Emission Integral Effect on Non-Invasive Blood Glucose Measurements Made Using Mid-Infrared Passive Spectroscopic Imaging
Abstract
1. Introduction
2. Materials and Methods
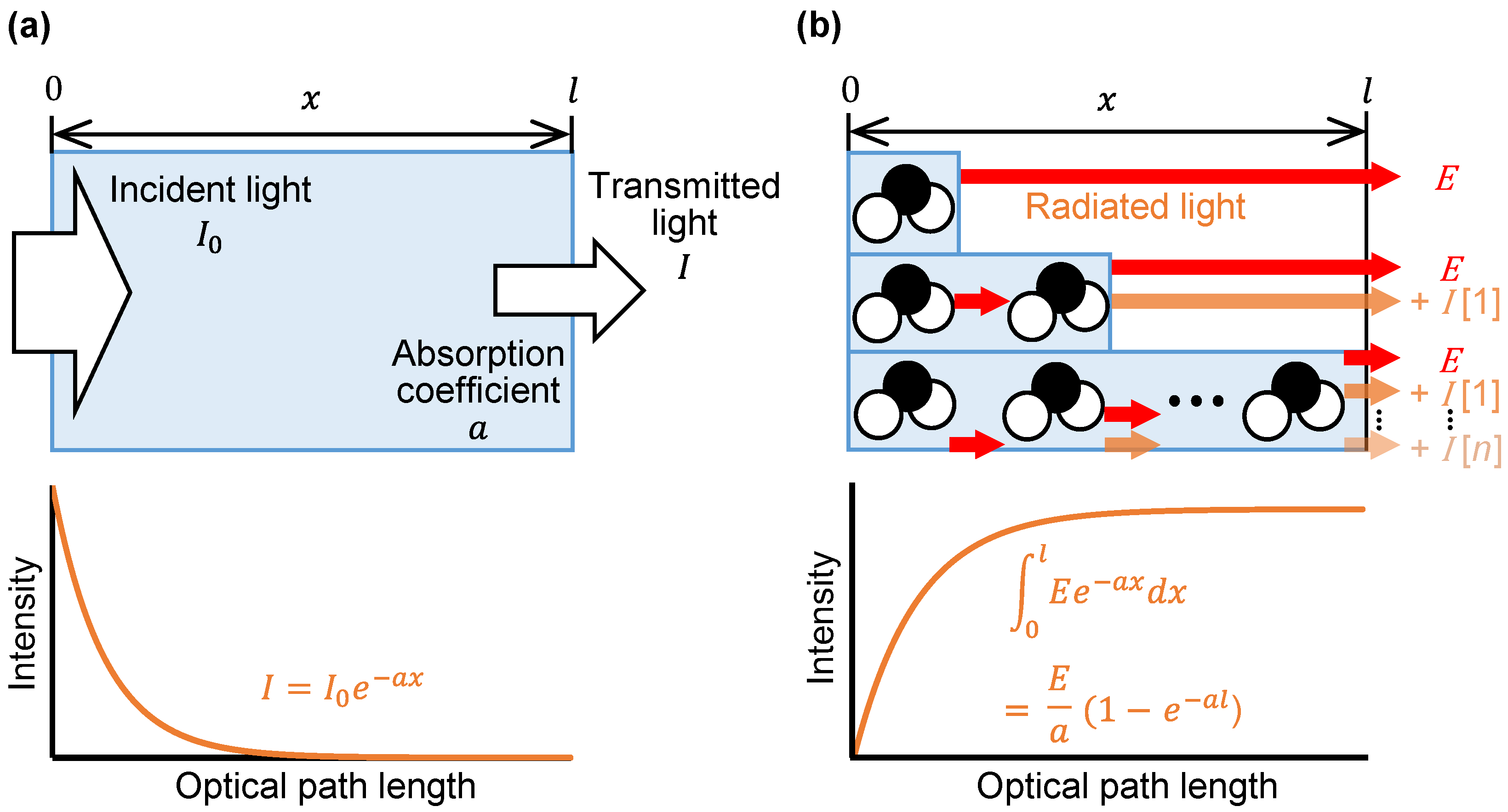
2.1. Derivation of the Emission Integral Effect
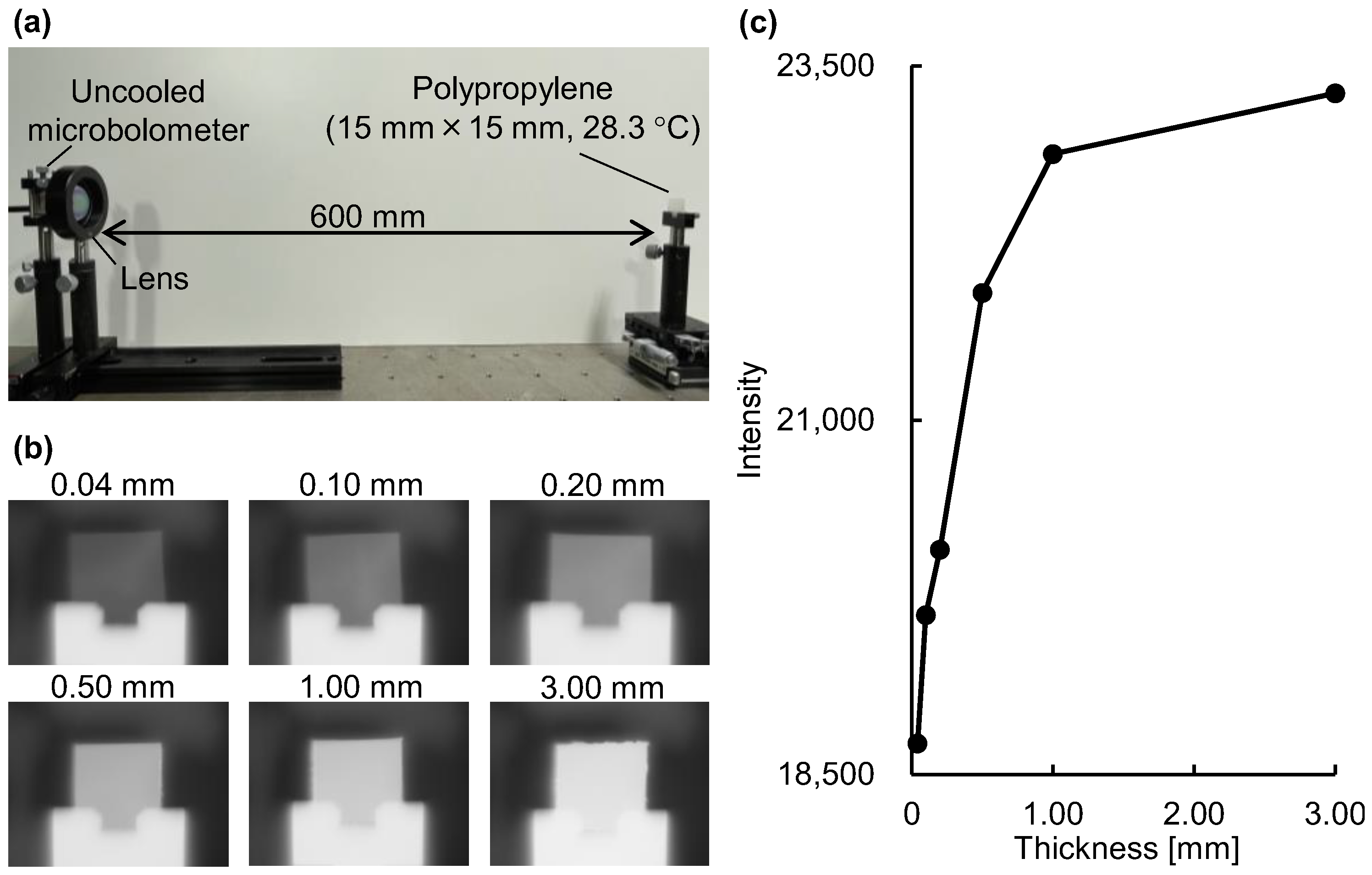
2.2. Experiments Using Polypropylene to Demonstrate the Emission Integral Effect
2.3. Internal Optics of the Apparatus Capable of Mid-Infrared Passive Spectroscopic Imaging
2.4. Measurement of Spectral Radiance and Radiance
2.5. Simulation of Spectral Radiance at Different Substance Thicknesses
2.6. Simulation of Spectral Radiance at Dermal Thickness
3. Results
3.1. Verification of the Emission Integral Effect on Radiance
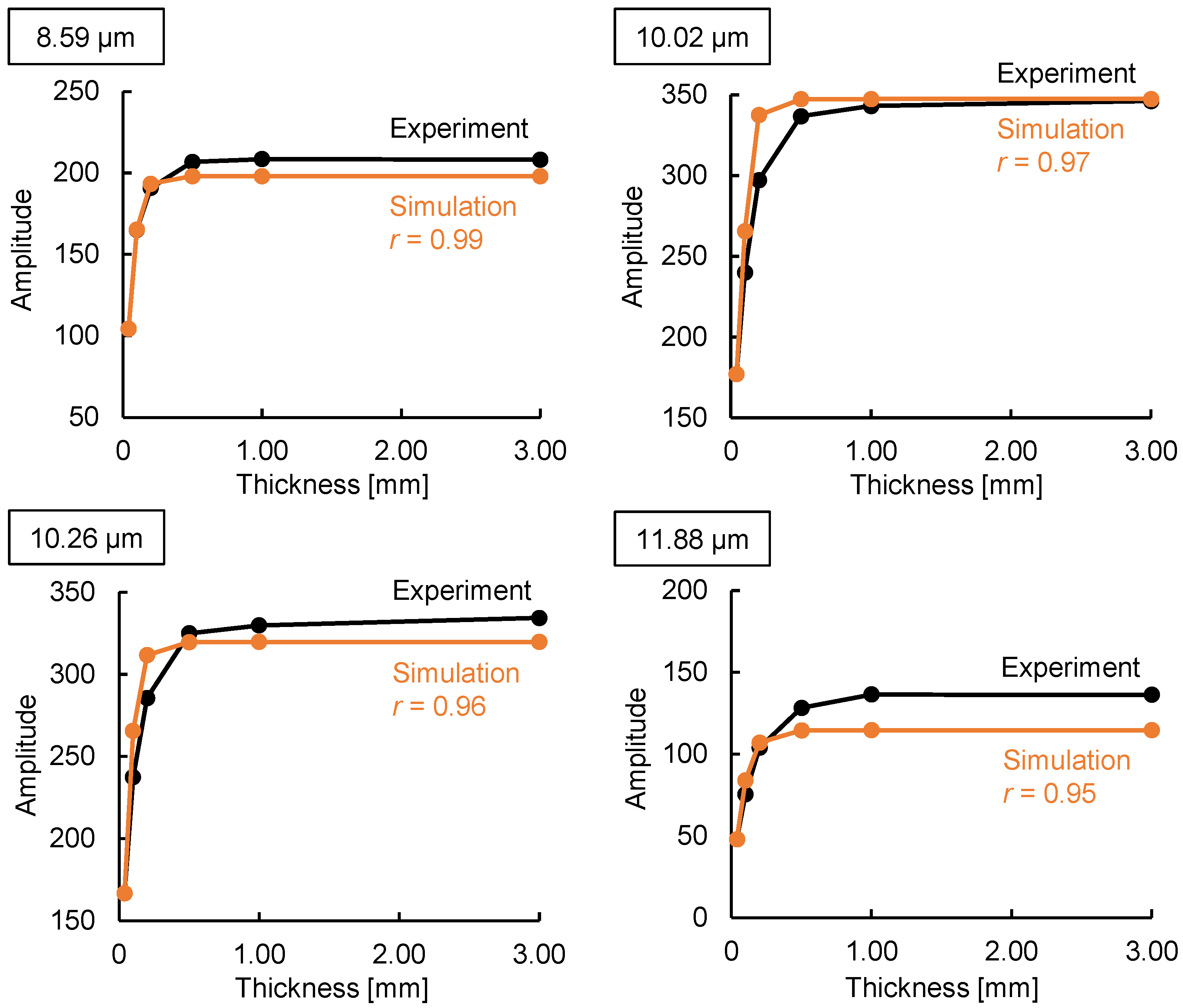
3.2. Verification of the Emission Integral Effect on Spectral Radiance
4. Discussion
4.1. Verification of the Emission Integral Effect Through Simulation
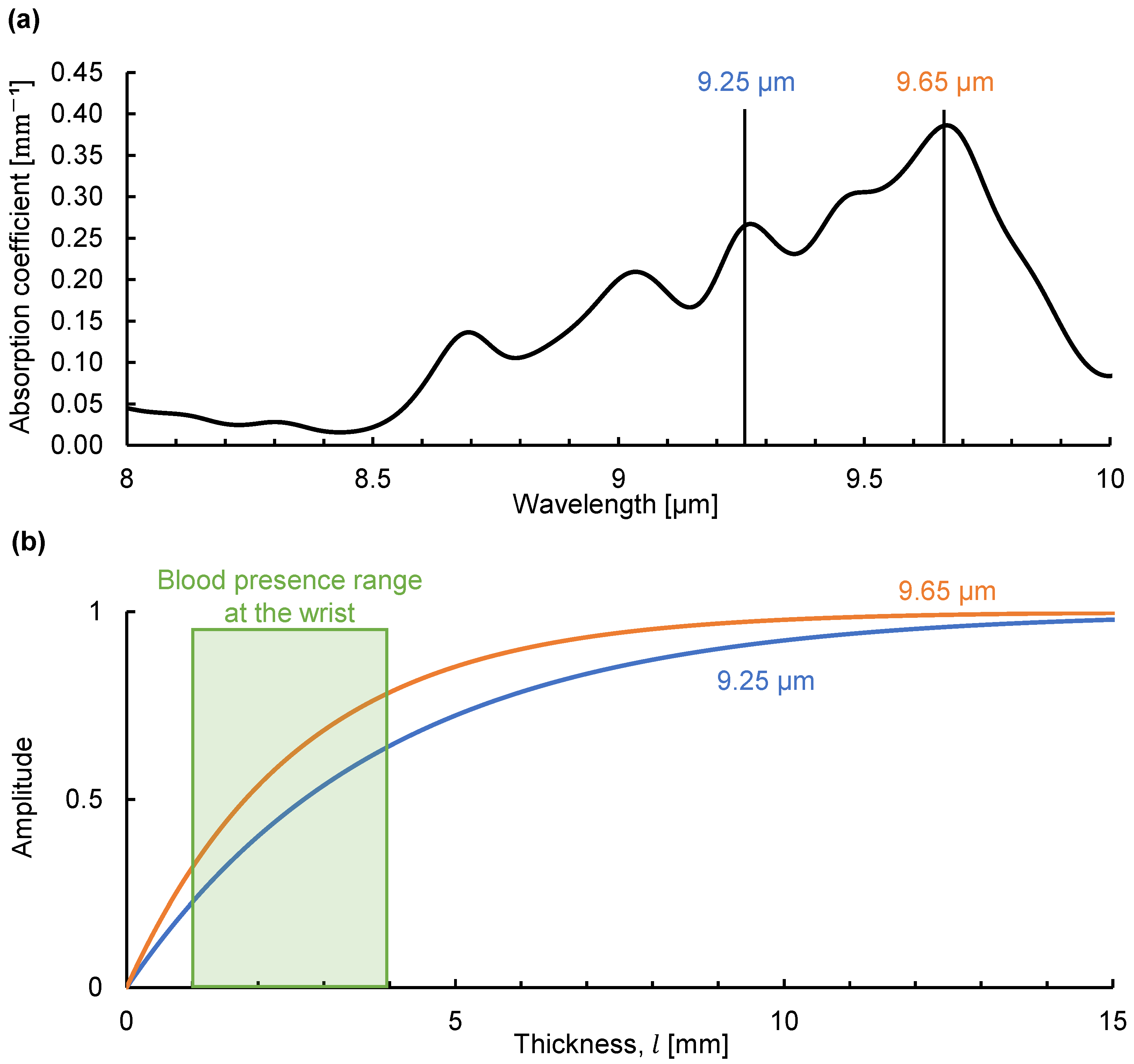
4.2. Emission Integral Effect on Blood Glucose at the Wrist
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ahmed, B.M.; Ali, M.E.; Masud, M.M.; Naznin, M. Recent trends and techniques of blood glucose level prediction for diabetes control. Smart Health 2024, 32, 100457. [Google Scholar] [CrossRef]
- Schleicher, E.; Gerdes, C.; Petersmann, A.; Müller-Wieland, D.; Müller, U.; Freckmann, G.; Heinemann, L.; Nauck, M.; Landgraf, R. Definition Classification and Diagnosis of Diabetes Mellitus. Exp. Clin. Endocrinol. Diabetes 2022, 130, S1–S8. [Google Scholar] [CrossRef] [PubMed]
- Villena Gonzales, W.; Mobashsher, A.T.; Abbosh, A. The progress of glucose monitoring—A review of invasive to minimally and non-invasive techniques, devices and sensors. Sensors 2019, 19, 800. [Google Scholar] [CrossRef]
- Arimoto, H.; Tarumi, M.; Yamada, Y. Instrumental requirements for non-invasive blood glucose measurement using NIR spectroscopy. Opt. Rev. 2003, 10, 161–165. [Google Scholar] [CrossRef]
- Maruo, K.; Yamada, Y. Near-infrared noninvasive blood glucose prediction without using multivariate analyses: Introduction of imaginary spectra due to scattering change in the skin. J. Biomed. Opt. 2015, 20, 047003. [Google Scholar] [CrossRef] [PubMed]
- Uwadaira, Y.; Ikehata, A.; Momose, A.; Miura, M. Identification of informative bands in the short-wavelength NIR region for non-invasive blood glucose measurement. Biomed. Opt. Express 2016, 7, 2729–2737. [Google Scholar] [CrossRef]
- Zheng, Y.; Gindy, N. Investigation of glucose non-invasive measurement based on NIR laser. Sens. Transducers 2006, 72, 769–785. [Google Scholar]
- Yadav, J.; Rani, A.; Singh, V.; Murari, B.M. Prospects and limitations of non-invasive blood glucose monitoring using near-infrared spectroscopy. Biomed. Signal Process. Control 2015, 18, 214–227. [Google Scholar] [CrossRef]
- Srichan, C.; Srichan, W.; Danvirutai, P.; Ritsongmuang, C.; Sharma, A.; Anutrakulchai, S. Non-invasively accuracy enhanced blood glucose sensor using shallow dense neural networks with NIR monitoring and medical features. Sci. Rep. 2022, 12, 1769. [Google Scholar] [CrossRef]
- Pleitez, M.; von Lilienfeld-Toal, H.; Mäntele, W. Infrared spectroscopic analysis of human interstitial fluid in vitro and in vivo using FT-IR spectroscopy and pulsed quantum cascade lasers (QCL): Establishing a new approach to non invasive glucose measurement. Spectrochim. Acta A Mol. Biomol. Spectrosc. 2012, 85, 61–65. [Google Scholar] [CrossRef]
- Yamakawa, K. Method for Measuring Concentration of Substance in Blood. U.S. patent 2021/0038125 A1, 11 February 2021. Available online: https://patents.google.com/patent/US20210038125A1 (accessed on 5 March 2025).
- Delbeck, S.; Heise, H.M. Evaluation of opportunities and limitations of mid-infrared skin spectroscopy for noninvasive blood glucose monitoring. J. Diabetes Sci. Technol. 2021, 15, 19–27. [Google Scholar] [CrossRef] [PubMed]
- Koyama, T.; Kino, S.; Matsuura, Y. Accuracy Improvement of Blood Glucose Measurement System Using Quantum Cascade Lasers. Opt. Photonics J. 2019, 9, 155–164. [Google Scholar] [CrossRef]
- Kitazaki, T.; Morimoto, Y.; Yamashita, S.; Anabuki, D.; Tahara, S.; Nishiyama, A.; Wada, K.; Ishimaru, I. Glucose emission spectra through mid-infrared passive spectroscopic imaging of the wrist for non-invasive glucose sensing. Sci. Rep. 2022, 12, 20558. [Google Scholar] [CrossRef] [PubMed]
- Buchert, J.M. Instrument and Method for Non-Invasive Monitoring of Human Tissue Analyte by Measuring the Body’s Infrared Radiation. U.S. Patent 5666956A, 16 September 1997. Available online: https://patents.google.com/patent/US5666956A/en (accessed on 5 March 2025).
- Malchoff, C.D.; Shoukri, K.; Landau, J.I.; Buchert, J.M. A novel noninvasive blood glucose monitor. Diabetes Care 2002, 25, 2268–2275. [Google Scholar] [CrossRef]
- Klonoff, D.C. Noninvasive blood glucose monitoring. Diabetes Care 1997, 20, 433–437. [Google Scholar] [CrossRef]
- Mueller, M.; Grunze, M.; Leiter, E.H.; Reifsnyder, P.C.; Klueh, U.; Kreutzer, D. Non-invasive glucose measurements in mice using mid-infrared emission spectroscopy. Sens. Actuators B Chem. 2009, 142, 502–508. [Google Scholar] [CrossRef]
- Inoue, Y.; Ishimaru, I.; Yasokawa, T.; Ishizaki, K.; Yoshida, M.; Kondo, M.; Kuriyama, S.; Masaki, T.; Nakai, S.; Takegawa, K.; et al. Variable phase-contrast fluorescence spectrometry for fluorescently stained cells. Appl. Phys. Lett. 2006, 89, 121103. [Google Scholar] [CrossRef]
- Ishimaru, I. Palm-sized (80-mm cube weighing 0.5 kg) low-price (several thousand USD) mid-infrared (wavelength 8–14 µm) Fourier spectroscopic imager. In Proceedings of the Imaging Spectrometry XXIII: Applications, Sensors, and Processing, San Diego, CA, USA, 11–15 August 2019; Volume 11130. [Google Scholar] [CrossRef]
- Qi, W.; Suzuki, Y.; Sato, S.; Fujiwara, M.; Kawashima, N.; Suzuki, S.; Abeygunawardhana, P.; Wada, K.; Nishiyama, A.; Ishimaru, I. Enhanced interference-pattern visibility using multislit optical superposition method for imaging-type two-dimensional Fourier spectroscopy. Appl. Opt. 2015, 54, 6254. [Google Scholar] [CrossRef]
- Tirosh, A.; Shai, I.; Tekes-Manova, D.; Israeli, E.; Pereg, D.; Shochat, T.; Kochba, I.; Rudich, A. Normal fasting plasma glucose levels and type 2 diabetes in young men. N. Engl. J. Med. 2005, 353, 1454–1462. [Google Scholar] [CrossRef]
- Raju, B.I.; Srinivasan, M.A. High-frequency ultrasonic attenuation and backscatter coefficients of in vivo normal human dermis and subcutaneous fat. Ultrasound Med. Biol. 2001, 27, 1543–1556. [Google Scholar] [CrossRef]
- Wang, Y.-J.; Chen, T.-Y.; Tsai, M.-C.; Wu, C.H. Noninvasive blood pressure monitor using strain gauges, a fastening band, and a wrist elasticity model. Sens. Actuators A Phys. 2016, 252, 198–208. [Google Scholar] [CrossRef]
- Zygmunt, D.; Joanna, G.; Natalie, P.; Nicole, H.; Jakub, K.; Agnieszka, L.; Katarzyna, S.; Bozena, K.; Mirosław, T. Ultrasound evaluation of the radial artery in young adults—A pilot study. Ann. Anat. 2021, 238, 151763. [Google Scholar] [CrossRef]
- Oltulu, P.; Ince, B.; Kokbudak, N.; Findik, S.; Kilinc, F. Measurement of epidermis, dermis, and total skin thicknesses from six different body regions with a new ethical histometric technique. Turk. J. Plast. Surg. 2018, 26, 56–61. [Google Scholar] [CrossRef]



| Ratio of 3σ to the Mean (%) | ||||||
|---|---|---|---|---|---|---|
| 0.04 mm | 0.10 mm | 0.20 mm | 0.50 mm | 1.00 mm | 3.00 mm | |
| 9.36 µm | 4.22 | 4.57 | 5.58 | 4.33 | 3.65 | 2.81 |
| 10.02 µm | 3.79 | 4.50 | 3.08 | 3.72 | 2.33 | 2.27 |
| 10.26 µm | 5.77 | 4.58 | 3.93 | 3.39 | 2.57 | 3.13 |
| 11.33 µm | 5.57 | 5.41 | 5.32 | 3.14 | 3.35 | 3.63 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anabuki, D.; Tahara, S.; Yano, H.; Nishiyama, A.; Wada, K.; Nishimura, A.; Ishimaru, I. Emission Integral Effect on Non-Invasive Blood Glucose Measurements Made Using Mid-Infrared Passive Spectroscopic Imaging. Sensors 2025, 25, 1674. https://doi.org/10.3390/s25061674
Anabuki D, Tahara S, Yano H, Nishiyama A, Wada K, Nishimura A, Ishimaru I. Emission Integral Effect on Non-Invasive Blood Glucose Measurements Made Using Mid-Infrared Passive Spectroscopic Imaging. Sensors. 2025; 25(6):1674. https://doi.org/10.3390/s25061674
Chicago/Turabian StyleAnabuki, Daichi, Shiori Tahara, Hibiki Yano, Akira Nishiyama, Kenji Wada, Akiko Nishimura, and Ichiro Ishimaru. 2025. "Emission Integral Effect on Non-Invasive Blood Glucose Measurements Made Using Mid-Infrared Passive Spectroscopic Imaging" Sensors 25, no. 6: 1674. https://doi.org/10.3390/s25061674
APA StyleAnabuki, D., Tahara, S., Yano, H., Nishiyama, A., Wada, K., Nishimura, A., & Ishimaru, I. (2025). Emission Integral Effect on Non-Invasive Blood Glucose Measurements Made Using Mid-Infrared Passive Spectroscopic Imaging. Sensors, 25(6), 1674. https://doi.org/10.3390/s25061674








