Anti-Interference Spectral Confocal Sensors Based on Line Spot
Abstract
1. Introduction
2. Principles and Structural Design
2.1. Measurement Principle
2.2. Overall Structural Design of Line Spot Spectral Confocal Sensor
3. Simulation Analysis
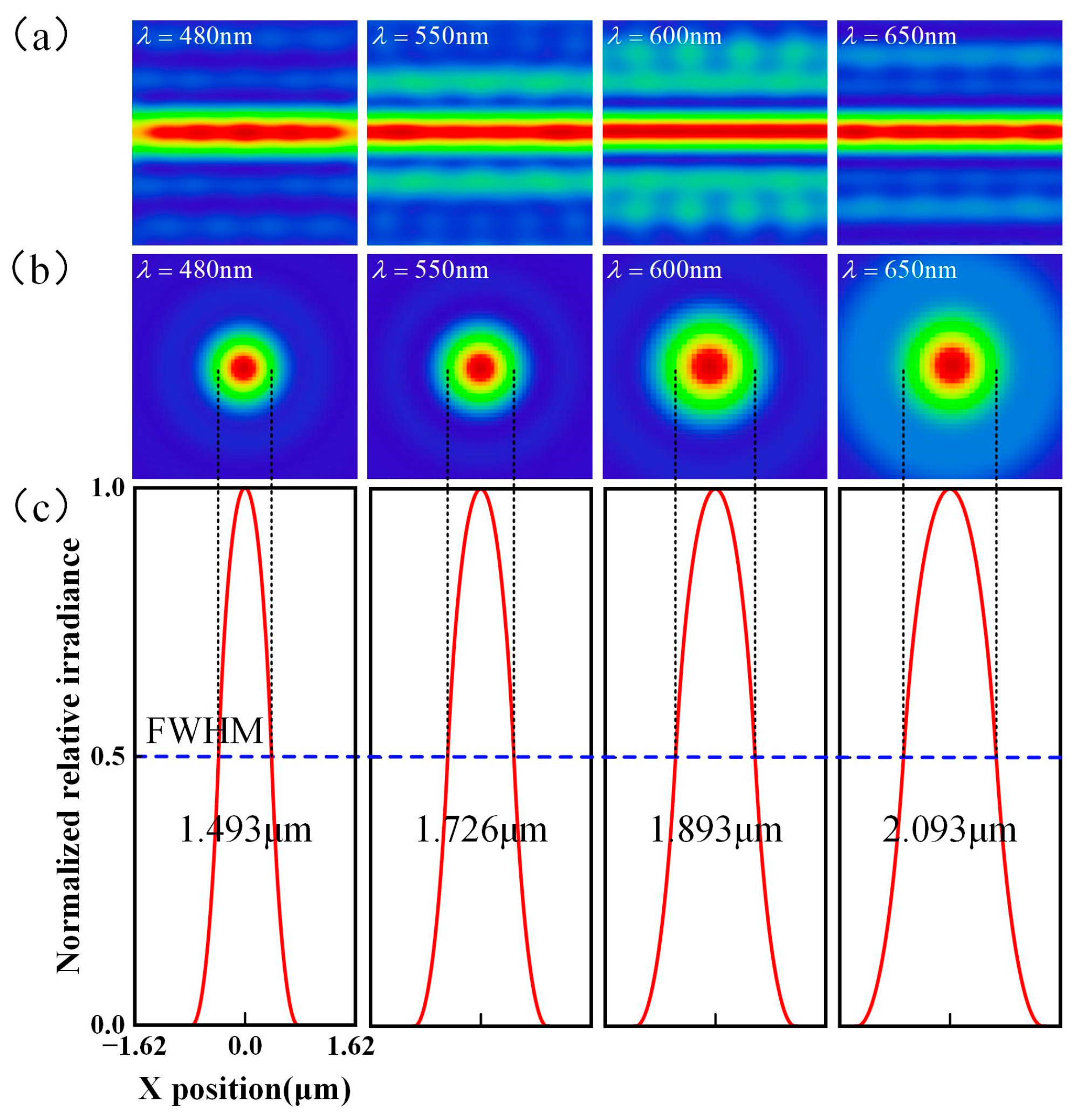
3.1. Simulation Analysis of Binary Diffractive Lens
3.2. Microscope Objective Simulation Analysis
3.3. Overall Structure Simulation Analysis
4. Experiment
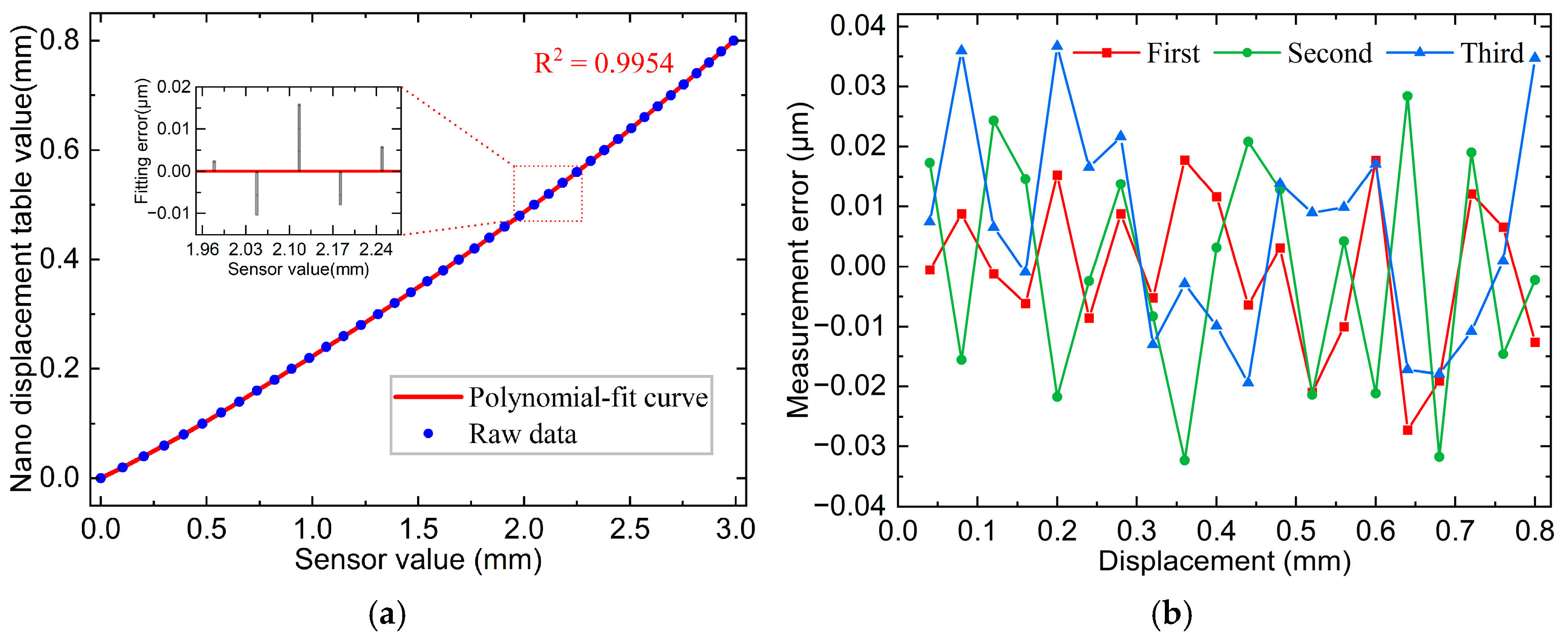
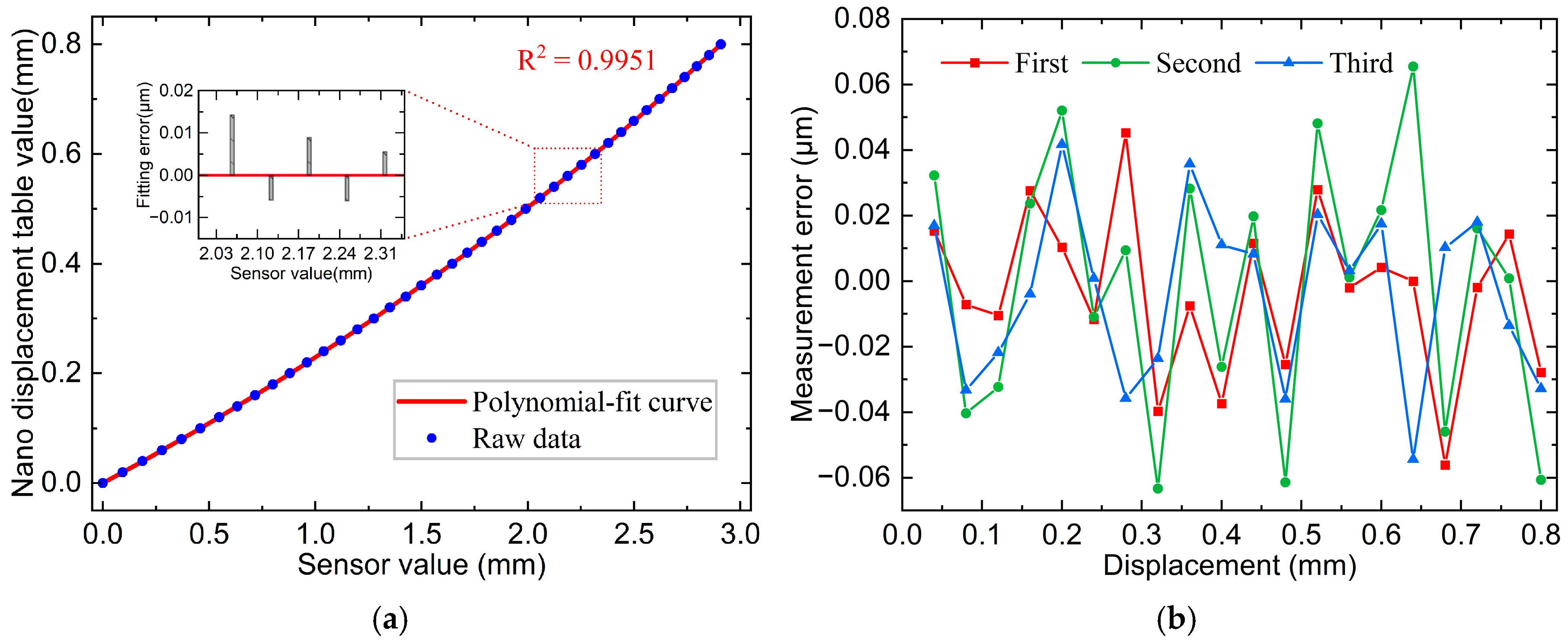
4.1. System Calibration Experiment
4.2. Axial Resolution Experiment
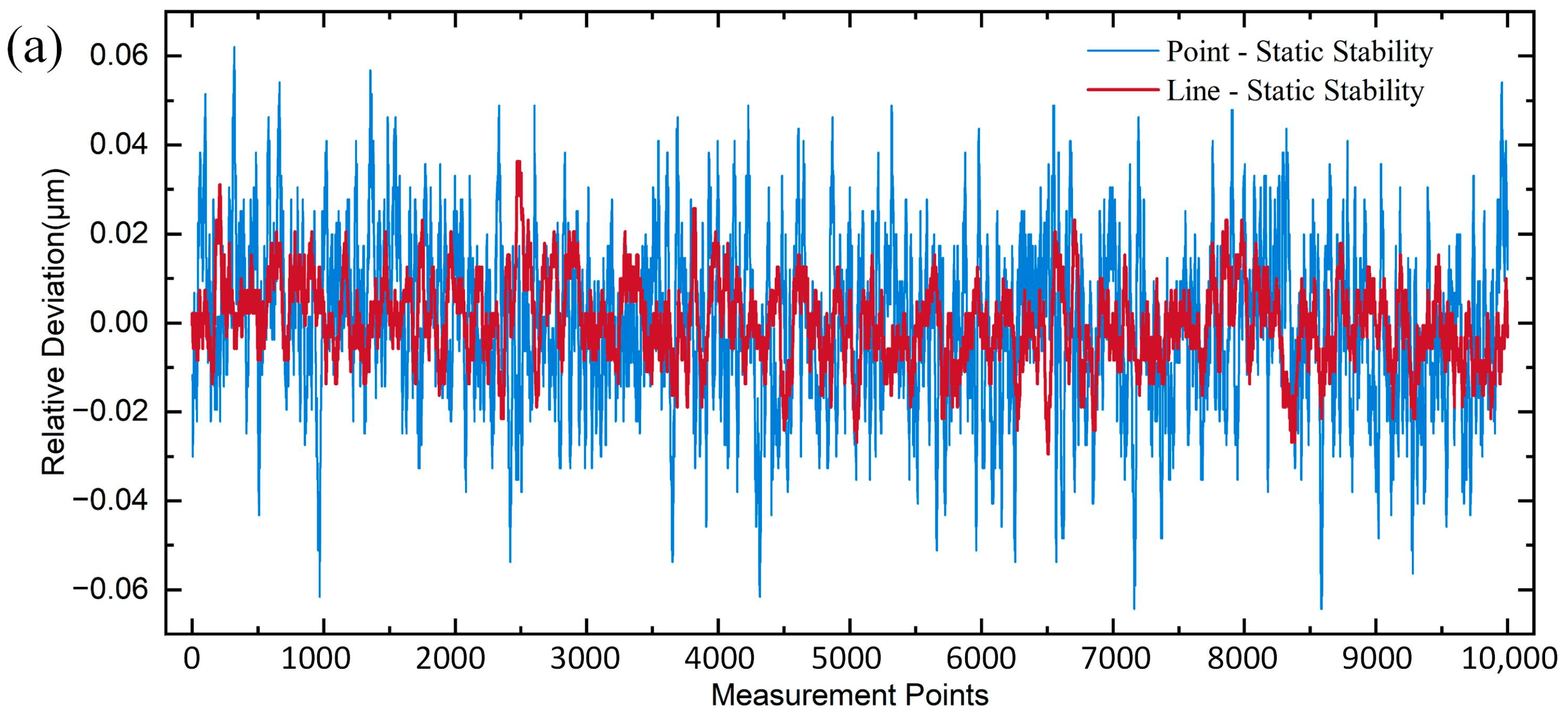
4.3. Static Stability Experiment
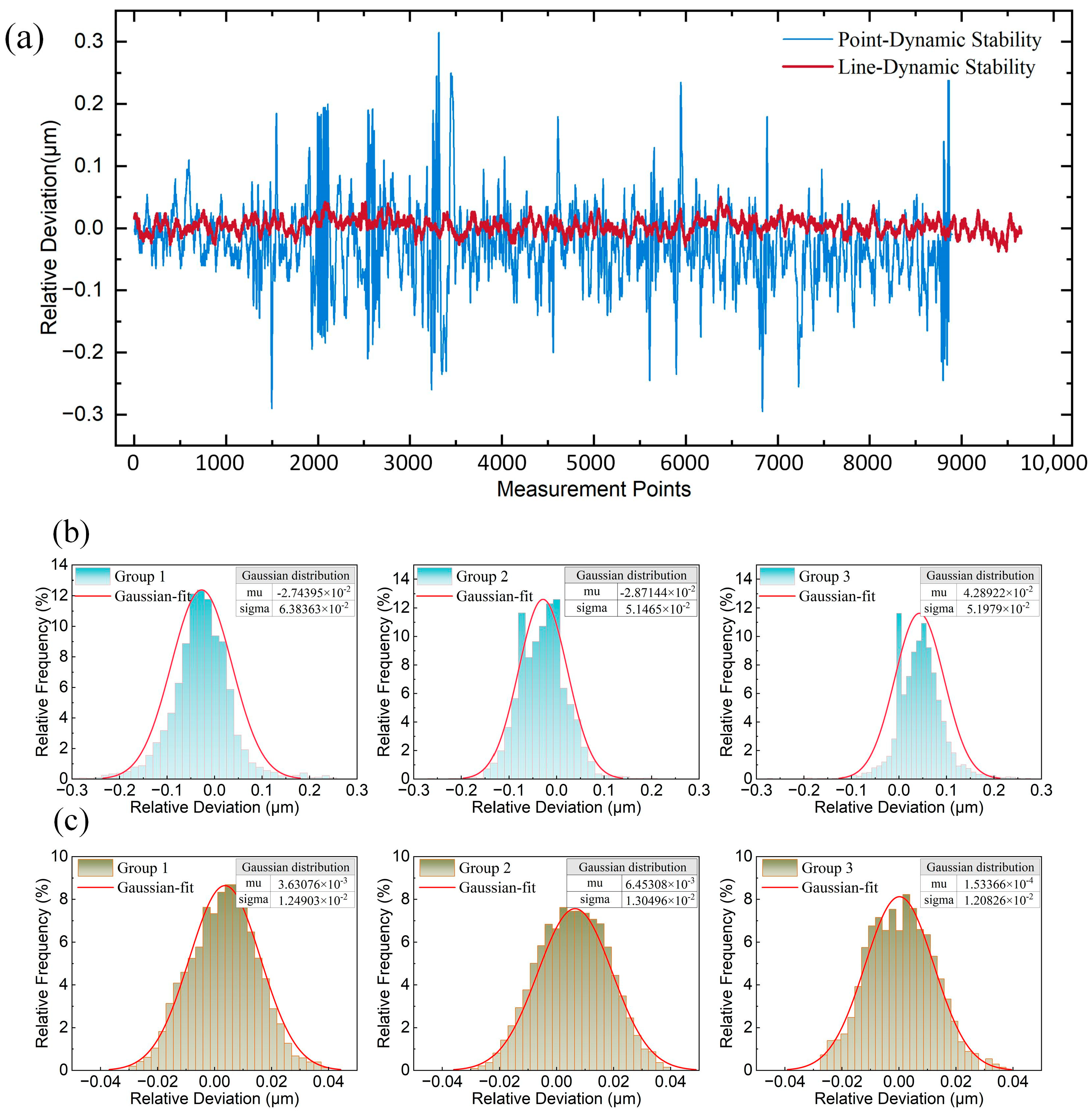
4.4. Dynamic Stability Experiment
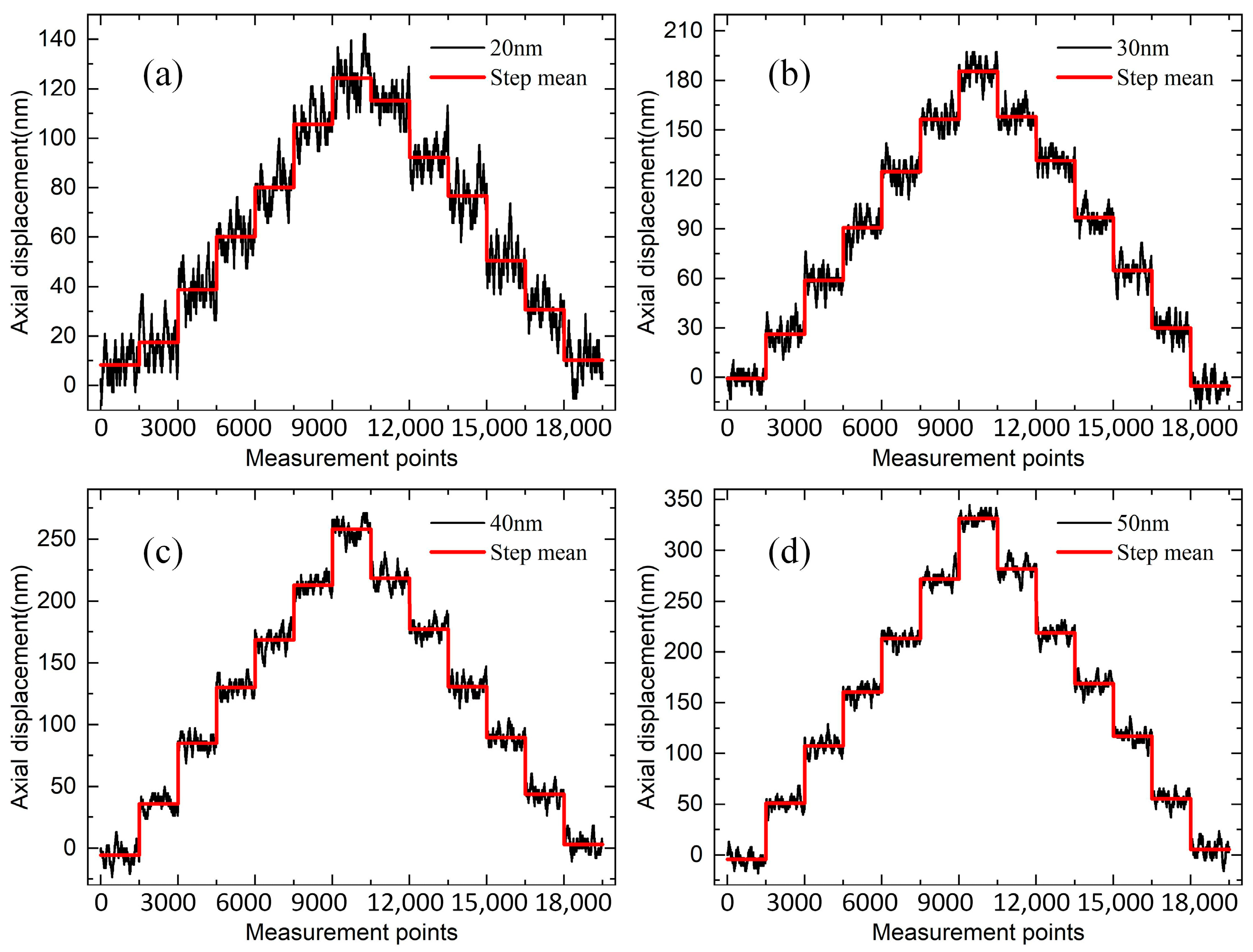
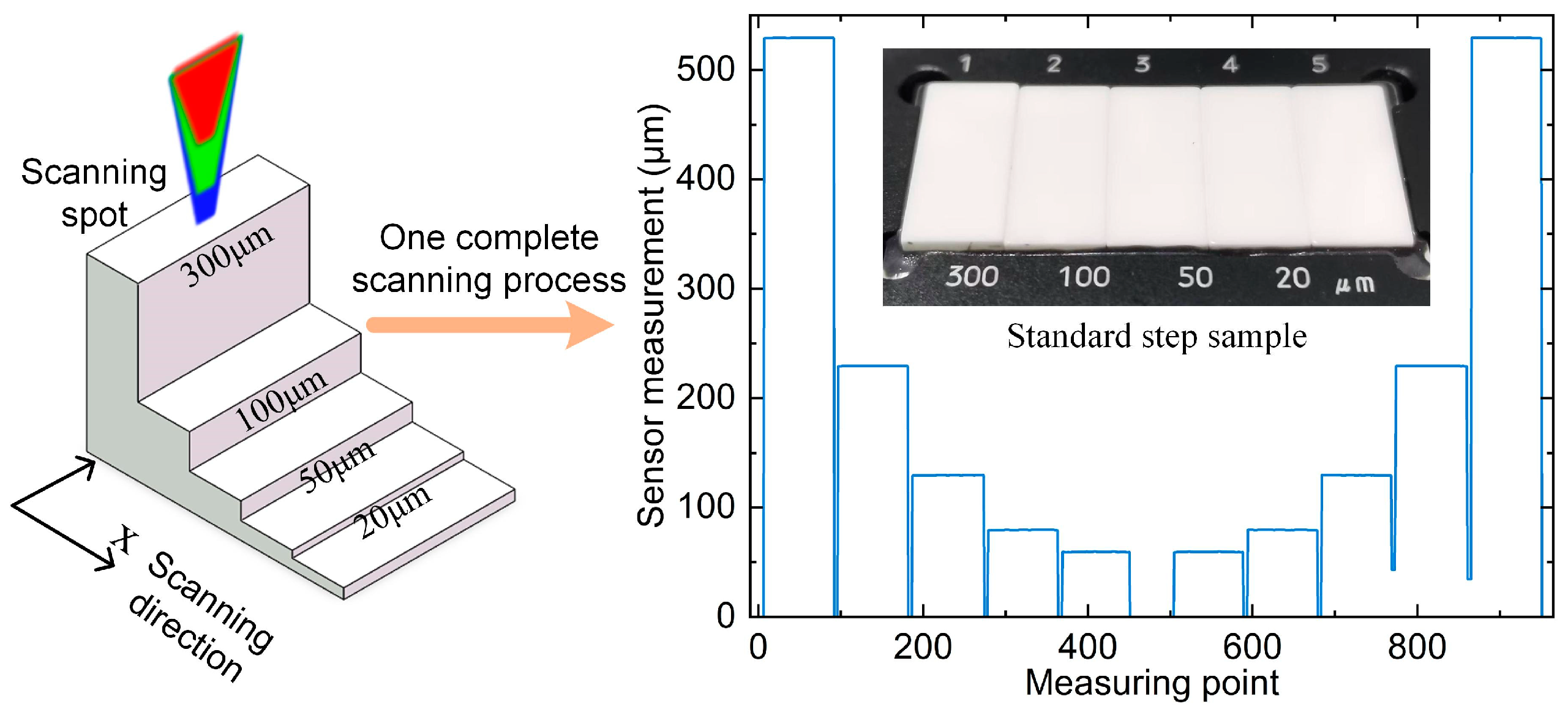
4.5. Standard Step Scanning Experiment
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, B.; Li, J.; Mao, X.; Sun, F.; Gao, X. Dynamic pressure surface deformation measurement based on a chromatic confocal sensor. Appl. Opt. 2023, 62, 1467–1474. [Google Scholar] [CrossRef] [PubMed]
- Shang, Z.; Wang, J.; Du, H.; Yin, P. High-precision measurement of gear tooth profile using line spectral confocal method. Measurement 2023, 223, 113779. [Google Scholar] [CrossRef]
- Lishchenko, N.; O’Donnell, G.E.; Culleton, M. Contactless Method for Measurement of Surface Roughness Based on a Chromatic Confocal Sensor. Machines 2023, 11, 836. [Google Scholar] [CrossRef]
- Haider, C.; Fuerst, M.E.; Laimer, M.; Csencsics, E.; Schitter, G. Range-Extended Confocal Chromatic Sensor System for Double-Sided Measurement of Optical Components with High Lateral Resolution. IEEE Trans. Instrum. Meas. 2022, 71, 1–8. [Google Scholar] [CrossRef]
- Titze, V.M.; Caixeiro, S.; Dinh, V.S.; König, M.; Rübsam, M.; Pathak, N.; Schumacher, A.-L.; Germer, M.; Kukat, C.; Niessen, C.M. Hyperspectral confocal imaging for high-throughput readout and analysis of bio-integrated microlasers. Nat. Protoc. 2024, 19, 928–959. [Google Scholar] [CrossRef]
- Sung, Y.; Wang, W. Hyperspectral confocal microscopy in the short-wave infrared range. Opt. Lett. 2023, 48, 3993–3996. [Google Scholar] [CrossRef]
- Santos, J.; Jakobsen, H.H.; Petersen, P.M.; Pedersen, C. Remote 3D Imaging and Classification of Pelagic Microorganisms with A Short-Range Multispectral Confocal LiDAR. Laser Photonics Rev. 2024, 18, 2301291. [Google Scholar] [CrossRef]
- Li, J.; Zhu, X.; Du, H.; Ji, Z.; Wang, K.; Zhao, M. Thickness measurement method for self-supporting film with double chromatic confocal probes. Appl. Opt. 2021, 60, 9447–9452. [Google Scholar] [CrossRef]
- Jiao, S.; Wang, S.; Gao, M.; Xu, M. Non-contact method of thickness measurement for thin-walled rotary shell parts based on chromatic confocal sensor. Measurement 2024, 224, 113794. [Google Scholar] [CrossRef]
- Chen, Z.; Xue, K.; Sun, C.; Liu, Y.; Tan, J. Measuring the profile of aircraft engine blades using spectral confocal sensors. Meas. Sci. Technol. 2024, 35, 075009. [Google Scholar] [CrossRef]
- Wang, Y.; Qin, Y.; Zhang, T.; Qin, H.; Wang, J.; Ding, W. LSTM-based spectral confocal signal processing method. Appl. Opt. 2024, 63, 7396–7401. [Google Scholar] [CrossRef]
- Wang, S.; Diao, K.; Liu, X. Spectral line confocal sensor signal analysis and correction: Unlocking reflectance difference sample measurements. Precis. Eng. 2024, 88, 759–766. [Google Scholar] [CrossRef]
- Xi, M.; Liu, H.; Li, D.; Wang, Y. Intensity response model and measurement error compensation method for chromatic confocal probe considering the incident angle. Opt. Lasers Eng. 2024, 172, 107858. [Google Scholar] [CrossRef]
- Zhang, H.; Li, Z.; Hu, H.; Sun, J.; Hou, Y.; Song, J. A method for measuring and adaptively correcting lens center thickness based on the chromatic confocal principle. Results Phys. 2024, 56, 107281. [Google Scholar] [CrossRef]
- Yang, W.; Du, J.; Qi, M.; Yan, J.; Cheng, M.; Zhang, Z. Design of Optical System for Ultra-Large Range Line-Sweep Spectral Confocal Displacement Sensor. Sensors 2024, 24, 723. [Google Scholar] [CrossRef]
- Liao, M.; Yang, Y.; Lu, X.; Li, H.; Zhang, J.; Wang, J.; Chen, Z. Off-Axis Co-Optical Path Large-Range Line Scanning Chromatic Confocal Sensor. Photonic Sens. 2024, 14, 240309. [Google Scholar] [CrossRef]
- Liu, T.; Wang, J.; Liu, Q.; Hu, J.; Wang, Z.; Wan, C.; Yang, S. Chromatic confocal measurement method using a phase Fresnel zone plate. Opt. Express 2022, 30, 2390–2401. [Google Scholar] [CrossRef] [PubMed]
- He, N.; Hu, H.; Cui, Z.; Xu, X.; Zhou, D.; Chen, Y.; Gong, P.; Chen, Y.; Kuang, C. Compact Chromatic Confocal Lens with Large Measurement Range. Sensors 2024, 24, 5122. [Google Scholar] [CrossRef]
- Zhou, W.; Sun, Y.; Li, W.; Bayanheshig; Wang, X.; Guo, L.; Liu, Z. Polarization-folding ultra-wide spectral chromatic confocal line displacement sensor based on a binary lens. Opt. Laser Technol. 2024, 177, 111152. [Google Scholar] [CrossRef]
- Sun, Z.; Huang, X.; Yang, C. Fiber chromatic confocal method with a tilt-coupling source module for axial super-resolution. Opt. Express 2023, 31, 39153–39168. [Google Scholar] [CrossRef]
- Li, C.; Li, K.; Liu, J.; Lv, Z.; Li, G.; Li, D. Design of a confocal dispersion objective lens based on the GRIN lens. Opt. Express 2022, 30, 44290–44299. [Google Scholar] [CrossRef] [PubMed]
- Falak, P.; Chan, J.H.-T.; Williamson, J.; Henning, A.; Lee, T.; Zahertar, S.; Holmes, C.; Beresna, M.; Martin, H.; Brambilla, G. An Ultracompact Metasurface and Specklemeter-Based Chromatic Confocal Sensor. IEEE Trans. Instrum. Meas. 2024, 73, 1–8. [Google Scholar] [CrossRef]
- Bai, J.; Li, J.; Wang, X.; Zhou, Q.; Ni, K.; Li, X. A new method to measure spectral reflectance and film thickness using a modified chromatic confocal sensor. Opt. Lasers Eng. 2022, 154, 107019. [Google Scholar] [CrossRef]
- Chen, H.-R.; Chen, L.-C. Full-field chromatic confocal microscopy for surface profilometry with sub-micrometer accuracy. Opt. Lasers Eng. 2023, 161, 107384. [Google Scholar] [CrossRef]
- Chen, X.; Nakamura, T.; Shimizu, Y.; Chen, C.; Chen, Y.-L.; Matsukuma, H.; Gao, W. A chromatic confocal probe with a mode-locked femtosecond laser source. Opt. Laser Technol. 2018, 103, 359–366. [Google Scholar] [CrossRef]
- Sato, R.; Chen, C.; Matsukuma, H.; Shimizu, Y.; Gao, W. A new signal processing method for a differential chromatic confocal probe with a mode-locked femtosecond laser. Meas. Sci. Technol. 2020, 31, 094004. [Google Scholar] [CrossRef]
- Chen, C.; Sato, R.; Shimizu, Y.; Nakamura, T.; Matsukuma, H.; Gao, W. A method for expansion of Z-directional measurement range in a mode-locked femtosecond laser chromatic confocal probe. Appl. Sci. 2019, 9, 454. [Google Scholar] [CrossRef]
- Sato, R.; Shimizu, Y.; Chen, C.; Matsukuma, H.; Gao, W. Investigation and improvement of thermal stability of a chromatic confocal probe with a mode-locked femtosecond laser source. Appl. Sci. 2019, 9, 4084. [Google Scholar] [CrossRef]
- Zhao, Y.; Chang, S.; Zhang, Y.; Xia, H. Application of the Hilbert-Huang transform to evaluate signals in chromatic confocal spectral interferometry. Appl. Opt. 2024, 63, 9088–9096. [Google Scholar] [CrossRef]
- Dai, J.; Zhong, W.; Zeng, W.; Jiang, X.; Chang, S.; Lu, W. Enhancing precision in line-scan chromatic confocal sensors through bimodal signal pattern. Opt. Laser Technol. 2025, 180, 111417. [Google Scholar] [CrossRef]
- Zhang, G.; Peng, J.; Jia, S.; Nie, T.; Zhou, X.; Yu, H. Non-contact measurement of insulating bearing coating thickness based on multi-sensor combination. Measurement 2023, 207, 112369. [Google Scholar] [CrossRef]


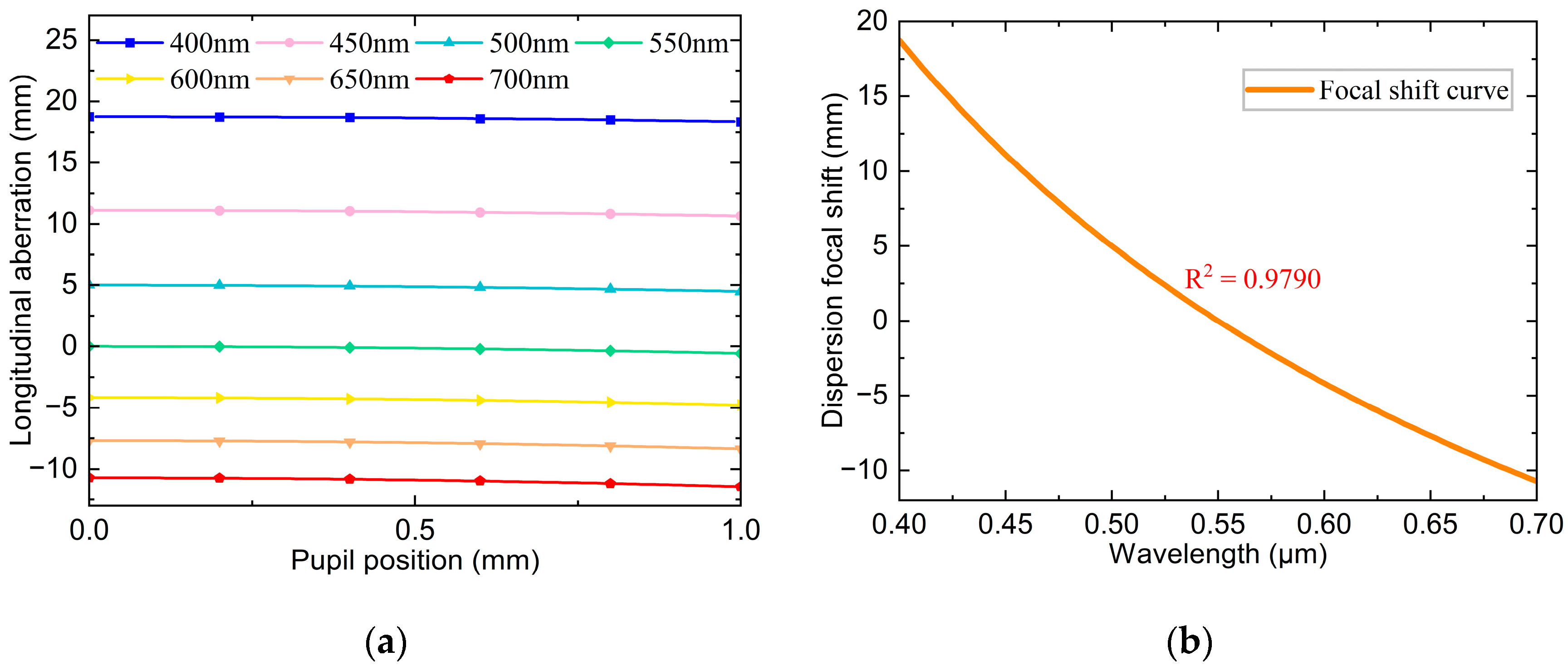
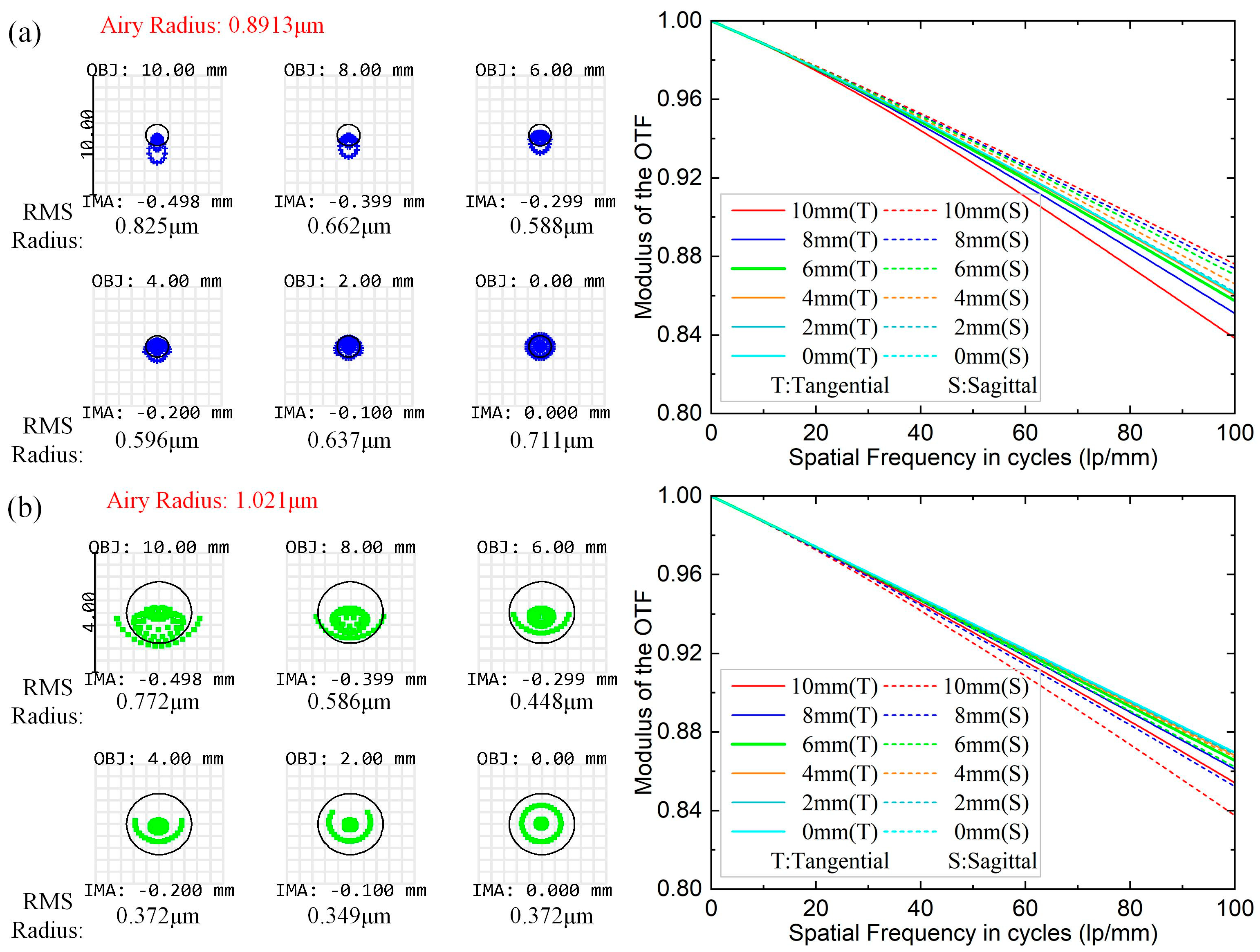
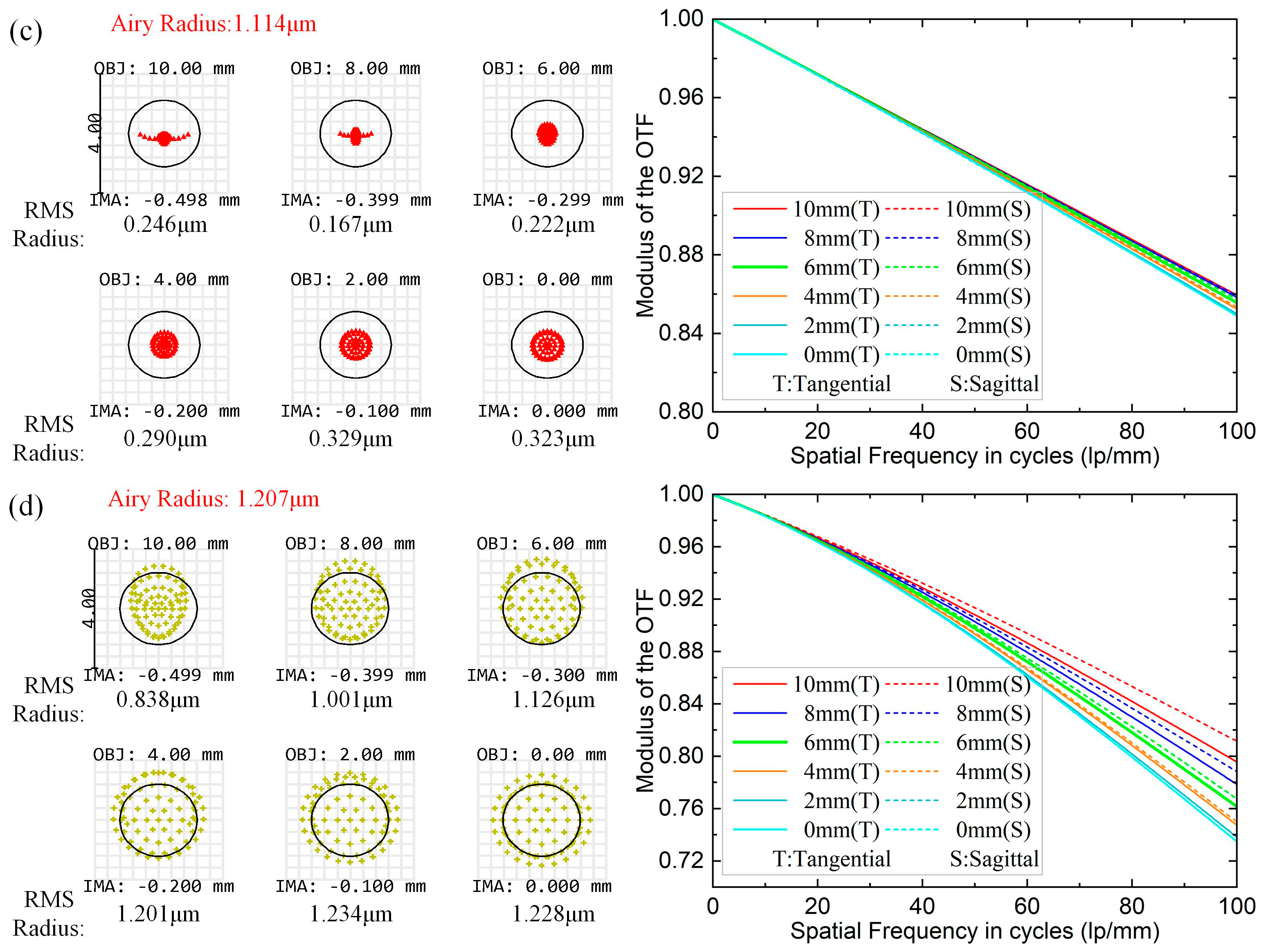
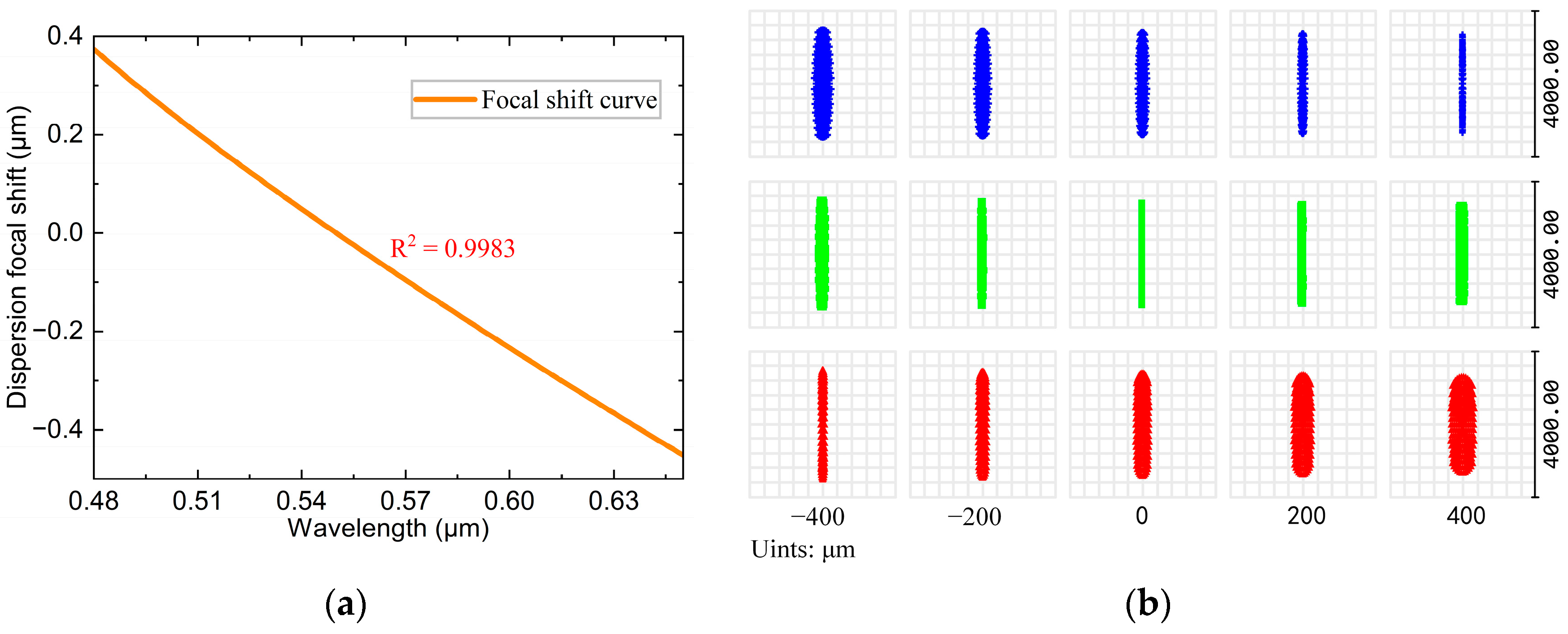



| Parameter | Value |
|---|---|
| Design center wavelength (nm) | 550 |
| Focal length (mm) | 50 |
| Diffraction level | −1 |
| Thicknesses (mm) | 1.2 |
| Surface Type | Thickness | Material | Radius | Norm Radius | P2 | P4 | P6 |
|---|---|---|---|---|---|---|---|
| Object | Inf | - | - | - | - | - | - |
| Standard | 1.2 | N-BK7 | 9.5 | - | - | - | - |
| Binary | 50.005 | - | 9.5 | 7.5 | 6425.396 | −333.748 | 80.760 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, B.; Li, J.; Luo, M.; Liang, F.; Hu, J. Anti-Interference Spectral Confocal Sensors Based on Line Spot. Sensors 2025, 25, 1337. https://doi.org/10.3390/s25051337
Wang B, Li J, Luo M, Liang F, Hu J. Anti-Interference Spectral Confocal Sensors Based on Line Spot. Sensors. 2025; 25(5):1337. https://doi.org/10.3390/s25051337
Chicago/Turabian StyleWang, Bo, Jiafu Li, Mingzhe Luo, Fengshuang Liang, and Jiacheng Hu. 2025. "Anti-Interference Spectral Confocal Sensors Based on Line Spot" Sensors 25, no. 5: 1337. https://doi.org/10.3390/s25051337
APA StyleWang, B., Li, J., Luo, M., Liang, F., & Hu, J. (2025). Anti-Interference Spectral Confocal Sensors Based on Line Spot. Sensors, 25(5), 1337. https://doi.org/10.3390/s25051337





