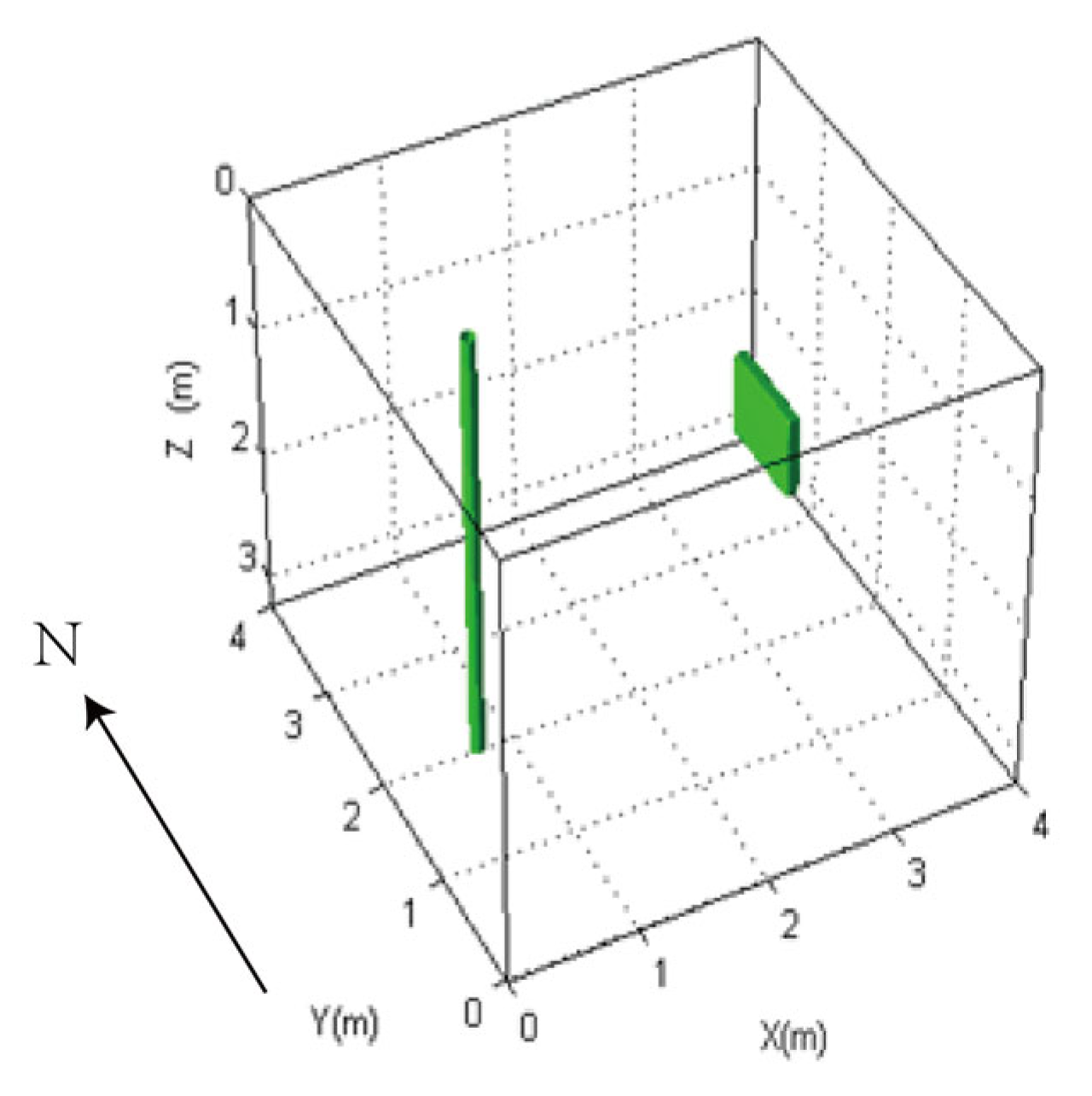
Figure 1.
Directional borehole sonde with one transmitting and four receiving antennas forming a uniform circular array (UCA), located in a water-filled borehole.
Figure 1.
Directional borehole sonde with one transmitting and four receiving antennas forming a uniform circular array (UCA), located in a water-filled borehole.
Figure 2.
Schematic diagram of a uniform linear array.
Figure 2.
Schematic diagram of a uniform linear array.
Figure 3.
Schematic diagram of ray distribution in the near−field blind zone.
Figure 3.
Schematic diagram of ray distribution in the near−field blind zone.
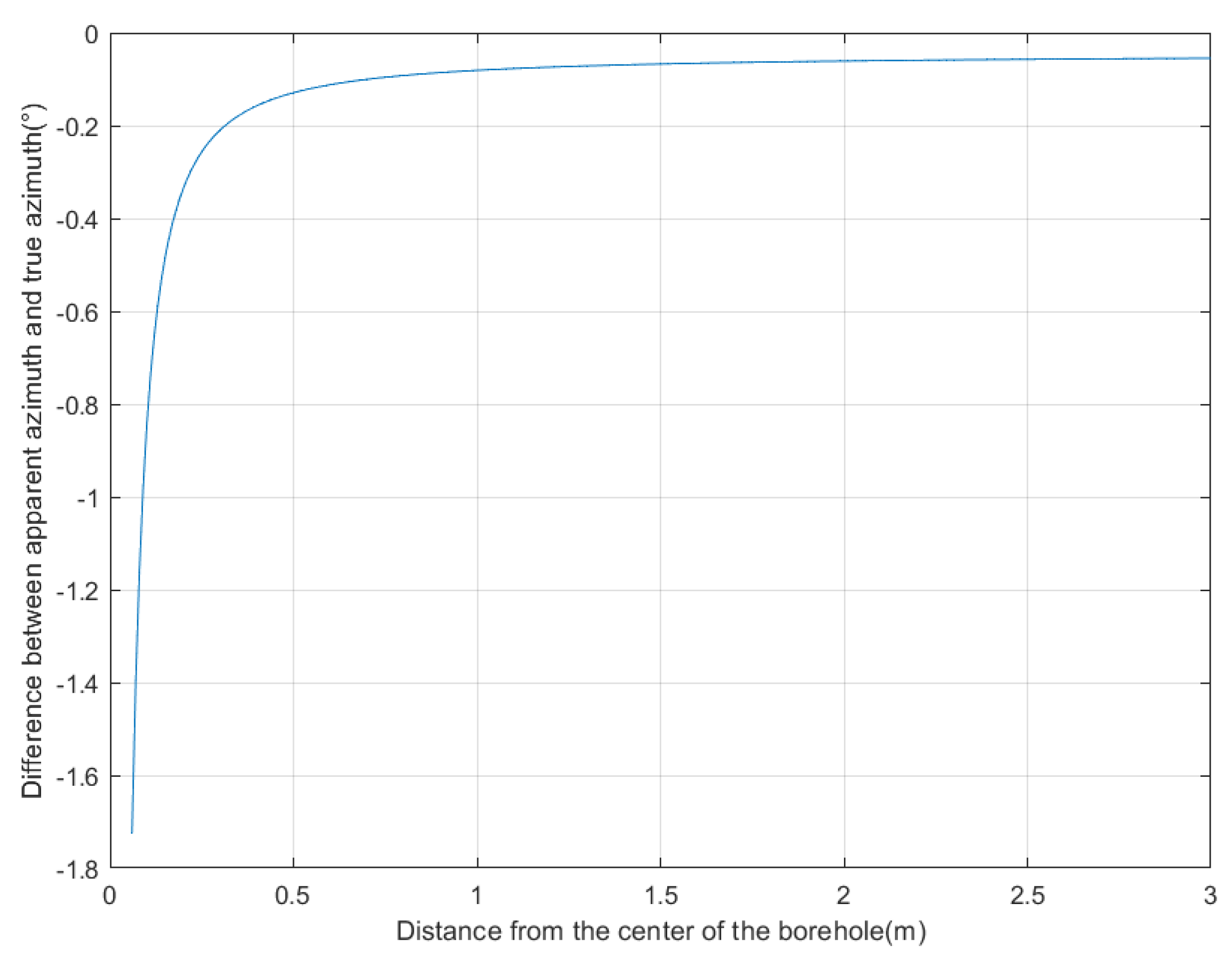
Figure 4.
The relationship between distance and azimuth identification error.
Figure 4.
The relationship between distance and azimuth identification error.
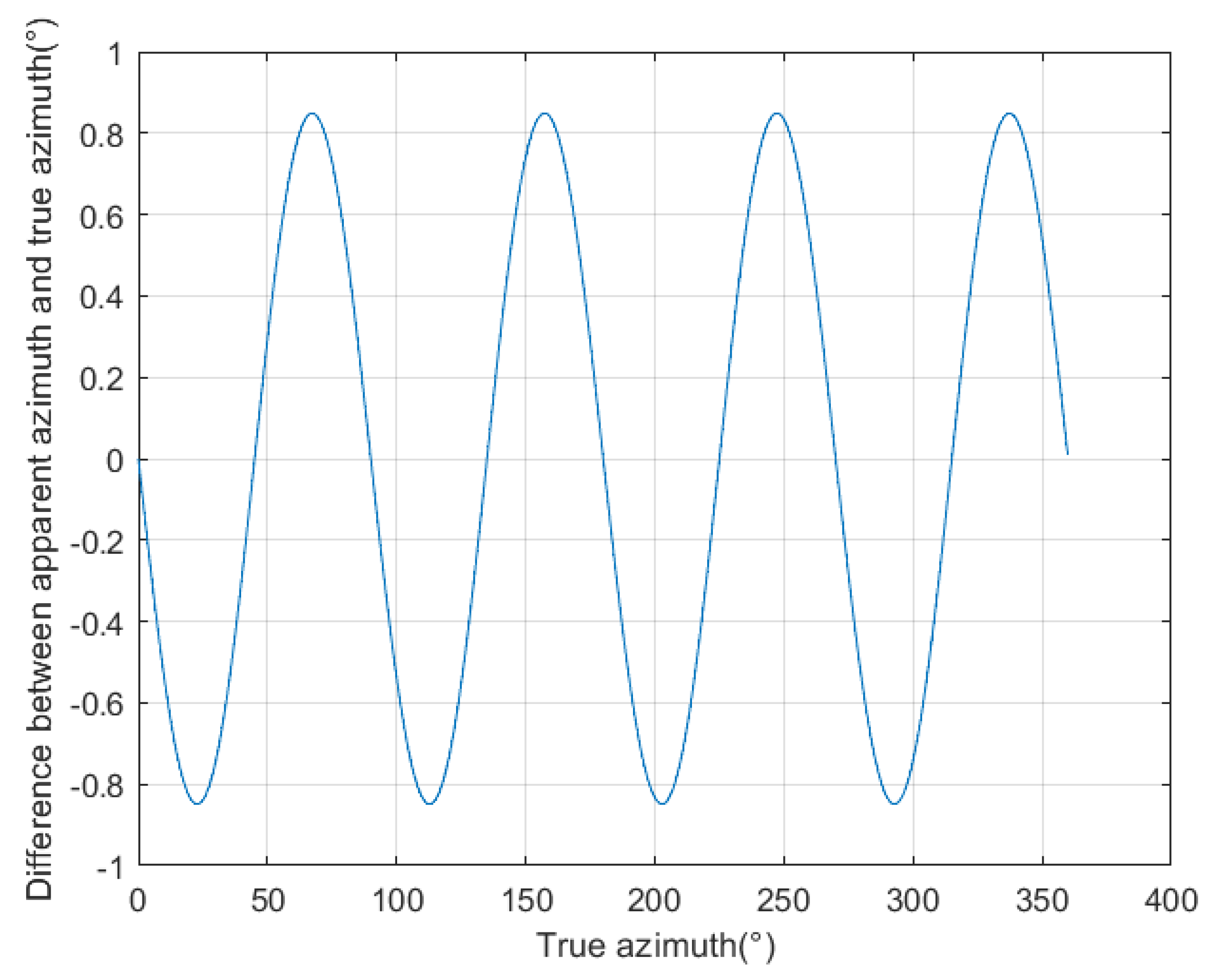
Figure 5.
The relationship between the target’s location and the error in location identification.
Figure 5.
The relationship between the target’s location and the error in location identification.
Figure 6.
Model diagram of the impact of different antenna center frequencies on the received signal of directional borehole radar.
Figure 6.
Model diagram of the impact of different antenna center frequencies on the received signal of directional borehole radar.
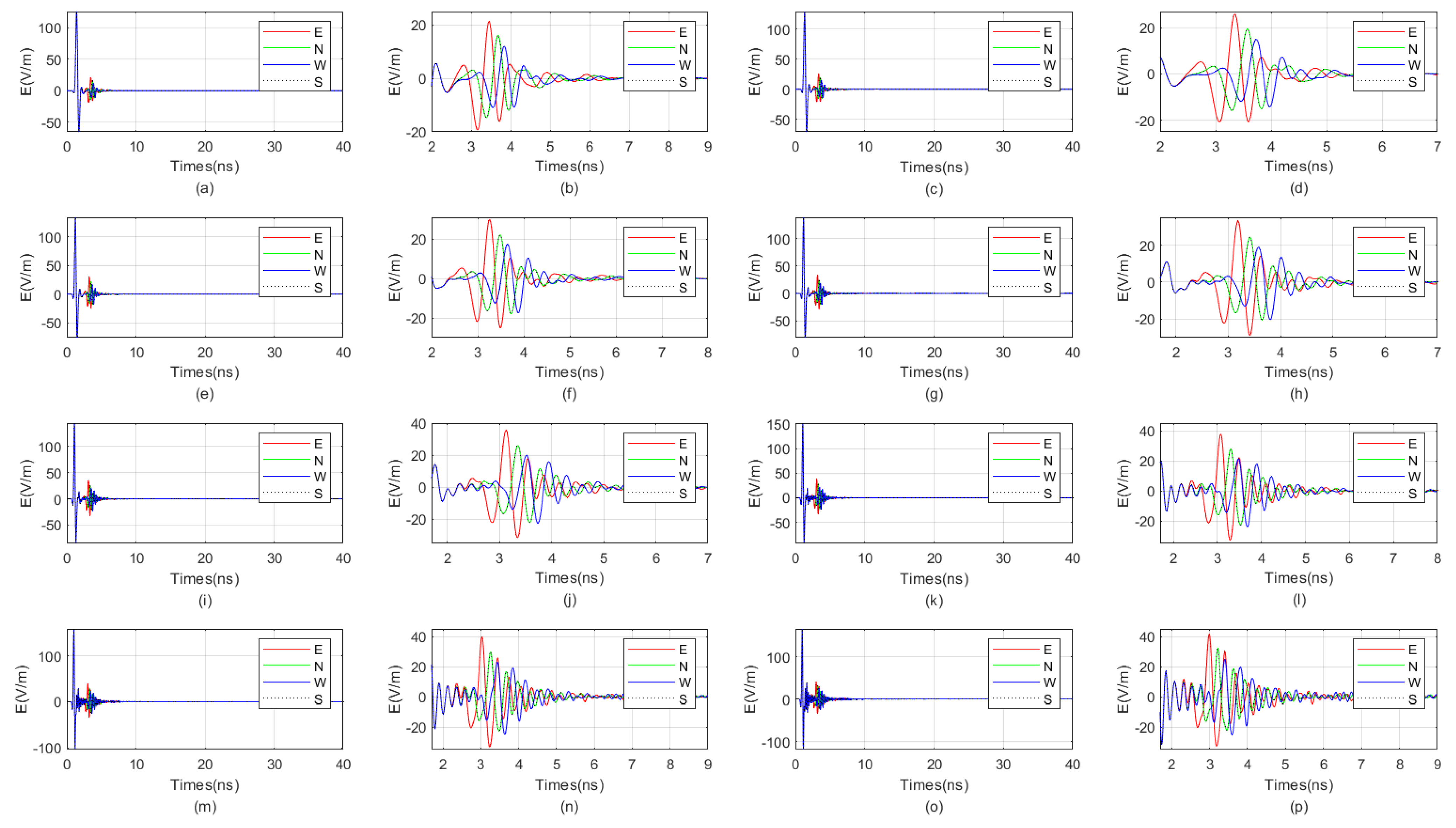
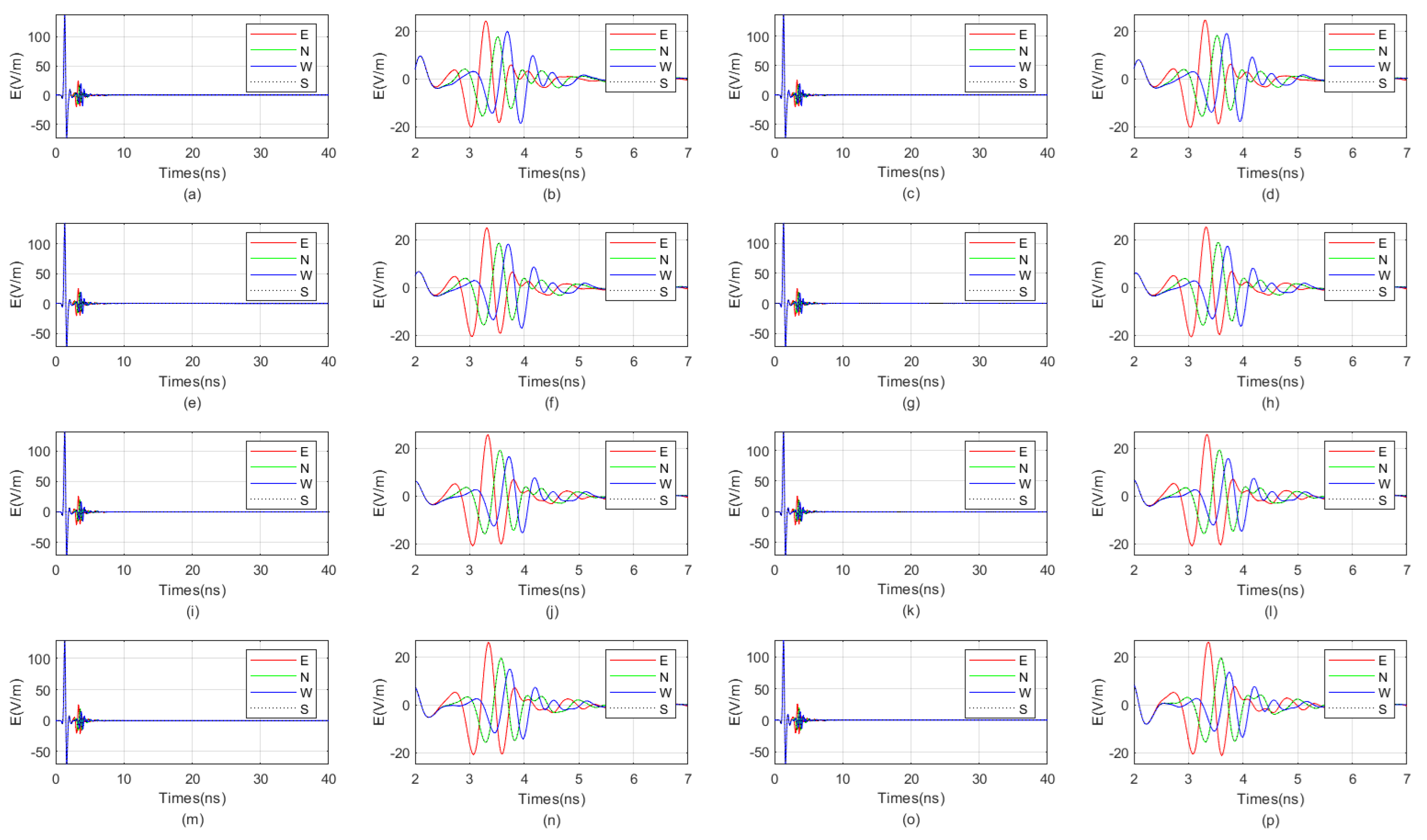
Figure 7.
The impact of the antenna center frequency, ranging from 100 MHz to 800 MHz, on the received signal of a directional borehole radar. (a) Received signal at an antenna center frequency of 100 MHz; (b) local amplification of the received signal at an antenna center frequency of 100 MHz; (c) received signal at an antenna center frequency of 200 MHz; (d) local amplification of the received signal at an antenna center frequency of 200 MHz; (e) received signal at an antenna center frequency of 300 MHz; (f) local amplification of the received signal at an antenna center frequency of 300 MHz; (g) received signal at an antenna center frequency of 400 MHz; (h) local amplification of the received signal at an antenna center frequency of 400 MHz; (i) received signal at an antenna center frequency of 500 MHz; (j) local amplification of the received signal at an antenna center frequency of 500 MHz; (k) received signal at an antenna center frequency of 600 MHz; (l) local amplification of the received signal at an antenna center frequency of 600 MHz; (m) received signal at an antenna center frequency of 700 MHz; (n) local amplification of the received signal at an antenna center frequency of 700 MHz; (o) received signal at an antenna center frequency of 800 MHz; (p) local amplification of the received signal at an antenna center frequency of 800 MHz.
Figure 7.
The impact of the antenna center frequency, ranging from 100 MHz to 800 MHz, on the received signal of a directional borehole radar. (a) Received signal at an antenna center frequency of 100 MHz; (b) local amplification of the received signal at an antenna center frequency of 100 MHz; (c) received signal at an antenna center frequency of 200 MHz; (d) local amplification of the received signal at an antenna center frequency of 200 MHz; (e) received signal at an antenna center frequency of 300 MHz; (f) local amplification of the received signal at an antenna center frequency of 300 MHz; (g) received signal at an antenna center frequency of 400 MHz; (h) local amplification of the received signal at an antenna center frequency of 400 MHz; (i) received signal at an antenna center frequency of 500 MHz; (j) local amplification of the received signal at an antenna center frequency of 500 MHz; (k) received signal at an antenna center frequency of 600 MHz; (l) local amplification of the received signal at an antenna center frequency of 600 MHz; (m) received signal at an antenna center frequency of 700 MHz; (n) local amplification of the received signal at an antenna center frequency of 700 MHz; (o) received signal at an antenna center frequency of 800 MHz; (p) local amplification of the received signal at an antenna center frequency of 800 MHz.
![Sensors 25 01252 g007 Sensors 25 01252 g007]()
Figure 8.
The impact of the antenna center frequency, ranging from 900 MHz to 1600 MHz, on the received signal of a directional borehole radar. (a) Received signal at an antenna center frequency of 900 MHz; (b) local amplification of the received signal at an antenna center frequency of 900 MHz; (c) received signal at an antenna center frequency of 1000 MHz; (d) local amplification of the received signal at an antenna center frequency of 1000 MHz; (e) received signal at an antenna center frequency of 1100 MHz; (f) local amplification of the received signal at an antenna center frequency of 1100 MHz; (g) received signal at an antenna center frequency of 1200 MHz; (h) local amplification of the received signal at an antenna center frequency of 1200 MHz; (i) received signal at an antenna center frequency of 1300 MHz; (j) local amplification of the received signal at an antenna center frequency of 1300 MHz; (k) received signal at an antenna center frequency of 1400 MHz; (l) local amplification of the received signal at an antenna center frequency of 1400 MHz; (m) received signal at an antenna center frequency of 1500 MHz; (n) local amplification of the received signal at an antenna center frequency of 1500 MHz; (o) received signal at an antenna center frequency of 1600 MHz; (p) local amplification of the received signal at an antenna center frequency of 1600 MHz.
Figure 8.
The impact of the antenna center frequency, ranging from 900 MHz to 1600 MHz, on the received signal of a directional borehole radar. (a) Received signal at an antenna center frequency of 900 MHz; (b) local amplification of the received signal at an antenna center frequency of 900 MHz; (c) received signal at an antenna center frequency of 1000 MHz; (d) local amplification of the received signal at an antenna center frequency of 1000 MHz; (e) received signal at an antenna center frequency of 1100 MHz; (f) local amplification of the received signal at an antenna center frequency of 1100 MHz; (g) received signal at an antenna center frequency of 1200 MHz; (h) local amplification of the received signal at an antenna center frequency of 1200 MHz; (i) received signal at an antenna center frequency of 1300 MHz; (j) local amplification of the received signal at an antenna center frequency of 1300 MHz; (k) received signal at an antenna center frequency of 1400 MHz; (l) local amplification of the received signal at an antenna center frequency of 1400 MHz; (m) received signal at an antenna center frequency of 1500 MHz; (n) local amplification of the received signal at an antenna center frequency of 1500 MHz; (o) received signal at an antenna center frequency of 1600 MHz; (p) local amplification of the received signal at an antenna center frequency of 1600 MHz.
![Sensors 25 01252 g008 Sensors 25 01252 g008]()
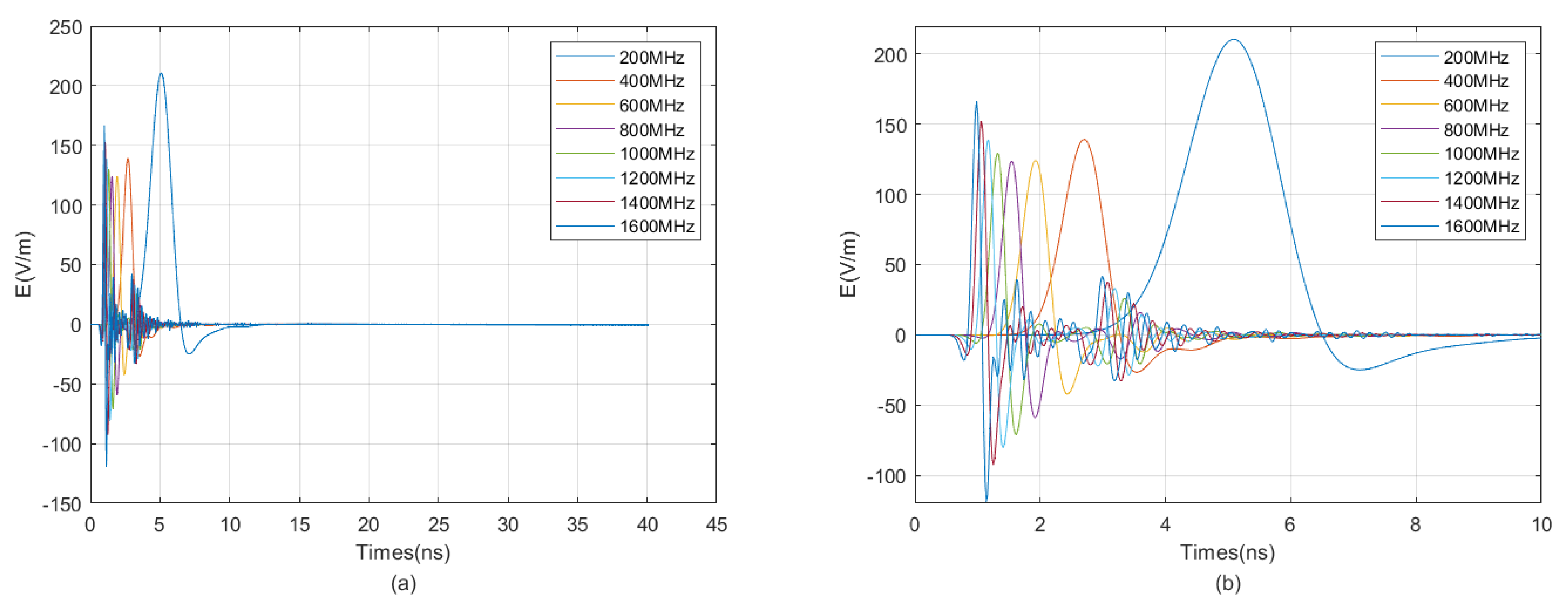
Figure 9.
Signal reception diagram of east-facing receiving antennas at various frequencies. (a) Global map; (b) local enlarged view.
Figure 9.
Signal reception diagram of east-facing receiving antennas at various frequencies. (a) Global map; (b) local enlarged view.
Figure 10.
When the target is located in the blind zone, the influence of the relative permittivity of the fluid in the borehole, ranging from 3 to 7, on the received signal of the directional borehole radar. (a) The received signal has a relative permittivity of 3 for the fluid inside the borehole; (b) local amplification diagram of the received signal with a relative permittivity of 3 for the fluid in the borehole; (c) the received signal has a relative permittivity of 3.5 for the fluid inside the borehole; (d) local amplification diagram of the received signal with a relative permittivity of 3.5 for the fluid in the borehole; (e) the received signal has a relative permittivity of 4 for the fluid inside the borehole; (f) local amplification diagram of the received signal with a relative permittivity of 4 for the fluid in the borehole; (g) the received signal has a relative permittivity of 4.5 for the fluid inside the borehole; (h) local amplification diagram of the received signal with a relative permittivity of 4.5 for the fluid in the borehole; (i) the received signal has a relative permittivity of 5 for the fluid inside the borehole; (j) local amplification diagram of the received signal with a relative permittivity of 5 for the fluid in the borehole; (k) the received signal has a relative permittivity of 5.5 for the fluid inside the borehole; (l) local amplification diagram of the received signal with a relative permittivity of 5.5 for the fluid in the borehole; (m) the received signal has a relative permittivity of 6 for the fluid inside the borehole; (n) local amplification diagram of the received signal with a relative permittivity of 6 for the fluid in the borehole; (o) the received signal has a relative permittivity of 7 for the fluid inside the borehole; (p) local amplification diagram of the received signal with a relative permittivity of 7 for the fluid in the borehole.
Figure 10.
When the target is located in the blind zone, the influence of the relative permittivity of the fluid in the borehole, ranging from 3 to 7, on the received signal of the directional borehole radar. (a) The received signal has a relative permittivity of 3 for the fluid inside the borehole; (b) local amplification diagram of the received signal with a relative permittivity of 3 for the fluid in the borehole; (c) the received signal has a relative permittivity of 3.5 for the fluid inside the borehole; (d) local amplification diagram of the received signal with a relative permittivity of 3.5 for the fluid in the borehole; (e) the received signal has a relative permittivity of 4 for the fluid inside the borehole; (f) local amplification diagram of the received signal with a relative permittivity of 4 for the fluid in the borehole; (g) the received signal has a relative permittivity of 4.5 for the fluid inside the borehole; (h) local amplification diagram of the received signal with a relative permittivity of 4.5 for the fluid in the borehole; (i) the received signal has a relative permittivity of 5 for the fluid inside the borehole; (j) local amplification diagram of the received signal with a relative permittivity of 5 for the fluid in the borehole; (k) the received signal has a relative permittivity of 5.5 for the fluid inside the borehole; (l) local amplification diagram of the received signal with a relative permittivity of 5.5 for the fluid in the borehole; (m) the received signal has a relative permittivity of 6 for the fluid inside the borehole; (n) local amplification diagram of the received signal with a relative permittivity of 6 for the fluid in the borehole; (o) the received signal has a relative permittivity of 7 for the fluid inside the borehole; (p) local amplification diagram of the received signal with a relative permittivity of 7 for the fluid in the borehole.
![Sensors 25 01252 g010 Sensors 25 01252 g010]()
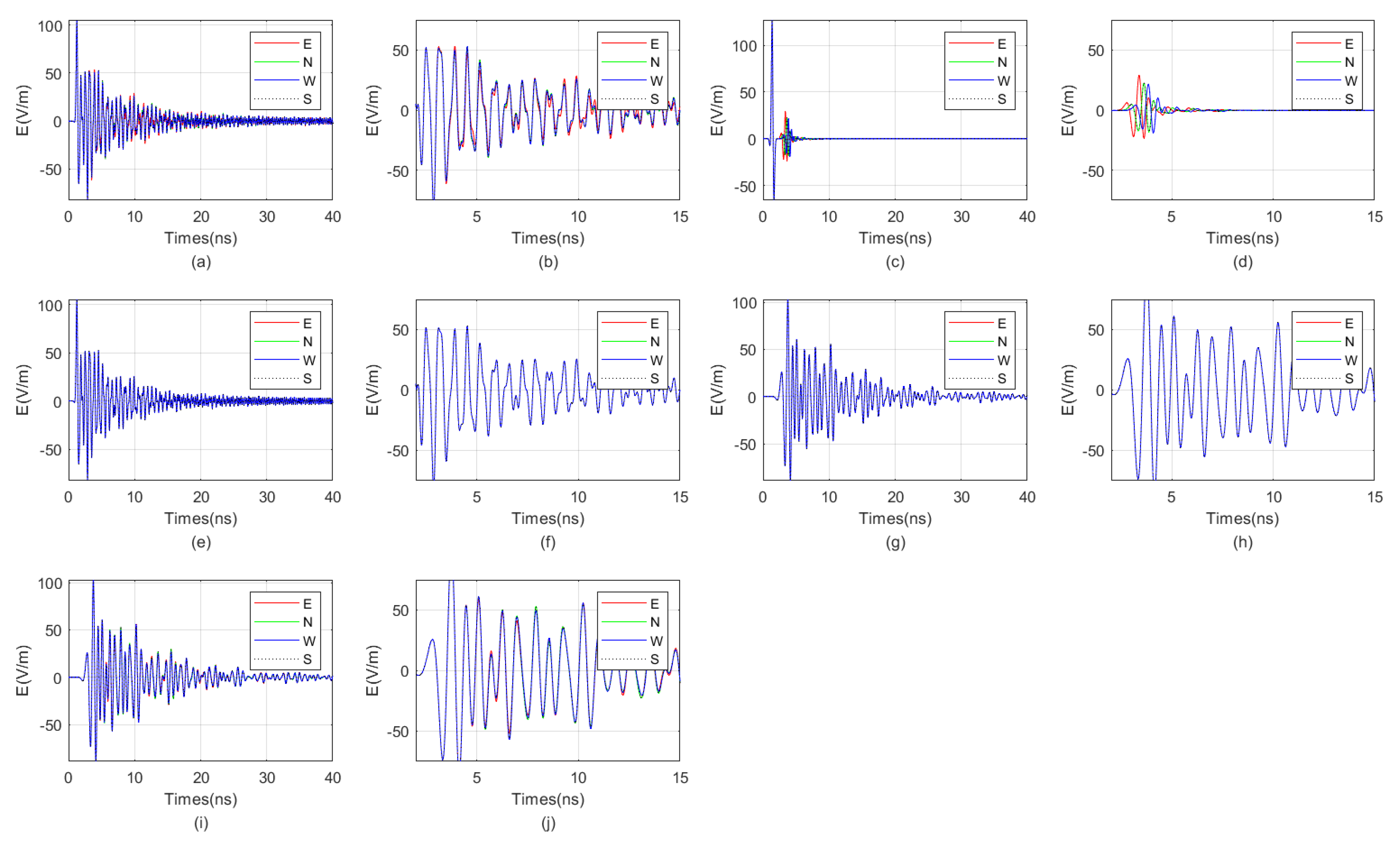
Figure 11.
When the target is located in the blind zones, the influence of the relative permittivity of the fluid in the borehole, ranging from 8 to 40, on the received signal of the directional borehole radar. (a) The received signal has a relative permittivity of 8 for the fluid inside the borehole; (b) local amplification diagram of the received signal with a relative permittivity of 8 for the fluid in the borehole; (c) the received signal has a relative permittivity of 9 for the fluid inside the borehole; (d) local amplification diagram of the received signal with a relative permittivity of 9 for the fluid in the borehole; (e) the received signal has a relative permittivity of 10 for the fluid inside the borehole; (f) local amplification diagram of the received signal with a relative permittivity of 10 for the fluid in the borehole; (g) the received signal has a relative permittivity of 15 for the fluid inside the borehole; (h) local amplification diagram of the received signal with a relative permittivity of 15 for the fluid in the borehole; (i) the received signal has a relative permittivity of 20 for the fluid inside the borehole; (j) local amplification diagram of the received signal with a relative permittivity of 20 for the fluid in the borehole; (k) the received signal has a relative permittivity of 25 for the fluid inside the borehole; (l) local amplification diagram of the received signal with a relative permittivity of 25 for the fluid in the borehole; (m) the received signal has a relative permittivity of 30 for the fluid inside the borehole; (n) local amplification diagram of the received signal with a relative permittivity of 30 for the fluid in the borehole; (o) the received signal has a relative permittivity of 40 for the fluid inside the borehole; (p) local amplification diagram of the received signal with a relative permittivity of 40 for the fluid in the borehole.
Figure 11.
When the target is located in the blind zones, the influence of the relative permittivity of the fluid in the borehole, ranging from 8 to 40, on the received signal of the directional borehole radar. (a) The received signal has a relative permittivity of 8 for the fluid inside the borehole; (b) local amplification diagram of the received signal with a relative permittivity of 8 for the fluid in the borehole; (c) the received signal has a relative permittivity of 9 for the fluid inside the borehole; (d) local amplification diagram of the received signal with a relative permittivity of 9 for the fluid in the borehole; (e) the received signal has a relative permittivity of 10 for the fluid inside the borehole; (f) local amplification diagram of the received signal with a relative permittivity of 10 for the fluid in the borehole; (g) the received signal has a relative permittivity of 15 for the fluid inside the borehole; (h) local amplification diagram of the received signal with a relative permittivity of 15 for the fluid in the borehole; (i) the received signal has a relative permittivity of 20 for the fluid inside the borehole; (j) local amplification diagram of the received signal with a relative permittivity of 20 for the fluid in the borehole; (k) the received signal has a relative permittivity of 25 for the fluid inside the borehole; (l) local amplification diagram of the received signal with a relative permittivity of 25 for the fluid in the borehole; (m) the received signal has a relative permittivity of 30 for the fluid inside the borehole; (n) local amplification diagram of the received signal with a relative permittivity of 30 for the fluid in the borehole; (o) the received signal has a relative permittivity of 40 for the fluid inside the borehole; (p) local amplification diagram of the received signal with a relative permittivity of 40 for the fluid in the borehole.
![Sensors 25 01252 g011 Sensors 25 01252 g011]()
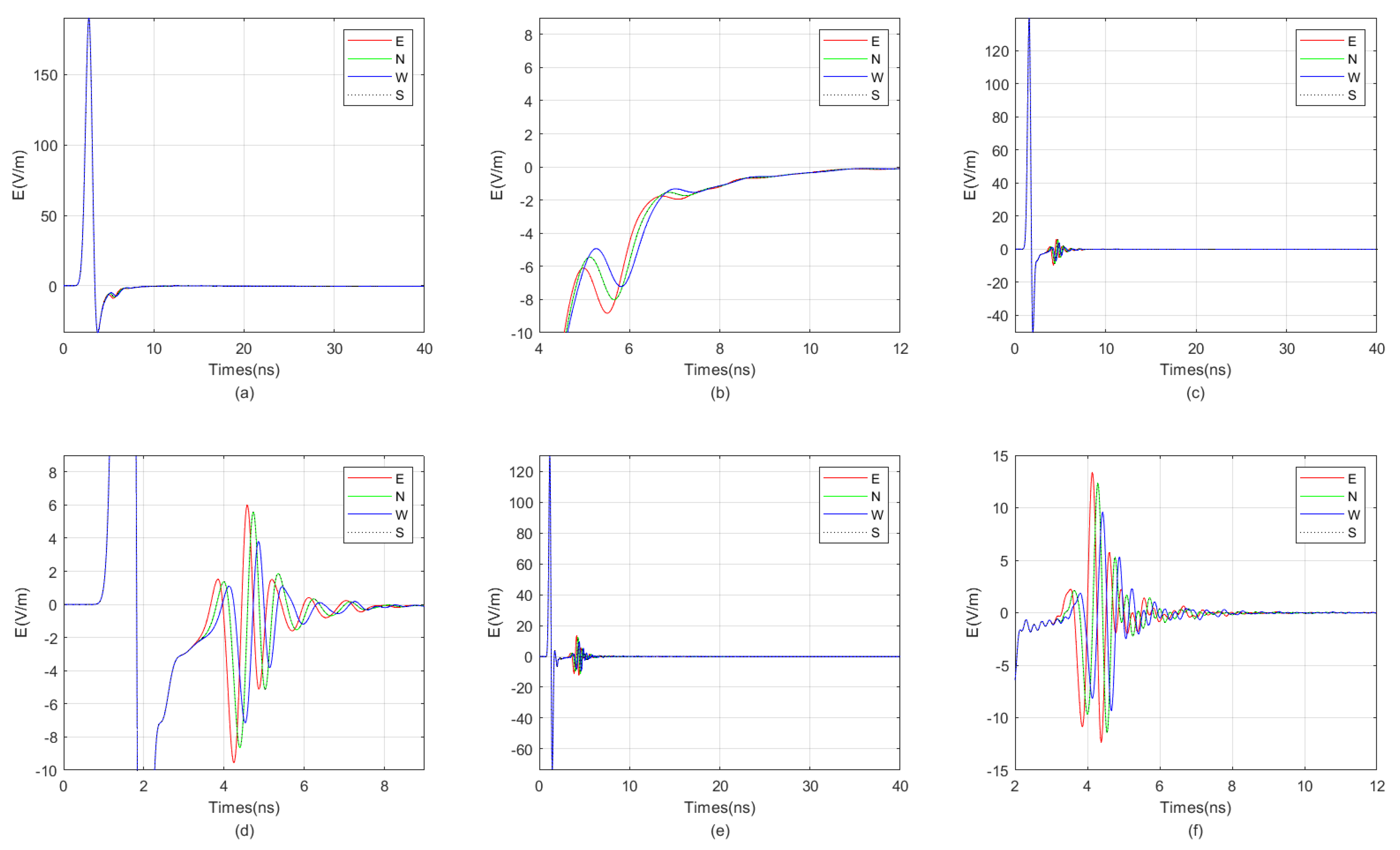
Figure 12.
Numerical simulation results of the cause of resonance when the relative permittivity of the fluid in the borehole is 80. There are (a) “borehole, probe tube, and target” conditions; (b) local magnification of “borehole, probe tube, and target” conditions; (c) “no borehole, no probe tube, but with target” conditions; (d) local magnification of “no borehole, no probe tube, but with target” conditions; (e) “with a wellbore and a probe tube, but no target” conditions; (f) local magnification of “with a wellbore and a probe tube, but no target” conditions; (g) “with a borehole, no probe tube, and no target” conditions; (h) local magnification of “with a borehole, no probe tube, and no target” conditions; (i) “with a borehole but no probe tube, there is a target” conditions; (j) local magnification of “with a borehole but no probe tube, there is a target” conditions.
Figure 12.
Numerical simulation results of the cause of resonance when the relative permittivity of the fluid in the borehole is 80. There are (a) “borehole, probe tube, and target” conditions; (b) local magnification of “borehole, probe tube, and target” conditions; (c) “no borehole, no probe tube, but with target” conditions; (d) local magnification of “no borehole, no probe tube, but with target” conditions; (e) “with a wellbore and a probe tube, but no target” conditions; (f) local magnification of “with a wellbore and a probe tube, but no target” conditions; (g) “with a borehole, no probe tube, and no target” conditions; (h) local magnification of “with a borehole, no probe tube, and no target” conditions; (i) “with a borehole but no probe tube, there is a target” conditions; (j) local magnification of “with a borehole but no probe tube, there is a target” conditions.
Figure 13.
Model diagram of the influence of target distance on the received signal of directional borehole radar.
Figure 13.
Model diagram of the influence of target distance on the received signal of directional borehole radar.
Figure 14.
When the target distances are 2.25 m and 1.75 m, the directional borehole radar receives signals. (a) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 2.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 2.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 2.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified. (g) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 300 MHz. (h) When the target distance is 1.75 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (i) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 600 MHz. (j) When the target distance is 1.75 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (k) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 900 MHz. (l) When the target distance is 1.75 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
Figure 14.
When the target distances are 2.25 m and 1.75 m, the directional borehole radar receives signals. (a) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 2.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 2.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 2.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 2.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified. (g) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 300 MHz. (h) When the target distance is 1.75 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (i) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 600 MHz. (j) When the target distance is 1.75 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (k) When the target distance is 1.75 m, the antenna receives a signal with a center frequency of 900 MHz. (l) When the target distance is 1.75 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
![Sensors 25 01252 g014 Sensors 25 01252 g014]()
Figure 15.
When the target distances are 1.25 m and 0.75 m, the directional borehole radar receives signals. (a) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 1.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 1.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 1.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified. (g) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 300 MHz. (h) When the target distance is 0.75 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (i) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 600 MHz. (j) When the target distance is 0.75 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (k) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 900 MHz. (l) When the target distance is 0.75 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
Figure 15.
When the target distances are 1.25 m and 0.75 m, the directional borehole radar receives signals. (a) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 1.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 1.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 1.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 1.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified. (g) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 300 MHz. (h) When the target distance is 0.75 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (i) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 600 MHz. (j) When the target distance is 0.75 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (k) When the target distance is 0.75 m, the antenna receives a signal with a center frequency of 900 MHz. (l) When the target distance is 0.75 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
![Sensors 25 01252 g015 Sensors 25 01252 g015]()
Figure 16.
When the target distances are 0.25 m, the directional borehole radar receives signals. (a) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 0.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 0.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 0.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
Figure 16.
When the target distances are 0.25 m, the directional borehole radar receives signals. (a) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 300 MHz. (b) When the target distance is 0.25 m, the antenna center frequency is 300 MHz, and the received signal is locally amplified. (c) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 600 MHz. (d) When the target distance is 0.25 m, the antenna center frequency is 600 MHz, and the received signal is locally amplified. (e) When the target distance is 0.25 m, the antenna receives a signal with a center frequency of 900 MHz. (f) When the target distance is 0.25 m, the antenna center frequency is 900 MHz, and the received signal is locally amplified.
Table 1.
Conclusion of this numerical simulation.
Table 1.
Conclusion of this numerical simulation.
| Blind Zones Range | Maximum Error Direction | Best Antenna Center Frequency | Optimal Borehole Fluid | The Farther the Target Distance |
|---|
| 2 m (1 GHz) | Odd multiples of 22.5° | 600 MHz–1100 MHz | Oil-base mud | the lower the optimal frequency |