Detection Method for Bolt Loosening Based on Summation Coefficient of Absolute Spectrum Ratio
Abstract
:1. Introduction
- A novel SCASR-based loosening detection indicator is proposed, providing an intuitive and effective measurement of bolt loosening severity, particularly excelling in early-stage detection.
- The method enables precise detection of bolt loosening severity using vibration and rotational speed data in the low-frequency range, facilitating a significantly more streamlined detection of complex bolted connection structures in motor systems.
- This method demonstrates excellent detection performance across vibration data in all three axes, further validating its applicability and reliability in multi-axial detection scenarios.
2. Impact of Bolt Loosening on Structural Natural Frequencies
2.1. Bolt Loosening Detection Principle and Simulation Verification
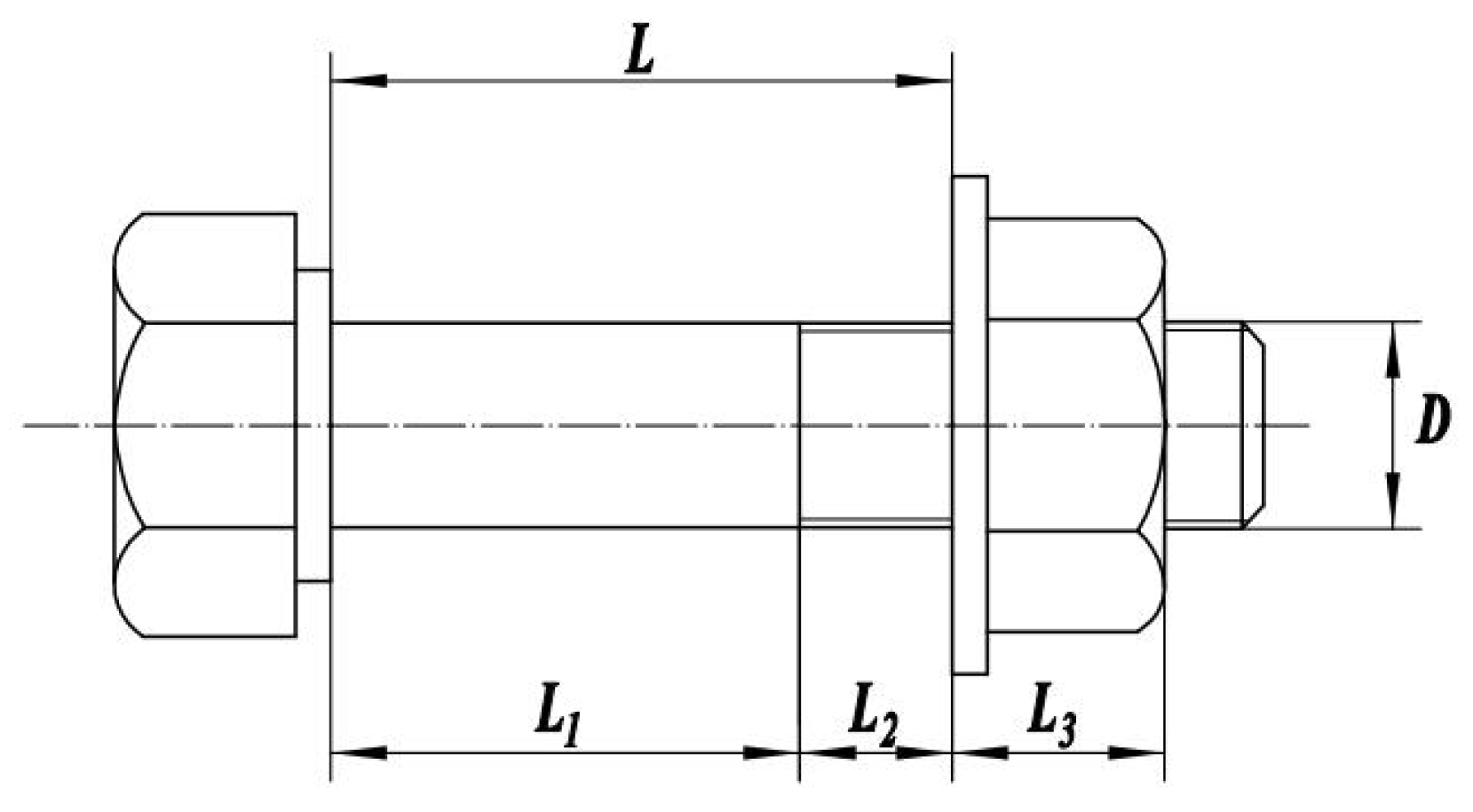
2.1.1. Calculation of Stiffness and Damping in Bolt Connections
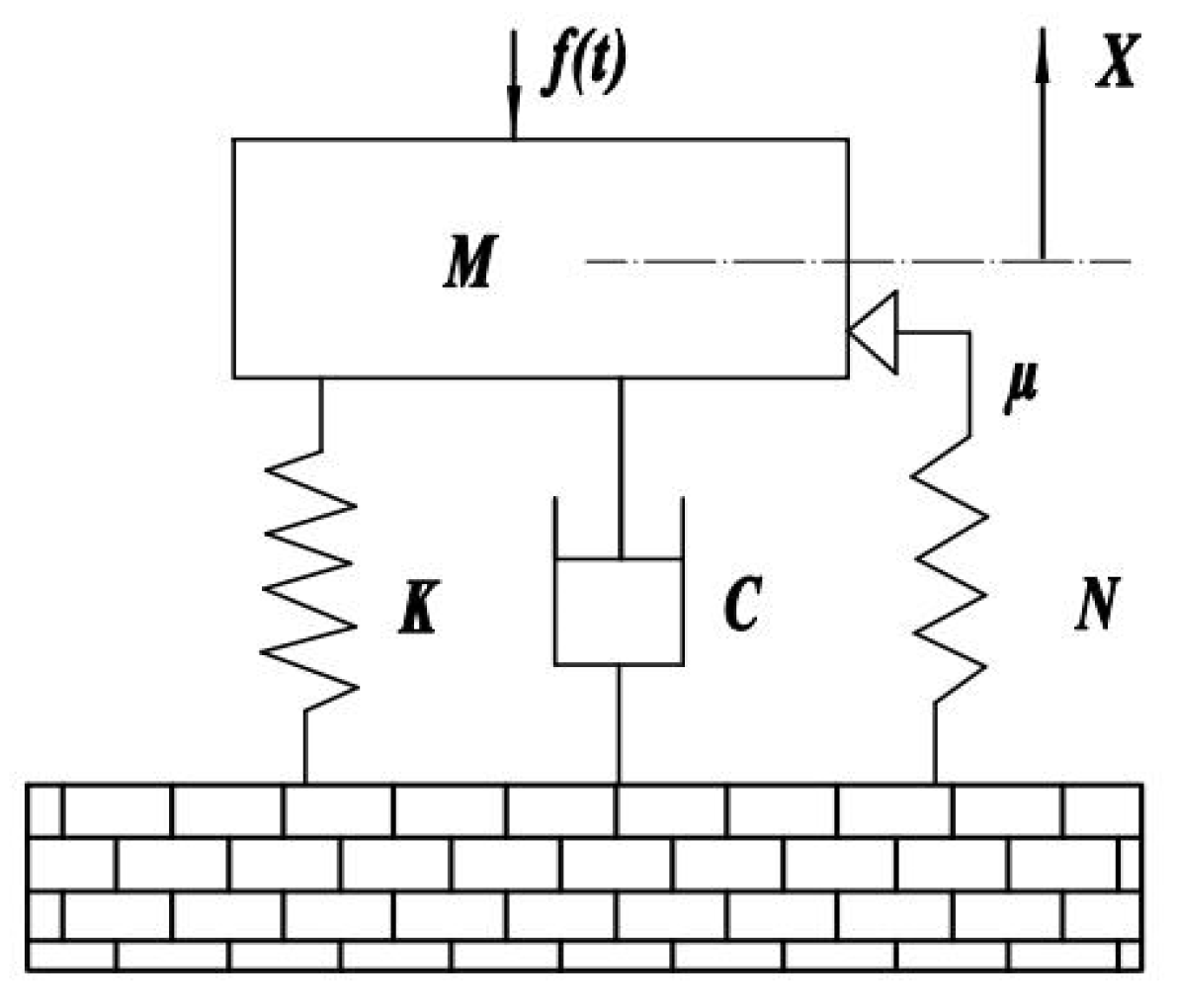
2.1.2. Motor Vibration Equation
2.1.3. Mechanical Model of the Motor Foundation
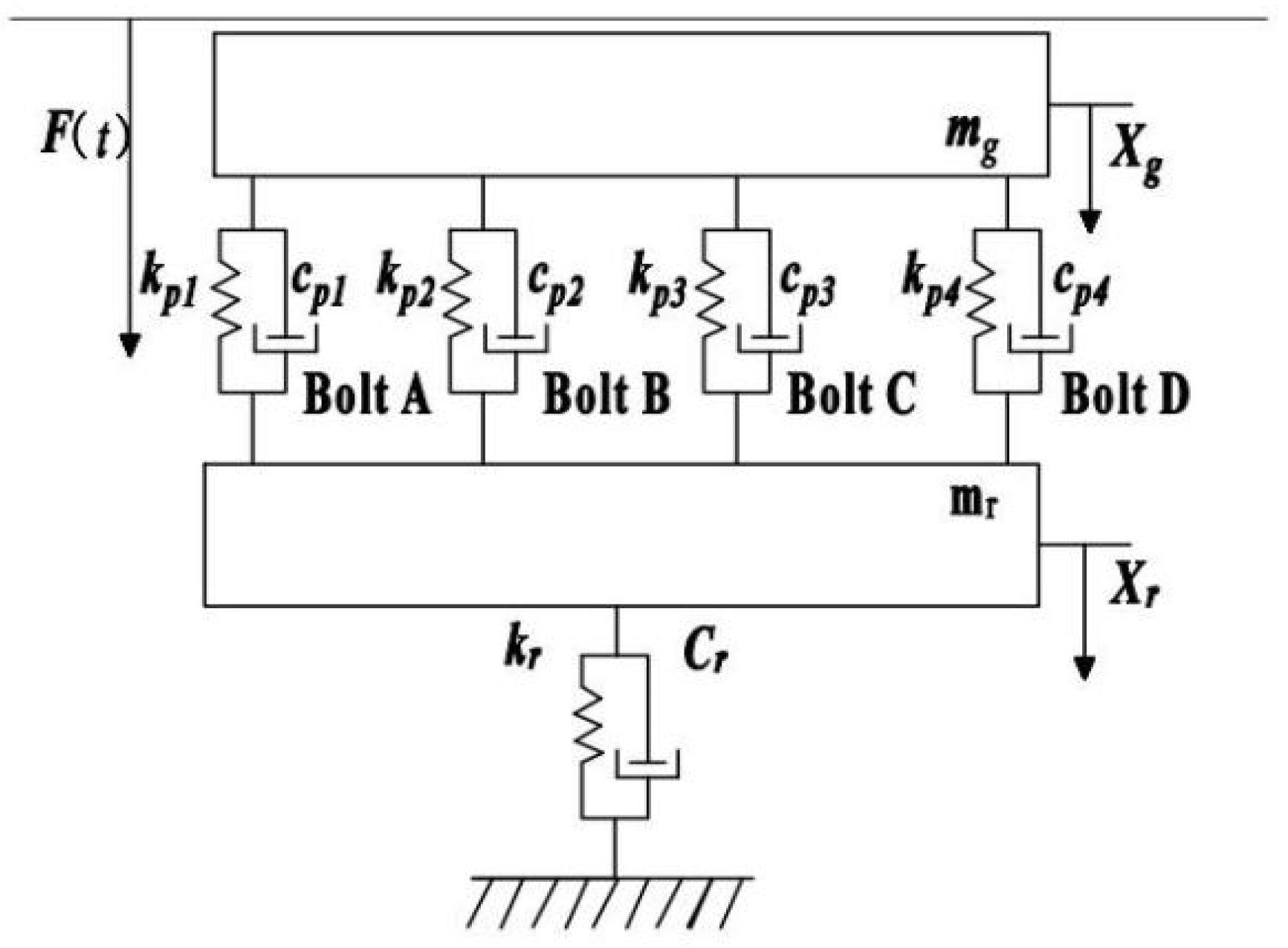
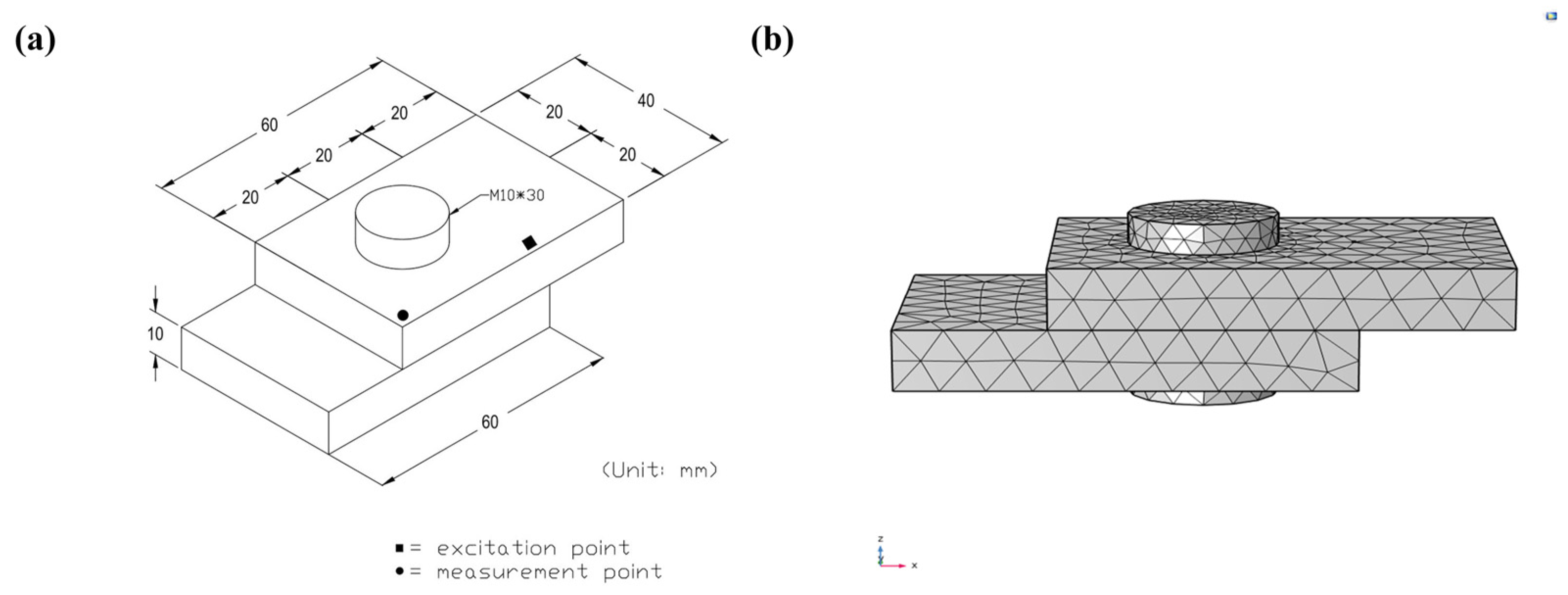
2.1.4. Simulation of the Experimental Multi-Bolt Connection System
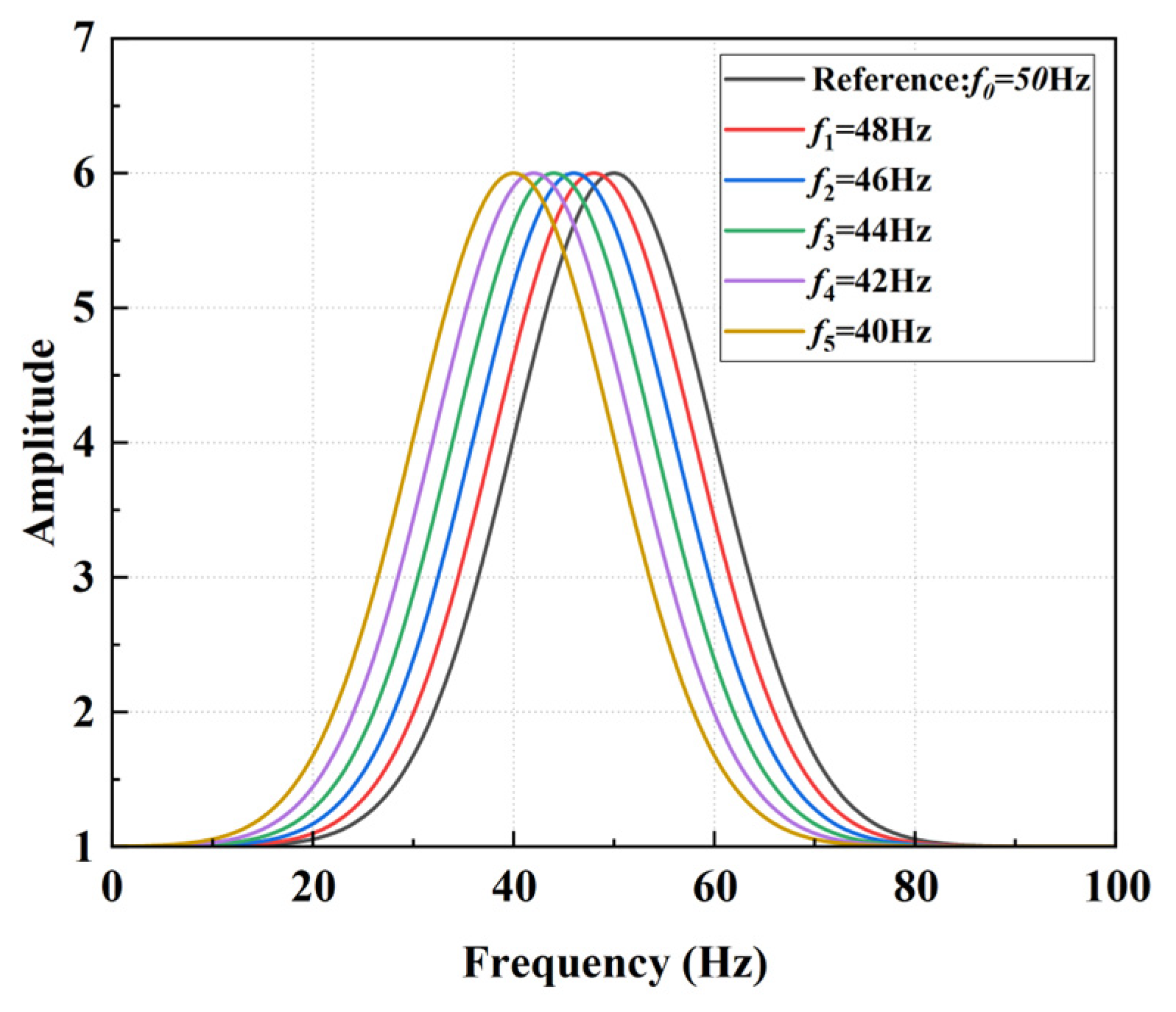
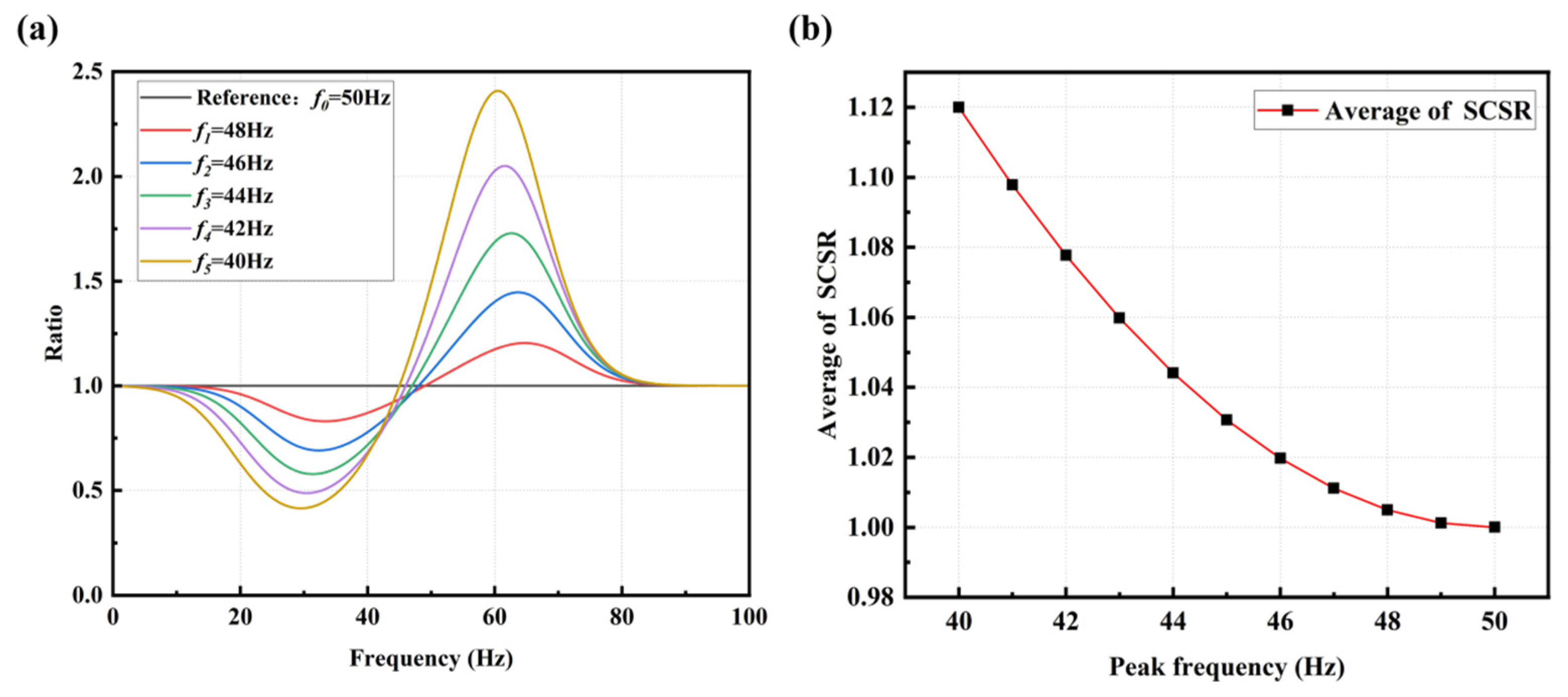
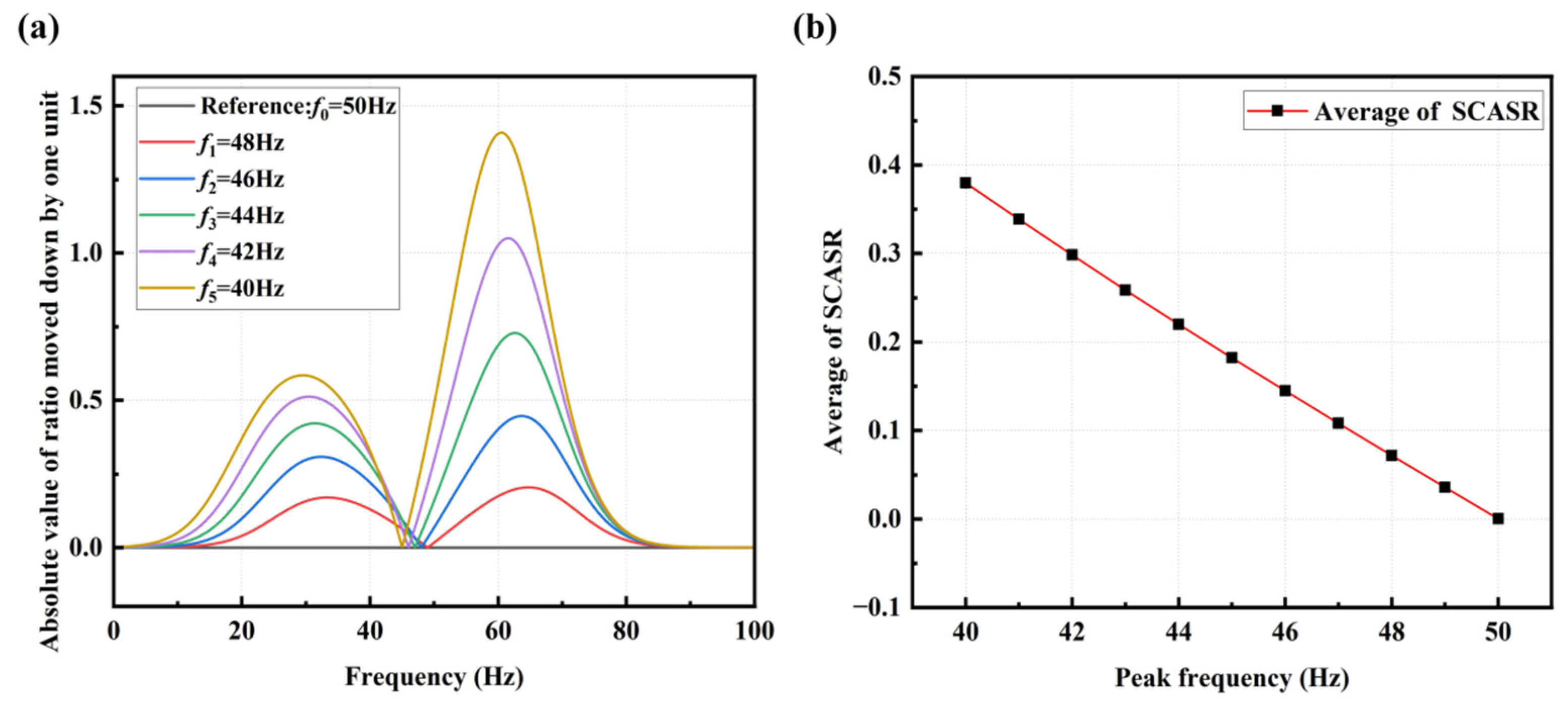
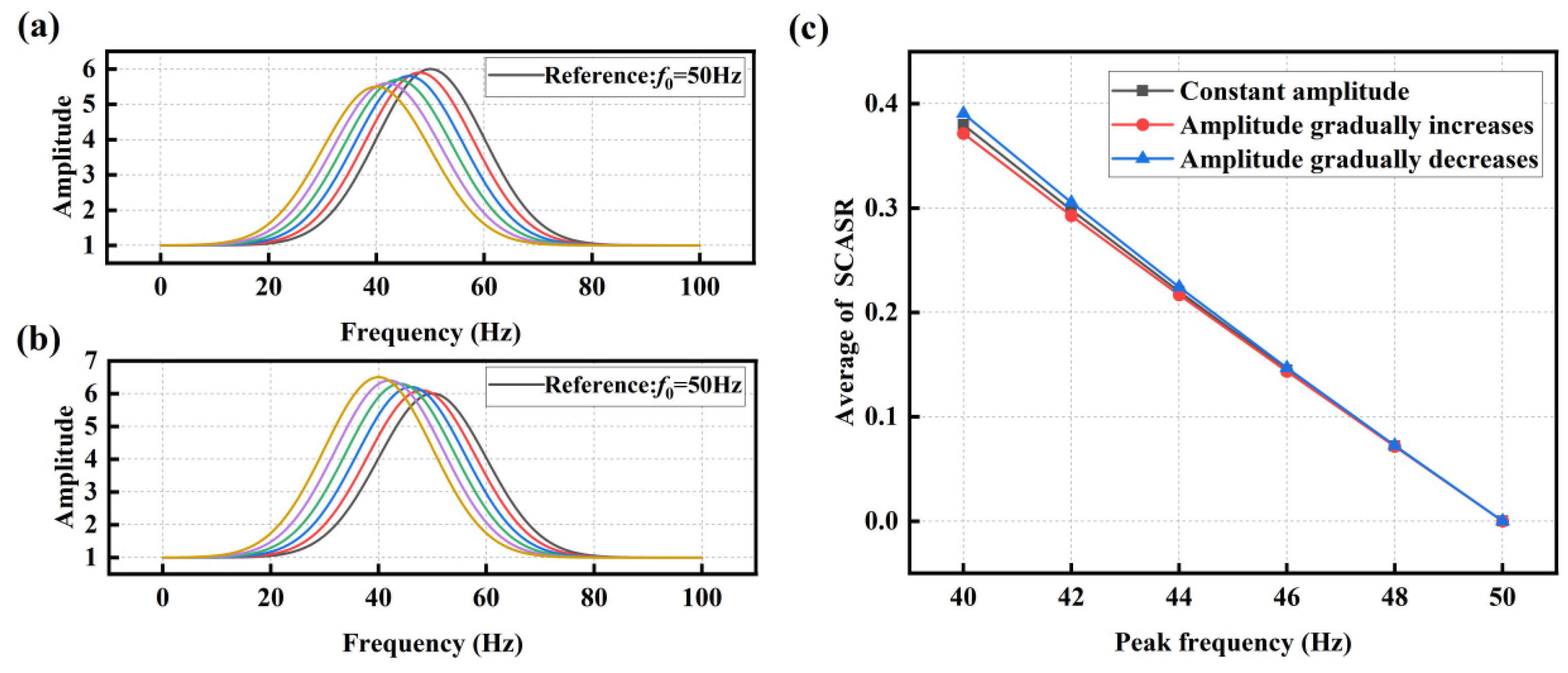
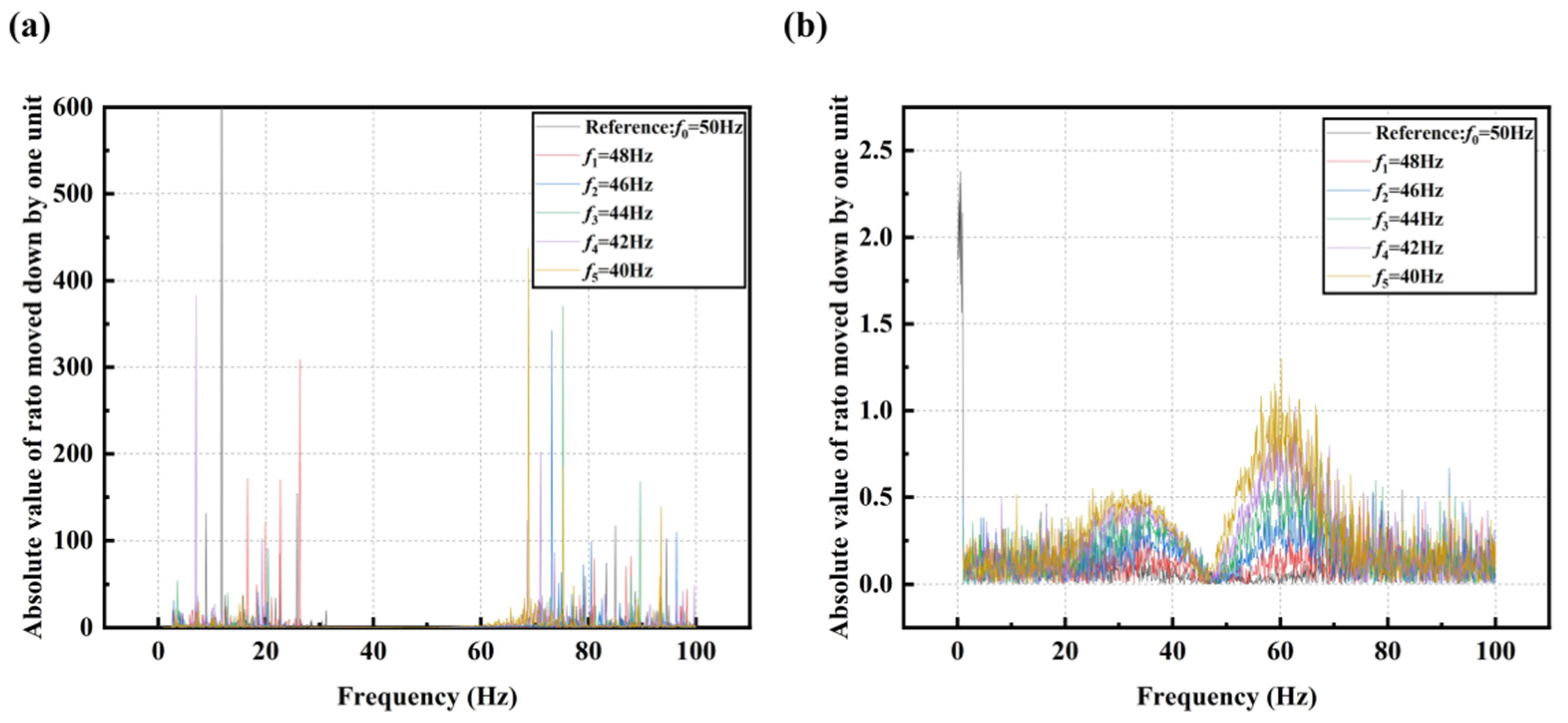
2.2. Principle of Detection Method Based on Summation Coefficient of Absolute Spectrum Ratio
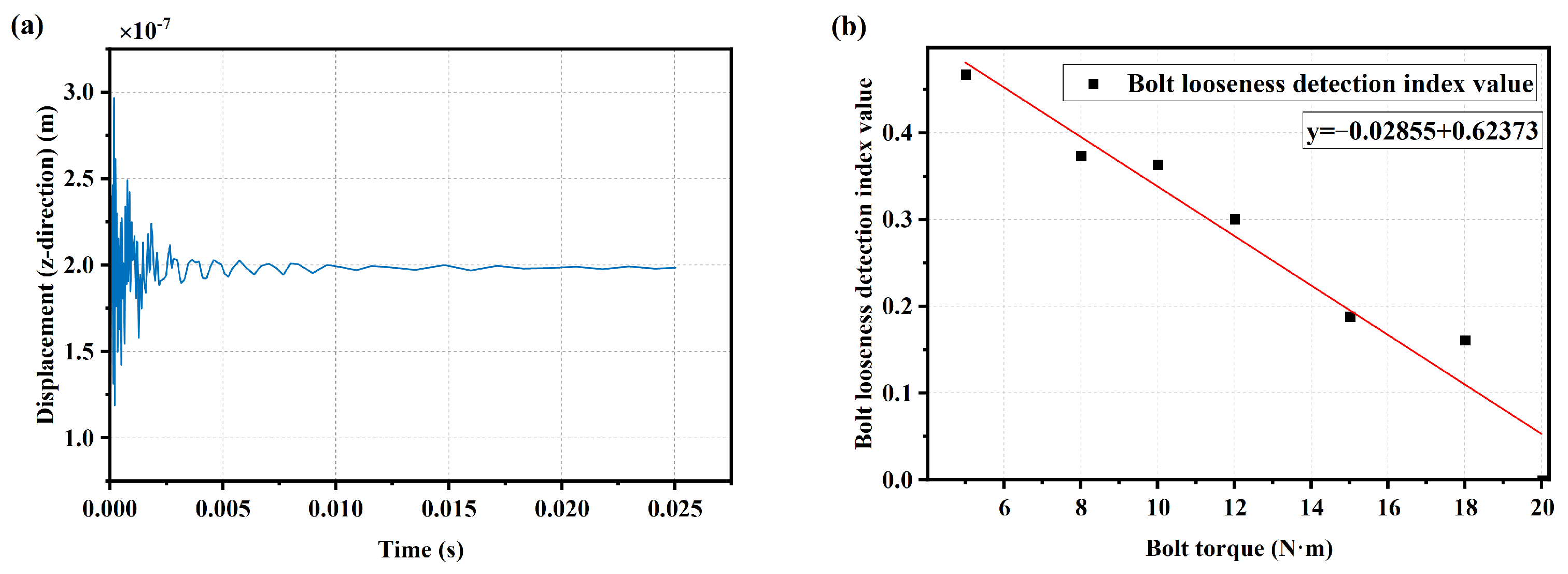
2.3. Simulation Validation of Detection Method in Simple Bolted Joint Structure
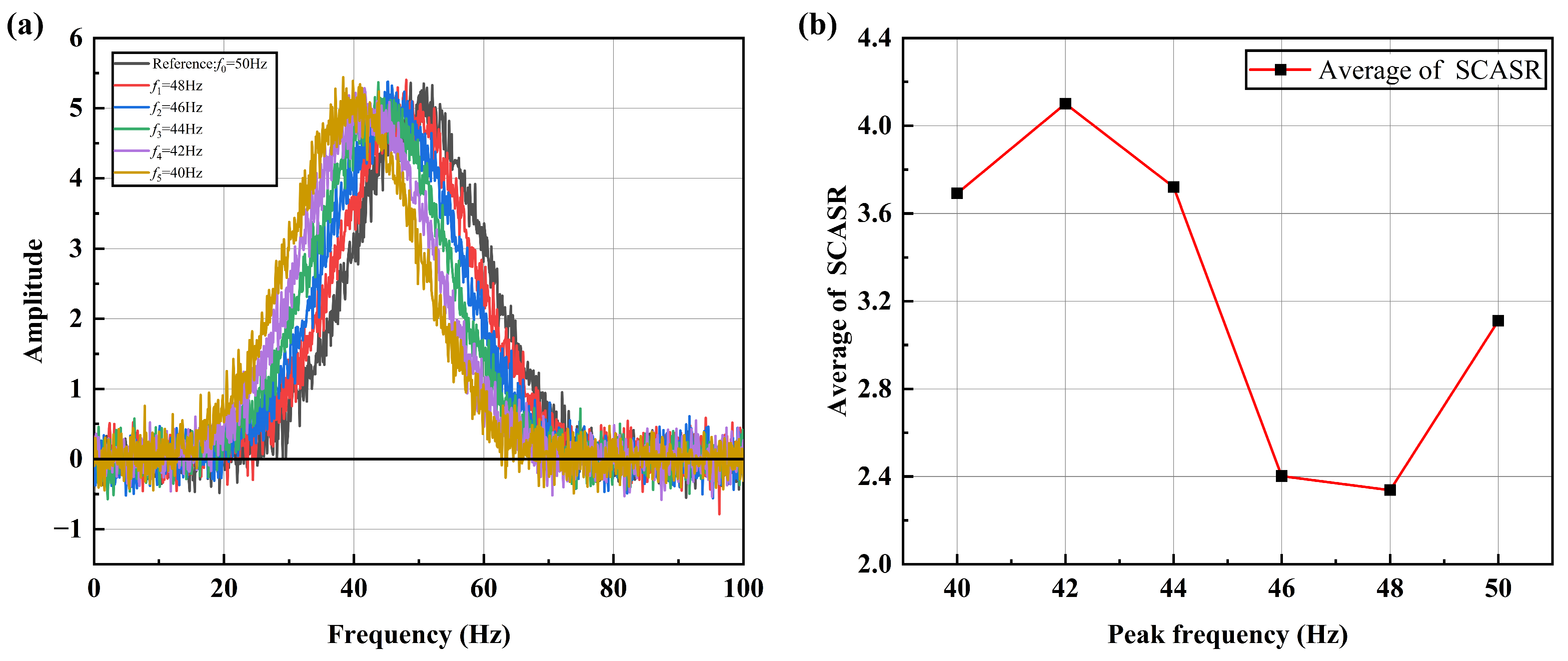
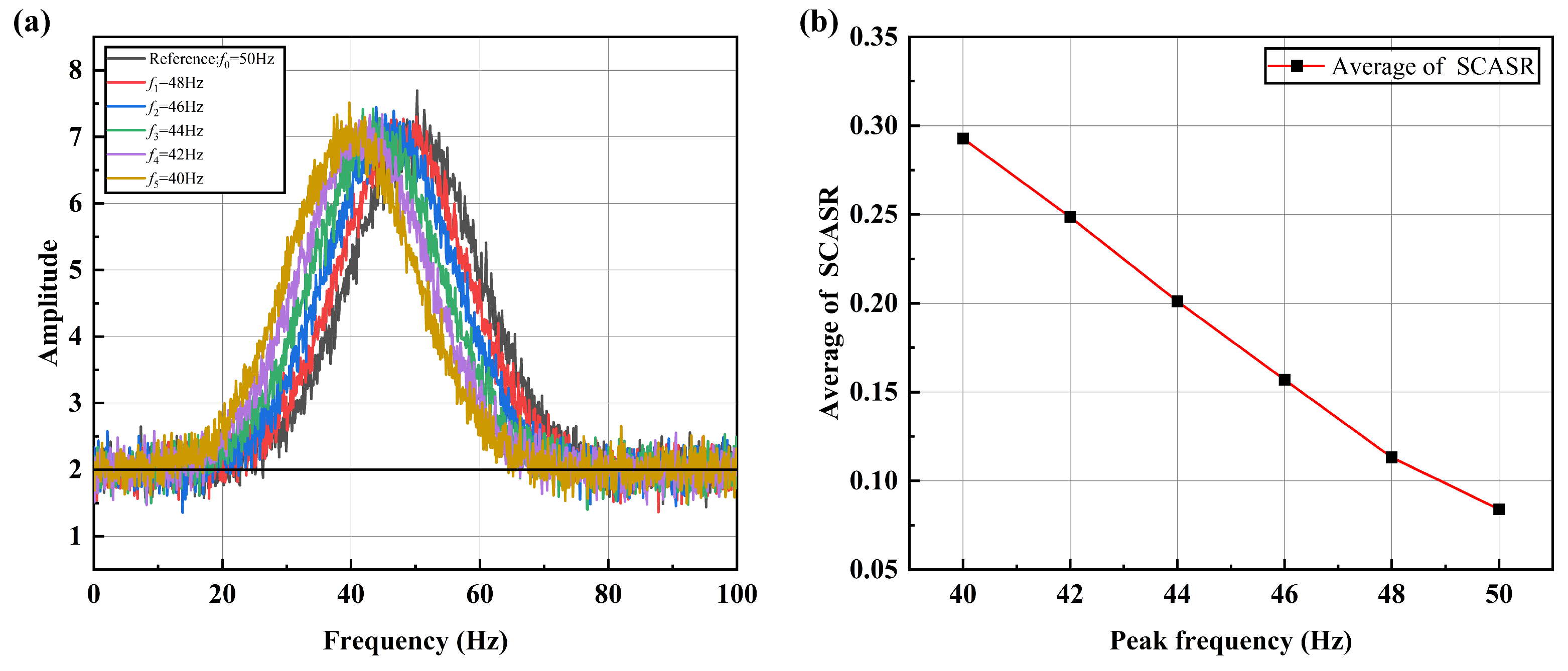
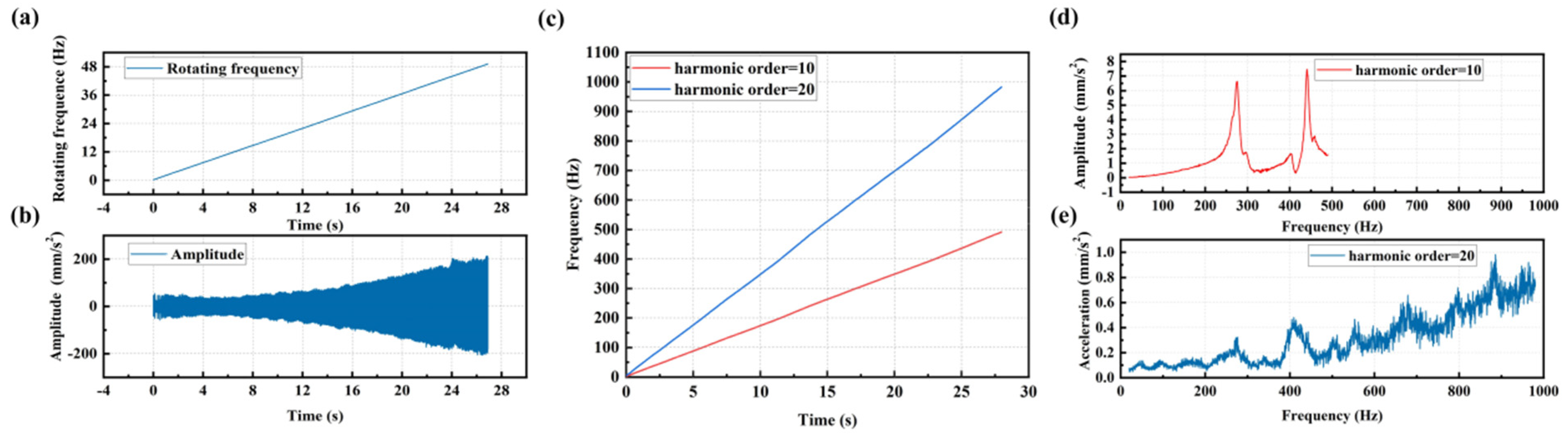
2.4. Spectrum Curve Analysis Based on Chirp Fourier Transform
2.4.1. The Principle of the Chirp Fourier Transform
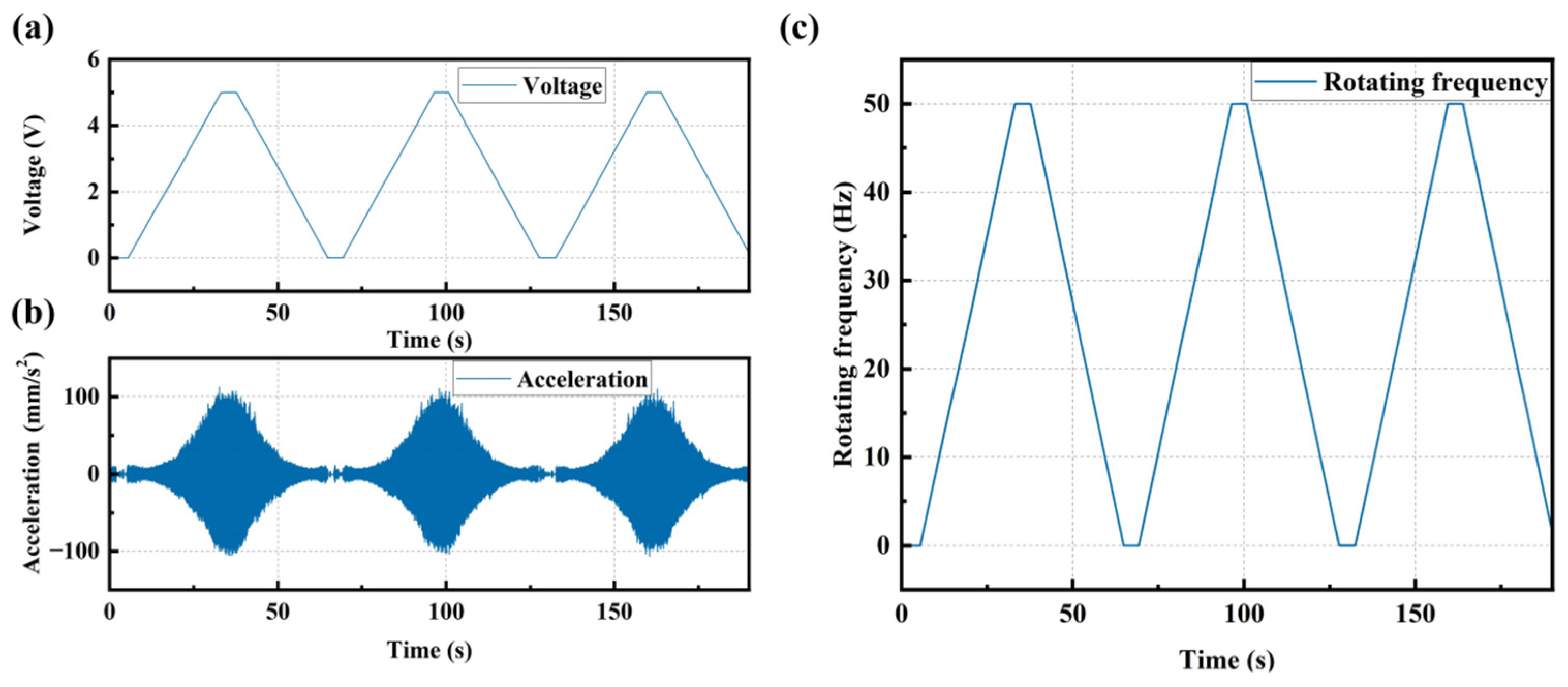
2.4.2. Spectrum Analysis for Vibration Data Using Linear-Frequency Excitation
3. Experiments and Discussions
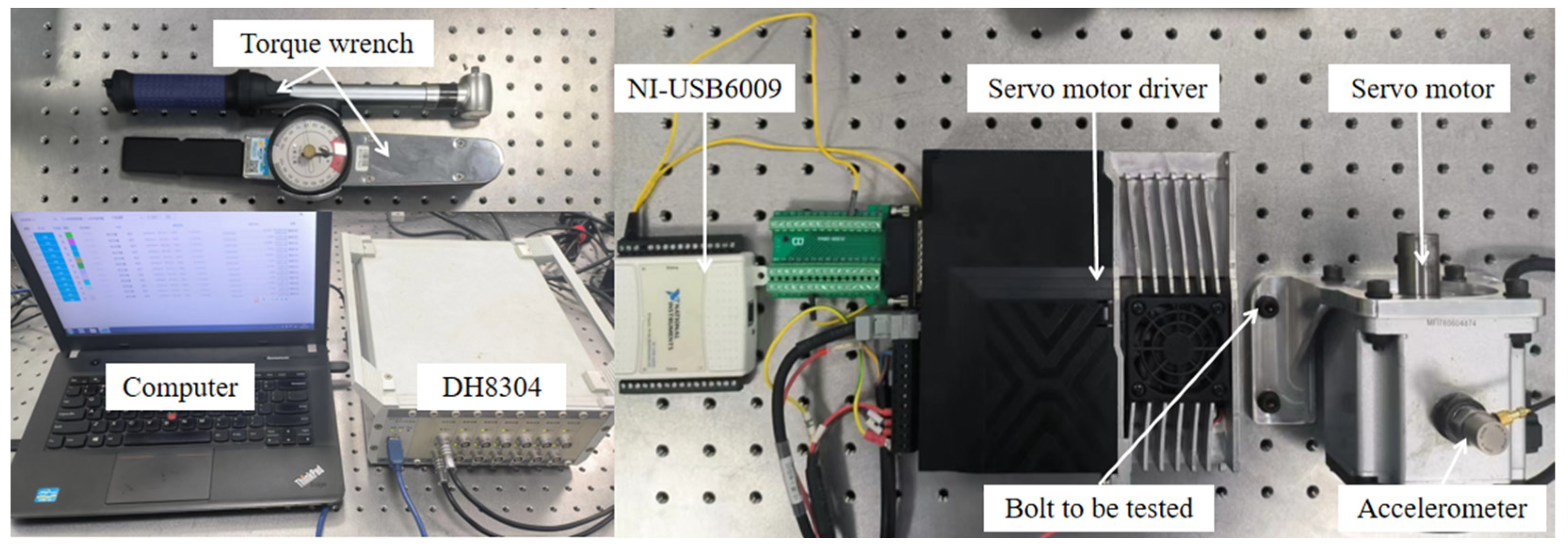
3.1. Description of the Experimental Setup
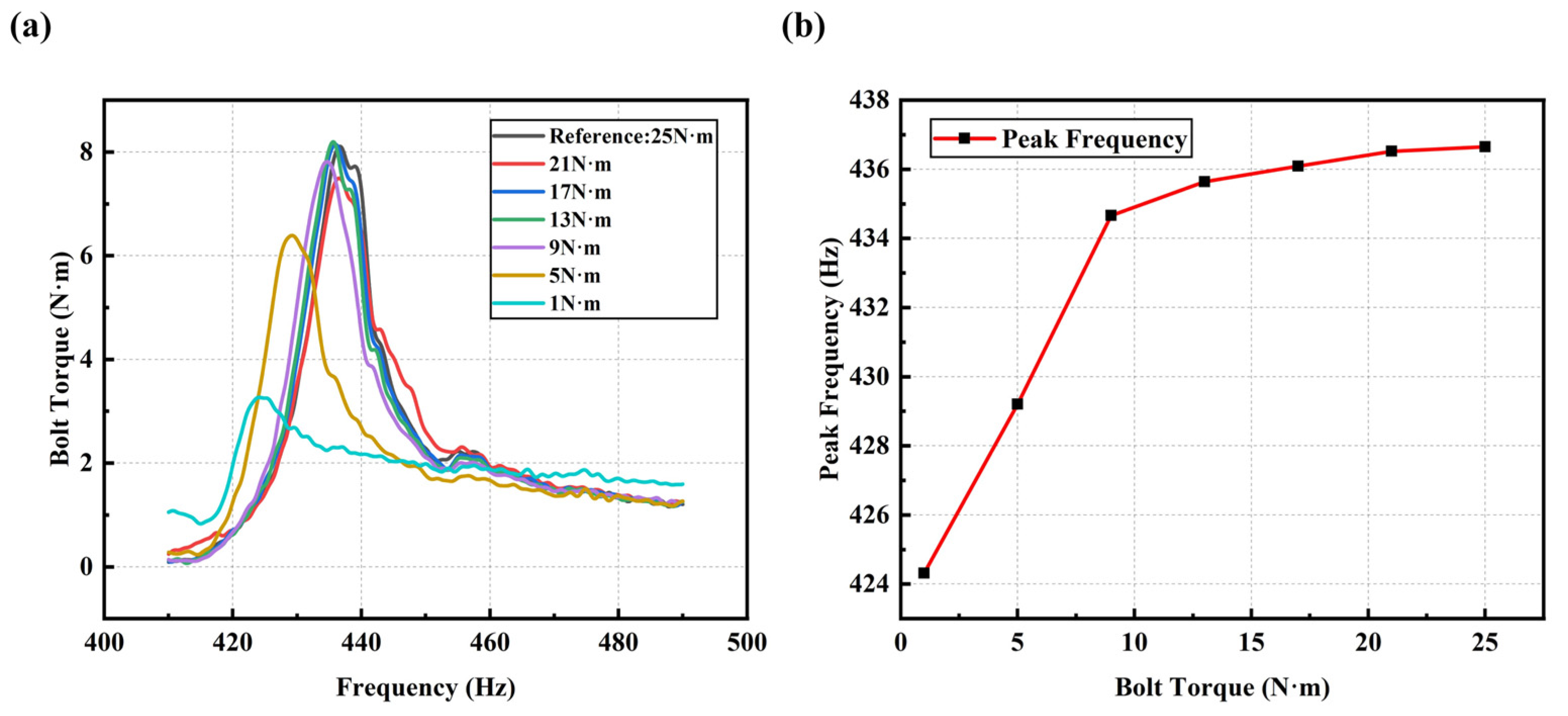
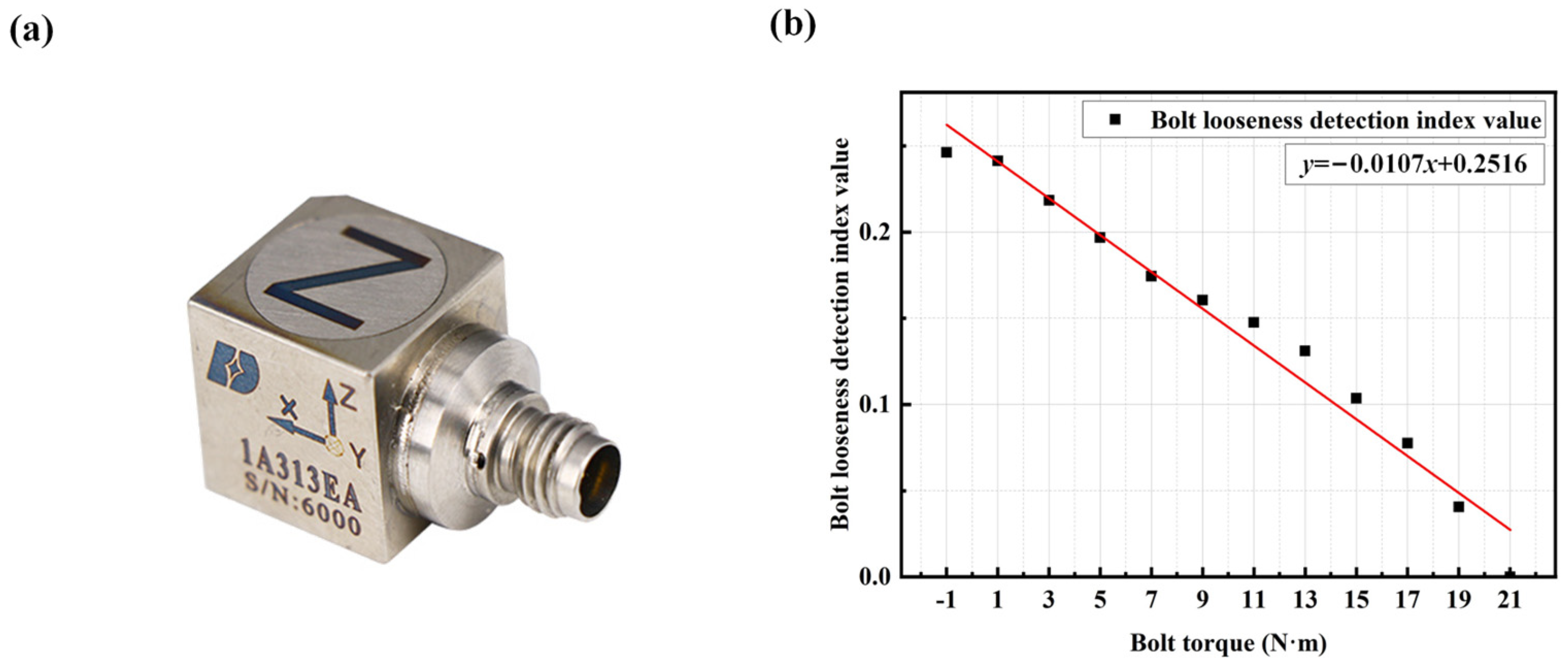
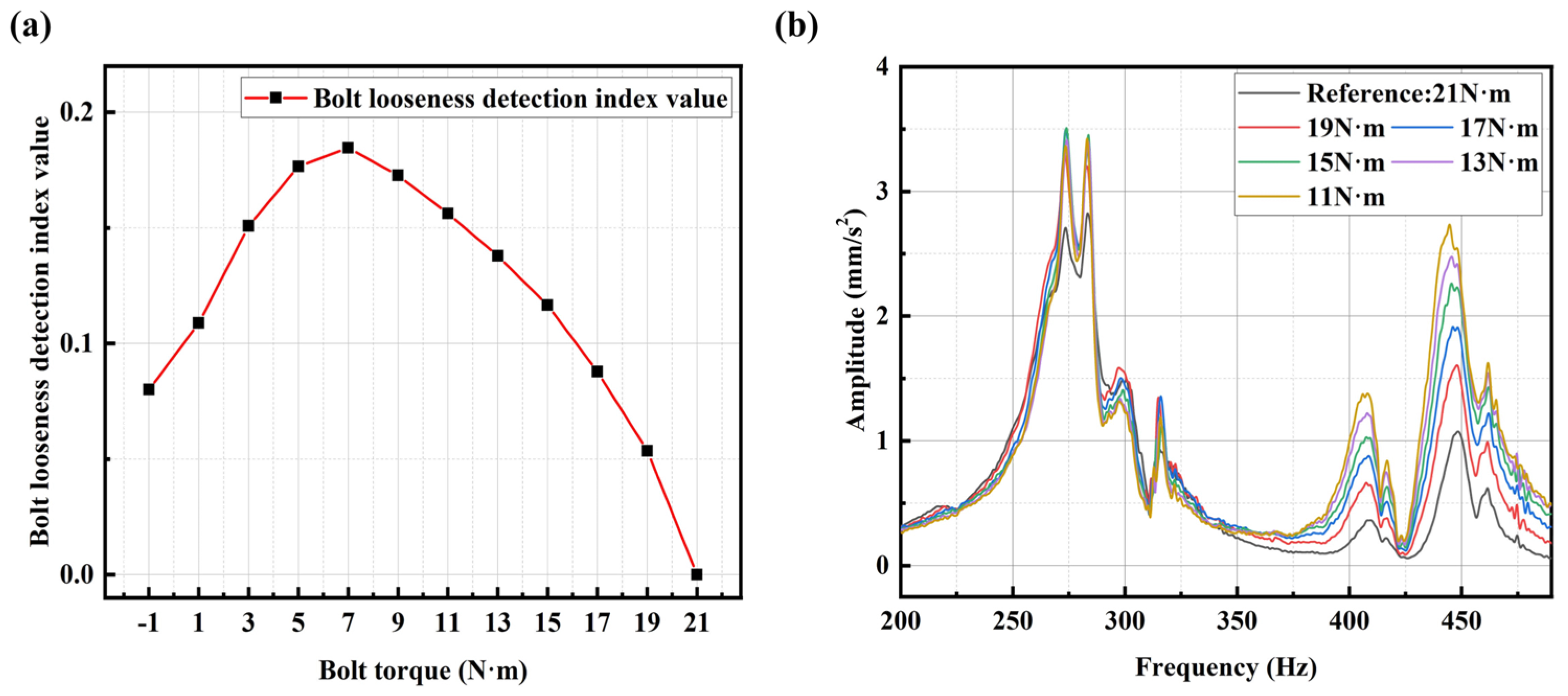
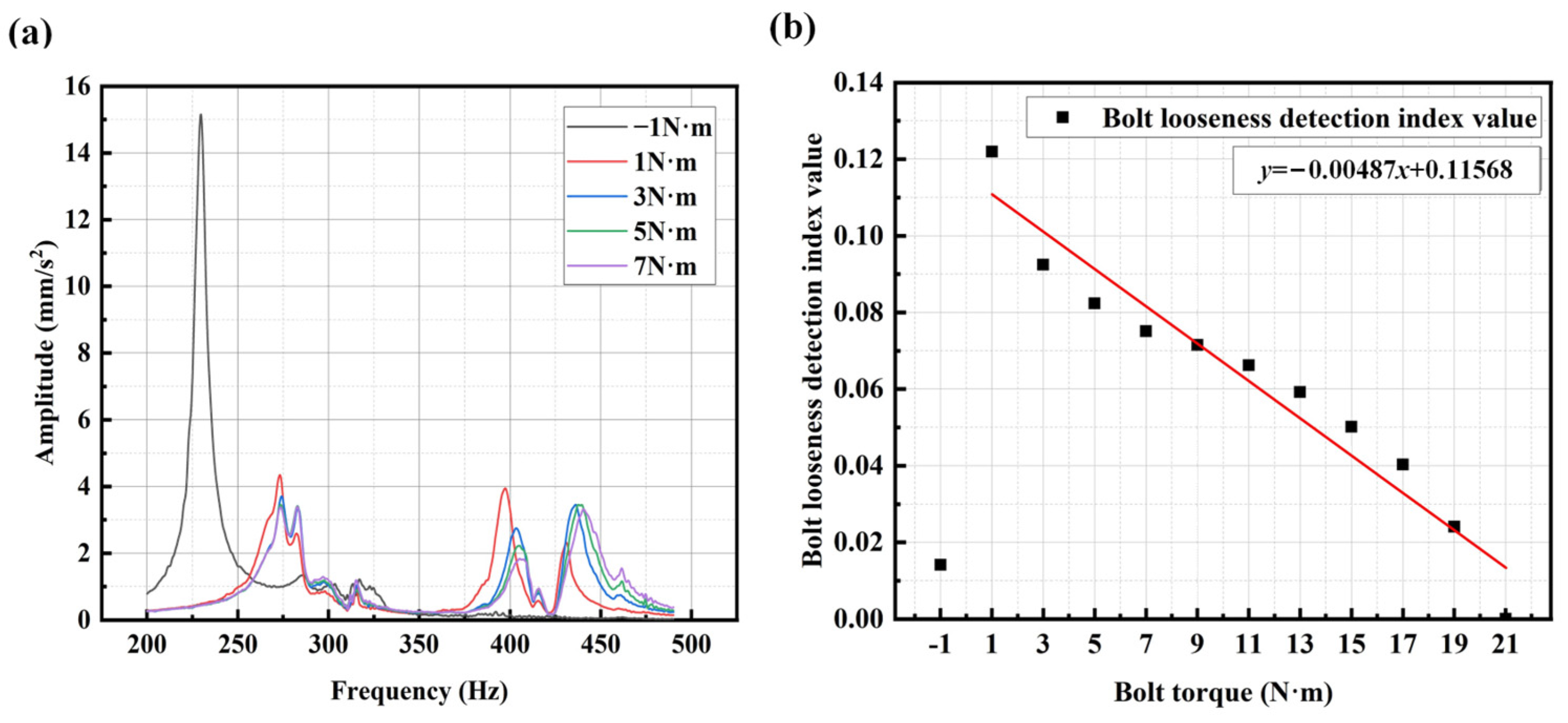
3.2. Experimental Analysis for Detecting Different Levels of Bolt Loosening
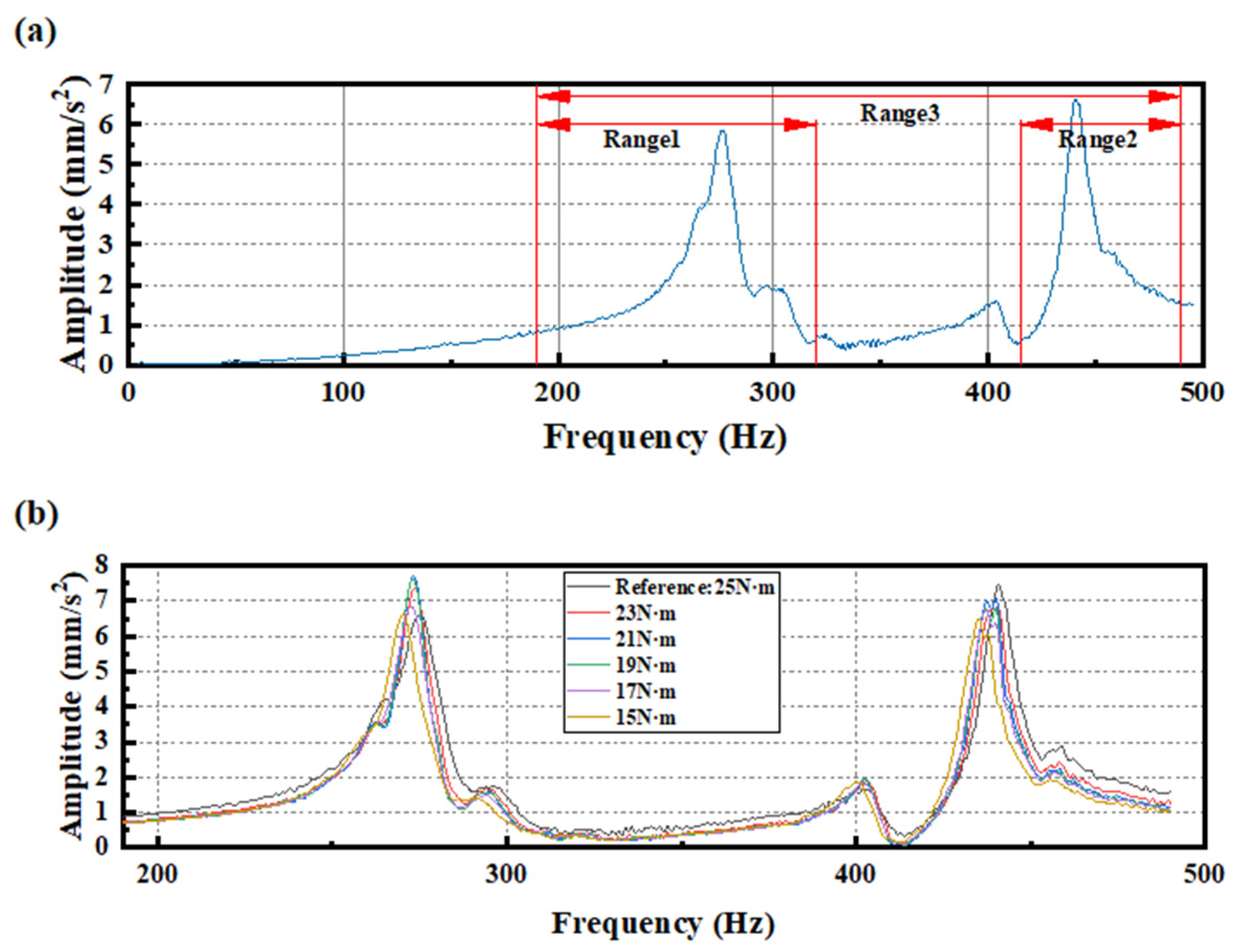
3.3. Analysis of Detection Performance at Different Frequency Ranges
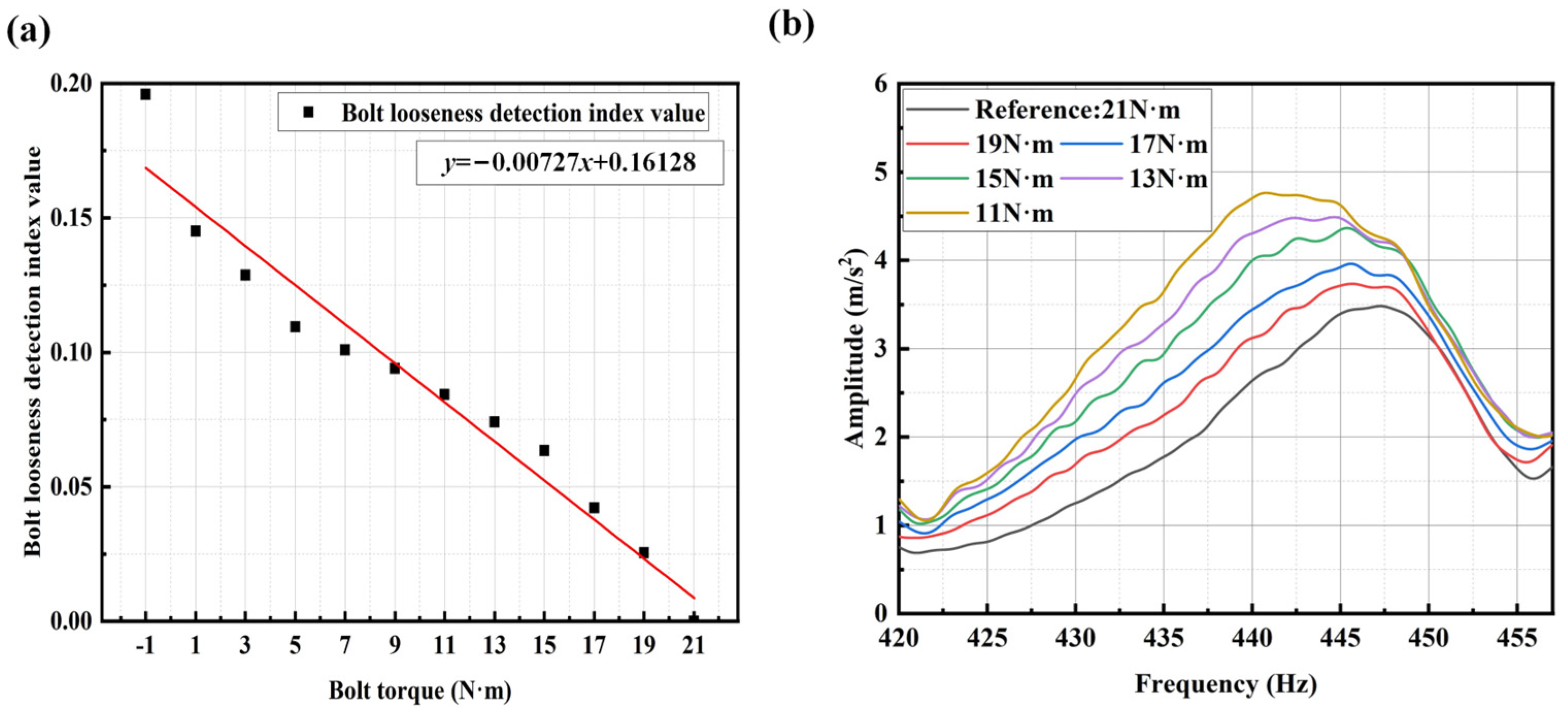
3.4. Analysis of Detection Performance on X-Axis and Y-Axis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nikravesh, S.M.Y.; Goudarzi, M. A Review Paper on Looseness Detection Methods in Bolted Structures. Lat. Am. J. Solids Struct. 2017, 14, 2153–2176. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, M.L.; Su, Z.Q.; Xiao, Y. Quantitative evaluation of residual torque of a loose bolt based on wave energy dissipation and vibro-acoustic modulation: A comparative study. J. Sound Vib. 2016, 383, 156–170. [Google Scholar] [CrossRef]
- Meyer, J.J.; Adams, D.E. Theoretical and experimental evidence for using impact modulation to assess bolted joints. Nonlinear Dyn. 2015, 81, 103–117. [Google Scholar] [CrossRef]
- Guannan, W.; Chao, X. Bolt looseness detection based on chaos ultrasonic excitation. J. Vib. Shock 2018, 37, 208–213. [Google Scholar]
- Zhang, M.R.; Tang, Z.F.; Yun, C.B.; Sui, X.D.; Chen, J.; Duan, Y.F. Bolt looseness detection using SH guided wave and wave energy transmission. Smart Mater. Struct. 2021, 30, 105015. [Google Scholar] [CrossRef]
- Wang, F.R.; Song, G.B. Bolt early looseness monitoring using modified vibro-acoustic modulation by time-reversal. Mech. Syst. Signal Process. 2019, 130, 349–360. [Google Scholar] [CrossRef]
- Qin, X.S.; Peng, C.; Zhao, G.Z.; Ju, Z.Y.; Lv, S.S.; Jiang, M.S.; Sui, Q.M.; Jia, L. Full life-cycle monitoring and earlier warning for bolt joint loosening using modified vibro-acoustic modulation. Mech. Syst. Signal Process. 2022, 162, 108054. [Google Scholar] [CrossRef]
- Gou, B.; Lu, Q.; Wang, B.; Wang, S. Bolt tightening force detection using outlier analysis of structural natural frequencies. J. Vib. Shock 2015, 34, 77–82. [Google Scholar]
- Razi, P.; Esmaeel, R.A.; Taheri, F. Improvement of a vibration-based damage detection approach for health monitoring of bolted flange joints in pipelines. Struct. Health Monit. 2013, 12, 207–224. [Google Scholar] [CrossRef]
- Demi, A.; Hui, L.; Hongping, Z.; Chao, W. EMI Based Experiment on Tunnel Segment Structural Bolt-looseness Damage Detection. J. Civ. Eng. Manag. 2016, 33, 39–42+56. [Google Scholar]
- Wang, T.; Yang, Z.; Shao, J.; Li, Y. Research on Bolt Loosen Detection Based on Piezoelectric Impedance Technology. Chin. J. Sens. Actuators 2014, 27, 1321–1325. [Google Scholar]
- Shao, J.H.; Wang, T.; Yin, H.Y.; Yang, D.; Li, Y.R. Bolt Looseness Detection Based on Piezoelectric Impedance Frequency Shift. Appl. Sci. 2016, 6, 298. [Google Scholar] [CrossRef]
- Wang, F.R.; Chen, Z.; Song, G.B. Monitoring of multi-bolt connection looseness using entropy-based active sensing and genetic algorithm-based least square support vector machine. Mech. Syst. Signal Process. 2020, 136, 106507. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, M.L.; Liao, Y.Z.; Su, Z.Q.; Xiao, Y. Contact acoustic nonlinearity (CAN)-based continuous monitoring of bolt loosening: Hybrid use of high-order harmonics and spectral sidebands. Mech. Syst. Signal Process. 2018, 103, 280–294. [Google Scholar] [CrossRef]
- Gong, T.; Yang, J.; Zhuang, X.; Liu, H. Review of experimental research on bolt looseness monitoring. Mach. Des. Manuf. 2024, 2, 354–363. [Google Scholar]
- Huang, J.Y.; Liu, J.H.; Gong, H.; Deng, X.J. A comprehensive review of loosening detection methods for threaded fasteners. Mech. Syst. Signal Process. 2022, 168, 108652. [Google Scholar] [CrossRef]
- Wu, J.; Jun, S.; Gen, Z.; Deqiang, Y.; Yuan, C. Detecting method for bolt looseness with average vibration response energy. Mech. Sci. Technol. Aerosp. Eng. 2022, 41, 992–997. [Google Scholar]
- Wang, J.; Sheng, J.; Jiang, J.; Wen, J.; Xiao, S.; Zhang, Z. Identification method of bolt looseness using STFT-IP time-frequency feature extraction technology. Noise Vib. Control 2021, 41, 108–112. [Google Scholar]
- Li, J.; Zhang, Y.; Fu, D.; Zhang, Y. Research on Detection Method and Test of Generator’s Bolt Loose Faults. Noise Vib. Control 2021, 41, 136–139. [Google Scholar]
- Cao, Z.; Tan, Z.; Jiang, D.; He, D.; Fei, Q. Loosening discrimination of a bolted connector under high-temperature vibration environment based on time-frequency analysis. J. Vib. Shock 2019, 38, 205–210. [Google Scholar]
- Xie, F.; Fu, Y.; Liu, K.; Xiao, Q.; Liu, H. Research on bolt looseness detection method based on multi-domain feature. J. Electron. Meas. Instrum. 2021, 35, 109–117. [Google Scholar]
- Huda, F.; Kajiwara, I.; Hosoya, N.; Kawamura, S. Bolt loosening analysis and diagnosis by non-contact laser excitation vibration tests. Mech. Syst. Signal Process. 2013, 40, 589–604. [Google Scholar] [CrossRef]
- Pal, D.K.; Basu, S.K. Surface preparation of machine tool slideways and its influence on the contact stiffness. Tribology 1973, 6, 172–177. [Google Scholar] [CrossRef]
- Xia, T.; Qu, J.; Liu, Y. A Joint Surface Contact Stiffness Model Considering Micro-Asperity Interaction. Aerospace 2024, 11, 472. [Google Scholar] [CrossRef]
- Sun, W.; Tan, L.F.; Su, N.X. Static Hysteresis Behavior Analysis and Stiffness and Damping Identification of Bolted Joints. J. Northeast. Univ. Nat. Sci. 2018, 39, 1743–1747. [Google Scholar]
- Elzahry, R.M. Optimum design of preloaded bolted joint under harmonic excitation. J. Sound Vib. 1986, 108, 455–470. [Google Scholar] [CrossRef]
- Zhang, Y. Response of vibration systems with uncertain transfer paths. Eng. Mech. 2008, 25, 133–136. [Google Scholar] [CrossRef]
- Li, Y.G.; Kong, X.N.; Gao, Y.Y. A Method for detecting bolt looseness base on probability density of vibration signals of two connected parts and principal component analysis. J. Vib. Shock 2015, 34, 63–67. [Google Scholar]
- Xiang, Z.H.; Huang, J.T. A controlled tap detection method for bolt tightness. J. Exp. Mech. 2012, 27, 545–551. [Google Scholar]
- Gelman, L.; Ottley, M. New processing techniques for transient signals with non-linear variation of the instantaneous frequency in time. Mech. Syst. Signal Process. 2006, 20, 1254–1262. [Google Scholar] [CrossRef]

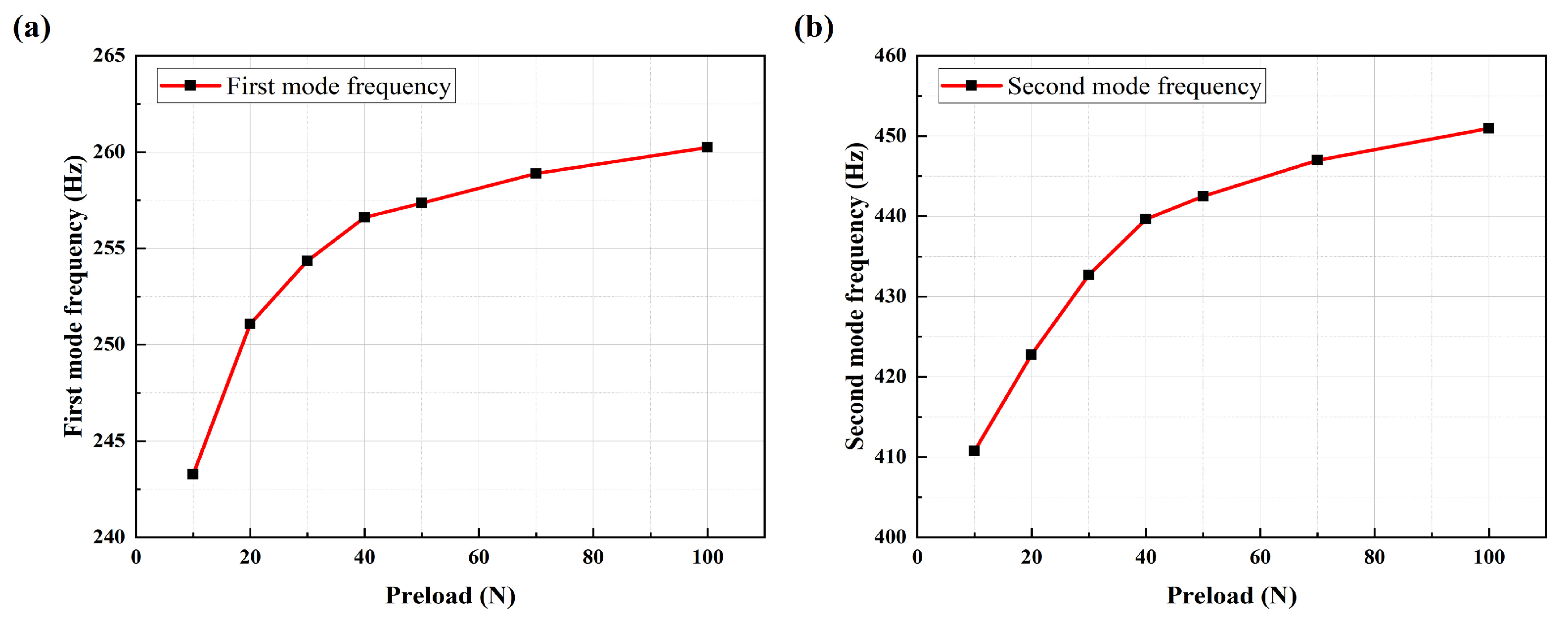


| Preload Force/N | First Mode Frequency/Hz | Second Mode Frequency/Hz | Third Mode Frequency/Hz | Fourth Mode Frequency/Hz |
|---|---|---|---|---|
| 10 | 243.27 | 410.77 | 792.06 | 1389.2 |
| 20 | 251.08 | 422.79 | 809.64 | 1415.5 |
| 30 | 254.35 | 432.69 | 825.20 | 1445.4 |
| 40 | 256.61 | 439.64 | 837.59 | 1472.1 |
| 50 | 257.35 | 442.49 | 843.41 | 1485.5 |
| 70 | 258.89 | 446.98 | 852.41 | 1506.3 |
| 100 | 260.24 | 450.95 | 860.67 | 1527.2 |
| 1000 | 264.35 | 458.05 | 877.17 | 1578.7 |
| 2000 | 264.87 | 458.21 | 877.71 | 1582.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, H.; Zhong, J.; Feng, B.; Chen, Y.; Zhong, S. Detection Method for Bolt Loosening Based on Summation Coefficient of Absolute Spectrum Ratio. Sensors 2025, 25, 246. https://doi.org/10.3390/s25010246
Guo H, Zhong J, Feng B, Chen Y, Zhong S. Detection Method for Bolt Loosening Based on Summation Coefficient of Absolute Spectrum Ratio. Sensors. 2025; 25(1):246. https://doi.org/10.3390/s25010246
Chicago/Turabian StyleGuo, Haoyang, Jianfeng Zhong, Bin Feng, Yulong Chen, and Shuncong Zhong. 2025. "Detection Method for Bolt Loosening Based on Summation Coefficient of Absolute Spectrum Ratio" Sensors 25, no. 1: 246. https://doi.org/10.3390/s25010246
APA StyleGuo, H., Zhong, J., Feng, B., Chen, Y., & Zhong, S. (2025). Detection Method for Bolt Loosening Based on Summation Coefficient of Absolute Spectrum Ratio. Sensors, 25(1), 246. https://doi.org/10.3390/s25010246







