Method and Verification of Liquid Cooling Heat Dissipation Based on Internal Heat Source of Airborne Long-Focus Aerial Camera
Abstract
1. Introduction
2. Models and Methods
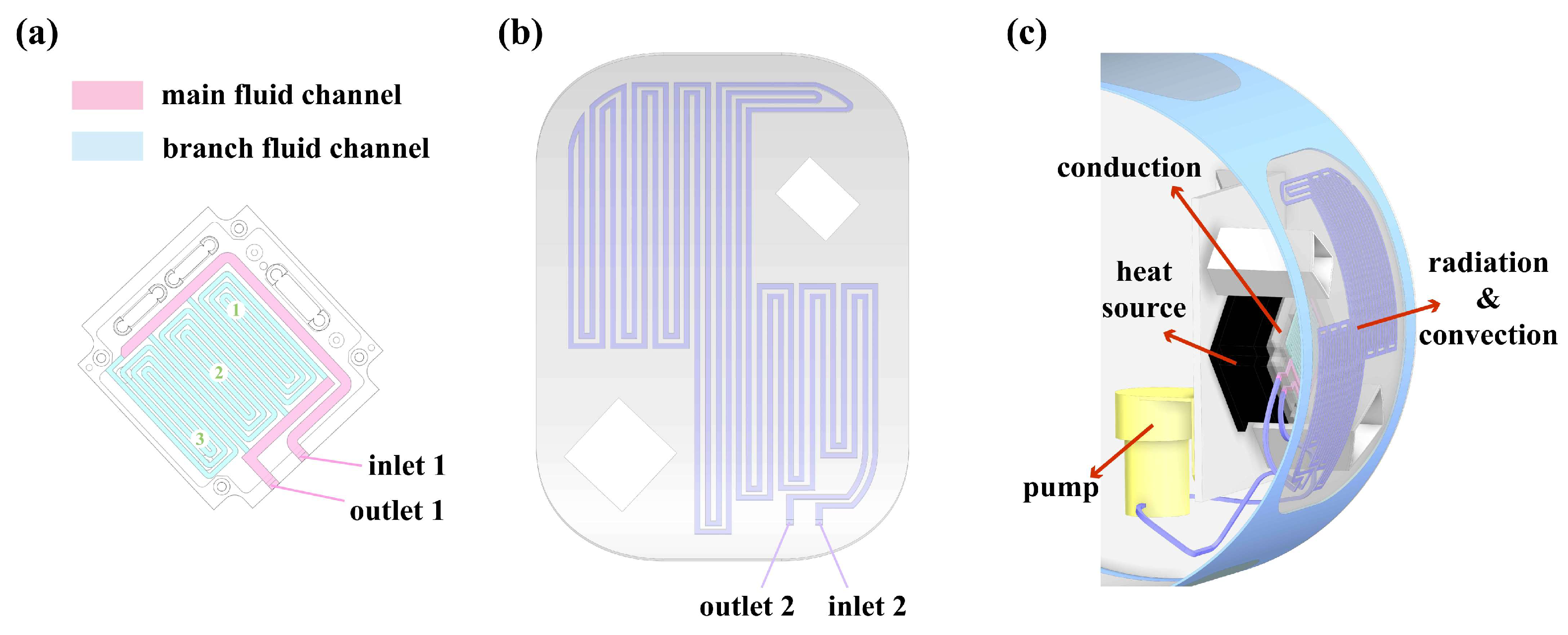
2.1. Liquid Cooling Structure Layout of the Aerial Camera
2.2. Theoretical Model
2.2.1. Heat Transfer Model
2.2.2. Turbulence Model
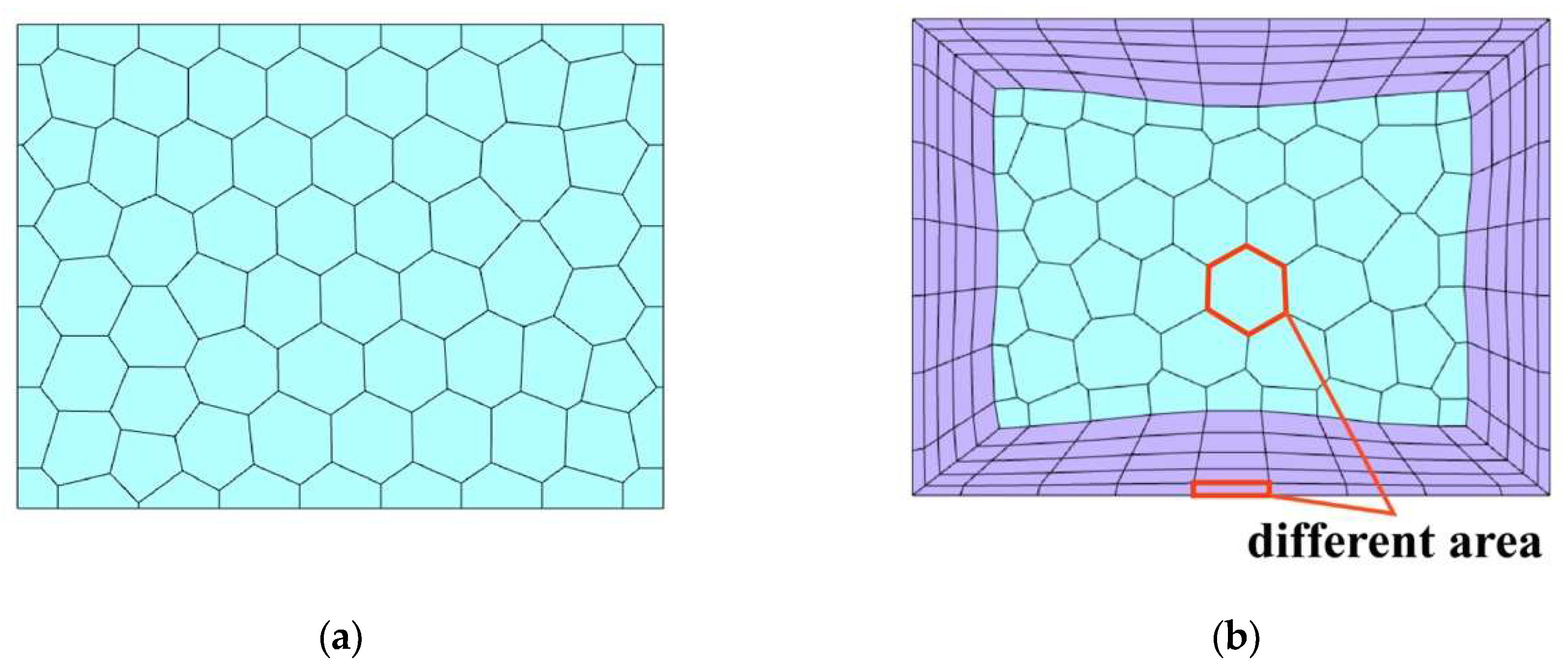
2.3. Dynamic Boundary Information Transfer Method Based on Grid Area Weighting
- Define the initial temperature = 273.15 (K) at the inlet boundary.
- Define the pointer to the inlet boundary face thread and the temperature variable index , which are passed by the solver to the program.
- Define the grid face variable , loop each face in the inlet face thread , index all grid faces in the loop, and complete the initial temperature boundary assignment.
- Call the execution macro after each iteration calculation, define the fluid domain variable and the face variable , and call the thread corresponding to the fluid domain outlet boundary face ID. The update period of the inlet boundary is defined according to the number of steps that can reach convergence. Define variables (representing the sum of the product of each grid face area and its corresponding stored temperature information), (representing the average temperature value weighted based on the grid faces area at the fluid outlet boundary), (representing the sum of grid faces area at the fluid outlet boundary), and define array to store the area data.
- When the fluid domain pointer is not explicitly passed to the program as a parameter, the fluid domain of the calculation domain is obtained by retrieving the domain pointer; the thread pointer points to the fluid domain outlet boundary face zone ID to avoid the fluid outlet boundary missing due to the randomness of each thread during parallel computation.
- When the number of iterations meets the convergence period, the face loop on the fluid outlet boundary face thread is executed. The actual area of a given face in the face thread can be calculated by Equation (8), where , , and are the three components of the area vector. Multiply and sum the area vector with the corresponding face temperature to obtain Equation (9), where is the number of face elements on the outlet boundary and is the area of the face . is the temperature information stored by the face , and Formula (10) represents the sum of the area on the outlet boundary face region.
- The average temperature value at the fluid outlet boundary weighted by grid area can be expressed as
3. Thermal Simulation and Thermal Test
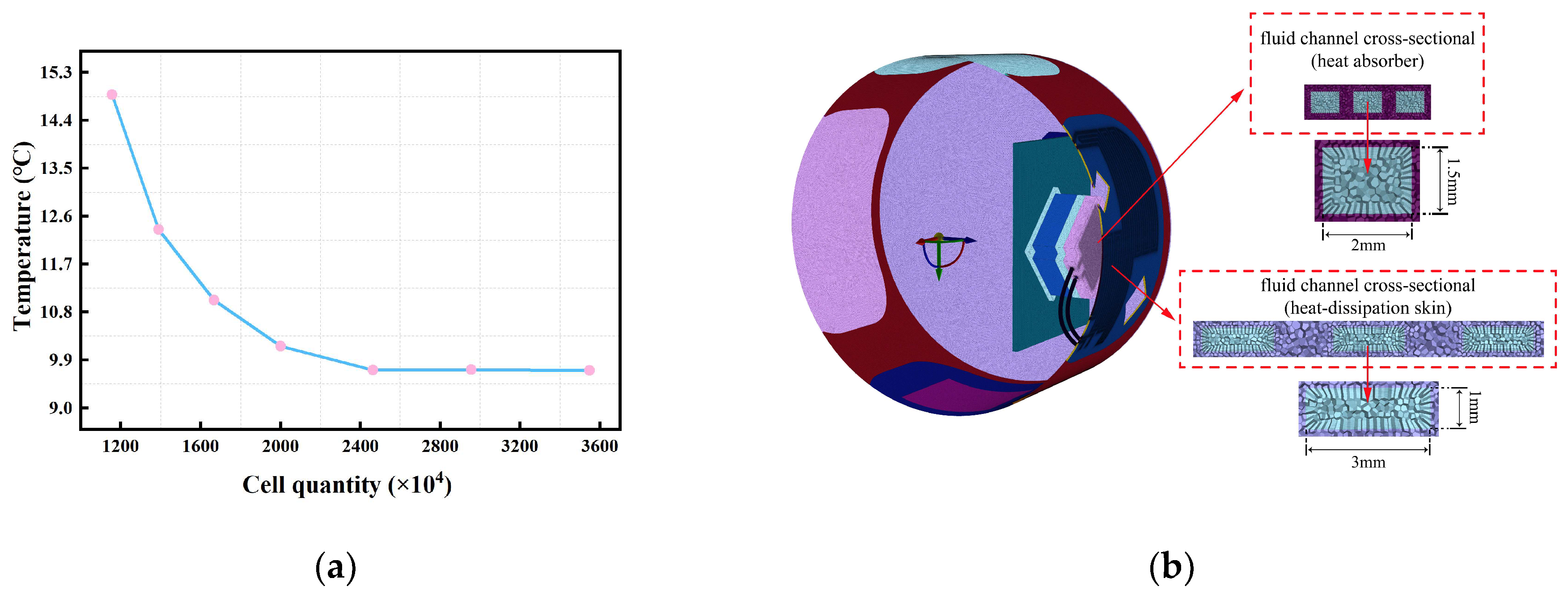
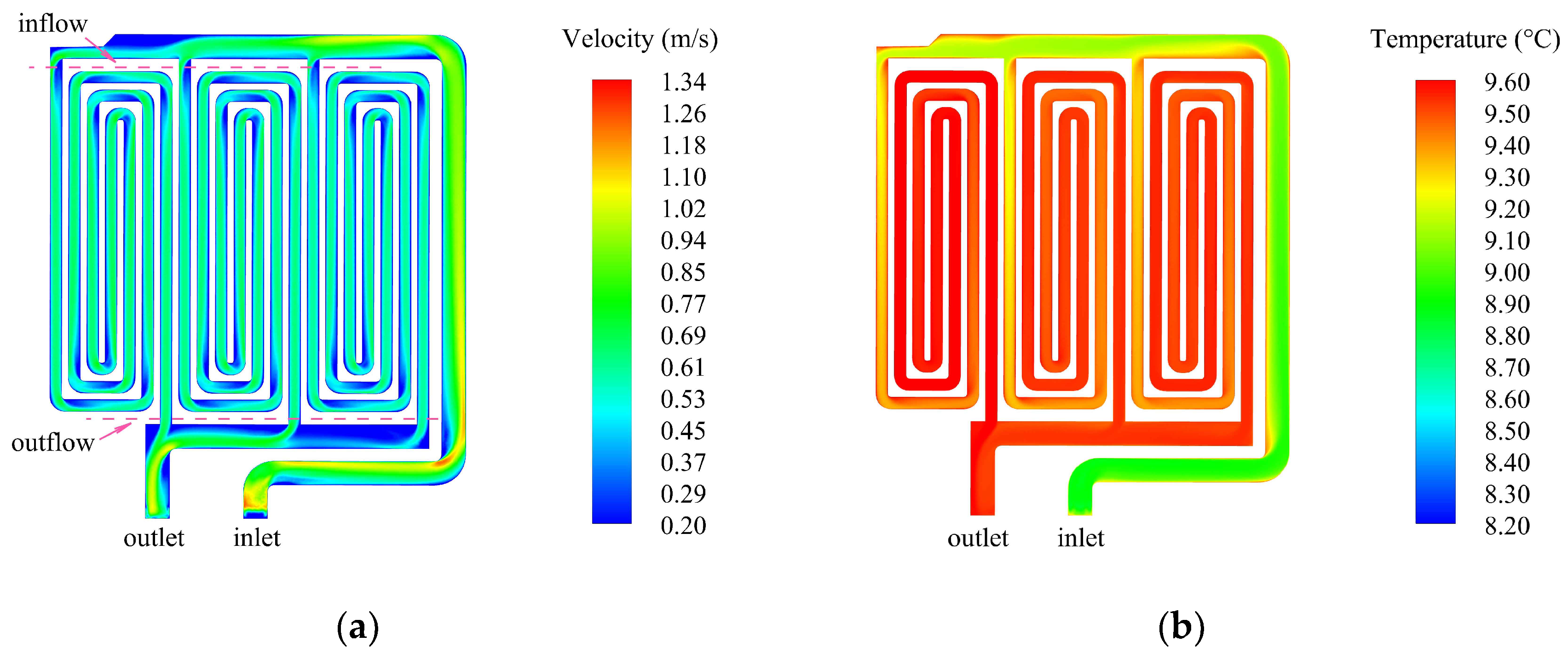
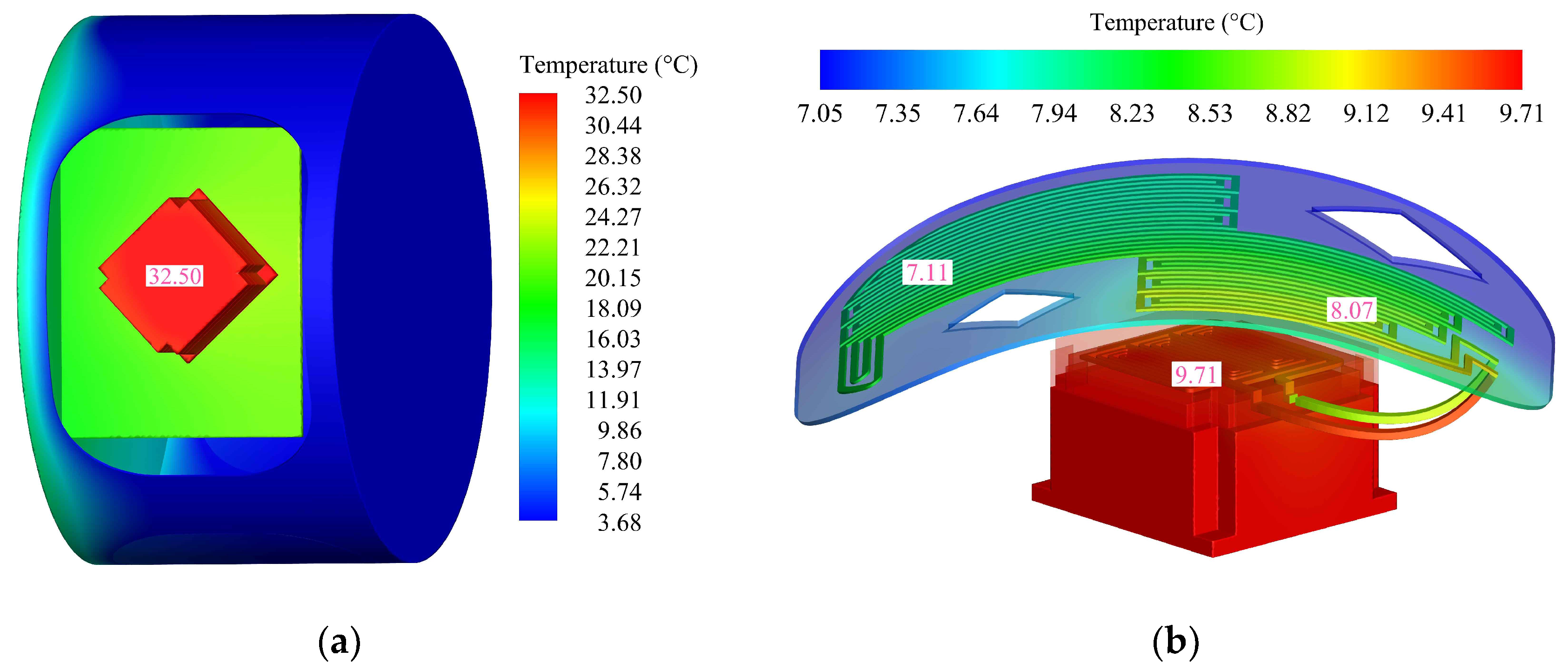
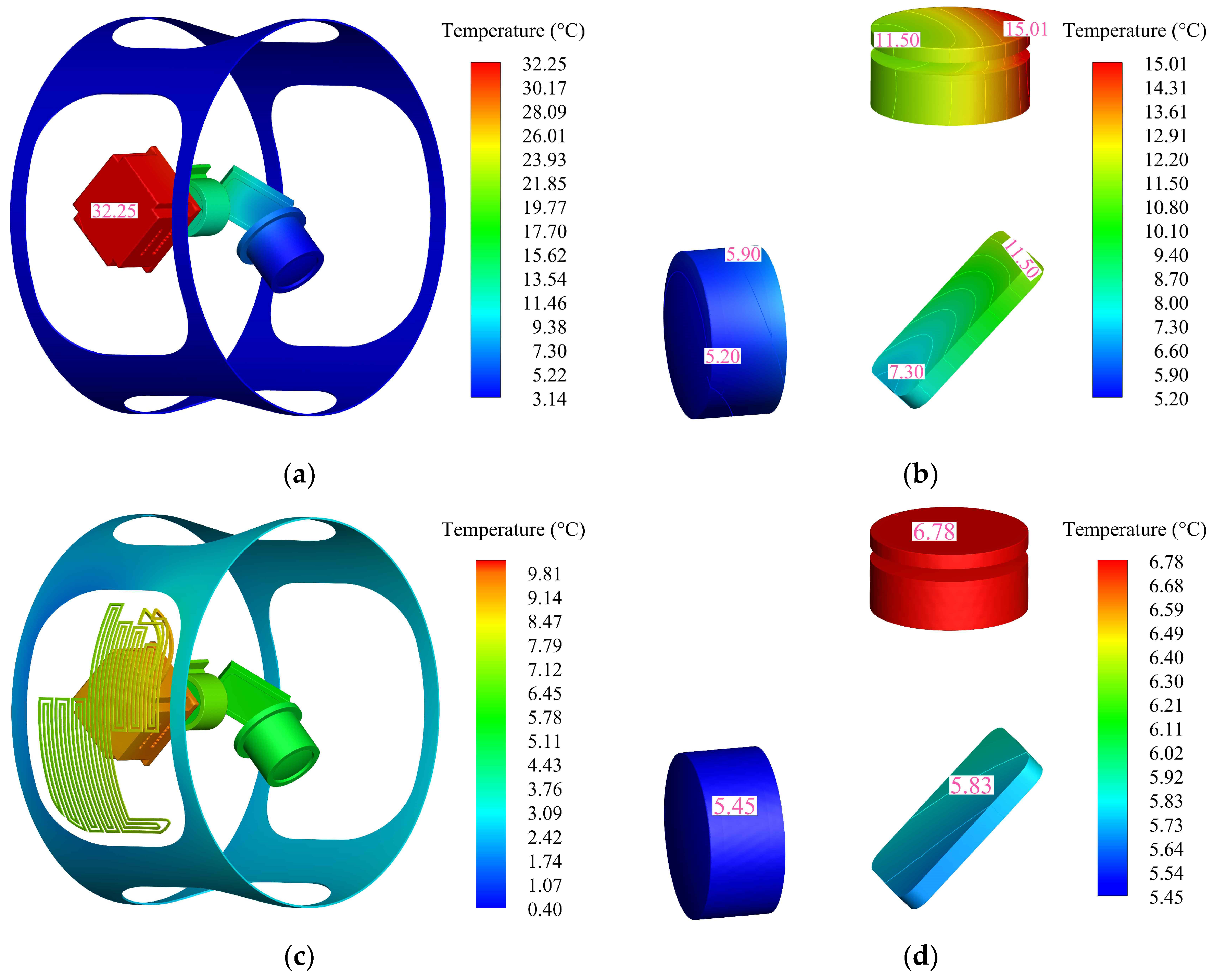
3.1. Thermal Simulation Analysis
3.2. Thermal Test
4. Optical System Simulation Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yuan, G.; Zheng, L.; Ding, Y.; Zhang, H.; Zhang, X.; Liu, X.; Sun, J. A Precise Calibration Method for Line Scan Cameras. IEEE Trans. Instrum. Meas. 2021, 70, 5013709. [Google Scholar] [CrossRef]
- Khan, A.; Imran, M.; Rashid, A. Aerial Camera Network for Observing Moving Targets. IEEE Sens. J. 2018, 18, 6847–6856. [Google Scholar] [CrossRef]
- Lin, J.; Wang, H.; Gu, Y.; Yi, A.; Wang, J.; Yang, Z. Design, Analysis and Preliminary Tests of a Linear Array CCD Aerial Camera for Ground Simulation. Optik 2020, 200, 163378. [Google Scholar] [CrossRef]
- Pitombeira, K.; Mitishita, E. Influence of On-Site Camera Calibration with Sub-Block of Images on the Accuracy of Spatial Data Obtained by PPK-Based UAS Photogrammetry. Remote Sens. 2023, 15, 3126. [Google Scholar] [CrossRef]
- Yang, Z.; Han, B.; Chen, W.; Gao, X. TMDiMP: Temporal Memory Guided Discriminative Tracker for UAV Object Tracking. Remote Sens. 2022, 14, 6351. [Google Scholar] [CrossRef]
- Colomina, I.; Molina, P. Unmanned Aerial Systems for Photogrammetry and Remote Sensing: A Review. ISPRS J. Photogramm. Remote Sens. 2014, 92, 79–97. [Google Scholar] [CrossRef]
- Nakada, R.; Takigawa, M.; Ohga, T.; Fujii, N. Verification of potency of aerial digital oblique cameras for aerial photogrammetry in japan. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2016, 41, 63–68. [Google Scholar] [CrossRef]
- Zhou, X.; Liu, H.; Li, Y.; Ma, M.; Lin, J. Analysis of the Influence of Vibrations on the Imaging Quality of an Integrated TDICCD Aerial Camera. Opt. Express 2021, 29, 18108. [Google Scholar] [CrossRef]
- Liu, X.; Yuan, D.; Song, L.; Yuan, G.; Zhang, H.; Ding, Y.; Zhang, C. Two-Mirror Aerial Mapping Camera Design with a Tilted-Aplanatic Secondary Mirror for Image Motion Compensation. Opt. Express 2023, 31, 4108. [Google Scholar] [CrossRef]
- Michels, G.J.; Genberg, V.L. Analysis of Thermally Loaded Transmissive Optical Elements. In Optical Modeling and Performance Predictions VI.; Kahan, M.A., Levine, M.B., Eds.; SPIE: San Diego, CA, USA, 2013; p. 88400C. [Google Scholar]
- Selımoglu, O.; Ekinci, M.; Karcı, O. Thermal Refocusing Method for Spaceborne High-Resolution Optical Imagers. Appl. Opt. 2016, 55, 4109. [Google Scholar] [CrossRef]
- Fan, Y.; Feng, W.; Ren, Z.; Liu, B.; Wang, D. Lumped Parameter Thermal Network Modeling and Thermal Optimization Design of an Aerial Camera. Sensors 2024, 24, 3982. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Zhou, Y.; Jiang, X.; Zuo, X.; Chen, M. Optimization of Thermal Control Design for Aerial Reflective Opto-Mechanical Structure. Sensors 2024, 24, 1194. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Z.; Sun, L.; Liu, F.; Liu, X.; Li, L.; Li, Q.; Hu, R. Engineering Design of an Active–Passive Combined Thermal Control Technology for an Aerial Optoelectronic Platform. Sensors 2019, 19, 5241. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Cheng, Z.; Jia, P.; Zhang, B.; Liu, X.; Hu, R. Impact of Thermal Control Measures on the Imaging Quality of an Aerial Optoelectronic Sensor. Sensors 2019, 19, 2753. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.-Y.; Ding, Y.-L.; Wu, Q.-W.; Jia, J.-Q.; Guo, L.; Wang, L.-H. Thermal Analysis and Design of the Aerial Camera’s Primary Optical System Components. Appl. Therm. Eng. 2012, 38, 40–47. [Google Scholar] [CrossRef]
- Yang, H.; Yuan, G.; Pan, J.; Zhou, D. Environmental Stability Design of the Aerial Mapping Camera Based on Multi-Dimensional Compound Structure. Sensors 2023, 23, 4421. [Google Scholar] [CrossRef]
- Liu, W.; Xu, Y.; Yao, Y.; Xu, Y.; Shen, H.; Ding, Y. Relationship Analysis between Transient Thermal Control Mode and Image Quality for an Aerial Camera. Appl. Opt. 2017, 56, 1028. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, B.; Chen, L.; Xu, B.; Gu, G. Thermal Design and Analysis of the High Resolution MWIR/LWIR Aerial Camera. Optik 2019, 179, 37–46. [Google Scholar] [CrossRef]
- Li, Y.; Yuan, G.; Xie, X.; Dong, L.; Yin, L. Multilayer Thermal Control for High-Altitude Vertical Imaging Aerial Cameras. Appl. Opt. 2022, 61, 5205–5214. [Google Scholar] [CrossRef]
- Deng, Y.; Liu, J. A Liquid Metal Cooling System for the Thermal Management of High Power LEDs. Int. Commun. Heat Mass Transf. 2010, 37, 788–791. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, C.; Pan, M. Design and Performance Research of Integrated Indirect Liquid Cooling System for Rack Server. Int. J. Therm. Sci. 2023, 184, 107951. [Google Scholar] [CrossRef]
- Xu, H.; Zhang, X.; Xiang, G.; Li, H. Optimization of Liquid Cooling and Heat Dissipation System of Lithium-Ion Battery Packs of Automobile. Case Stud. Therm. Eng. 2021, 26, 101012. [Google Scholar] [CrossRef]
- Ni, T.; Si, J.; Li, F.; Pan, C.; Li, D.; Pan, M.; Guan, W. Performance Analysis on the Liquid Cooling Plate with the New Tesla Valve Capillary Channel Based on the Fluid Solid Coupling Simulation. Appl. Therm. Eng. 2023, 232, 120977. [Google Scholar] [CrossRef]
- Chang, M.; Lai, B.; Wang, H.; Bai, J.; Mao, Z. Comprehensive Efficiency Analysis of Air-Cooled vs Water-Cooled Electric Motor for Unmanned Aerial Vehicle. Appl. Therm. Eng. 2023, 225, 120226. [Google Scholar] [CrossRef]
- Haarla, J.; Ala-Laurinaho, J.; Viikari, V. Scalable 3-D-Printable Antenna Array With Liquid Cooling for 28 GHz. IEEE Trans. Antennas Propagat. 2023, 71, 5067–5078. [Google Scholar] [CrossRef]
- Wang, D.; Li, Z.; Lin, J.; Lu, M.; Li, Y.; Ran, T. Thermal-Optical Characteristics Analysis of an Aerial Camera Optical System. Appl. Opt. 2022, 61, 8190. [Google Scholar] [CrossRef]
- Xue, Z.; Wang, C.; Yu, Y.; Wang, P.; Zhang, H.; Sui, Y.; Li, M.; Luo, Z. Integrated Optomechanical Analyses and Experimental Verification for a Thermal System of an Aerial Camera. Appl. Opt. 2019, 58, 6996–7005. [Google Scholar] [CrossRef]









| Materials | Fusing Point | Boiling Point | Density | Specific Heat | Viscosity | Thermal Conductivity |
|---|---|---|---|---|---|---|
| °C | °C | kg/m3 | J/(kg·K) | Pa·s | W/(m·K) | |
| Aluminum alloy | — | — | 2800 | 904 | — | 142 |
| ZTC4 | — | — | 4400 | 577 | — | 8.8 |
| N-OCTANE | −57 | 125 | 762.39 | 2013.146 | 0.002166 | 0.153 |
| Inlet Temperature | Outlet Temperature | Error |
|---|---|---|
| 8.82588 °C | 8.82721 °C | 0.015% |
| Flow Rate (g/s) | Branch Channel 1 | Branch Channel 2 | Branch Channel 3 |
|---|---|---|---|
| Inflow | 1.220 | 1.216 | 1.224 |
| Outflow | 1.220 | 1.216 | 1.224 |
| Operating Conditions | Simulation (°C) | Test (°C) | Error | ||||||
|---|---|---|---|---|---|---|---|---|---|
| natural heat dissipation | t0 | t0 | t0 | ||||||
| 32.50 | 31.45 | 3.34% | |||||||
| liquid cooling heat dissipation | t0 | t1 | t2 | t0 | t1 | t2 | t0 | t1 | t2 |
| 9.71 | 8.07 | 7.11 | 9.25 | 7.63 | 6.68 | 4.97% | 5.77% | 6.44% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuwen, Z.; Li, X.; Yuan, G.; Li, H.; Zhang, J.; Zhang, M.; Ding, Y. Method and Verification of Liquid Cooling Heat Dissipation Based on Internal Heat Source of Airborne Long-Focus Aerial Camera. Sensors 2024, 24, 6714. https://doi.org/10.3390/s24206714
Yuwen Z, Li X, Yuan G, Li H, Zhang J, Zhang M, Ding Y. Method and Verification of Liquid Cooling Heat Dissipation Based on Internal Heat Source of Airborne Long-Focus Aerial Camera. Sensors. 2024; 24(20):6714. https://doi.org/10.3390/s24206714
Chicago/Turabian StyleYuwen, Ziming, Xinyang Li, Guoqin Yuan, Haixing Li, Jichao Zhang, Mingqiang Zhang, and Yalin Ding. 2024. "Method and Verification of Liquid Cooling Heat Dissipation Based on Internal Heat Source of Airborne Long-Focus Aerial Camera" Sensors 24, no. 20: 6714. https://doi.org/10.3390/s24206714
APA StyleYuwen, Z., Li, X., Yuan, G., Li, H., Zhang, J., Zhang, M., & Ding, Y. (2024). Method and Verification of Liquid Cooling Heat Dissipation Based on Internal Heat Source of Airborne Long-Focus Aerial Camera. Sensors, 24(20), 6714. https://doi.org/10.3390/s24206714





