A SAW Wireless Passive Sensing System for Rotating Metal Parts
Abstract
1. Introduction
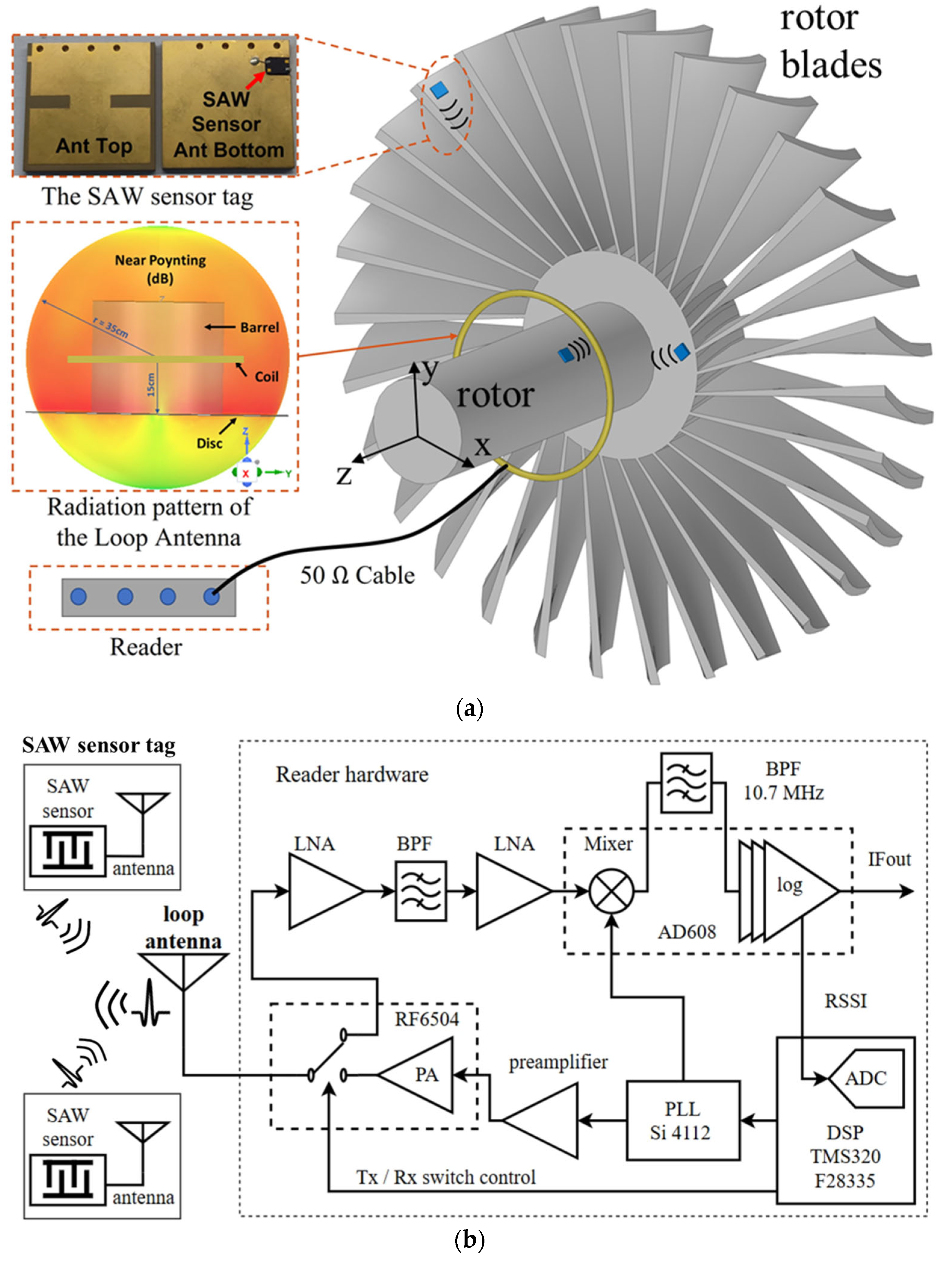
2. Theories and System Design Methods
2.1. Optimization of the Loop Antenna Performance
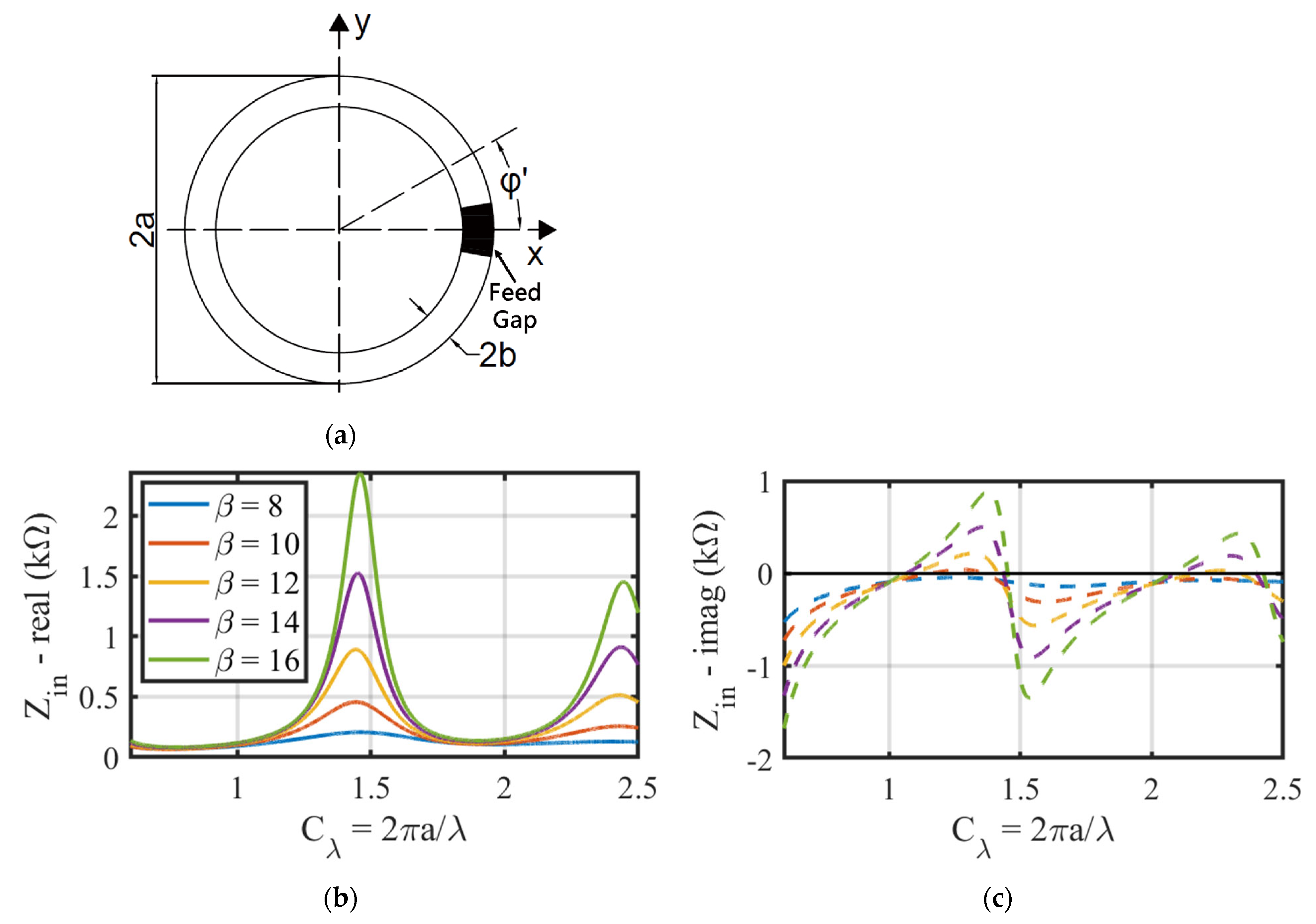
2.1.1. The Impedance of the Loop Antenna in Free Space
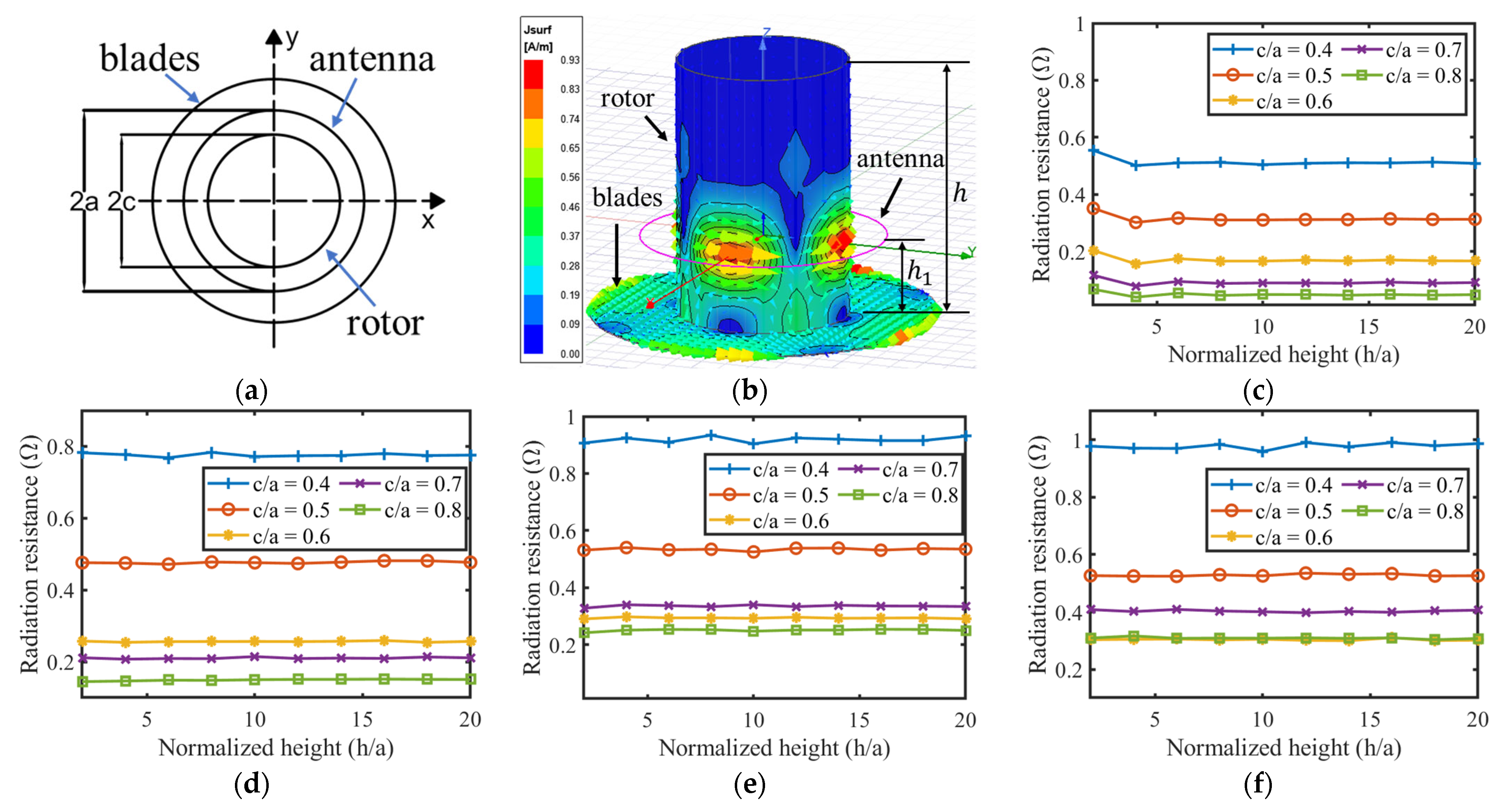
2.1.2. Loop Antenna Integrated with a Rotor
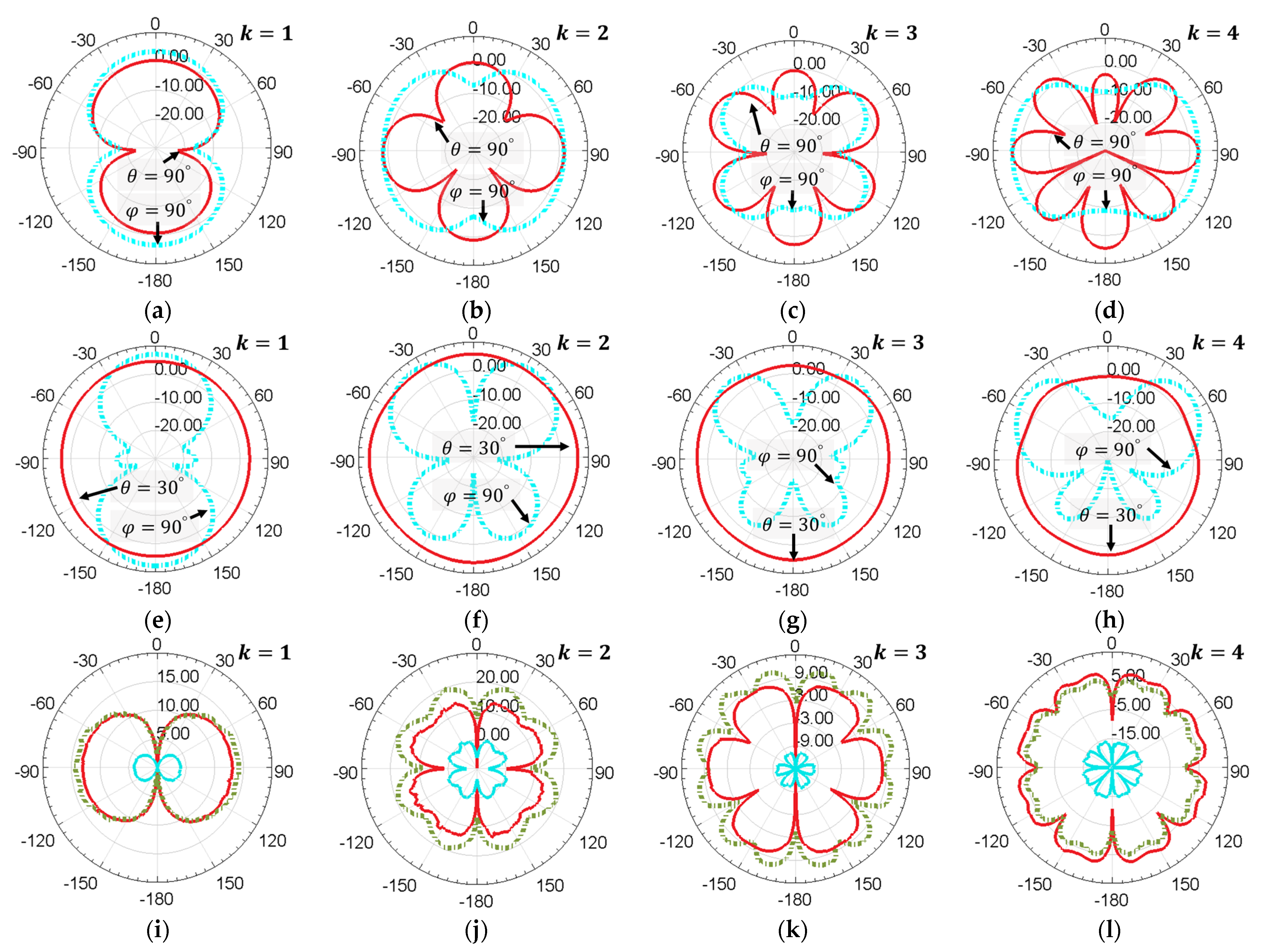
2.1.3. Efficiency and Radiation Patten of the Loop Antenna
2.2. Optimization for the SAW Senor Tag
2.2.1. Considerations for the SAW Antenna
2.2.2. Design of the SAW Temperature Sensor
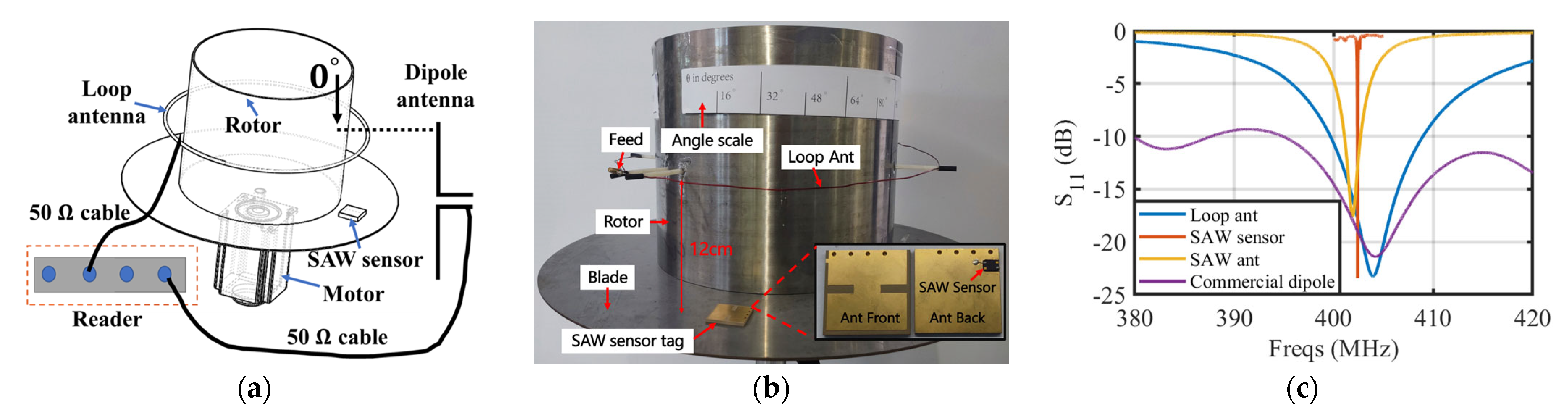
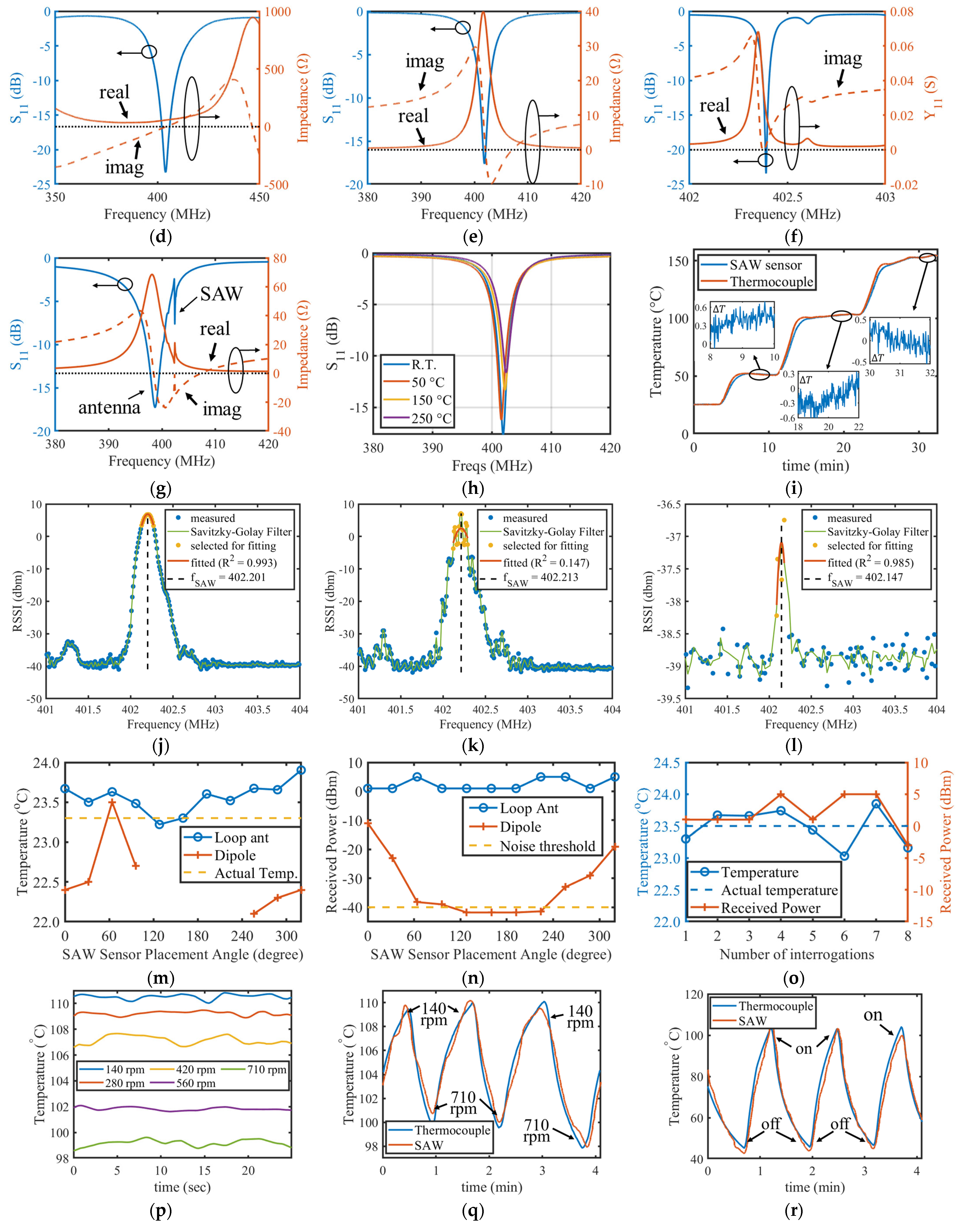
3. Experimental Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hong, Y.; Jia, P.; Guan, X.; Xiong, J.; Liu, W.; Zhang, H.; Li, C. Wireless Passive High-Temperature Sensor Readout System for Rotational-Speed Measurement. J. Sens. 2021, 2021, 6656527. [Google Scholar] [CrossRef]
- Ossété Gombé, B.; Goavec Mérou, G.; Breschi, K.; Guyennet, H.; Friedt, J.-M.; Felea, V.; Medjaher, K. A SAW wireless sensor network platform for industrial predictive maintenance. J. Intell. Manuf. 2019, 30, 1617–1628. [Google Scholar] [CrossRef]
- Merkulov, A.; Shvetsov, A.; Zhgoon, S. SAW-Based Wireless Measurements of the Fast-Varying Deformations in Rotating Vibrating Objects. In Proceedings of the IEEE International Ultrasonics Symposium (IUS), Glasgow, UK, 6–9 October 2019; pp. 2545–2548. [Google Scholar] [CrossRef]
- Binder, A.; Fachberger, R. Wireless SAW Temperature Sensor System for High-Speed High-Voltage Motors. IEEE Sens. J. 2011, 11, 966–970. [Google Scholar] [CrossRef]
- Zhou, X.; Tan, Q.; Liang, X.; Lin, B.; Guo, T.; Gan, y. Novel Multilayer SAW Temperature Sensor for Ultra-High Temperature Environments. Micromachines 2021, 12, 643. [Google Scholar] [CrossRef] [PubMed]
- Silva, D.; Mendes, J.; Pereira, A.; Gégot, F.; Alves, L. Measuring Torque and Temperature in a Rotating Shaft Using Commercial SAW Sensors. Sensors 2017, 17, 1547. [Google Scholar] [CrossRef] [PubMed]
- Kalinin, V.; Beckley, J.; Makeev, I. High-speed reader for wireless resonant SAW sensors. In Proceedings of the European Frequency and Time Forum, Gothenburg, Sweden, 23–27 April 2012; pp. 428–435. [Google Scholar] [CrossRef]
- Zhang, C.; Xie, Y. The Closed-Form Solution of Frequency Shift for an HF RFID Coil Antenna in Metallic Environments. IEEE Internet Things J. 2018, 5, 3927–3941. [Google Scholar] [CrossRef]
- Kocur, D.; Fortes, J.; Švecová, M. Multiple moving person tracking by UWB sensors: The effect of mutual shielding per-sons and methods reducing its impacts. EURASIP J. Wirel. Commun. Netw. 2017, 1, 68. [Google Scholar] [CrossRef]
- Guan, K.; Zhong, Z.; Alonso, J.; Briso-Rodríguez, C. Measurement of Distributed Antenna Systems at 2.4 GHz in a Realistic Subway Tunnel Environment. IEEE Trans. Veh. Technol. 2012, 61, 834–837. [Google Scholar] [CrossRef]
- Wang, Q.; Debbarma, D.; Lo, A.; Cao, Z.; Niemegeers, I.; Heemstra de Groot, S. Distributed Antenna System for Mitigating Shadowing Effect in 60 GHz WLAN. Wirel. Pers. Commun. 2015, 82, 811–832. [Google Scholar] [CrossRef]
- Merlo, J.M.; Mghabghab, S.R.; Nanzer, J.A. Wireless Picosecond Time Synchronization for Distributed Antenna Arrays. IEEE Trans. Microw. Theory Tech. 2023, 71, 1720–1731. [Google Scholar] [CrossRef]
- Chen, X.; He, X.; Zhang, Y.; Gao, B. Angle Measurement Method Based on Cross-Orthogonal Hall Sensor. In Proceedings of the IEEE 16th International Conference on Electronic Measurement & Instruments (ICEMI), Harbin, China, 9–11 August 2023. [Google Scholar] [CrossRef]
- Kumar, R.; Sontakke, B.A.; Anoop, C.S. A Hall Effect Based Through Shaft Angle Sensor—Analysis and Signal Conditioning. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Auckland, New Zealand, 20–23 May 2019; pp. 1–6. [Google Scholar] [CrossRef]
- Lee, Y.; Wu, R.; Xu, S. Applications of linear Hall-effect sensors on angular measurement. In Proceedings of the IEEE International Conference on Control Applications (CCA), Denver, CO, USA, 28–30 September 2011; pp. 479–482. [Google Scholar] [CrossRef]
- Alpert, H.S.; Chapin, C.A.; Dowling, K.M.; Benbrook, S.R.; Köck, H.; Ausserlechner, U.; Senesky, D.G. Sensitivity of 2DEG-based Hall-effect sensors at high temperatures. Rev. Sci. Instrum. 2020, 91, 2. [Google Scholar] [CrossRef] [PubMed]
- Leeladhar, M.; Panigrahi, R.K.; Kartikeyan, M.V. A low profile planar MIMO antenna with polarization diversity for LTE 1800. Microw. Opt. Technol. Lett. 2017, 59, 533–538. [Google Scholar] [CrossRef]
- Liu, Y.; Jiu, B.; Xia, X.-G.; Liu, H.; Zhang, L. Height Measurement of Low-Angle Target Using MIMO Radar Under Multipath Interference. IEEE Trans. Aerosp. Electron. Syst. 2017, 54, 808–818. [Google Scholar] [CrossRef]
- Boccard, J.M.; Reindl, L.M. Ceramic and magnetic loop antennas for wireless interrogation of SAW resonators on rotating machinery. In Proceedings of the IEEE International Workshop on Antenna Technology (iWAT), Tucson, AZ, USA, 5–7 March 2012; pp. 112–115. [Google Scholar] [CrossRef]
- Kalinin, V.; Lohr, R.; Leigh, A.; Bown, G. Application of Passive SAW Resonant Sensors to Contactless Measurement of the Output Engine Torque in Passenger Cars. In Proceedings of the IEEE International Frequency Control Symposium Joint with the 21st European Frequency and Time Forum, Geneva, Switzerland, 29 May 2007; pp. 499–504. [Google Scholar] [CrossRef]
- Kalinin, V. Wireless physical SAW sensors for automotive applications. In Proceedings of the IEEE International Ultrasonics Symposium, Orlando, FL, USA, 19 October 2011; pp. 212–221. [Google Scholar] [CrossRef]
- Kalinin, V.; Leigh, A.; Stopps, A.; Artigao, E. Resonant SAW torque sensor for wind turbines. In Proceedings of the Joint European Frequency and Time Forum & International Frequency Control Symposium (EFTF/IFC), Prague, Czech Republic, 21–25 July 2013; pp. 462–465. [Google Scholar] [CrossRef]
- Storer, J.E. Impedance of Thin-Wire Loop Antennas Fourier Series Solution for the Current Distribution. Trans. Am. Inst. Electr. Part I Commun. Electron. 1956, 75, 606–619. [Google Scholar] [CrossRef]
- Akhtyamov, E.R.; Gorbatov, V.I.; Ivliev, A.D.; Polev, V.F.; Kurichenko, A.A. High-Temperature Thermophysical Properties of Alloys of the Nickel–Vanadium System in Solid State. High Temp. 2023, 61, 36–44. [Google Scholar] [CrossRef]
- Lankford, A.; Hust, J. Update of thermal conductivity and electrical resistivity of electrolytic iron, tungsten, and stainless steel. Stand. Ref. Mater. 1984, 260, 53. [Google Scholar] [CrossRef]
- Tourette, S.; Collin, G.; Le Thuc, P.; Luxey, C.; Staraj, R. Small meandered PIFA associated with SAW passive sensor for monitoring inner temperature of a car exhaust header. In Proceedings of the IEEE International Workshop on Antenna Technology, Santa Monica, CA, USA, 2–4 March 2009; pp. 1–4. [Google Scholar] [CrossRef]
- Zakaria, M.R.; Farhi Shamsuddin, M.A.; Hashim, U.; Adam, T.; Al-Mufti, A.W. Design and Fabrication of IDT Surface Acoustic Wave Device for Biosensor Application. In Proceedings of the 5th International Conference on Intelligent Systems, Modelling and Simulation, Langkawi, Malaysia, 27–29 January 2014; pp. 760–764. [Google Scholar] [CrossRef]
- Ye, X.; Wang, Q.; Fang, L.; Wang, X.; Liang, B. Comparative study of SAW temperature sensor based on different piezoelectric materials and crystal cuts for passive wireless measurement. In Proceedings of the SENSORS IEEE, Waikoloa, HI, USA, 1–4 November 2010; pp. 585–588. [Google Scholar] [CrossRef]
- Slobodnik, A.J. Surface acoustic waves and SAW materials. Proc. IEEE 1976, 64, 581–595. [Google Scholar] [CrossRef]
- Pinheiro, T.; Morais, M.; Silvestre, S.; Carlos, E.; Coelho, J.; Almeida, H.V.; Barquinha, P.; Fortunato, E.; Martins, R. Direct Laser Writing: From Materials Synthesis and Conversion to Electronic Device Processing. Adv. Mater. 2024, 36, 2402014. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Zhou, Z.; Li, B.; Wang, C.; Liu, Q. Progresses on new generation laser direct writing technique. Mater. Today Nano 2021, 16, 100142. [Google Scholar] [CrossRef]



| k | Designed Frequency (MHz) | Simulated Frequency (MHz) | Simulated Radiation Resistance (Ω) | Frequency Error (%) |
|---|---|---|---|---|
| 1 | 400 | 426.35 | 156.60 | +6.59 |
| 2 | 420.35 | 205.46 | +5.09 | |
| 3 | 417.35 | 228.14 | +4.34 | |
| 4 | 416.15 | 251.35 | +4.04 |
| Parameter Symbols | Definitions | Values for Simulation |
|---|---|---|
| Radius of the turbine rotor | Normalized by antenna radius | |
| Height of the turbine rotor | ||
| Distance between the loop antenna and turbine blades | Fixed at 15 cm | |
| Fixed at 14 | ||
| Antenna wavelength | Fixed at 75 cm |
| Material Conductivity (MS/m) | k | Simulated Frequency (MHz) | Δf (MHz) | Simulated Radiation Resistance (Ω) | ΔR (Ω) |
|---|---|---|---|---|---|
| 1.0 (For stainless steel at high temperature) | 1 | 405.28 | −0.70 | 15.99 | +3.20 |
| 2 | 402.46 | −0.38 | 45.21 | +2.60 | |
| 3 | 401.12 | −0.24 | 83.47 | +2.79 | |
| 4 | 400.90 | −0.18 | 108.42 | +2.72 | |
| 2.8 (For nickel at high temperature) | 1 | 405.60 | −0.38 | 14.53 | +1.73 |
| 2 | 402.64 | −0.21 | 44.02 | +1.41 | |
| 3 | 401.23 | −0.13 | 82.15 | +1.47 | |
| 4 | 400.98 | −0.10 | 107.20 | +1.50 |
| Simulation Model | k | Max Gain (dB) | Max Gain Position |
|---|---|---|---|
| Loop antenna in free space in Figure 4a–d | 1 | 3.46 | |
| 2 | 3.00 | ||
| 3 | 4.59 | ||
| 4 | 5.75 | ||
| Loop antenna integrated with turbine rotor in Figure 4e–h | 1 | 7.76 | |
| 2 | 6.55 | ||
| 3 | 6.97 | ||
| 4 | 7.36 |
| Antenna types | Gain | Size | Bandwidth | Metal Environment |
|---|---|---|---|---|
| PIFA | Low | Small | Medium | Good |
| Loop coupler | Very low | Medium | Medium | Good |
| Helical | Medium | Medium | Wide | Poor |
| Dipole | High | Large | Wide | Poor |
| Parameters | Value | Explanation |
|---|---|---|
| Distance from the first via to the edge of the substrate | ||
| Gap between the vias | ||
| Distance from via array to the edge of the substrate | ||
| Feed width | ||
| Feed position | ||
| Slot width | ||
| Slot depth | ||
| Total length of the top layer graphic | ||
| Total width of the top layer graphic |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Ding, J.; Wang, B.; Gao, F.; Dong, S.; Jin, H. A SAW Wireless Passive Sensing System for Rotating Metal Parts. Sensors 2024, 24, 6703. https://doi.org/10.3390/s24206703
Zhou Y, Ding J, Wang B, Gao F, Dong S, Jin H. A SAW Wireless Passive Sensing System for Rotating Metal Parts. Sensors. 2024; 24(20):6703. https://doi.org/10.3390/s24206703
Chicago/Turabian StyleZhou, Yue, Jing Ding, Bingji Wang, Feng Gao, Shurong Dong, and Hao Jin. 2024. "A SAW Wireless Passive Sensing System for Rotating Metal Parts" Sensors 24, no. 20: 6703. https://doi.org/10.3390/s24206703
APA StyleZhou, Y., Ding, J., Wang, B., Gao, F., Dong, S., & Jin, H. (2024). A SAW Wireless Passive Sensing System for Rotating Metal Parts. Sensors, 24(20), 6703. https://doi.org/10.3390/s24206703









