Research on the NI-MLA Method for Enhancing the Spot Position Detection Accuracy of Quadrant Detectors Under Atmospheric Turbulence
Abstract
1. Introduction
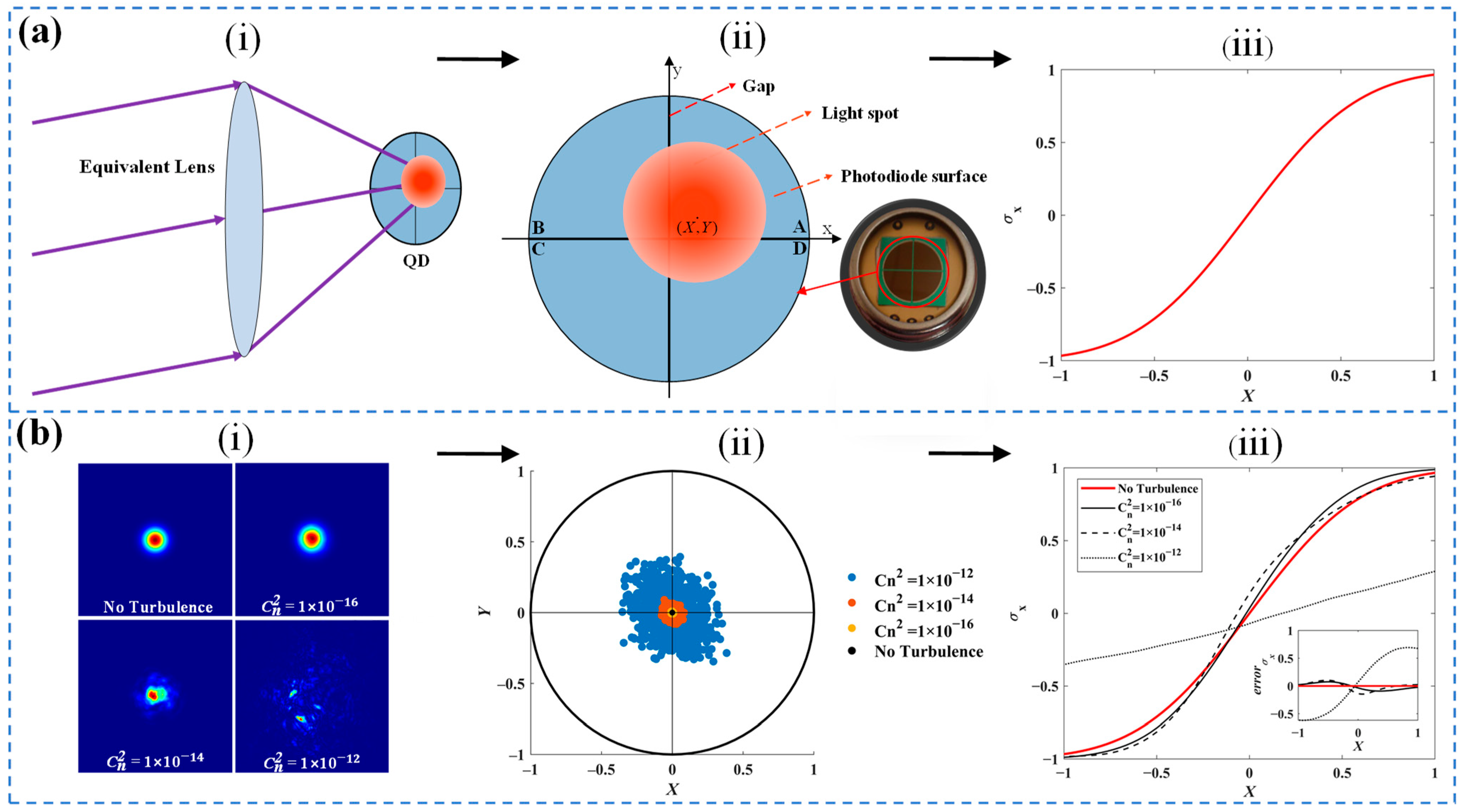
2. Analysis of the Atmospheric Turbulence Influence on QD
3. Verification of the NI-MLA System’s AMC and Analysis of the QD Computation Curve
3.1. Theoretical Analysis of the AMC
3.2. Simulation Verification of the AMC and Analysis of the QD Computation Curve
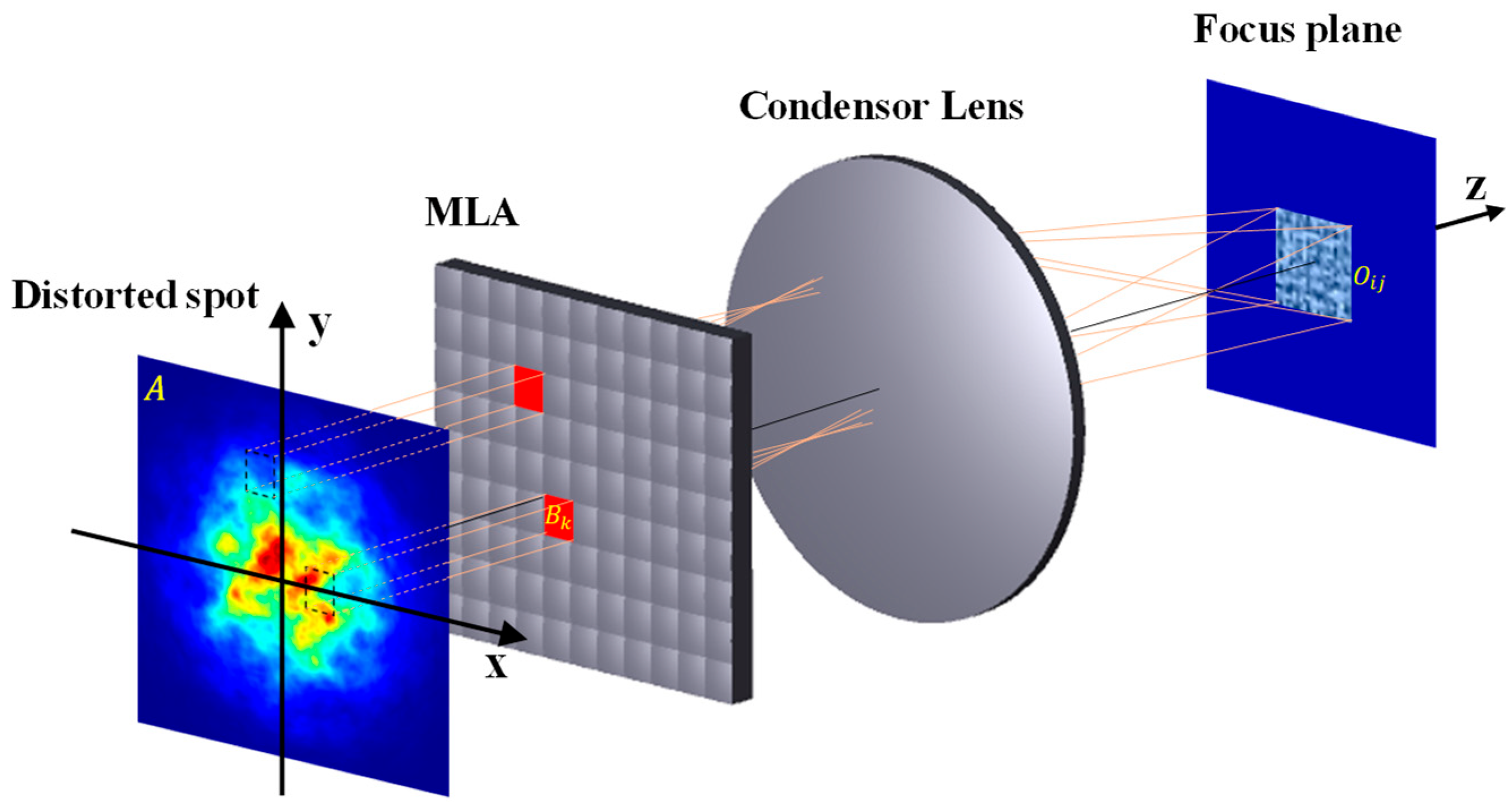
4. Verification of the NI-MLA System Mitigating SPDRE-QD Under Turbulence
4.1. Theoretical Analysis
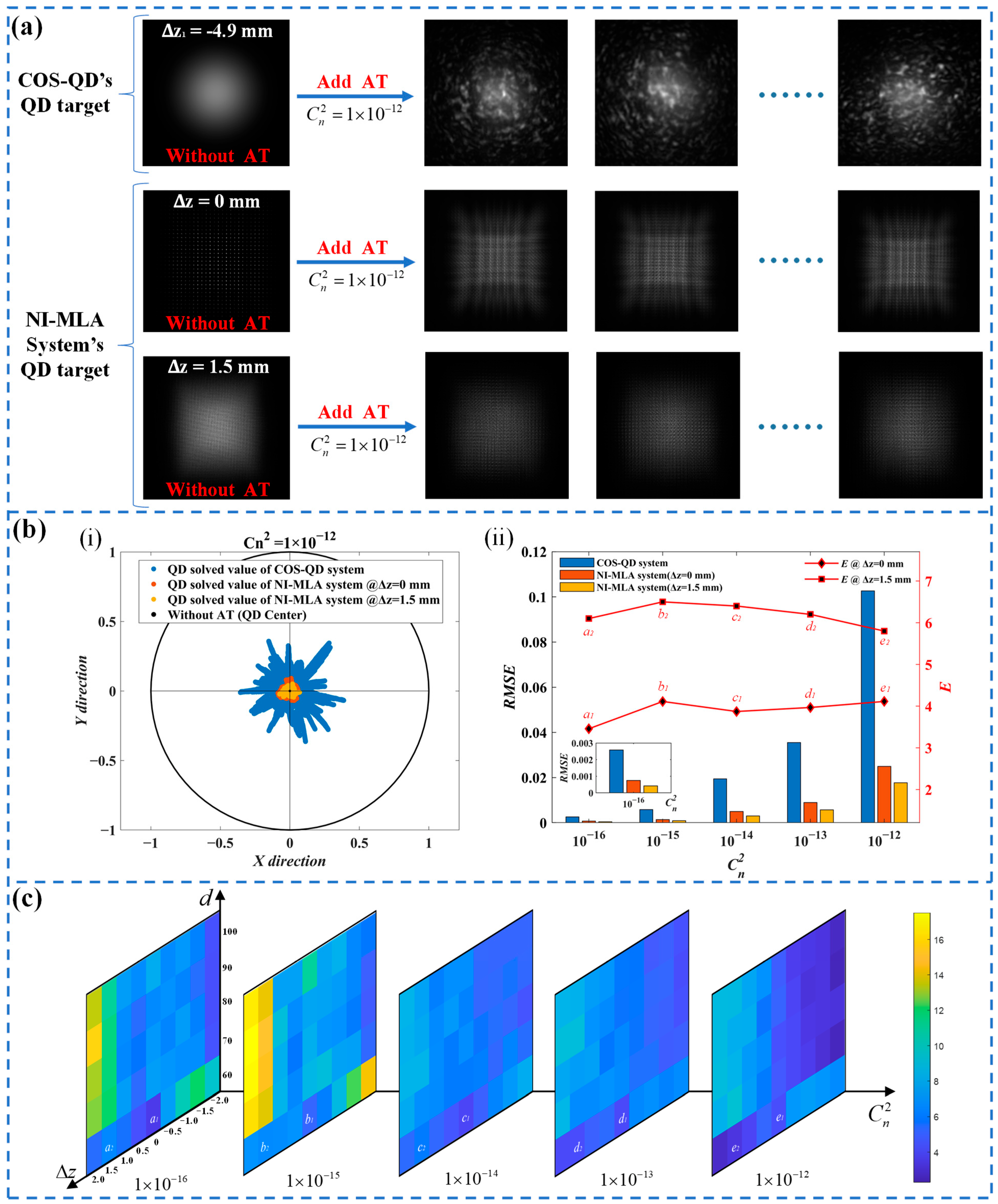
4.2. Simulation Verification
5. Experimental Results
5.1. Experimental Setup
5.2. Experimental Verification of the AMC of the NI-MLA System
5.3. QD Computation Curve Characteristics
5.4. Turbulence Mitigation Effect of NI-MLA System
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Safi, H.; Dargahi, A.; Cheng, J. Beam Tracking for UAV-Assisted FSO Links with a Four-Quadrant Detector. IEEE Commun. Lett. 2021, 25, 3908–3912. [Google Scholar] [CrossRef]
- Ocampos-Guillen, A.; Gomez-Garcia, J.; Denisenko, N. Double-loop wavefront tilt correction for free-space quantum key distribution. IEEE Access 2019, 7, 114033–114041. [Google Scholar] [CrossRef]
- Wang, S.; Li, L.; Chen, W. Improving seeking precision by utilizing ghost imaging in a semi-active quadrant detection seeker. Chin. J. Aeronaut. 2021, 34, 171–176. [Google Scholar] [CrossRef]
- Vo, Q.; Zhang, X.; Fang, F. Extended the linear measurement range of four-quadrant detector by using modified polynomial fitting algorithm in micro-displacement measuring system. Opt. Laser Technol. 2019, 112, 332–338. [Google Scholar] [CrossRef]
- Li, Y.; Geng, T.; Gao, S. On the error performance and channel capacity of a uniquely decodable coded FSO system over Malaga turbulence with pointing errors. Opt. Express 2023, 31, 34264–34279. [Google Scholar] [CrossRef]
- Ding, G.; Du, X.; Du, H. Performance analysis of OSSK-UWOC systems considering pointing errors and channel estimation errors. Opt. Express 2024, 32, 3606–3618. [Google Scholar] [CrossRef]
- Tang, Q.; Zhang, Y.; Chen, D. Research on the spectral phase correction method for the atmospheric detection in open space. Opt. Express 2018, 26, 193286–193299. [Google Scholar] [CrossRef]
- Mattsson, C.; Bertilsson, K.; Thung, S. Manufacturing and characterization of a modified four-quadrant position sensitive detector for out-door applications. Nucl. Instrum. Methods Phys. Res. 2004, 531, 134–139. [Google Scholar] [CrossRef]
- Hao, H.; Zhao, Y.; Huang, Y. A compact multi-pixel superconducting nanowire single-photon detector array supporting gigabit space-to-ground communications. Light Sci. Appl. 2024, 13, 25–37. [Google Scholar] [CrossRef]
- Bertilsson, K.; Dubaric, E.; Thung, S. Simulation of a low atmospheric-noise modified four-quadrant position sensitive detector. Nucl. Instrum. Methods Phys. Res. 2001, 466, 183–187. [Google Scholar] [CrossRef]
- Cui, S.; Soh, Y. Analysis and improvement of Laguerre–Gaussian beam position estimation using quadrant detectors. Opt. Lett. 2011, 36, 1692–1694. [Google Scholar] [CrossRef]
- Hermosa, N.; Aiello, A.; Woerdman, J. Quadrant detector calibration for vortex beams. Opt. Lett. 2011, 36, 409–411. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, S.; Xian, H. Analytical calibration of slope response of Zernike modes in a Shack-Hartmann wavefront sensor based on matrix product. Opt. Lett. 2022, 47, 1466–1469. [Google Scholar] [CrossRef]
- Lee, X.; Moreno, I.; Sun, C. High-performance LED street lighting using microlens arrays. Opt. Express 2013, 21, 10612–10621. [Google Scholar] [CrossRef]
- Zheng, X.; Dai, S.; Zhao, S. Partially coherent laser beam shaping in a zoom homogenizer. Opt. Express 2023, 31, 18444–18453. [Google Scholar] [CrossRef]
- Hou, M.; Gong, S.; Li, X. Forecasting the atmospheric refractive index structure constant profile with an altitude-time correlations-inspired deep learning model. Opt. Express 2023, 31, 2426–2444. [Google Scholar] [CrossRef]
- Dickey, F.; Holswade, H. Laser Beam Shaping: Theory and Techniques. Vacuum 2001, 62, 390–391. [Google Scholar] [CrossRef]
- Bich, A.; Holmes, A.; Meunier, M. Multifunctional Micro-Optical Elements for Laser Beam Homogenizing and Beam Shaping. Proc. SPIE 2008, 6879, 123–134. [Google Scholar] [CrossRef]
- Lindlein, N. Simulation of micro-optical systems including microlens arrays. J. Opt. A Pure Appl. Opt. 2002, 4, S1. [Google Scholar] [CrossRef]
- Wippermann, F.; Zeitner, U.; Dannberg, P. Beam homogenizers based on chirped microlens arrays. Opt. Express 2007, 15, 6218–6231. [Google Scholar] [CrossRef]
- Do, P.; Suzumoto, R.; Carrasco-Casado, A. Modeling of piezoelectric actuator’s hysteresis and its effect on the control accuracy of a LEO-to-GEO laser-communication for a small satellite. Proc. SPIE 2021, 11852, 1414–1423. [Google Scholar] [CrossRef]
- Yongbin, Z.; Huimin, C.; Zongtan, Z. Angle Measurement of Objects outside the Linear Field of View of a Strapdown Semi-Active Laser Seeker. Sensors 2018, 18, 1673–1685. [Google Scholar] [CrossRef]








| Parameter Name | Symbol | Value |
|---|---|---|
| MLA sub-aperture size | p | 300 μm |
| MLA sub-aperture focal length | f1 | 5 mm |
| Number of MLA sub-aperture | a | 50 × 50 |
| The focal length of the condenser lens | ff | 25 mm |
| Distance between MLA and CL | d1 | 60 mm |
| QD target surface defocus | 0 and 1.5 mm |
| Category | Parameter | ||
|---|---|---|---|
| NI-MLA system’s AMC | R2 | 0.9955 | 0.9997 |
| //mm | 24.95/25.00 mm | 22.70/22.90 mm | |
| NI-MLA system’s QD computation curve characteristics | 0.0261 | 0.0112 | |
| 1.4205 | 1.5733 | ||
| /mm | 0.0180 mm | 0.0041 mm |
| Category | Parameter Name | Symbol | Value |
|---|---|---|---|
| COS-QD system | focal length | 25 mm | |
| effective aperture | P | 10 mm | |
| defocus | −4.92 mm | ||
| Light source | wavelength | λ | 632.8 nm |
| waist radius | 1.2 mm | ||
| Atmospheric parameters | refractive index structure constant | ||
| atmospheric channel length | D | 1 km |
| /mm | /mm | /mm | |||||
|---|---|---|---|---|---|---|---|
| COS-QD | NI-MLA | COS-QD | NI-MLA | COS-QD | NI-MLA | ||
| −2.00 | −5.81 | 0.0155 | 0.0194 | 1.3727 | 1.4908 | 0.0043 | 0.0061 |
| −1.50 | −5.69 | 0.0162 | 0.0205 | 1.4035 | 1.5143 | 0.0046 | 0.0068 |
| −1.00 | −5.56 | 0.0170 | 0.0211 | 1.4356 | 1.5533 | 0.0048 | 0.0071 |
| −0.50 | −5.43 | 0.0177 | 0.0224 | 1.4692 | 1.6015 | 0.0050 | 0.0079 |
| 0.00 | −5.30 | 0.0186 | 0.0274 | 1.5045 | 1.6309 | 0.0052 | 0.0171 |
| 0.50 | −5.17 | 0.0195 | 0.0145 | 1.5415 | 1.6818 | 0.0055 | 0.0045 |
| 1.00 | −5.05 | 0.0204 | 0.0139 | 1.5804 | 1.7179 | 0.0057 | 0.0036 |
| 1.50 | −4.92 | 0.0215 | 0.0123 | 1.6212 | 1.7509 | 0.0060 | 0.0033 |
| 2.00 | −4.79 | 0.0226 | 0.0105 | 1.6643 | 1.8157 | 0.0063 | 0.0028 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Gao, S.; Wu, J.; Chen, Y.; Ma, L.; Yu, X.; Wang, X.; Li, R. Research on the NI-MLA Method for Enhancing the Spot Position Detection Accuracy of Quadrant Detectors Under Atmospheric Turbulence. Sensors 2024, 24, 6684. https://doi.org/10.3390/s24206684
Liu Z, Gao S, Wu J, Chen Y, Ma L, Yu X, Wang X, Li R. Research on the NI-MLA Method for Enhancing the Spot Position Detection Accuracy of Quadrant Detectors Under Atmospheric Turbulence. Sensors. 2024; 24(20):6684. https://doi.org/10.3390/s24206684
Chicago/Turabian StyleLiu, Zuoyu, Shijie Gao, Jiabin Wu, Yunshan Chen, Lie Ma, Xichang Yu, Ximing Wang, and Ruipeng Li. 2024. "Research on the NI-MLA Method for Enhancing the Spot Position Detection Accuracy of Quadrant Detectors Under Atmospheric Turbulence" Sensors 24, no. 20: 6684. https://doi.org/10.3390/s24206684
APA StyleLiu, Z., Gao, S., Wu, J., Chen, Y., Ma, L., Yu, X., Wang, X., & Li, R. (2024). Research on the NI-MLA Method for Enhancing the Spot Position Detection Accuracy of Quadrant Detectors Under Atmospheric Turbulence. Sensors, 24(20), 6684. https://doi.org/10.3390/s24206684





