Theoretical and Experimental Investigations on High-Precision Micro-Low-Gravity Simulation Technology for Lunar Mobile Vehicle
Abstract
1. Introduction
2. Theoretical Model and Methods
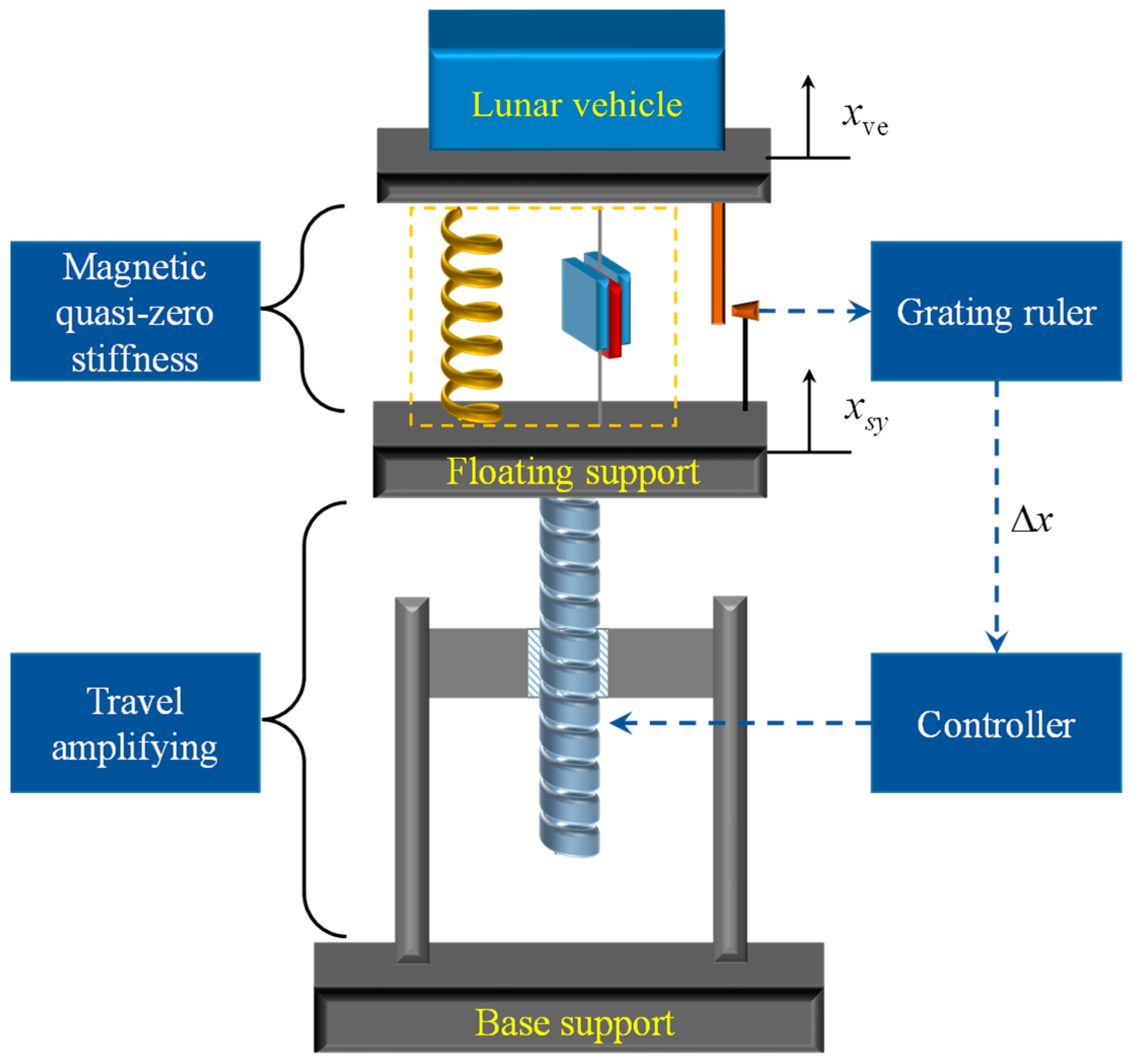
2.1. Design of Micro-Low-Gravity Simulation System for Lunar Vehicle
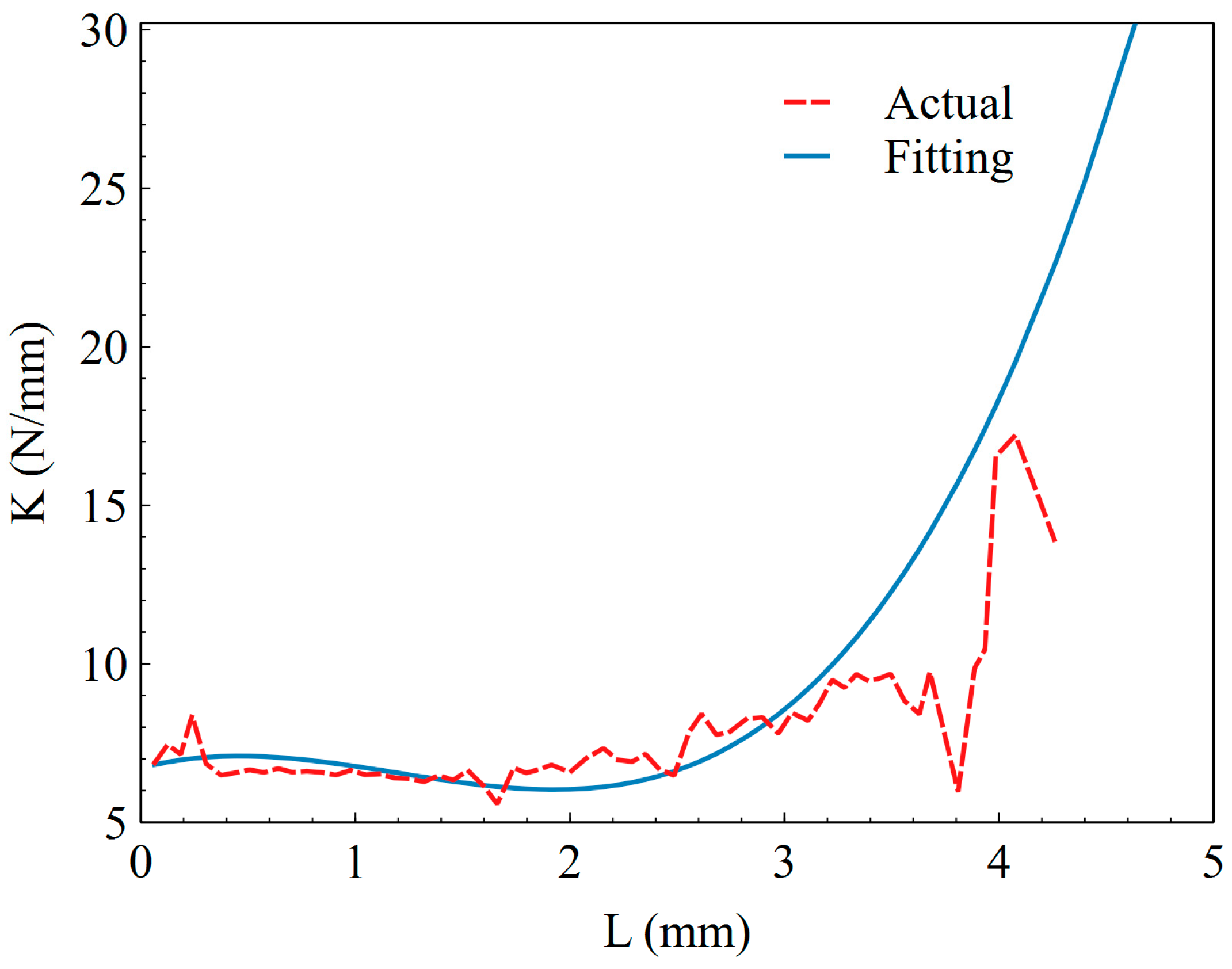
2.2. Principle of Magnetic Quasi-Zero Stiffness
2.3. M-QZS Vertical Constant Force System
2.3.1. Dynamic Model
2.3.2. Control System Design and Active Control Strategy
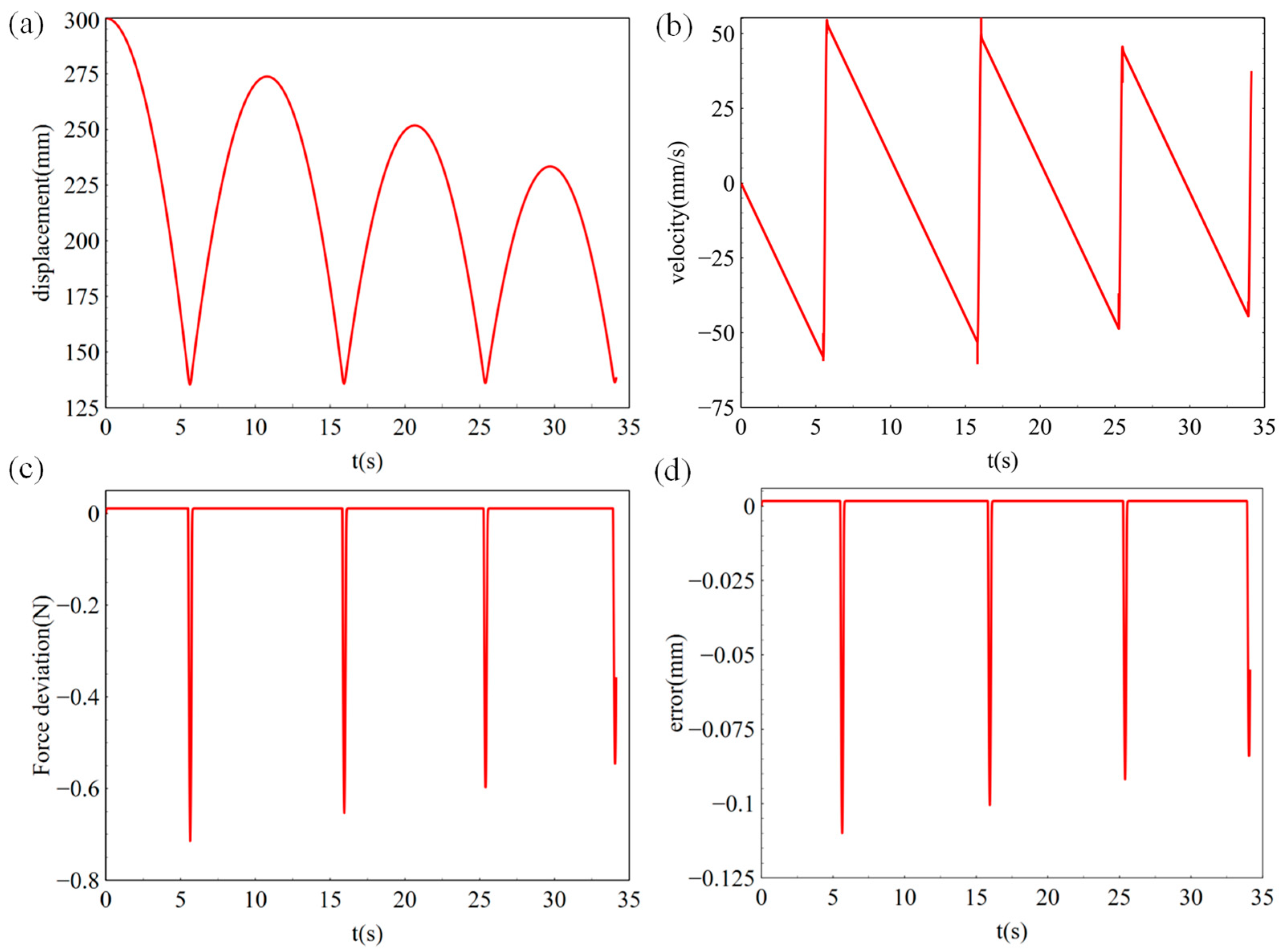
2.4. Dynamic Simulation of Micro-Low-Gravity Simulation System
3. Design and Performance Evaluation Experiment
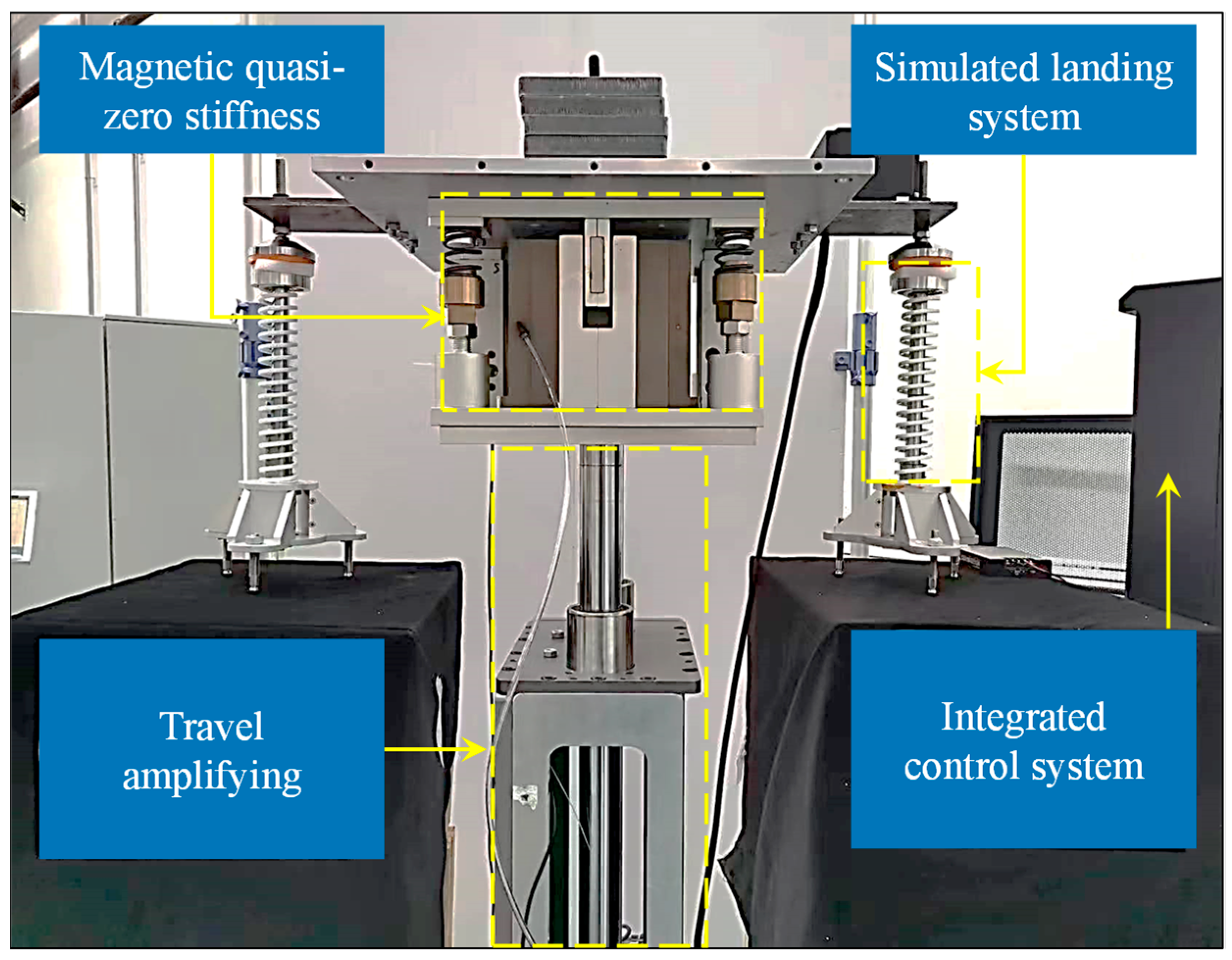
3.1. System Architecture
3.1.1. Structure Composition
3.1.2. Integrated Control System
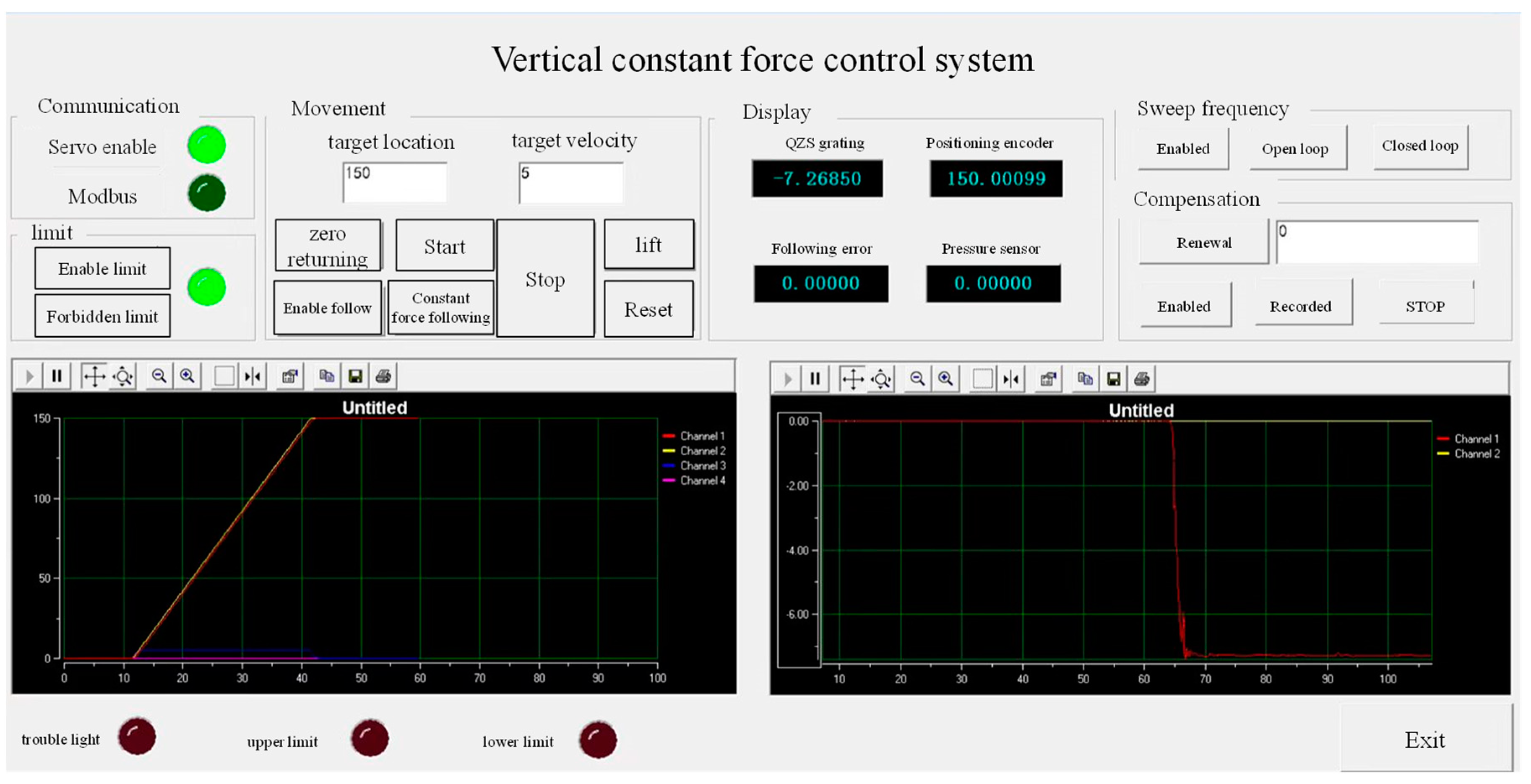
3.2. Test Method
4. Results and Discussion
4.1. Simulation Results
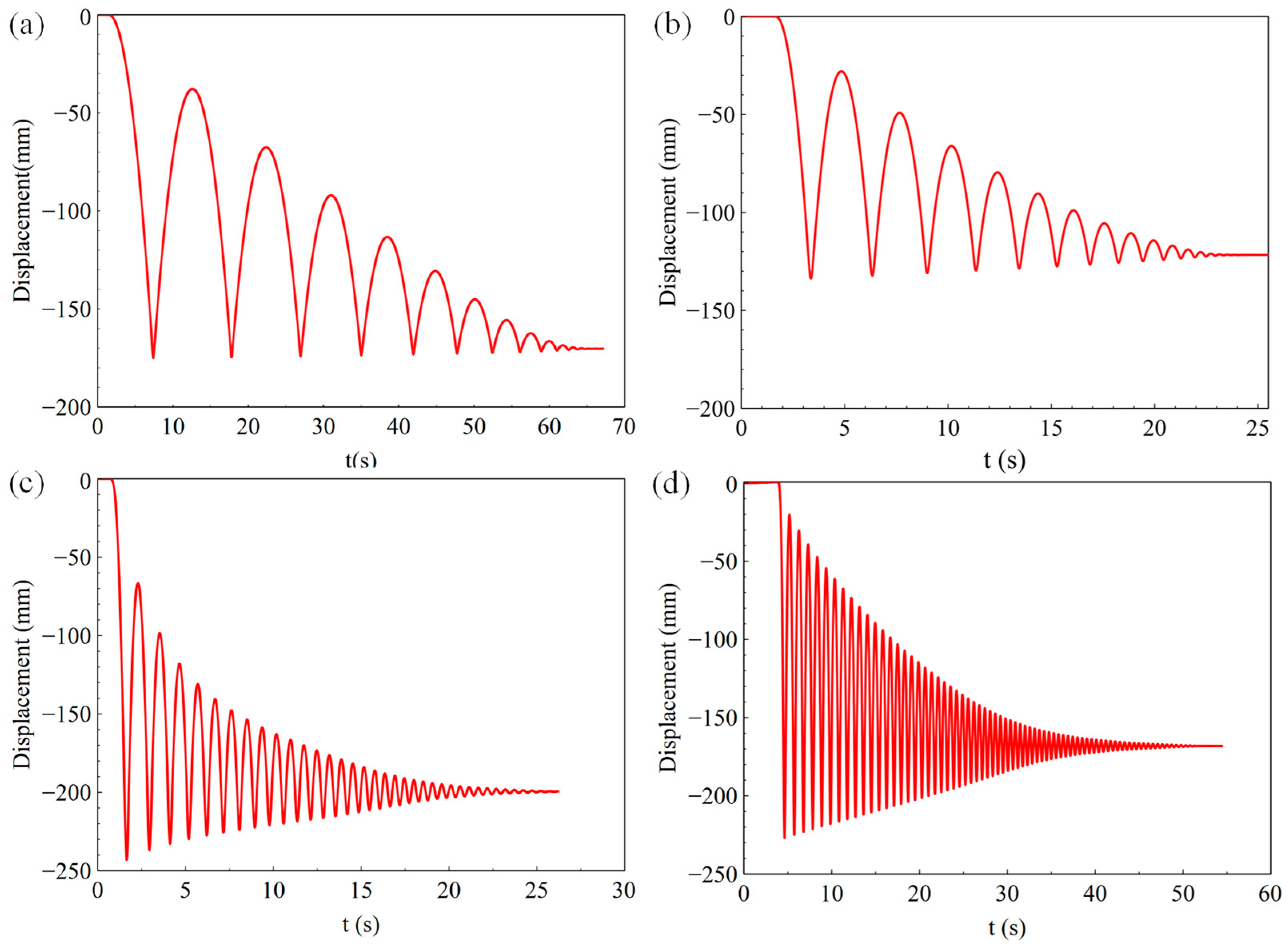
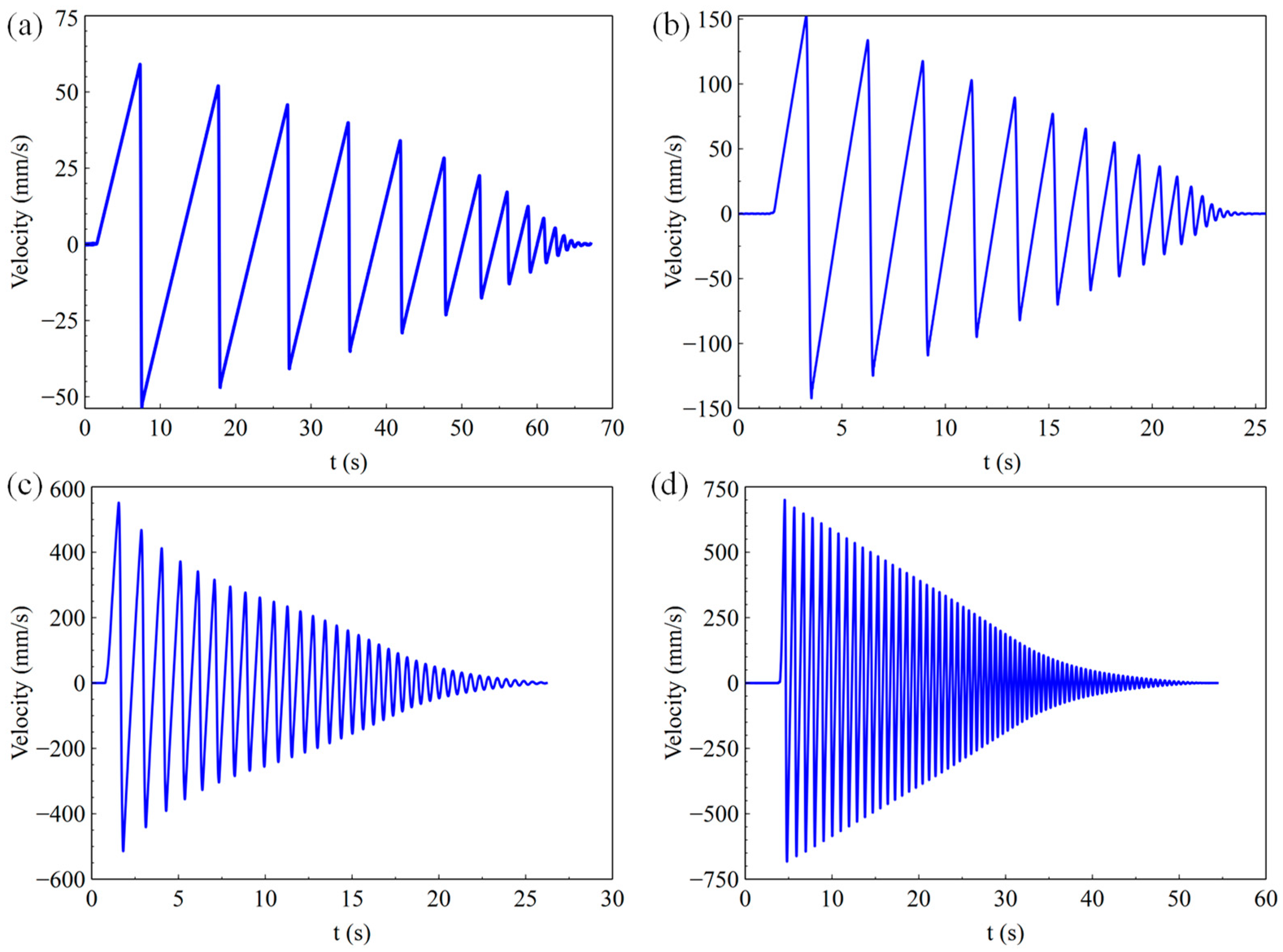
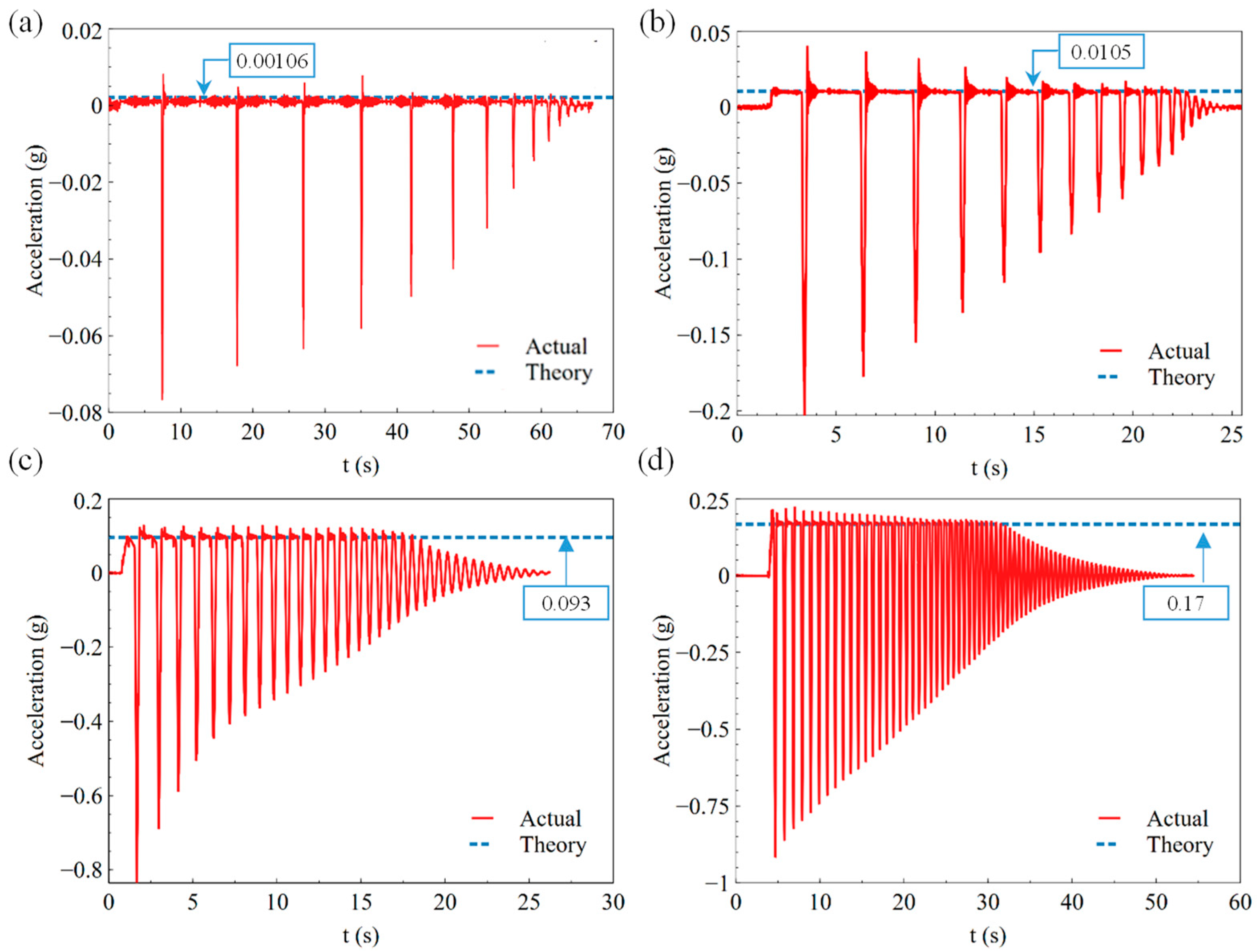
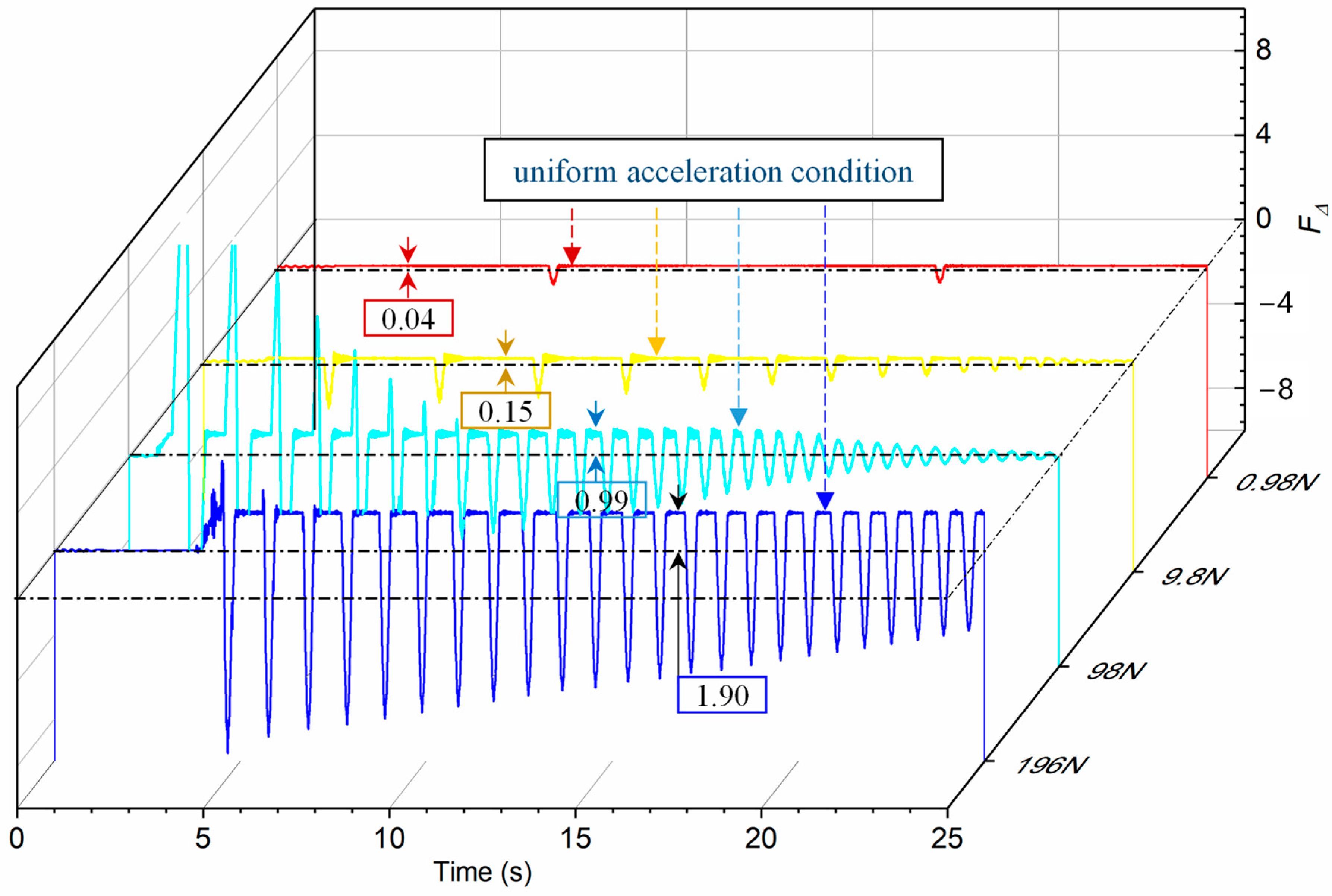
4.2. Experimental Results
4.3. Discussion
5. Conclusions
- The non-contact/frictionless M-QZS mechanism was combined with the stroke amplification structure, which realized high-precision and high-dynamic constant force maintenance in the vertical direction. It provided a new method for the micro-low-gravity simulation test of lunar vehicles on the ground.
- A position control method based on fuzzy PID parameter tuning was proposed, and the dynamic characteristics of the system were simulated and analyzed using joint simulation technology, which verified the feasibility and accuracy of the control model.
- The prototype of the micro-low-gravity simulation test system for the lunar vehicle was built, and landing buffer tests under different external forces were carried out. The results show that the system had good stability and high accuracy under different external loads. The force sensitivity was better than 0.1%, and the constant force error was less than 0.1%. The test object bounced up many times during the landing buffer test, which successfully reproduced the phenomenon of the lunar vehicle touchdown rebound during landing in a slight micro-low-gravity environment.
- Later, the research on vertical adaptive adjustment during horizontal movement of the lunar vehicle will be carried out. The performance of the test system to maintain vertical constant force accuracy will be further verified when the lunar vehicle walks on the undulating road surface in the next step.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lund, T. Lunar roving vehicle and exploration of the moon. In Early Exploration of the Moon: Ranger to Apollo, Luna to Lunniy Korabl; Springer: Berlin/Heidelberg, Germany, 2018; pp. 303–324. [Google Scholar]
- Wysor, R. Preliminary design study of a lunar gravity simulator. In Proceedings of the National Symposium on Saturn V/Apollo and Beyond, Huntsville, AL, USA, 11–14 June 1967. [Google Scholar]
- Hirt, C.; Kuhn, M. Convergence and divergence in spherical harmonic series of the gravitational field generated by high-resolution planetary topography-A case study for the Moon. J. Geophys. Res. Planets 2017, 122, 1727–1746. [Google Scholar] [CrossRef]
- Liu, Z. Single-Cable Gravity Compensation for Planetary Rovers and Experimental Researches; Harbin Institute of Technology: Harbin, China, 2013. [Google Scholar]
- Silva, A.F.B.; Santos, A.V.; Meggiolaro, M.A.; Neto, M.S. A rough terrain traction control technique for all-wheel-drive mobile robots. J. Braz. Soc. Mech. Sci. Eng. 2010, 32, 489–501. [Google Scholar] [CrossRef]
- Han, O.; Kienholz, D.; Janzen, P.; Kidney, S. Gravity-off-loading system for large-displacement ground testing of spacecraft mechanisms. In Proceedings of the 40th Aerospace Mechanisms Symposium, Mountain View, CA, USA, 12 May 2010. [Google Scholar]
- Kemurdjian, A.; Khakhanov, U. Development of simulation means for a gravity forces. In Robotics 2000; American Society Civil Engineers: Virginia, VA, USA, 2000; pp. 220–225. [Google Scholar]
- Liu, Z.; Gao, H.; Deng, Z. Design of the Low Gravity Simulation System for Planetary Rovers. Robot 2013, 35, 750–756. [Google Scholar] [CrossRef]
- Li, S.; Jiang, X.; Tao, T. Guidance summary and assessment of the Chang’e-3 powered descent and landing. J. Spacecr. Rocket. 2016, 53, 258–277. [Google Scholar] [CrossRef]
- Sun, Z.Z.; Zhang, H.; Jia, Y.; Xueying, W.U.; Shen, Z.R.; Ren, D.P.; Dang, Z.L.; Wang, C.; Zheng, G.U.; Chen, B.C. Ground validation technologies for Chang’E-3 lunar spacecraft. Sci. Sin. 2014, 44, 369. [Google Scholar]
- Hockman, B.J.; Frick, A.; Reid, R.G.; Nesnas, I.A.; Pavone, M. Design, control, and experimentation of internally-actuated rovers for the exploration of low-gravity planetary bodies. J. Field Robot. 2017, 34, 5–24. [Google Scholar] [CrossRef]
- Ren, D.; Li, J.; Zhang, Z.; Du, Y.; Huang, H.; Shu, Y.; Peng, J.; Ruan, J.; Zhang, W.; Zhang, G. Ground-test validation technologies for Chang’e-5 lunar probe. Sci. Sin. Technol. 2021, 51, 778–787. [Google Scholar] [CrossRef]
- Guo, L.; Wang, X.; Fan, R.L.; Bi, F. Review on Development of High-Static–Low-Dynamic-Stiffness Seat Cushion Mattress for Vibration Control of Seating Suspension System. Appl. Sci. 2020, 10, 2887. [Google Scholar] [CrossRef]
- Carrella, A.; Brennan, M.; Waters, T. Static analysis of a passive vibration isolator with quasi-zero-stiffness characteristic. J. Sound Vib. 2007, 301, 678–689. [Google Scholar] [CrossRef]
- Tang, R.; Huang, H.; Huang, Z. Design and experiment of a suspension device based on quasi-zero-stiffness technology for microgravity simulation. Spacecarft Environ. Eng. 2017, 34, 222–228. [Google Scholar]
- Niu, F.; Liu, Z.; Gao, H.; Yu, H.; Li, N.; Deng, Z. An enhanced cascade constant-force system based on an improved low-stiffness mechanism. Mech. Mach. Theory 2022, 167, 104544. [Google Scholar] [CrossRef]
- Zang, L.; Zhong, Q.; Chen, Y.; Hou, W.; Zhao, B.; Wu, Y. Effect of coating thickness on tribological properties of manganese phosphate conversion coating in different motion conditions. Tribol. Int. 2022, 176, 107894. [Google Scholar] [CrossRef]
- Zhou, R.; Zhou, Y.; Chen, X.; Hou, W.; Wang, C.; Wang, H.; Jiang, W. Gravity compensation method via magnetic quasi-zero stiffness combined with a quasi-zero deformation control strategy. Sci. China Technol. Sci. 2022, 65, 1738–1748. [Google Scholar] [CrossRef]
- Robertson, W.S.; Kidner, M.; Cazzolato, B.S.; Zander, A.C. Theoretical design parameters for a quasi-zero stiffness magnetic spring for vibration isolation. J. Sound Vib. 2009, 326, 88–103. [Google Scholar] [CrossRef]
- Wu, J.; Zeng, L.; Han, B.; Zhou, Y.; Luo, X.; Li, X.; Chen, X.; Jiang, W. Analysis and design of a novel arrayed magnetic spring with high negative stiffness for low-frequency vibration isolation. Int. J. Mech. Sci. 2022, 216, 106980. [Google Scholar] [CrossRef]
- Wu, W.; Chen, X.; Shan, Y. Analysis and experiment of a vibration isolator using a novel magnetic spring with negative stiffness. J. Sound Vib. 2014, 333, 2958–2970. [Google Scholar] [CrossRef]
- Allag, H.; Yonnet, J.-P. 3-D analytical calculation of the torque and force exerted between two cuboidal magnets. IEEE Trans. Magn. 2009, 45, 3969–3972. [Google Scholar] [CrossRef]
- Abushagur, A.A.; Arsad, N.; Reaz, M.I.; Bakar, A.A. Advances in bio-tactile sensors for minimally invasive surgery using the fibre Bragg grating force sensor technique: A survey. Sensors 2014, 14, 6633–6665. [Google Scholar] [CrossRef] [PubMed]
- Valle, P. Reduced gravity testing of robots (and humans) using the active response gravity offload system. In Proceedings of the International Conference on Intelligent Robots and Systems, Vancouver, BC, Canada, 24–28 September 2017. [Google Scholar]
- Zhang, H. Control Method Research Based on Suspended Low-Gravity Environment Simulation; Harbin Institute of Technology: Harbin, China, 2021. [Google Scholar]
- Li, H. Research of Zero Gravity Multidimensional Unfolding Test Apparatus; Zhejiang University of Technology: Hangzhou, China, 2015. [Google Scholar]





| Parameters | Value |
|---|---|
| external force (N) | 0.98 |
| mass of lunar vehicle (kg) | 94.5 |
| falling height (mm) | 170 |
| Parts | Parameters | Value |
|---|---|---|
| Servo motor | Power (kW) | 7.5 |
| Torque (Nm) | 48 | |
| rated speed (rpm) | 1500 | |
| Turn-screw | Stroke (mm) | 480 |
| Lead (mm) | 50 |
| Parameters | Value |
|---|---|
| mass of the weight (kg) | 0.1 |
| 1 | |
| 10 | |
| 20 | |
| mass of the lunar vehicle (kg) | 94.5 |
| falling height (mm) | 170 |
| Falling Parameters | Simulation | Experimental |
|---|---|---|
| Acceleration (g) | 0.001058 | 0.00106 ± 0.001 |
| Max velocity (mm/s) | 58.504 | 59.37 |
| First rebound period (s) | 10.59 | 10.39 |
| First rebound amplitude (mm) | 264.73 | 262.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, W.; Hao, Y.; Wang, C.; Chen, L.; Li, G.; Zhao, B.; Wang, H.; Wei, Q.; Xu, S.; Feng, K.; et al. Theoretical and Experimental Investigations on High-Precision Micro-Low-Gravity Simulation Technology for Lunar Mobile Vehicle. Sensors 2023, 23, 3458. https://doi.org/10.3390/s23073458
Hou W, Hao Y, Wang C, Chen L, Li G, Zhao B, Wang H, Wei Q, Xu S, Feng K, et al. Theoretical and Experimental Investigations on High-Precision Micro-Low-Gravity Simulation Technology for Lunar Mobile Vehicle. Sensors. 2023; 23(7):3458. https://doi.org/10.3390/s23073458
Chicago/Turabian StyleHou, Weijie, Yongbo Hao, Chang Wang, Lei Chen, Guangping Li, Baoshan Zhao, Hao Wang, Qingqing Wei, Shuo Xu, Kai Feng, and et al. 2023. "Theoretical and Experimental Investigations on High-Precision Micro-Low-Gravity Simulation Technology for Lunar Mobile Vehicle" Sensors 23, no. 7: 3458. https://doi.org/10.3390/s23073458
APA StyleHou, W., Hao, Y., Wang, C., Chen, L., Li, G., Zhao, B., Wang, H., Wei, Q., Xu, S., Feng, K., & Zang, L. (2023). Theoretical and Experimental Investigations on High-Precision Micro-Low-Gravity Simulation Technology for Lunar Mobile Vehicle. Sensors, 23(7), 3458. https://doi.org/10.3390/s23073458







