Split-Ring Resonator Based Sensor for the Detection of Amino Acids in Liquids
Abstract
1. Introduction
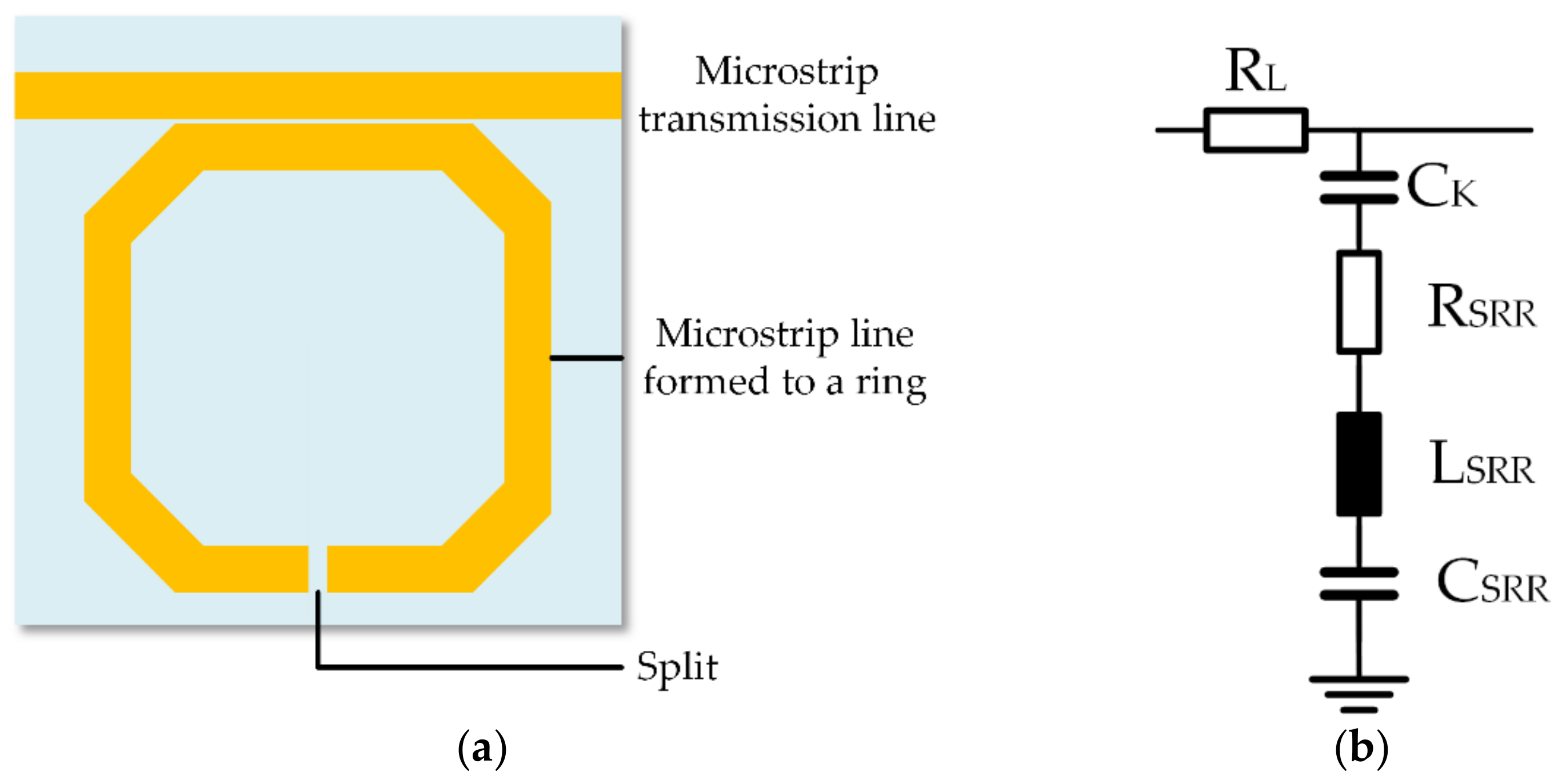
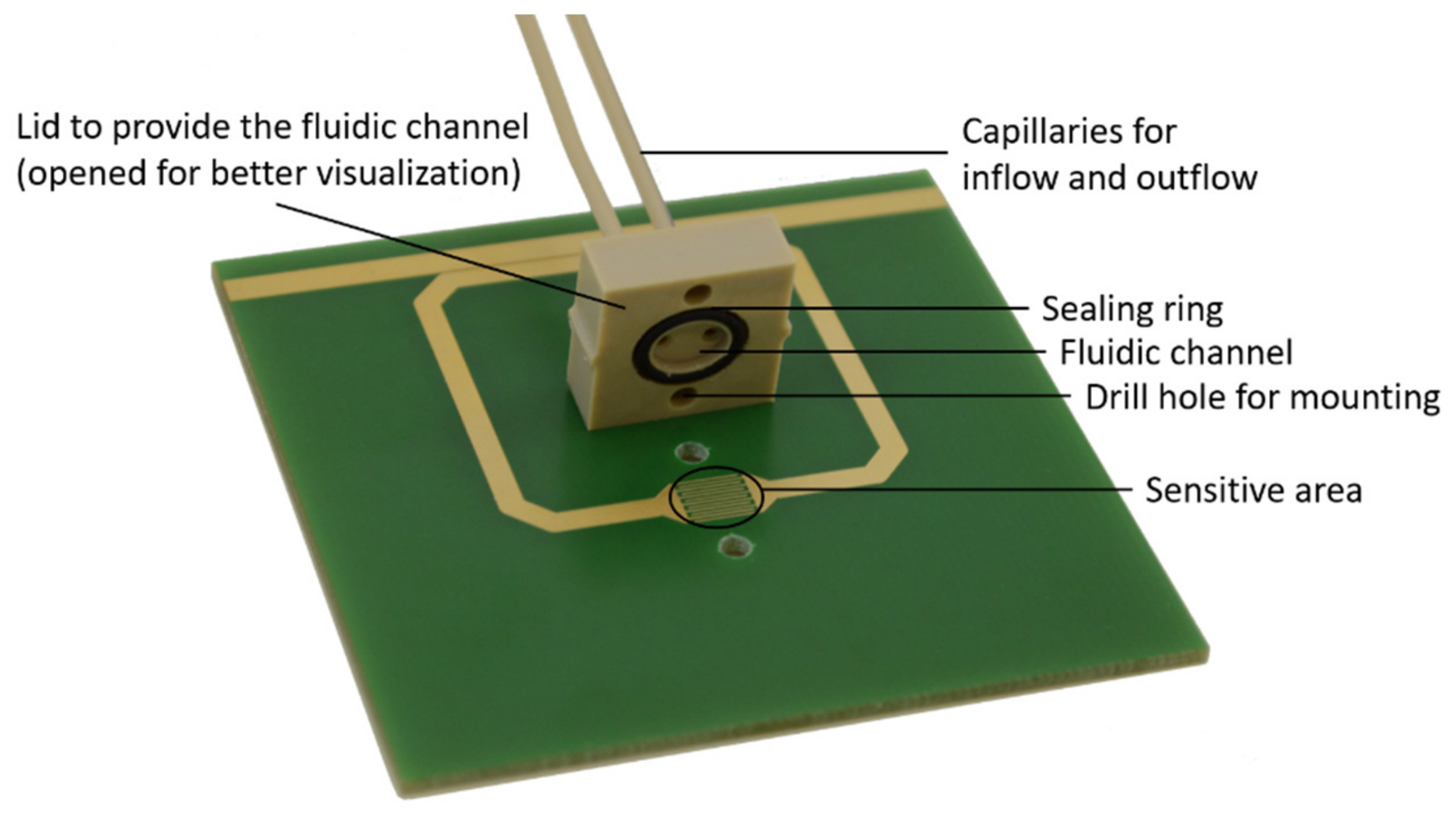
2. Materials and Methods
3. Results
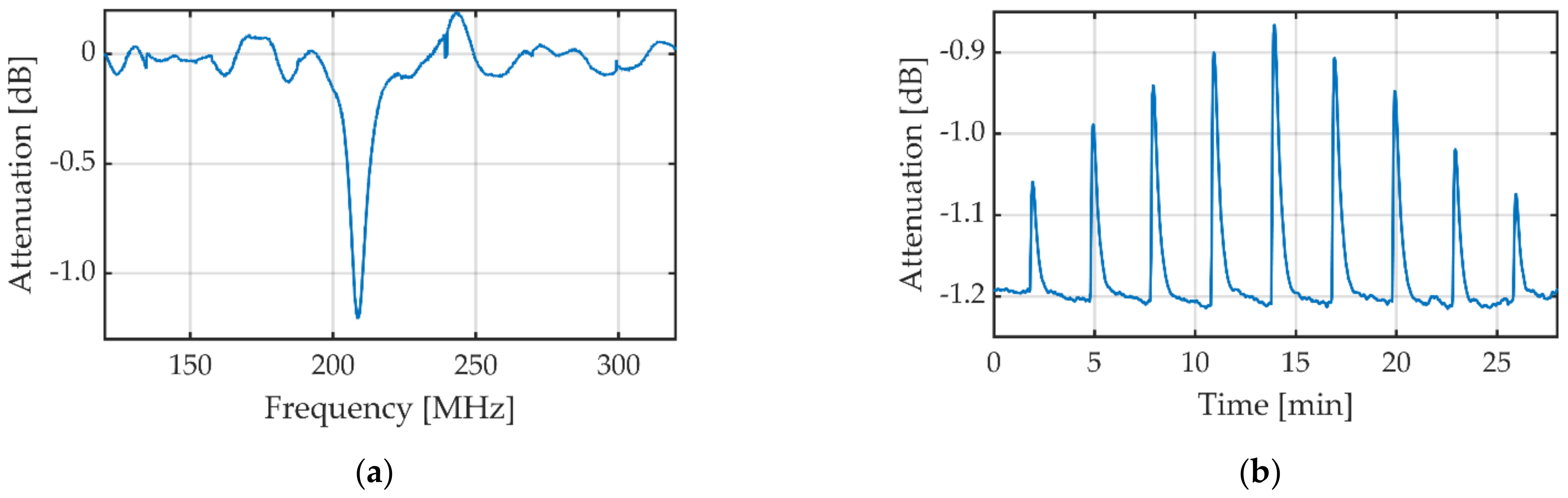
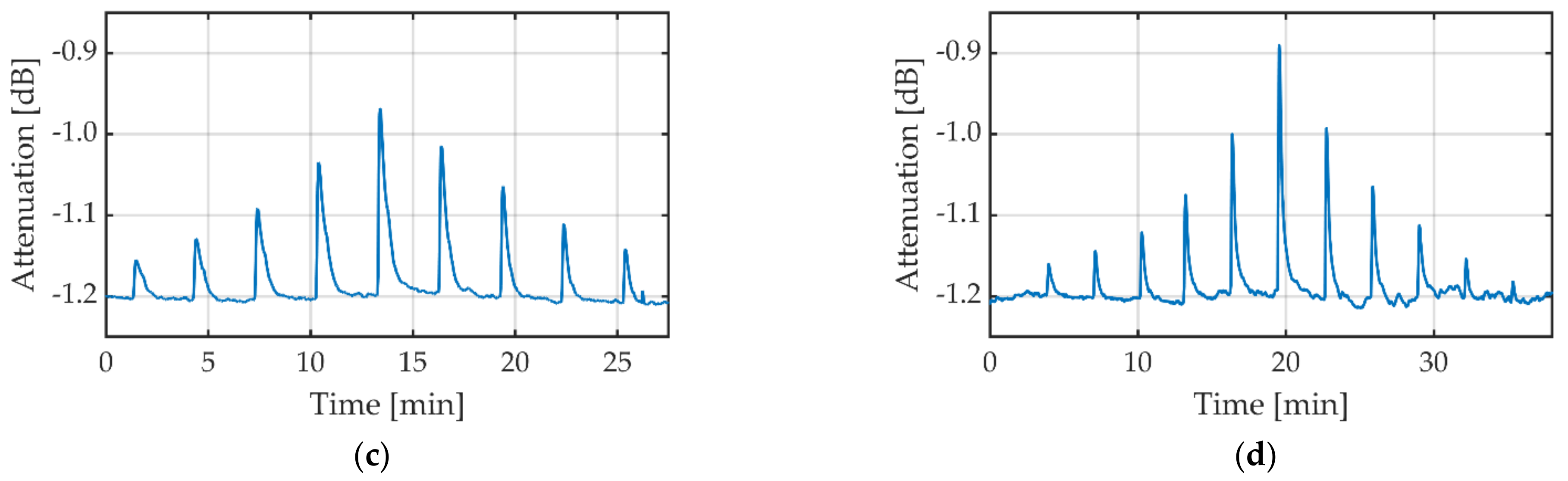
3.1. Evaluation Method
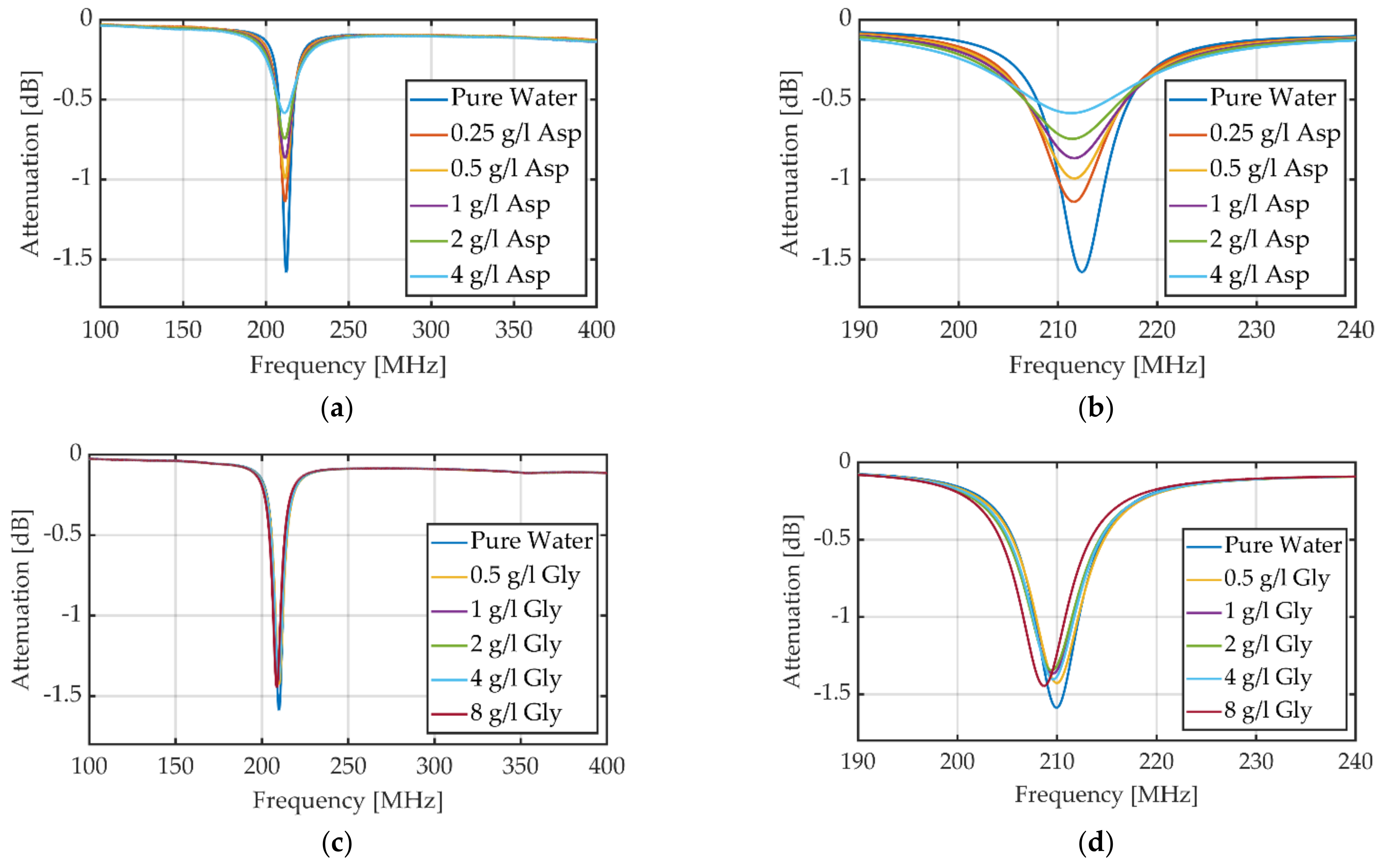
3.2. Measuring Amino Acids
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A



References
- Puentes, M.; Weiß, C.; Schüßler, M.; Jakoby, R. Sensor Array Based on Split Ring Resonators for Analysis of Organic Tissues. In Proceedings of the 2011 IEEE MTT-S International Microwave Symposium, Baltimore, MD, USA, 5–10 June 2011; pp. 1–4. [Google Scholar] [CrossRef]
- Naqui, J.; Durán-Sindreu, M.; Martín, F. Alignment and Position Sensors Based on Split Ring Resonators. Sensors 2012, 12, 11790–11797. [Google Scholar] [CrossRef]
- Albishi, A.; Ramahi, O.M. Detection of surface and subsurface cracks in metallic and non-metallic materials using a complementary split-ring resonator. Sensors 2014, 14, 19354–19370. [Google Scholar] [CrossRef] [PubMed]
- Reinecke, T.; Walter, J.-G.; Kobelt, T.; Ahrens, A.; Scheper, T.; Zimmermann, S. Design and evaluation of split-ring resonators for aptamer-based biosensors. J. Sens. Sens. Syst. 2018, 7, 101–111. [Google Scholar] [CrossRef]
- Lee, H.-J.; Lee, H.-S.; Yoo, K.-H.; Yook, J.-G. DNA sensing using split-ring resonator alone at microwave regime. J. Appl. Phys. 2010, 108, 14908. [Google Scholar] [CrossRef]
- Lee, H.-J.; Lee, J.-H.; Moon, H.-S.; Jang, I.-S.; Choi, J.-S.; Yook, J.-G.; Jung, H.-I. A planar split-ring resonator-based microwave biosensor for label-free detection of biomolecules. Sens. Actuators B Chem. 2012, 169, 26–31. [Google Scholar] [CrossRef]
- Torun, H.; Cagri Top, F.; Dundar, G.; Yalcinkaya, A.D. An antenna-coupled split-ring resonator for biosensing. J. Appl. Phys. 2014, 116, 124701. [Google Scholar] [CrossRef]
- Withayachumnankul, W.; Jaruwongrungsee, K.; Tuantranont, A.; Fumeaux, C.; Abbott, D. Metamaterial-based microfluidic sensor for dielectric characterization. Sens. Actuators A Phys. 2013, 189, 233–237. [Google Scholar] [CrossRef]
- Ebrahimi, A.; Withayachumnankul, W.; Al-Sarawi, S.F.; Abbott, D. High-Sensitivity Metamaterial-Inspired Sensor for Microfluidic Dielectric Characterization. IEEE Sens. J. 2014, 14, 1345–1351. [Google Scholar] [CrossRef]
- Hitzemann, M.; Dehning, K.J.; Gehl, A.V.; Sterr, E.-F.; Zimmermann, S. Fast Readout of Split-Ring Resonators Made Simple and Low-Cost for Application in HPLC. Electronics 2022, 11, 1139. [Google Scholar] [CrossRef]
- Dehning, K.J.; Hitzemann, M.; Sterr, E.-F.; Zimmermann, S. P8.9-Split-Ring Resonator as Detector for Liquid Chromatography. In Proceedings of the 15. Dresdner Sensor-Symposium 2021, Dresden, Germany, 6–8 December 2021; pp. 285–289. [Google Scholar] [CrossRef]
- Verma, A.; Bhushan, S.; Tripathi, P.N.; Goswami, M.; Singh, B.R. A defected ground split ring resonator for an ultra-fast, selective sensing of glucose content in blood plasma. J. Electromagn. Waves Appl. 2017, 31, 1049–1061. [Google Scholar] [CrossRef]
- Camli, B.; Kusakci, E.; Lafci, B.; Salman, S.; Torun, H.; Yalcinkaya, A. A Microwave Ring Resonator Based Glucose Sensor. Procedia Eng. 2016, 168, 465–468. [Google Scholar] [CrossRef]
- Ye, W.; Zhao, W.-S.; Wang, J.; Wang, D.-W.; Wang, G. A Split-Ring Resonator-Based Planar Microwave Sensor for Microfluidic Applications. In Proceedings of the 2022 IEEE MTT-S International Microwave Biomedical Conference (IMBioC), Suzhou, China, 16–18 May 2022; IEEE: Piscataway, NJ, USA, 2022; pp. 34–36, ISBN 978-1-6654-2340-3. [Google Scholar]
- Wu, W.-J.; Zhao, W.-S. A Quality Factor Enhanced Microwave Sensor Based on Modified Split-Ring Resonator for Microfluidic Applications. IEEE Sens. J. 2022, 22, 22582–22590. [Google Scholar] [CrossRef]
- Yeo, J.; Lee, J.-I. High-Sensitivity Microwave Sensor Based on an Interdigital-Capacitor-Shaped Defected Ground Structure for Permittivity Characterization. Sensors 2019, 19, 498. [Google Scholar] [CrossRef]
- Ye, W.; Wang, D.-W.; Wang, J.; Wang, G.; Zhao, W.-S. An Improved Split-Ring Resonator-Based Sensor for Microfluidic Applications. Sensors 2022, 22, 8534. [Google Scholar] [CrossRef]
- Bao, X.; Zhang, M.; Ocket, I.; Bao, J.; Kil, D.; Liu, Z.; Puers, R.; Schreurs, D.; Nauwelaers, B. Integration of Interdigitated Electrodes in Split-Ring Resonator for Detecting Liquid Mixtures. IEEE Trans. Microw. Theory Techn. 2020, 68, 2080–2089. [Google Scholar] [CrossRef]
- Schulz, G.E.; Schirmer, R.H. Principle of Protein Structure; Springer: New York, NY, USA, 1979; ISBN 978-0-387-90334-7. [Google Scholar]
- Fürst, P.; Stehle, P. What Are the Essential Elements Needed for the Determination of Amino Acid Requirements in Humans? J. Nutr. 2004, 134, 1558S–1565S. [Google Scholar] [CrossRef]
- Larson, T.M.; Gawlitzek, M.; Evans, H.; Albers, U.; Cacia, J. Chemometric evaluation of on-line high-pressure liquid chromatography in mammalian cell cultures: Analysis of amino acids and glucose. Biotechnol. Bioeng. 2002, 77, 553–563. [Google Scholar] [CrossRef]
- Kabus, P.; Koch, G. Quantitative Determination of Amino Acids in Tissue Culture Cells by High Performance Liquid Chromatography. Biochem. Biophys. Res. Commun. 1982, 2, 783–790. [Google Scholar] [CrossRef]
- Askretkov, A.D.; Klishin, A.A.; Zybin, D.I.; Orlova, N.V.; Kholodova, A.V.; Lobanova, N.V.; Seregin, Y.A. Determination of Twenty Proteinogenic Amino Acids and Additives in Cultural Liquid by High-Performance Liquid Chromatography. J. Anal. Chem. 2020, 75, 1038–1045. [Google Scholar] [CrossRef]
- Salazar, A.; Keusgen, M.; von Hagen, J. Amino acids in the cultivation of mammalian cells. Amino Acids 2016, 48, 1161–1171. [Google Scholar] [CrossRef]
- Kaspar, H.; Dettmer, K.; Gronwald, W.; Oefner, P.J. Advances in amino acid analysis. Anal. Bioanal. Chem. 2009, 393, 445–452. [Google Scholar] [CrossRef] [PubMed]
- Lippmann, M.; Hermeling, L.; Hitzemann, M.; Dehning, K.J.; Zimmermann, S. Electronics for Continuously Measuring the Resonance Frequency and Attenuation of a Split-Ring Resonator. In Proceedings of the 16. Dresdner Sensor-Symposium 2022, Dresden, Germany, 5–7 December 2022; pp. 177–182. [Google Scholar] [CrossRef]
- Dehning, K.J.; Hitzemann, M.; Gossmann, A.; Zimmermann, S. Split-Ring Resonator for measuring low amounts of glutamic acid in pure water. In Proceedings of the 16. Dresdner Sensor-Symposium 2022, Dresden, Germany, 5–7 December 2022; pp. 132–135. [Google Scholar] [CrossRef]
- Multi-CB. Basic Design Rules für Leiterplatten v2.4—05.2021. Available online: https://www.multi-circuit-boards.eu/fileadmin/user_upload/downloads/leiterplatten_design-hilfe/Miltu-CB-Leiterplatten_Basic-Design-Rules-pdf (accessed on 7 December 2022).
- Apelblat, A.; Manzurola, E.; Orekhova, Z. Electrical Conductance Studies in Aqueous Solutions with Aspartic Ions. J. Solut. Chem. 2008, 37, 97–105. [Google Scholar] [CrossRef]
- Pethig, R. Dielectric Properties of Biological Materials: Biophysical and Medical Applications. IEEE Trans. Electr. Insul. 1984, EI-19, 453–474. [Google Scholar] [CrossRef]
- Rohde & Schwarz. R&S®ZNL Vector Network Analyzer Specifications. Available online: https://scdn.rohde-schwarz.com/ur/pws/dl_downloads/dl_common_library/dl_brochures_and_datasheets/pdf_1/ZNL_dat-sw_en_3607-1071-22_v0700.pdf (accessed on 7 December 2022).
- Taylor, W.R. The Classification of Amino Acid Conservation. J. Theor. Biol. 1986, 119, 205–218. [Google Scholar] [CrossRef]



| Amino Acid | Sensitivities of Different Evaluation Methods | ||
|---|---|---|---|
| Tracking Resonance Frequency [MHz/M] | Attenuation at Fixed Frequency [dB/M] * | ||
| Resonance | Turning Point | ||
| Aspartic Acid | 45.27 | 98.93 | 12.01 |
| Glycine | 20.76 | 12.21 | 4.04 |
| Lysine | 170.17 | 39.33 | 7.45 |
| Threonine | 34.81 | 28.91 | 7.85 |
| Amino Acid | Limit of Detection [µM] of Different Evaluation Methods | ||
|---|---|---|---|
| Tracking Resonance Frequency | Attenuation at Fixed Frequency | ||
| Resonance | Turning Point | ||
| Aspartic Acid | 4431.18 | 122.50 | 3721.72 |
| Glycine | 9660.91 | 992.21 | 11,070.18 |
| Lysine | 1178.87 | 308.17 | 5996.93 |
| Threonine | 5763.30 | 419.18 | 5692.34 |
| Amino Acid | Short | Isoelectric Point [32] | Sensitivity | Limit of Detection |
|---|---|---|---|---|
| Aspartic Acid | Asp | 2.85 | 97.49 dB/M | 122.50 µM |
| Glutamic Acid | Glu | 3.22 | 115.94 dB/M | 105.44 µM |
| Glutamine | Gln | 5.65 | 47.57 dB/M | 624.50 µM |
| Glycine | Gly | 5.97 | 12.21 dB/M | 992.21 µM |
| Histidine | His | 7.47 | 52.83 dB/M | 229.38 µM |
| Isoleucine | Ile | 5.94 | 7.75 dB/M | 1563.28 µM |
| Lysine | Lys | 9.59 | 39.33 dB/M | 308.17 µM |
| Methionine | Met | 5.74 | 8.06 dB/M | 1504.06 µM |
| Threonine | Thr | 5.60 | 28.91 dB/M | 419.18 µM |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dehning, K.J.; Hitzemann, M.; Gossmann, A.; Zimmermann, S. Split-Ring Resonator Based Sensor for the Detection of Amino Acids in Liquids. Sensors 2023, 23, 645. https://doi.org/10.3390/s23020645
Dehning KJ, Hitzemann M, Gossmann A, Zimmermann S. Split-Ring Resonator Based Sensor for the Detection of Amino Acids in Liquids. Sensors. 2023; 23(2):645. https://doi.org/10.3390/s23020645
Chicago/Turabian StyleDehning, Kirsten J., Moritz Hitzemann, Alexander Gossmann, and Stefan Zimmermann. 2023. "Split-Ring Resonator Based Sensor for the Detection of Amino Acids in Liquids" Sensors 23, no. 2: 645. https://doi.org/10.3390/s23020645
APA StyleDehning, K. J., Hitzemann, M., Gossmann, A., & Zimmermann, S. (2023). Split-Ring Resonator Based Sensor for the Detection of Amino Acids in Liquids. Sensors, 23(2), 645. https://doi.org/10.3390/s23020645







