Optimization of an Inductive Displacement Transducer
Abstract
:1. Introduction
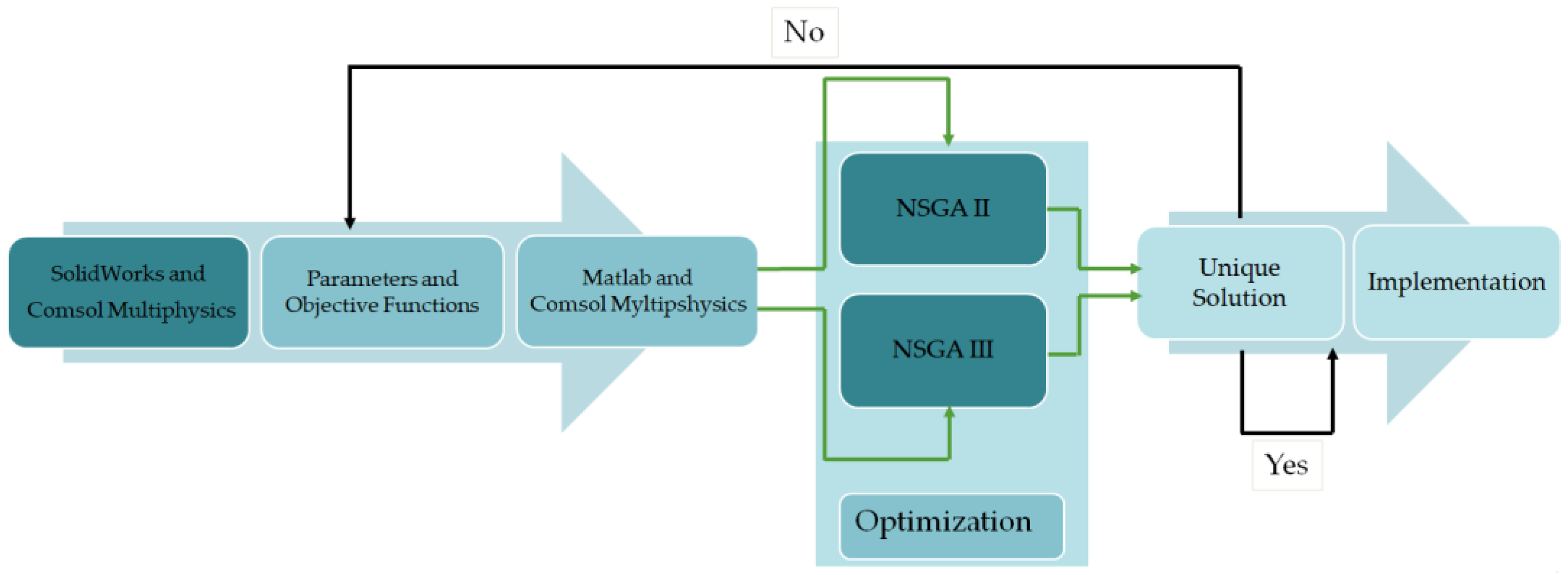
2. Materials and Methods
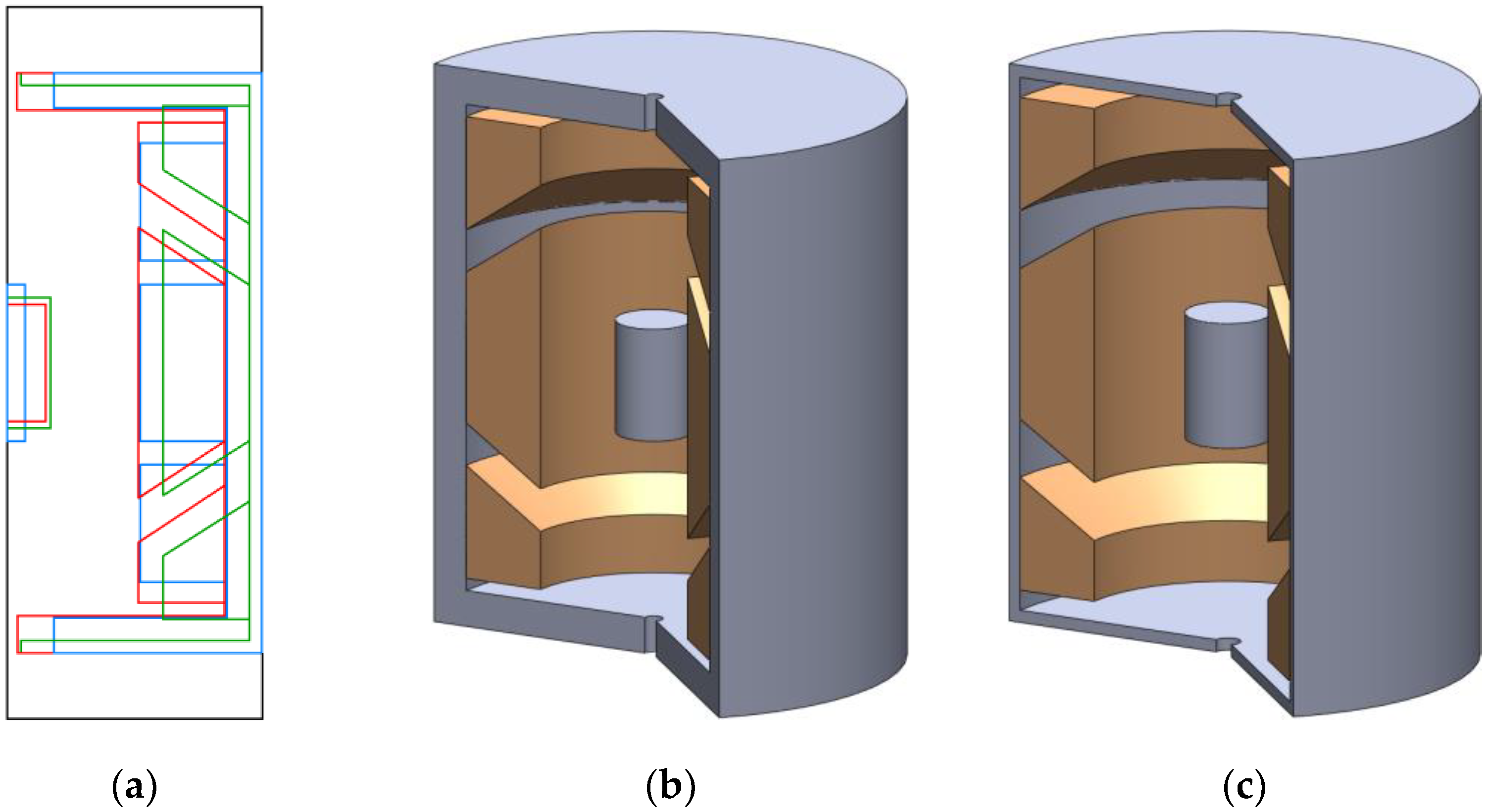
2.1. The First Approach
2.2. The Second Approach—Extending the Operational Range from 0 to 18 (mm)
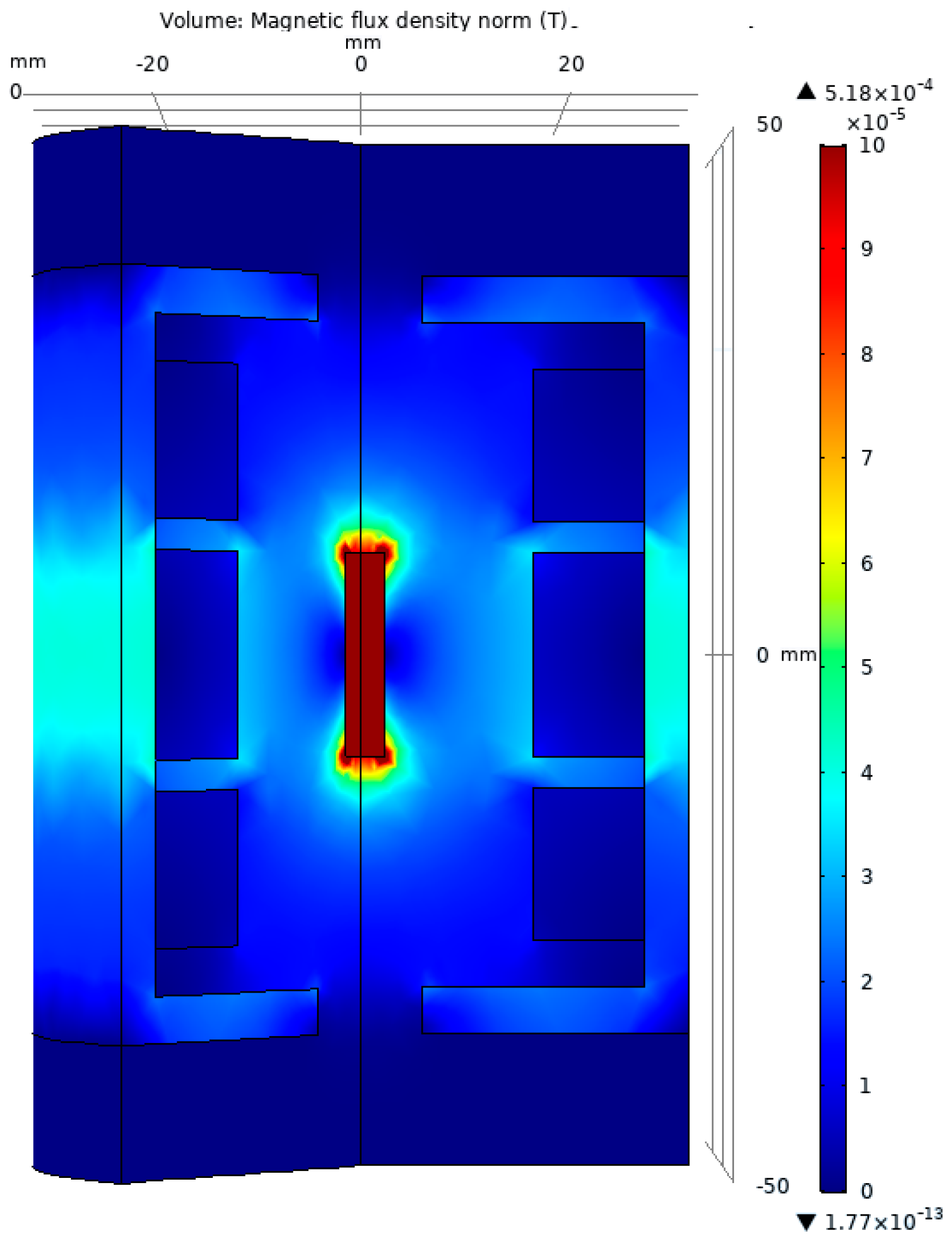
3. Results
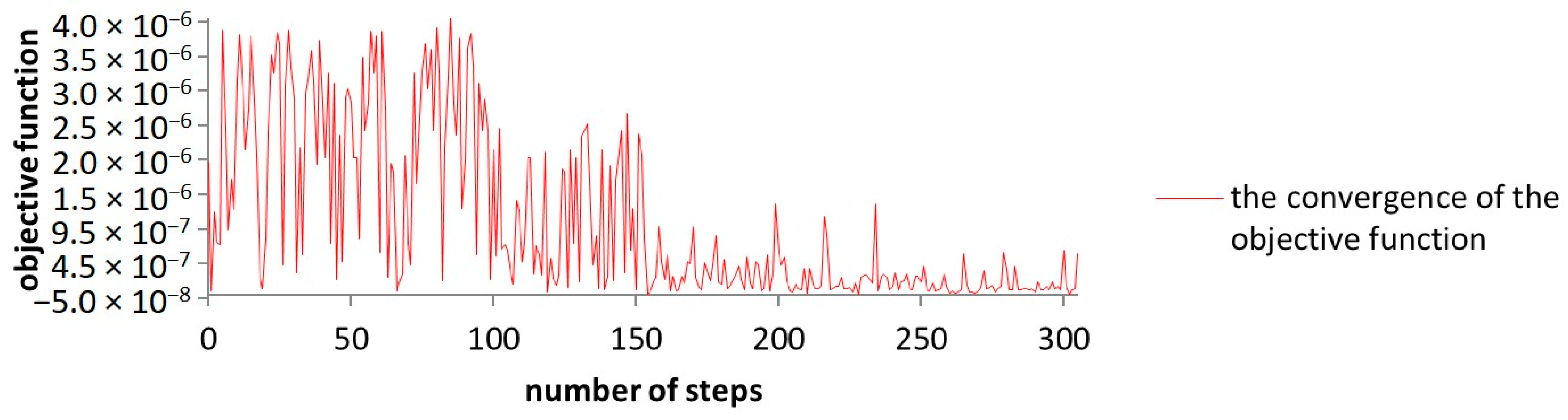
3.1. NSGA-II Optimization
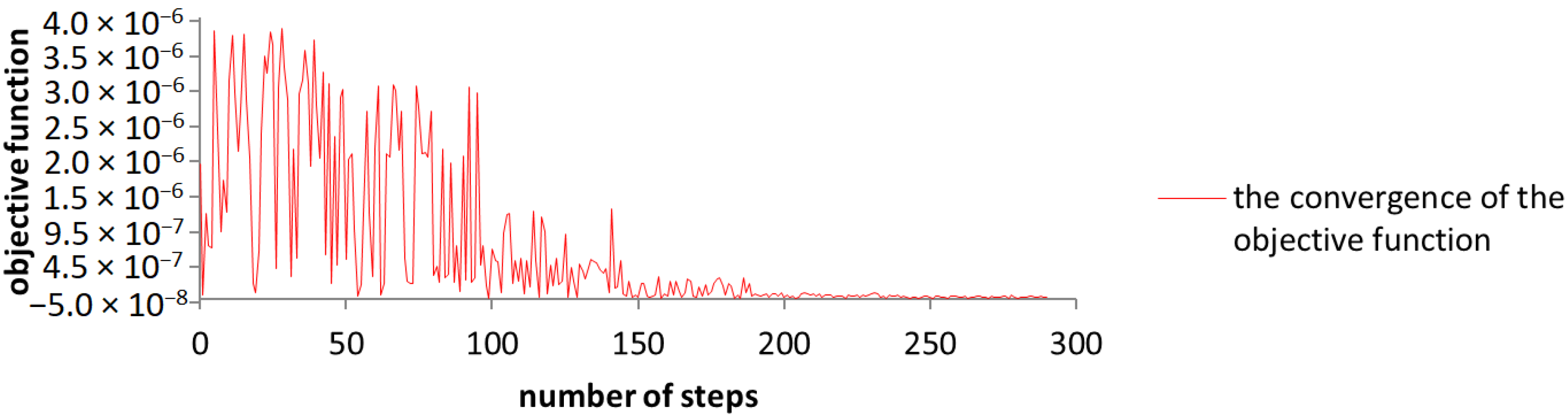
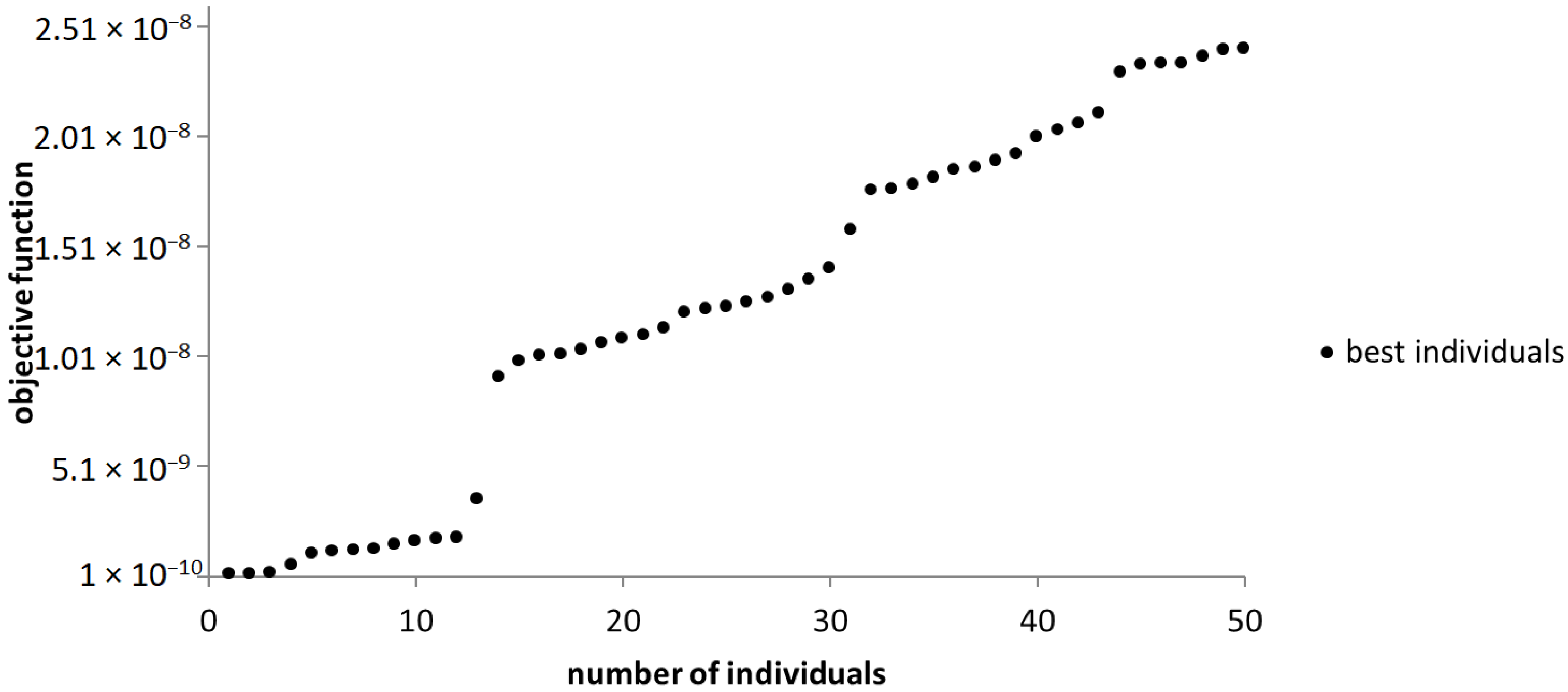
3.2. NSGA-III Optimization
4. Discussion
4.1. The Discussion Based on the NSGA-II Optimization
4.2. The Discussion Based on the NSGA-III Optimization
4.3. Results Comparison
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Petchmaneelumka, W.; Koodtalang, W.; Songsuwankit, K.; Riewruja, V. Linear range extension for LVDT using analog lookup table. In Proceedings of the 2018 International Conference on Engineering, Applied Sciences, and Technology (ICEAST), Phuket, Thailand, 4–7 July 2018; pp. 1–4. [Google Scholar] [CrossRef]
- Ara, K. A Differential Transformer with Temperature- and Excitation-Independent Output. IEEE Trans. Instrum. Meas. 1972, 21, 249–255. [Google Scholar] [CrossRef]
- Topa, V.; Munteanu, C.; De May, G.; Deconinck, J.; Simion, E. Optimal Design of the Electromagnetic Devices Using Numerical Methods; VUB University Press: Brussels, Belgium, 2000; pp. 110–115. [Google Scholar]
- Sykulski, J.K.; Sykulska, E.; Hughes, S.T. Application of finite element modelling in LVDT design. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 1992, 11, 73–76. [Google Scholar] [CrossRef]
- Wang, Z.; Duan, Z. The research of LVDT nonlinearity data compensation based on RBF neural network. In Proceedings of the 2008 7th World Congress on Intelligent Control and Automation, Chongqing, China, 25–27 June 2008; pp. 4591–4594. [Google Scholar] [CrossRef]
- Mishra, S.K.; Panda, G.; Das, D.P.; Pattanaik, S.K.; Meher, M.R. A novel method of designing LVDT using artificial neural network. In Proceedings of the 2005 International Conference on Intelligent Sensing and Information Processing, Chennai, India, 4–7 January 2005; pp. 223–227. [Google Scholar] [CrossRef]
- Mishra, S.K.; Panda, G.; Das, D.P. A Novel Method of Extending the Linearity Range of Linear Variable Differential Transformer Using Artificial Neural Network. IEEE Trans. Instrum. Meas. 2010, 59, 947–953. [Google Scholar] [CrossRef]
- Das, S.; Das, D.P.; Behera, S.K. Enhancing the linearity of LVDT by two-stage functional link artificial neural network with high accuracy and precision. In Proceedings of the 2013 IEEE 8th Conference on Industrial Electronics and Applications (ICIEA), Melbourne, VIC, Australia, 19–21 June 2013. [Google Scholar] [CrossRef]
- Angkeaw, K.; Chunchay, S.; Thongdit, P. A Wide Linearity Range of LVDT Using Approximate Function. In Proceedings of the 2021 18th International Conference on Electrical Engineering/Electronics, Computer, Telecommunications and Information Technology (ECTI-CON), Chiang Mai, Thailand, 19–22 May 2021. [Google Scholar] [CrossRef]
- Rerkratn, A.; Tongcharoen, J.; Petchmaneelumka, W.; Riewruja, V. Linear-Range Extension for Linear Variable Differential Transformer Using Hyperbolic Sine Function. Sensors 2022, 22, 3674. [Google Scholar] [CrossRef] [PubMed]
- Drandić, A.; Frljić, S.; Trkulja, B. Methodology for Eddy Current Losses Calculation in Linear Variable Differential Transformers (LVDTs). Sensors 2023, 23, 1760. [Google Scholar] [CrossRef] [PubMed]
- Saurav, S.; Murugan, S. Selection of Material for Magnetic Core of LVDT for High Temperature Application. In Proceedings of the 2019 2nd International Conference on Intelligent Computing, Instrumentation and Control Technologies (ICICICT), Kannur, India, 5–6 July 2019. [Google Scholar] [CrossRef]
- Félix, M.; Lizárraga, A.; Islas, A.; González, A. Analysis of a ferrofluid core LVDT displacement sensor. In Proceedings of the IECON 2010—36th Annual Conference on IEEE Industrial Electronics Society, Glendale, AZ, USA, 7–10 November 2010. [Google Scholar] [CrossRef]
- Liu, X.; Wang, H.; Yang, X.; Lai, J. Prediction and Evaluation of Fatigue Strength via Mechanical Behavior of Materials. J. Chin. Soc. Mech. Eng. Trans. Chin. Inst. Eng. Ser. C 2022, 43, 229–238. [Google Scholar]
- Liu, X.; Wu, Q.; Su, S.; Wang, Y. Evaluation and prediction of material fatigue characteristics under impact loads: Review and prospects. Int. J. Struct. Integr. 2022, 13, 251–277. [Google Scholar] [CrossRef]
- Narayanan, G. Probabilistic fatigue model for cast alloys of aero engine applications. Int. J. Struct. Integr. 2021, 12, 454–469. [Google Scholar] [CrossRef]
- Mociran, B.; Oglejan, R. Methods of Interconnecting Designing Programs Simulation and Optimization in Engineering. In Proceedings of the 2018 International Conference and Exposition on Electrical and Power Engineering (EPE), Iasi, Romania, 18–19 October 2018; pp. 475–478. [Google Scholar] [CrossRef]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A fast and elitist multiobjective genetic algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Ibrahim, A.; Rahnamayan, S.; Martin, M.V.; Deb, K. EliteNSGA-III: An improved evolutionary many-objective optimization algorithm. In Proceedings of the IEEE Congress on Evolutionary Computation (CEC), Vancouver, BC, Canada, 24–29 July 2016; pp. 973–982. [Google Scholar] [CrossRef]









| Initial Model | Value |
|---|---|
| Length of the secondary coil—p1 | 15 (mm) |
| Length of the magnetic core—p2 | 20 (mm) |
| Width of the magnetic core—p3 | 2.25 (mm) |
| Thickness of the casing—p4 | 4.50 (mm) |
| Distance casing–secondary coil—p5 | 4.50 (mm) |
| Width of the casing—p6 | 26.50 (mm) |
| Standardized position of the magnetic core—p7 | 0, 2, 4, 6, 8, 10, 12, 14, 16, 18 (mm) |
| Length of the secondary coils that maintains the same values—p8 | 15 (mm) |
| Length of the primary coil that maintains the same values—p9 | 20 (mm) |
| Height of the LVDT that maintains the same values—p10 | 74 (mm) |
| Radius of the LVDT from the center of the magnetic core, at shell extremity, that maintains the same values—p11 | 32.50 (mm) |
| Value of the objective function—f(p0) | 1.7334 × 10−8 (V2) |
| Parameter 2D Cross Section NSGA-II | Value |
|---|---|
| Length of the secondary coil | 7.69 (mm) |
| Length of the magnetic core | 14.87 (mm) |
| Width of the magnetic core | 4.90 (mm) |
| Width of the casing | 31.24 (mm) |
| Thickness of the casing | 4.76 (mm) |
| Distance casing–secondary coil | 1.61 (mm) |
| Standardized position of the magnetic core | 0, 2, 4, 6, 8, 10, 12, 14, 16, 18 (mm) |
| Value of the objective function | 2.37856 × 10−10 (V2) |
| Parameter 2D Cross Section NSGA-III | Value |
|---|---|
| Length of the secondary coil | 8.06 (mm) |
| Length of the magnetic core | 16.56 (mm) |
| Width of the magnetic core | 5.53 (mm) |
| Width of the casing | 30.76 (mm) |
| Thickness of the casing | 1.62 (mm) |
| Distance casing–secondary coil | 2.62 (mm) |
| Standardized position of the magnetic core | 0, 2, 4, 6, 8, 10, 12, 14, 16, 18 (mm) |
| Value of the objective function | 2.29 × 10−9 (V2) |
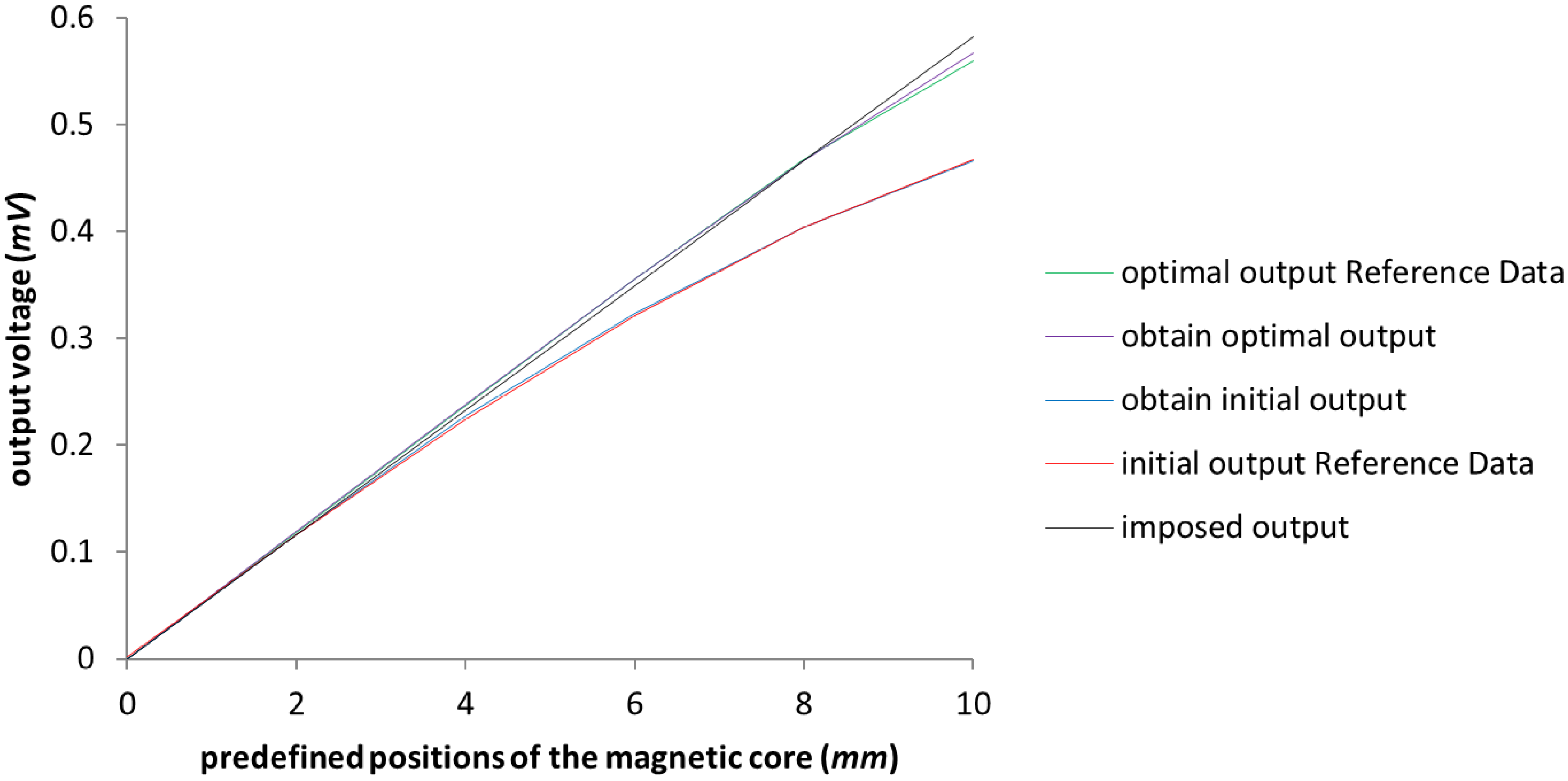
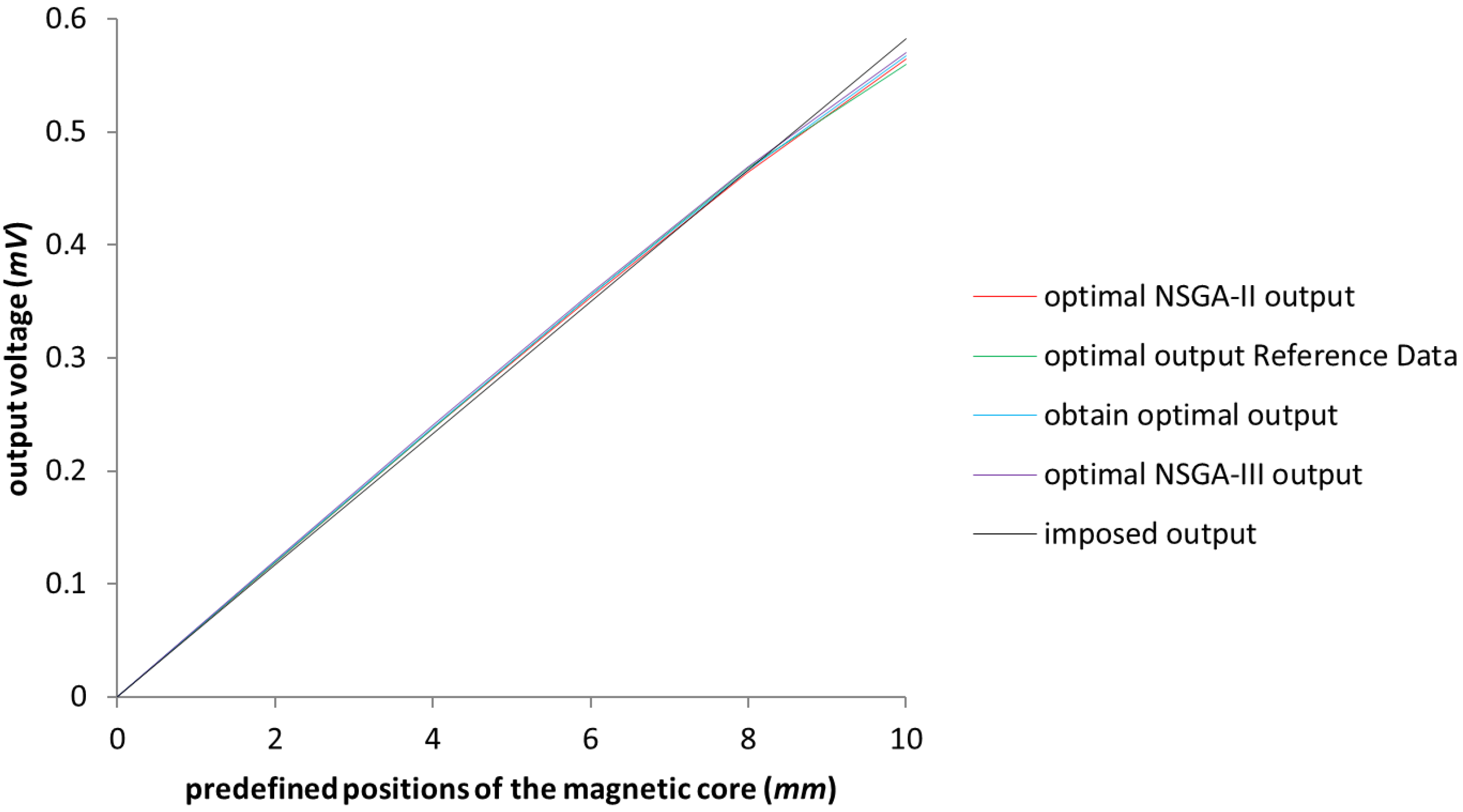
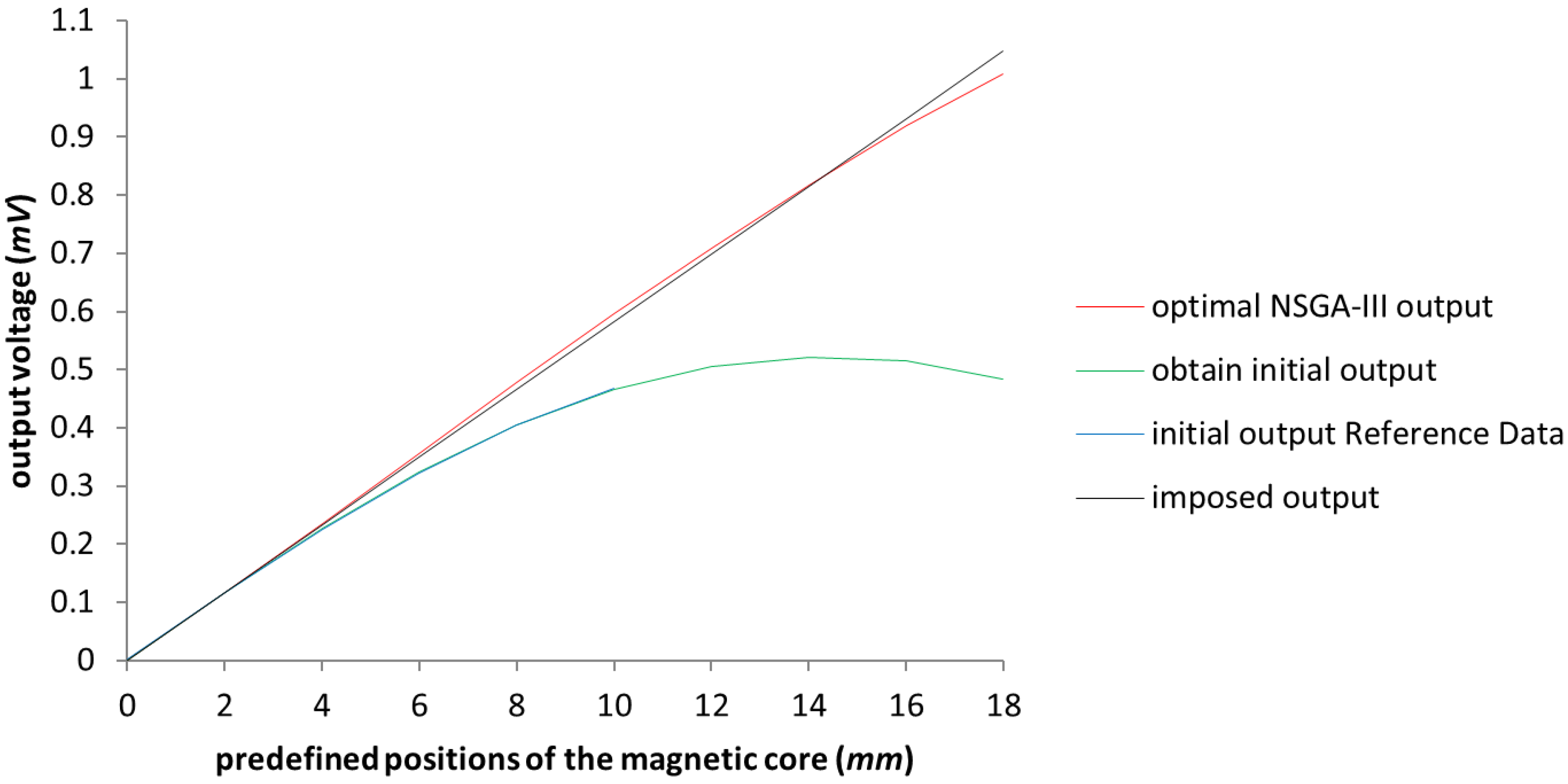
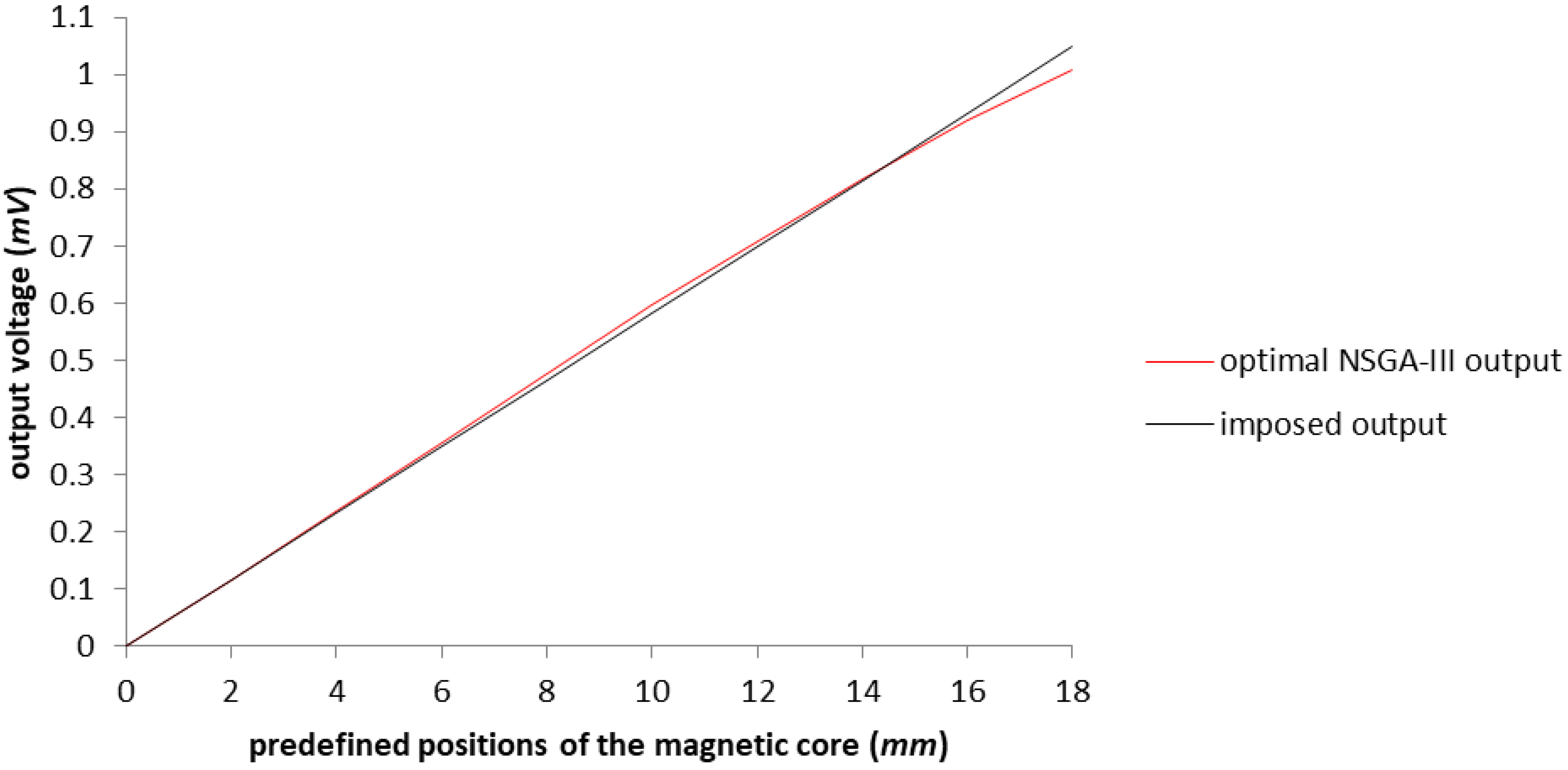
| (mm) | 0 | 2 | 4 | 6 | 8 | 10 | 12 | 14 | 16 | 18 |
|---|---|---|---|---|---|---|---|---|---|---|
| Linear val (mV) | 0 | 0.1164 | 0.2329 | 0.3493 | 0.4658 | 0.5822 | 0.6987 | 0.8151 | 0.9316 | 1.0479 |
| Original output in [3] (mV) | 0 | 0.1164 | 0.225 | 0.3215 | 0.404 | 0.4673 | No data | No data | No data | No data |
| Optimal output in [3] (mV) | 0 | 0.1183 | 0.2375 | 0.3555 | 0.4679 | 0.5596 | No data | No data | No data | No data |
| Obtain val (mV) | 0 | 0.01167 | 0.2264 | 0.3233 | 0.4041 | 0.4656 | 0.5056 | 0.5218 | 0.5152 | 0.4847 |
| NSGA-II val (mV) | 0 | 0.1139 | 0.2299 | 0.3467 | 0.4675 | 0.5866 | 0.7039 | 0.8188 | 0.9281 | 1.0361 |
| NSGA-III val (mV) | 0 | 0.1160 | 0.2354 | 0.3549 | 0.4770 | 0.5968 | 0.7089 | 0.8171 | 0.9191 | 1.0074 |
| Initial rel. er (%) | 0 | 0.2975 | 2.8902 | 8.0255 | 15.268 | 25.049 | 38.187 | 56.225 | 80.796 | 116.23 |
| Rel.er. NSGA-II (%) | 0 | 2.2208 | 1.2641 | 0.7458 | 0.3620 | 0.7536 | 0.7491 | 0.4509 | 0.3721 | 1.1442 |
| Rel.er. NSGA-III (%) | 0 | 0.3270 | 1.0766 | 1.5950 | 2.3495 | 2.4415 | 1.4515 | 0.2427 | 1.3585 | 4.0294 |
| Parameters | Initial Model | NSGA-II | NSGA-III |
|---|---|---|---|
| p₁ (mm) | 15 | 7.69 | 8.06 |
| p₂ (mm) | 20 | 14.87 | 16.56 |
| p₃ (mm) | 2.25 | 4.90 | 5.53 |
| p₄ (mm) | 4.50 | 31.24 | 30.76 |
| p₅ (mm) | 4.50 | 4.76 | 1.62 |
| p₆ (mm) | 26.50 | 1.61 | 2.62 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mociran, B.; Gliga, M. Optimization of an Inductive Displacement Transducer. Sensors 2023, 23, 8152. https://doi.org/10.3390/s23198152
Mociran B, Gliga M. Optimization of an Inductive Displacement Transducer. Sensors. 2023; 23(19):8152. https://doi.org/10.3390/s23198152
Chicago/Turabian StyleMociran, Bogdan, and Marian Gliga. 2023. "Optimization of an Inductive Displacement Transducer" Sensors 23, no. 19: 8152. https://doi.org/10.3390/s23198152
APA StyleMociran, B., & Gliga, M. (2023). Optimization of an Inductive Displacement Transducer. Sensors, 23(19), 8152. https://doi.org/10.3390/s23198152








