Energy Harvesting System Whose Potential Is Mapped with the Modified Fibonacci Function
Abstract
1. Introduction
2. Mathematical Model Formulation
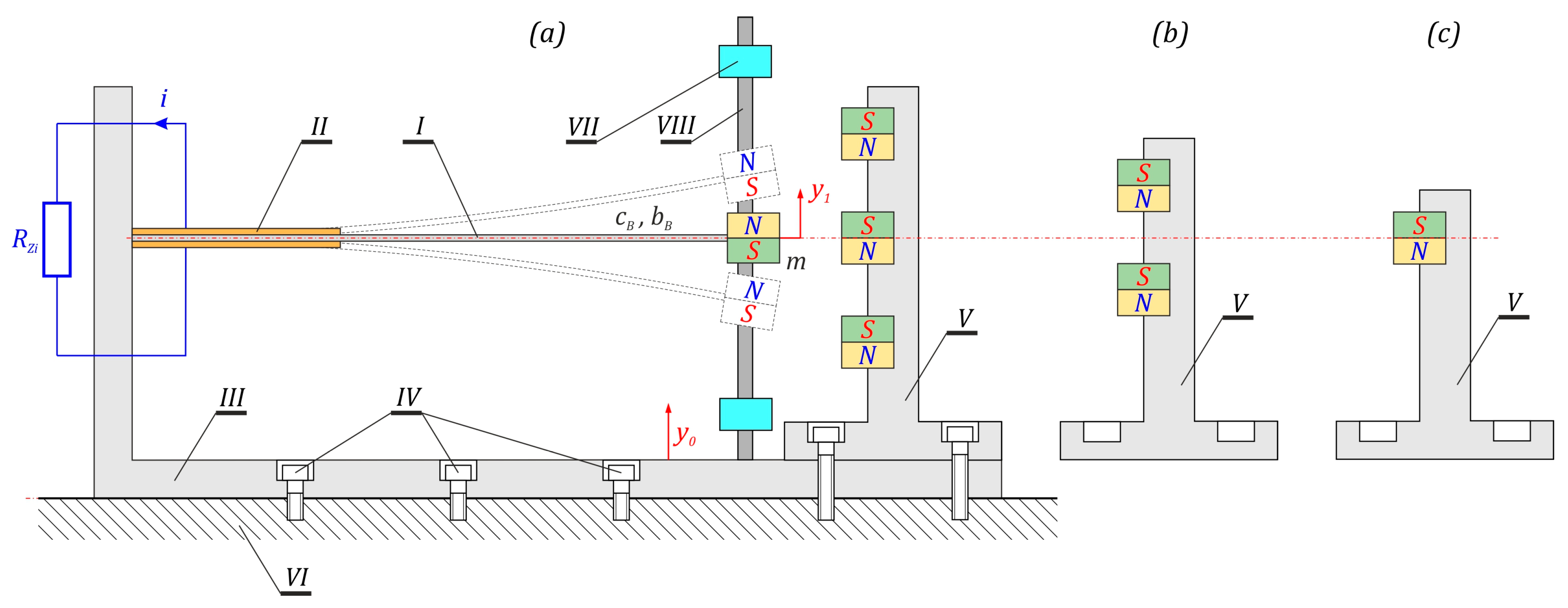
2.1. Model of the Flexible Vibration Limiter
- , φ ≈ 1.61803—golden ratio;
- —parameter defining the variable stiffness of the bumpers.
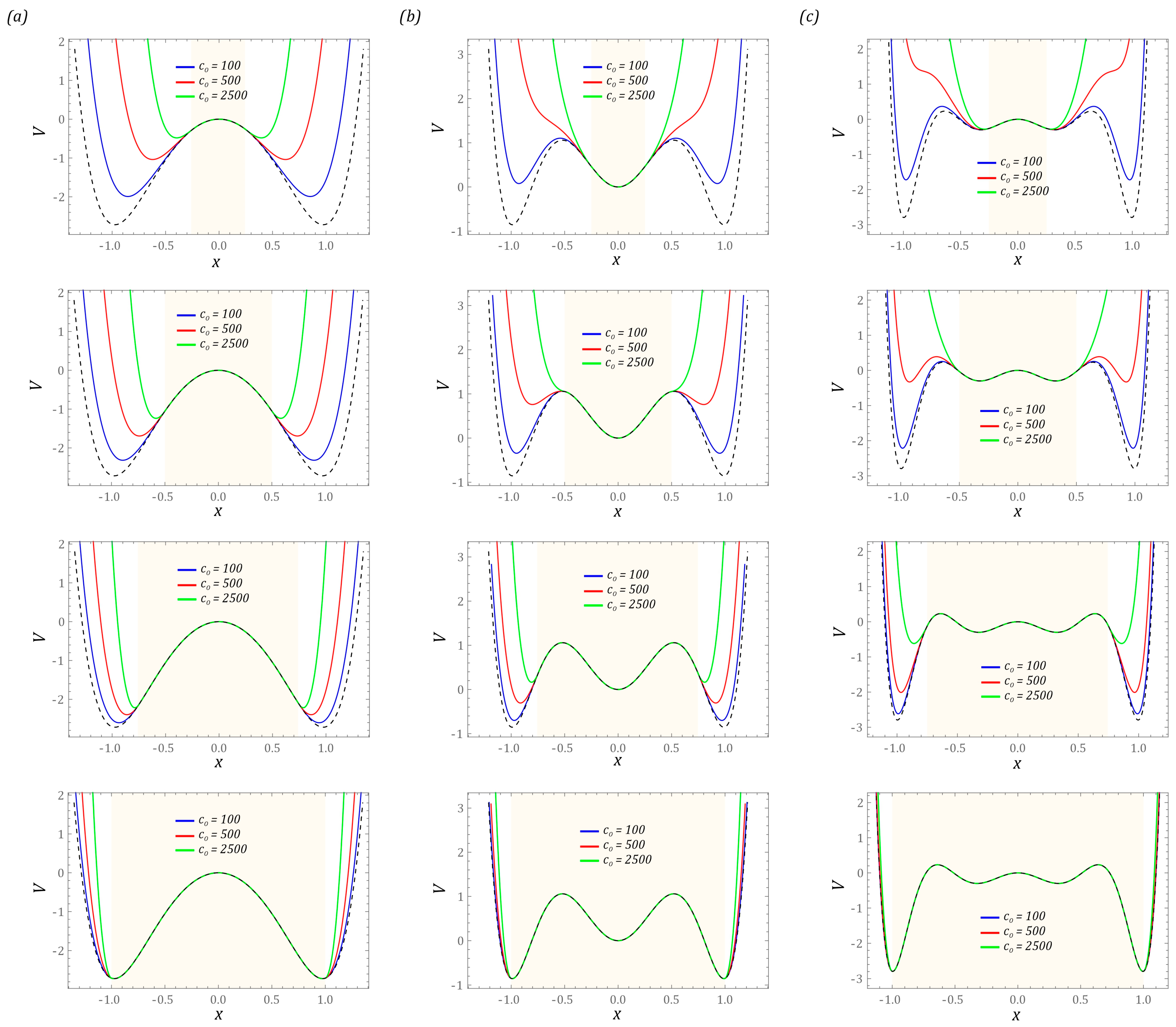
2.2. Dimensionless Mathematical Model of Energy Harvesting Systems
3. Results of Numerical Calculations
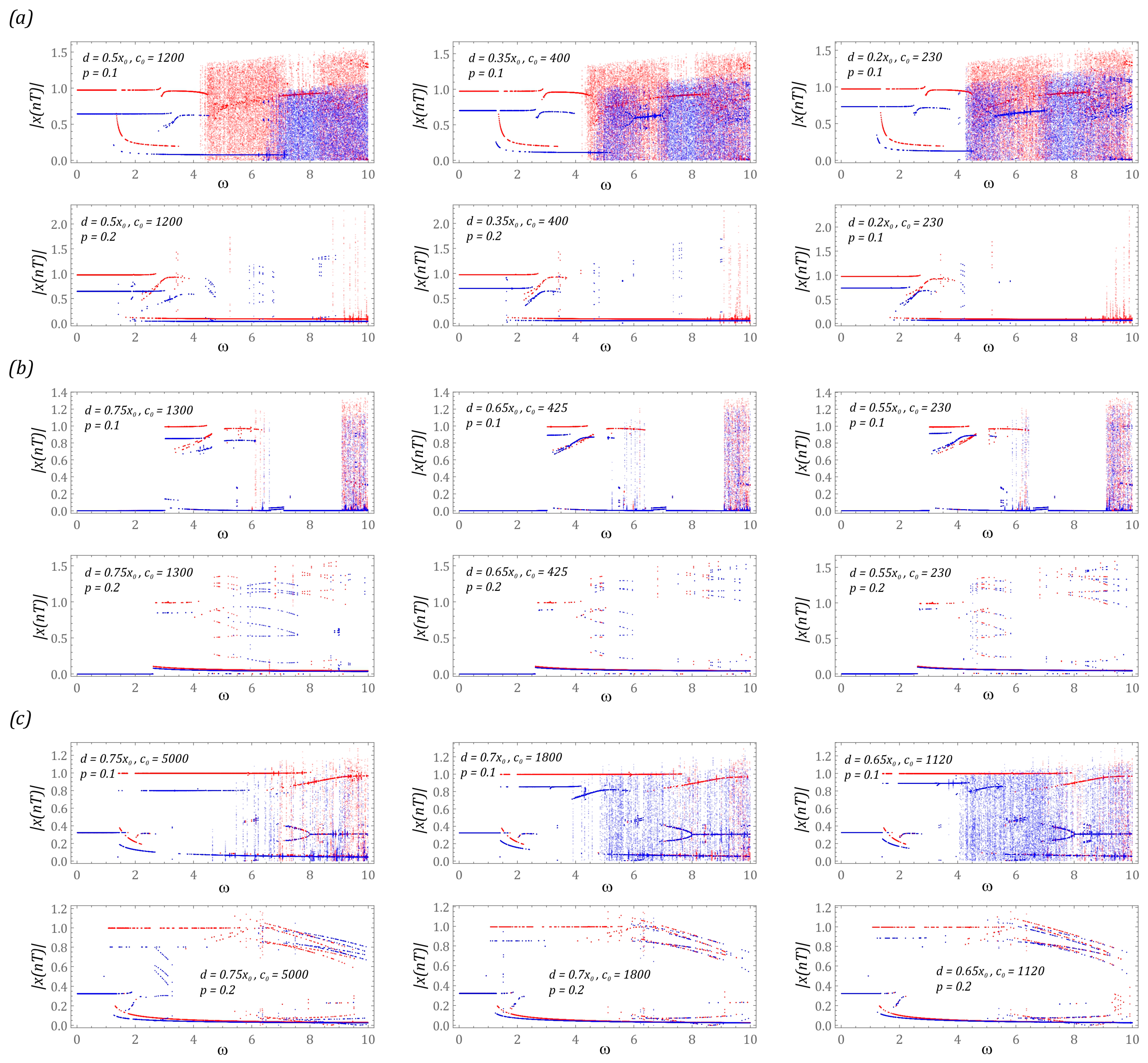
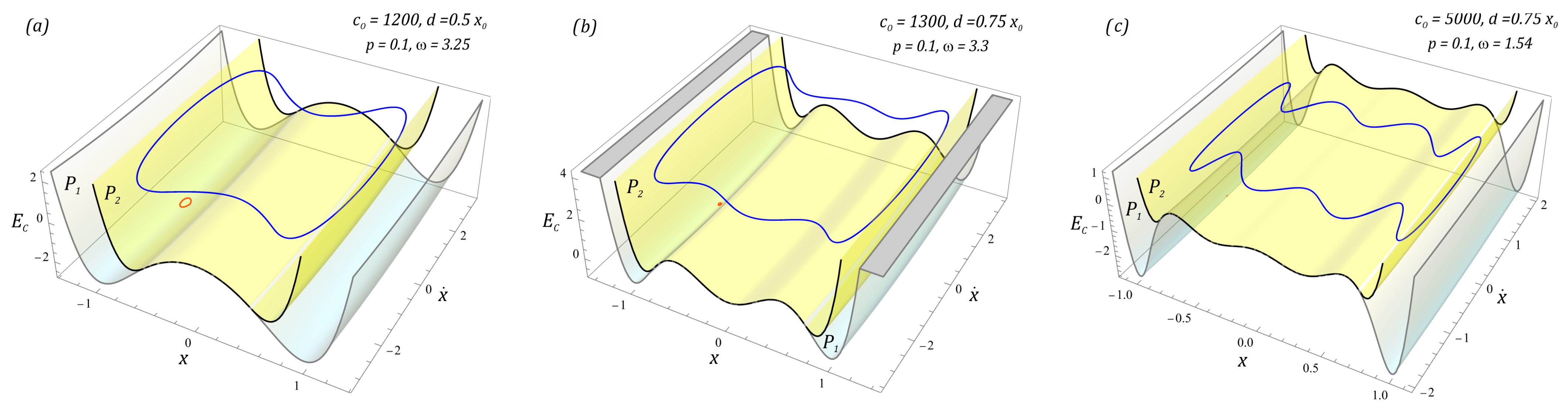
3.1. Periodicity of Solutions and Transient Chaos
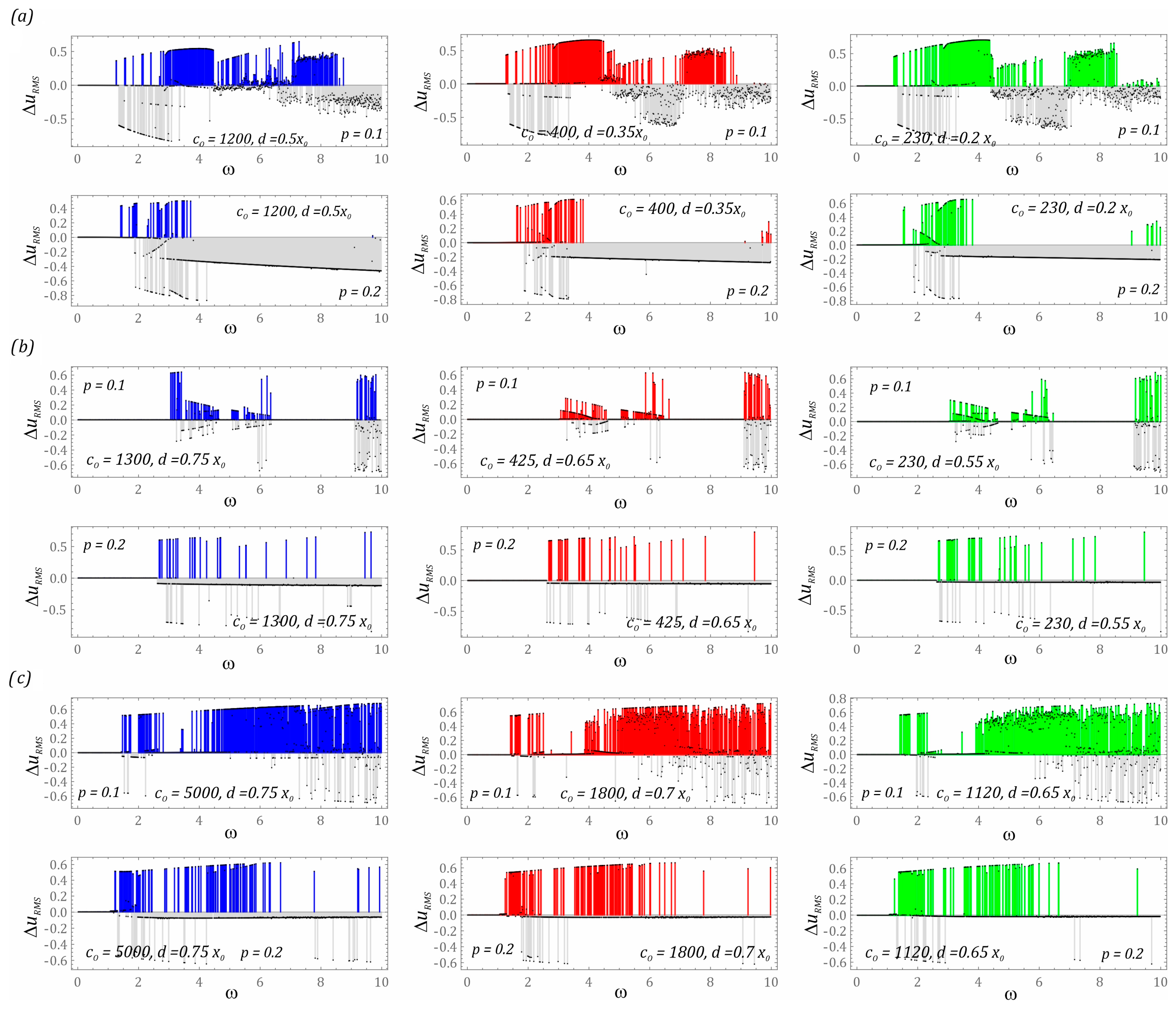
3.2. Effectiveness of Energy Harvesting
4. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, S.; Lallart, M.; Erturk, A. Multistable vibration energy harvesters: Principle, progress, and perspectives. J. Sound Vib. 2022, 528, 116886. [Google Scholar] [CrossRef]
- Lallart, M.; Zhou, S.; Yang, Z.; Yan, L.; Li, K.; Chen, Y. Coupling mechanical and electrical nonlinearities: The effect of synchronized discharging on tristable energy harvesters. Appl. Energy 2020, 266, 114516. [Google Scholar] [CrossRef]
- Hadaš, Z.; Janak, L.; Smilek, J. Virtual prototypes of energy harvesting systems for industrial applications. Mech. Syst. Signal. Process. 2018, 110, 152–164. [Google Scholar] [CrossRef]
- Caban, J.; Vrabel, J.; Górnicka, D.; Nowak, R.; Jankiewicz, M.; Matijosius, J.; Palka, M. Overview of Energy Harvesting Technologies Used in Road Vehicles. Energies 2023, 16, 3787. [Google Scholar] [CrossRef]
- Ye, J.; Ding, G.; Wu, X.; Zhou, M.; Wang, J.; Chen, Y.; Yu, Y. Development and performance research of PSN-PZT piezoelectric ceramics based on road vibration energy harvesting technology. Mater. Today Commun. 2023, 34, 105135. [Google Scholar] [CrossRef]
- Zhang, X.; Pan, H.; Qi, L.; Zhang, Z.; Yuan, Y.; Lui, Y. A renewable energy harvesting system using a mechanical vibration rectifier (MVR) for railroads. Appl. Energy 2017, 204, 1535–1543. [Google Scholar] [CrossRef]
- Sodano, H.A.; Inman, D.J.; Park, G. A review of power harvesting from vibration using piezoelectric materials. Shock Vibrat. Dig. 2004, 36, 197–206. [Google Scholar] [CrossRef]
- Beeby, S.P.; Tudor, M.J.; White, N.M. Energy harvesting vibration sources for microsystems applications. Meas. Sci. Technol. 2006, 17, R175. [Google Scholar] [CrossRef]
- Priya, S. Advances in energy harvesting using low profile piezoelectric transducers. J. Electroceram. 2007, 19, 167–184. [Google Scholar] [CrossRef]
- Daqaq, M.F.; Masana, R.; Erturk, A.; Quinn, D.D. On the role of nonlinearities in vibratory energy harvesting: A critical review and discussion. Appl. Mech. Rev. 2014, 66, 040801. [Google Scholar] [CrossRef]
- Ma, X.; Li, H.; Zhou, S.; Yang, Z.; Litak, G. Characterizing nonlinear characteristics of asymmetric tristable energy harvesters. Mech. Syst. Signal Process. 2022, 168, 108612. [Google Scholar] [CrossRef]
- Giri, A.M.; Ali, S.F.; Arockiarajan, A. Dynamics of symmetric and asymmetric potential well-based piezoelectric harvesters: A comprehensive review. J. Intell. Mater. Syst. Struct. 2020, 32, 1881–1947. [Google Scholar] [CrossRef]
- Gu, L.; Livemore, C. Impact-driven, frequency up-converting coupled vibration energy harvesting device for low frequency operation. Smart Mater. Struct. 2011, 20, 045004. [Google Scholar] [CrossRef]
- Le, C.P.; Halvorsen, E.; Sorasen, O.; Yeatman, E.M. Microscale electrostatic energy harvester using internal impacts. J. Intell. Mater. Syst. Struct. 2012, 23, 1409. [Google Scholar] [CrossRef]
- Soliman, M.S.M.; Abdel-Rahman, E.M.; El-Saadany, E.F.; Mansour, R.R. A wideband vibration-based energy harvester. J. Micromech. Microeng. 2008, 18, 115021. [Google Scholar] [CrossRef]
- Nowak, R.; Pietrzakowski, M. Experimental and Simulation Investigations of the Cantilever Beam Energy Harvester. Solid State Phenom. 2016, 248, 249–255. [Google Scholar] [CrossRef]
- Shahruz, S.M. Design of mechanical band-pass filters for energy scavenging. J. Sound Vib. 2006, 292, 987–998. [Google Scholar] [CrossRef]
- Nowak, R.; Pietrzakowski, M.; Rumianek, P. Influence of design parameters on bending piezoelectric harvester effectiveness: Static approach. Mech. Syst. Signal Process. 2020, 143, 106833. [Google Scholar] [CrossRef]
- Tang, L.; Yang, Y. A multiple-degree-of-freedom piezoelectric energy harvesting model. J. Intell. Mater. Syst. Struct. 2012, 23, 1631–1647. [Google Scholar] [CrossRef]
- Rajarathinam, M.; Aravindan, M.; Vinothkrishnan, V.; Ali, S.F. Coupled piezo-multiple electromagnetic energy harvesting. Mech. Adv. Mater. Struc. 2022, 1–20. [Google Scholar] [CrossRef]
- Ahmad, M.M.; Khan, N.M.; Khan, F.U. Multi-degrees of freedom energy harvesting for broad-band vibration frequency range: A review. Sens. Actuators A Phys. 2022, 344, 113690. [Google Scholar] [CrossRef]
- Liu, X.; He, L.; Liu, R.; Hu, D.; Zhang, L.; Cheng, G. Piezoelectric energy harvesting systems using mechanical tuning techniques featured. Rev. Sci. Instrum. 2023, 94, 031501. [Google Scholar] [CrossRef]
- Erturk, A.; Hoffmann, J.; Inman, D.J. A piezomagnetoelastic structure for broadband vibration energy harvesting. Appl. Phys. Lett. 2009, 94, 254102. [Google Scholar] [CrossRef]
- Cottone, F.; Vocca, H.; Gammaitoni, L. Nonlinear energy harvesting. Phys. Rev. Lett. 2009, 102, 080601. [Google Scholar] [CrossRef] [PubMed]
- Rubes, O.; Chalupa, J.; Ksica, F.; Hadas, Z. Development and experimental validation of self-powered wireless vibration sensor node using vibration energy harvester. Mech. Syst. Signal Process. 2021, 160, 107890. [Google Scholar] [CrossRef]
- Huguet, T.; Badel, A.; Druet, O.; Lallart, M. Drastic bandwidth enhancement of bistable energy harvesters: Study of subharmonic behaviors and their stability robustness. Appl. Energy 2018, 226, 607–617. [Google Scholar] [CrossRef]
- Morel, A.; Brenes, A.; Gibus, D.; Lefeuvre, E.; Gasnier, P.; Pillonnet, G.; Badel, A. A comparative study of electrical interfaces for tunable piezoelectric vibration energy harvesting. Smart Mater. Struct. 2022, 31, 045016. [Google Scholar] [CrossRef]
- Mohanty, A.; Parida, S.; Behera, R.K.; Roy, T. Vibration energy harvesting: A review. J. Adv. Dielectr. 2019, 9, 1930001. [Google Scholar] [CrossRef]
- Zhou, S.; Cao, J.; Inman, D.J.; Lin, J.; Liu, S.; Wang, Z. Broadband tristable energy harvester: Modeling and experiment verification. Appl. Energy 2014, 133, 33–39. [Google Scholar] [CrossRef]
- Fang, S.; Zhou, S.; Yurchenko, D.; Yang, T.; Liao, W.H. Multistability phenomenon in signal processing, energy harvesting, composite structures, and metamaterials: A review. Mech. Syst. Signal Process. 2022, 166, 108419. [Google Scholar] [CrossRef]
- Margielewicz, J.; Gąska, D.; Litak, G.; Wolszczak, P.; Yurchenko, D. Nonlinear dynamics of a new energy harvesting system with quasi-zero stiffness. Appl. Energy 2022, 307, 118159. [Google Scholar] [CrossRef]
- Huguet, T.; Lallart, M.; Badel, A. Orbit jump in bistable energy harvesters through buckling level modification. Mech. Syst. Signal Process. 2019, 128, 202–215. [Google Scholar] [CrossRef]
- Sosna, P.; Rubeš, O.; Hadaš, Z. Verification and analysis of advanced tuneable nonlinear vibration energy harvester. Mech. Syst. Signal Process. 2023, 189, 110096. [Google Scholar] [CrossRef]
- Maamer, B.; Boughamoura, A.; Fath El-Bab, A.M.R.; Francis, L.A.; Tounsi, F. A Review on Design Improvements and Techniques for Mechanical Energy Harvesting Using Piezoelectric and Electromagnetic Schemes. Energy Convers. Manag. 2019, 199, 111973. [Google Scholar] [CrossRef]
- Giusa, F.; Giuffrida, A.; Trigona, C.; Andò, B.; Bulsara, A.R.; Baglio, S. “Random Mechanical Switching Harvesting on Inductor”: A Novel Approach to Collect and Store Energy from Weak Random Vibrations with Zero Voltage Threshold. Sens. Actuators A Phys. 2013, 198, 35–45. [Google Scholar] [CrossRef]
- Covaci, C.; Gontean, A. Piezoelectric Energy Harvesting Solutions: A Review. Sensors 2020, 20, 3512. [Google Scholar] [CrossRef]
- Liu, H.; Lee, C.; Kobayashi, T.; Tay, C.J.; Quan, C. Investigation of a MEMS Piezoelectric Energy Harvester System with a Frequency-Widened-Bandwidth Mechanism Introduced by Mechanical Stoppers. Smart Mater. Struct. 2012, 21, 035005. [Google Scholar] [CrossRef]
- Liu, S.; Cheng, Q.; Zhao, D.; Feng, L. Theoretical Modeling and Analysis of Two-Degree-of-Freedom Piezoelectric Energy Harvester with Stopper. Sens. Actuators A Phys. 2016, 245, 97–105. [Google Scholar] [CrossRef]
- Hu, G.; Tang, L.; Das, R.; Marzocca, P. A Two-Degree-of-Freedom Piezoelectric Energy Harvester with Stoppers for Achieving Enhanced Performance. Int. J. Mech. Sci. 2018, 149, 500–507. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, Q.; Wang, W. Low-Frequency Wideband Vibration Energy Harvesting by Using Frequency up-Conversion and Quin-Stable Nonlinearity. J. Sound Vib. 2017, 399, 169–181. [Google Scholar] [CrossRef]
- Fan, Y.; Ghayesh, M.H.; Lu, T.F. Enhanced Nonlinear Energy Harvesting Using Combined Primary and Parametric Resonances: Experiments with Theoretical Verifications. Energy Convers. Manag. 2020, 221, 113061. [Google Scholar] [CrossRef]
- Liu, W.; Liu, C.; Ren, B.; Zhu, Q.; Hu, G.; Yang, W. Bandwidth Increasing Mechanism by Introducing a Curve Fixture to the Cantilever Generator. Appl. Phys. Lett. 2016, 109, 043905. [Google Scholar] [CrossRef]
- Salazar, R.; Serrano, M.; Abdelkefi, A. Fatigue in piezoelectric ceramic vibrational energy harvesting: A review. Appl. Energy 2020, 270, 115161. [Google Scholar] [CrossRef]
- Junak, G. Low-cycle fatigue characteristics of selected titanium, magnesium and aluminium alloys. Arch. Metall. Mater. 2018, 63, 1949–1955. [Google Scholar] [CrossRef]
- Sedighi, H.M.; Shirazi, K.H.; Zare, J. Novel equivalent function for deadzone nonlinearity: Applied to analytical solution of beam vibration using He’s Parameter Expanding Method. Lat. Am. J. Solids Struct. 2012, 9, 443–451. [Google Scholar] [CrossRef]
- Margielewicz, J.; Gąska, D.; Litak, G. Modelling of the gear backlash. Nonlinear Dyn. 2019, 97, 355–368. [Google Scholar] [CrossRef]
- Jiang, D.M. Application of Fibonacci sine and cosine function to a nonlinear differential–difference equation. Appl. Math. Comput. 2010, 217, 3898–3902. [Google Scholar] [CrossRef]
- Biban, G.; Chugh, R.; Panwar, A. Image encryption based on 8D hyperchaotic system using Fibonacci Q-Matrix. Chaos Solitons Fractals 2023, 170, 113396. [Google Scholar] [CrossRef]
- Ham, S.; Roh, M.; Lee, H.; Ha, S. Multibody dynamic analysis of a heavy load suspended by a floating crane with constraint-based wire rope. Ocean Eng. 2015, 109, 145–160. [Google Scholar] [CrossRef]
- Karnopp, D.C.; Margolis, D.L.; Rosenberg, R.C. System Dynamics: Modelling and Simulation of Mechatronic Systems, 5th ed.; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Geitner, G.H.; Komurgoz, G. Generic power split modelling for compound epicyclic four-speed gears. Mech. Mach. Theory 2017, 116, 50–68. [Google Scholar] [CrossRef]







| Name | Symbol | Value | ||
|---|---|---|---|---|
| Inertial element (mass) loading the beam | m | 0.01 kg | ||
| Energy losses in the cantilever system | bB | 0.02 Nsm−1 | ||
| Cantilever beam stiffness | cB | 15 Nm−1 | ||
| Scaling parameters | x0 | 0.0253 m | 0.03 m | 0.0307 m |
| Physical parameters defining potential barriers | c1 c2 c3 c4 | 144 Nm−1 35 × 107 Nm−5 | 243 Nm−1 1.35 × 106 Nm−3 1.2 × 109 Nm−5 | 210 Nm−1 2.7 × 106 Nm−3 7.8 × 109 Nm−5 5496 × 109 Nm−7 |
| Load resistance | Rp | 1.1 MΩ | ||
| Piezoelectric capacity | CP | 72 nF | ||
| Electromechanical constant of piezoelectric converter | kP | 3.985 × 10−5 NV−1 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Margielewicz, J.; Gąska, D.; Litak, G.; Caban, J.; Dudziak, A.; Ma, X.; Zhou, S. Energy Harvesting System Whose Potential Is Mapped with the Modified Fibonacci Function. Sensors 2023, 23, 6593. https://doi.org/10.3390/s23146593
Margielewicz J, Gąska D, Litak G, Caban J, Dudziak A, Ma X, Zhou S. Energy Harvesting System Whose Potential Is Mapped with the Modified Fibonacci Function. Sensors. 2023; 23(14):6593. https://doi.org/10.3390/s23146593
Chicago/Turabian StyleMargielewicz, Jerzy, Damian Gąska, Grzegorz Litak, Jacek Caban, Agnieszka Dudziak, Xiaoqing Ma, and Shengxi Zhou. 2023. "Energy Harvesting System Whose Potential Is Mapped with the Modified Fibonacci Function" Sensors 23, no. 14: 6593. https://doi.org/10.3390/s23146593
APA StyleMargielewicz, J., Gąska, D., Litak, G., Caban, J., Dudziak, A., Ma, X., & Zhou, S. (2023). Energy Harvesting System Whose Potential Is Mapped with the Modified Fibonacci Function. Sensors, 23(14), 6593. https://doi.org/10.3390/s23146593








