Detection and Identification for Void of Concrete Structure by Air-Coupled Impact-Echo Method
Abstract
1. Introduction
2. Theory
2.1. Theory of Void Damage Model
2.2. Acoustic Modal Theory
2.3. Quantitative Index
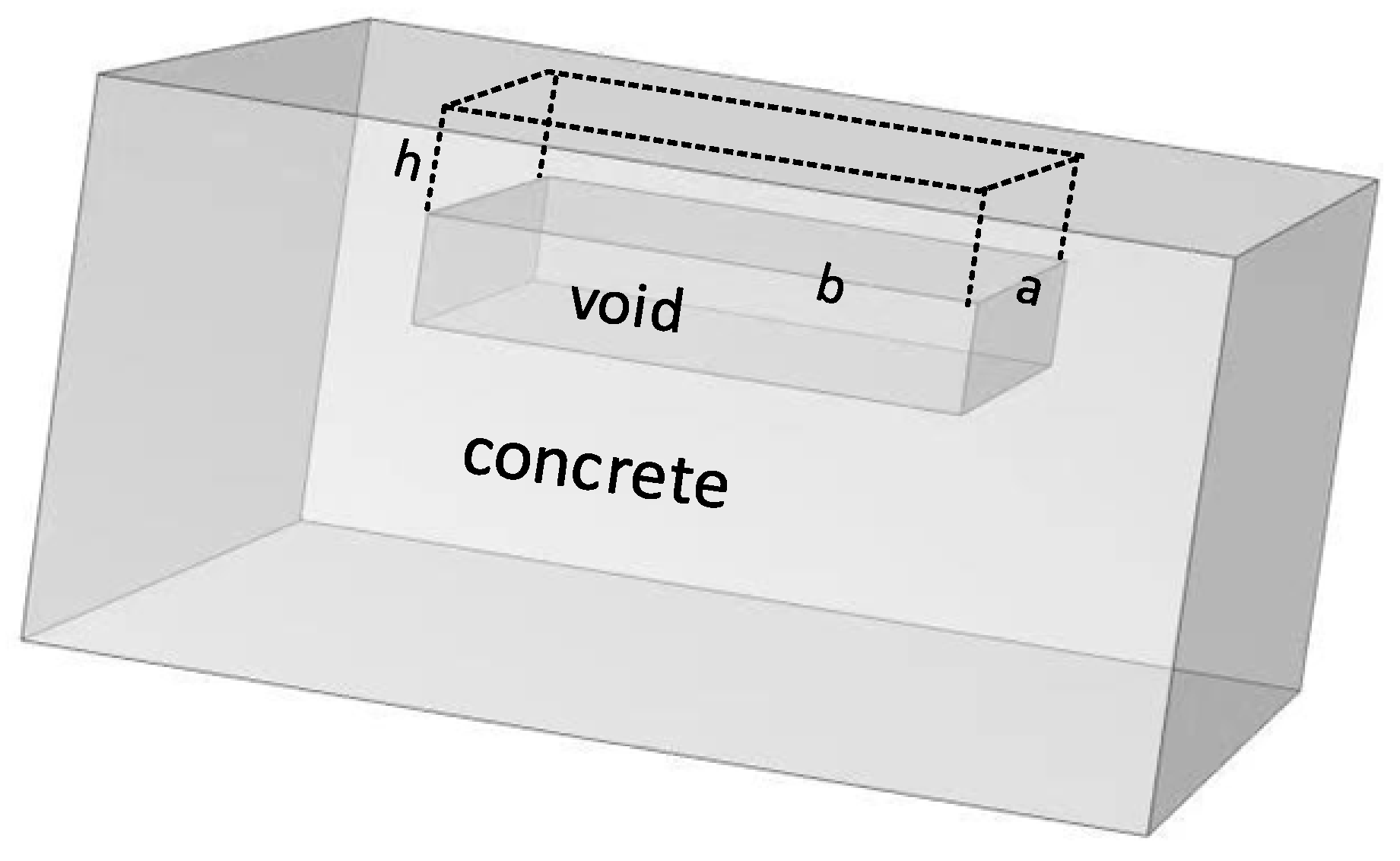
3. Finite Element Model (FEM) for Concrete Void
3.1. Parameters of FEM
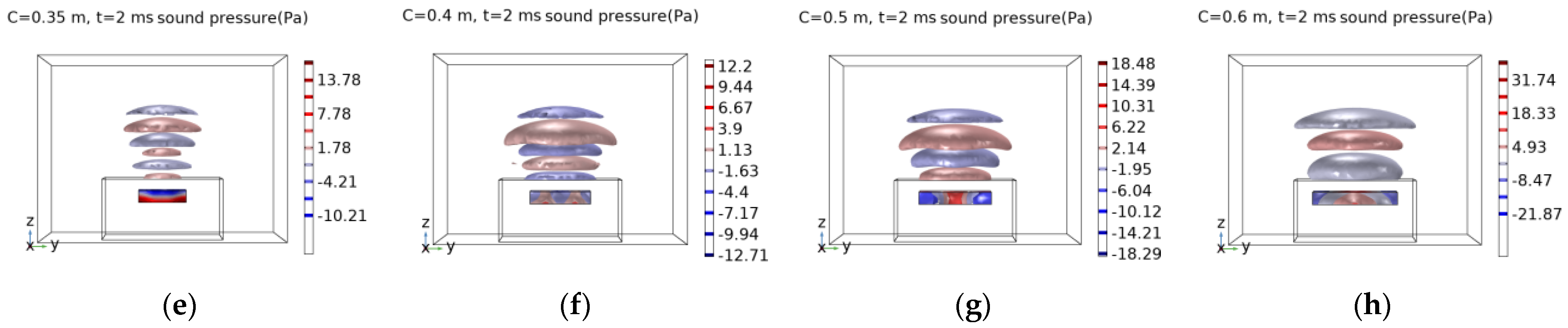
3.2. Sound Field Analysis of the FEM
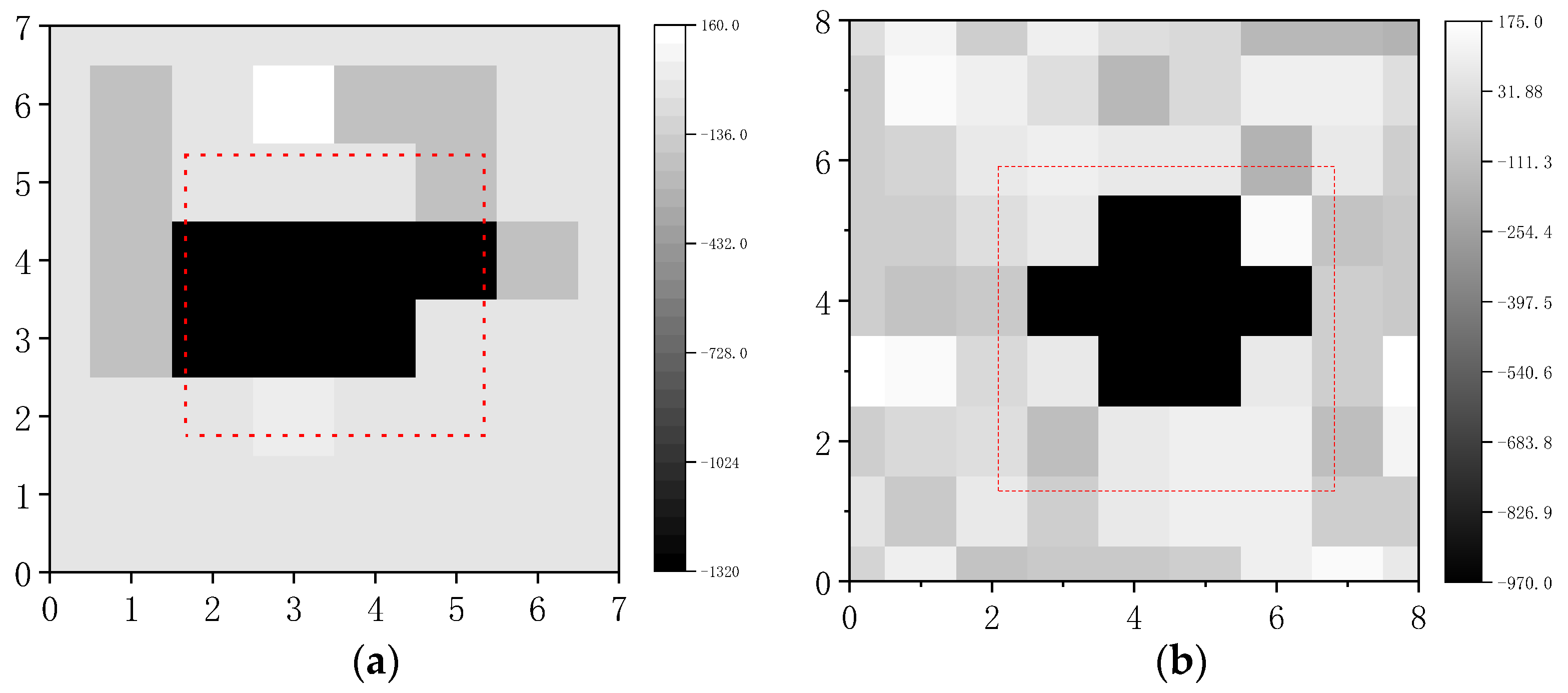
3.2.1. Analysis for the Influence of Width on Sound Field
3.2.2. Analysis for the Influence of Depth on Sound Field
3.3. Frequency-Domain Analysis of the FEM
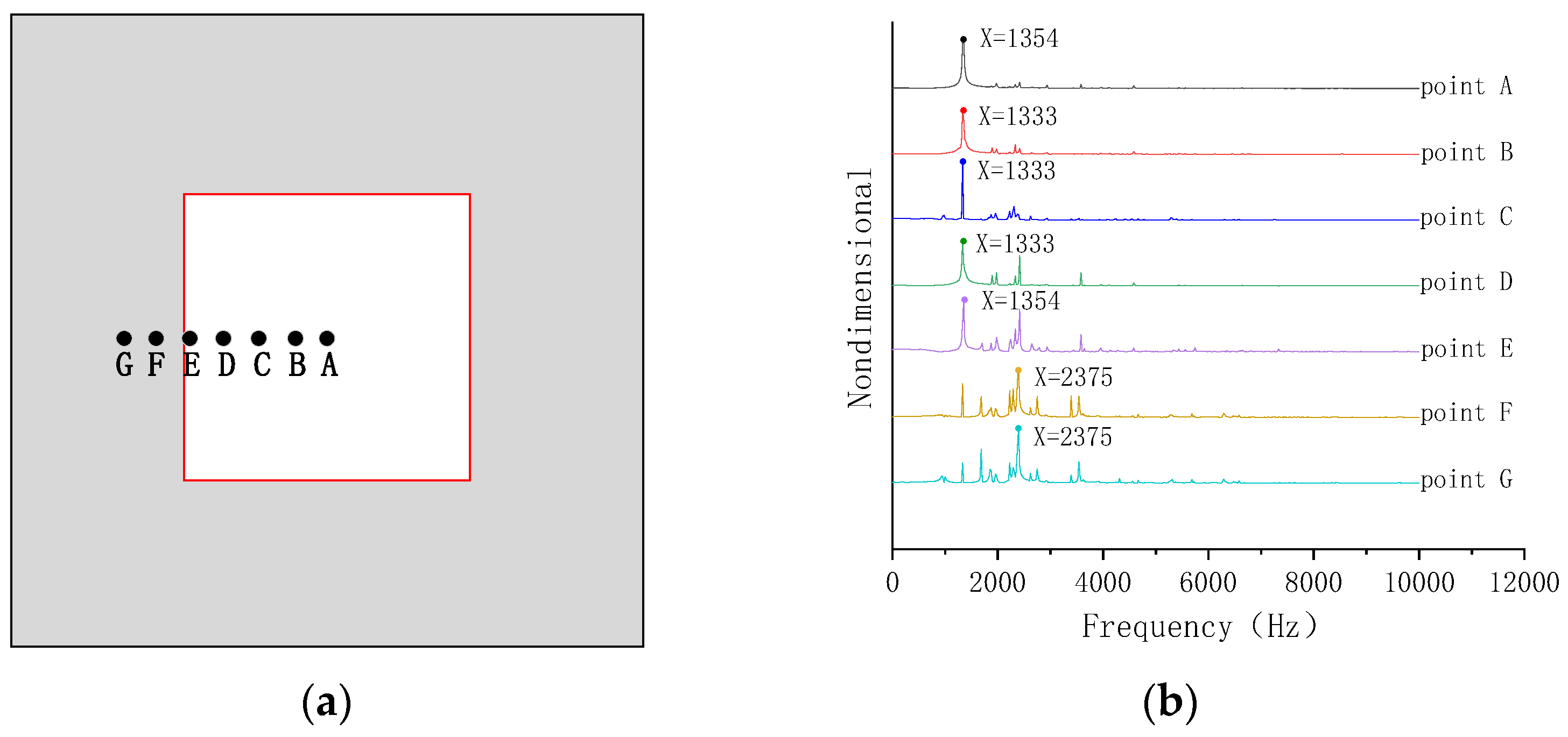
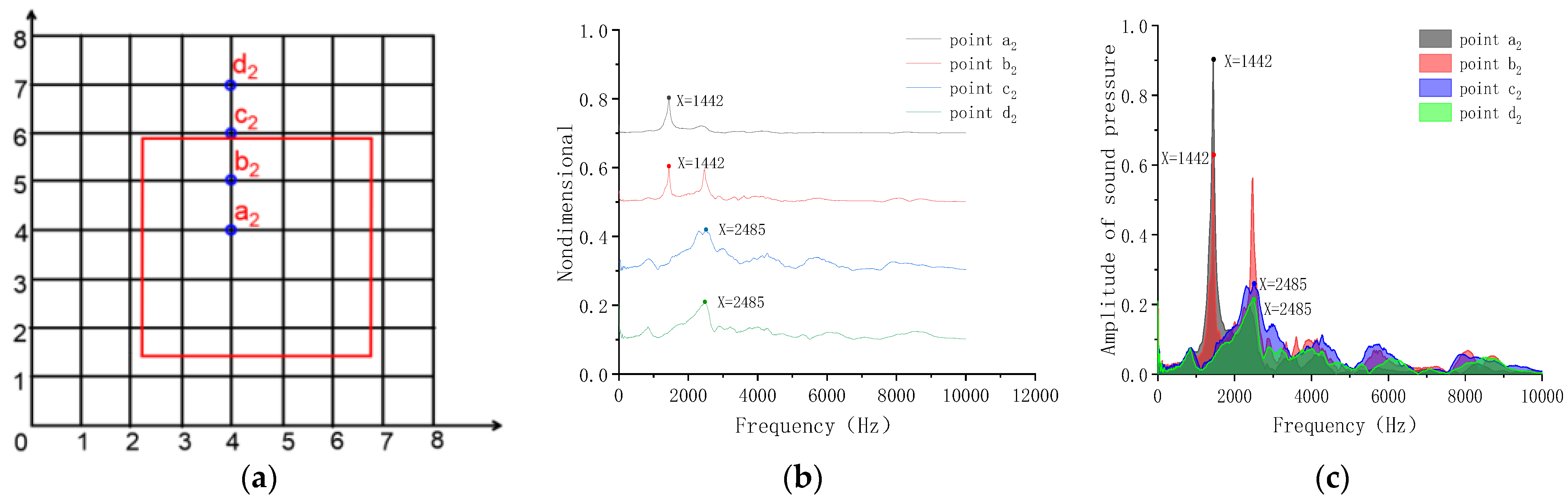
3.3.1. The Influence of Different Excitation Positions
3.3.2. The Influence of Different Void Sizes
4. Experimental Verification
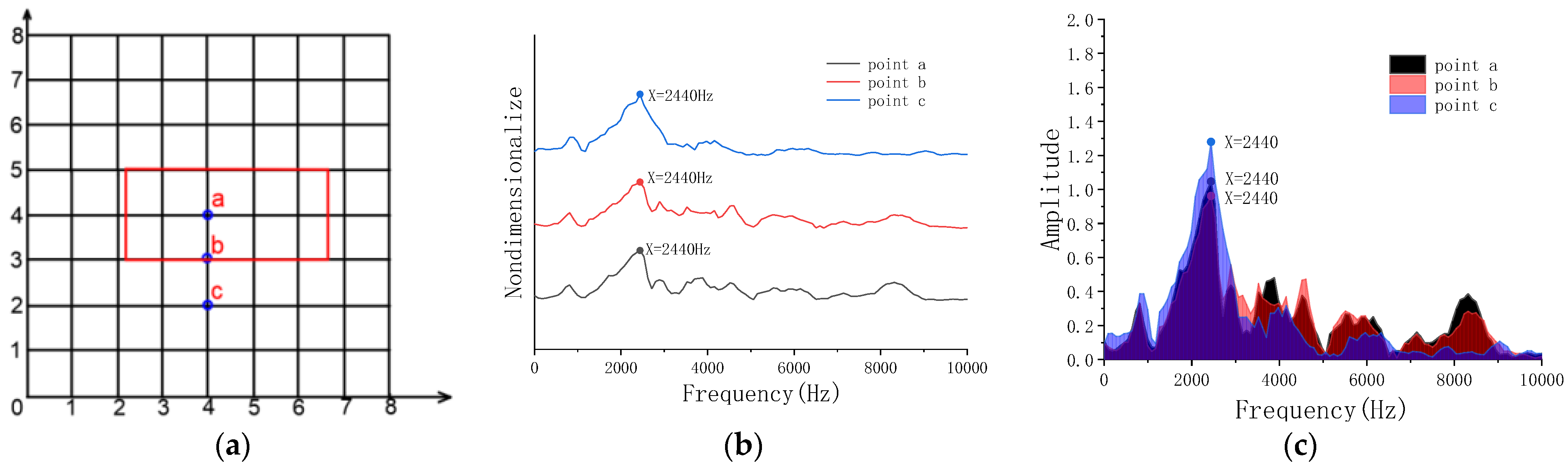
4.1. Experiment
4.2. Frequency-Domain Analysis of Experiment
5. Result Analysis and the Identification Method
5.1. Result Analysis of Experiment and FEM
5.2. The Method and Effect of Identification
6. Discussion
7. Conclusions
- (1)
- In this paper, numerical simulation and experiments are described for a void depth of less than 0.4 m. The results show that, compared with the void depth, the influence of the width on peak frequency increases significantly. When the void width is greater than 0.30 m, the peak frequency decreases with the increase in void width, and the change is obvious.
- (2)
- It is found that the acoustic peak frequency can effectively judge a concrete void depth of less than 0.4 m by numerical simulation. The method of peak frequency can be used identify a void with a width greater than 0.3 m in a concrete structure.
- (3)
- The main engineering value of this study is that the threshold value can be used to quickly judge whether there is a void in a concrete structure through single-point excitation. When multipoint scanning is used, the void range can be quickly estimated.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wei, X.; Zhang, Z. Analysis and nondestructive detection on diseases (defects) of high-speed railway ballastless track. Railw. Stand. Des. 2011, 3, 38–40. [Google Scholar]
- Kaewunruen, S.; AbdelHadi, M.; Kongpuang, M.; Pansuk, W.; Remennikov, A.M. Digital Twins for Managing Railway Bridge Maintenance, Resilience, and Climate Change Adaptation. Sensors 2023, 23, 252. [Google Scholar] [CrossRef]
- Xu, Q.; Zhang, Z.; Fang, Z.; Lou, P.; Lin, Q.; Li, W. Effects of uneven settlement of pier and abutment on dynamic characteristics of longitudinal connected slab ballastless track on bridge. J. Vib. Shock. 2019, 38, 112–118. [Google Scholar]
- Tian, X.; Du, Y.; Zhao, W. Detection and identification of mortar void in the ballastless track of high-speed railway based on transient impact characteristics. Vib. Shock. 2019, 38, 148–153. [Google Scholar]
- Yang, Y.; Lu, J.; Li, R.; Tian, X.; Zhao, W. Analysis on edge estimation of functional layer defect in ballastless track based on Burg power spectrum. Vib. Shock. 2020, 39, 1–6. [Google Scholar]
- Zhang, H.; Yu, Q.; Lv, Y. Application study of quality testing of the arch-rib concrete of concrete-filled steel tube arch bridge by ultrasonic transmission method. China Civ. Eng. J. 2004, 37, 50–53. [Google Scholar]
- Liu, Q.; Xiong, Z. Two methods of testing defect in concrete-filled steel tubular. J. Wuhan Univ. Technol. 2005, 27, 38–40. [Google Scholar]
- Ding, R. Research on fiber sensing of health monitoring for steel tube-confined concrete arch bridge. China Civ. Eng. J. 2005, 38, 69–74. [Google Scholar]
- Chen, T.; Zhao, W.; Li, R.; Yang, Y.; Tian, X. Study on Identification of CA Mortar Layer Void Based on Convolution Neural Network. Railw. Stand. Des. 2021, 65, 77–82. [Google Scholar]
- Leśnicki, K.J.; Kim, J.-Y.; Kurtis, K.E.; Jacobs, L.J. Characterization of ASR damage in concrete using nonlinear impact resonance acoustic spectroscopy technique. NDT E Int. 2011, 44, 721–727. [Google Scholar] [CrossRef]
- Bodnar, J.L.; Nicolas, J.L.; Candore, J.C.; Detalle, V. Non-destructive testing by infrared thermography under random excitation and ARMA analysis. Int. J. Thermophys. 2012, 33, 2011–2015. [Google Scholar] [CrossRef]
- Yang, Y.; Zhao, W.; Du, Y.; Zhang, H. Analysison directional featrure extraction for the target identification of shallow subsurface based on curvelet tranform. DYNA 2017, 92, 293–299. [Google Scholar] [CrossRef] [PubMed]
- Zhu, J.; Popovics, J.S. Air-coupled impact-echo method for ndt of concrete. Am. Inst. Phys. 2006, 820, 1351–1357. [Google Scholar]
- Oh, T.; Popovics, J.S.; Ham, S.; Shin, S.W. Improved interpretation of vibration responses from concrete delamination defects using air–coupled impact resonance tests. J. Eng. Mech. ASCE 2013, 139, 315–324. [Google Scholar] [CrossRef]
- Oh, T.; Popovics, J.S. Application of impact resonance c–scan stack images to evaluate bridge deck conditions. J. Infrastruct. Syst. 2015, 21, 1–8. [Google Scholar] [CrossRef]
- Oh, T.; Popovics, J.S. Practical visualization of local vibration data collected over large concrete elements. Comput.-Aided Civ. Infrastruct. Eng. 2015, 30, 68–81. [Google Scholar] [CrossRef]
- Sun, H.; Zhu, J.; Ham, S. Acoustic evaluation of concrete delaminations using ball–chain impact excitation. Acoust. Soc. Am. J. 2017, 141, EL477–EL481. [Google Scholar] [CrossRef]
- Zhang, G.; Harichandran, R.S.; Ramuhalli, P. Automatic delamination detection of concrete bridge decks using impact signals. J. Bridge Eng. 2012, 17, 951–954. [Google Scholar] [CrossRef]
- Liu, M. Diagnosis of Interface Debonding in Steel–Concrete Composite Structures Using Air–Coupled Impact Resonance Test. Master’s Thesis, Hunan University, Changsha, China, 2018. [Google Scholar]
- Shin, S.W.; Popovics, J.S.; Oh, T. Cost effective air-coupled impact-echo sensing for rapid detection of delamination damage in concrete structures. Adv. Struct. Eng. 2012, 15, 887–895. [Google Scholar] [CrossRef]
- Kim, G.; Kim, J.Y.; Kurtis, K.E.; Jacobs, L.J.; Le Pape, Y.; Guimaraes, M. Quantitative evaluation of carbonation in concrete using nonlinear ultrasound. Mater. Struct. 2016, 49, 399–409. [Google Scholar] [CrossRef]
- Dou, S.; He, L.; Zheng, J.; Wang, N. Application of Modal Analysis in Acoustic-vibration Detection of Tunnel Secondary Liner Void. J. Railw. Eng. Soc. 2018, 35, 52–57. [Google Scholar]
- Peng, Y.; Chen, J.; Pan, B.; Zhang, X. Application Study of Acoustic-vibration in Detection of Rigid Pavement Slab Cavern. J. Highw. Transp. Res. Dev. 2005, 22, 54–65. [Google Scholar]
- Warburton, G.B. The Vibration of Rectangular Plates; Institute of Mechanical Engineers: London, UK, 1954; pp. 371–384. [Google Scholar]
- Leissa, A.W. The free vibration of rectangular plates. J. Sound Vib. 1973, 31, 257–293. [Google Scholar] [CrossRef]
- Ventsel, E.S.; Krauthammer, T. Thin Plates and Shells: Theory, Analysis, and Application; Marcel Dekker, Inc.: New York, NY, USA, 2001. [Google Scholar]
- Mitchell, A.K.; Hazell, C.R. A simple frequency formula for clamped rectangular plates. J. Sound Vib. 1987, 118, 271–281. [Google Scholar] [CrossRef]
- Hazell, C.R.; Mitchell, A.K. Experimental eigenvalues and mode shapes for flat clamped plates. Exp. Mech. 1986, 26, 209–216. [Google Scholar] [CrossRef]
- Cheng, C.; Sansalone, M. The impact-echo response of concrete plates containing delaminations—Numerical, experimental and field studies. Mater. Struct. 1993, 26, 274–285. [Google Scholar] [CrossRef]
- Zhao, S. Research on Delamination Detection in Concrete Bridge Decks Using Impact-Echo Method. Master’s Thesis, Dalian University of Technology, Dalian, China, 2021. [Google Scholar]






| Materials | Velocity of Sound (m/s) | Density (kg/m3) | Elastic Modulus (Pa) | Poisson Ratio |
|---|---|---|---|---|
| Concrete | 4000 | 2500 | 3.0 × 1010 | 0.2 |
| Air | 343 | / | / | / |
| f | Theory | FEM | Experiment |
|---|---|---|---|
| Model B | 1862 Hz | 1892 Hz | 1860 Hz |
| Model C | 1551 Hz | 1354 Hz | 1442 Hz |
| Δf (Hz) | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 2 | 0 | 0 | 0 | 20 | 0 | 0 | 0 | 0 |
| 3 | 0 | −220 | −1320 | −1320 | −1320 | 0 | 0 | 0 |
| 4 | 0 | −220 | −1320 | −1320 | −1320 | −1320 | −200 | 0 |
| 5 | 0 | −220 | 0 | 0 | 0 | −220 | 0 | 0 |
| 6 | 0 | −220 | 0 | 160 | −1040 | −220 | 0 | 0 |
| 7 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Δf (Hz) | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|---|
| 0 | −29 | 86 | −100 | −86 | −86 | −57 | 86 | 129 | 71 |
| 1 | 43 | −71 | 57 | −43 | 71 | 100 | 86 | −57 | −57 |
| 2 | −43 | 0 | 14 | −129 | 57 | 86 | 100 | −114 | 114 |
| 3 | 157 | 129 | 0 | 71 | −957 | −970 | 57 | −43 | 171 |
| 4 | −57 | −100 | −86 | −957 | −957 | −957 | −957 | −43 | −86 |
| 5 | −43 | −57 | 14 | 71 | −957 | −957 | 143 | −100 | −71 |
| 6 | −43 | −29 | 71 | 86 | 57 | 71 | −171 | 71 | −57 |
| 7 | −57 | 129 | 86 | 14 | −157 | −14 | 100 | 100 | 29 |
| 8 | 14 | 114 | −57 | 100 | 29 | −14 | −157 | −143 | −176 |
| Width of Void | Depth of Void | k | |
|---|---|---|---|
| Model A | 0.45 m | 0.10 m | 39.5% |
| Model B | 0.35 m | 0.06 m | 57.1% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ju, J.; Tian, X.; Zhao, W.; Yang, Y. Detection and Identification for Void of Concrete Structure by Air-Coupled Impact-Echo Method. Sensors 2023, 23, 6018. https://doi.org/10.3390/s23136018
Ju J, Tian X, Zhao W, Yang Y. Detection and Identification for Void of Concrete Structure by Air-Coupled Impact-Echo Method. Sensors. 2023; 23(13):6018. https://doi.org/10.3390/s23136018
Chicago/Turabian StyleJu, Jinghui, Xiushu Tian, Weigang Zhao, and Yong Yang. 2023. "Detection and Identification for Void of Concrete Structure by Air-Coupled Impact-Echo Method" Sensors 23, no. 13: 6018. https://doi.org/10.3390/s23136018
APA StyleJu, J., Tian, X., Zhao, W., & Yang, Y. (2023). Detection and Identification for Void of Concrete Structure by Air-Coupled Impact-Echo Method. Sensors, 23(13), 6018. https://doi.org/10.3390/s23136018







