Analogical Assessment of Train-Induced Vibration and Radiated Noise in a Proposed Theater
Abstract
1. Introduction
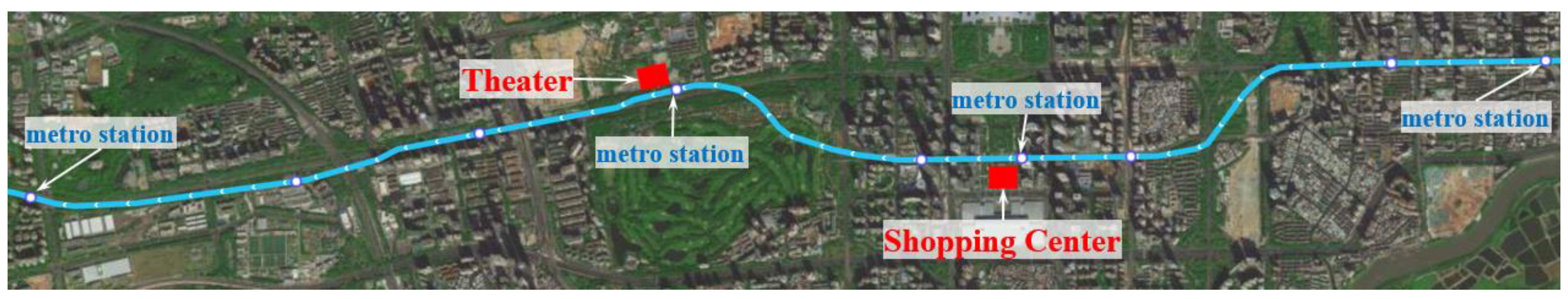
2. Overview of the Theater
3. Assessment Methods
3.1. Experimental Tools
3.2. Vibration Assessment Method
3.3. Radiated Noise Assessment Methods
3.3.1. Equivalent Continuous A-Weighted Sound Pressure
3.3.2. Noise Rating Number
3.4. Allowable Vibration and Noise Levels
4. Measurements and Analysis in the Theater Construction Site
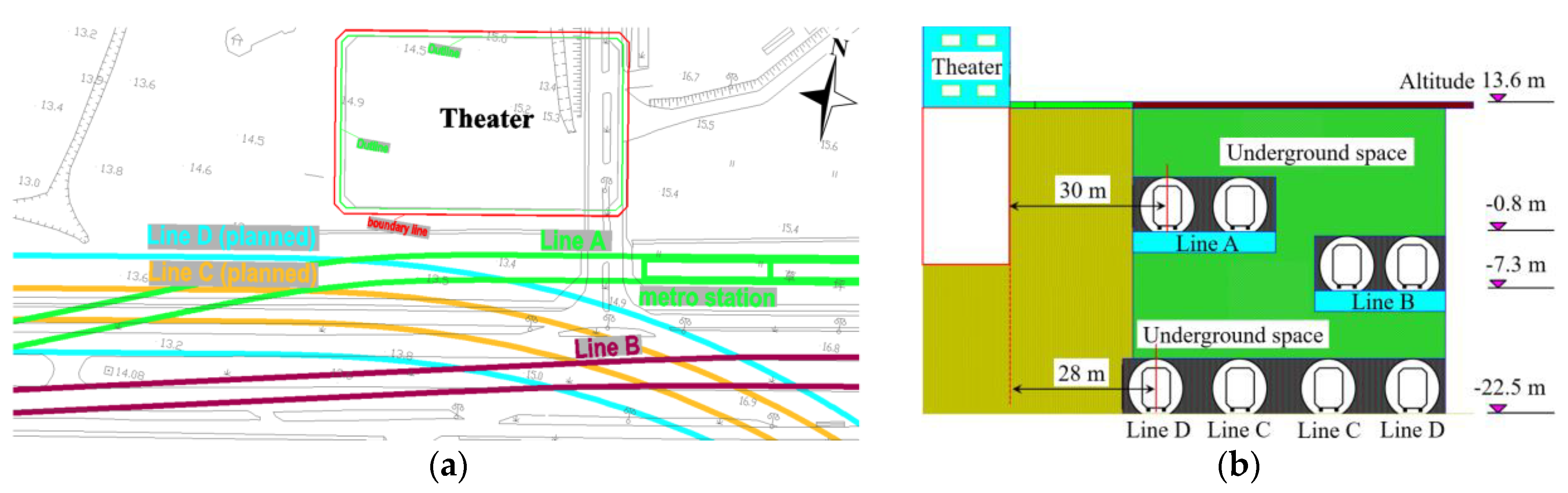
4.1. Metro Information
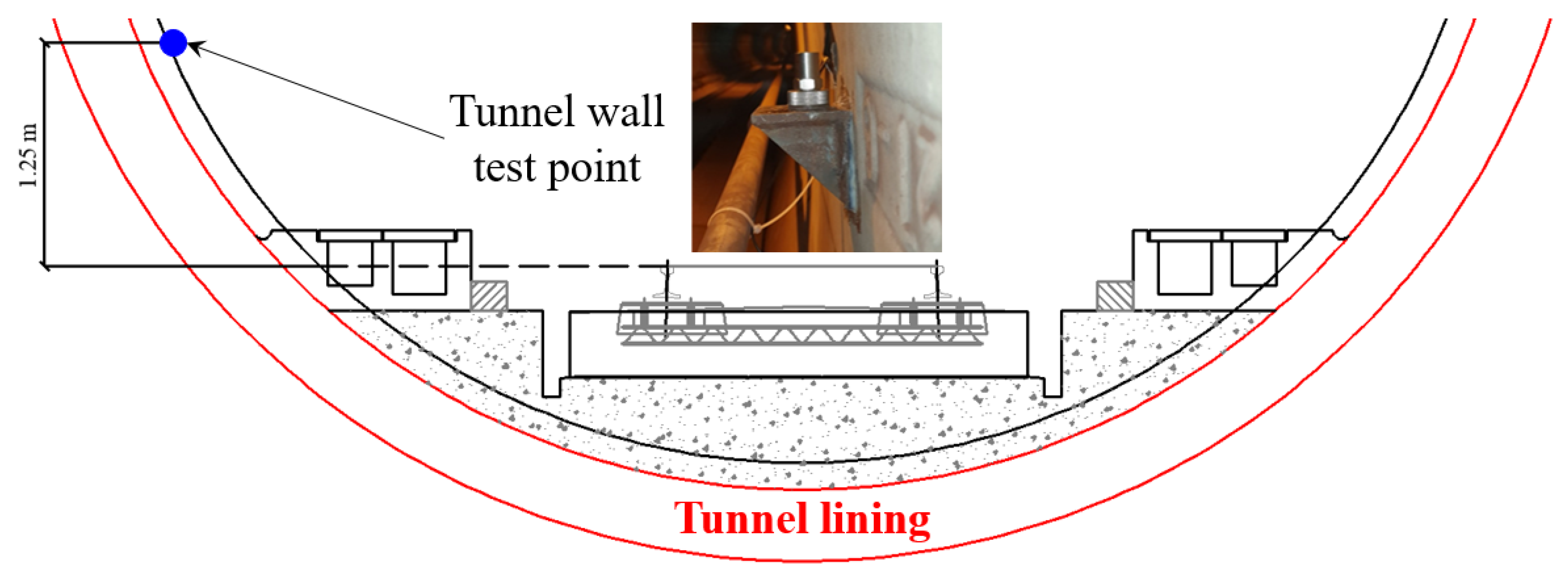
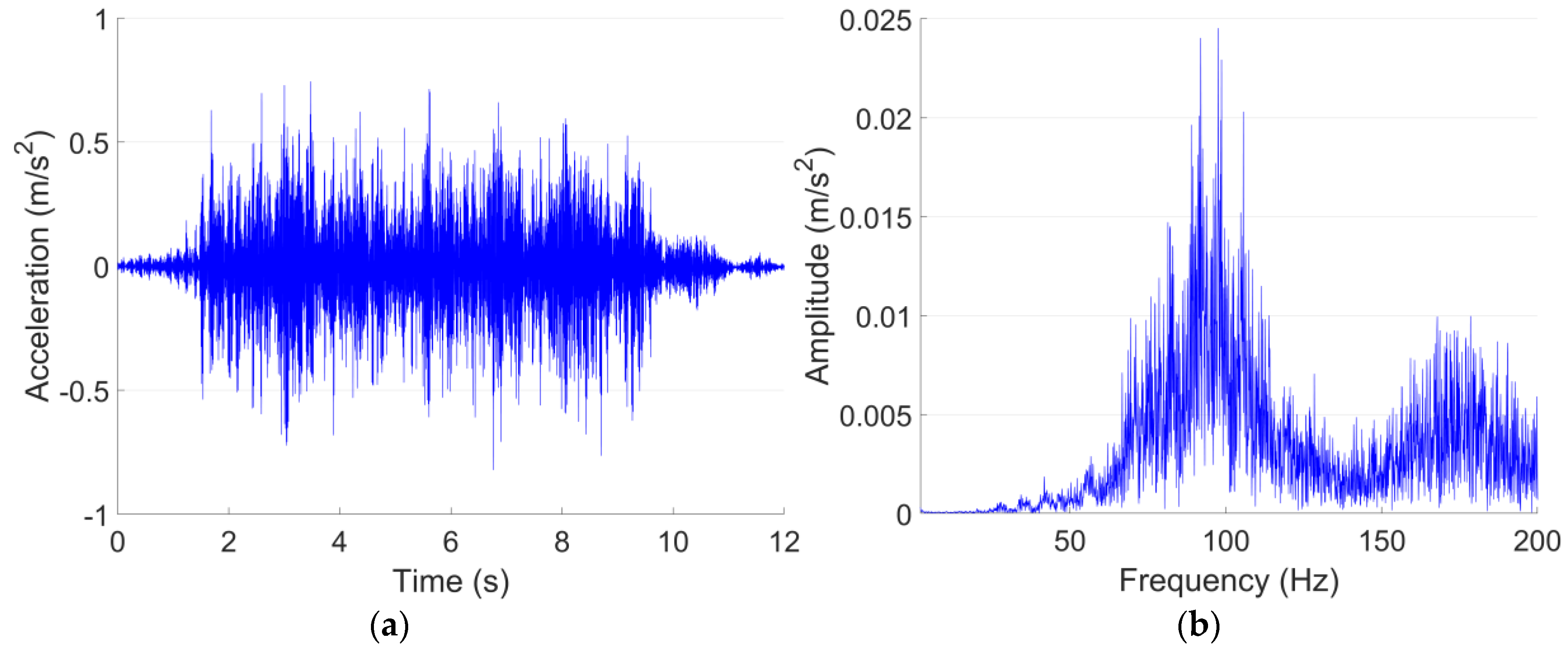
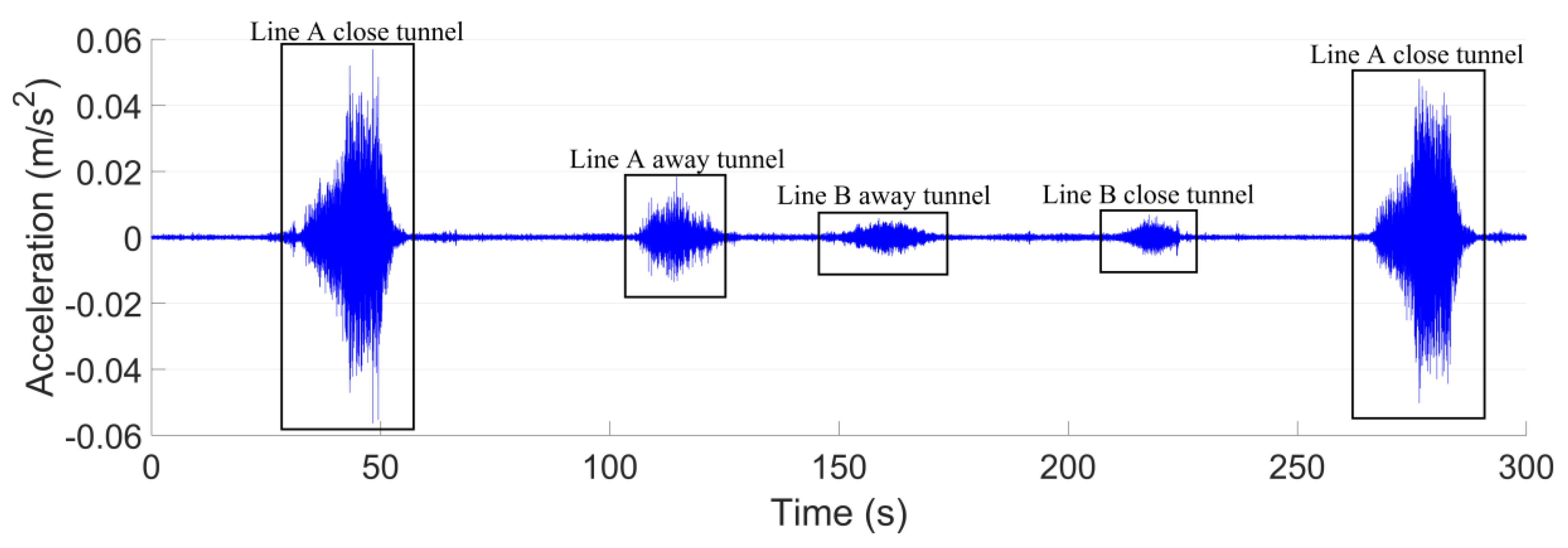
4.2. In-Track Vibration Measurements and Analysis
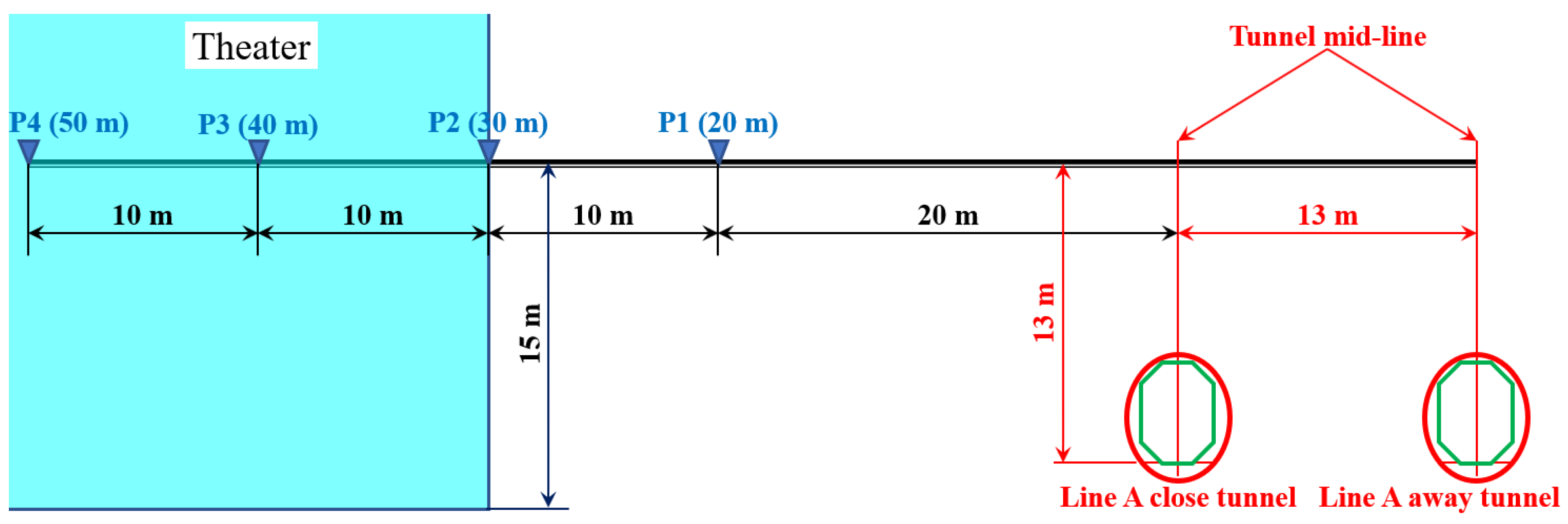
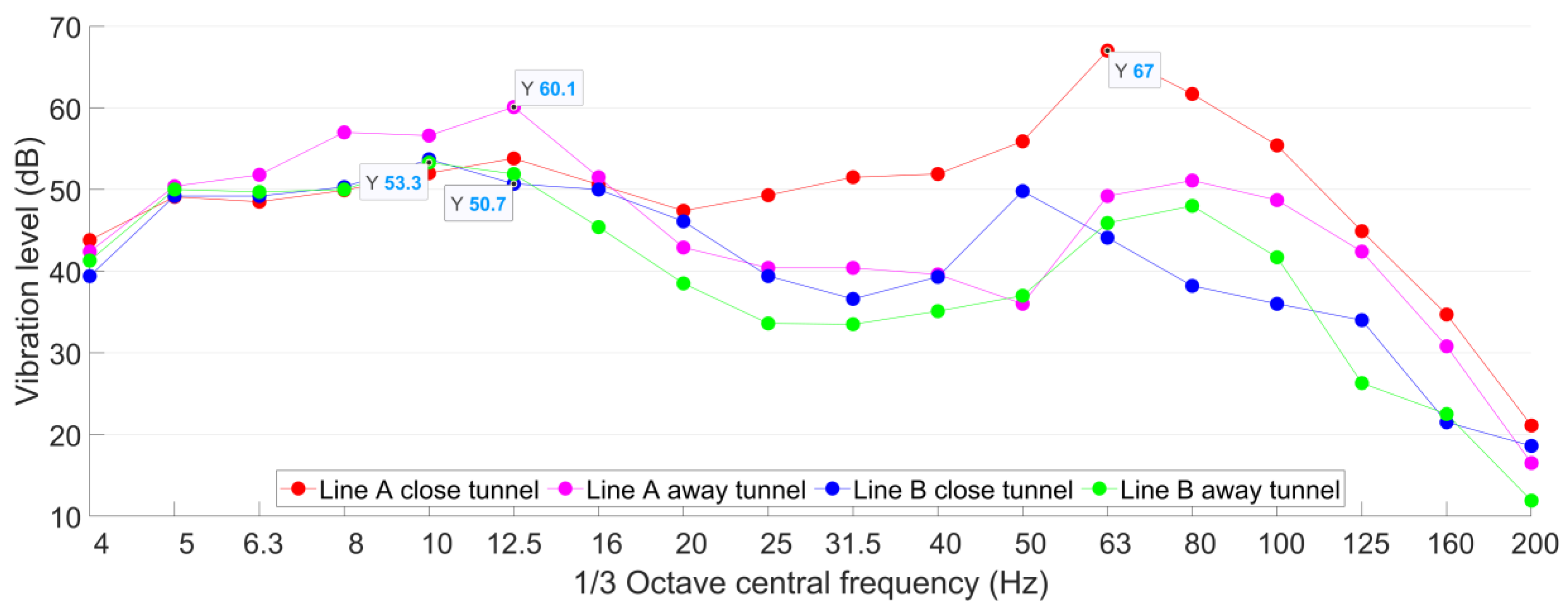
4.3. Ground Vibration Tests and Analysis
5. Measurements and Analysis in the Analogical Building
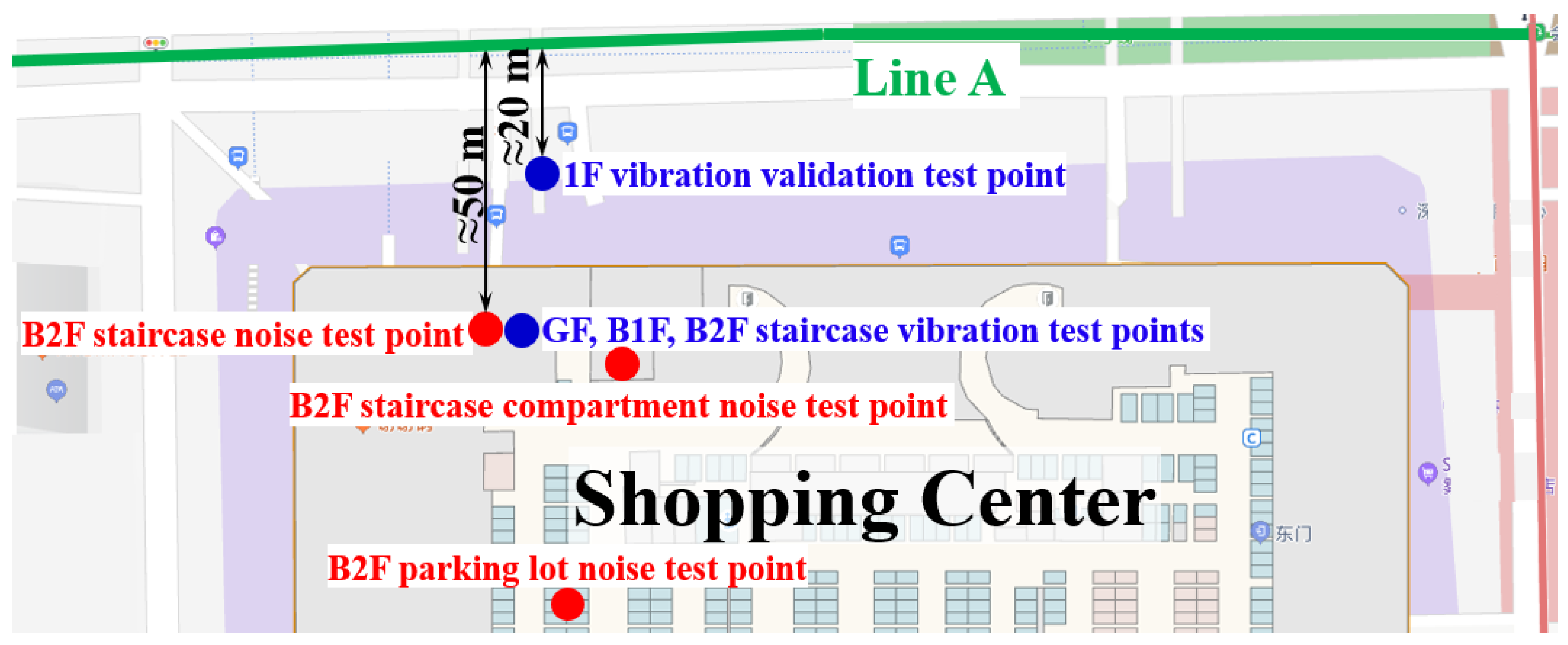
5.1. Analogical Building Selection
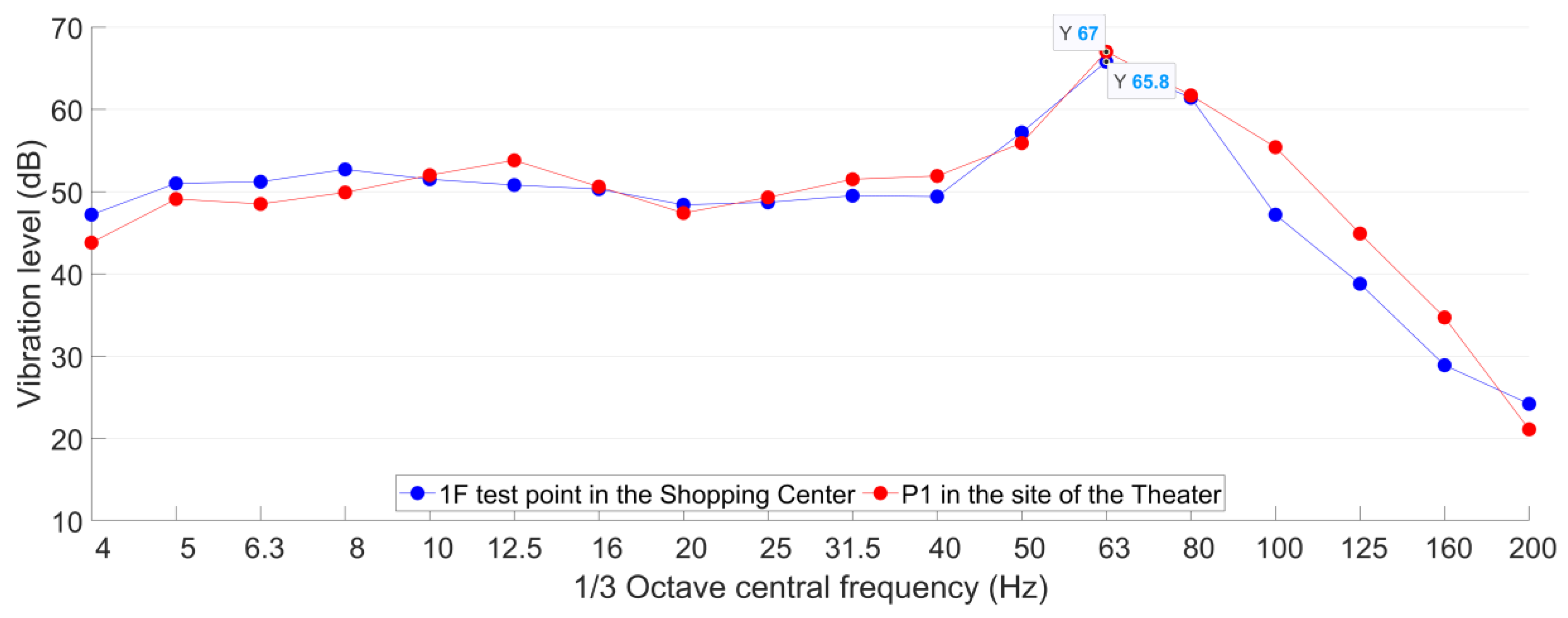
5.2. Ground Vibration Validation
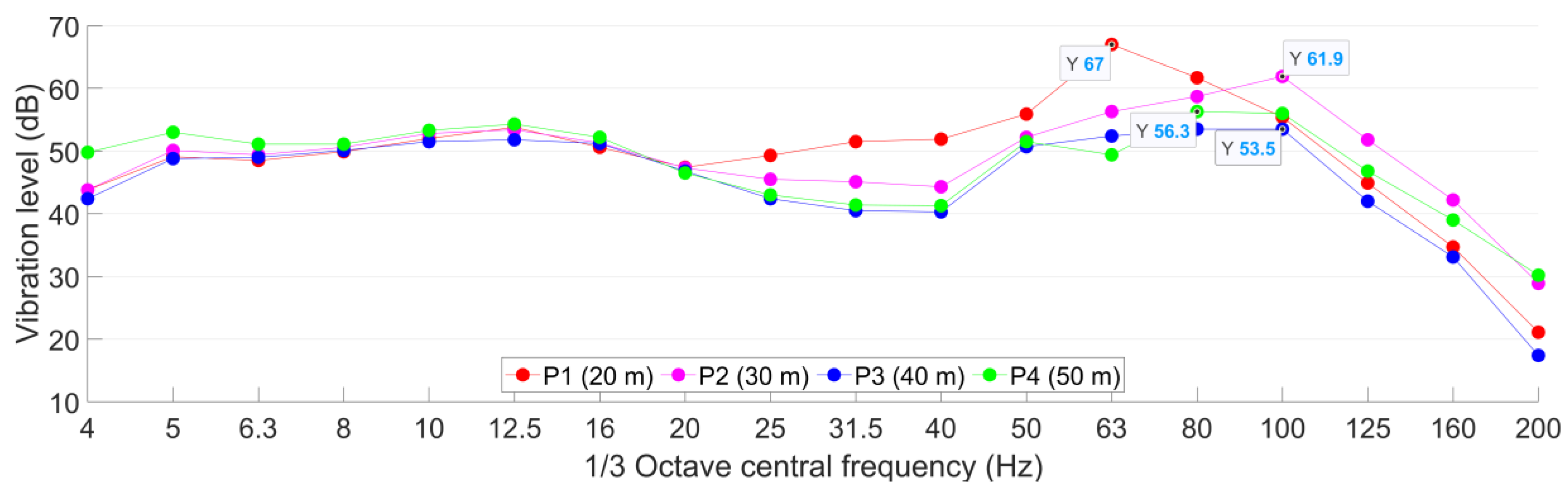
5.3. Analogical Measurements and Analysis
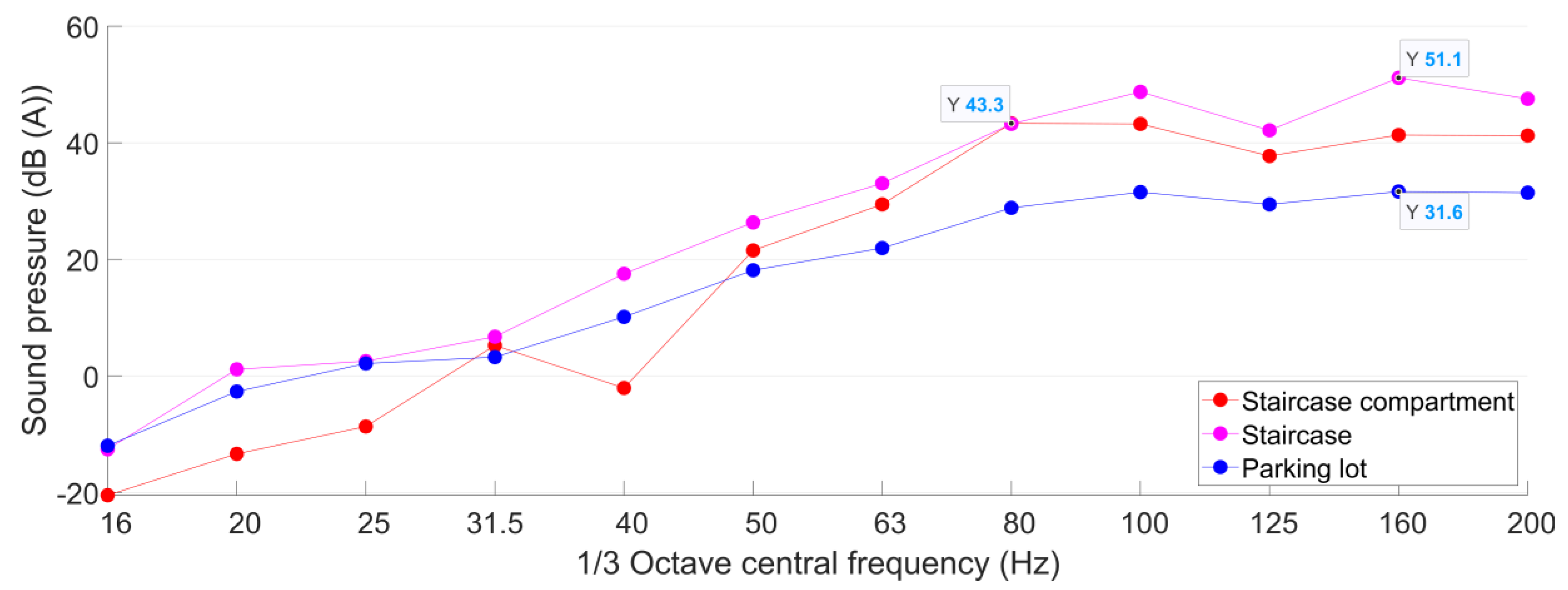
- The vibration and noise measurements and analysis in the public area such as the staircase, staircase compartment and parking lot to simulate the public area of the theater;
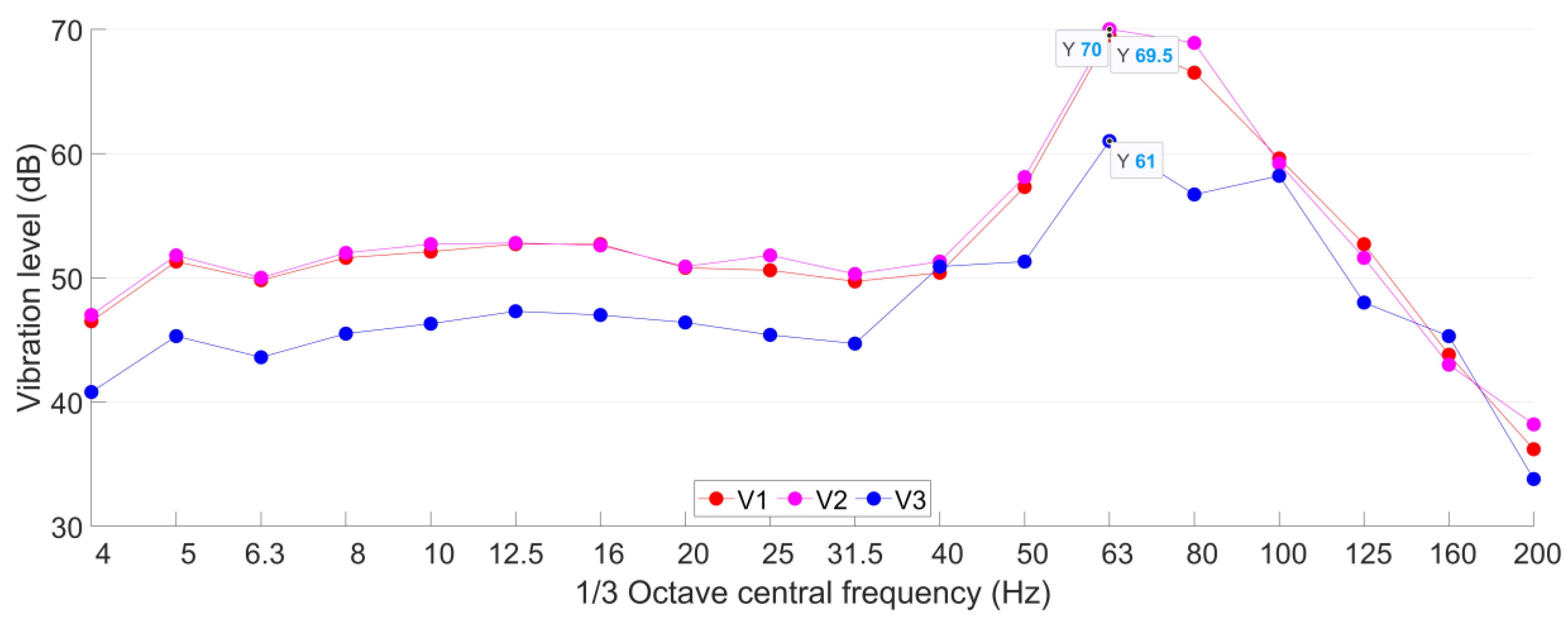
- The vibration and noise measurements and analysis in the cinema simulate the dream theater and the multi-functional performing space in the theater.
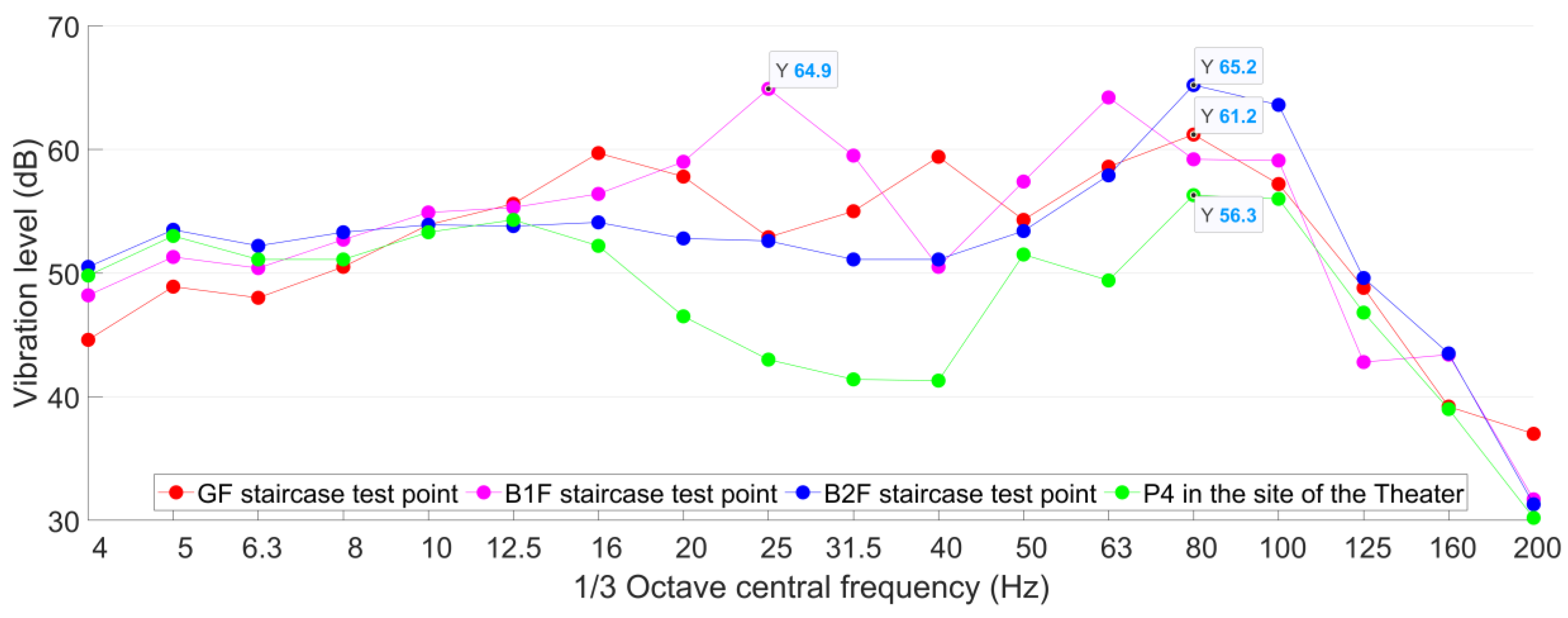
5.3.1. Public Area Measurements and Analysis
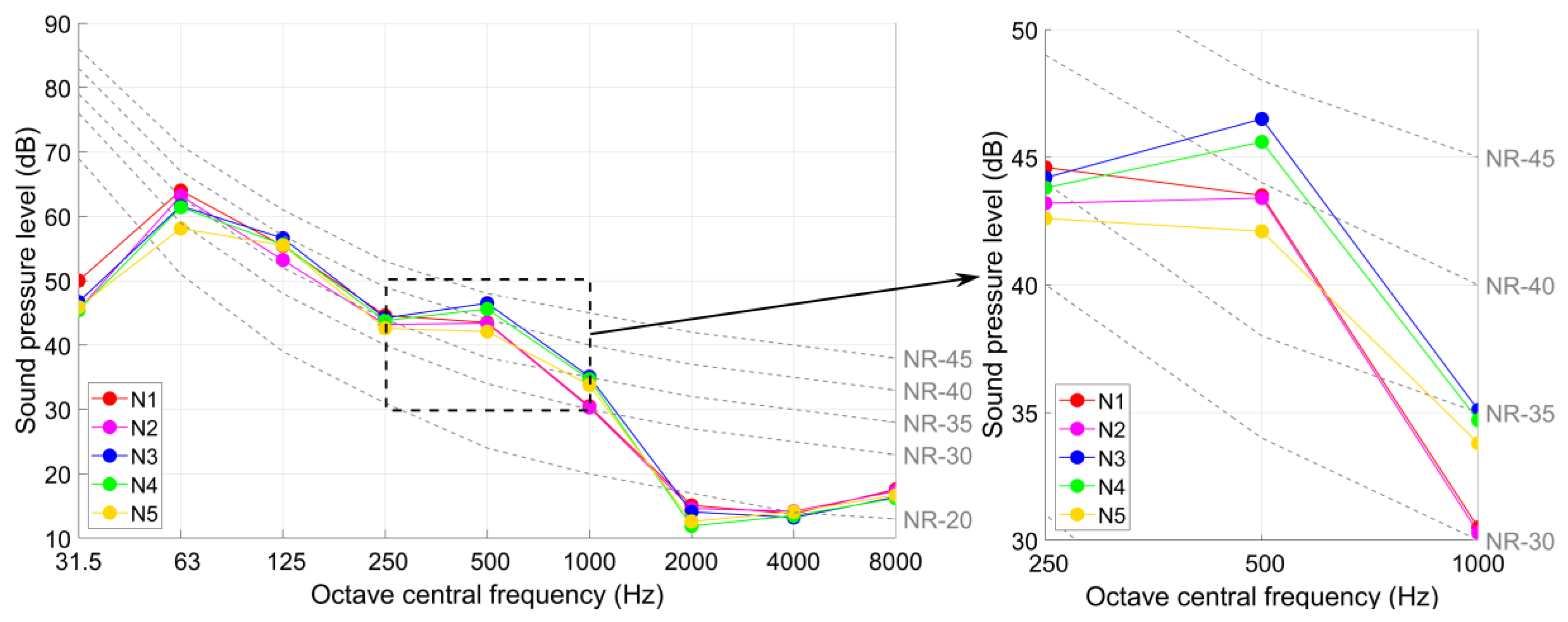
5.3.2. Cinema Measurements and Analysis
6. Conclusions and Discussion
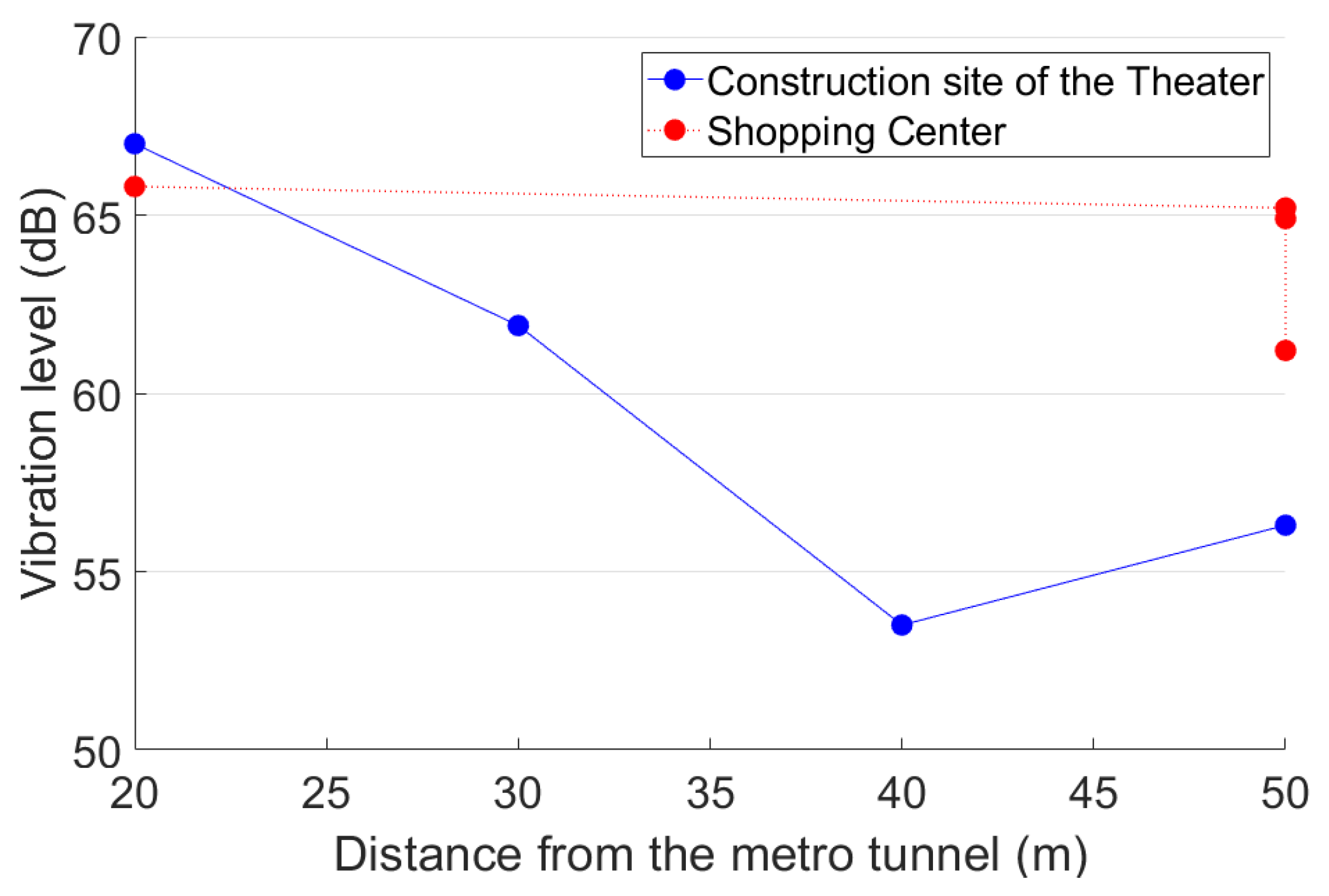
- The train-induced vibration presents higher responses in the analogical building. With the same horizontal distance (50 m) from the metro tunnel, the ground vibration in the staircase of the analogical building was up to 65 dB, which is 9 dB higher than that in the construction site of the theater. In the cinema of the analogical building (60 m from the metro tunnel), the train-induced ground vibration was up to 70 dB, which is already 5 dB higher than the daytime allowable level for the theater. Such results indicate that the expected vibration level in the theater will be much higher than now measured at the construction site, which can be explained by the excavation of soil that reduced the soil damping effect.
- The highest equivalent continuous A sound pressure obtained in the public area of the analogical building was 55 dB (A), which is 10 dB (A) higher than the allowable level of the theater. The noise rating number in the cinema of the analogical building was up to NR-43. Compared with the allowable level of NR-20 for the dream theater and multi-functional performing space, the noise reduction requirement for the theater is more than 23 dB, which puts forward a challenging task for the theater anti-vibration design and construction.
- The expected high impact of train-induced vibration and radiated noise on the proposed theater is not only due to the mutual positional relationship between the theater and the metro tunnel, but also due to the lack of anti-vibration design of the current metro line. Such a fact puts forward requirements on the planned metro lines. To guarantee the stage effect of the theater, vibration mitigation measures have to be fully considered.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Xia, H.; Cao, Y.; De Guido, R.; Geert, D. Environmental problems of vibrations induced by railway traffic. Front. Archit. Civ. Eng. China 2007, 1, 142–152. [Google Scholar] [CrossRef]
- Connolly, D.P.; Marecki, G.P.; Kouroussis, G.; Thalassinakis, I.; Woodward, P.K. The growth of railway ground vibration problems—A review. Sci. Total Environ. 2016, 568, 1276–1282. [Google Scholar] [CrossRef] [PubMed]
- Sadeghi, J.; Esmaeili, M.H. Safe distance of cultural and historical buildings from subway lines. Soil Dyn. Earthq. Eng. 2017, 96, 89–103. [Google Scholar] [CrossRef]
- Ma, M.; Liu, W.; Qian, C.; Deng, G.; Li, Y. Study of the train-induced vibration impact on a historic Bell Tower above two spatially overlapping metro lines. Soil Dyn. Earthq. Eng. 2016, 81, 58–74. [Google Scholar] [CrossRef]
- Qu, S.; Yang, J.; Zhu, S.; Zhai, W.; Kouroussis, G. A hybrid methodology for predicting train-induced vibration on sensitive equipment in far-field buildings. Transp. Geotech. 2021, 31, 100682. [Google Scholar] [CrossRef]
- Merideno, I.; Nieto, J.; Gil-Negrete, N.; Giménez Ortiz, J.G.; Landaberea, A.; Iartza, J. Theoretical prediction of the damping of a railway wheel with sandwich-type dampers. J. Sound Vib. 2014, 333, 4897–4911. [Google Scholar] [CrossRef]
- Li, W.; Wang, A.; Gao, X.; Ju, L.; Liu, L. Development of multi-band tuned rail damper for rail vibration control. Appl. Acoust. 2021, 184, 108370. [Google Scholar] [CrossRef]
- Gao, X.; Wang, A.; Liu, L.; He, Y.; Ju, L. Analysis of failure mechanism of W1-type fastening clip in high speed railway and structure study of damping composite. Eng. Fail. Anal. 2020, 118, 104848. [Google Scholar] [CrossRef]
- Jin, H.; Liu, W.; Zhou, S. Optimization of Vibration Reduction Ability of Ladder Tracks by FEM Coupled with ACO. Shock Vib. 2015, 2015, 84827. [Google Scholar] [CrossRef]
- Han, J.; He, Y.; Wang, J.; Xiao, X. Simulation and experimental study on vibration and acoustic characteristics of a continuous supported embedded track. Appl. Acoust. 2021, 180, 108103. [Google Scholar] [CrossRef]
- Lombaert, G.; Degrande, G.; Vanhauwere, B.; Vandeborght, B.; François, S. The control of ground-borne vibrations from railway traffic by means of continuous floating slabs. J. Sound Vib. 2006, 297, 946–961. [Google Scholar] [CrossRef]
- Zhao, C.; Shi, D.; Zheng, J.; Niu, Y.; Wang, P. New floating slab track isolator for vibration reduction using particle damping vibration absorption and bandgap vibration resistance. Constr. Build. Mater. 2022, 336, 127561. [Google Scholar] [CrossRef]
- Liang, L.; Li, X.; Yin, J.; Wang, D.; Gao, W.; Guo, Z. Vibration characteristics of damping pad floating slab on the long-span steel truss cable-stayed bridge in urban rail transit. Eng. Struct. 2019, 191, 92–103. [Google Scholar] [CrossRef]
- Alabbasi, S.; Hussein, M.; Abdeljaber, O.; Avci, O. A numerical and experimental investigation of a special type of floating-slab tracks. Eng. Struct. 2020, 215, 110734. [Google Scholar] [CrossRef]
- Zhao, Z.; Wei, K.; Ding, W.; Du, W.; Li, H. UM-SIMULINK Co-simulation for the vibration reduction optimization of a magnetorheological damping steel-spring floating slab track. Constr. Build. Mater. 2021, 307, 124923. [Google Scholar] [CrossRef]
- Ma, M.; Li, M.; Qu, X.; Zhang, H. Effect of passing metro trains on uncertainty of vibration source intensity: Monitoring tests. Measurement 2022, 193, 110992. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, W.; Zhang, W.; Li, F.; Du, Y. Train-Induced Vibration Monitoring of Track Slab under Long-Term Temperature Load Using Fiber-Optic Accelerometers. Sensors 2021, 21, 787. [Google Scholar] [CrossRef]
- Auersch, L. Different Types of Continuous Track Irregularities as Sources of Train-Induced Ground Vibration and the Importance of the Random Variation of the Track Support. Appl. Sci. 2022, 12, 1463. [Google Scholar] [CrossRef]
- Hasap, A.; Noraphaiphipaksa, N.; Kanchanomai, C. Influence of malposition on the performance of elastic rail clip: Toe load, stress, and friction. Structures 2020, 28, 2661–2670. [Google Scholar] [CrossRef]
- Zou, C.; Wang, Y.; Tao, Z. Train-Induced Building Vibration and Radiated Noise by Considering Soil Properties. Sustainability 2020, 12, 937. [Google Scholar] [CrossRef]
- Hildebrand, R. Effect of soil stabilization on audible band railway ground vibration. Soil Dyn. Earthq. Eng. 2004, 24, 411–424. [Google Scholar] [CrossRef]
- Auersch, L. Train-induced ground vibration due to the irregularities of the soil. Soil Dyn. Earthq. Eng. 2021, 140, 106438. [Google Scholar] [CrossRef]
- Huang, S.; Chen, Y.; Zou, C.; Jian, S. Train-induced environmental vibrations by considering different building foundations along curved track. Transp. Geotechn. 2022, 35, 100785. [Google Scholar] [CrossRef]
- Tao, Z.; Wang, Y.; Sanayei, M.; Moore, J.A.; Zou, C. Experimental study of train-induced vibration in over-track buildings in a metro depot. Eng. Struct. 2019, 198, 109473. [Google Scholar] [CrossRef]
- Cao, Z.; Guo, T.; Zhang, Z.; Li, A. Measurement and analysis of vibrations in a residential building constructed on an elevated metro depot. Measurement 2018, 125, 394–405. [Google Scholar] [CrossRef]
- Zou, C.; Wang, Y.; Moore, J.A.; Sanayei, M. Train-induced field vibration measurements of ground and over-track buildings. Sci. Total Environ. 2017, 575, 1339–1351. [Google Scholar] [CrossRef]
- Sheng, T.; Bian, X.; Liu, G.; Xiao, C.; Chen, Y.; Li, Y. Experimental study on the sandbag isolator of buildings for subway-induced vertical vibration and radiated air-borne noise. Geotext. Geomembr. 2020, 48, 504–515. [Google Scholar] [CrossRef]
- Zhao, C.; Zheng, J.; Sang, T.; Wang, L.; Yi, Q.; Wang, P. Computational analysis of phononic crystal vibration isolators via FEM coupled with the acoustic black hole effect to attenuate railway-induced vibration. Constr. Build. Mater. 2021, 283, 122802. [Google Scholar] [CrossRef]
- Connolly, D.P.; Alves Costa, P.; Kouroussis, G.; Galvin, P.; Woodward, P.K.; Laghrouche, O. Large scale international testing of railway ground vibrations across Europe. Soil Dyn. Earthq. Eng. 2015, 71, 1–12. [Google Scholar] [CrossRef]
- Kouroussis, G.; Conti, C.; Verlinden, O. Experimental study of ground vibrations induced by Brussels IC/IR trains in their neighbourhood. Mech. Ind. 2013, 14, 99–105. [Google Scholar] [CrossRef]
- Mouzakis, C.; Vogiatzis, K.; Zafiropoulou, V. Assessing subway network ground borne noise and vibration using transfer function from tunnel wall to soil surface measured by muck train operation. Sci. Total Environ. 2019, 650, 2888–2896. [Google Scholar] [CrossRef]
- Sanayei, M.; Maurya, P.; Moore, J.A. Measurement of building foundation and ground-borne vibrations due to surface trains and subways. Eng. Struct. 2013, 53, 102–111. [Google Scholar] [CrossRef]
- Zou, C.; Wang, Y.; Wang, P.; Guo, J. Measurement of ground and nearby building vibration and noise induced by trains in a metro depot. Sci. Total Environ. 2015, 536, 761–773. [Google Scholar] [CrossRef]
- Ibrahim, Y.E.; Nabil, M. Finite element analysis of multistory structures subjected to train-induced vibrations considering soil-structure interaction. Case Stud. Constr. Mater. 2021, 15, e00592. [Google Scholar] [CrossRef]
- López-Mendoza, D.; Romero, A.; Connolly, D.P.; Galvín, P. Scoping assessment of building vibration induced by railway traffic. Soil Dyn. Earthq. Eng. 2017, 93, 147–161. [Google Scholar] [CrossRef]
- Lopes, P.; Costa, P.A.; Ferraz, M.; Calçada, R.; Cardoso, A.S. Numerical modeling of vibrations induced by railway traffic in tunnels: From the source to the nearby buildings. Soil Dyn. Earthq. Eng. 2014, 61, 269–285. [Google Scholar] [CrossRef]
- Guo, T.; Cao, Z.; Zhang, Z.; Li, A. Numerical simulation of floor vibrations of a metro depot under moving subway trains. J. Vib. Control 2018, 24, 4353–4366. [Google Scholar] [CrossRef]
- Lopes, P.; Alves Costa, P.; Calçada, R.; Silva Cardoso, A. Numerical Modeling of Vibrations Induced in Tunnels: A 2.5D FEM-PML Approach. In Traffic Induced Environmental Vibrations and Controls: Theory and Application; Xia, H., Calçada, R., Eds.; Nova Science Publishers: Hauppauge, NY, USA, 2013; pp. 133–166. [Google Scholar]
- Kouroussis, G.; Vogiatzis, K.E.; Connolly, D.P. A combined numerical/experimental prediction method for urban railway vibration. Soil Dyn. Earthq. Eng. 2017, 97, 377–386. [Google Scholar] [CrossRef]
- JGJ/T 170-2009; Standard for Limit and Measuring Method of Building Vibration and Radiated Noise Caused by Urban Rail Transit. China Architecture & Building Press: Beijing, China, 2009. (In Chinese)
- GB/T 50356-2005; Code for Architectural Acoustical Design of Theater, Cinema and Multi-Use Auditorium. China Planning Press: Beijing, China, 2005. (In Chinese)
- ISO 2631-1:1997; Mechanical Vibration and Shock—Evaluation of Human Exposure to Whole-Body Vibration—Part 1: General Requirements. International Organization for Standardization: Geneva, Switzerland, 1997.
- IEC 61672-1:2013; Electroacoustics—Sound Level Meters—Part 1 Specifications. British Standards Institution: London, UK, 2013.
- ISO/R 1996:1971; Acoustics—Assessment of Noise with Respect to Community Response. International Organization for Standardization: Geneva, Switzerland, 1971.
- HJ 453-2018; Technical Guidelines for Environmental Impact Assessment—Urban Rail Transit. China Environmental Press: Beijing, China, 2019. (In Chinese)







| Sensor Type | Test Object | Measuring Range | Resolution | Frequency Range |
|---|---|---|---|---|
| Accelerometer | Tunnel wall and ground acceleration | ±7 m/s2 | 0.00025 m/s2 | 0.1–500 Hz |
| Sound level meter | Radiated noise | 20–146 dB | 0.01 dB | 10–20,000 Hz |
| Octave Frequency (Hz) | 31.5 | 63 | 125 | 250 | 500 | 1000 | 2000 | 4000 | 8000 |
| Sound Pressure Level (dB (A)) | 69 | 51 | 39 | 31 | 24 | 20 | 17 | 14 | 13 |
| Items\Lines | Line A | Line B |
|---|---|---|
| Train type/Axle load | Metro Type A/17 tones | |
| Marshalling number | 6 | 8 |
| Train length | 140 m | 184 m |
| Passing velocity | 60 km/h | 110 km/h |
| Tunnel construction method | Cut and cover | Shield |
| Track/Rail type | Ordinary monolithic track bed/CN 60 | |
| Depth of rail top | 15 m | 21 m |
| Shortest distance from the theater | 30 m | 80 m |
| Items | Theater | Shopping Center |
|---|---|---|
| Metro track type | The ordinary integral track bed | |
| Train velocity | 60 km/h | |
| The horizontal distance from Line A | ≈30 m | |
| Tunnel rail surface depth | 13 m | 15 m |
| Structure foundation depth | 15 m | 12 m |
| Geological condition | Plain fill, grit, gravelly clay, completely decomposed granite | |
| Composition at the bottom of tunnels | Gravelly clay | |
| B2F Noise Test Point | Radiated Noise | Background Noise | Difference | Amendment | Amended Noise |
|---|---|---|---|---|---|
| Staircase | 55 | 37 | 18 | 0 | 55 |
| Staircase compartment | 49 | 30 | 19 | 0 | 49 |
| Parking lot | 39 | 32 | 7 | 1 | 38 |
| Test Points | N1 | N2 | N3 | N4 | N5 |
|---|---|---|---|---|---|
| LAeq (dB (A)) | 42 | 40 | 42 | 41 | 40 |
| NR value | NR-40 | NR-40 | NR-43 | NR-42 | NR-38 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, X.; Xiao, Y.; Jiang, H.; Guo, Y.; Yu, M.; Tan, W. Analogical Assessment of Train-Induced Vibration and Radiated Noise in a Proposed Theater. Sensors 2023, 23, 505. https://doi.org/10.3390/s23010505
Liu X, Xiao Y, Jiang H, Guo Y, Yu M, Tan W. Analogical Assessment of Train-Induced Vibration and Radiated Noise in a Proposed Theater. Sensors. 2023; 23(1):505. https://doi.org/10.3390/s23010505
Chicago/Turabian StyleLiu, Xiangming, Yuchun Xiao, Huihuang Jiang, Yunlong Guo, Mengwen Yu, and Wanzhong Tan. 2023. "Analogical Assessment of Train-Induced Vibration and Radiated Noise in a Proposed Theater" Sensors 23, no. 1: 505. https://doi.org/10.3390/s23010505
APA StyleLiu, X., Xiao, Y., Jiang, H., Guo, Y., Yu, M., & Tan, W. (2023). Analogical Assessment of Train-Induced Vibration and Radiated Noise in a Proposed Theater. Sensors, 23(1), 505. https://doi.org/10.3390/s23010505







