Suppression Method of Optical Noises in Resonator-Integrated Optic Gyroscopes
Abstract
:1. Introduction
2. The Basic Configuration of the RIOG
3. Noise and Suppression
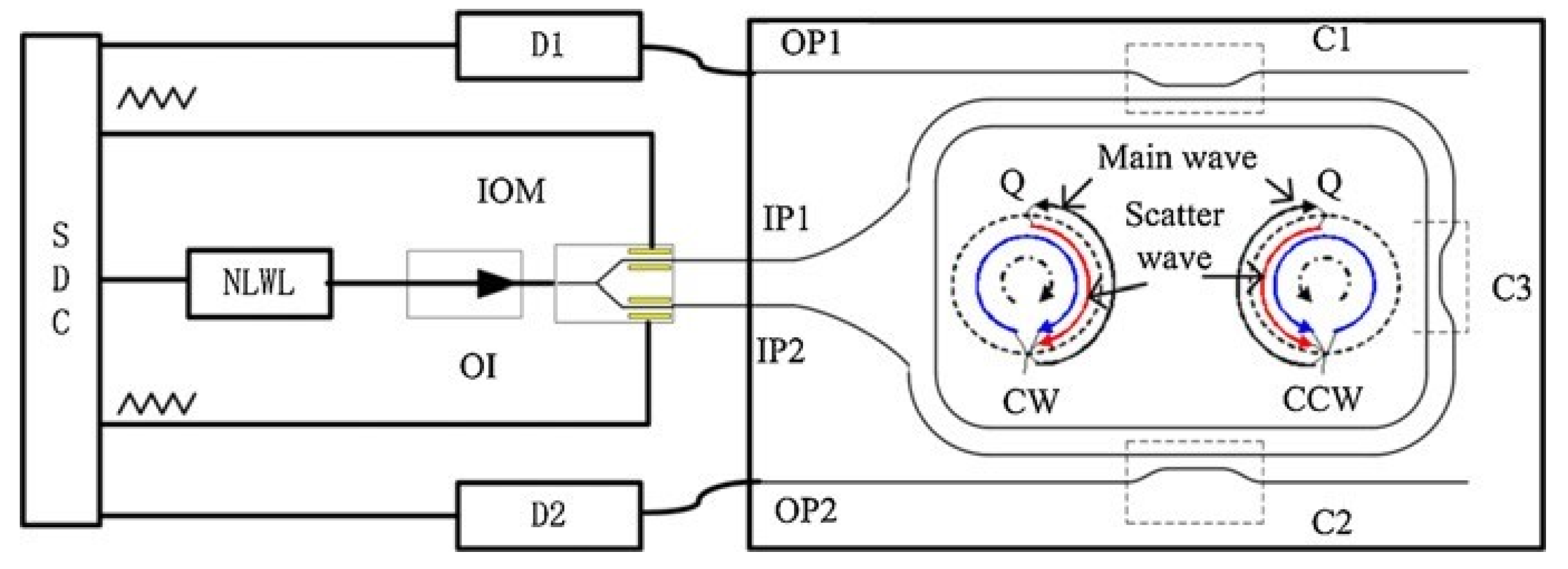
3.1. Rayleigh Backscattering Noise
3.1.1. The Intensity of Rayleigh Backscattering Noise
3.1.2. Suppression Method of Rayleigh Backscattering Noise in RIOG
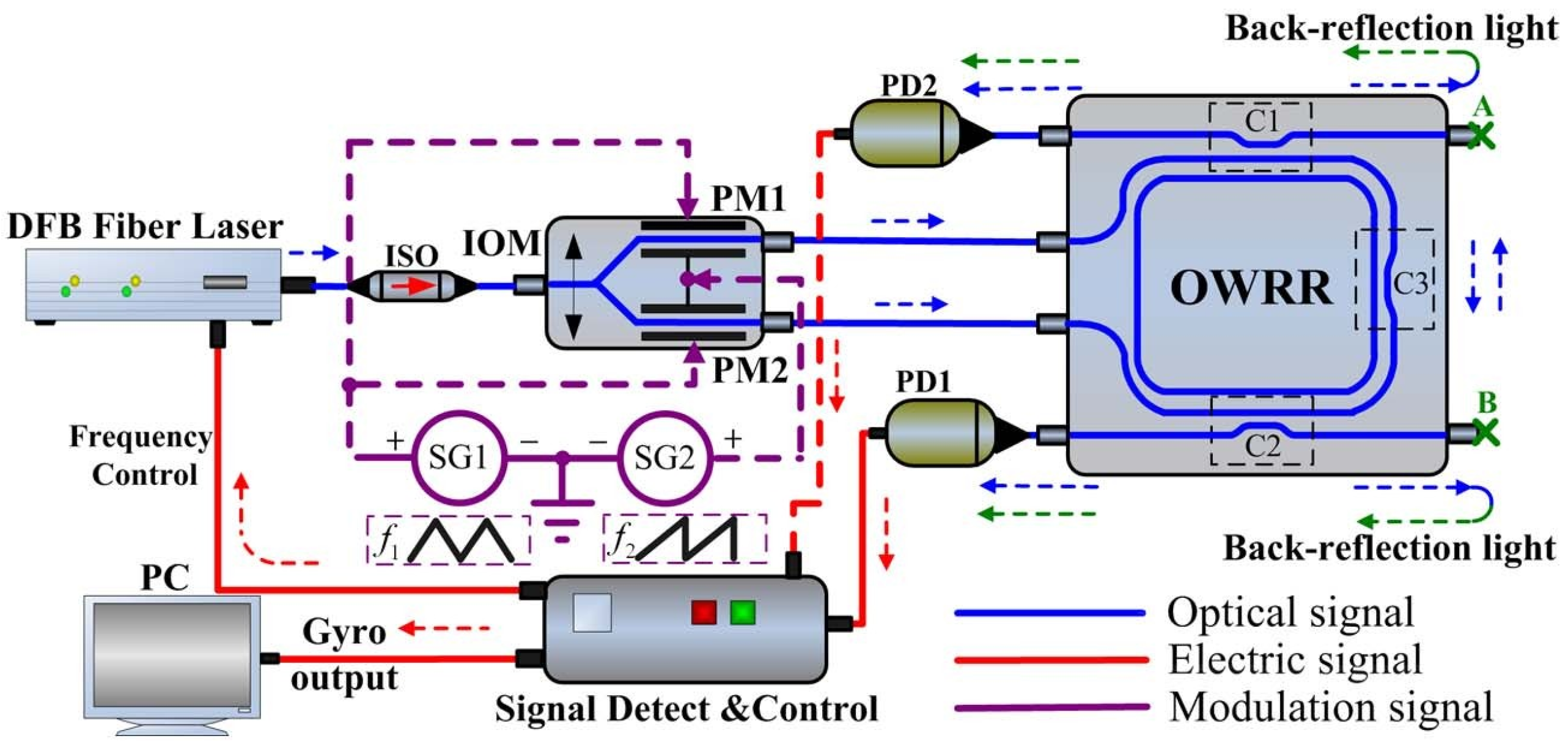
3.2. Back-Reflection Noise
3.2.1. The Back-Reflection Noise
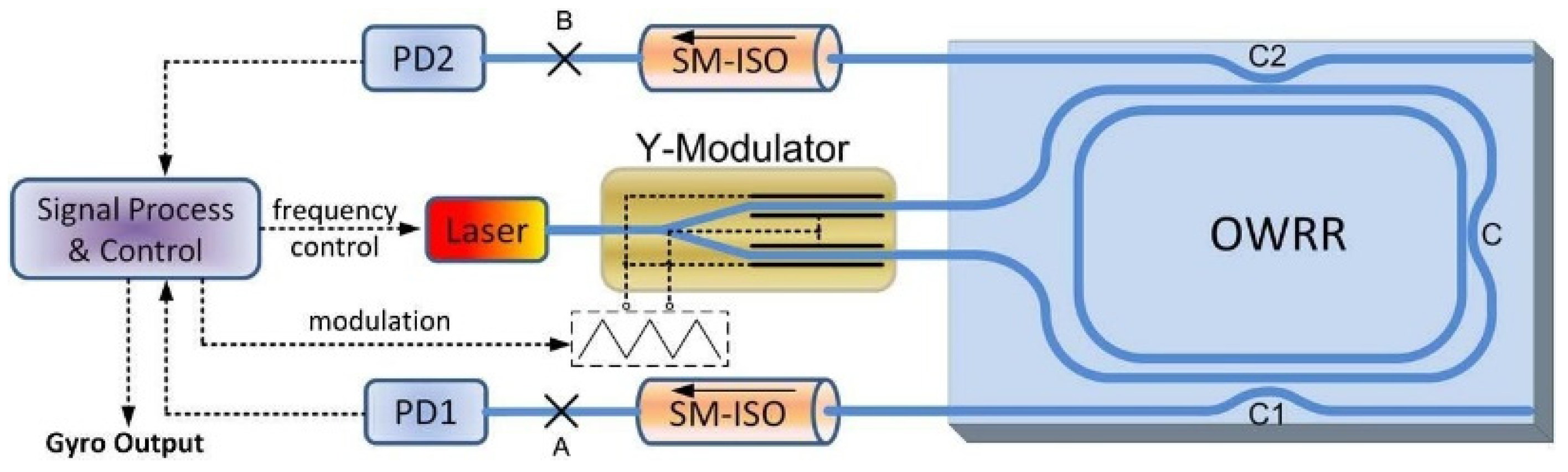
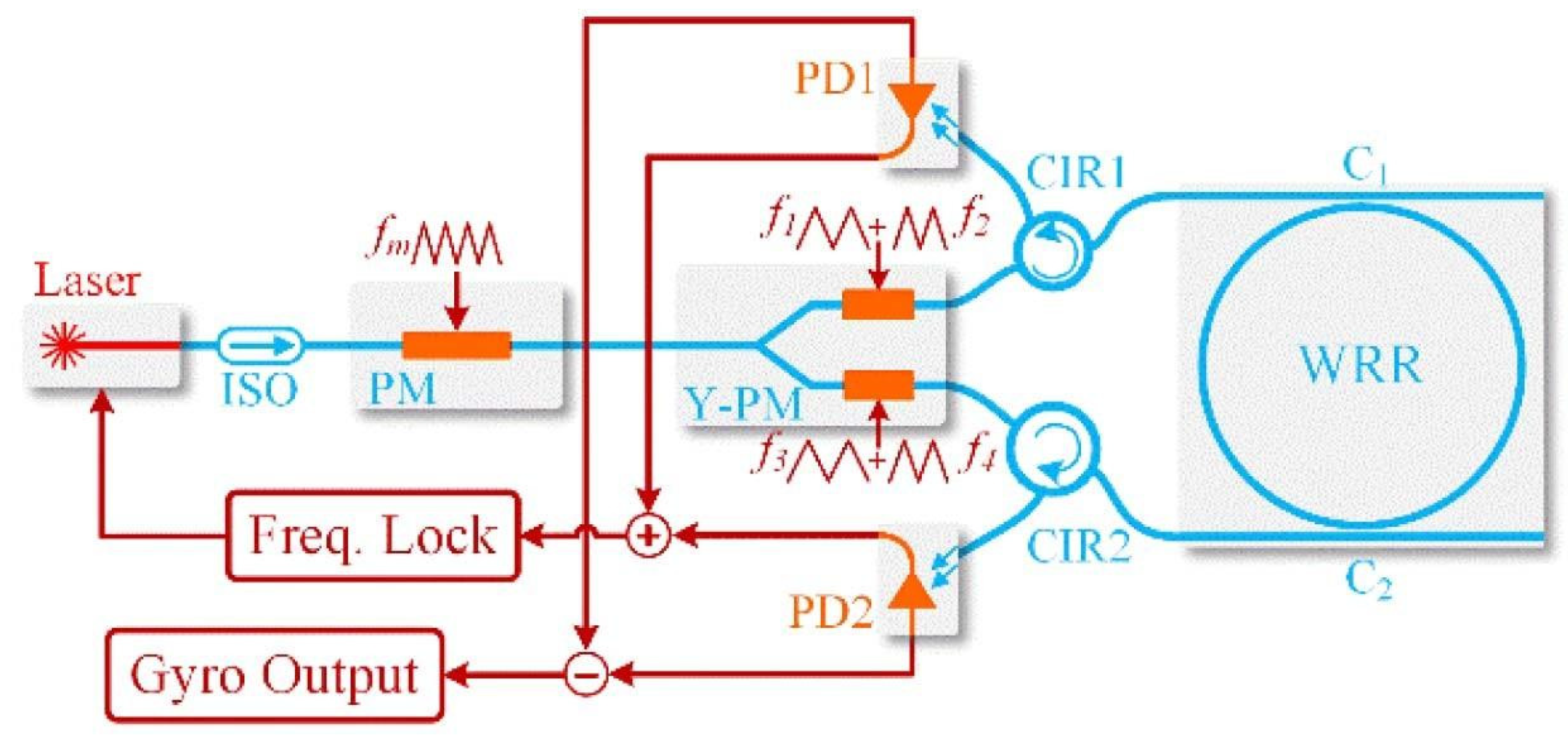
3.2.2. Suppression Method of Back-Reflection Noise in RIOG
3.3. Optical Kerr Effect
3.3.1. Nonlinear Kerr Noise
3.3.2. Suppression Method of Kerr-Induced Noise in RIOG
3.4. Polarization Noise
3.4.1. Nonreciprocal Polarization Fluctuation Noise
3.4.2. Suppression Method of Polarization Fluctuation-Induced Noise in RIOG
3.5. Laser Frequency Noise
4. New Noise Suppression Technology
5. Summary of Noise Suppression
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Arditty, H.J.; Lefevre, H.C. Fiber-Optic Gyroscopes. In New Directions in Guided Wave and Coherent Optics; Ostrowsky, D.B., Spitz, E., Eds.; NATO ASI Series; Springer: Dordrecht, The Netherlands, 1984; pp. 299–333. ISBN 978-94-010-9550-1. [Google Scholar]
- Passaro, V.M.N.; Cuccovillo, A.; Vaiani, L.; De Carlo, M.; Campanella, C.E. Gyroscope Technology and Applications: A Review in the Industrial Perspective. Sensors 2017, 17, 2284. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lefevre, H.C. The Fiber-Optic Gyroscope: Actually Better than the Ring-Laser Gyroscope? In Proceedings of the OFS International Conference on Optical Fiber Sensor, Beijing, China, 15–17 October 2012. [Google Scholar]
- Baretela, M.; Heckman, D.W. Interferometric Fiber Optic Gyro Technology (IFOG). IEEE Aerosp. Electron. Syst. Mag. 2000, 15, 23–28. [Google Scholar] [CrossRef]
- Qian, K.; Tang, J.; Guo, H.; Liu, W.; Liu, J.; Xue, C.; Zheng, Y.; Zhang, C. Under-Coupling Whispering Gallery Mode Resonator Applied to Resonant Micro-Optic Gyroscope. Sensors 2017, 17, 100. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, Y.M.; Yang, F.H.; Yan, W.; Han, W.H.; Li, Z.F. Asymmetry Analysis of the Resonance Curve in Resonant Integrated Optical Gyroscopes. Sensors 2019, 19, 3305. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, Y.; Ma, H.; Jin, Z. Ultra-Low-Loss Spliceless Fiber Ring Resonators for Resonant Micro-Optic Gyroscopes. In Proceedings of the CLEO Pacific Rim Conference, Hong Kong, 29 July–3 August 2018; p. Th3L.4. [Google Scholar]
- Post, E.J. Sagnac Effect. Rev. Mod. Phys. 1967, 39, 475–493. [Google Scholar] [CrossRef]
- Arditty, H.J.; Lefèvre, H.C. Sagnac Effect in Fiber Gyroscopes. Opt. Lett. 1981, 6, 401–403. [Google Scholar] [CrossRef]
- Iwatsuki, K.; Hotate, K.; Higashiguchi, M. Effect of Rayleigh Backscattering in an Optical Passive Ring-Resonator Gyro. Appl. Opt. 1984, 23, 3916. [Google Scholar] [CrossRef]
- Hotate, K.; Takiguchi, K.; Hirose, A. Adjustment-Free Method to Eliminate the Noise Induced by the Backscattering in an Optical Passive Ring-Resonator Gyro. IEEE Photonics Technol. Lett. 1990, 2, 75–77. [Google Scholar] [CrossRef]
- Yu, H.; Zhang, C.; Feng, L.; Hong, L.; Lei, M. Limitation of Rotation Sensing in IORG by Rayleigh Backscattering Noise. Europhys. Lett. 2011, 95, 64001. [Google Scholar] [CrossRef]
- Ma, H.; He, Z.; Hotate, K. Reduction of Backscattering Induced Noise by Carrier Suppression in Waveguide-Type Optical Ring Resonator Gyro. J. Lightwave Technol. 2011, 29, 85–90. [Google Scholar] [CrossRef]
- He, Y.; Yang, F.; Yan, W.; Li, Z. Phase Modulation Techniques for Suppressing Backscattering Noise in Resonator Integrated Optic Gyroscopes. Chin. Opt. 2019, 12, 1403–1417. [Google Scholar] [CrossRef]
- Mao, H.; Ma, H.; Jin, Z. Polarization Maintaining Silica Waveguide Resonator Optic Gyro Using Double Phase Modulation Technique. Opt. Express 2011, 19, 4632–4643. [Google Scholar] [CrossRef] [PubMed]
- Yu, H.; Zhang, C.; Feng, L.; Hong, L.; Liu, H.; Wang, J. Rayleigh Backscatter Noise in Integrated Optical Resonance Gyro. Optik 2012, 123, 1364–1369. [Google Scholar] [CrossRef]
- Nakazawa, M. Rayleigh Backscattering Theory for Single-Mode Optical Fibers. J. Opt. Soc. Am. 1983, 73, 1175–1180. [Google Scholar] [CrossRef]
- Zhi, Y.; Feng, L.; Wang, J.; Tang, Y. Reduction of Backscattering Noise in a Resonator Integrated Optic Gyro by Double Triangular Phase Modulation. Appl. Opt. 2015, 54, 114. [Google Scholar] [CrossRef]
- Ma, H.; Wang, W.; Ren, Y.; Jin, Z. Low-Noise Low-Delay Digital Signal Processor for Resonant Micro Optic Gyro. IEEE Photonics Technol. Lett. 2013, 25, 198–201. [Google Scholar] [CrossRef]
- Wang, H.; Liu, W.; Pan, Z.; Tao, Y.; Niu, J.; Tang, J.; Liu, J. Suppression of Backscattering-Induced Noise in a Resonator Optic Gyro by the Dual-Frequency Modulation Method. Opt. Commun. 2020, 459, 124766. [Google Scholar] [CrossRef]
- Mao, H.; Ma, H.; Jin, Z. Resonator Micro-Optic Gyroscope Based on the Double Phase Modulation Technique. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CL, USA, 16–21 May 2010. [Google Scholar]
- Niu, J.; Liu, W.; Pan, Z.; Tao, Y.; Zhou, Y.; Xing, E.; Tang, J.; Liu, J. The Noise Suppression in Resonant Micro Optic Gyroscopes Based on Dual Light Sources Method. Opt. Commun. 2021, 488, 126839. [Google Scholar] [CrossRef]
- Fei, Y.; He, Y.-M.; Li, Z.-F.; Yang, F.-H.; Liu, W. Backreflections in Resonant Micro-Optic Gyroscope. J. Phys. Commun. 2018, 2, 095010. [Google Scholar] [CrossRef]
- Feng, L.; Lei, M.; Liu, H.; Zhi, Y.; Wang, J. Suppression of Backreflection Noise in a Resonator Integrated Optic Gyro by Hybrid Phase-Modulation Technology. Appl. Opt. 2013, 52, 1668. [Google Scholar] [CrossRef]
- Wang, J.; Feng, L.; Wang, Q.; Wang, X.; Jiao, H. Reduction of Angle Random Walk by In-Phase Triangular Phase Modulation Technique for Resonator Integrated Optic Gyro. Opt. Express 2016, 24, 5463. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Feng, L.; Zhi, Y.; Liu, H.; Wang, W.; Lei, M. Reduction of Backreflection Noise in Resonator Micro-Optic Gyro by Integer Period Sampling. Appl. Opt. 2013, 52, 7712–7717. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Feng, L.; Li, H.; Wang, X.; Jia, Y.; Liu, D. Enhanced Differential Detection Technique for the Resonator Integrated Optic Gyro. Opt. Lett. 2018, 43, 2941. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Feng, L.; Wang, Q.; Jiao, H.; Wang, X. Suppression of Backreflection Error in Resonator Integrated Optic Gyro by the Phase Difference Traversal Method. Opt. Lett. 2016, 41, 1586–1589. [Google Scholar] [CrossRef]
- Iwatsuki, K.; Hotate, K.; Higashiguchi, M. Kerr Effect in an Optical Passive Ring-Resonator Gyro. J. Lightwave Technol. 1986, 4, 645–651. [Google Scholar] [CrossRef]
- Takiguchi, K.; Hotate, K. Partially Digital-Feedback Scheme and Evaluation of Optical Kerr-Effect Induced Bias in Optical Passive Ring-Resonator Gyro. IEEE Photonics Technol. Lett. 1991, 3, 679–681. [Google Scholar] [CrossRef]
- Takiguchi, K.; Hotate, K. Method to Reduce the Optical Kerr-Effect-Induced Bias in an Optical Passive Ring-Resonator Gyro. IEEE Photonics Technol. Lett. 1992, 4, 203–206. [Google Scholar] [CrossRef]
- Ciminelli, C.; Dell’Olio, F.; Armenise, M.N. High-Q Spiral Resonator for Optical Gyroscope Applications: Numerical and Experimental Investigation. IEEE Photonics J. 2012, 4, 1844–1854. [Google Scholar] [CrossRef]
- Li, X.; Zhang, J.; Ma, H.; Jin, Z. Test and Analysis of the Optical Kerr-Effect in Resonant Micro-Optic Gyros. IEEE Photonics J. 2014, 6, 1–7. [Google Scholar] [CrossRef]
- Yin, S.; Liu, W.; Xing, E.; Pan, Z.; Tao, Y.; Zhu, J.; Tang, J.; Liu, J. Suppression of Laser Intensity Fluctuation in Resonator Optical Gyro by a Simple Light Intensity Feedback Technique. Opt. Eng. 2020, 59, 036112. [Google Scholar] [CrossRef]
- Ma, H.; Li, X.; Zhang, G.; Jin, Z. Reduction of Optical Kerr-Effect Induced Error in a Resonant Micro-Optic Gyro by Light-Intensity Feedback Technique. Appl. Opt. 2014, 53, 3465. [Google Scholar] [CrossRef] [PubMed]
- Niu, J.; Liu, W.; Pan, Z.; Tao, Y.; Zhou, Y.; Xing, E.; Liu, J.; Tang, J. Reducing Backscattering and the Kerr Noise in a Resonant Micro-Optic Gyro Using Two Independent Lasers. Appl. Opt. 2021, 60, 2761. [Google Scholar] [CrossRef] [PubMed]
- Bergh, R.A.; Lefevre, H.C.; Shaw, H.J. Compensation of the Optical Kerr Effect In Fiber-Optic Gyroscopes. Opt. Lett. 1982, 7, 282–284. [Google Scholar] [CrossRef] [PubMed]
- Iwatsuki, K.; Hotate, K.; Higashiguchi, M. Eigenstate of Polarization in a Fiber Ring Resonator and Its Effect in an Optical Passive Ring-Resonator Gyro. Appl. Opt. 1986, 25, 2606. [Google Scholar] [CrossRef]
- Tench, R.; Delavaux, J.-M.; Tzeng, L.; Smith, R.; Buhl, L.; Alferness, R. Performance Evaluation of Waveguide Phase Modulators for Coherent Systems at 1.3 and 1.5 Μm. J. Lightwave Technol. 1987, 5, 492–501. [Google Scholar] [CrossRef]
- Wang, X.; He, Z.; Hotate, K. Reduction of Polarization-Fluctuation Induced Drift in Resonator Fiber Optic Gyro by a Resonator with Twin 90° Polarization-Axis Rotated Splices. Opt. Express 2010, 18, 1677. [Google Scholar] [CrossRef]
- Yan, Y.; Chen, Y.; Ma, H.; Jin, Z. Polarization-Fluctuation Induced Drift in Resonator Micro Optic Gyro. In Proceedings of the 2012 Asia Communications and Photonics Conference (ACP), Guangzhou, China, 7–10 November 2012; IEEE: Piscataway, NJ, USA, 2012. [Google Scholar]
- Ma, H.; Yu, X.; Jin, Z. Reduction of Polarization-Fluctuation Induced Drift in Resonator Fiber Optic Gyro by a Resonator Integrating in-Line Polarizers. Opt. Lett. 2012, 37, 3342. [Google Scholar] [CrossRef]
- Ma, H.; Yan, Y.; Chen, Y.; Jin, Z. Improving Long-Term Stability of a Resonant Micro-Optic Gyro by Reducing Polarization Fluctuation. IEEE Photonics J. 2012, 4, 2372–2381. [Google Scholar] [CrossRef]
- Yu, X.; Ma, H.; Jin, Z. Improving Thermal Stability of a Resonator Fiber Optic Gyro Employing a Polarizing Resonator. Opt. Express 2013, 21, 358. [Google Scholar] [CrossRef]
- Liang, N.; Lijun, G.; Mei, K.; Tuoyuan, C. Waveguide-Type Optical Passive Ring Resonator Gyro Using Frequency Modulation Spectroscopy Technique. J. Semicond. 2014, 35, 124008. [Google Scholar]
- Ma, H.; Zhang, J.; Chen, Z.; Jin, Z. Tilted Waveguide Gratings and Implications for Optical Waveguide-Ring Resonator. J. Lightwave Technol. 2015, 33, 4176–4183. [Google Scholar] [CrossRef]
- Yan, Y.; Ma, H.; Jin, Z. Reducing Polarization-Fluctuation Induced Drift in Resonant Fiber Optic Gyro by Using Single-Polarization Fiber. Opt. Express 2015, 23, 2002. [Google Scholar] [CrossRef] [PubMed]
- Posner, M.T.; Mennea, P.L.; Podoliak, N.; Horak, P.; Gates, J.C.; Smith, P.G.R. Integrated Polarizing Coupler Based on Tilted Gratings. In Proceedings of the Advanced Photonics 2016 (IPR, NOMA, Sensors, Networks, SPPCom, SOF), Optical Society of America, Vancouver, NA, Canada, 18–20 July 2016; p. IW3B.3. [Google Scholar]
- Zhang, J.; Ma, H.; Li, H.; Jin, Z. Single-Polarization Fiber-Pigtailed High-Finesse Silica Waveguide Ring Resonator for a Resonant Micro-Optic Gyroscope. Opt. Lett. 2017, 42, 3658. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; Zhang, J.; Ma, H.; Jin, Z. Evaluation of Polarization Characteristics of the Fiber-Pigtailed Waveguide-Type Ring Resonator and Implications for Resonant Micro-Optic Gyroscopes. J. Lightwave Technol. 2019, 37, 2425–2434. [Google Scholar] [CrossRef]
- Ma, H.; Yan, Y.; Wang, L.; Chang, X.; Jin, Z. Laser Frequency Noise Induced Error in Resonant Fiber Optic Gyro Due to an Intermodulation Effect. Opt. Express 2015, 23, 25474. [Google Scholar] [CrossRef] [PubMed]
- Duan, R.; Feng, L.; Jiao, H.; Wang, X. Research on Reducing the Influence of Laser Frequency Noise on Resonator Optical Gyro. IEEE Sens. J. 2017, 17, 2422–2427. [Google Scholar] [CrossRef]
- Zhang, G.; Yu, Z.; Xu, Z.; Jin, Z. Laser Frequency Noise Measurement in a Resonant Fiber Optic Gyro. Optik 2018, 175, 296–303. [Google Scholar] [CrossRef]
- Yang, T.; Fei, Y.; Li, Z.; Wen, L.; Yan, L.; Wang, X.; Yang, F. Comparison between Interferometric and Resonant Integrated Optic Gyroscopes. Laser Optoelectron. Prog. 2016, 53, 080601. [Google Scholar] [CrossRef]
- Xie, C.; Tang, J.; Cui, D.; Wu, D.; Zhang, C.; Li, C.; Zhen, Y.; Xue, C.; Liu, J. Resonant Microsphere Gyroscope Based on a Double Faraday Rotator System. Opt. Lett. 2016, 41, 4783. [Google Scholar] [CrossRef]
- Xu, X.; Song, N.; Zhang, Z.; Jin, J. Backward Secondary-Wave Coherence Errors in Photonic Bandgap Fiber Optic Gyroscopes. Sensors 2016, 16, 851. [Google Scholar] [CrossRef] [Green Version]
- Jiao, H.; Feng, L.; Zhang, C.; Liu, N.; Zhang, Y.; Ma, H. Design of Low-Crosstalk Polarizing Resonator and Homologous Multi-Frequency Differential Detection for Hollow-Core Photonic-Crystal Fiber Optic Gyro. Opt. Express 2019, 27, 19536–19547. [Google Scholar] [CrossRef] [PubMed]
- Jiao, H.; Feng, L.; Liu, N.; Yang, Z. Improvement of Long-Term Stability of Hollow-Core Photonic-Crystal Fiber Optic Gyro Based on Single-Polarization Resonator. Opt. Express 2018, 26, 8645–8655. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Li, Y.; Ma, R.; Zhao, L.; Dong, X. Study on the High-Birefringence Hollow-Core Anti-Resonant Fiber with Semicircular Cladding. Int. J. Opt. 2021, 2021, 5520142. [Google Scholar] [CrossRef]
- Yang, K.Y.; Oh, D.Y.; Lee, S.H.; Yang, Q.-F.; Yi, X.; Shen, B.; Wang, H.; Vahala, K. Bridging Ultrahigh-Q Devices and Photonic Circuits. Nat. Photon. 2018, 12, 297–302. [Google Scholar] [CrossRef] [Green Version]
- Liang, W.; Ilchenko, V.S.; Savchenkov, A.A.; Dale, E.; Eliyahu, D.; Matsko, A.B.; Maleki, L. Resonant Microphotonic Gyroscope. Optica 2017, 4, 114–117. [Google Scholar] [CrossRef]
- Li, Z.; He, N.; Sun, X.; Jin, C.; Liu, C.; Wu, X. Analysis of Resonance Asymmetry Phenomenon in Resonant Fiber Optic Gyro. Sensors 2018, 18, 696. [Google Scholar] [CrossRef] [Green Version]
- Lei, M.; Feng, L.; Zhi, Y.; Liu, H.; Wang, J.; Ren, X.; Su, N. Current Modulation Technique Used in Resonator Micro-Optic Gyro. Appl. Opt. 2013, 52, 307–313. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, S.; Liu, Z.; Zhang, X.; He, Y.; Ma, Y.; Zhang, Y.; Zhang, Z.; Liu, Y. High-Frequency Characterization of High-Speed Modulators and Photodetectors in a Link with Low-Speed Photonic Sampling. J. Semicond. 2021, 42, 042303. [Google Scholar] [CrossRef]
- Yang, Z.; Jin, X.; Ma, H.; Jin, Z. CORDIC Algorithm Based Digital Detection Technique Applied in Resonator Fiber Optic Gyroscope. Opt. Fiber Technol. 2009, 15, 328–331. [Google Scholar] [CrossRef]
- Hotate, K.; Harumoto, M. Resonator Fiber Optic Gyro Using Digital Serrodyne Modulation. J. Lightwave Technol. 1997, 15, 466–473. [Google Scholar] [CrossRef]
- Jin, Z.; Yu, X.; Ma, H. Closed-Loop Resonant Fiber Optic Gyro with an Improved Digital Serrodyne Modulation. Opt. Express 2013, 21, 26578. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Kishi, M.; He, Z.; Hotate, K. Resonator Fiber Optic Gyro with Bipolar Digital Serrodyne Modulation Scheme. In 22nd International Conference on Optical Fiber Sensors, Pts 1–3; Liao, Y., Jin, W., Sampson, D.D., Yamauchi, R., Chung, Y., Nakamura, K., Rao, Y., Eds.; International Society for Optics and Photonics: Bellingham, WA, USA, 2012; Volume 8421, p. 84210A. [Google Scholar]
- Zhu, J.; Liu, W.; Pan, Z.; Tao, Y.; Yin, S.; Tang, J.; Liu, J. Combined Frequency-Locking Technology of a Digital Integrated Resonator Optic Gyroscope with a Phase-Modulated Feedback Loop. Appl. Opt. 2019, 58, 9914. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Feng, L.; Tang, Y.; Zhi, Y. Resonator Integrated Optic Gyro Employing Trapezoidal Phase Modulation Technique. Opt. Lett. 2015, 40, 155. [Google Scholar] [CrossRef] [PubMed]
- Lei, M.; Feng, L.; Zhi, Y. Impact of Inflection Points on the Performance of Resonator Integrated Optic Gyro. Optik 2014, 125, 508–510. [Google Scholar] [CrossRef]
- Takahashi, M.; Tai, S.; Kyuma, K.; Hamanaka, K. Effect of Reflections on the Drift Characteristics of a Fiber-Optic Passive Ring-Resonator Gyro. Opt. Lett. 1988, 13, 413. [Google Scholar] [CrossRef]
- Arditty, H.; Shaw, H.J.; Chodorow, M.; Kompfner, R. Re-Entrant Fiberoptic Approach To Rotation Sensing. In Laser Inertial Rotation Sensors; Ezekiel, S., Knausenberger, G.E., Eds.; SPIE: Bellingham, WA, USA, 1978; pp. 138–148. [Google Scholar]
- Lefèvre, H.C. The Fiber-Optic Gyroscope: Achievement and Perspective. Gyroscopy Navig. 2012, 3, 223–226. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light, 7th expanded ed.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1999; ISBN 978-0-521-64222-4. [Google Scholar]
- Zhang, X.; Zhou, K. Open-Loop Experiments of Resonator Micro-Optic Gyro. Optoelectron. Lett. 2009, 5, 97–100. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, C.; Gao, S.; Wang, T.; Lin, T.; Li, X. Application of Fast Dynamic Allan Variance for the Characterization of FOGs-Based Measurement While Drilling. Sensors 2016, 16, 2078. [Google Scholar] [CrossRef] [Green Version]
- Tan, M.; Xu, X.; Wu, J.; Nguyen, T.G.; Chu, S.T.; Little, B.E.; Morandotti, R.; Mitchell, A.; Moss, D.J. Photonic Radio Frequency Channelizers Based on Kerr Optical Micro-Combs. J. Semicond. 2021, 42, 041302. [Google Scholar] [CrossRef]
- Ezekiel, S.; Hellwarth, R.W.; Davis, J.L. Observation of Intensity-Induced Nonreciprocity in a Fiber-Optic Gyroscope. Opt. Lett. 1982, 7, 457. [Google Scholar] [CrossRef]
- Philen, D.L.; White, I.A.; Kuhl, J.F.; Mettler, S.C. Single-Mode Fiber OTDR: Experiment and Theory. IEEE Trans. Microw. Theory Tech. 1982, 30, 1487–1496. [Google Scholar] [CrossRef]
- Jin, Z.; Zhang, G.; Mao, H.; Ma, H. Resonator Micro Optic Gyro with Double Phase Modulation Technique Using an FPGA-Based Digital Processor. Opt. Commun. 2012, 285, 645–649. [Google Scholar] [CrossRef]
- Ren, Y.; Jin, Z.; Chen, Y.; Ma, H. Optimization of the Resonant Frequency Servo Loop Technique in the Resonator Micro Optic Gyro. J. Zhejiang Univ.-Sci. C 2011, 12, 942–950. [Google Scholar] [CrossRef]
- Strandjord, L.K.; Sanders, G.A. Resonator Fiber Optic Gyro Employing a Polarization-Rotating Resonator. In Fiber Optic Gyros: 15th Anniversary Confence; International Society for Optics and Photonics: Bellingham, WA, USA, 1992; Volume 1585, pp. 163–172. [Google Scholar] [CrossRef]
- Suzuki, K.; Kaneko, T.; Yoshida, H.; Obi, Y.; Fujimori, H.; Morita, H. Crystal Structure and Magnetic Properties of the Compound MnN. J. Alloy. Compd. 2000, 306, 66–71. [Google Scholar] [CrossRef]
- Kaiser, T.J.; Cardarelli, D.; Walsh, J.G. Experimental Developments in the RFOG. In Fiber Optic and Laser Sensors VIII; International Society for Optics and Photonics: Bellingham, WA, USA, 1991; Volume 1367, pp. 121–126. [Google Scholar]
- Khial, P.P.; White, A.D.; Hajimiri, A. Nanophotonic Optical Gyroscope with Reciprocal Sensitivity Enhancement. Nat. Photonics 2018, 12, 671–675. [Google Scholar] [CrossRef] [Green Version]
- Lei, M.; Feng, L.; Zhi, Y.; Liu, H. Effect of Intensity Variation of Laser in Resonator Integrated Optic Gyro. Appl. Opt. 2013, 52, 4576–4581. [Google Scholar] [CrossRef]
- Qiu, T.; Wu, J.; Strandjord, L.K.; Sanders, G.A. Performance of Resonator Fiber Optic Gyroscope Using External-Cavity Laser Stabilization and Optical Filtering. In 23rd International Conference on Optical Fibre Sensors; López-Higuera, J.M., Jones, J.D.C., López-Amo, M., Santos, J.L., Eds.; International Society for Optics and Photonics: Bellingham, WA, USA, 2014; p. 91570B. [Google Scholar]
- Li, H.; Ni, P.; Wang, Q.; Feng, C.; Feng, L. Analysis and Optimization of Dynamic Performance for Resonant Integrated Optical Gyroscope. J. Lightwave Technol. 2021, 39, 1858–1866. [Google Scholar] [CrossRef]
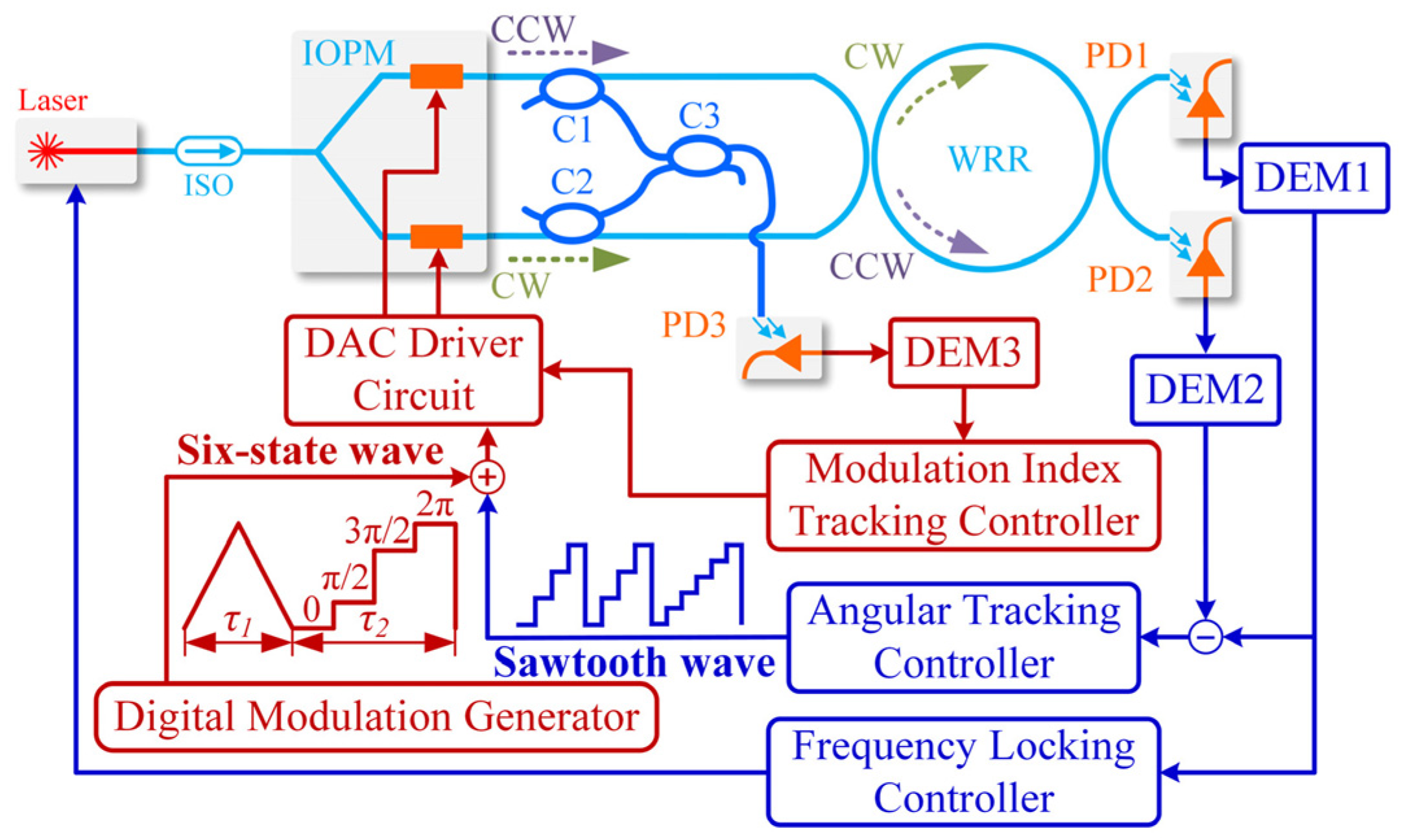
- Wang, Q.; Li, H.; Wang, P.; Deng, K.; Feng, L. Modulation Index Stabilization Technique of Integrated Optic Phase Modulator Used in Resonant Integrated Optic Gyro. Opt. Express 2019, 27, 3460–3471. [Google Scholar] [CrossRef]
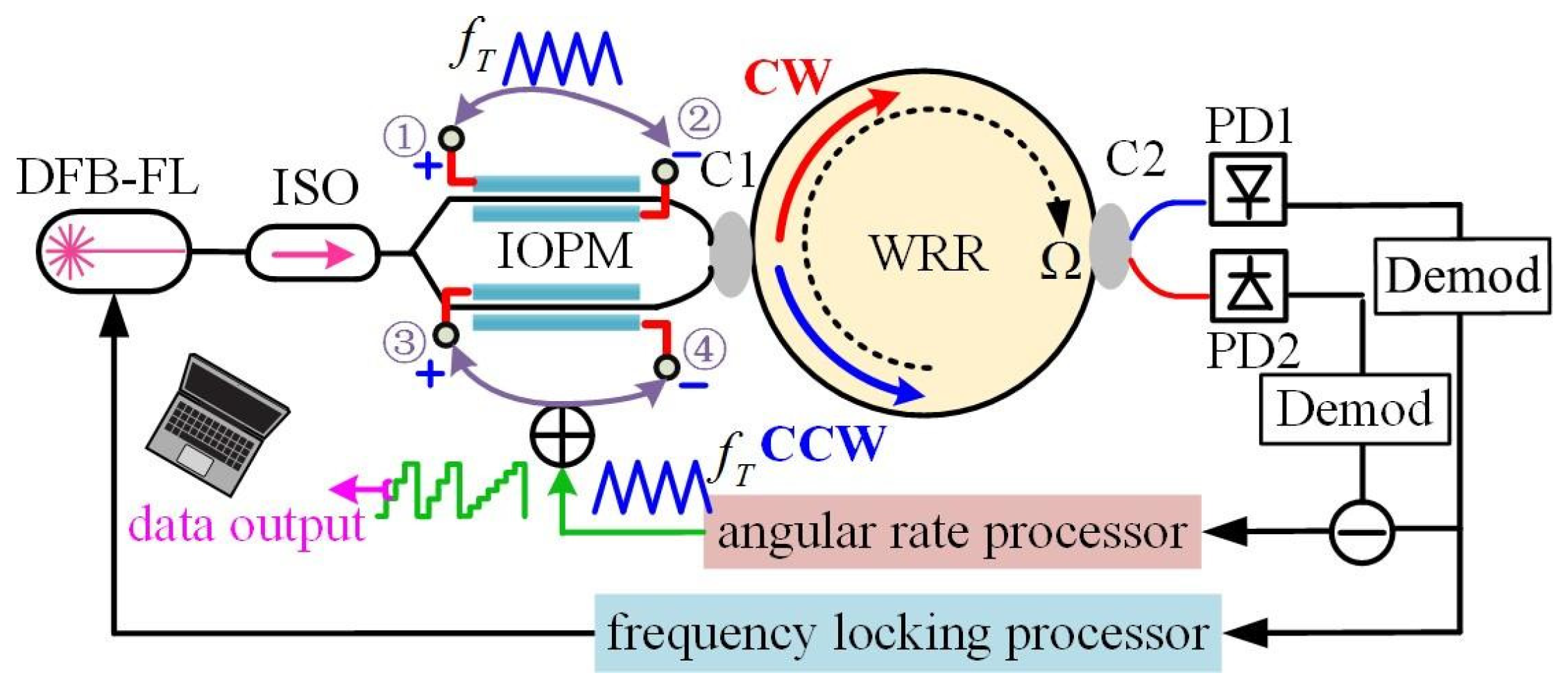
- Li, H.; Liu, L.; Lin, Z.; Wang, Q.; Wang, X.; Feng, L. Double Closed-Loop Control of Integrated Optical Resonance Gyroscope with Mean-Square Exponential Stability. Opt. Express 2018, 26, 1145. [Google Scholar] [CrossRef]
- Geng, J.; Yang, L.; Zhao, S.; Zhang, Y. Resonant Micro-Optical Gyro Based on Self-Injection Locking. Opt. Express 2020, 28, 32907–32915. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Y.; Wang, J.; Lai, J. A Survey of FPGA Design for AI Era. J. Semicond. 2020, 41, 021402. [Google Scholar] [CrossRef]
- Luo, C.; Sit, M.-K.; Fan, H.; Liu, S.; Luk, W.; Guo, C. Towards Efficient Deep Neural Network Training by FPGA-Based Batch-Level Parallelism. J. Semicond. 2020, 41, 022403. [Google Scholar] [CrossRef]
- Wu, C.; Lin, Y.; Ye, S.; Zhang, G.; Ma, H.; Jin, Z. Synchronous Digital Quadrature Demodulation Technique for Resonant Optic Gyroscopes. In Proceedings of the 2018 Asia Communications and Photonics Conference (ACP), Hangzhou, China, 26–29 October 2018; pp. 1–3. [Google Scholar]





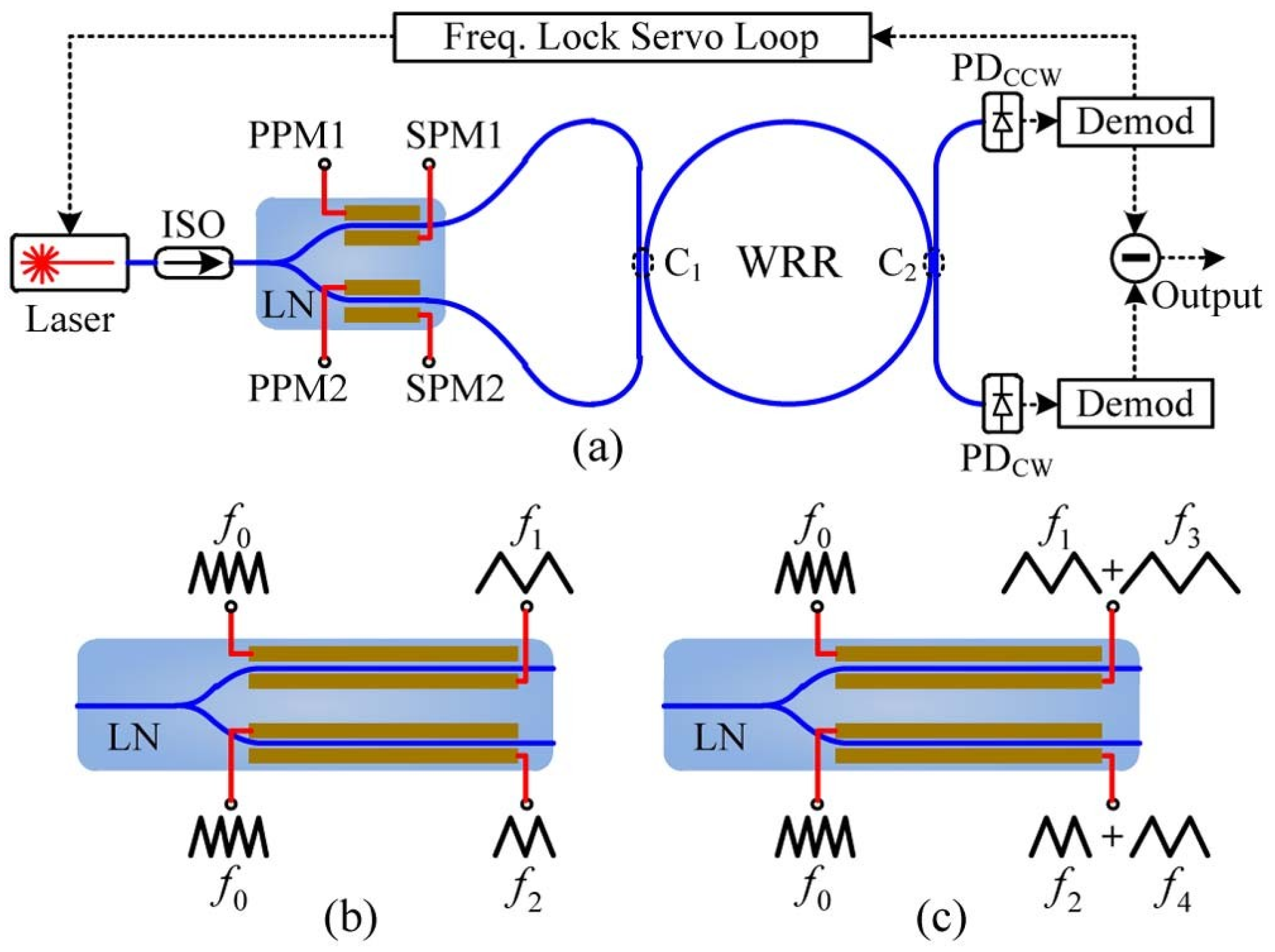

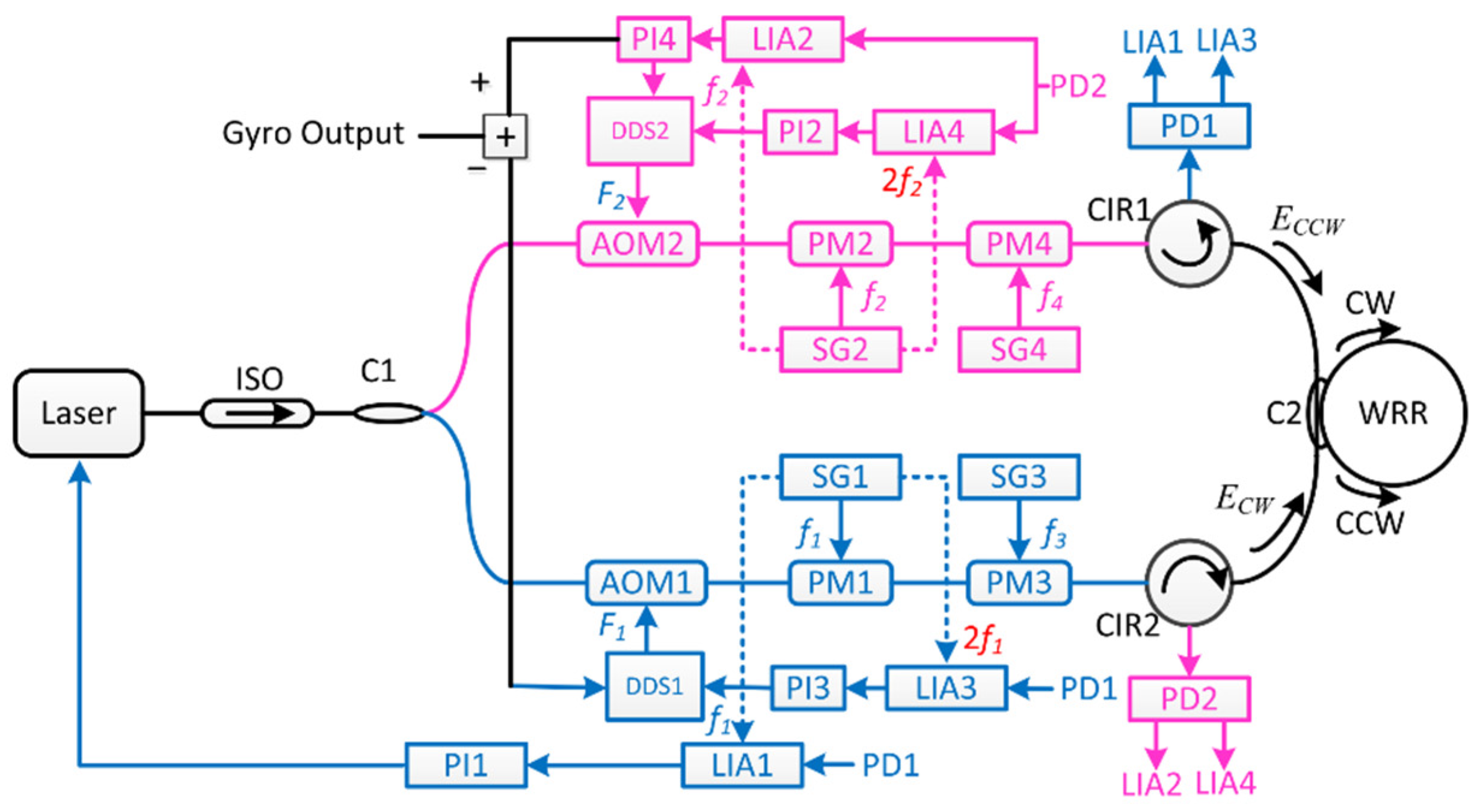
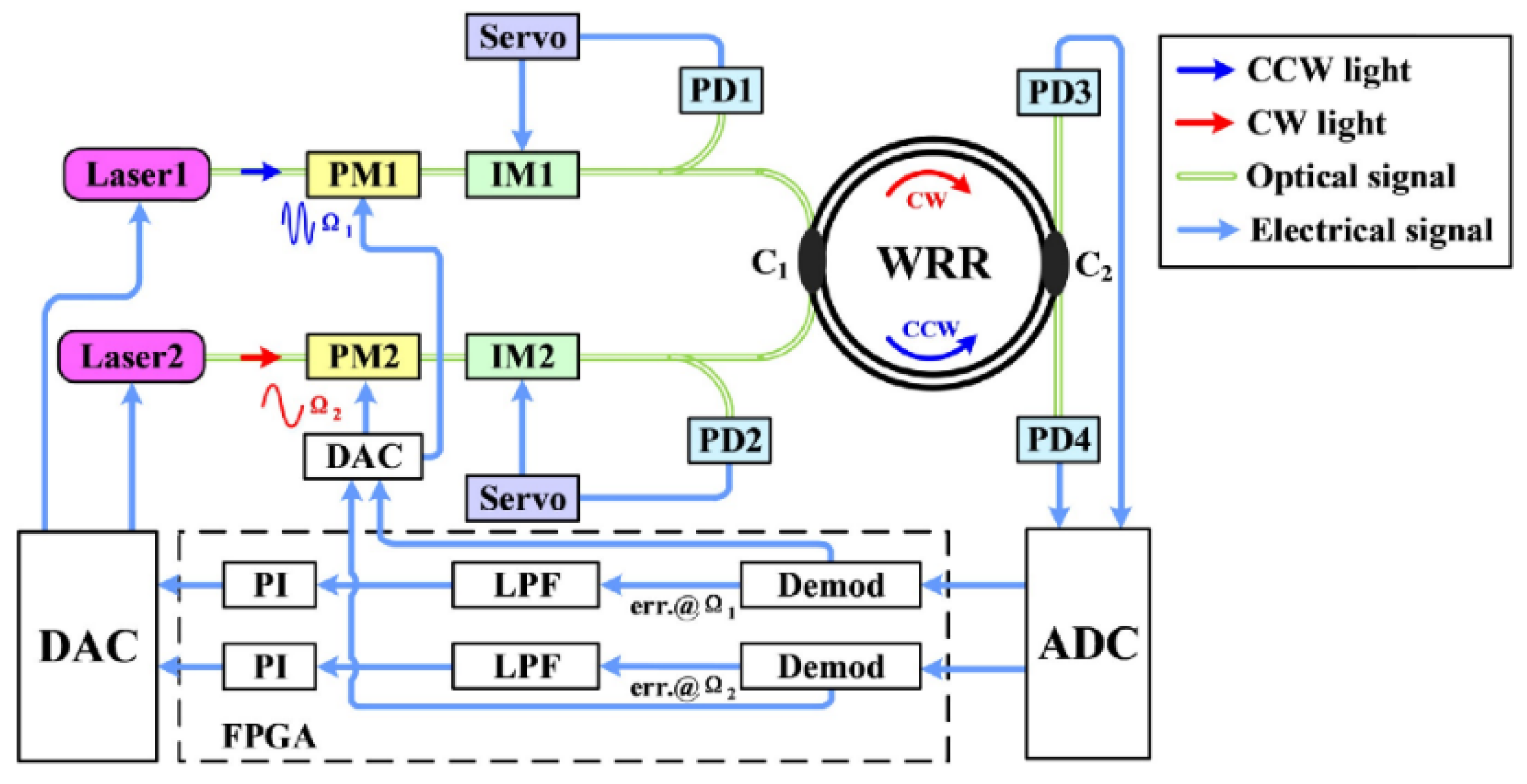
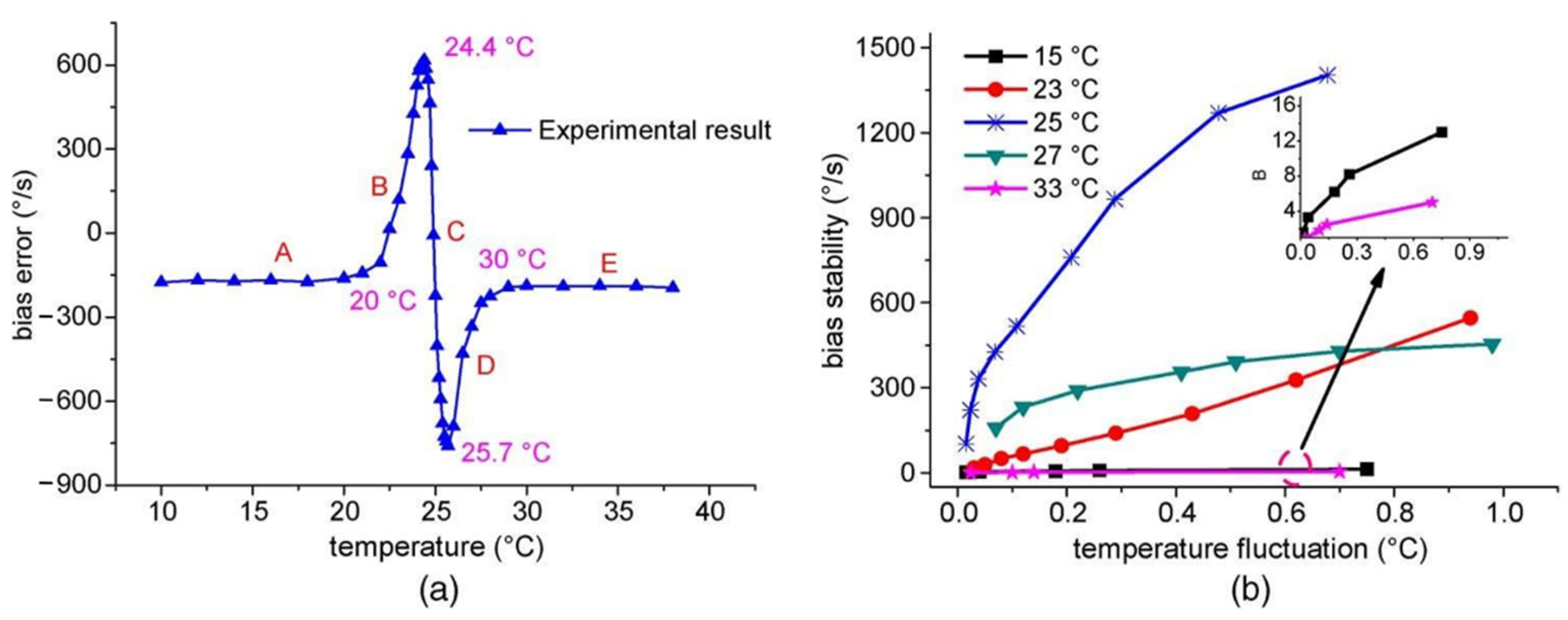




| Noise | Technology | Short-Term Bias Stability | Long-Term Bias Stability |
|---|---|---|---|
| backscattering noise | SPMT with triangular wave [12] | - | 0.71708°/s |
| SPMT with sinusoidal wave [13] | 0.46°/s | - | |
| DPMT with sinusoidal wave [21] | 3.14 × 10−3 rad/s | - | |
| dual light sources [22] | - | 0.00448°/s | |
| back-reflection noise | HPMT with triangular wave and sawtooth wave [24] | - | 0.22°/s |
| integer period sampling [26] | 0.067°/s | 0.41°/s | |
| enhanced differential detection technique [27] | - | 0.0029°/s | |
| phase difference traversal [28] | 0.0055°/s | 0.013°/s | |
| Kerr noise | light intensity feedback loop [34] | - | 16.94°/h |
| HPMT and the light intensity feedback loop [35] | - | - | |
| dual light sources and light intensity feedback loop [36] | - | 9.06°/h | |
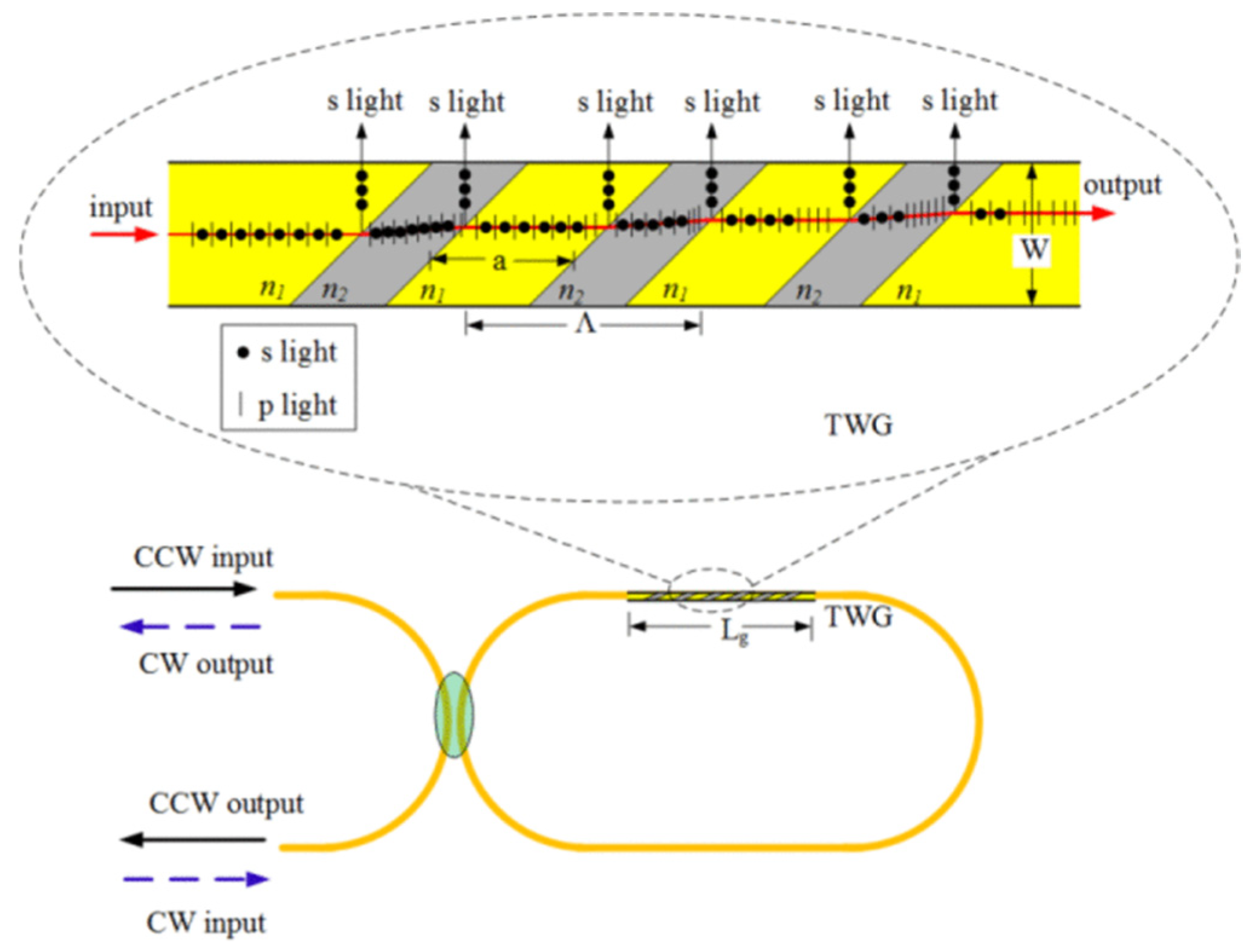
| polarization noise | tilted waveguide gratings [46] | - | - |
| single-polarization fiber [49] | - | 0.004°/s | |
| reciprocal sensitivity enhancement [86] | - | - | |
| laser frequency noise | - | - | - |
| new noise suppression technology | closed-loop signal detection [89] | - | - |
| modulation index stabilization technique [90] | - | - | |
| double closed-loop control system [91] | - | 7.04°/h | |
| self-injection locking technique [92] | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuai, X.; Wei, L.; Yang, F.; Yan, W.; Li, Z.; Wang, X. Suppression Method of Optical Noises in Resonator-Integrated Optic Gyroscopes. Sensors 2022, 22, 2889. https://doi.org/10.3390/s22082889
Kuai X, Wei L, Yang F, Yan W, Li Z, Wang X. Suppression Method of Optical Noises in Resonator-Integrated Optic Gyroscopes. Sensors. 2022; 22(8):2889. https://doi.org/10.3390/s22082889
Chicago/Turabian StyleKuai, Xuebao, Lei Wei, Fuhua Yang, Wei Yan, Zhaofeng Li, and Xiaodong Wang. 2022. "Suppression Method of Optical Noises in Resonator-Integrated Optic Gyroscopes" Sensors 22, no. 8: 2889. https://doi.org/10.3390/s22082889
APA StyleKuai, X., Wei, L., Yang, F., Yan, W., Li, Z., & Wang, X. (2022). Suppression Method of Optical Noises in Resonator-Integrated Optic Gyroscopes. Sensors, 22(8), 2889. https://doi.org/10.3390/s22082889







