Analysis of Electromagnetic Properties of New Graphene Partial Discharge Sensor Electrode Plate Material
Abstract
:1. Introduction
2. Electromagnetic Properties
2.1. Electrical Conductivity
2.2. Dielectric Constant
2.3. Magnetic Permeability
2.4. Refractive Index
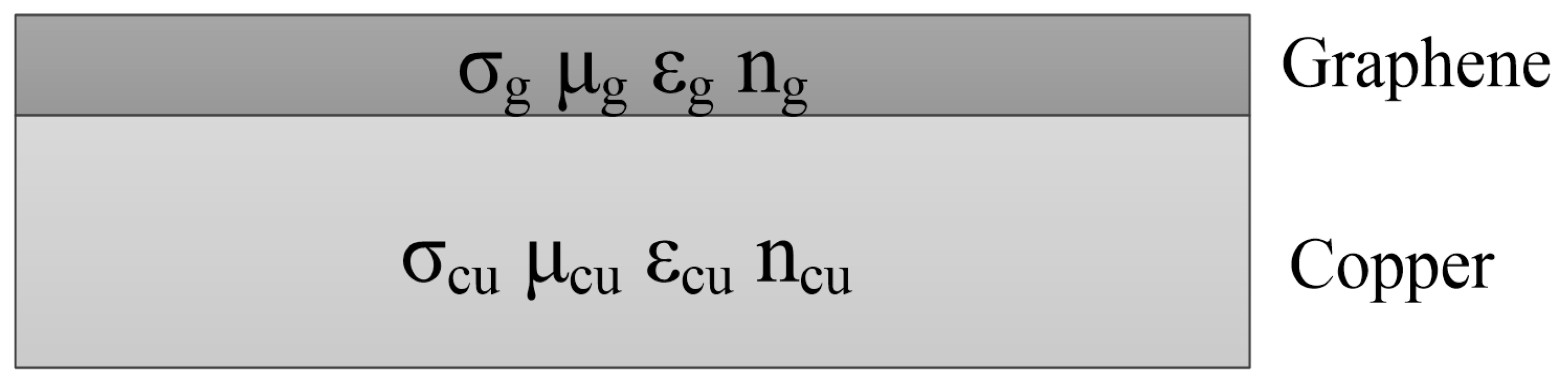
3. Electromagnetic Properties of Graphene Copper-Clad
3.1. Electromagnetic Properties of Graphene
3.1.1. Electrical Conductivity of Graphene
3.1.2. Dielectric Constant of Graphene
3.1.3. Magnetic Permeability and Refractive Index of Graphene
3.2. Electromagnetic Properties of Copper
4. Analysis of Influencing Factors
4.1. Scattering Rate
4.2. Electrochemical Potential
4.3. Temperature
4.4. Frequency
4.5. Factors Influencing the Electromagnetic Properties of Copper
5. Computational Analysis
5.1. Electrical Conductivity
5.2. Dielectric Constant
5.3. Refractive Index
6. Prediction Models of Electromagnetic Properties
6.1. Prediction Model of Conductivity
6.2. Prediction Model of Dielectric Constant
6.3. Prediction Model of Refractive Index
7. Simulation and Experimental Analysis
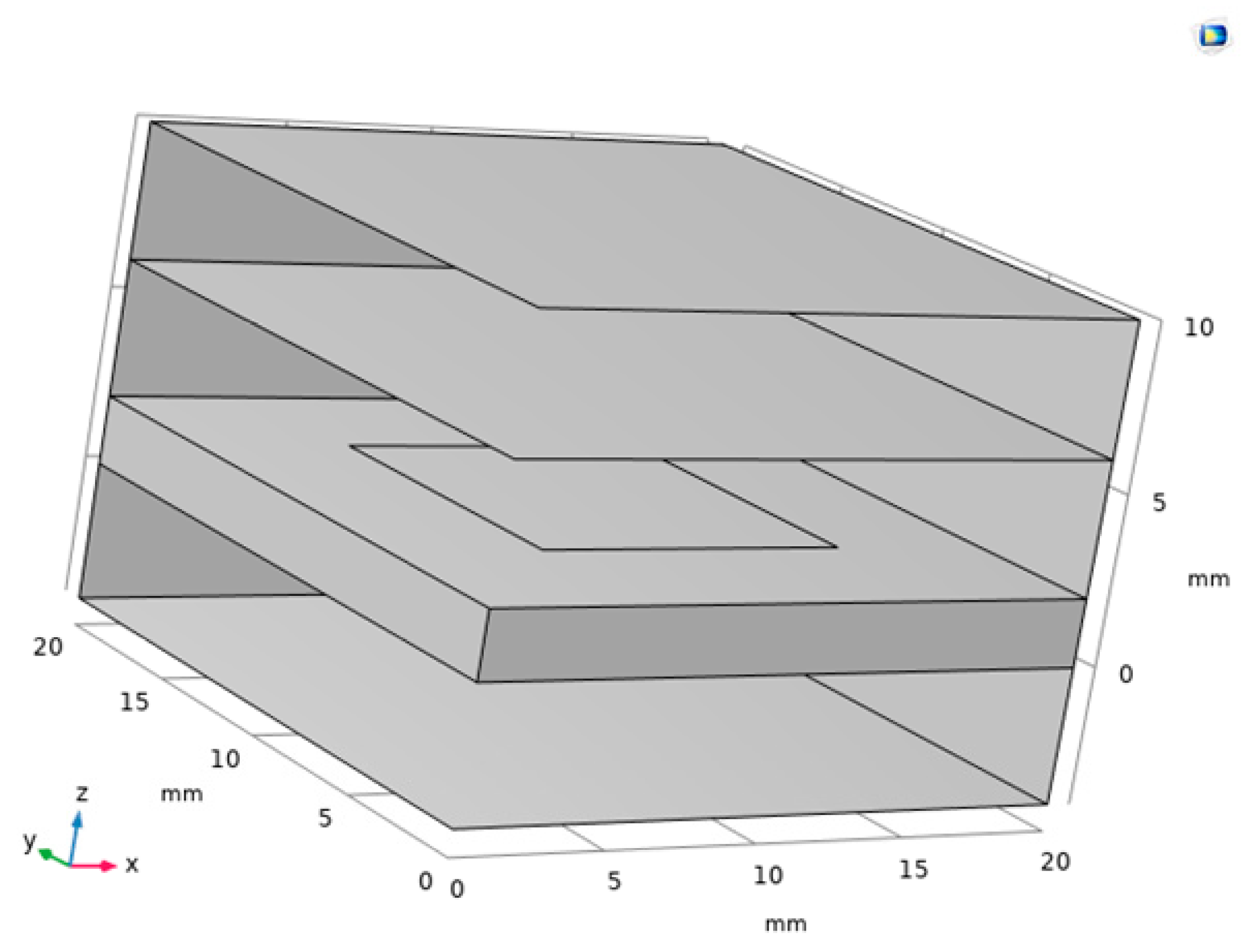
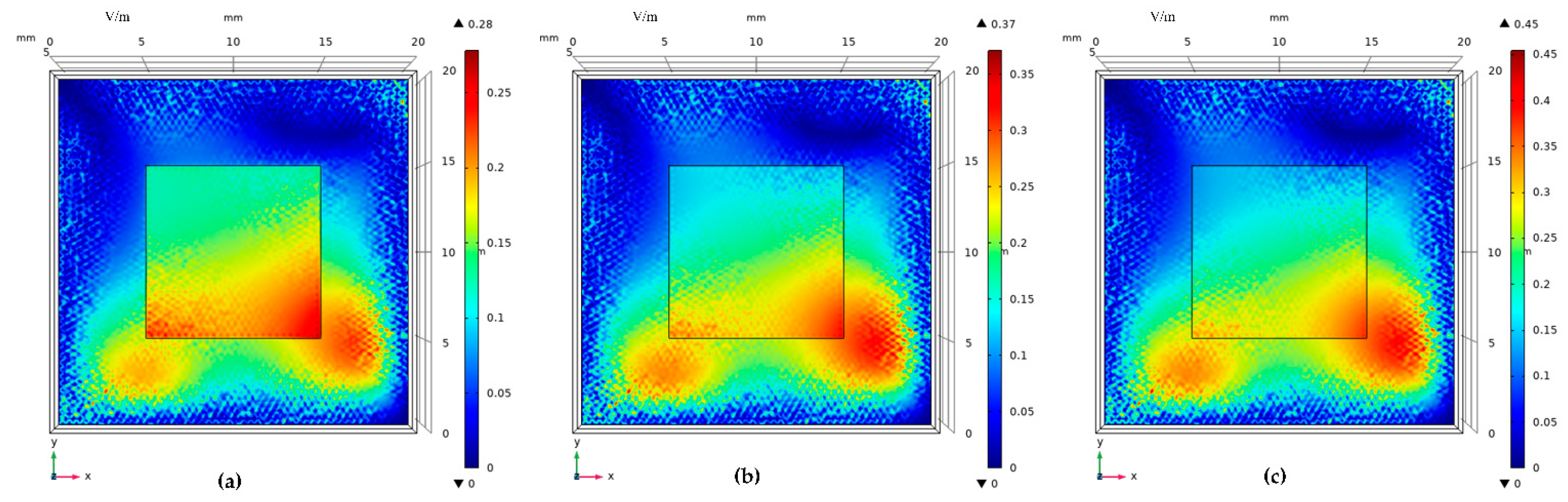
7.1. Simulation Analysis
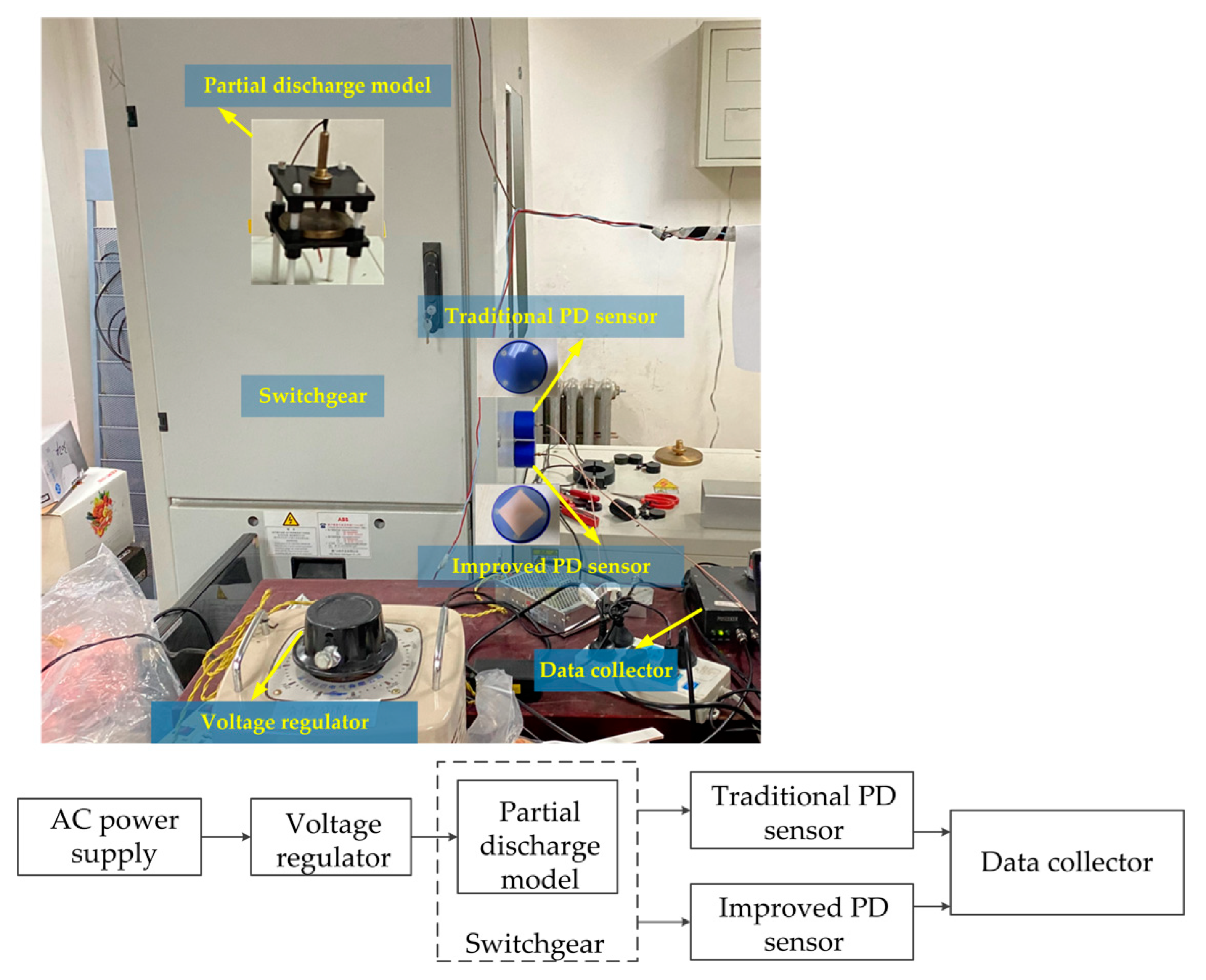
7.2. Experimental Analysis
8. Conclusions
- (1)
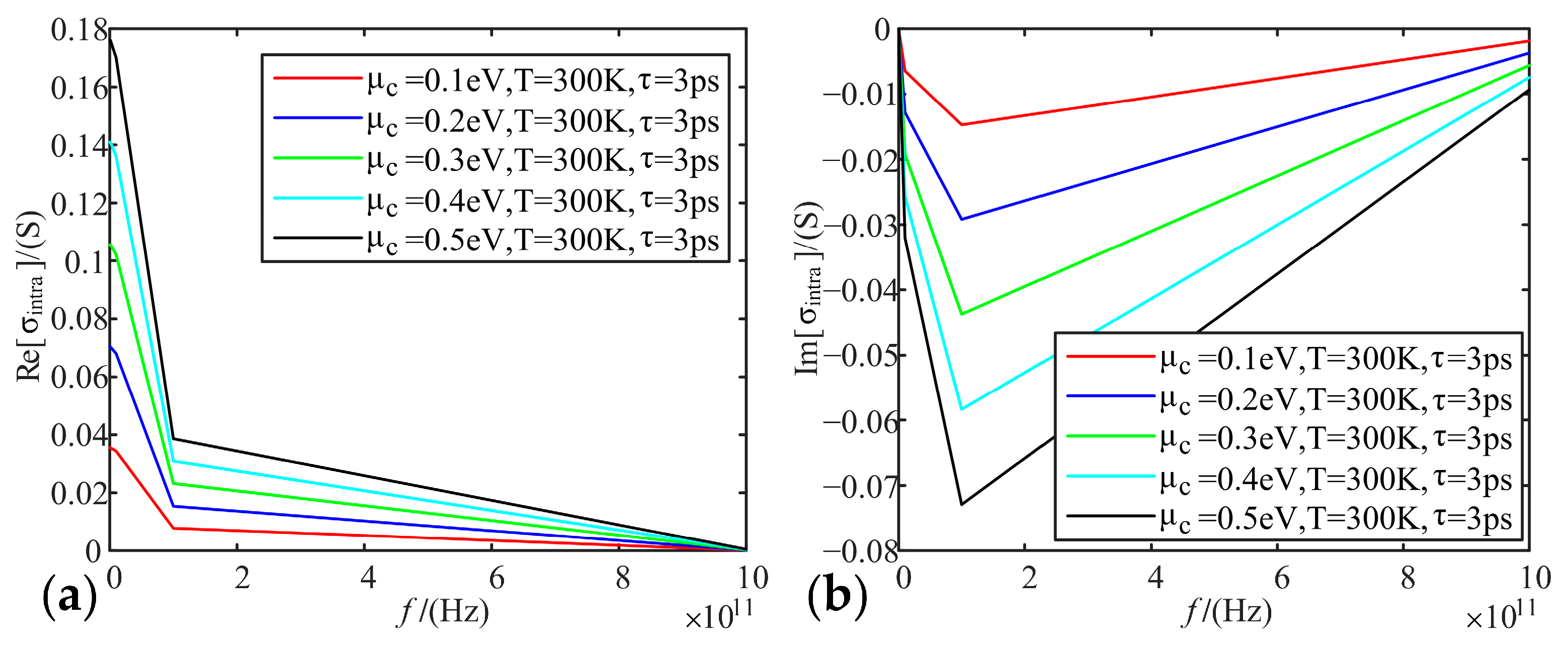
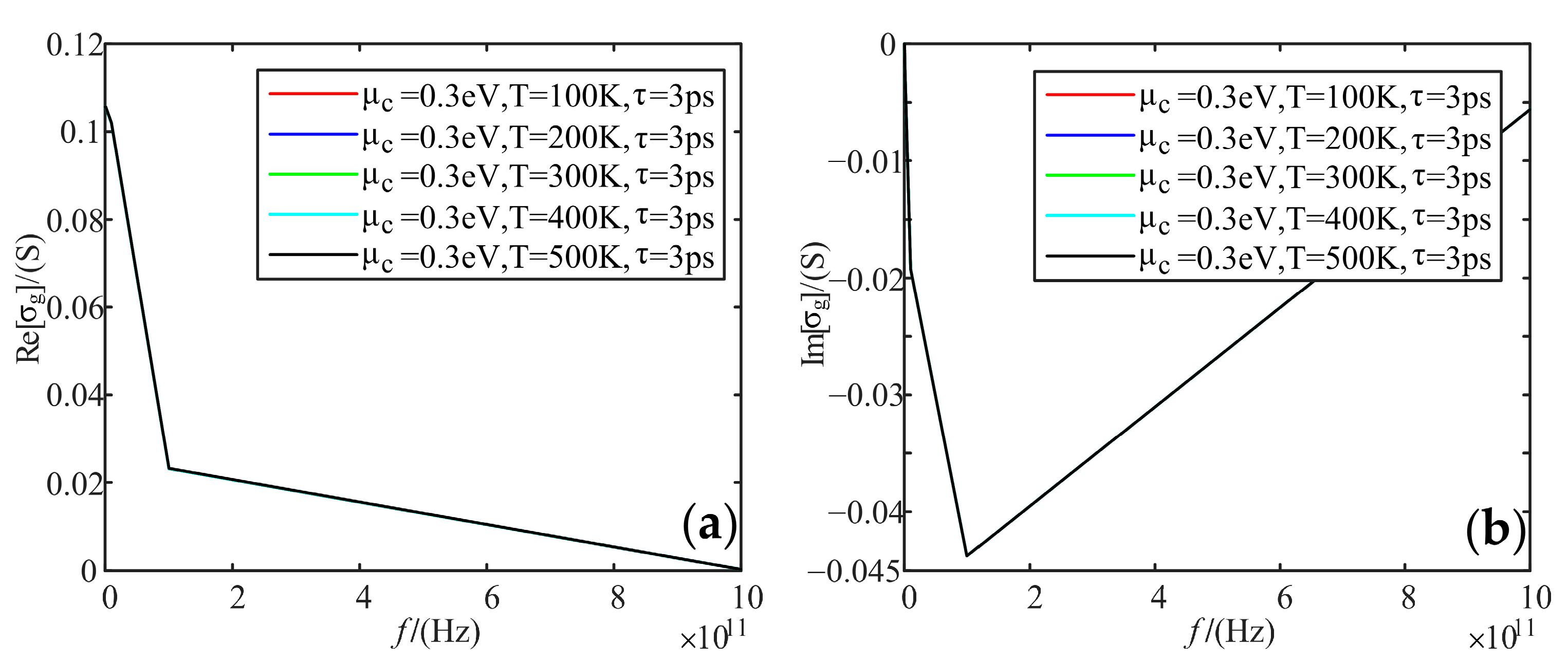
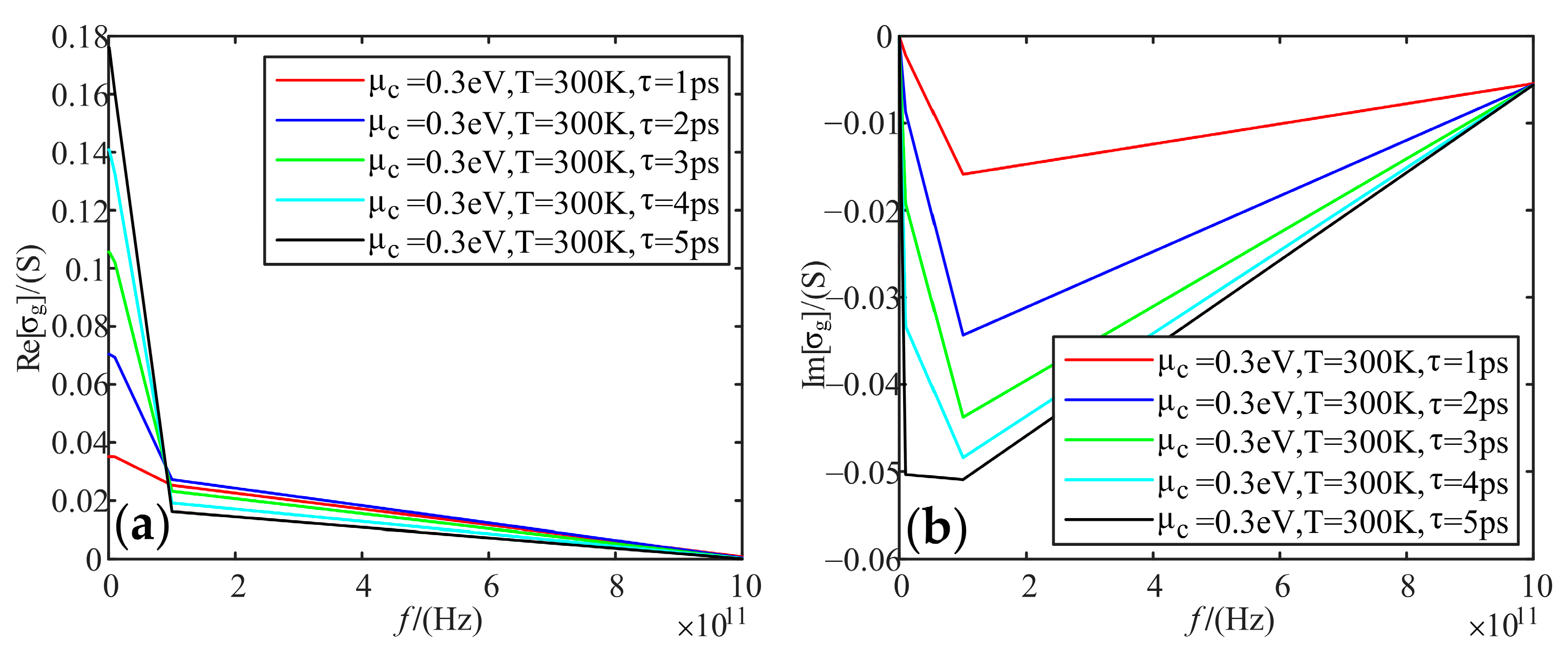
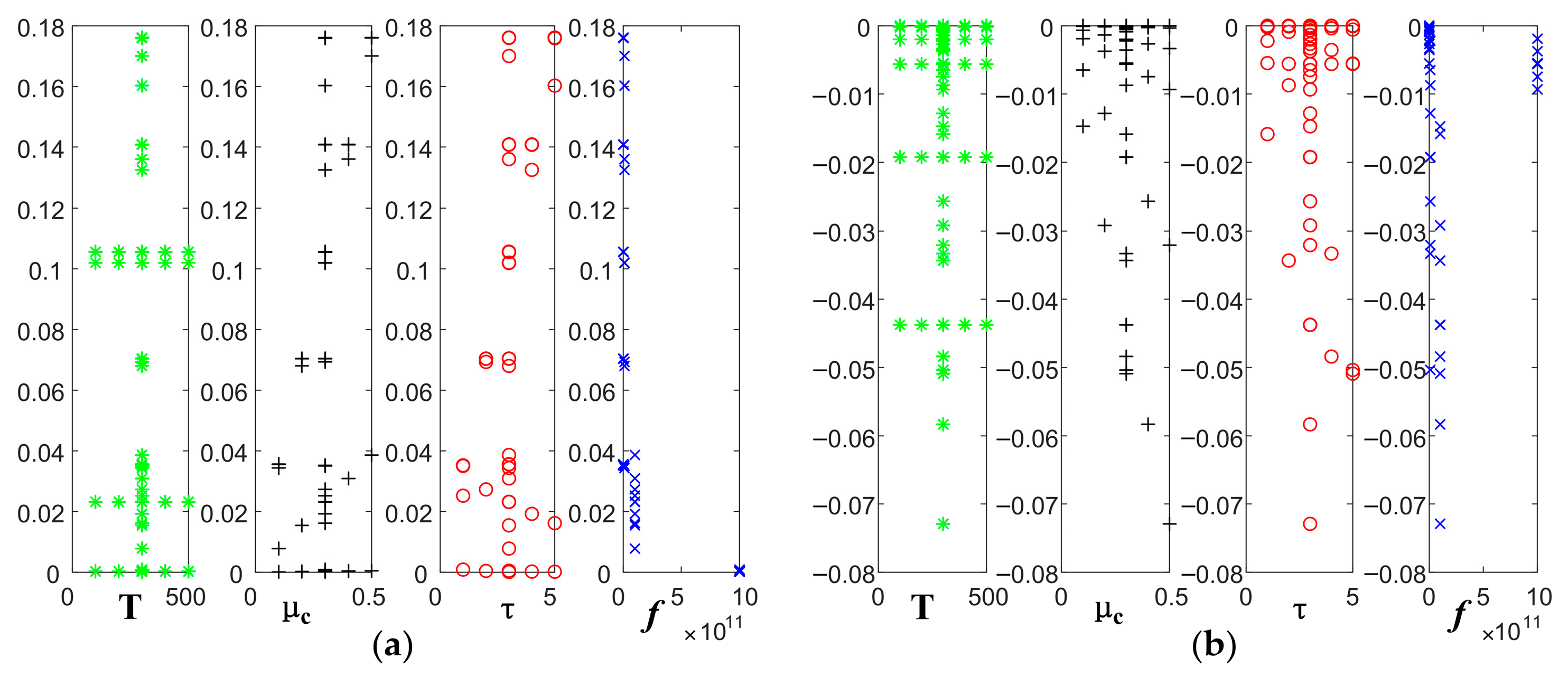
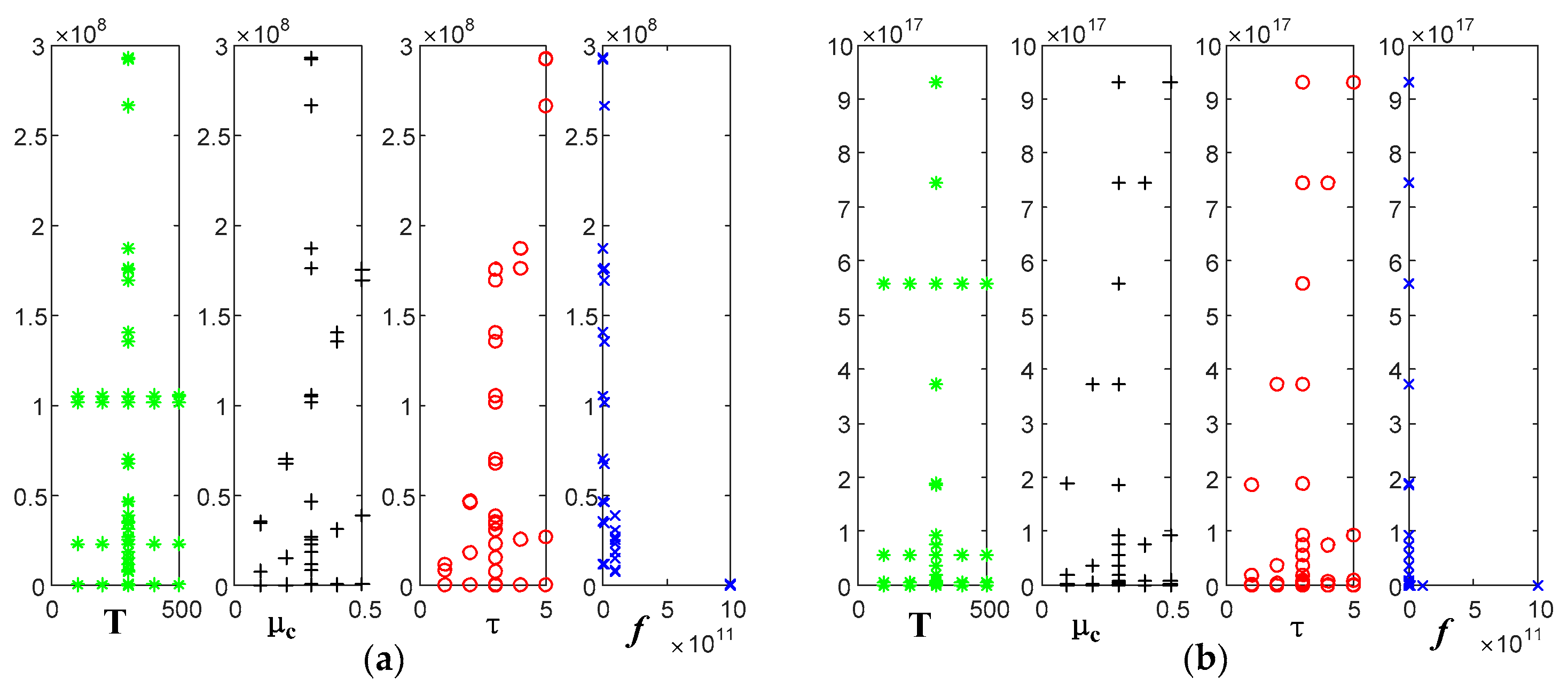
- As for the conductivity, the higher the frequency, the smaller the real part of the conductivity, and the imaginary part firstly decreases and then increases. The higher the electrochemical potentials, the larger the real part of the conductivity, and the smaller the imaginary part. Temperature does not affect the real and imaginary part of the conductivity of graphene. When the relaxation time increases, the real part of the conductivity increases, and the imaginary part decreases;
- (2)
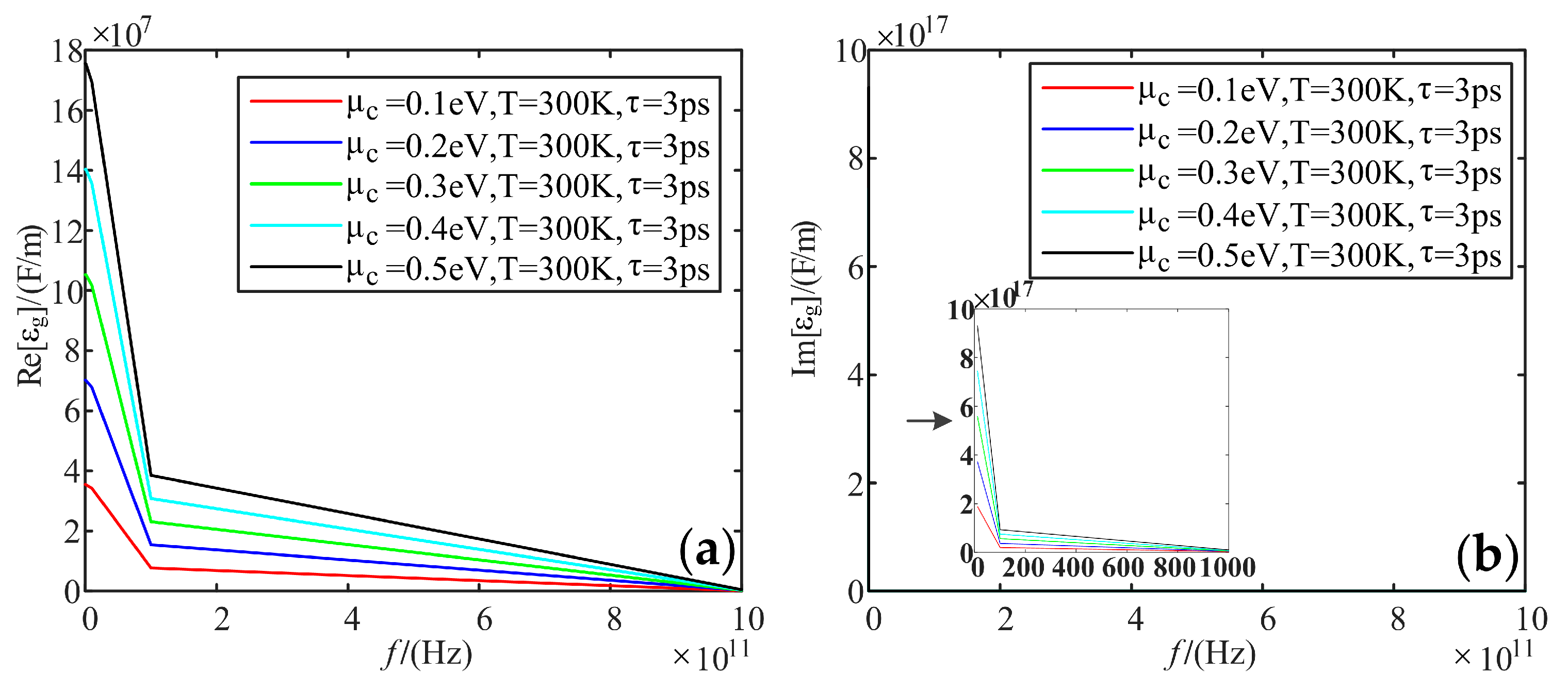
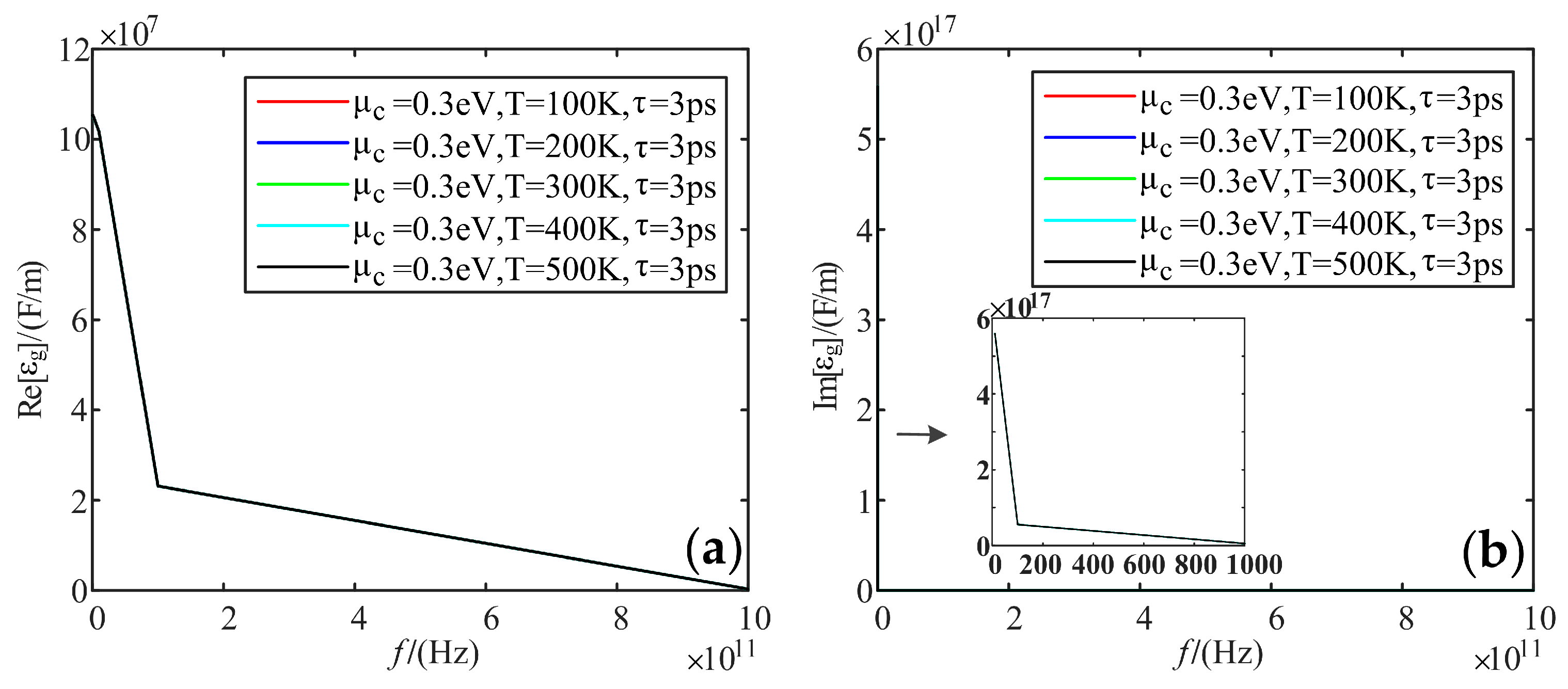
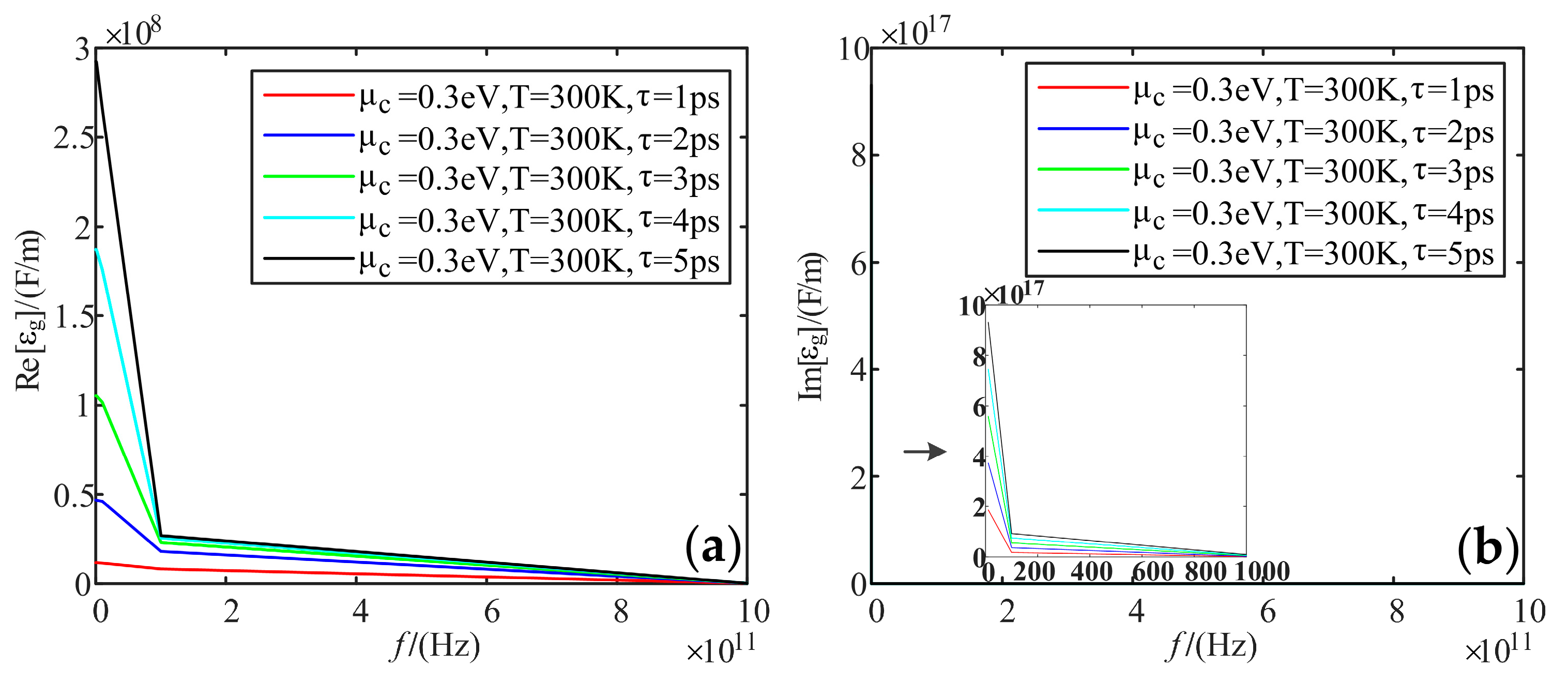
- As for the dielectric constant, the higher the frequency, the less the real part gradually decreases. The imaginary part has a huge value in the low-frequency band, and its value tends to 0 in the high-frequency band. The higher the electrochemical potentials, the larger the real part of the dielectric constant. Temperature does not affect the real and imaginary parts of the dielectric constant. When the relaxation time increases, the real part and imaginary part of the dielectric constant increase;
- (3)
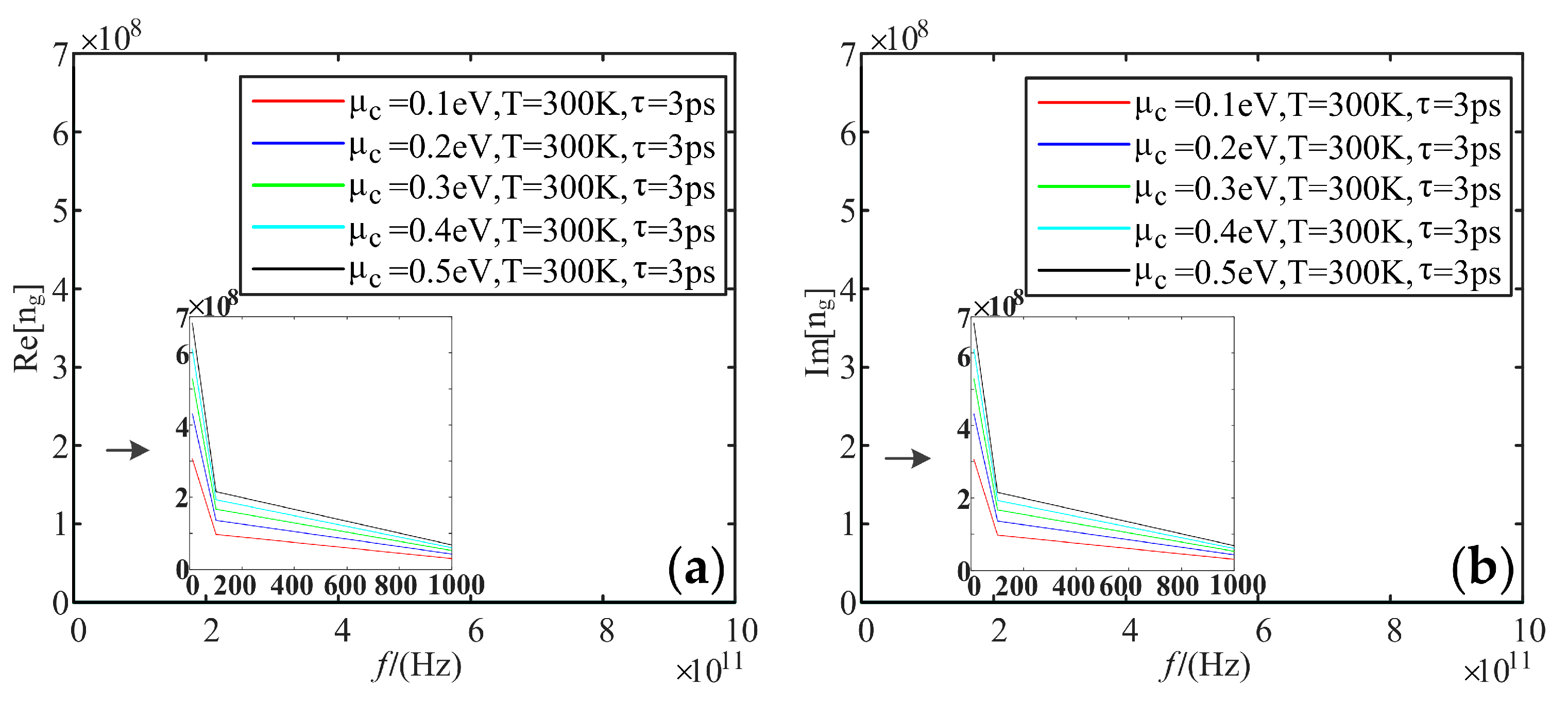
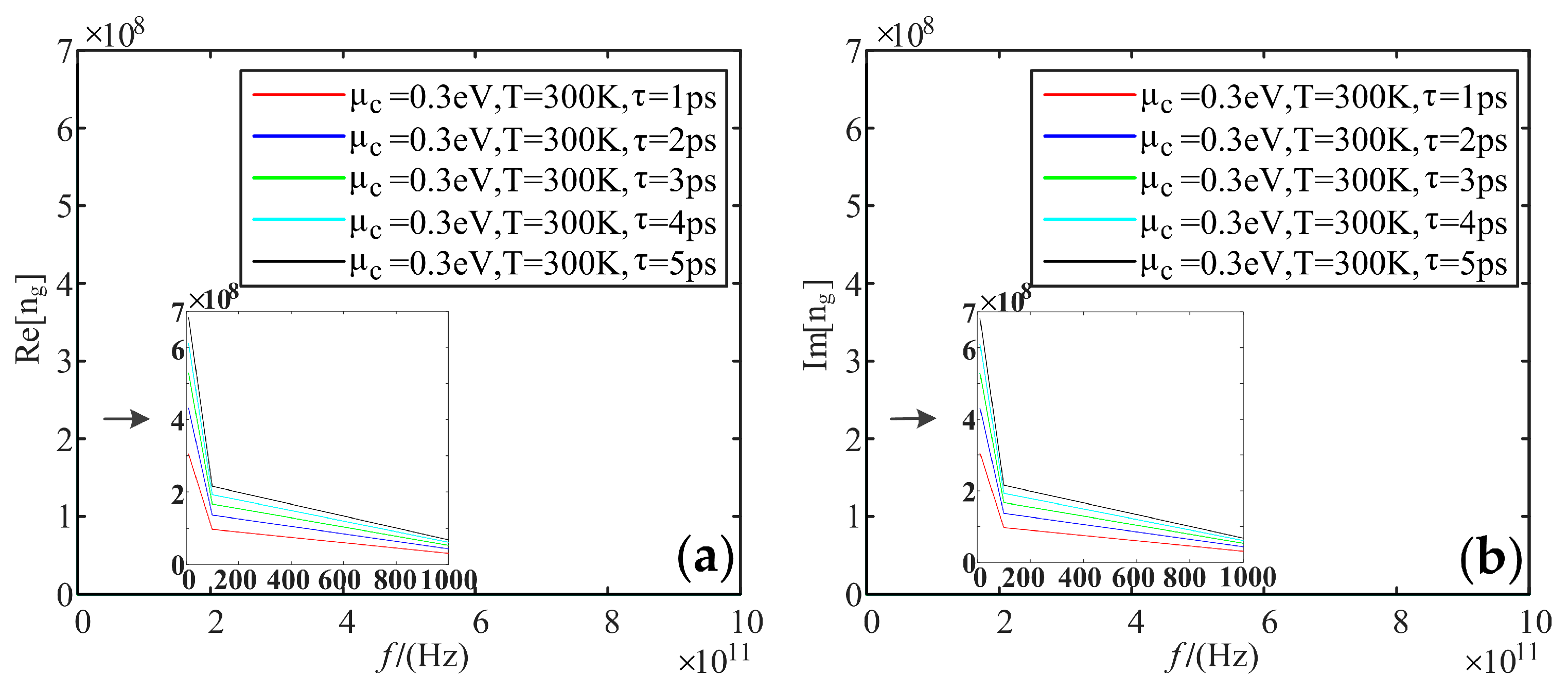
- As for the refractive index, because of the large frequency span of the sampling frequency band, the real and imaginary parts of the refractive index are larger when the frequency is small, but the high-frequency band tends to be 0. Additionally, the real and imaginary parts increase with the electrochemical potentials and relaxation time, and these two parts cannot reflect the effects of temperature.
- (4)
- A multiple linear regression model based on the least-squares method is established for the three electromagnetic properties, which can further simplify the calculation of electromagnetic properties and provide a good fit to the data.
- (5)
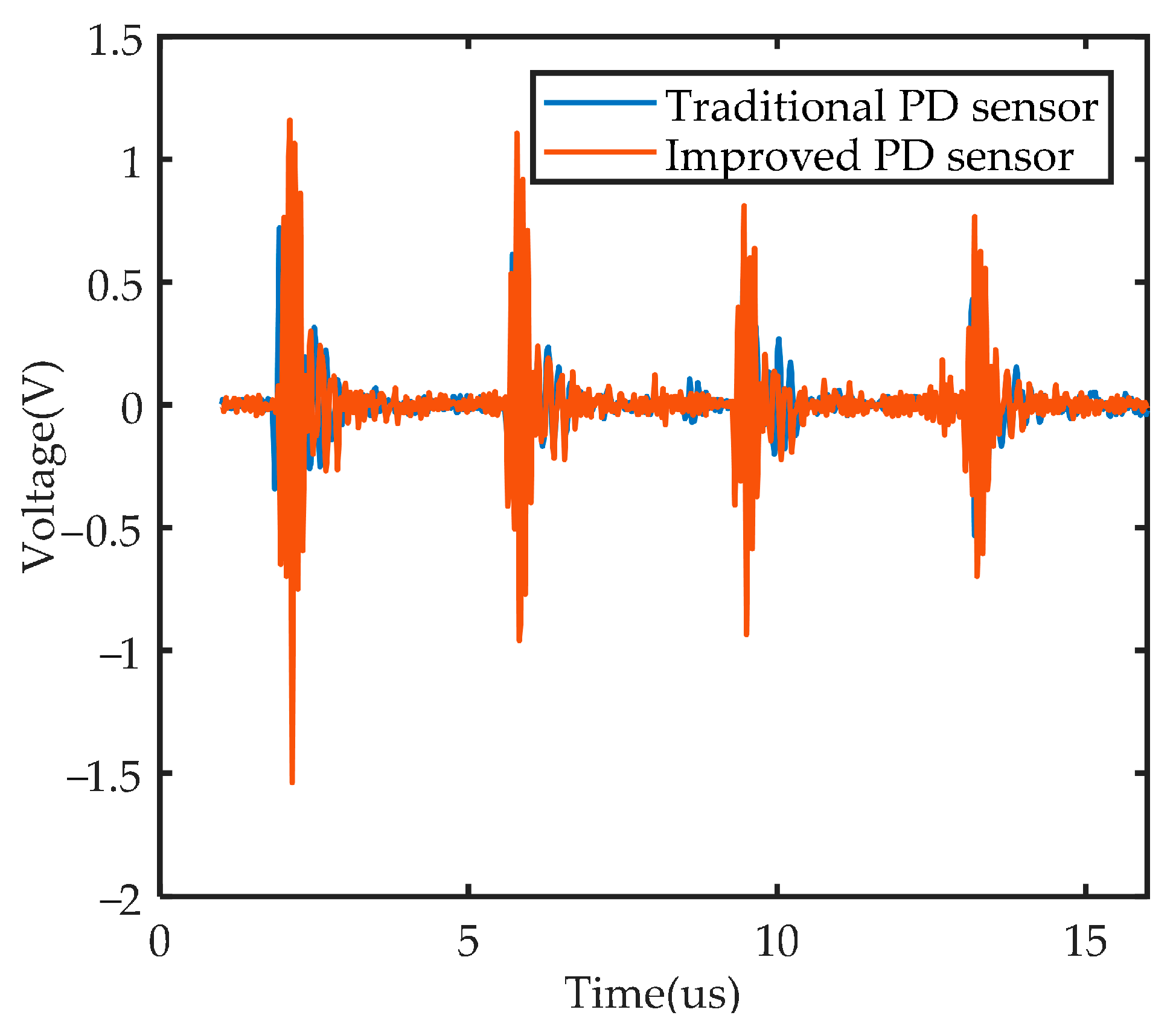
- The simulation and experiment verify that the graphene partial discharge sensor can better absorb the partial discharge signal compared with the traditional partial discharge sensor.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Electrical conductivity, dielectric constant, magnetic permeability, and refractive index | |
| Frequency, w = 2πf | |
| Electrochemical potential | |
| Scattering rate | |
| Relaxation time | |
| Temperature | |
| Imaginary unit | |
| Electron charge | |
| Planck constant | |
| Angular Planck constant, ħ = h/2π | |
| Energy | |
| Fermi–Dirac distribution function | |
| Simplification factor. | |
| Simplification factor. | |
| Vacuum dielectric constant. ε0 = 8.854187817 × 10−12 F/m | |
| Vacuum magnetic permeability. μ0 = 4π × 10−7 H/m | |
| Relative dielectric constant | |
| Relative magnetic permeability | |
| Thickness | |
| Three-dimensional conductivity | |
| Two-dimensional conductivity. σ2D = σ3D/t | |
| Electricity of graphene. σ2D = σg | |
| The real part of the graphene conductivity | |
| The imaginary part of the graphene conductivity | |
| The dielectric constant of graphene | |
| The real part of the graphene dielectric constant | |
| The imaginary part of the graphene dielectric constant | |
| The magnetic permeability of graphene | |
| Refractive index of graphene | |
| The real part of the graphene refractive index | |
| The imaginary part of the graphene refractive index | |
| Intra-band conductivity | |
| Inter-band conductivity | |
| Graphene layers number | |
| Boltzmann constant | |
| The propagation speed of electromagnetic waves in the medium | |
| Carrier concentration | |
| The velocity of the electromagnetic wave in a vacuum | |
| Fermi velocity |
References
- Subramaniam, A.; Sahoo, A.; Manohar, S.S.; Raman, S.J.; Panda, S.K. Switchgear Condition Assessment and Lifecycle Management: Standards, Failure Statistics, Condition Assessment, Partial Discharge Analysis, Maintenance Approaches, and Future Trends. IEEE Electr. Insul. Mag. 2021, 37, 27–41. [Google Scholar] [CrossRef]
- Tang, J.; Zhou, S.; Pan, C. A Denoising Algorithm for Partial Discharge Measurement Based on the Combination of Wavelet Threshold and Total Variation Theory. IEEE Trans. Instrum. Meas. 2020, 69, 3428–3441. [Google Scholar] [CrossRef]
- Zhao, M.; Cao, X.; Zhou, K.; Fu, Y.; Li, X.; Wan, L. Flexible Sensor Array Based on Transient Earth Voltage for Online Partial Discharge Monitoring of Cable Termination. Sensors 2020, 20, 6646. [Google Scholar] [CrossRef] [PubMed]
- Montanari, G.C.; Ghosh, R.; Cirioni, L.; Galvagno, G.; Mastroeni, S. Partial Discharge Monitoring of Medium Voltage Switchgears: Self-Condition Assessment Using an Embedded Bushing Sensor. IEEE Trans. Power Deliv. 2022, 37, 85–92. [Google Scholar] [CrossRef]
- Lu, B.X.; Huang, W.X.; Xiong, J.; Song, L.J. The Study on a New Method for Detecting Corona Discharge in Gas Insulated Switchgear. IEEE Trans. Instrum. Meas. 2022, 71, 9000208. [Google Scholar] [CrossRef]
- Zhang, X.R.; Shi, M.X.; Cai, J.; Li, J.H. A Novel Partial Discharge Detection Method for Power Transformers on Site Adopting Its Component as Ultra-High Frequency Sensor. IEEE Trans. Power Deliv. 2019, 34, 2269–2271. [Google Scholar] [CrossRef]
- Yadam, R.Y.; Ramanujam, S.; Arunachalam, K. An Ultrawideband Conical Monopole with Radome for Detection of Partial Discharges. IEEE Sens. J. 2021, 21, 18764–18772. [Google Scholar] [CrossRef]
- Nobrega, L.A.M.M.; Xavier, G.V.R.; Aquino, M.V.D.; Serres, A.J.R.; Albuquerque, C.C.R.; Costa, E.G. Design and Development of a Bio-Inspired UHF Sensor for Partial Discharge Detection in Power Transformers. Sensors 2019, 19, 653. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.; Dong, M.; Ren, M.; Huang, W.; Zhou, J.; Gao, X.; Albarracín, R. Partial Discharge Monitoring on Metal-Enclosed Switchgear with Distributed Non-Contact Sensors. Sensors 2018, 18, 551. [Google Scholar] [CrossRef] [Green Version]
- Tong, M.J.; Jiang, Y.; Wang, L.Y.; Tang, C.Y. Frictional characteristics of graphene layers with embedded nanopores. Nanotechnology 2021, 32, 345701. [Google Scholar] [CrossRef]
- Mu, S.N.; Liu, Q.R.; Kidkhunthod, P.; Zhou, X.L. Molecular grafting towards high-fraction active nanodots implanted in N-doped carbon for sodium dual-ion batteries. Natl. Sci. Rev. 2021, 8, nwaa178. [Google Scholar] [CrossRef] [PubMed]
- Lu, C.J.; Zhu, R.R.; Yu, F.H.; Jiang, X.L. Gear rotational speed sensor based on FeCoSiB/Pb(Zr,Ti)O3 magnetoelectric composite. Meas. J. Int. Meas. Confed. 2021, 168, 108409. [Google Scholar] [CrossRef]
- Wang, S.L.; Hong, J.S.; Liu, S.P.; Li, X.Y. Design of an Antenna Radome Based on a Kind of Graphene Frequency Selective Surface. In Proceedings of the 2018 International Conference on Microwave and Millimeter Wave Technology (ICMMT), Chengdu, China, 7–11 May 2018; pp. 1–3. [Google Scholar]
- Uzlu, B.; Wang, Z.; Lukas, S.; Otto, M.; Lemme, M.C.; Neumaier, D. Gate-tunable graphene-based Hall sensors on flexible substrates with increased sensitivity. Sci. Rep. 2019, 9, 18059. [Google Scholar] [CrossRef] [PubMed]
- Pezeshki, A.; Hamdi, A.; Yang, Z.; Lubio, A.; Shackery, I.; Ruediger, A.; Razzari, L.; Orgiu, E. Effect of Extrinsic Disorder on the Magnetoresistance Response of Gated Single-Layer Graphene Devices. ACS Appl. Mater. Interfaces 2021, 13, 26152–26160. [Google Scholar] [CrossRef] [PubMed]
- An, H.; Li, D.; Yang, S.; Wen, X.; Zhang, C.; Cao, Z.; Wang, J. Design and Performance Verification of a Space Radiation Detection Sensor Based on Graphene. Sensors 2021, 21, 7753. [Google Scholar] [CrossRef]
- Yu, H.; Xiang, P.; Zhu, Y.; Huang, S.; Luo, X. Polarization Insensitive Broadband Concentric- Annular-Strip Octagonal Terahertz Graphene Metamaterial Absorber. IEEE Photonics J. 2022, 14, 4600906. [Google Scholar] [CrossRef]
- Yan, J.; Huang, Y.; Wei, C.; Zhang, N.; Liu, P. Covalently bonded polyaniline/graphene composites as high-performance electromagnetic (EM) wave absorption materials. Compos. Part A Appl. Sci. Manuf. 2017, 99, 121–128. [Google Scholar] [CrossRef]
- Ullah, Z.; Nawi, I.; Witjaksono, G.; Tansu, N.; Khattak, M.I.; Junaid, M.; Siddiqui, M.A.; Magsi, S.A. Dynamic Absorption Enhancement and Equivalent Resonant Circuit Modeling of Tunable Graphene-Metal Hybrid Antenna. Sensors 2020, 20, 3187. [Google Scholar] [CrossRef]
- Judd, M.D.; Yang, L.; Hunter, I.B.B. Partial discharge monitoring for power transformers using UHF sensors. Part 2: Field experience. IEEE Electr. Insul. Mag. 2005, 21, 5–13. [Google Scholar] [CrossRef]
- Alaee, R.; Farhat, M.; Rockstuhl, C.; Lederer, F. A perfect absorber made of a graphene micro-ribbon metamaterial. Opt. Express 2012, 20, 28017–28024. [Google Scholar] [CrossRef] [Green Version]
- Gombor, T.; Pávó, J. Modeling of frequency selective surfaces using impedance type boundary condition. IEEE Trans. Magn. 2014, 50, 165–168. [Google Scholar] [CrossRef]
- Yao, Y.; Kats, M.A.; Genevet, P.; Yu, N.; Song, Y.; Kong, J.; Capasso, F. Broad electrical tuning of graphene-loaded optical antennas. In Proceedings of the 2013 Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 9–14 June 2013; pp. 1–2. [Google Scholar]
- Gusynin, V.P.; Sharapov, S.G.; Carbotte, J.P. Sum rules for the optical and Hall conductivity in graphene. Phys. Rev. B Condens. Matter Mater. Phys. 2007, 75, 165407. [Google Scholar] [CrossRef] [Green Version]


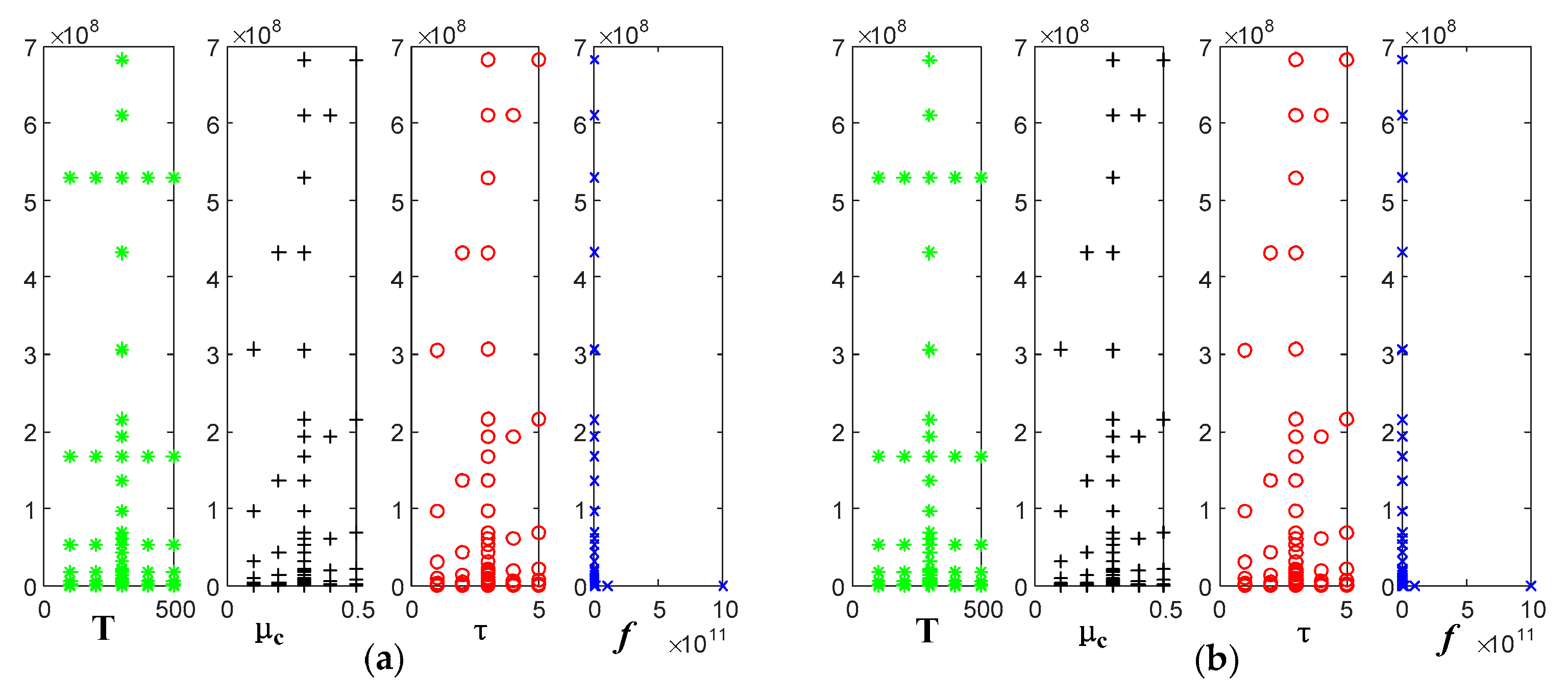


| Hz | kHz | MHz | GHz | THz |
|---|---|---|---|---|
| 1 Hz, 10 Hz | 1 kHz, 10 kHz, 100 kHz | 1 MHz, 10 MHz, 100 MHz | 1 GHz, 10 GHz, 100 GHz | 1 THz |
| Electromagnetic Properties | Part | T | μc | τ | f |
|---|---|---|---|---|---|
| Conductivity | Re | \ | + | + | − |
| Im | \ | − | − | − + | |
| Dielectric constant | Re | \ | + | + | − |
| Im | \ | + | + | − | |
| Refractive index | Re | \ | + | + | − |
| Im | \ | + | + | − |
| Material | T (K) | μc (eV) | τ (fs) | F (MHz) | σ (S/m) | ε |
|---|---|---|---|---|---|---|
| Graphene | 300 | 0.5 | 65 | 30, 60, 90 | \ | \ |
| Copper | \ | \ | \ | 30, 60, 90 | 5.998 × 107 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Wu, Z. Analysis of Electromagnetic Properties of New Graphene Partial Discharge Sensor Electrode Plate Material. Sensors 2022, 22, 2550. https://doi.org/10.3390/s22072550
Zhang H, Wu Z. Analysis of Electromagnetic Properties of New Graphene Partial Discharge Sensor Electrode Plate Material. Sensors. 2022; 22(7):2550. https://doi.org/10.3390/s22072550
Chicago/Turabian StyleZhang, Huiyuan, and Zhensheng Wu. 2022. "Analysis of Electromagnetic Properties of New Graphene Partial Discharge Sensor Electrode Plate Material" Sensors 22, no. 7: 2550. https://doi.org/10.3390/s22072550
APA StyleZhang, H., & Wu, Z. (2022). Analysis of Electromagnetic Properties of New Graphene Partial Discharge Sensor Electrode Plate Material. Sensors, 22(7), 2550. https://doi.org/10.3390/s22072550





