Appendix A
1D—dissipative tunneling in an external electric field. The role of the thermostat (of the heat-bath), (see also [
1,
26]).
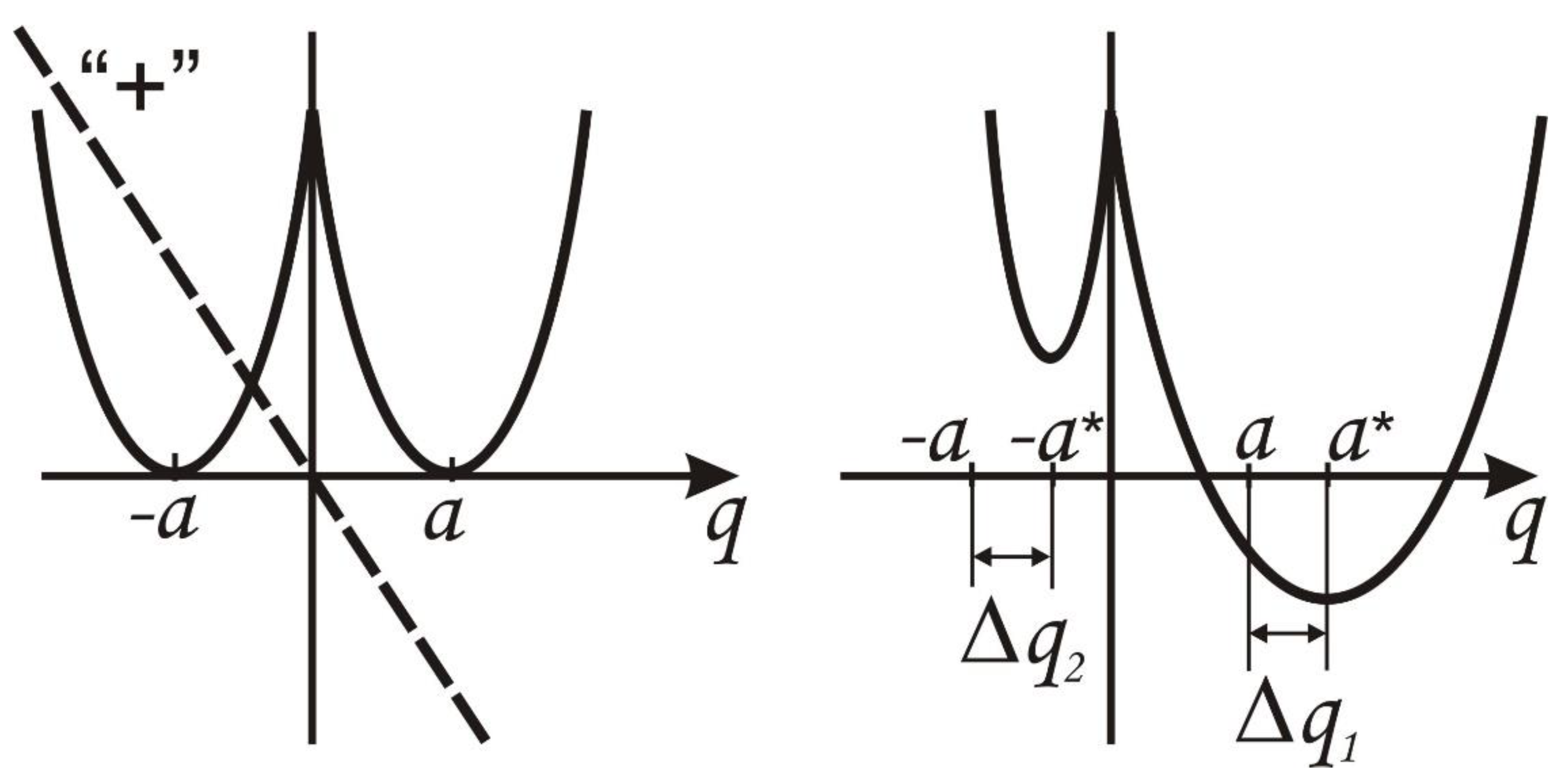
Let us consider the influence of an electric field on a two-well model oscillatory 1D potential (
Figure A1).
Figure A1.
Effect of an electric field on a symmetric double-well oscillatory potential.
Figure A1.
Effect of an electric field on a symmetric double-well oscillatory potential.
Taking into account the influence of an electric field on the symmetric double-well model oscillatory potential can be represented in the next form:
The electric field changes the symmetry of the potential and the minima shift:
Then the renormalized potential takes the form:
The values of the shifted minima (
Figure A1) are equal to:
and the shift of the minima turns out to be proportional to the field strength:
In this case, the shifts of the minima turn out to be the same in magnitude:
In the considered model, the top of the potential barrier is fixed:
but there is a corresponding shift in the value of the left minimum, and as a consequence, the barrier effectively decreases:
Since the subsequent consideration assumes the usage of the semiclassical instanton approximation when calculating the tunneling probability in a double-well oscillatory potential, we will assume that the barrier value cannot be too small in comparison with the sub-barrier transfer length; therefore, there is a natural limitation on the magnitude of the electric field strength:
In the case when the initial potential turns out to be asymmetric, the situation is similar with a correction for the initial asymmetry parameter (
Figure A2).
Figure A2.
Effect of an electric field on an asymmetric double-well oscillatory potential.
Figure A2.
Effect of an electric field on an asymmetric double-well oscillatory potential.
The initial potential asymmetry is determined by the parameter
:
where
; in this case, the displacements of the minima in the external electric field are determined by the parameters:
The values of the shifted minima are defined as:
The change in the asymmetry of the potential, as in the previous case, is proportional to the magnitude of the field:
At a certain value of the external field, the initially asymmetric potential with a deeper left-hand well can become symmetric
:
from here:
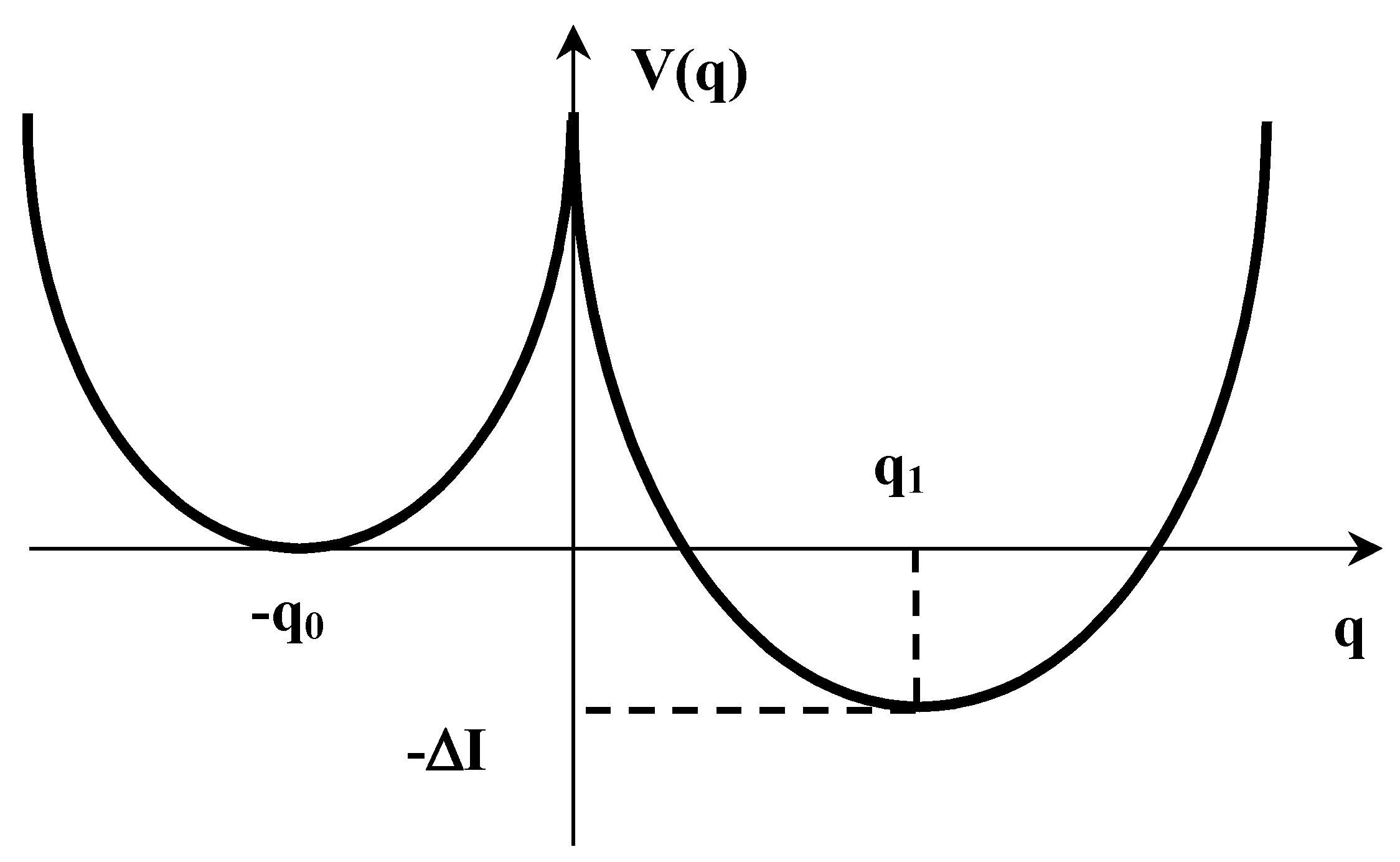
In order to use the standard model to determine the probability of dissipative tunneling, we will use the following notation for the renormalized double-well oscillatory potential in an external electric field:
,
. Then the model renormalized 1D potential can be represented in the standard form (
Figure A3).
Taking into account the results obtained earlier in [
1,
2,
3,
4,
26], the model Hamiltonian of the system can be written as
Figure A3.
Potential energy of a particle along the tunneling coordinate.
Figure A3.
Potential energy of a particle along the tunneling coordinate.
where
The probability of particle tunneling per unit time can be found in the semiclassical approximation. It is necessary that the de Broglie wavelength of the particle be much less than the characteristic linear scale of the potential. For this, it is quite sufficient that the height of the barrier be much greater than the energy of zero-point vibrations in the well of the initial state [
1,
26]. In addition to the semiclassical approximation, we must assume that the decay is quasi-stationary (for more details see [
1,
26]), that is, the width of the level
, from which the particle tunnels should be much less than the zero-point vibration energy. For the case of a nonzero temperature, the decay probability per unit time is defined as
here
—is the statistical sum of the system, which, due to the decay, is a complex quantity. A discussion of the justification of this expression for the multidimensional case is given in [
1,
26]. The appearance of the imaginary part in the statistical sum in the case of a two-well potential due to strong dissipation was considered in [
1,
26]. With a weak interaction with the oscillators of the medium, coherent tunneling oscillations are possible [
1,
26] (which are not considered in this work).
The calculation of the probability of 1D—dissipative tunneling (A12) in the form
accurate to the preexponential factor
(here
—is the one-instanton semiclassical action) in the one-instanton quasi-classical approximation in the model two-well oscillatory potential is given in [
26].
To calculate
(A12) in the form
, following the author’s work [
26], it is convenient to represent
in the form of an integral over trajectories [
1,
26]:
Since we are not interested in the states of the oscillators in the initial and final states, then along the trajectories
and according to the initial conditions
, (here
or
, where
and
are assumed to be equal to unity), we can integrate. Then the action functional depends only on the trajectory
:
where
here the potential is renormalized, i.e., the so-called adiabatic potential was introduced (for a discussion of this issue, see [
1,
26]). The kernel of the integral term in (A14) depends only on the parameters of the oscillators. The Fourier coefficients
in the expansion of the kernel
in a Fourier series are defined as
here
is the Matsubara frequency. Now, for the convenience of the calculation, we will shift the coordinate
so that the maximum of the potential
is at the point
, i.e.,
The type of potential (A18) is shown in
Figure A3.
We now turn to the calculation of the semiclassical action in the one-instanton approximation. The partition function
can be calculated in the semiclassical approximation. It is assumed that the main contribution to the action
is made by the trajectory
(instanton), which minimizes the action functional (A14) and obeys the Euler—Lagrange equation:
moreover, the trajectory
is sought on the class of periodic functions
The type of
is determined from the nature of the motion of the particle in the potential
. The particle begins to move (in the case of zero temperature) at the top of the potential
, i.e., at the point
, then passes the minimum point (
) at the moment of time
and reaches the value
(in the case of a symmetric potential) at the moment of time
. Then the particle repeats the trajectory in reverse order. Such a trajectory is called instanton [
1,
26]. It is noteworthy that the magnitude of the action on the trajectory
does not depend on the position of the instanton center
. Time
is determined from the condition
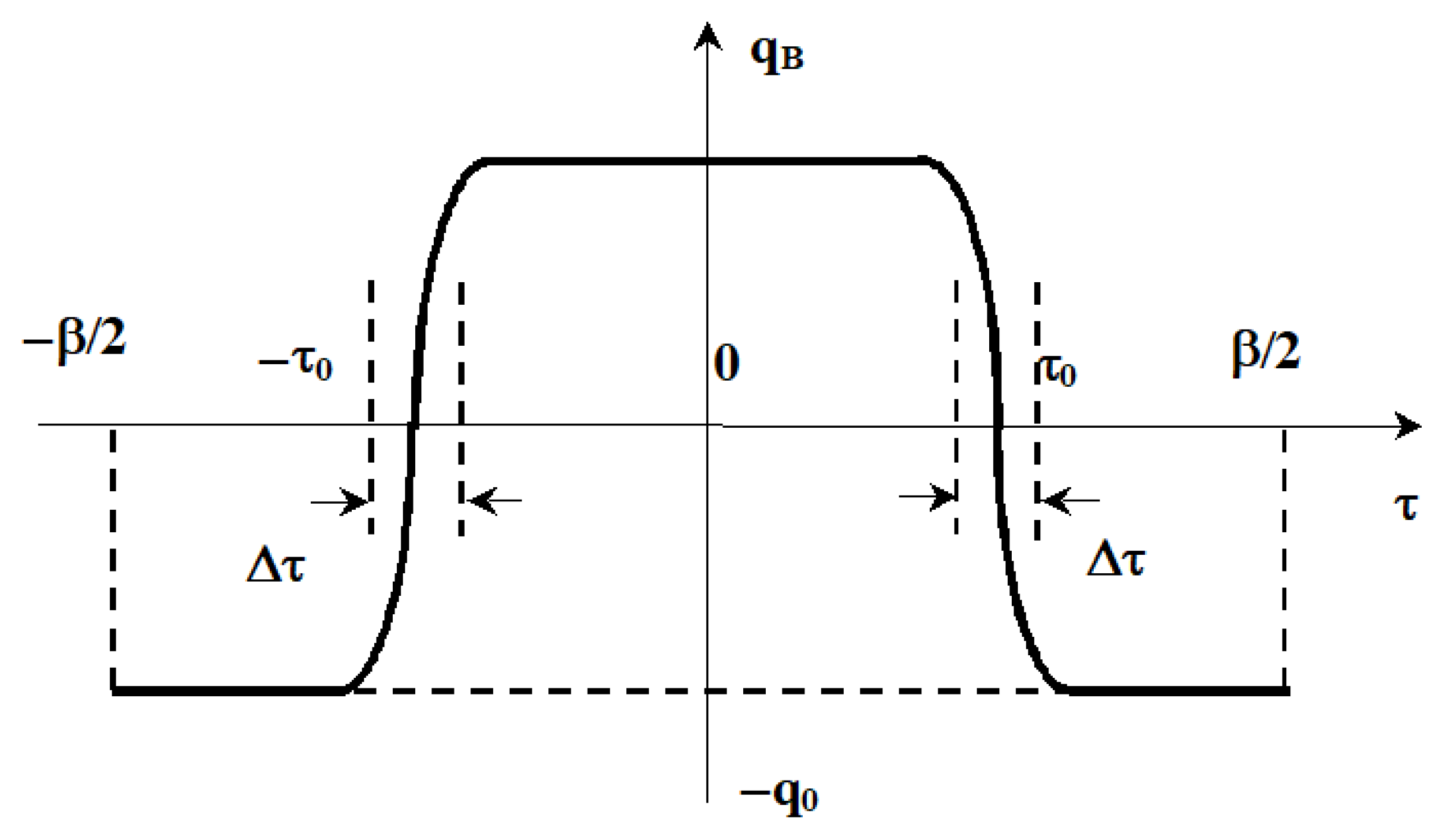
The trajectory
is shown in
Figure A4, where
is the center of the instanton,
is the width of the instanton.
Figure A4.
Instanton trajectory .
Figure A4.
Instanton trajectory .
The introduction of time
greatly facilitates the solution of Equation (A20), since the step functions of the coordinate can be replaced by the corresponding functions of time:
We will look for the trajectory
in the form of an expansion in a Fourier series:
Expanding also the
-functions and the kernel
in Fourier series, we obtain equations for the Fourier coefficients
, which can be solved exactly. Then
where
—Matsubara frequency, and
is determined from the relation (A16). Next, we substitute (A25) in the expression for the action. Then we find
Thus, the semiclassical action in the one-instanton approximation is determined analytically. Several special cases for the kernel
will be analyzed below. Consider a situation where there is no interaction with the environment, i.e.,
. This case corresponds to one-dimensional tunneling. Then,
, determined from Equations (A22) and (A25) is
The action is found from expressions (A26) and (A27):
Now let’s move on to calculating the preexponential factor. The preexponential factor is determined by the contribution of trajectories close to the instanton. To do this, we have to expand the action to a quadratic term in deviations
and integrate in the functional space [
1,
26]. Then the tunneling probability per unit time can be written as
means that the zero eigenvalue corresponding to the zero instanton mode is omitted. Note that the derivation of this formula assumes the approximation of an ideal instanton gas [
1,
26]
where
is the width of the transition from the positive value of the trajectory to the negative one (
Figure A4). For the sake of rigor, we note that the trajectory
is the sum of two trajectories—an instanton and an anti-instanton. If
is large, then we can assume that instanton and anti-instanton interact weakly. However, for small
, this approximation is incorrect. Therefore, we should talk not about an ideal instanton gas, but about a rarefied gas of instanton—anti-instanton pairs. We define the width
as
In (A31)
means the calculation of the product of the eigenvalues of the following equation [
1,
26]:
The second derivative of the potential with respect to the coordinate is taken either at the instanton (semiclassical trajectory) or at the minimum point of the metastable potential. For our potential
In doing so, we used the condition
First, we calculate the eigenvalues of Equation (A35) at
. The eigenfunctions, as in the case of instanton, are sought in the class of periodic functions. Expanding the trajectory and the kernel
in Fourier series, we obtain the eigenvalues
of Equation (A35):
Next, we find the product of the eigenvalues of Equation (A35) on the instanton trajectory. Now the eigenvalue equation has the form
We also seek a solution to this equation in the class of periodic functions. Expanding
, the
-functions and
in Fourier series and integrating over
with a factor
, we find
where
From Equation (A40) you can find
; substitution
in
and
gives two eigenvalue equations:
Taking into account from (A25), that
Equations (A42) and (A43) can be reduced to the form
The first equation contains an eigenvalue
, corresponding to the zero mode; it should be excluded from the product of roots. According to Vieta’s theorem, the product of the roots of the first equation (without
) can be found exactly:
For the final calculation of the preexponential factor, it remains to find the normalization of the zero mode,
, determined by formula (A32). Considering that
and substituting (A49) into the integrand (A32), we obtain
Taking into account (A47), (A48) and (A50), we can calculate the pre-exponential factor
Thus, the problem of particle tunneling in the model potential (A18) in the one-instanton approximation has been solved analytically. The exponent is determined by the semiclassical action (A26), and the preexponential factor is determined by the expression (A51). In this case, the quasi-stationarity condition (A23) (ideal gas of instanton—anti-instanton pairs) imposes restrictions on the temperature and other parameters of the system.
Let us now investigate the influence of low-frequency oscillations of the medium on the probability of a particle transition in a system with a selected tunneling coordinate. In this case, we will consider several important special cases of the spectrum of vibrations of molecules of the medium in relation to the results obtained at the beginning of this section and relating to the transition probability of a particle interacting with a “thermostat” or the heat-bath in system (A18) with a selected tunneling coordinate.
Consider several special cases for the kernel .
(a) Let there be no interaction with the environment, i.e.,
. This situation corresponds to one-dimensional tunneling. Expressions for action in this case (A28), (A29) were obtained above. The pre-exponential factor
(A51) has the form
When
, i.e., at
When
, i.e., at
(symmetrical wells)
From (A54) it is seen that at low temperatures
That is the preexponential factor diverges. This fact should not discourage, since at low temperatures for symmetric wells the quasi-stationarity condition (A33) is violated and the semiclassical approximation is unfair, since at zero tunneling energy, there is no wave going to infinity. The theory is limited by the approximation of a free gas of instanton—anti-instanton pairs, i.e., the next inequality is true
where
—barrier height.
(b) An important case of the spectral phonon density is the ohmic damping approximation, i.e.,
. This case corresponds to the viscous motion of a particle in the classical limit, where the transition probability per unit time was calculated by Kramers [
1,
26]. At low temperatures, the series in the expression for the action can be summed up by the Euler-McCloren method [
1,
26], and
where
here
is Euler’s constant and
.
Of particular interest is the case of a symmetric potential, i.e., when
and
. Then the action diverges at low temperatures:
This divergence may indicate the localization of the particle in the well of the metastable state. The phenomenon of particle localization (zero quantum limit in the rate constant) is completely absent in the Einstein model of medium oscillations [
1,
26] and is a property of a large number of low-frequency medium oscillations responsible for the viscous motion of a tunneling particle. It should be noted that in this case the action can be calculated exactly not only in the low-temperature limit:
here
is the logarithmic derivative of the
(of the Euler function) [
1,
26]. Note that at
, there is no particle localization.
The pre-exponential factor can be calculated exactly at low temperatures and at any
:
where
and
are determined by expressions (A58). At
In this case, the preexponential factor is calculated exactly at an arbitrary temperature; however, due to the cumbersomeness of the expression, we will not present it.
Consider the complete expression for the tunneling rate
where
From (A64) it can be seen that, depending on the sign of the exponent at the factor , three cases are possible:
(1) Let
then, with a sufficiently strong damping, the particle is localized in the well of the initial state. This phenomenon is not observed when simulating a chemical reaction with a two-frequency model [
1,
26]. Localization of a particle means a violation of symmetry for the right and left positions. A similar temperature dependence was obtained for the two-level model [
1,
26].
(2) For the inverse to (A65) inequality at low temperatures, the quasi-stationarity condition is violated, and the temperature is limited by inequality (A33).
(3) At tunneling rate is independent of temperature.
The condition for the theory applicability for ohmic damping has the form
(c) For a symmetric form of the potential, the action can be calculated exactly (instanton action (A26) at
,
), if we choose
in the form of the Drude approximation [
1,
26]:
where
—the boundary value of the frequency for the vibrational spectrum. In this case
where
,
and
- absolute values of the roots of the following algebraic equation:
At low temperatures (
,
)
As in the previous case, there is a divergence of the action at low temperatures. This divergence is associated with a linear dependence of at , i.e., the divergence is determined by small frequencies.
The preexponential factor can also be calculated exactly, but we will not give a complete expression for , but only note that the temperature dependence of the decay probability completely coincides with the case of ohmic damping. The same dependence on viscosity also remains.
Such a model is of interest in the theory of tunneling of color centers [
1,
26] in solids, provided that the medium affects the particle motion due to coupling with acoustic phonons. In addition, in this case, for a symmetric potential, the action can be calculated exactly. We present only its asymptotic behavior at low temperatures:
where
(
)—absolute values of the roots of an algebraic equation
In this case, no divergence of the action is observed and it takes on a finite value at low temperatures. The form of the spectrum (A71) at small values of depends in a quadratic manner on , which at low temperatures leads to renormalization of the mass of the tunneling particle, and the problem is thus reduced to one-dimensional tunneling.
The pre-exponential factor is
where
—some factor that does not depend on temperature (we will not present its expression due to its cumbersomeness). From (A74) it follows that the preexponential factor diverges at low temperatures as
, however, in contrast to the exponential divergence in the one-dimensional case, here the divergence is weakened to a power law due to the presence of viscous motion of the oscillators of the medium. In fact, the divergence is eliminated by the condition of applicability of inequality (A33), i.e., by approximation of the quasi-stationarity of the kinetic process.
Let us consider (A29) taking into account the interaction with one local phonon mode (). For simplicity, we will assume that this interaction is sufficiently small, i.e., and . In this case , where ; and .
Then we can obtain an expression for the semiclassical action taking into account the local mode of the medium—thermostat (of the heat -bath) in the given dimensionless variables:
where
,
;
—renormalized asymmetry parameter. In addition, the influence of the local mode of the thermostat—medium (of the heat-bath) is taken into account through the following parameters:
where
To calculate the preexponential factor taking into account the influence of the local mode
of the medium-thermostat (of the heat-bath), we use the previously obtained general expression [
1,
26] (A51). In this case, as in the case of calculating the semiclassical instanton (Euclidean) action taking into account the local mode
, we use that
where
Then, to calculate the preexponential factor, we will take into account that in the general expression for
(A51)
And the following transformation of expressions occurs:
where
The expression in the denominator (A51) is converted to the form:
where
have been defined above.
Introducing, as in the case of the action calculation, taking into account the local mode of the medium-thermostat (of the heat-bath), the coefficients:
and also, considering that
where
we obtain the final analytical expression for the preexponent taking into account the influence of the local mode of the medium-thermostat (of the heat-bath):
For subsequent numerical estimates, we use the introduction of dimensionless parameters
,
,
Condition (A33), which limits the applicability of the considered approximation, for studying tunneling in semiconductor quantum dots gives the following estimates. The applicability of the semiclassical instanton approximation [
1,
2,
3,
4,
26] in the study of the temperature dependence of the tunneling probability Γ for QDs based on InSb can be estimated in the semiclassical approximation by comparing the characteristic size of the system with the de Broglie wavelength of the tunneling particle, or in the framework of the rarefied gas approximation pairs “instanton—anti-instanton” [
1,
2,
3,
4,
26].
where
—barrier height,
—effective mass of a tunneling electron.
The first inequality compares the QD radius
with the de Broglie wavelength of the tunneling particle; the second formula demonstrates the applicability of the approximation of a rarefied gas of “instanton–anti-instanton” pairs [
1,
26]. Both inequalities are fulfilled simultaneously at
and
, which may correspond to QD based on InSb. As shown in the work [
27], the suppression of Coulomb effects can occur if the starting energy of the particle in the QD significantly exceeds the energy of the Coulomb repulsion:
. Supplementing this condition with a limitation on the magnitude of the electric field strength
for QDs made of InSb, we can obtain the following value of the strength:
.
As a result, an expression for the probability of one-dimensional tunneling transport is found analytically [
1,
26]:
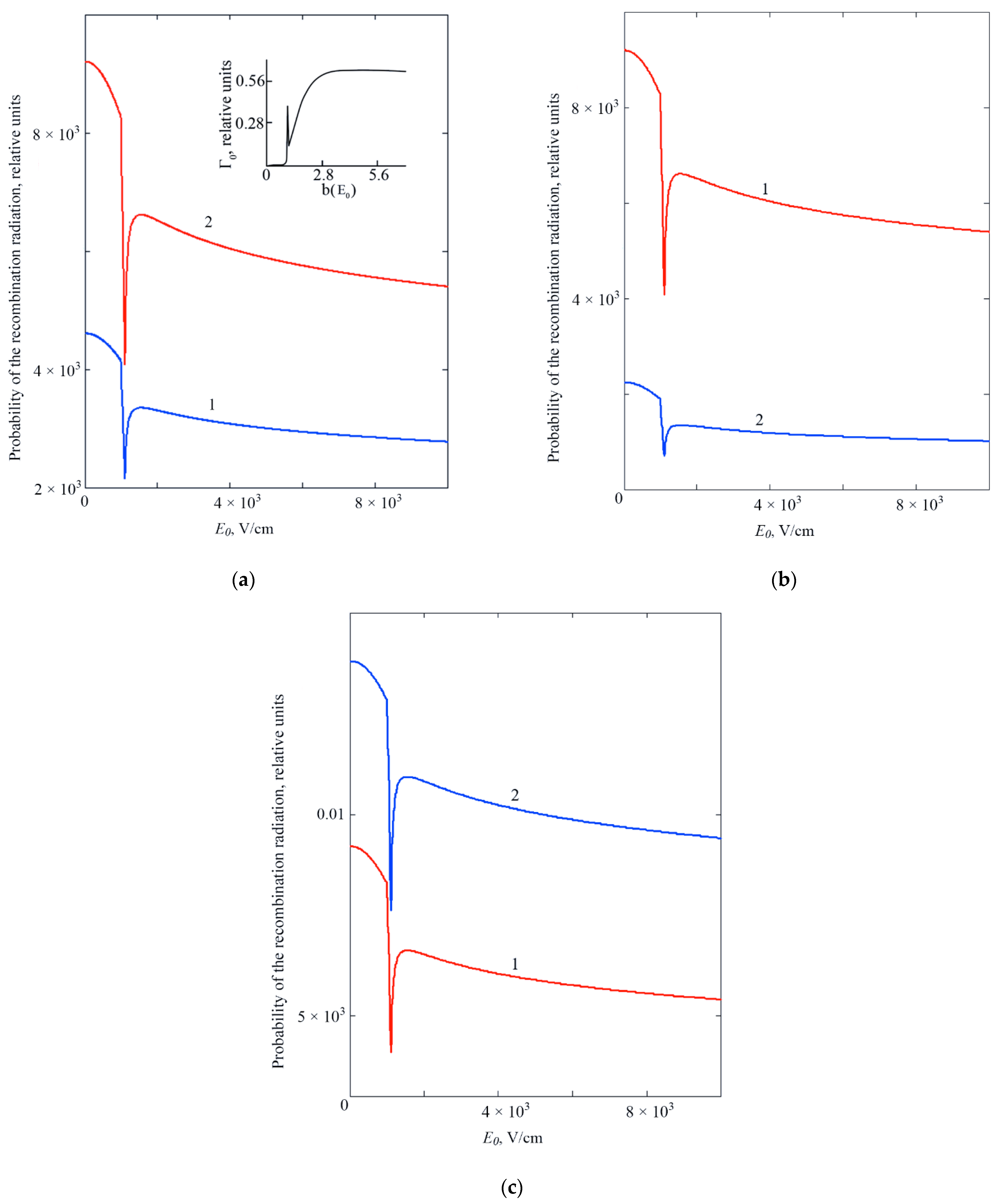
The inset to
Figure 3a of the text of the article shows a graph containing a single peak for the case of a symmetric double-well potential at a certain value of the external electric field strength, obtained as the field dependence of the probability of dissipative tunneling (A77). This peak, obtained theoretically, qualitatively coincides with individual experimental tunneling I–V characteristics for single QDs in the combined AFM/STM system [
1].