Research on Inherent Frequency and Vibration Characteristics of Sandwich Piezoelectric Ceramic Transducer
Abstract
1. Introduction
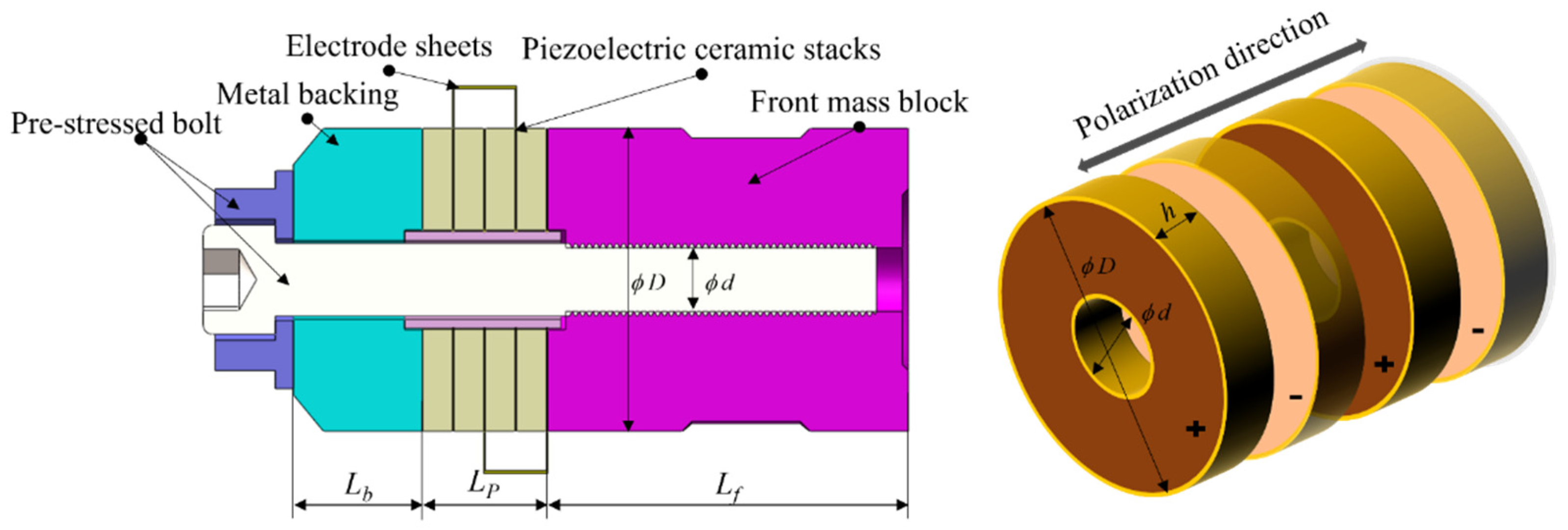
2. Transducer Structure and Equivalent Models
- The motion of the sandwich transducer along the axial direction is uniform, which means that the vibration caused by the piezoelectric ceramic is generated as a plane wave.
- It is assumed that the contact of the surface is perfect. Therefore, the reflection of the wave is neglected.
- Each ceramic piece in the piezoelectric ceramic stack is excited by a continuous sinusoidal wave, and the signal is in the same phase.
- The length of the transducer is more than three times its diameter, so the transverse Poisson effect is ignored and the transducer can be simplified to a one-dimensional model.
- The effect of the chamfer and thread of the transducer can be ignored; the transducer will be regarded as equal to the outside diameter.
- The acoustic impedance of air is much smaller than the transducer, so it is ignored. When the transducer is operating in the air, it is referred to as being under the “no-load” condition, and its stress is considered to be zero.
- The matched upper part is considered to be a solid cylinder with the center bolt integrated to simplify the boundary conditions.
3. Analysis Method of Transducer Inherent Frequency
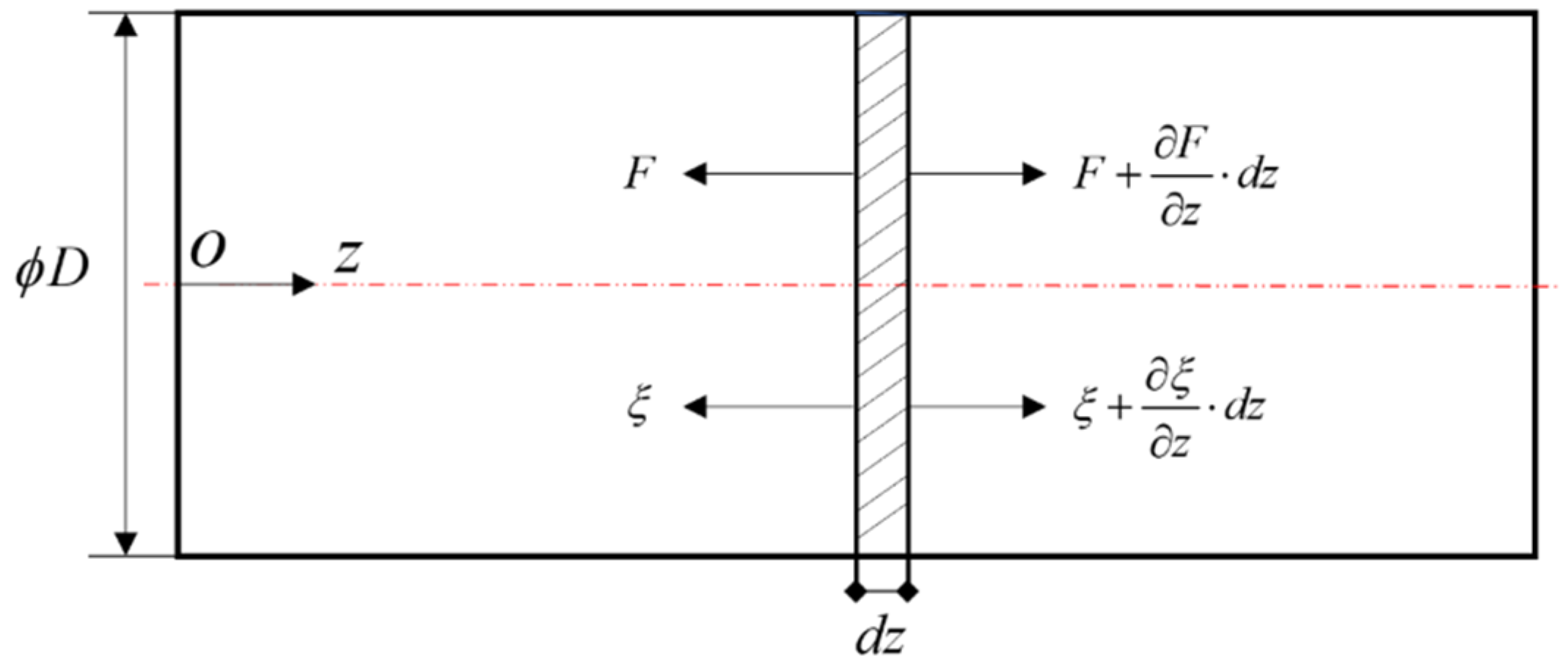
3.1. Mechanical Vibration Equation of Piezoelectric Ceramic Stack
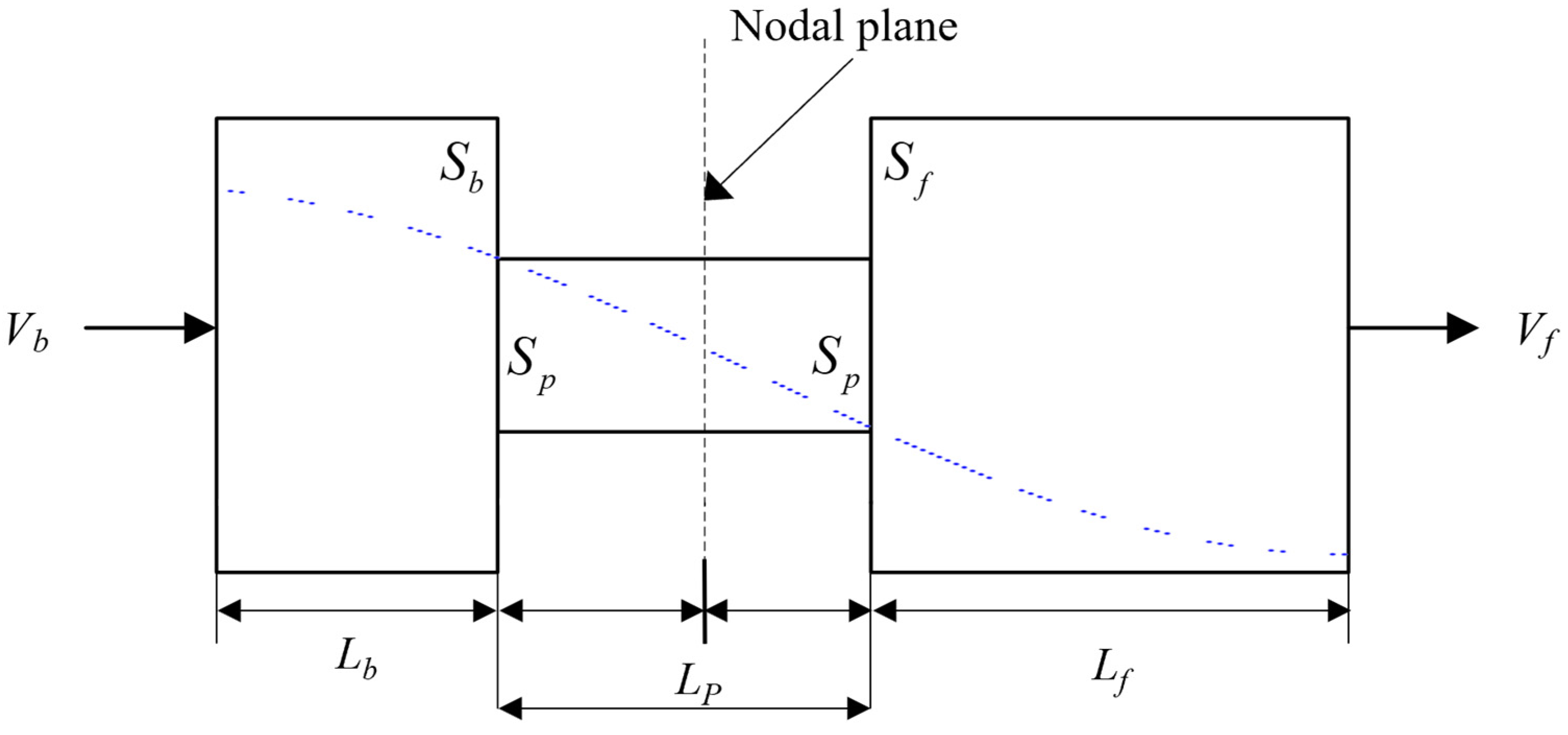
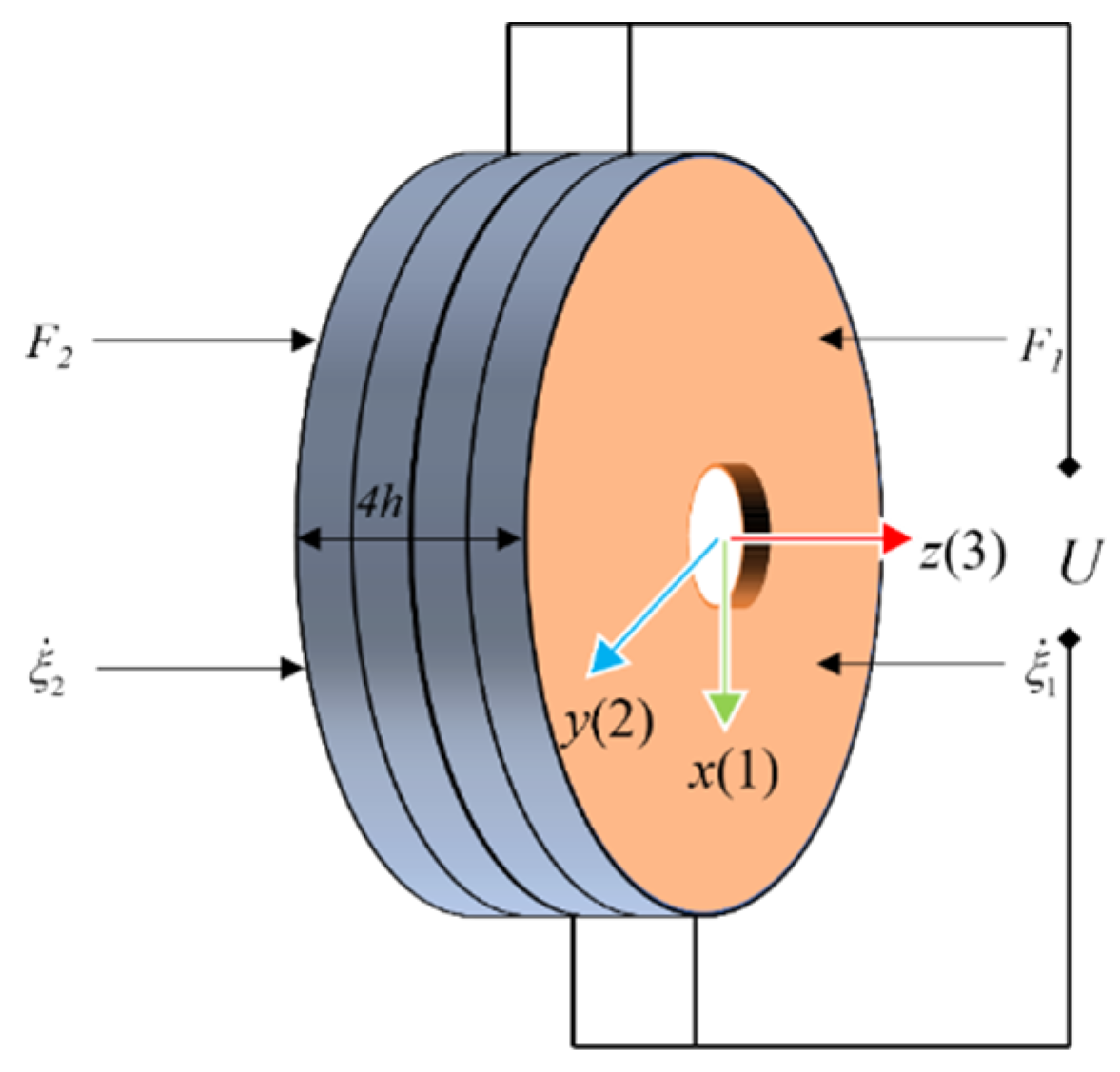
3.2. Vibration Model of Transducer
3.3. Equivalent Elastic Modulus Method of Transducer
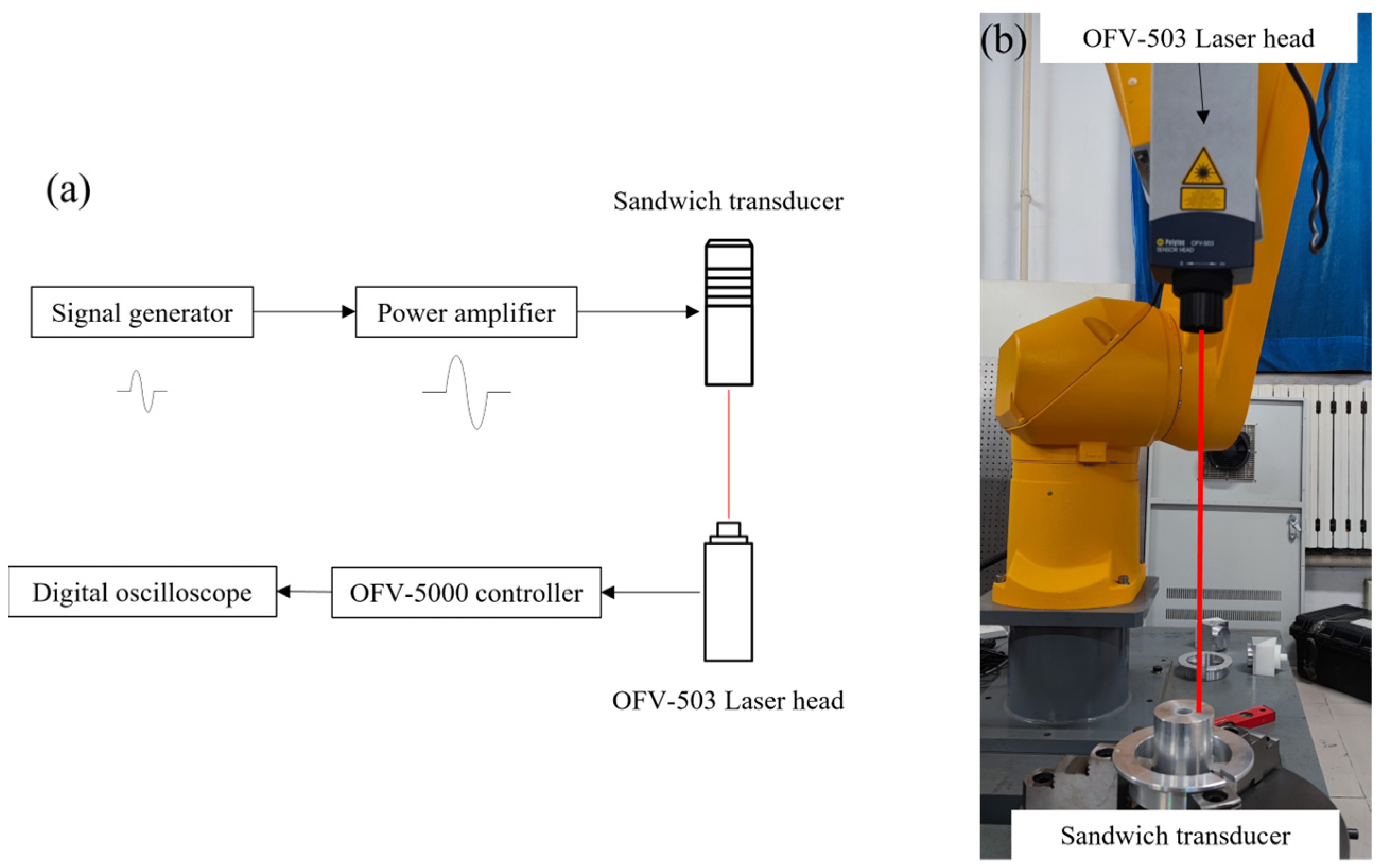
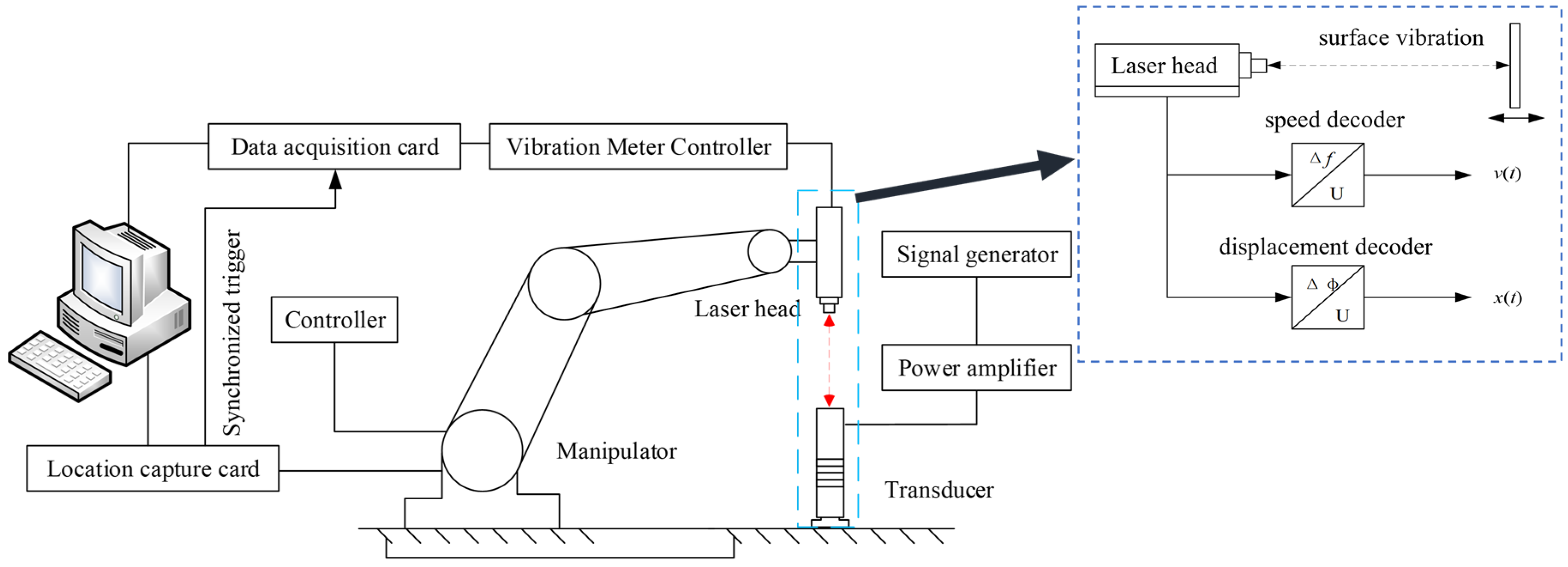
4. Experimental Methods
4.1. Design and Inherent Frequency Measurement of Piezoelectric Transducer
4.2. Measurement of Transducer Working State
5. Results and Discussion
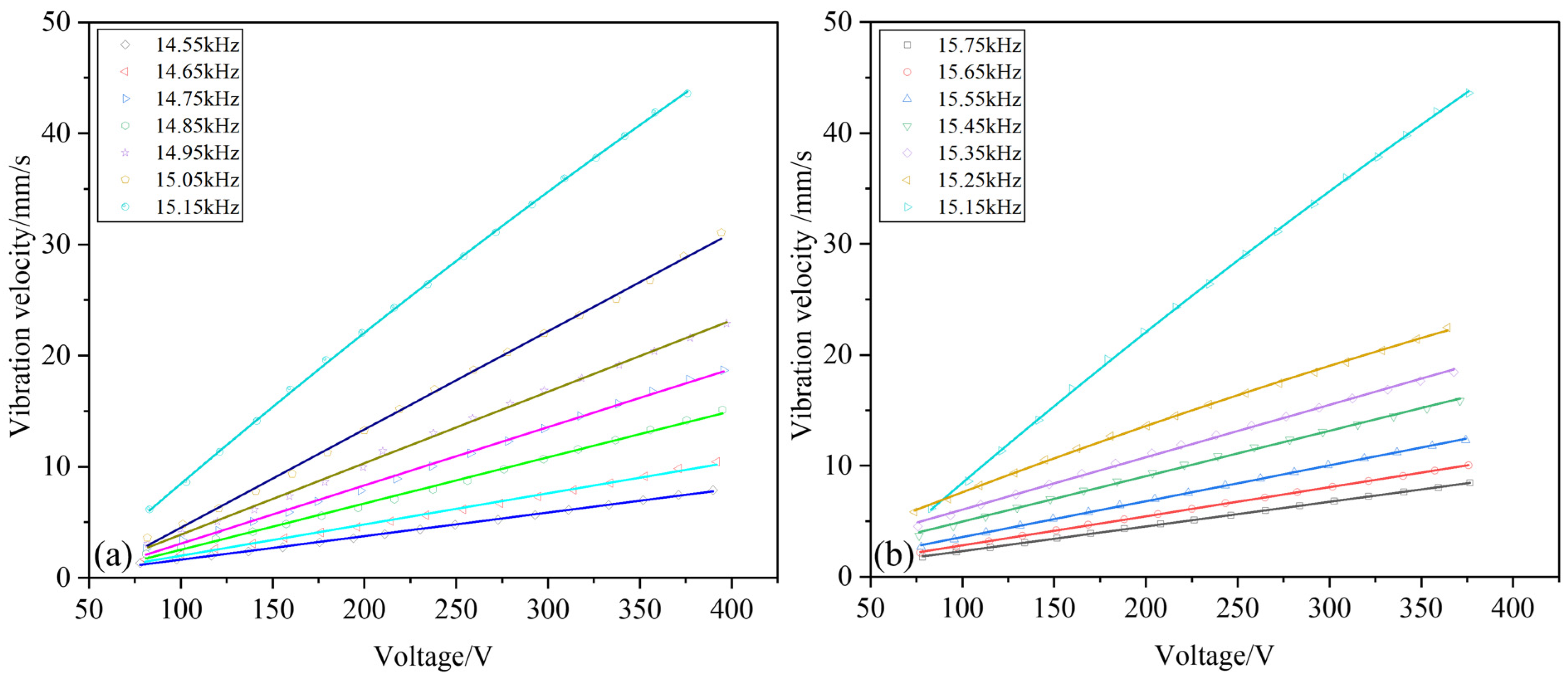
5.1. Transducer Inherent Frequency Analysis
5.2. Working Performance Measurement and Analysis of Sandwich Piezoelectric Transducer
5.3. Measurement of Working State of Sandwich Transducer
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pang, Y.; Feng, P.; Wang, J.; Zha, H.; Xu, J. Performance analysis of the longitudinal-torsional ultrasonic milling of Ti-6Al-4V. Int. J. Adv. Manuf. Technol. 2021, 113, 1255–1266. [Google Scholar] [CrossRef]
- Ying, N.; Feng, J.; Bo, Z.; Guofu, G.; Jing-jing, N. Theoretical investigation of machining-induced residual stresses in longitudinal torsional ultrasonic–assisted milling. Int. J. Adv. Manuf. Technol. 2020, 108, 3689–3705. [Google Scholar] [CrossRef]
- Lü, S.; Wu, S.; Dai, W.; Lin, C.; An, P. The indirect ultrasonic vibration process for rheo-squeeze casting of A356 aluminum alloy. J. Mater. Process. Technol. 2012, 212, 1281–1287. [Google Scholar] [CrossRef]
- Haghayeghi, R.; Heydari, A.; Kapranos, P. The effect of ultrasonic vibrations prior to high pressure die-casting of AA7075. Mater. Lett. 2015, 153, 175–178. [Google Scholar] [CrossRef]
- Zohrevand, M.; Aghaie-Khafri, M.; Forouzan, F.; Vuorinen, E. Internal stress relief and microstructural evolution by ultrasonic treatment of austeno-ferritic 2205 duplex stainless steel. Mater. Sci. Eng. A 2021, 815, 141290. [Google Scholar] [CrossRef]
- Zhang, Q.; Yu, L.; Shang, X.; Zhao, S. Residual stress relief of welded aluminum alloy plate using ultrasonic vibration. Ultrasonics 2020, 107, 106164. [Google Scholar] [CrossRef]
- Du, L.; Wang, Q.; Zhang, X. Reduction of internal stress in SU-8 photoresist layer by ultrasonic treatment. Sci. China Technol. Sci. 2010, 53, 3006–3013. [Google Scholar] [CrossRef]
- Gu, B.; Jin, Z.; Kong, D.; Lai, J.; Yang, Z.; Pan, L. Reduction of pulsed-laser surface irradiation induced residual stress using ultrasonic vibration method. Int. J. Adv. Manuf. Technol. 2017, 88, 755–765. [Google Scholar] [CrossRef]
- Song, W.-Y.; Xu, C.-G.; Pan, Q.-X.; Li, P.-L.; Wang, L.; Yu, T. The Residual Stress and Deformation Control of TC4 Thin-Walled Outer Ring Components by Ultrasonic Regulation. Machines 2022, 10, 598. [Google Scholar] [CrossRef]
- Lin, S.; Xu, C. Analysis of the sandwich ultrasonic transducer with two sets of piezoelectric elements. Smart Mater. Struct. 2008, 17, 065008. [Google Scholar] [CrossRef]
- Meng, X.; Lin, S. Analysis of a Cascaded Piezoelectric Ultrasonic Transducer with Three Sets of Piezoelectric Ceramic Stacks. Sensors 2019, 19, 580. [Google Scholar] [CrossRef]
- Hu, J.; Lin, S.; Zhang, X.; Wang, Y. Radially Sandwiched Composite Transducers Composed of the Radially Polarized Piezoelectric Ceramic Circular Ring and Metal Rings. Acta Acust. United Acust. 2014, 100, 418–426. [Google Scholar] [CrossRef]
- Bloomfield, P.E. Multilayer transducer transfer matrix formalism. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2002, 49, 1300–1311. [Google Scholar] [CrossRef] [PubMed]
- Xue, Y.; Bolton, J.S.; Liu, Y. Modeling and coupling of acoustical layered systems that consist of elements having different transfer matrix dimensions. J. Appl. Phys. 2019, 126, 165102. [Google Scholar] [CrossRef]
- Wang, L.; Hofmann, V.; Bai, F.; Jin, J.; Twiefel, J. Modeling of coupled longitudinal and bending vibrations in a sandwich type piezoelectric transducer utilizing the transfer matrix method. Mech. Syst. Signal Process. 2018, 108, 216–237. [Google Scholar] [CrossRef]
- Zhang, Y.; Tu, Z.; Lu, T.-F.; Al-Sarawi, S. A simplified transfer matrix of multi-layer piezoelectric stack. J. Intell. Mater. Syst. Struct. 2017, 28, 595–603. [Google Scholar] [CrossRef]
- Fu, B.; Li, C.; Zhang, J.; Huang, Z.; Hemsel, T.; Bo, F.; Chao, L.; Jianming, Z.; Zhenwei, H.; Tobias, H. Modeling of Piezoelectric Langevin Transducers by Using Mixed Transfer Matrix Methods. J. Korean Phys. Soc. 2010, 57, 929–932. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, T.-F.; Al-Sarawi, S. Formulation of a simple distributed-parameter model of multilayer piezoelectric actuators. J. Intell. Mater. Syst. Struct. 2016, 27, 1485–1491. [Google Scholar] [CrossRef]
- Abdullah, A.; Pak, A. Correct Prediction of the Vibration Behavior of a High Power Ultrasonic Transducer by FEM Simulation. Int. J. Adv. Manuf. Technol. 2008, 39, 21–28. [Google Scholar] [CrossRef]
- Abdullah, A.; Pak, A.; Abdullah, M.M.; Shahidi, A.; Malaki, M. Study of the behavior of ultrasonic piezo-ceramic actuators by simulations. Electron. Mater. Lett. 2014, 10, 37–42. [Google Scholar] [CrossRef]
- Pérez-Sánchez, A.; Segura, J.A.; Rubio-Gonzalez, C.; Baldenegro-Pérez, L.A.; Soto-Cajiga, J.A. Numerical design and analysis of a langevin power ultrasonic transducer for acoustic cavitation generation. Sens. Actuators Phys. 2020, 311, 112035. [Google Scholar] [CrossRef]
- Jiang, X.; Zhu, X.; Li, S.; Geng, D.; Zhang, D. Design of Sandwich Transducer Based on the Equivalent Length Algorithm. Comput. Mater. Contin. 2019, 61, 23–28. [Google Scholar] [CrossRef]
- Wei, X.; Yang, Y.; Yao, W.; Zhang, L. PSpice Modeling of a Sandwich Piezoelectric Ceramic Ultrasonic Transducer in Longitudinal Vibration. Sensors 2017, 17, 2253. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.-C. A comparative assessment of classification methods for resonance frequency prediction of Langevin piezoelectric transducers. Appl. Math. Model. 2011, 35, 3334–3344. [Google Scholar] [CrossRef]
- Karafi, M.; Kamali, S. A continuum electro-mechanical model of ultrasonic Langevin transducers to study its frequency response. Appl. Math. Model. 2021, 92, 44–62. [Google Scholar] [CrossRef]
- Wang, J.; Qin, L.; Song, W.; Shi, Z.; Song, G. Electromechanical Characteristics of Radially Layered Piezoceramic/Epoxy Cylindrical Composite Transducers: Theoretical Solution, Numerical Simulation, and Experimental Verification. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2018, 65, 1643–1656. [Google Scholar] [CrossRef]
- Piao, C.; Kim, J.O. Vibration characteristics of an ultrasonic transducer of two piezoelectric discs. Ultrasonics 2017, 74, 72–80. [Google Scholar] [CrossRef]
- Lin, S. Coupled vibration of isotropic metal hollow cylinders with large geometrical dimensions. J. Sound Vib. 2007, 305, 308–316. [Google Scholar] [CrossRef]
- Meng, X.; Lin, S. Analysis on coupled vibration of piezoelectric ceramic stack with two piezoelectric ceramic elements. J. Acoust. Soc. Am. 2019, 146, 2170–2178. [Google Scholar] [CrossRef]
- Karafi, M.R.; Mirshabani, S.A. An Analytical Approach to Design of Ultrasonic Transducers Considering Lateral Vibrations. J. Stress Anal. 2019, 3, 47–58. [Google Scholar] [CrossRef]
- Abdullah, A.; Shahini, M.; Pak, A. An approach to design a high power piezoelectric ultrasonic transducer. J. Electroceramics 2009, 22, 369–382. [Google Scholar] [CrossRef]
- Bhuiyan, M.S.H.; Choudhury, I.A.; Dahari, M.; Nukman, Y.; Dawal, S.Z. Application of acoustic emission sensor to investigate the frequency of tool wear and plastic deformation in tool condition monitoring. Measurement 2016, 92, 208–217. [Google Scholar] [CrossRef]
- Zhang, H.; Pei, Y.-C.; Liu, Q.-J. A measuring method for the resonant frequency of flexural horn-type transducer using non-contacting piezoelectric sensor. Measurement 2020, 164, 108016. [Google Scholar] [CrossRef]
- Derusova, D.A.; Vavilov, V.P.; Druzhinin, N.V.; Kolomeets, N.P.; Chulkov, A.O.; Rubtsov, V.E.; Kolubaev, E.A. Investigating vibration characteristics of magnetostrictive transducers for air-coupled ultrasonic NDT of composites. NDT E Int. 2019, 107, 102151. [Google Scholar] [CrossRef]
- Wu, J.; Mizuno, Y.; Nakamura, K. Vibration characteristics of polymer-based Langevin transducers. Smart Mater. Struct. 2018, 27, 095013. [Google Scholar] [CrossRef]
- Al-Budairi, H.; Lucas, M.; Harkness, P. A design approach for longitudinal–torsional ultrasonic transducers. Sens. Actuators A Phys. 2013, 198, 99–106. [Google Scholar] [CrossRef]
- Albareda, A.; Casals, J.A.; Pérez, R.; de Espinosa, F.M. Nonlinear Measurements of High Power 1–3 Piezo-Air-Transducers with Burst Excitation. Ferroelectrics 2002, 273, 47–52. [Google Scholar] [CrossRef]
- DeAngelis, D.A.; Schulze, G.W. Performance of PIN-PMN-PT Single Crystal Piezoelectric versus PZT8 Piezoceramic Materials in Ultrasonic Transducers. Phys. Procedia 2015, 63, 21–27. [Google Scholar] [CrossRef]





| Cross-Sectional Position | Boundary Conditions |
|---|---|
| The right side of the cross-section | |
| The left side of the cross-section | |
| Materials | Velocity of Sound m/s | Density kg/m3 | Elastic Modulus Gpa | Poisson’s Ratio | Elastic Constants SD33 1010 m2/N | Piezoelectric Voltage Constants g33 10−3 V·m/N |
|---|---|---|---|---|---|---|
| PZT-8 | 3100 | 7600 | 72 | - | 8.5 | 25.4 |
| duralumin | 5200 | 2790 | 72 | 0.34 | - | - |
| C45E4 steel | 5170 | 7850 | 200 | 0.28 | - | - |
| Structure of the Transducer | Velocity of Sound (m/s) | Density (Kg/m3) | Elastic Modulus E (Gpa) |
|---|---|---|---|
| Piezoelectric Ceramics PZT-8 | 3367 | 7632 | 88.52 |
| Aluminum front mass block | 5019 | 3272 | 72 |
| Aluminum back mass block | 5019 | 3272 | 72 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, Y.; Xu, C.; Pan, Q.; Yu, Q.; Xiao, D. Research on Inherent Frequency and Vibration Characteristics of Sandwich Piezoelectric Ceramic Transducer. Sensors 2022, 22, 9431. https://doi.org/10.3390/s22239431
Lu Y, Xu C, Pan Q, Yu Q, Xiao D. Research on Inherent Frequency and Vibration Characteristics of Sandwich Piezoelectric Ceramic Transducer. Sensors. 2022; 22(23):9431. https://doi.org/10.3390/s22239431
Chicago/Turabian StyleLu, Yuren, Chunguang Xu, Qinxue Pan, Quanpeng Yu, and Dingguo Xiao. 2022. "Research on Inherent Frequency and Vibration Characteristics of Sandwich Piezoelectric Ceramic Transducer" Sensors 22, no. 23: 9431. https://doi.org/10.3390/s22239431
APA StyleLu, Y., Xu, C., Pan, Q., Yu, Q., & Xiao, D. (2022). Research on Inherent Frequency and Vibration Characteristics of Sandwich Piezoelectric Ceramic Transducer. Sensors, 22(23), 9431. https://doi.org/10.3390/s22239431






