Performance of Vehicular Visible Light Communications under the Effects of Atmospheric Turbulence with Aperture Averaging
Abstract
1. Introduction
2. System Description
2.1. AT Parameters and Models
2.1.1. AT Parameters
- i.
- Weak regime, < 1;
- ii.
- Moderate regime, ~1;
- iii.
- Strong regime, ;
- iv.
- Saturation regime, ∞.
2.1.2. AT Models
2.2. VLC System
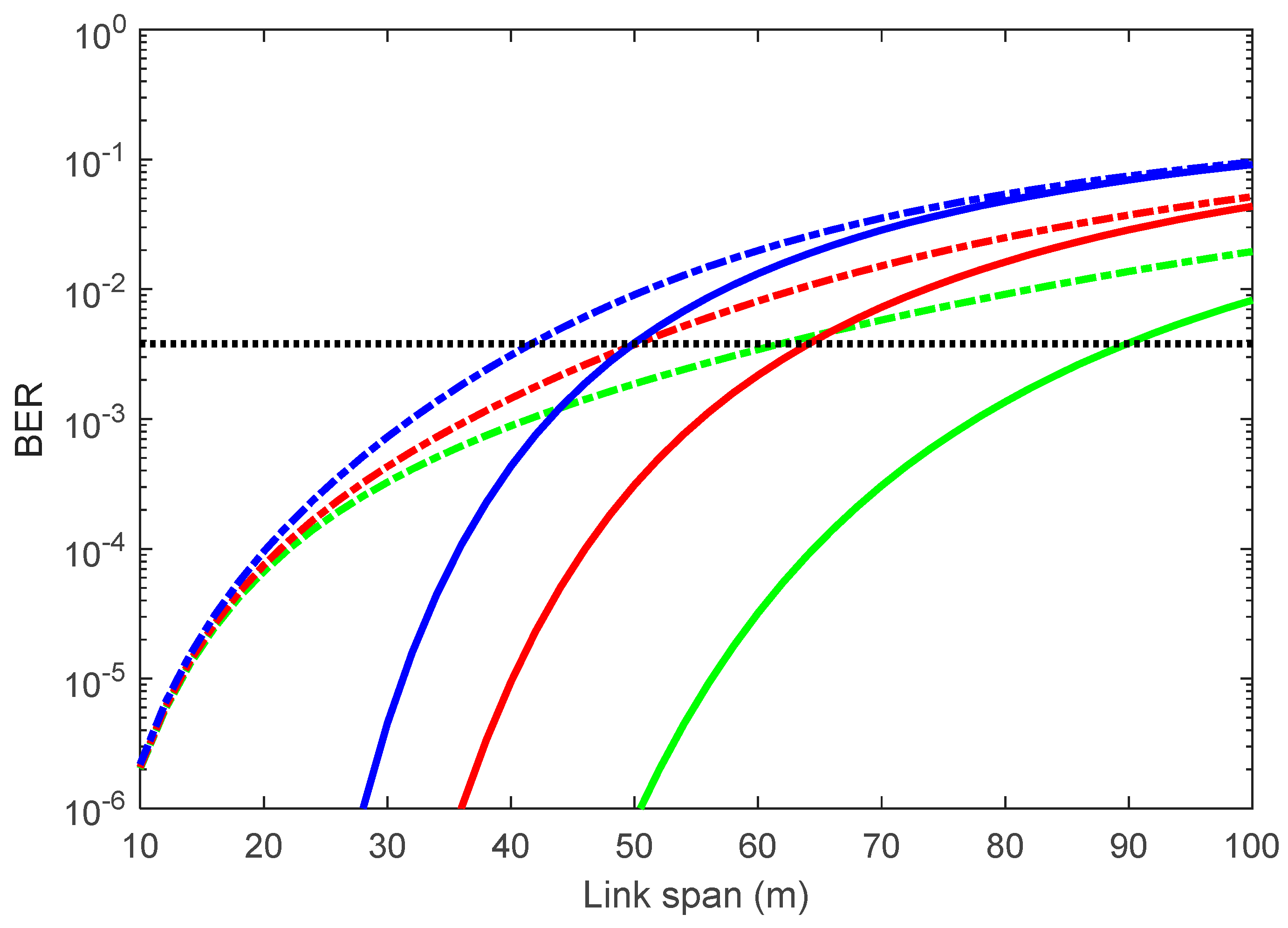
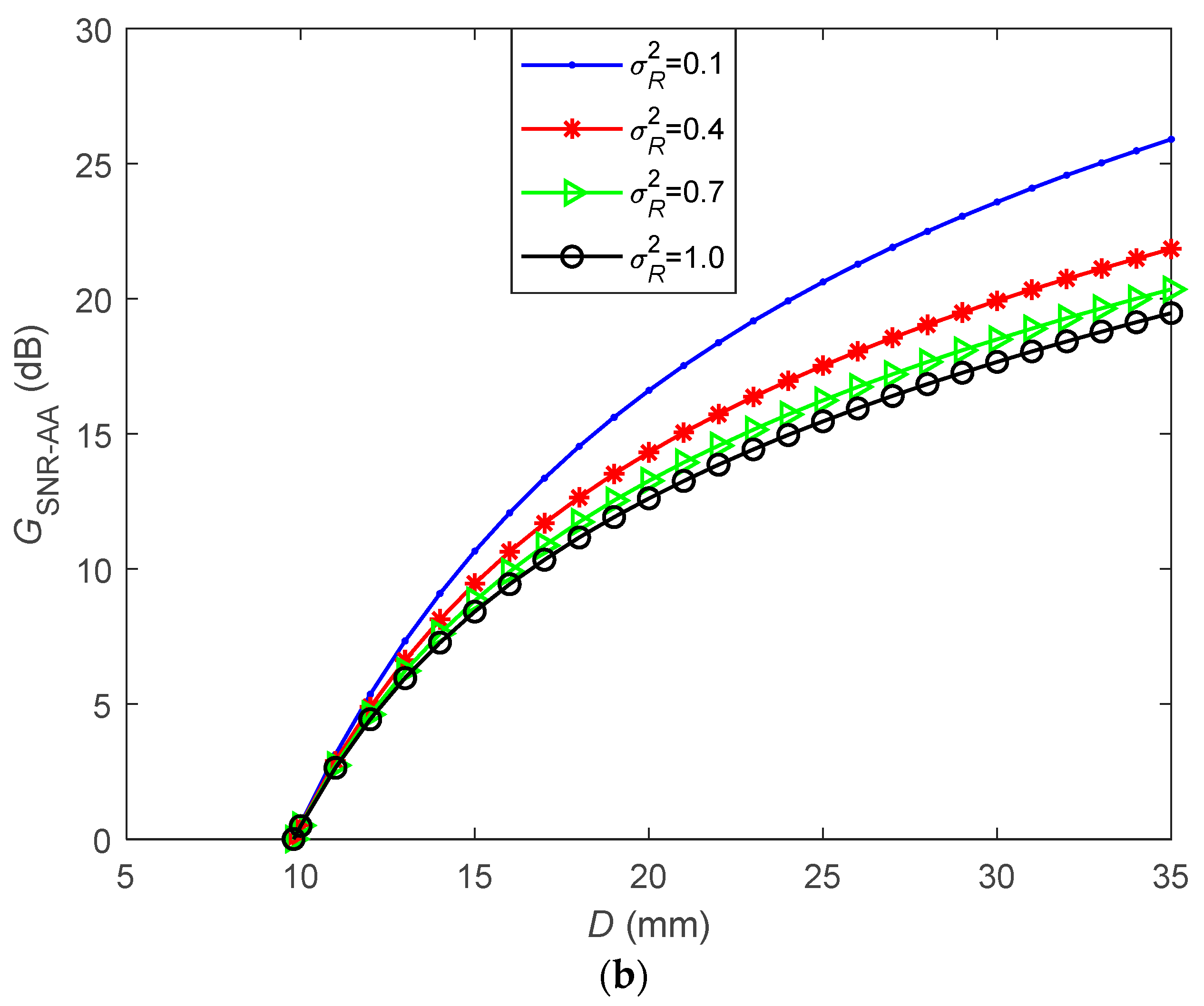
3. Simulation of AT Effects with AA
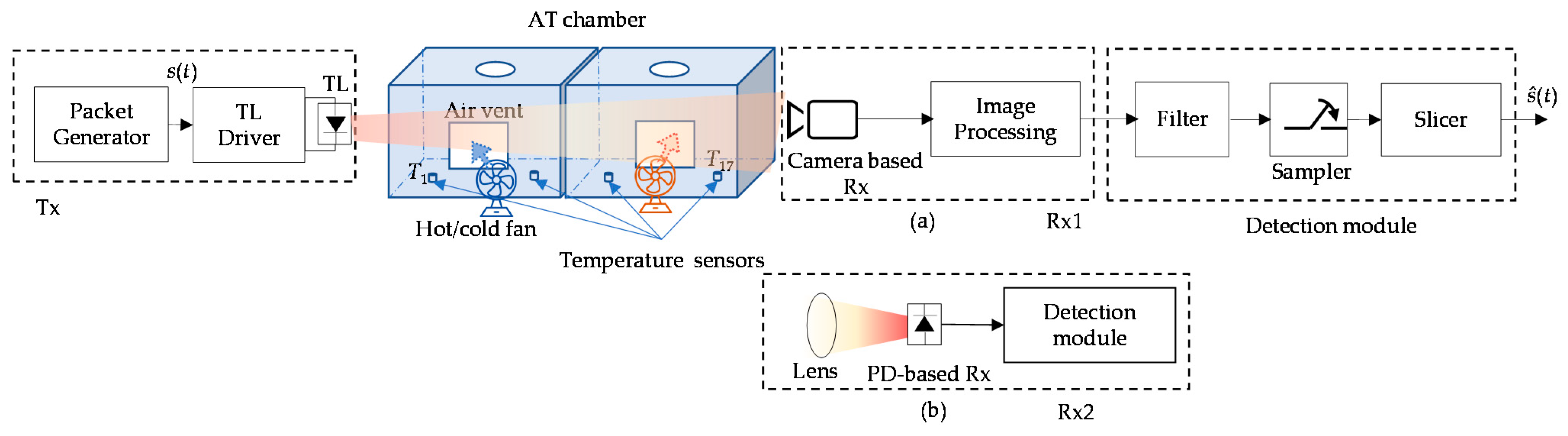
4. System Setup
4.1. Experimental Testbed
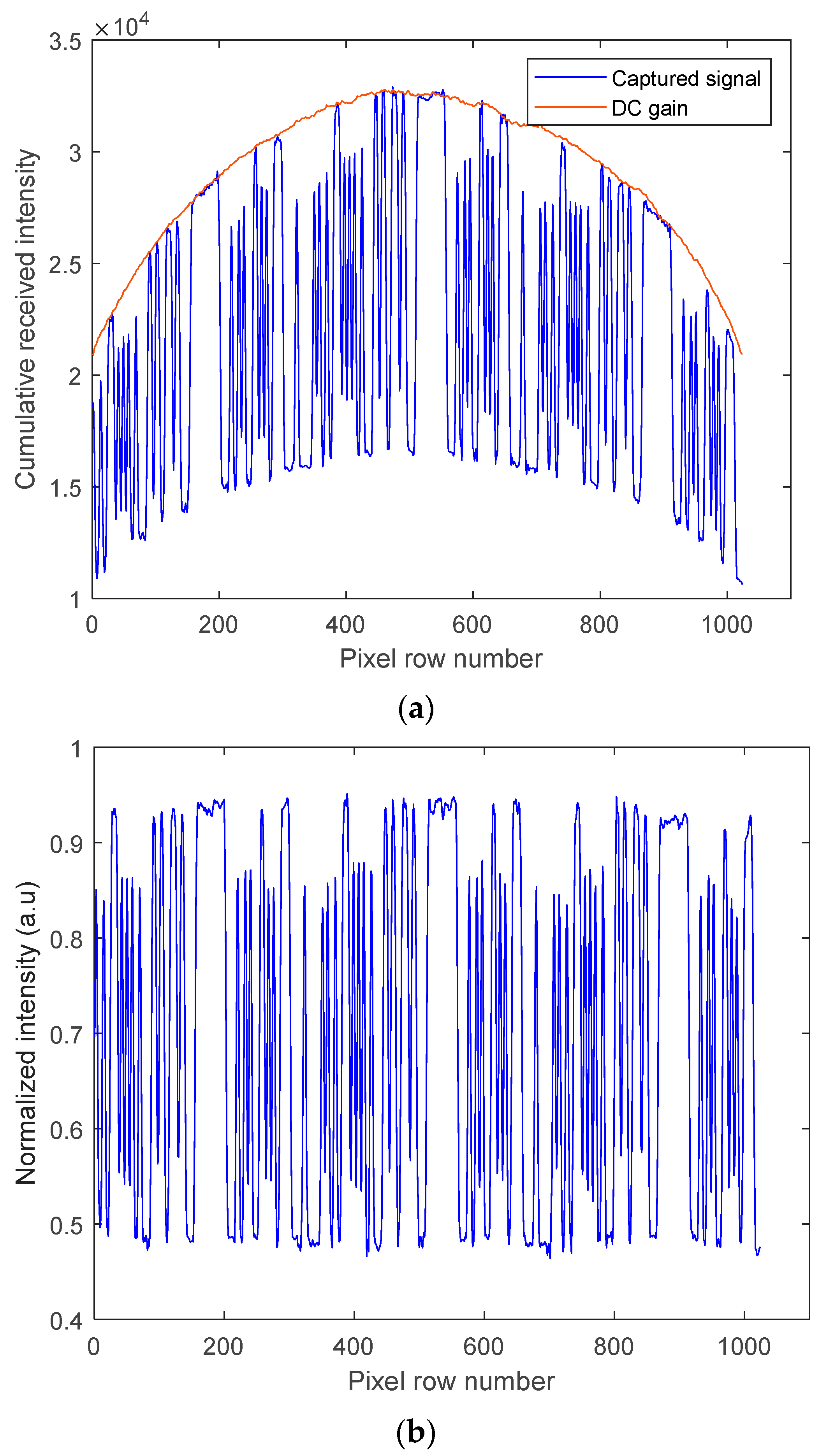
4.2. Signal Extraction
| Algorithm 1. The image processing algorithm for signal extraction. | |
| Input: Captured data frames and the DC signal only frames | |
| Output: | |
| 1 | For eachdo |
| 2 | Read sized frame = [. The RGB components of denoted as respectively, i = 1, 2, …, U and j = 1, 2, …, V represents the pixels indices of captured frame, and c = 1, 2, 3. |
| 3 | Apply grayscale conversion by calibrating the RGB components together over c, resulting . |
| 4 | Accumulate intensities for all pixels at each row where . |
| 5 | Estimate the averaged DC value by repeating previous steps on . |
| 6 | Calibrate swith respect to the averaged DC value |
| 7 | Resample with respect to the packet length |
| 8 | End. |
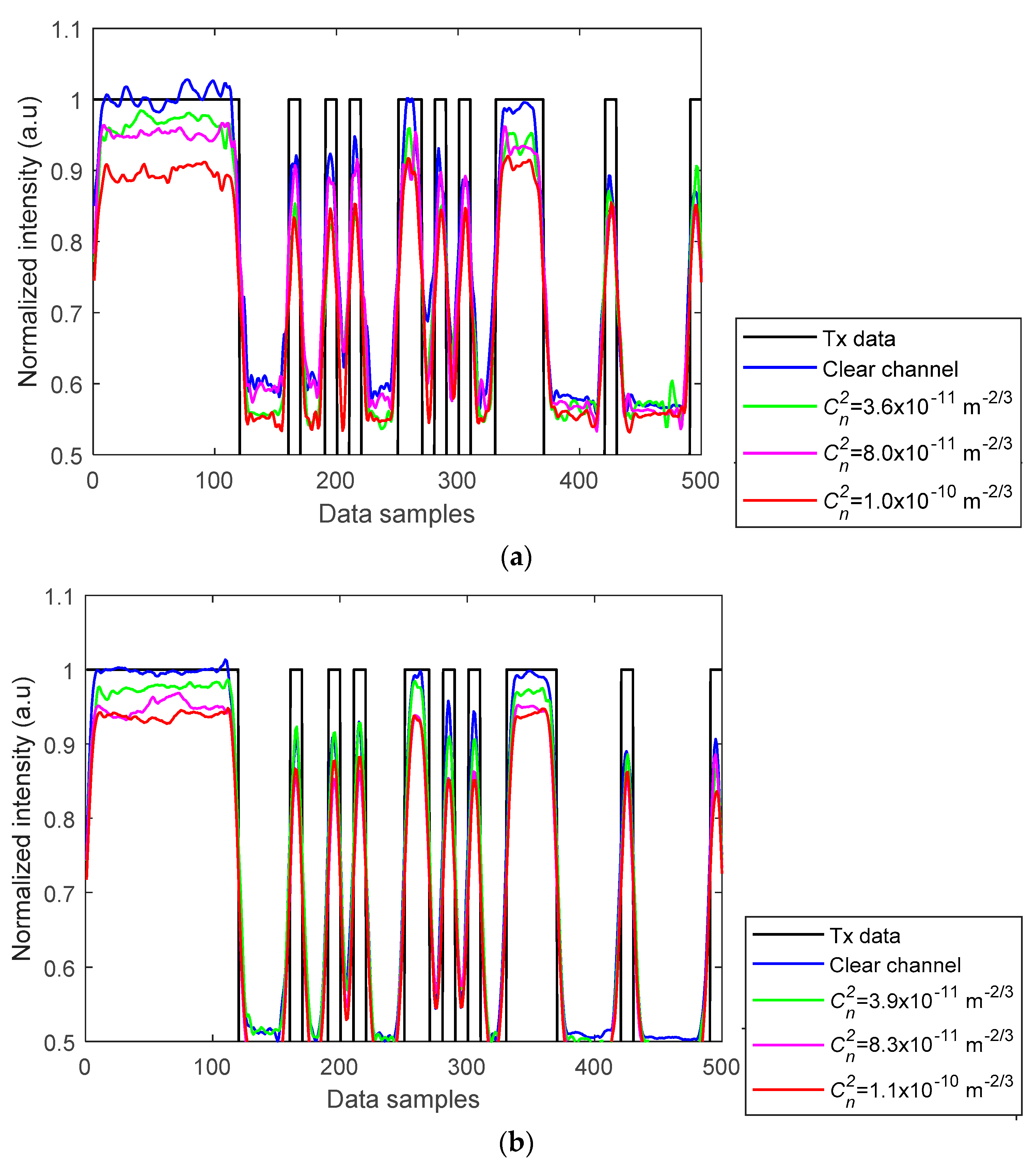
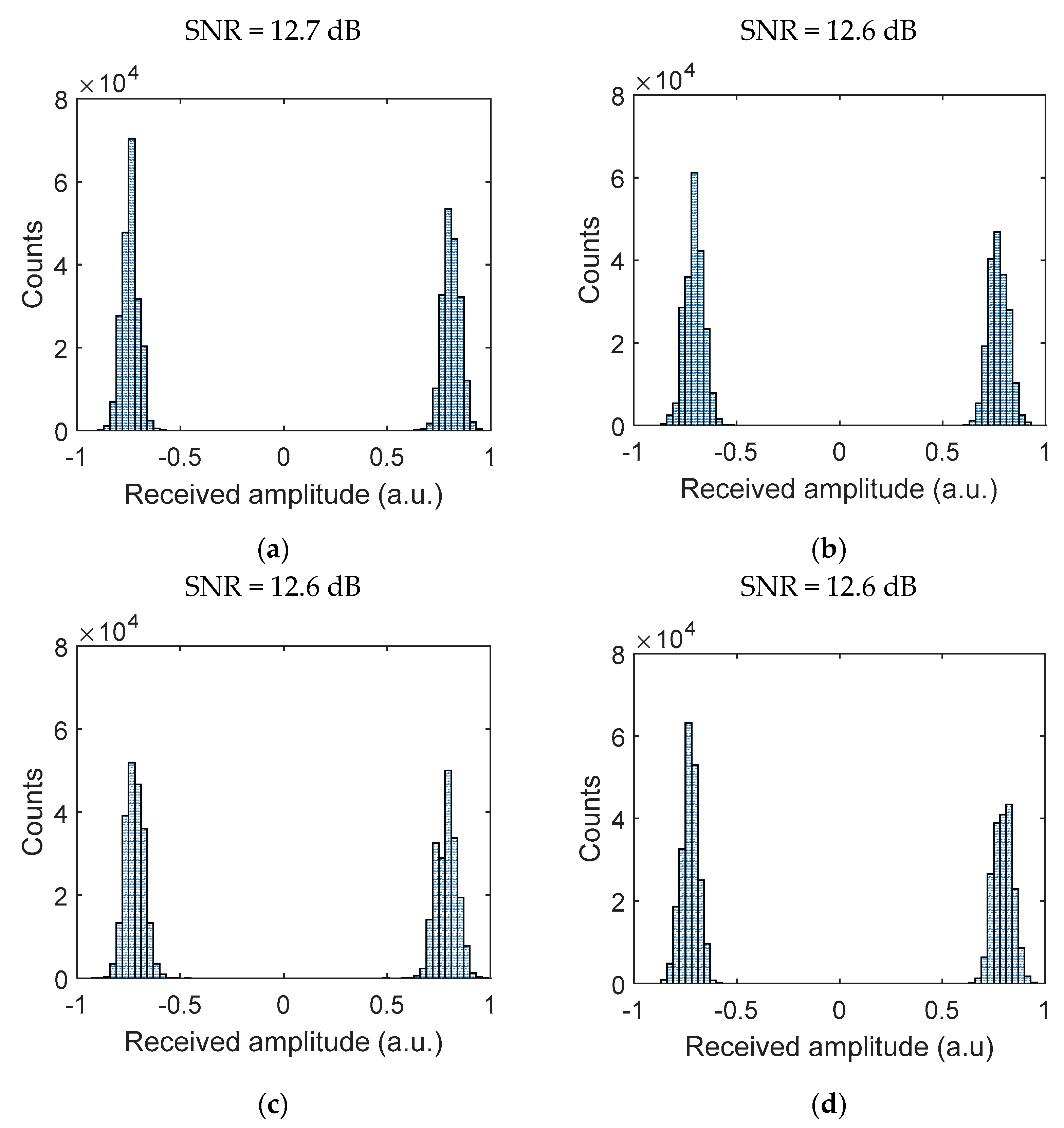
5. Results and Discussions
5.1. Camera-Based Rx
5.2. PD-Based Rx
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, S.; Lim, A.; Bevly, D. An empirical study of DSRC V2V performance in truck platooning scenarios. Digit. Commun. Netw. 2016, 2, 233–244. [Google Scholar] [CrossRef]
- Shen, W.-H.; Tsai, H.-M. Testing vehicle-to-vehicle visible light communications in real-world driving scenarios. In Proceedings of the 2017 IEEE Vehicular Networking Conference (VNC), Torino, Italy, 27–29 November 2017; pp. 187–194. [Google Scholar]
- Vivek, N.; Srikanth, S.V.; Saurabh, P.; Vamsi, T.P.; Raju, K. On field performance analysis of IEEE 802.11p and WAVE protocol stack for V2V & V2I communication. In Proceedings of the International Conference on Information Communication and Embedded Systems (ICICES2014), Chennai, India, 27–28 February 2014; pp. 1–6. [Google Scholar]
- Xu, Z.; Li, X.; Zhao, X.; Zhang, M.H.; Wang, Z. DSRC versus 4G-LTE for Connected Vehicle Applications: A Study on Field Experiments of Vehicular Communication Performance. J. Adv. Transp. 2017, 2017, 1–10. [Google Scholar] [CrossRef]
- Bazzi, A.; Masini, B.M.; Zanella, A.; Calisti, A. Visible light communications as a complementary technology for the internet of vehicles. Comput. Commun. 2016, 93, 39–51. [Google Scholar] [CrossRef]
- Car Lighting District. Halogen vs. HID vs. LED—Which is Best? 2018. Available online: https://www.carlightingdistrict.com/blogs/news/halogen-vs-hid-vs-led-which-is-best (accessed on 1 April 2019).
- Karunatilaka, D.; Zafar, F.; Kalavally, V.; Parthiban, R. LED Based Indoor Visible Light Communications: State of the Art. IEEE Commun. Surv. Tutor. 2015, 17, 1649–1678. [Google Scholar] [CrossRef]
- Pathak, P.H.; Feng, X.; Hu, P.; Mohapatra, P. Visible Light Communication, Networking, and Sensing: A Survey, Potential and Challenges. IEEE Commun. Surv. Tutor. 2015, 17, 2047–2077. [Google Scholar] [CrossRef]
- Cailean, A.M.; Dimian, M. Impact of IEEE 802.15. 7 Standard on Visible Light Communications Usage In Automotive Applications. IEEE Commun. Mag. 2017, 55, 169–175. [Google Scholar] [CrossRef]
- Cailean, A.-M.; Dimian, M. Current Challenges for Visible Light Communications Usage in Vehicle Applications: A Survey. IEEE Commun. Surv. Tutor. 2017, 19, 2681–2703. [Google Scholar] [CrossRef]
- Eso, E.; Younus, O.I.; Ghassemlooy, Z.; Zvanovec, S.; Abadi, M.M. Performances of Optical Camera-based Vehicular Communications under Turbulence Conditions. In Proceedings of the 2020 12th International Symposium on Communication Systems, Networks and Digital Signal Processing (CSNDSP), Porto, Portugal, 20–22 July 2020; pp. 1–5. [Google Scholar]
- Guo, L.-D.; Cheng, M.-J. Visible light propagation characteristics under turbulent atmosphere and its impact on communication performance of traffic system. In Proceedings of the 14th National Conference on Laser Technology and Optoelectronics (LTO 2019), Shanghai, China, 17 May 2019; 2019; Volume 11170, p. 1117047. [Google Scholar]
- Martinek, R.; Danys, L.; Jaros, R. Visible Light Communication System Based on Software Defined Radio: Performance Study of Intelligent Transportation and Indoor Applications. Electron. 2019, 8, 433. [Google Scholar] [CrossRef]
- Zheng, X.-T.; Guo, L.-X.; Cheng, M.-J.; Li, J.-T. Average BER of Maritime Visible Light Communication System in Atmospheric Turbulent Channel *. In Proceedings of the 2018 Cross Strait Quad-Regional Radio Science and Wireless Technology Conference (CSQRWC), Xuzhou, China, 21–24 July 2018; pp. 1–3. [Google Scholar] [CrossRef]
- Matus, V.; Eso, E.; Teli, S.R.; Perez-Jimenez, R.; Zvanovec, S. Experimentally Derived Feasibility of Optical Camera Communications under Turbulence and Fog Conditions. Sensors 2020, 20, 757. [Google Scholar] [CrossRef]
- Cahyadi, W.A.; Chung, Y.H.; Ghassemlooy, Z.; Hassan, N.B. Optical Camera Communications: Principles, Modulations, Potential and Challenges. Electronics 2020, 9, 1339. [Google Scholar] [CrossRef]
- Teli, S.R.; Zvanovec, S.; Ghassemlooy, Z. Performance evaluation of neural network assisted motion detection schemes implemented within indoor optical camera based communications. Opt. Express 2019, 27, 24082–24092. [Google Scholar] [CrossRef]
- Saeed, N.; Guo, S.; Park, K.-H.; Al-Naffouri, T.Y.; Alouini, M.-S. Optical camera communications: Survey, use cases, challenges, and future trends. Phys. Commun. 2019, 37, 100900. [Google Scholar] [CrossRef]
- Teli, S.R.; Matus, V.; Zvanovec, S.; Perez-Jimenez, R.; Vitek, S.; Ghassemlooy, Z. Optical Camera Communications for IoT–Rolling-Shutter Based MIMO Scheme with Grouped LED Array Transmitter. Sensors 2020, 20, 3361. [Google Scholar] [CrossRef]
- Younus., O.I.; Hassan, N.B.; Ghassemlooy, Z.; Haigh, P.A.; Zvanovec, S.; Alves, L.N.; Le Minh, H. Data Rate Enhancement in Optical Camera Communications Using an Artificial Neural Network Equaliser. IEEE Access 2020, 8, 42656–42665. [Google Scholar] [CrossRef]
- Lee, H.-Y.; Lin, H.-M.; Wei, Y.-L.; Wu, H.-I.; Tsai, H.-M.; Lin, K.C.-J. RollingLight: Enabling Line-of-Sight Light-to-Camera Communications. In Proceedings of the 13th Annual International Conference on Mobile Systems, Applications, and Services, Florence, Italy, 19–22 May 2015; pp. 167–180. [Google Scholar]
- Nguyen, T.; Islam, A.; Hossan, T.; Jang, Y.M. Current Status and Performance Analysis of Optical Camera Communication Technologies for 5G Networks. IEEE Access 2017, 5, 4574–4594. [Google Scholar] [CrossRef]
- Hassan, N.B.; Ghassemlooy, Z.; Zvanovec, S.; Biagi, M.; Vegni, A.M.; Zhang, M.; Luo, P. Non-Line-of-Sight MIMO Space-Time Division Multiplexing Visible Light Optical Camera Communications. J. Lightw. Technol. 2019, 37, 2409–2417. [Google Scholar] [CrossRef]
- Eso, E.; Teli, S.; Hassan, N.B.; Vitek, S.; Ghassemlooy, Z.; Zvanovec, S. 400 m rolling-shutter-based optical camera communications link. Opt. Lett. 2020, 45, 1059–1062. [Google Scholar] [CrossRef]
- Ghassemlooy, Z.; Popoola, W.; Rajbhandari, S. Optical Wireless Communication: System and Channel Modelling with MATLAB, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Nor, N.A.M.; Fabiyi, E.; Abadi, M.M.; Tang, X.; Ghassemlooy, Z.; Burton, A.; Mohd, N.N.A. Investigation of moderate-to-strong turbulence effects on free space optics A laboratory demonstration. In Proceedings of the 2015 13th International Conference on Telecommunications (ConTEL), Graz, Austria, 13–15 July 2015; pp. 1–5. [Google Scholar]
- Andrews, L.C.; Phillips, R.L. Laser Beam Propagation through Random Media, 2nd ed.; SPIE Press: Washington, DC, USA, 2005. [Google Scholar]
- Ghassemlooy, Z.; Le Minh, H.; Rajbhandari, S.; Perez, J.; Ijaz, M. Performance Analysis of Ethernet/Fast-Ethernet Free Space Optical Communications in a Controlled Weak Turbulence Condition. J. Light. Technol. 2012, 30, 2188–2194. [Google Scholar] [CrossRef]
- Andrews, L.; Phillips, R.; Hopen, C. Theory of Optical Scintillation with Applications, 1st ed.; SPIE Optical Engineering Press: Bellingham, WA, USA, 2001. [Google Scholar]
- Al-Habash, M.; Phillips, R.; Andrews, L. Mathematical model for the irradiance probability density function of a laser beam propagating through turbulent media. Opt. Eng. 2001, 40, 1554–1562. [Google Scholar] [CrossRef]
- Sandalidis, H.G.; Chatzidiamantis, N.D.; Karagiannidis, G.K. A Tractable Model for Turbulence- and Misalignment-Induced Fading in Optical Wireless Systems. IEEE Commun. Lett. 2016, 20, 1904–1907. [Google Scholar] [CrossRef]
- Jurado-Navas, A.; Garrido-Balsells, J.M.; Paris, J.F.; Castillo-Vazquez, M.; Puerta-Notario, A. Further insights on Málaga distribution for atmospheric optical communications. In Proceedings of the 2012 International Workshop on Optical Wireless Communications (IWOW), Pisa, Italy, 22 October 2012; pp. 1–3. [Google Scholar]
- Kahn, J.M.; Barry, J.R. Wireless infrared communications. Proc. IEEE 1997, 85, 265–298. [Google Scholar] [CrossRef]
- Komine, T.; Nakagawa, M. Fundamental analysis for visible-light communication system using LED lights. IEEE Trans. Consum. Electron. 2004, 50, 100–107. [Google Scholar] [CrossRef]
- Hui, R. Introduction to Fiber-Optic Communications; Academic Press: Cambridge, MA, USA, 2020; pp. 125–154. [Google Scholar]
- Haddad, O.; Khalighi, A.; Zvanovec, S. Channel Characterization for Optical Extra-WBAN Links Considering Local and Global User Mobility; SPIE OPTO: San Francisco, CA, USA, 2020. [Google Scholar]
- Farahneh, H.; Kamruzzaman, S.M.; Fernando, X. Differential Receiver as a Denoising Scheme to Improve the Performance of V2V-VLC Systems. In Proceedings of the 2018 IEEE International Conference on Communications Workshops (ICC Workshops), Kansas City, MO, USA, 20–24 May 2018; pp. 1–6. [Google Scholar]
- Paudel, R. Modelling and Analysis of Free Space Optical Link for Ground-to-Train Communications. Ph.D. Thesis, Northumbria University, Newcastle upon Tyne, UK, 2014. [Google Scholar]
- O’Brien, D.C.; Faulkner, G.; Le Minh, H.; Bouchet, O.; El Tabach, M.; Wolf, M.; Walewski, J.W.; Randel, S.; Nerreter, S.; Franke, M.; et al. Gigabit optical wireless for a Home Access Network. In Proceedings of the 2009 IEEE 20th International Symposium on Personal, Indoor and Mobile Radio Communications, Tokyo, Japan, 13–16 September 2009; pp. 1–5. [Google Scholar]
- Khalighi, M.-A.; Schwartz, N.; Aitamer, N.; Bourennane, S. Fading Reduction by Aperture Averaging and Spatial Diversity in Optical Wireless Systems. J. Opt. Commun. Netw. 2009, 1, 580–593. [Google Scholar] [CrossRef]
- Huynh-Thu, Q.; Ghanbari, M. Scope of validity of PSNR in image/video quality assessment. Electron. Lett. 2008, 44, 800–801. [Google Scholar] [CrossRef]
- Yang, G.; Khalighi, M.-A.; Ghassemlooy, Z.; Bourennane, S. Performance evaluation of receive-diversity free-space optical communications over correlated Gamma–Gamma fading channels. Appl. Opt. 2013, 52, 5903–5911. [Google Scholar] [CrossRef]
- Nationwide Vehicle Contracts. Understanding Car Size and Dimensions. 2020. Available online: www.nationwidevehiclecontracts.co.uk/blog/understanding-car-size-and-dimensions (accessed on 1 March 2021).
- Lee, I.E.; Ghassemlooy, Z.; Ng, W.P.; Khalighi, M.-A.; Liaw, S.-K. Effects of aperture averaging and beam width on a partially coherent Gaussian beam over free-space optical links with turbulence and pointing errors. Appl. Opt. 2015, 55, 1–9. [Google Scholar] [CrossRef]
- Uysal, M.; Li, J.; Yu, M. Error rate performance analysis of coded free-space optical links over gamma-gamma atmospheric turbulence channels. IEEE Trans. Wirel. Commun. 2006, 5, 1229–1233. [Google Scholar] [CrossRef]
- IDS Imaging and Development Systems GmbH: Brighter Images Thanks to gain- How to work with gain. Available online: https://en.ids-imaging.com/tl_files/downloads/techtip/TechTip_Gain_EN.pdf (accessed on 13 January 2020).



| Parameter | Value |
|---|---|
| 0.43 A/W | |
| 0.1–1 | |
| 30–60° | |
| 10–100 m | |
| Bandwidth B | 5 MHz |
| 0.5 W | |
| Diameter of OC D | 20–35 mm |
| Tx wavelength | 630 nm |
| Diameter of PD | m |
| 0° | |
| 298 K | |
| 100 W/m2 | |
| 50 ohms | |
| 5 nA | |
| 1 |
| Description | Value | |
|---|---|---|
| Tx | Tx peak wavelength | 630 nm |
| Tx bias current | 98 mA | |
| Transmit power | 32.4 mW | |
| PD Rx | Thorlabs PDA100A2 | |
| Responsivity | 0.43 A/W at 630 nm | |
| PD area | ||
| Bandwidth @ 0 dB gain | 11 MHz | |
| Noise equivalent power @ 960 nm | ||
| Lens focal length f | 25 mm | |
| Lens diameter D | 25 mm | |
| Camera Rx | Thorlabs DCC1645C-HQ | |
| Camera shutter speed | 600 µs | |
| Camera gain factors | 1.07, 3.96 | |
| Lens focal length f | 130 mm | |
| Lens aperture | f 5.6 | |
| Samples per frame | 2588 | |
| Pixel clock | 10 MHz | |
| Camera frame rate | 6.25 fps | |
| Camera resolution | 1280 × 1024 | |
| Packet Generator | Data format | NRZ-OOK |
| Packet generator sample rate | 11.125 kHz | |
| Number of samples per bit | 10 | |
| Channel | AT chamber dimension | 33 × 35 × 720 cm3 |
| PD | 0.2619 | |
| 0.5304 | ||
| 0.6714 | ||
| Camera | 0.2417 | |
| 0.2619 | ||
| 0.5304 | ||
| 0.5371 | ||
| 0.5573 | ||
| 0.7386 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eso, E.; Ghassemlooy, Z.; Zvanovec, S.; Sathian, J.; Abadi, M.M.; Younus, O.I. Performance of Vehicular Visible Light Communications under the Effects of Atmospheric Turbulence with Aperture Averaging. Sensors 2021, 21, 2751. https://doi.org/10.3390/s21082751
Eso E, Ghassemlooy Z, Zvanovec S, Sathian J, Abadi MM, Younus OI. Performance of Vehicular Visible Light Communications under the Effects of Atmospheric Turbulence with Aperture Averaging. Sensors. 2021; 21(8):2751. https://doi.org/10.3390/s21082751
Chicago/Turabian StyleEso, Elizabeth, Zabih Ghassemlooy, Stanislav Zvanovec, Juna Sathian, Mojtaba Mansour Abadi, and Othman Isam Younus. 2021. "Performance of Vehicular Visible Light Communications under the Effects of Atmospheric Turbulence with Aperture Averaging" Sensors 21, no. 8: 2751. https://doi.org/10.3390/s21082751
APA StyleEso, E., Ghassemlooy, Z., Zvanovec, S., Sathian, J., Abadi, M. M., & Younus, O. I. (2021). Performance of Vehicular Visible Light Communications under the Effects of Atmospheric Turbulence with Aperture Averaging. Sensors, 21(8), 2751. https://doi.org/10.3390/s21082751










