Calibration Analysis of High-G MEMS Accelerometer Sensor Based on Wavelet and Wavelet Packet Denoising
Abstract
1. Introduction
2. Algorithm
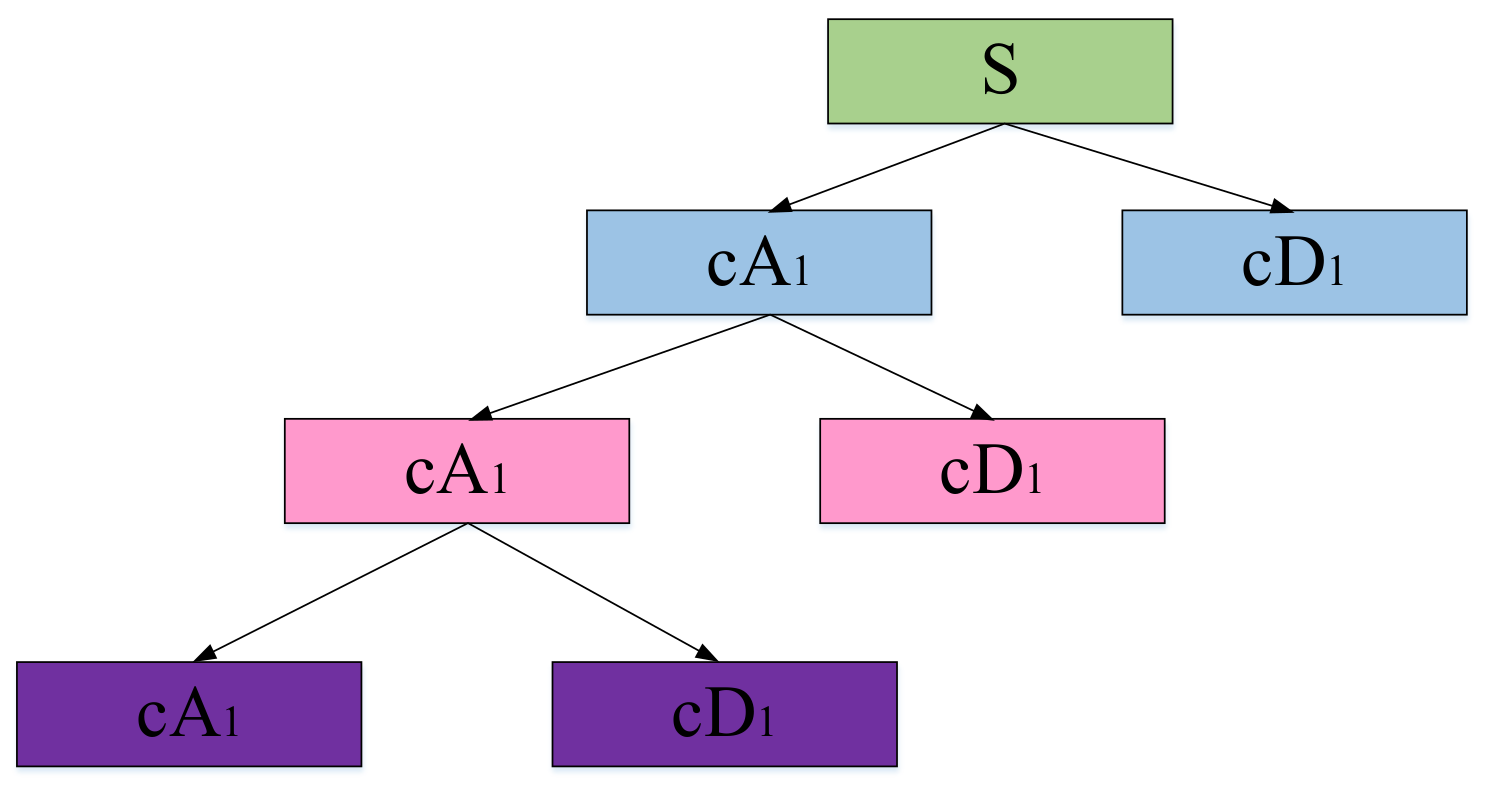
2.1. Wavelet Adaptive Decomposition
2.2. Wavelet Packet “Best Tree”
2.3. Threshold Function
3. High-G MEMS Accelerometer
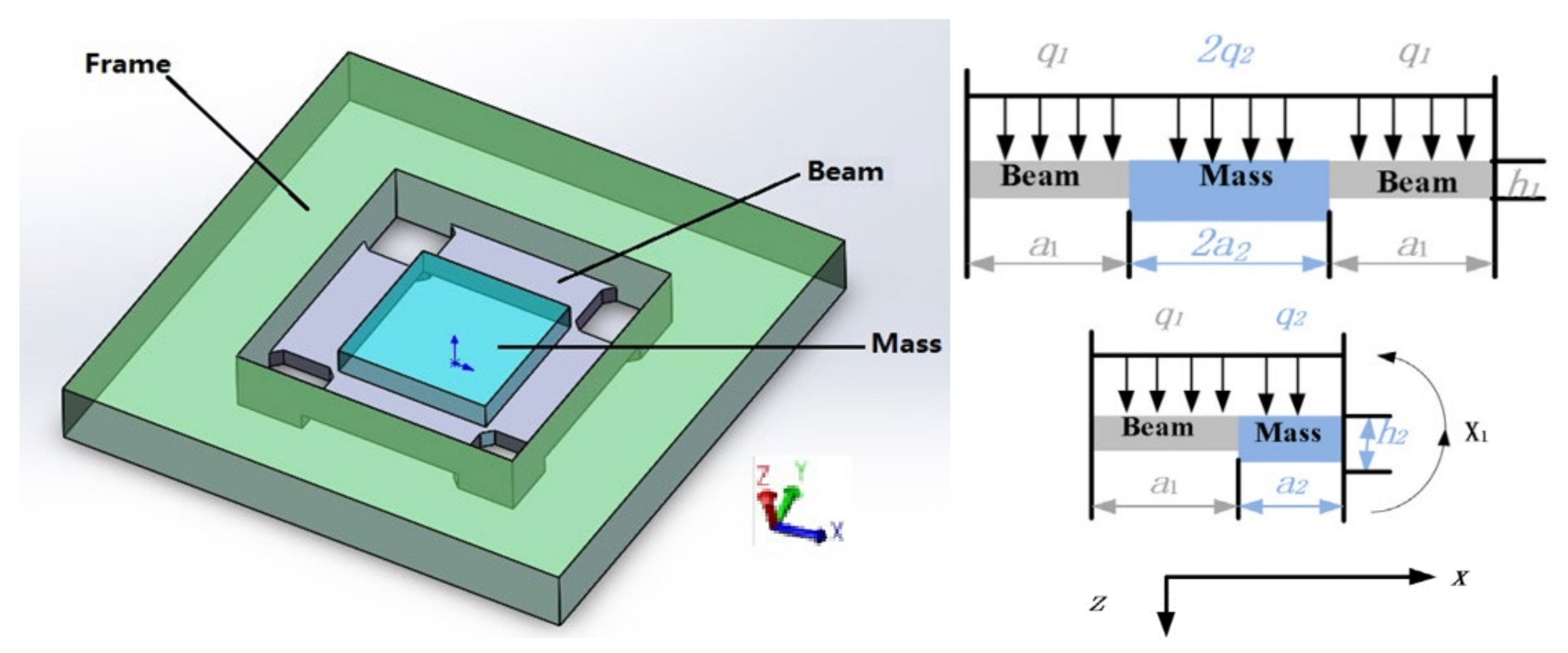
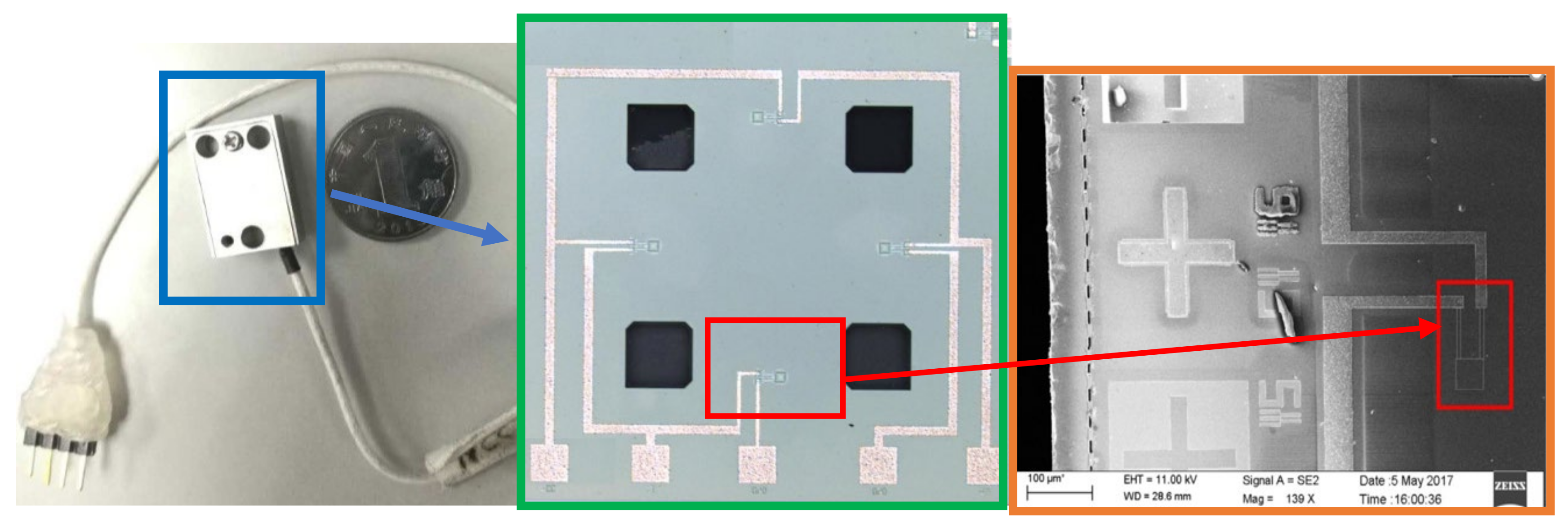
3.1. Structure and Structural Parameters of the HGMA
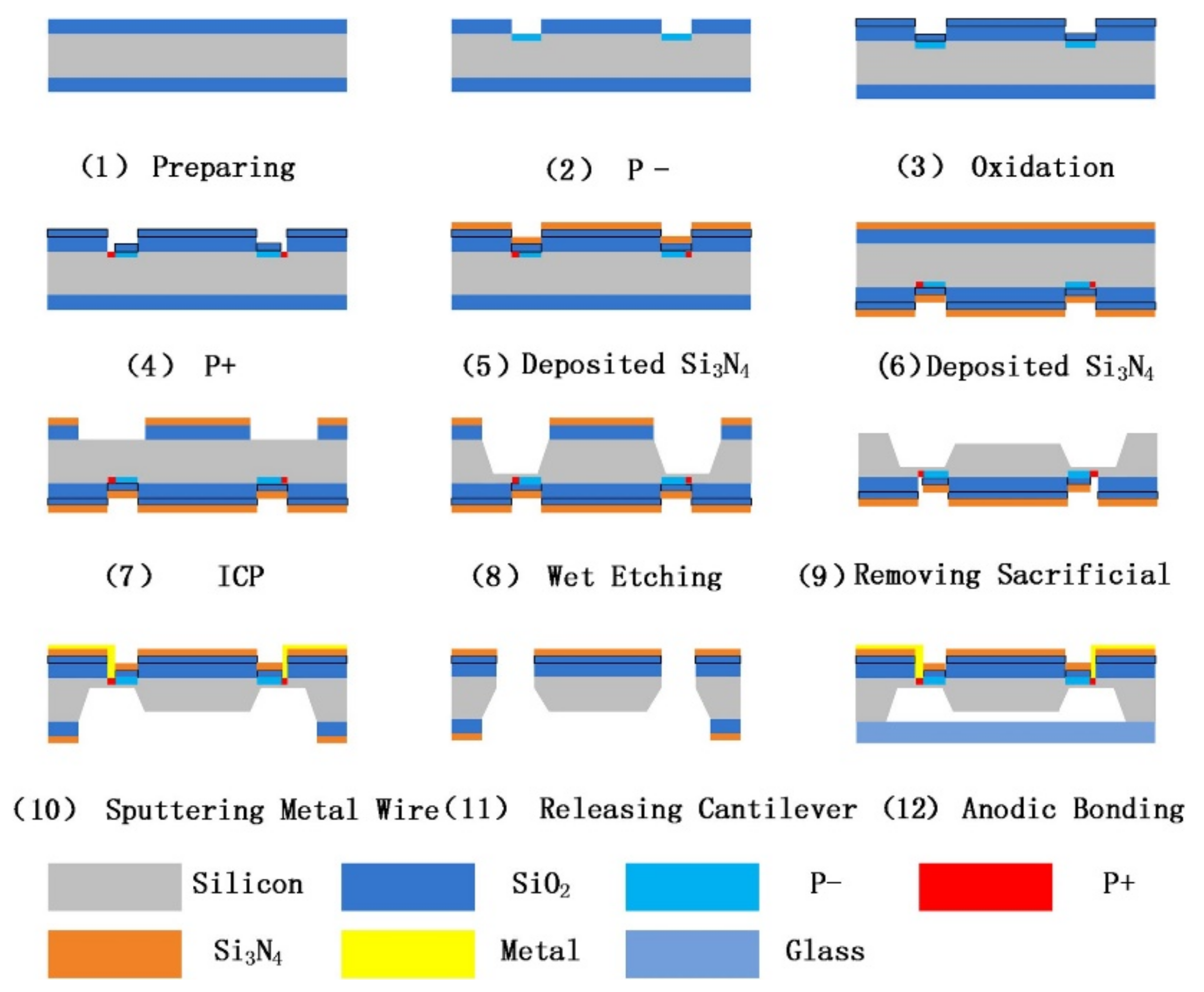
3.2. Process of the HGMA
4. Experiment Analysis
4.1. Simulation
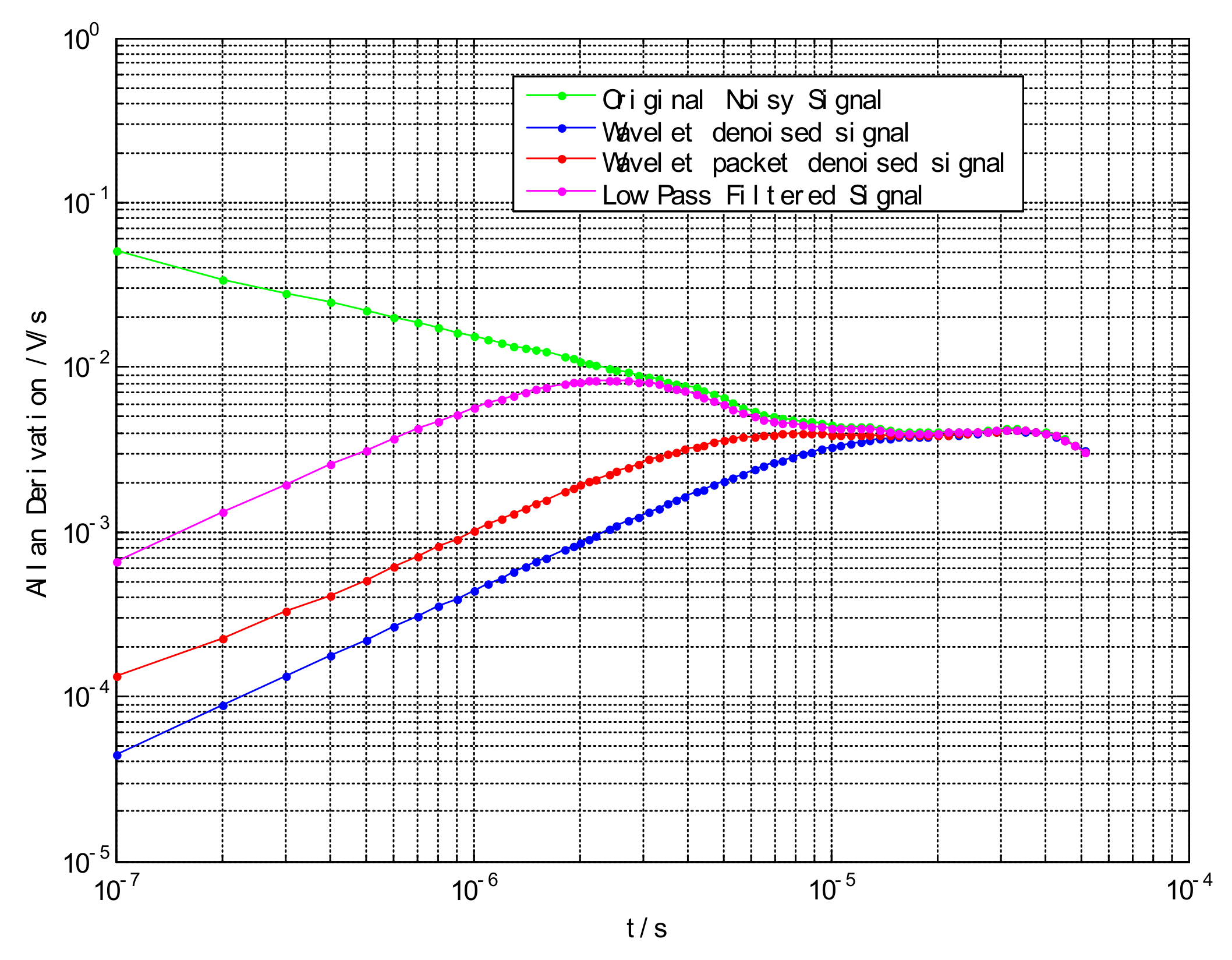
4.2. Experiment and Discussion
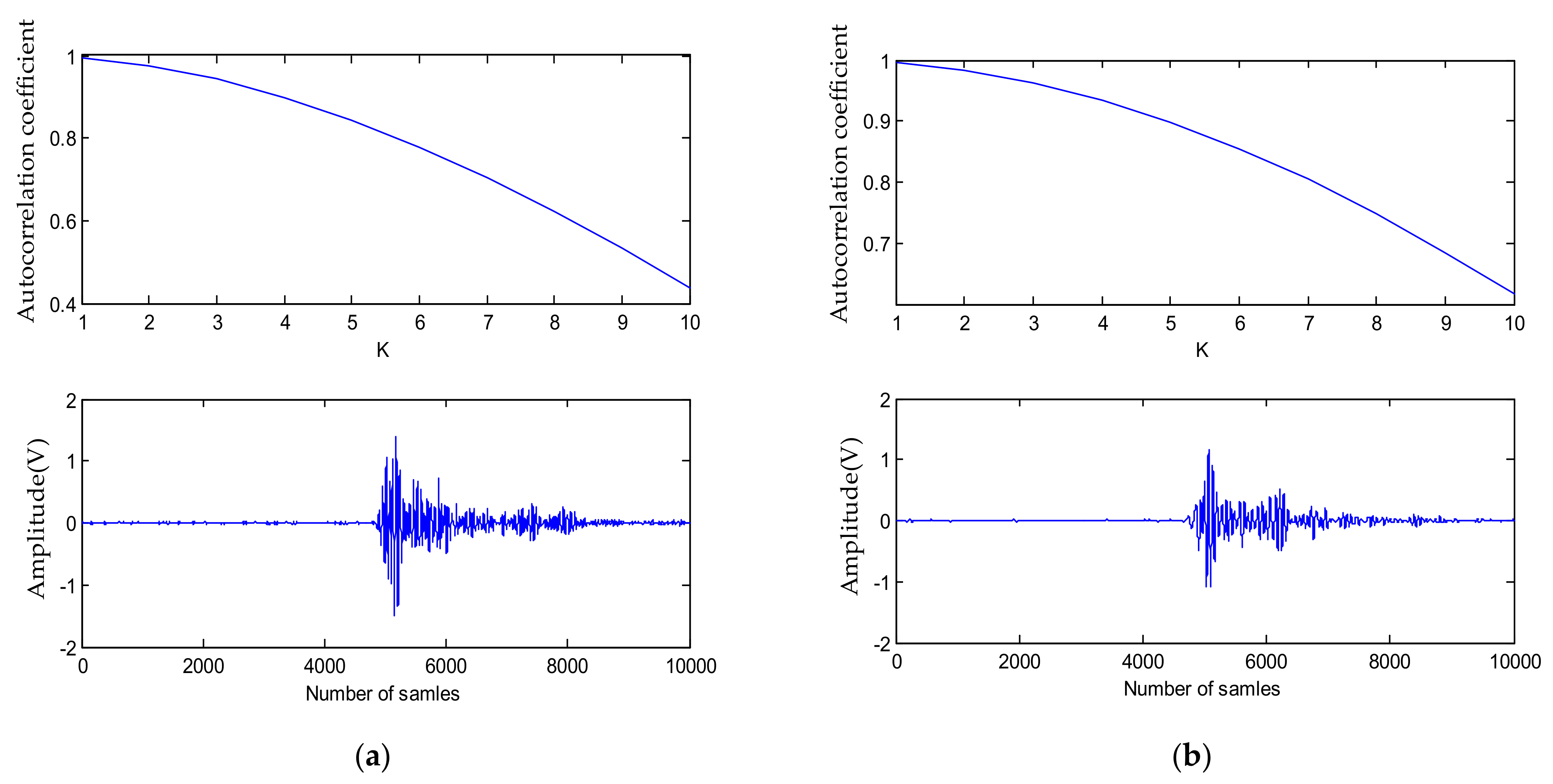
4.2.1. Experiment Analysis
- Reflect and keep the power in the first sideline cutoff frequency, which means keeping the real peak amplitude at the calibration shock peak frequency.
- Pick up the frequency information of the resonant frequency of HGMA, and this is helpful to recognize the mechanical characteristic of the HGMA sensor.
- Cut off the high-frequency noise during the HGMA calibration.
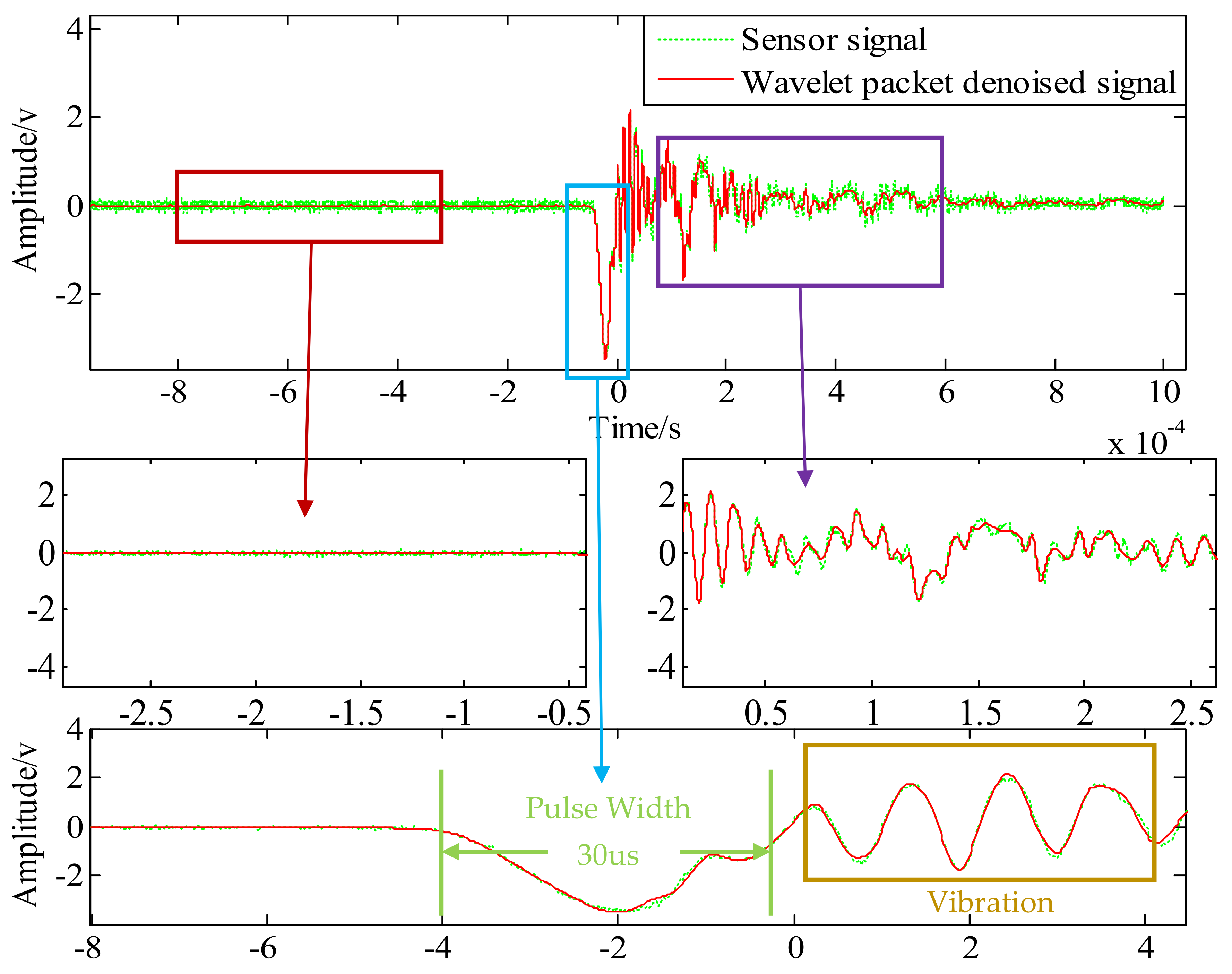
4.2.2. Static Calibration
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bang, W.C.; Chang, W.; Kang, K.-H.; Cjoi, E.-S.; Potanin, A.; Kim, D.-Y. Self-contained spatial input device for wearable computers. In Proceedings of the Seventh IEEE International Symposium on Wearable Computers, White Plains, NY, USA, 21–23 October 2003; pp. 26–34, ISBN 0-7695-2034-0. [Google Scholar]
- Shen, S.C.; Chen, C.J.; Huang, H.J. A new calibration method for MEMS inertial sensor module. In Proceedings of the 2010 11th IEEE International Workshop on Advanced Motion Control (AMC), Nagaoka, Japan, 21–24 March 2010; pp. 106–111. [Google Scholar] [CrossRef]
- Cao, H.; Zhang, Y.; Han, Z.; Shao, X.; Gao, J.; Huang, K.; Shi, Y.; Tang, J.; Shen, C.; Liu, J. Pole-zero temperature compensation circuit design and experiment for dual-mass MEMS gyroscope bandwidth expansion. IEEE/ASME Trans. Mechatron. 2019, 24, 677–688. [Google Scholar] [CrossRef]
- Guo, H.; Chen, Y.; Wu, D.; Zhao, R.; Tang, J.; Ma, Z.; Xue, C.; Zhang, W.; Liu, J. Plasmon-enhanced sensitivity of spin-based sensors based on a diamond ensemble of nitrogen vacancy color centers. Opt. Lett. 2017, 42, 403–406. [Google Scholar] [CrossRef] [PubMed]
- Cao, H.; Zhang, Y.; Shen, C.; Liu, Y.; Wang, X. Temperature energy influence compensation for MEMS vibration gyroscope based on RBF NN-GA-KF method. Shock Vib. 2018. [Google Scholar] [CrossRef]
- Yuan, K.; Guo, W.; Su, Y.; Shi, Y.; Lei, J.; Guo, H. Study on several key problems in shock calibration of high-g accelerometers using Hopkinson bar. Sens. Actuators A Phys. 2017, 258, 1–13. [Google Scholar] [CrossRef]
- Makowski, M.; Napieralski, A.; Pekoslawski, B.; Pietrzak, P. Adaptive subband filtering method for MEMS accelerometer noise reduction. Sens. Transducers J. 2008, 3, 14–24. [Google Scholar]
- Pietrzak, P. Application of micromachined accelerometers for vibration measurements in condition evaluation systems for large rotating machines. Zeszyty Naukowe. Elektryka. Politechnika Łódzka. 2007, 111, 81–87. [Google Scholar]
- Skaloud, J. Optimizing Georeferencing of Airborne Survey Systems by INS/DGPS. Doctoral Thesis, University of Calgary, Calgary, AB, Canada, March 1999. [Google Scholar]
- Bhatt, D.; Aggarwal, P.; Bhattacharya, P.; Devabhaktuni, V.K. An enhanced MEMS error modeling approach based on nu-support vector regression. Sensors 2012, 12, 9448–9466. [Google Scholar] [CrossRef] [PubMed]
- Lu, Q.; Pang, L.; Huang, H.; Shen, C.; Cao, H.; Shi, Y.; Liu, J. High-G calibration denoising method for High-G MEMS accelerometer based on EMD and wavelet threshold. Micromachines 2019, 10, 134. [Google Scholar] [CrossRef] [PubMed]
- El-Diasty, M.; El-Rabbany, A.; Pagiatakis, S. Temperature variation effects on stochastic characteristics for low-cost MEMS-based inertial sensor error. Meas. Sci. Technol. 2007, 18, 3321–3328. [Google Scholar] [CrossRef]
- Zhang, R.; Bouman, C.A.; Thibault, J.-B.; Sauer, K.D. Gaussian mixture Markov random field for image denoising and reconstruction. In Proceedings of the 2013 IEEE Global Conference on Signal and Information Processing, Austin, TX, USA, 3–5 December 2013; pp. 1089–1092. [Google Scholar]
- Zapata, O.; Pedreros, F.; Torres, S.N. An experimental validation of the Gauss-Markov model for nonuniformity noise in infrared focal plane array sensors. In Proceedings of the SPIE Defense, Security, and Sensing, Baltimore, MD, USA, 18 May 2012; Volume 8355, p. 47. [Google Scholar]
- Li, Y.; Xu, C.; Yi, L.; Fang, R. A data-driven approach for denoising GNSS position time series. J. Geod. 2017, 92, 905–922. [Google Scholar] [CrossRef]
- Shen, C.; Yang, J.; Tang, J.; Liu, J.; Cao, H. Note: Parallel processing algorithm of temperature and noise error for micro-electro-mechanical system gyroscope based on variational mode decomposition and augmented nonlinear differentiator. Rev. Sci. Instrum. 2018, 89, 076107. [Google Scholar] [CrossRef] [PubMed]
- Messer, S.R.; Agzarian, J.; Abbott, D. Optimal wavelet denoising for smart biomonitor systems. In Proceedings of the Smart Materials and MEMS, Melbourne, Australia, 16 March 2001; Volume 4236, pp. 66–79. [Google Scholar] [CrossRef]
- Xu, X.; Luo, M.; Tan, Z.; Pei, R. Echo signal extraction method of laser radar based on improved singular value decomposition and wavelet threshold denoising. Infrared Phys. Technol. 2018, 92, 327–335. [Google Scholar] [CrossRef]
- Chen, D.; Han, J. Application of wavelet neural network in signal processing of MEMS accelerometers. Microsyst. Technol. 2010, 17, 1–5. [Google Scholar] [CrossRef]
- Yan, Z.; Hou, B.; Zhang, J.; Shen, C.; Shi, Y.; Tang, J.; Cao, H.; Liu, J. MEMS accelerometer calibration denoising method for hopkinson bar system based on LMD-SE-TFPF. IEEE Access 2019, 7, 113901–113915. [Google Scholar] [CrossRef]
- Zhu, M.; Pang, L.; Xiao, Z.; Shen, C.; Cao, H.; Shi, Y.; Liu, J. Temperature drift compensation for High-G MEMS accelerometer based on RBF NN improved method. Appl. Sci. 2019, 9, 695. [Google Scholar] [CrossRef]
- Huang, J.; Tian, G.; Xie, J.; Li, H.; Chen, X. Self-adaptive decomposition level de-noising method based on wavelet transform. TELKOMNIKA Indones. J. Electr. Eng. 2012, 10, 1015–1020. [Google Scholar] [CrossRef]
- Cao, H.; Zhang, Z.; Zheng, Y.; Guo, H.; Zhao, R.; Shi, Y.; Chou, X. A New joint denoising algorithm for High-G calibration of MEMS accelerometer based on VMD-PE-wavelet threshold. Shock Vib. 2021, 2021, 1–16. [Google Scholar] [CrossRef]
- Lu, Q.; Shen, C.; Cao, H.; Shi, Y.; Liu, Y. Fusion algorithm-based temperature compensation method for High-G MEMS accelerometer. Shock Vib. 2019, 2019, 3154845. [Google Scholar] [CrossRef]
- Shi, Y.; Zhao, Y.; Feng, H.; Cao, H.; Tang, J.; Li, J.; Zhao, R.; Liu, J. Design, fabrication and calibration of a high-G MEMS accelerometer. Sens. Actuators A Phys. 2018, 279, 733–742. [Google Scholar] [CrossRef]
- Shi, Y.; Wen, X.; Zhao, Y.; Zhao, R.; Cao, H.; Liu, J. Investigation and experiment of high shock packaging technology for High-G MEMS accelerometer. IEEE Sens. J. 2020, 1. [Google Scholar] [CrossRef]
- Zu, J.; Ma, T.H.; Pei, D.X. New Concept Dynamic Test, 1st ed.; National Defense Industry Press: Beijing, China, 2016; pp. 106–109. ISBN 978-7-118-10775-3. [Google Scholar]








| Mode Shapes | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Resonant Frequency/kHz | 408 | 667 | 671 | 1119 |
| Denoising Method | SNR | RMSE |
|---|---|---|
| Wavelet Threshold Denoising | 20.412 | 0.200 |
| Wavelet Packet Threshold Denoising | 12.875 | 0.151 |
| LPF Denoising | 17.829 | 0.270 |
| Parameter | Sensor Signal | Wavelet Threshold Denoising | Wavelet Packet Threshold Denoising |
|---|---|---|---|
| Peak (g) | 34,070 | 35,225 | 34,759 |
| Integral Value | 3.297 × 10−4 | 2.959 × 10−4 | 3.084 × 10−4 |
| SNR | 11.518 | 13.042 | |
| RMSE | 0.109 | 0.0916 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Y.; Zhang, J.; Jiao, J.; Zhao, R.; Cao, H. Calibration Analysis of High-G MEMS Accelerometer Sensor Based on Wavelet and Wavelet Packet Denoising. Sensors 2021, 21, 1231. https://doi.org/10.3390/s21041231
Shi Y, Zhang J, Jiao J, Zhao R, Cao H. Calibration Analysis of High-G MEMS Accelerometer Sensor Based on Wavelet and Wavelet Packet Denoising. Sensors. 2021; 21(4):1231. https://doi.org/10.3390/s21041231
Chicago/Turabian StyleShi, Yunbo, Juanjuan Zhang, Jingjing Jiao, Rui Zhao, and Huiliang Cao. 2021. "Calibration Analysis of High-G MEMS Accelerometer Sensor Based on Wavelet and Wavelet Packet Denoising" Sensors 21, no. 4: 1231. https://doi.org/10.3390/s21041231
APA StyleShi, Y., Zhang, J., Jiao, J., Zhao, R., & Cao, H. (2021). Calibration Analysis of High-G MEMS Accelerometer Sensor Based on Wavelet and Wavelet Packet Denoising. Sensors, 21(4), 1231. https://doi.org/10.3390/s21041231







