Strength Development Monitoring of Cemented Paste Backfill Using Guided Waves
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Specimens for Test
2.2.1. Background
2.2.2. Specimens for Guided Wave Test
2.2.3. Specimens for Uniaxial Compression Strength (UCS) Test
2.3. Guided Wave Test
2.3.1. Test Set-Up and Procedure
2.3.2. Guided Wave Velocity and Attenuation Data Processing
2.4. UCS Test
3. Results and Discussion
3.1. Optimization of Excitation Wave
3.1.1. Optimization of the Excitation Wave Cycle
3.1.2. Optimization of the Excitation Wave Frequency
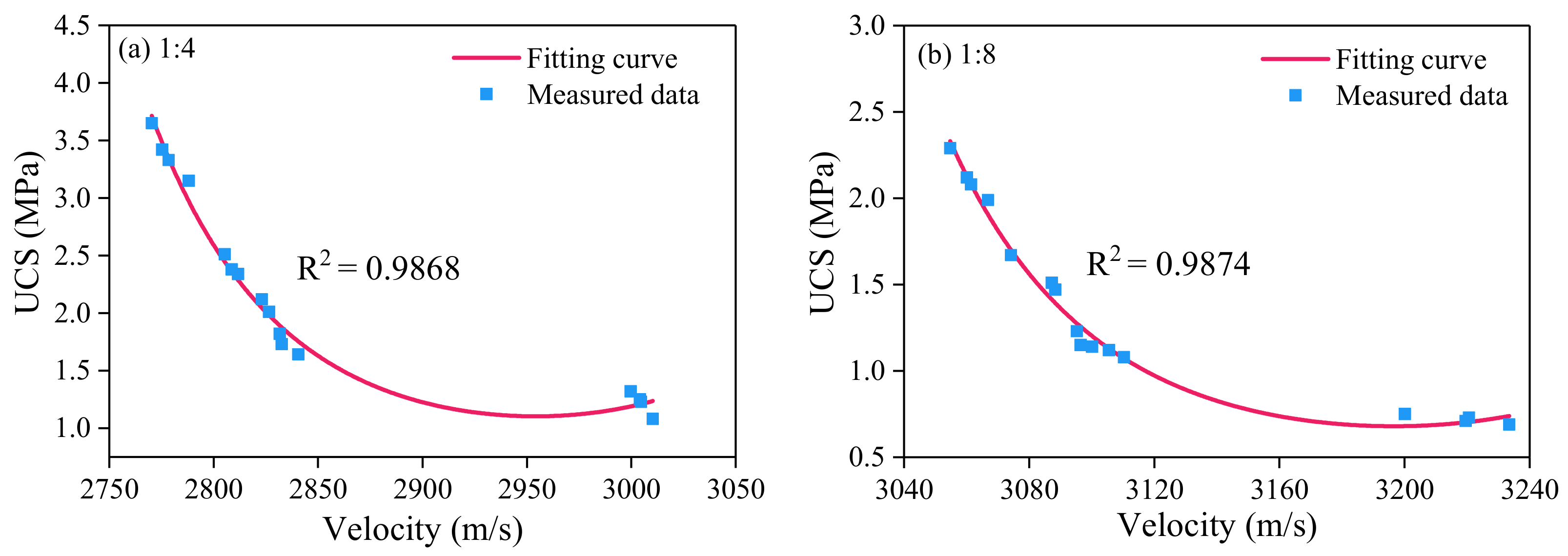
3.2. Relationship between UCS and Velocity of Guided Wave
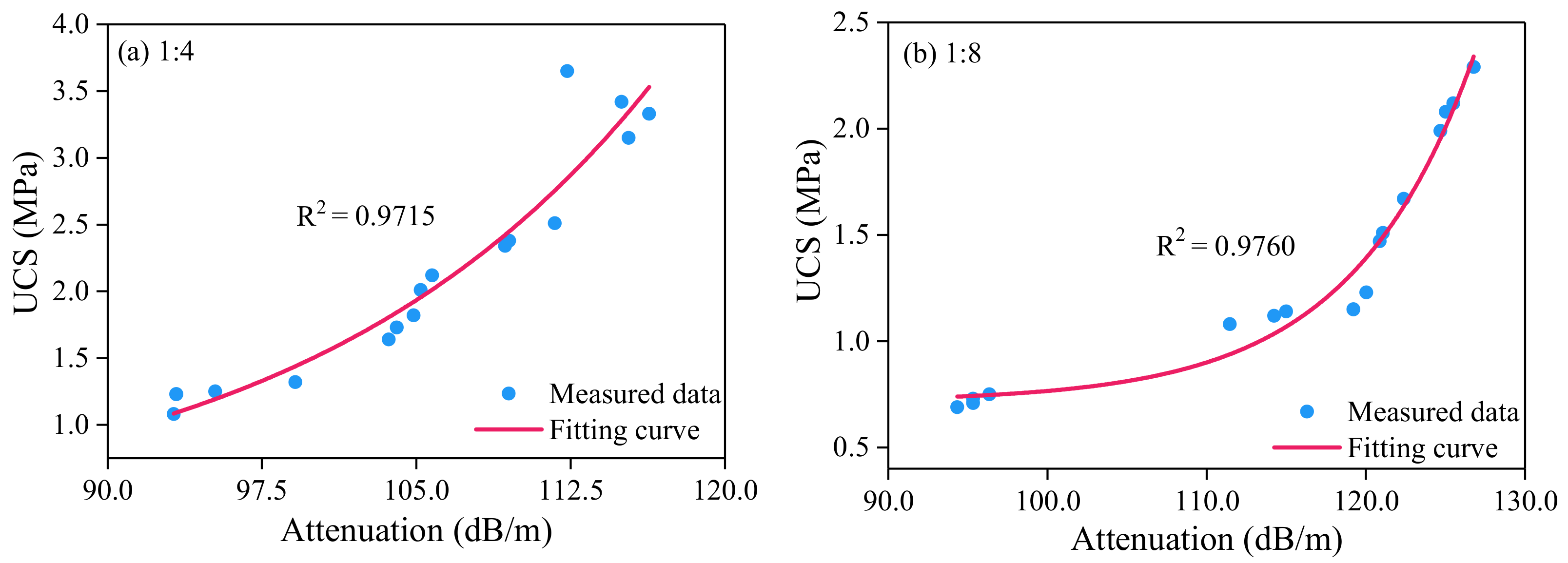
3.3. Relationship between UCS and Guided Wave Attenuation
4. Conclusions
- (1)
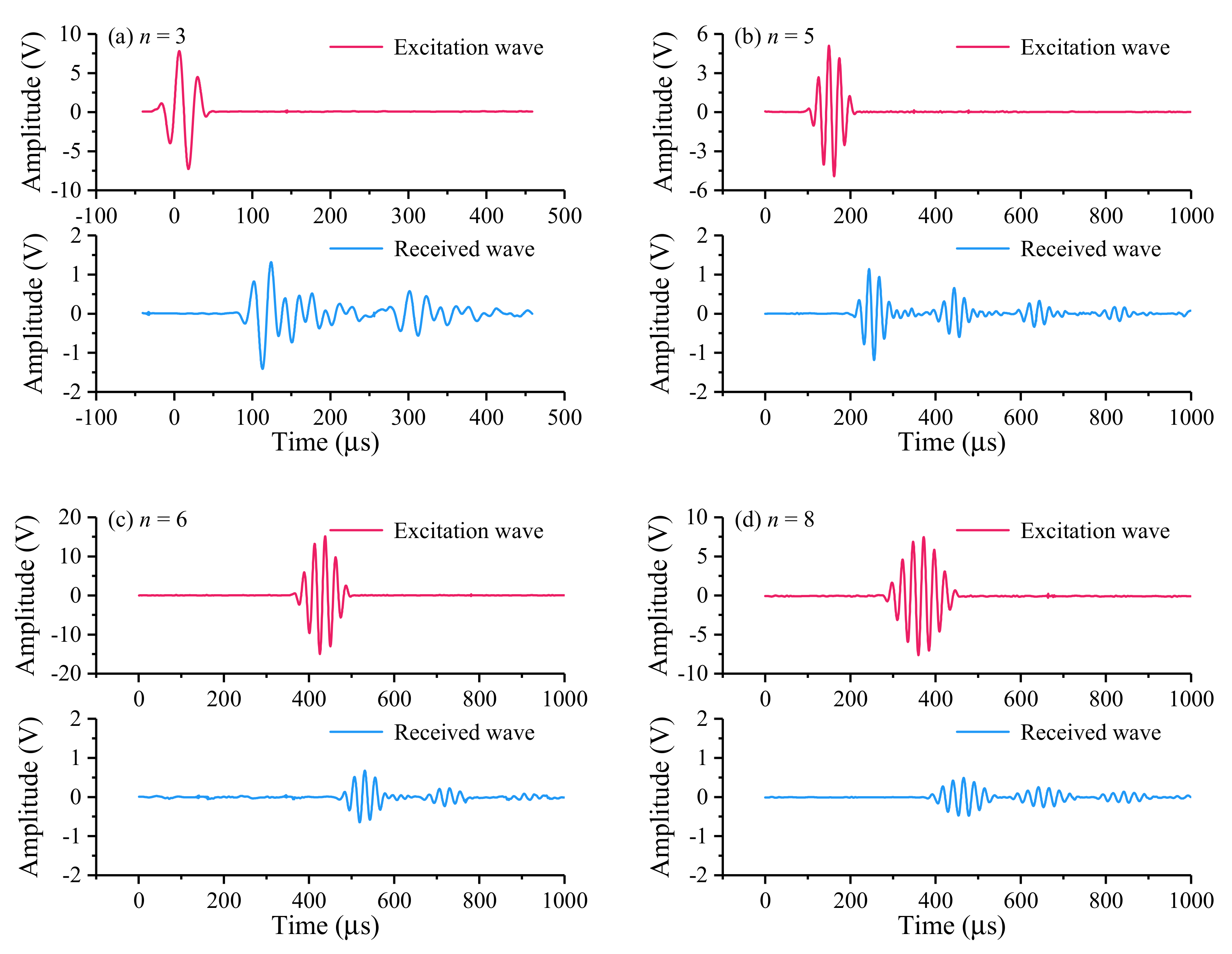
- Guided waves with a frequency of 40 kHz and cycle numbers of 3, 5, 6, and 8 were used to detect cemented paste backfill samples. The results show that the larger cycle of the excitation wave, the easier the excitation wave and received wave overlap; they are difficult to distinguish. Combined with the attenuation of guided waves, the optimal detection cycle of the guided wave was determined to be 5.
- (2)
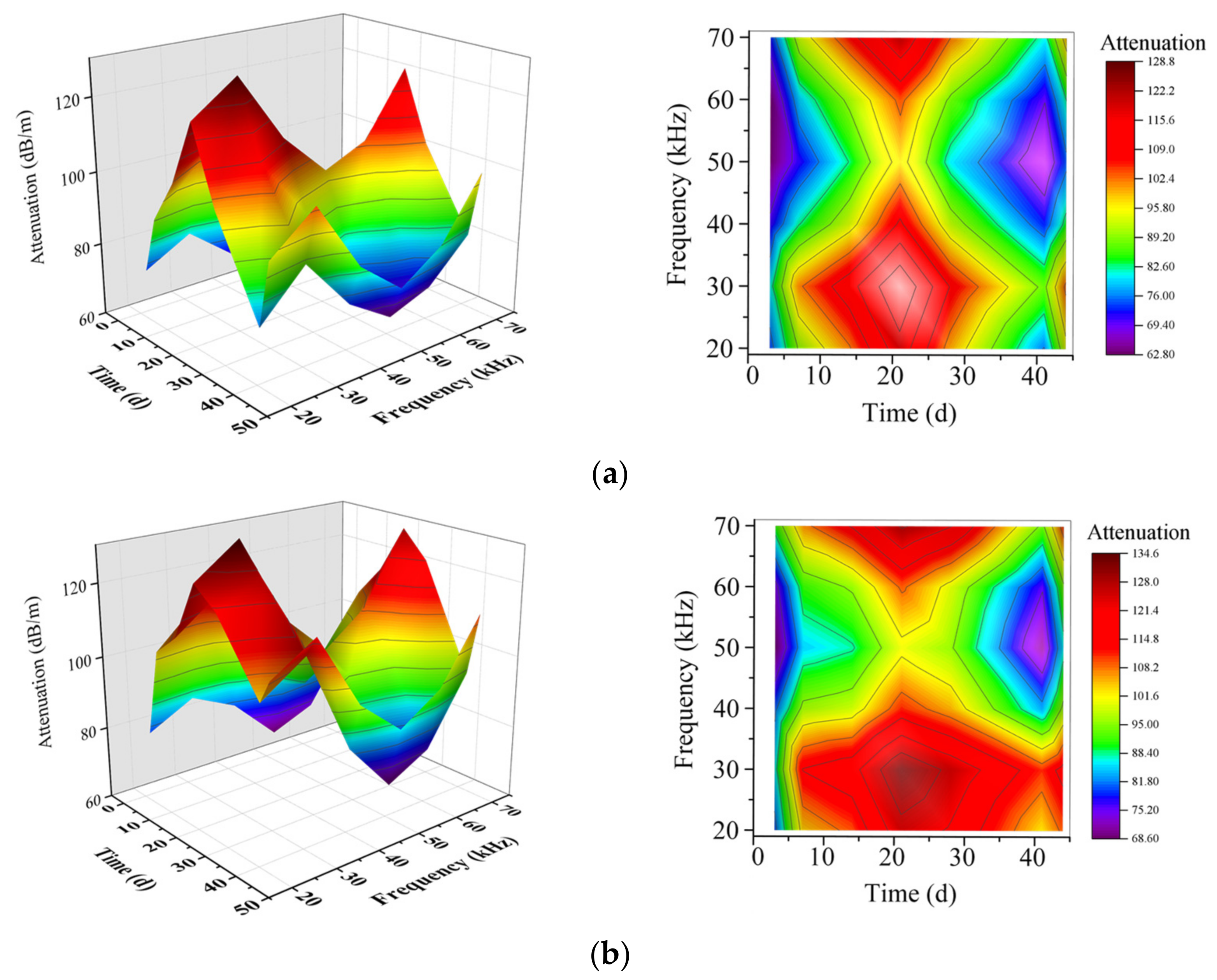
- Guided waves with frequencies of 20–70 kHz were used to test samples A and B, respectively. The general trend of wave velocity at each frequency decreases with curing time and will finally be stable. However, with the increase of the curing period, the attenuation of guided waves in CPB increases first and then decreases. The test results indicate that both the velocity and attenuation of guided wave change most obviously at the frequency of 30 kHz. Therefore, 30 kHz is the optimal guided wave frequency for cemented paste backfill monitoring.
- (3)
- Through the uniaxial compression test and guided wave test, the Mechanical and acoustic parameters of CPB were studied. The relationship between strength and wave velocity, strength and attenuation, and the corresponding exponential function fitting equations were found.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nasir, O.; Fall, M. Modeling the heat development in hydrating CPB structures. Comput. Geotech. 2009, 36, 1207–1218. [Google Scholar] [CrossRef]
- Benzaazoua, M.; Fall, M.; Belem, T. A contribution to understanding the hardening process of cemented pastefill. Miner. Eng. 2004, 17, 141–152. [Google Scholar] [CrossRef]
- Fall, M.; Benzaazoua, M.; Saa, E.G. Mix proportioning of underground cemented tailings backfill. Tunn. Undergr. Space Technol. 2008, 23, 80–90. [Google Scholar] [CrossRef]
- Yilmaz, E.; Kesimal, A.; Ercidi, B. Strength Development of Paste Backfill Simples at Long-Term Using Different Binders. In Proceedings of the 8th Symposium MineFill04, Beijing, China, 20–21 September 2004; pp. 281–285. [Google Scholar]
- Sivakugan, N.; Rankine, R.M.; Rankine, K.J.; Rankine, K.S. Geotechnical considerations in mine backfilling in Australia. J. Clean. Prod. 2006, 14, 1168–1175. [Google Scholar] [CrossRef]
- Fall, M.; Pokharel, M. Coupled effects of sulphate and temperature on the strength development of cemented tailings backfills: Portland cement-paste backfill. Cem. Concr. Compos. 2010, 32, 819–828. [Google Scholar] [CrossRef]
- Pagé, P.; Li, L.; Yang, P.; Simon, R. Numerical investigation of the stability of a base-exposed sill mat made of cemented backfill. Int. J. Rock Mech. Min. Sci. 2019, 114, 195–207. [Google Scholar] [CrossRef]
- Qi, C.C.; Fourie, A. Cemented paste backfill for mineral tailings management: Review and future perspectives. Miner. Eng. 2019, 144, 106025. [Google Scholar] [CrossRef]
- Fall, M.; Adrien, D.; Célestin, J.C.; Pokharel, M.; Touré, M. Saturated hydraulic conductivity of cemented paste backfill. Miner. Eng. 2009, 22, 1307–1317. [Google Scholar] [CrossRef]
- Jiang, H.; Yi, H.; Yilmaz, E.; Liu, S.; Qiu, J. Ultrasonic evaluation of strength properties of cemented paste backfill: Effects of mineral admixture and curing temperature. Ultrasonics 2020, 100, 105983. [Google Scholar] [CrossRef] [PubMed]
- He, C.F.; Zheng, M.F.; Lv, Y. Development applications and challenges in ultrasonic guided waves testing technology. Chin. J. Sci. Instrum. 2016, 37, 1713–1735. [Google Scholar] [CrossRef]
- Alleyne, D.N.; Lowe, M.J.S.; Cawley, P. The reflection of guided waves from circumferential notches in pipes. J. Appl. Mech. 1998, 65, 635–641. [Google Scholar] [CrossRef]
- Fan, Z.; Lowe, M.J.S. Elastic waves guided by a welded joint in a plate. Proc. R. Soc. A Math. Phys. Eng. Sci. 2009, 465, 2053–2068. [Google Scholar] [CrossRef]
- Pettit, J.R.; Walker, A.; Cawley, P.; Lowe, M.J.S. A stiffness reduction method for efficient absorption of waves at boundaries for use in commercial finite element codes. Ultrasonics 2014, 54, 1868–1879. [Google Scholar] [CrossRef] [PubMed]
- Leinov, E.; Lowe, M.J.S.; Cawley, P. Investigation of guided wave propagation and attenuation in pipe buried in sand. J. Sound Vib. 2015, 347, 96–114. [Google Scholar] [CrossRef] [Green Version]
- Leinov, E.; Lowe, M.J.S.; Cawley, P. Ultrasonic isolation of buried pipes. J. Sound Vib. 2016, 363, 225–239. [Google Scholar] [CrossRef] [Green Version]
- Huthwaite, P.; Ribichini, R.; Cawley, P.; Lowe, M.J. Mode selection for corrosion detection in pipes and vessels via guided wave tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. Control. 2013, 60, 1165–1177. [Google Scholar] [CrossRef] [PubMed]
- Simonetti, F.; Alqaradawi, M.Y. Guided ultrasonic wave tomography of a pipe bend exposed to environmental conditions: A long-term monitoring experiment. NDT E Int. 2019, 105, 1–10. [Google Scholar] [CrossRef]
- Liu, Z.H.; Liu, S.; Wu, B.; Zhang, Y.N.; He, C.F. Experimental research on acoustoelastic effect of ultrasonic guided waves in prestressing steel strand. Chin. J. Mech. Eng. 2010, 46, 22–28. (In Chinese) [Google Scholar] [CrossRef]
- Liu, Z.; Liu, S.; Wu, B.; Zhang, Y.; He, C. Propagation characteristics of high order longitudinal modes in steel strands and their applications. Acta Mech. Solida Sin. 2008, 21, 573–579. [Google Scholar] [CrossRef]
- Wang, W.B.; Feng, Z.J.; Ai, M.Y.; Wang, K. Image reconstruction of phased ultrasonic guided wave testing for storage tank floor defect. Nondestruct. Test. 2010, 32, 847–849. (In Chinese) [Google Scholar]
- Liu, Z.H.; Zhang, Y.N.; He, C.F.; Cao, C.; Wu, B. Development of magnetostrictive transmitter and receiver for exciting ultrasonic longitudinal guided waves in steel strands. Chin. J. Appl. Mech. 2009, 26, 507–512. (In Chinese) [Google Scholar]
- Liu, Z.H.; Yu, F.X.; Yu, H.T.; He, C.F.; Wu, B. Ultrasonic guided wave technology based on group velocity calibration and its application for defect detection in composite plates. J. Mech. Eng. 2012, 48, 8–15. (In Chinese) [Google Scholar] [CrossRef]
- Wu, B.; Li, Y.; Zheng, Y.; He, C.F. Thickness measurement of surface attachment on plate with SH wave. J. Mech. Eng. 2012, 48, 78–84. (In Chinese) [Google Scholar] [CrossRef]
- Liu, Z.H.; Xu, Y.Z.; He, C.F.; Wu, B. Experimental study on defect imaging based on single Lamb wave mode in plate-like structures. Eng. Mech. 2014, 31, 232–238. (In Chinese) [Google Scholar] [CrossRef]
- Liu, Z.H.; Wu, B.; He, C.F.; Wang, X.Y. Torsional mode of ultrasonic guided wave propagation characteristics in pipes with viscoelastic coatings. J. Basic Sci. Eng. 2005, 13, 291–299. (In Chinese) [Google Scholar] [CrossRef]
- Deng, F.; Wu, B.; He, C.F. A time-reversal defect-identifying method for guided wave inspection in pipes. J. Press. Vessel. Technol. 2008, 130, 021503. [Google Scholar] [CrossRef]
- Yu, J.G.; Wu, B.; Chen, G.Q. Wave characteristics in functionally graded piezoelectric hollow cylinders. Arch. Appl. Mech. 2009, 79, 807–824. [Google Scholar] [CrossRef]
- Liu, Z.H.; He, C.F.; Wu, B.; Wang, X.Y. Experimental research on longitudinal mode selection in pipes using angle beam probes. Eng. Mech. 2009, 26, 246–250. (In Chinese) [Google Scholar]
- Madenga, V.; Zou, D.H.; Zhang, C. Effects of curing time and frequency on ultrasonic wave velocity in grouted rock bolts. J. Appl. Geophys. 2006, 59, 79–87. [Google Scholar] [CrossRef]
- Cui, Y.; Zou, D.H. Numerical simulation of attenuation and group velocity of guided ultrasonic wave in grouted rock bolts. J. Appl. Geophys. 2006, 59, 337–344. [Google Scholar] [CrossRef]
- Zou, D.H.; Cui, Y.; Madenga, V. Effects of frequency and grouted length on the behavior of guided ultrasonic waves in rock bolts. Int. J. Rock Mech. Min. Sci. 2007, 44, 813–819. [Google Scholar] [CrossRef]
- Zou, D.H.; Cui, Y. A new approach for field instrumentation in grouted rock bolt monitoring using guided ultrasonic waves. J. Appl. Geophys. 2011, 75, 506–512. [Google Scholar] [CrossRef]
- Cui, Y.; Zou, D.H. Assessing the effects of insufficient rebar and missing grout in grouted rock bolts using guided ultrasonic waves. J. Appl. Geophys. 2012, 79, 64–70. [Google Scholar] [CrossRef]
- Wang, C.; He, W.; Ning, J.G.; Zhang, C.S. Propagation properties of guided wave in the anchorage structure of rock bolts. J. Appl. Geophys. 2009, 69, 131–139. [Google Scholar] [CrossRef]
- Zima, B.; Kedra, R. Reference-free determination of debonding length in reinforced concrete beams using guided wave propagation. Constr. Build. Mater. 2019, 207, 291–303. [Google Scholar] [CrossRef]
- Li, J.S.; Lu, Y.; Guan, R.Q.; Qu, W.Z. Guided waves for debonding identification in CFRP-reinforced concrete beams. Constr. Build. Mater. 2017, 131, 388–399. [Google Scholar] [CrossRef]
- Xu, W.B.; Pan, W.D.; Ding, M.L. Experiment on evolution of microstructures and long-term strength model of cemented backfill mass. J. Cent. South Univ. 2015, 46, 2333–2341. (In Chinese) [Google Scholar] [CrossRef]
- Torzaghi, K.; Peck, R.B. Soil Mechanics in Engineering Practice; John Wiley & Sons: New York, NY, USA, 1948; p. 22. [Google Scholar]
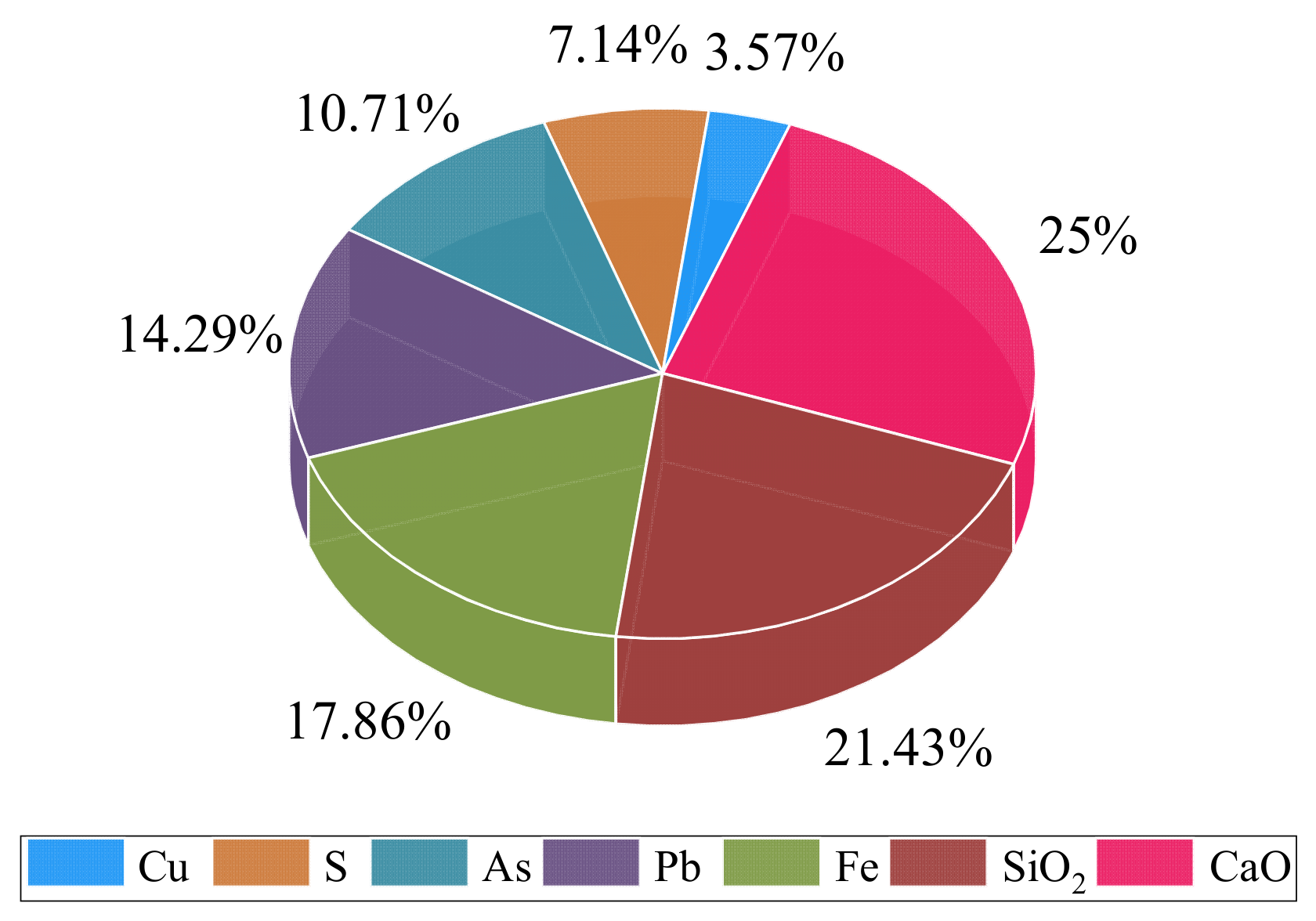
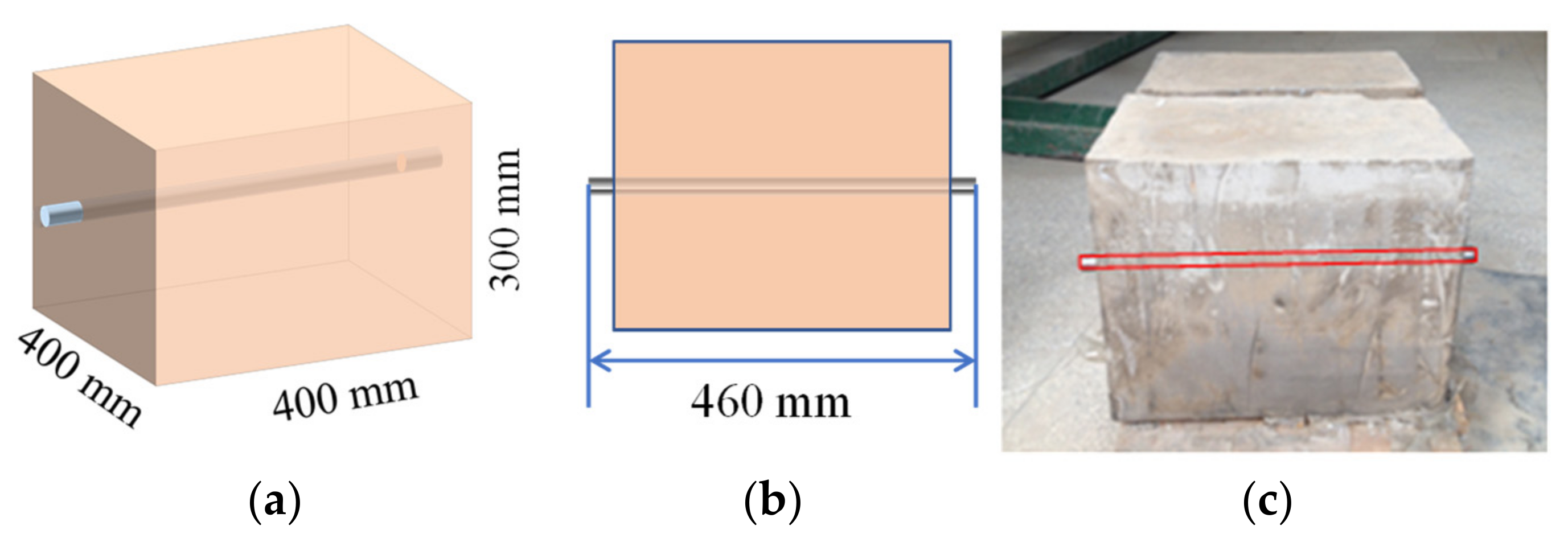

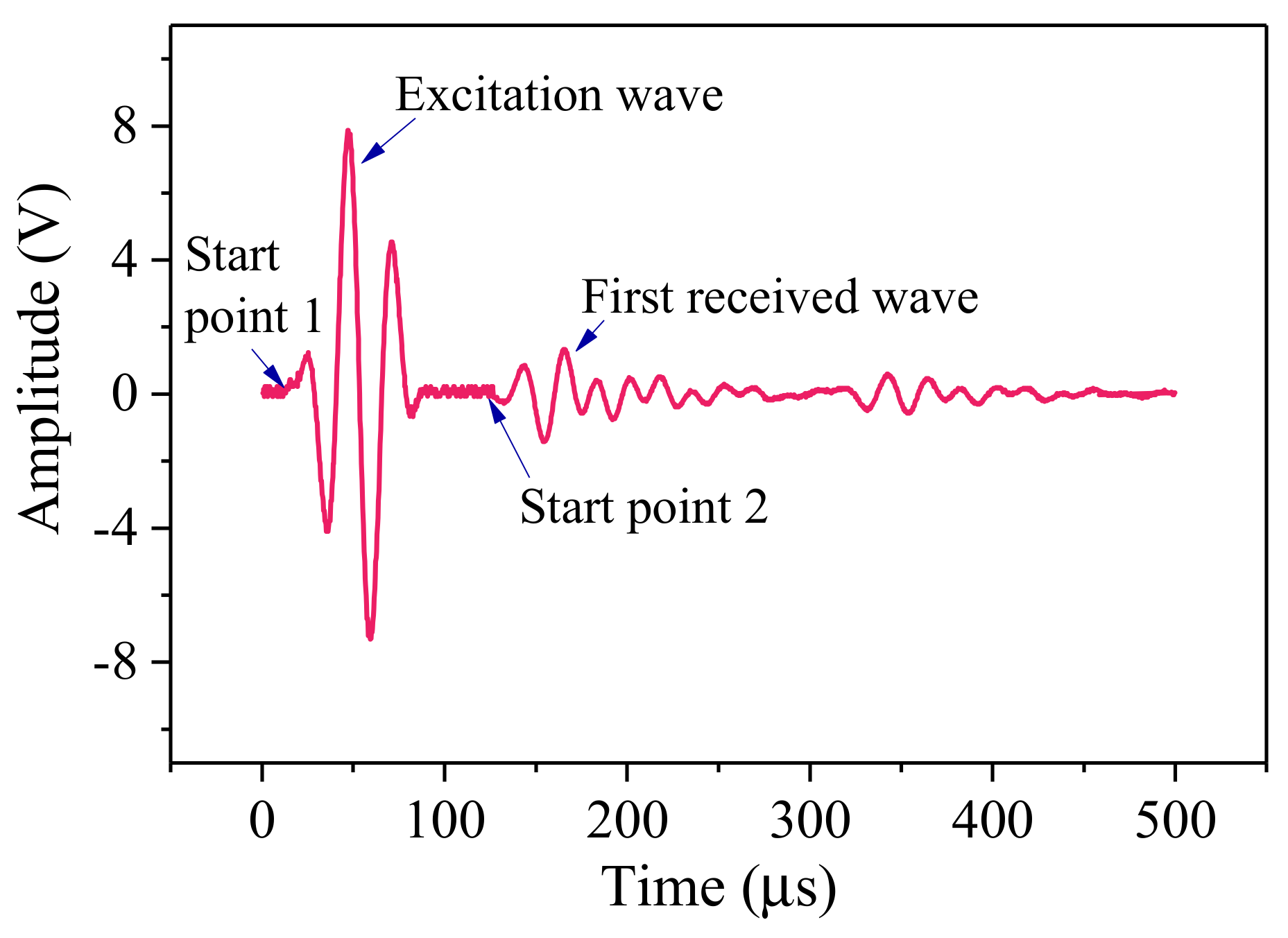
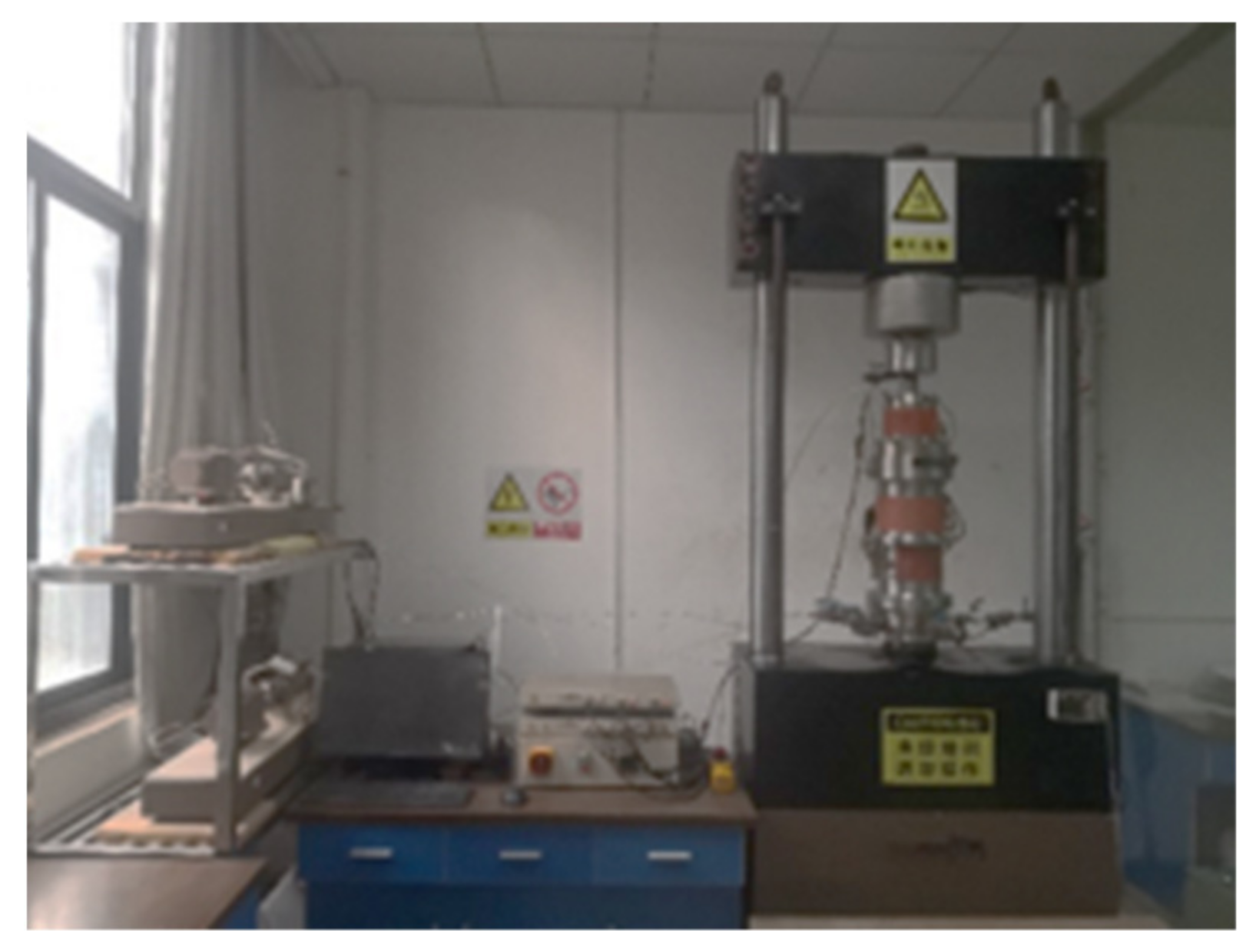

| Particle Size/um | 0~33 | 33~45 | 45~74 | >74 | |
| Content/% | 38.82 | 4.31 | 14.71 | 42.16 | 100 |
| UCS/MPa | ||||
|---|---|---|---|---|
| 3 d | 7 d | 14 d | 28 d | |
| Cement-tailings Ratio (1:4) | 1.25 | 1.73 | 2.12 | 3.42 |
| 1.23 | 2.01 | 2.15 | 3.33 | |
| 1.08 | 1.82 | 2.38 | 3.15 | |
| 1.32 | 1.64 | 2.34 | 3.65 | |
| Cement-tailings Ratio (1:8) | 0.69 | 1.14 | 1.67 | 2.12 |
| 0.73 | 1.12 | 1.47 | 2.08 | |
| 0.71 | 1.15 | 1.23 | 1.99 | |
| 0.75 | 1.08 | 1.51 | 2.29 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, W.; Zheng, C.; Li, S.; Shi, W.; Zhao, K. Strength Development Monitoring of Cemented Paste Backfill Using Guided Waves. Sensors 2021, 21, 8499. https://doi.org/10.3390/s21248499
He W, Zheng C, Li S, Shi W, Zhao K. Strength Development Monitoring of Cemented Paste Backfill Using Guided Waves. Sensors. 2021; 21(24):8499. https://doi.org/10.3390/s21248499
Chicago/Turabian StyleHe, Wen, Changsong Zheng, Shenhai Li, Wenfang Shi, and Kui Zhao. 2021. "Strength Development Monitoring of Cemented Paste Backfill Using Guided Waves" Sensors 21, no. 24: 8499. https://doi.org/10.3390/s21248499
APA StyleHe, W., Zheng, C., Li, S., Shi, W., & Zhao, K. (2021). Strength Development Monitoring of Cemented Paste Backfill Using Guided Waves. Sensors, 21(24), 8499. https://doi.org/10.3390/s21248499






