Fluid-Solid Interaction Simulation Methodology for Coriolis Flowmeter Operation Analysis
Abstract
1. Introduction
2. Methods
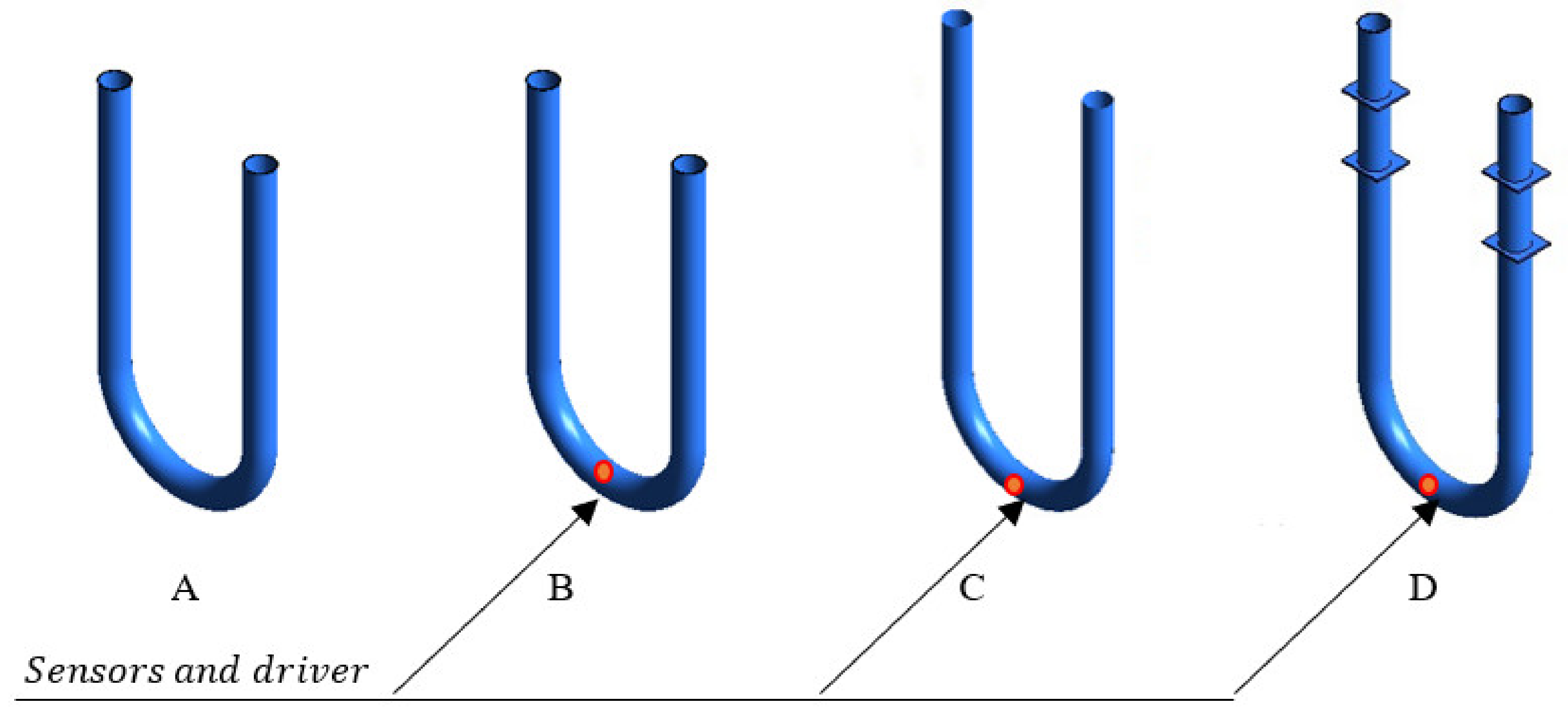
2.1. Flow Meter Configuration
2.2. Numerical Analysis Methodology
2.3. Computational Mesh and Boundary Conditions
3. Results
3.1. Numerical Verification
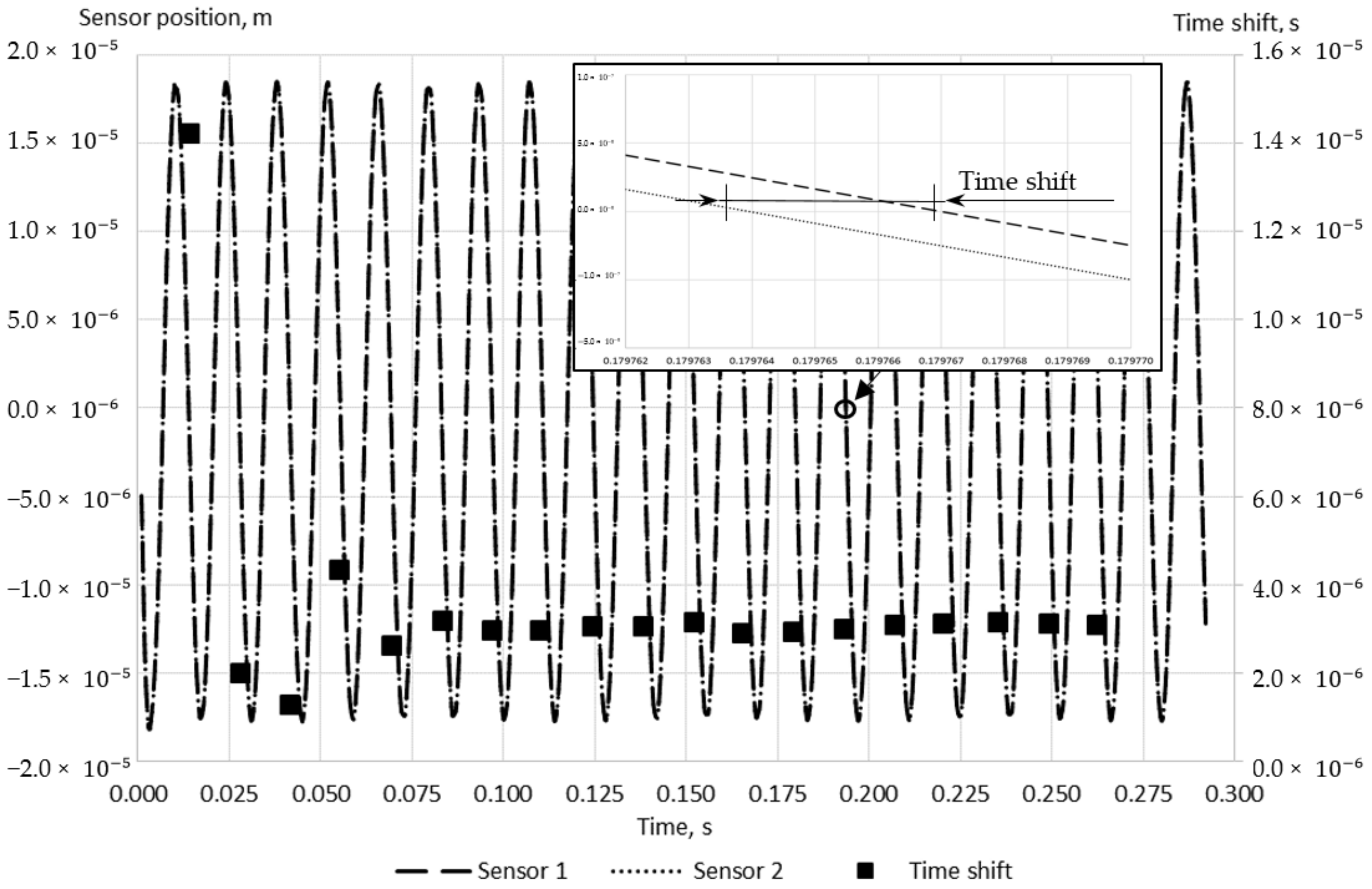
3.2. The Investigation of the Linearity between Flow Speed and Time Shift
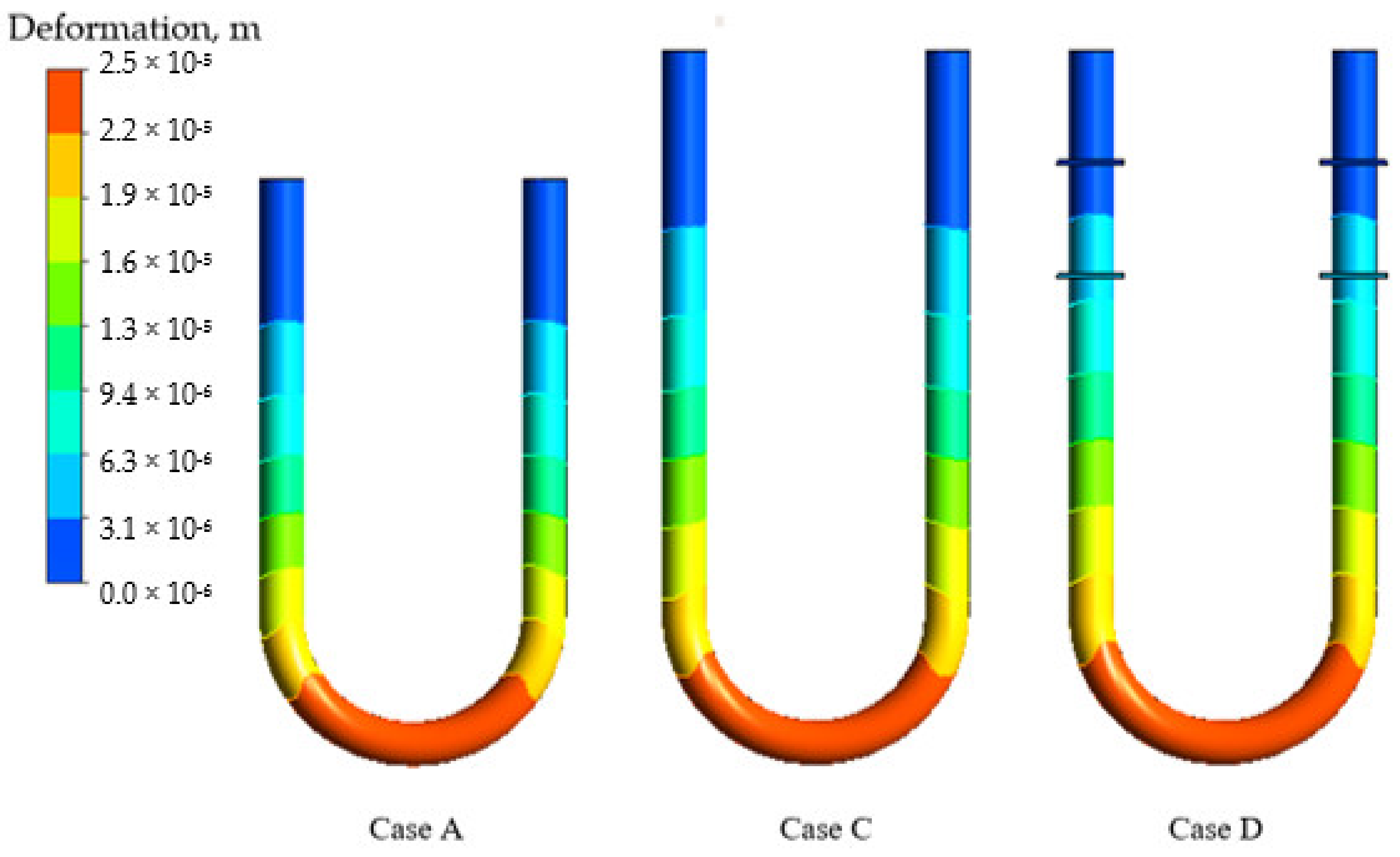
3.3. The Investigation of Acceptable CFM Configuration Simplification
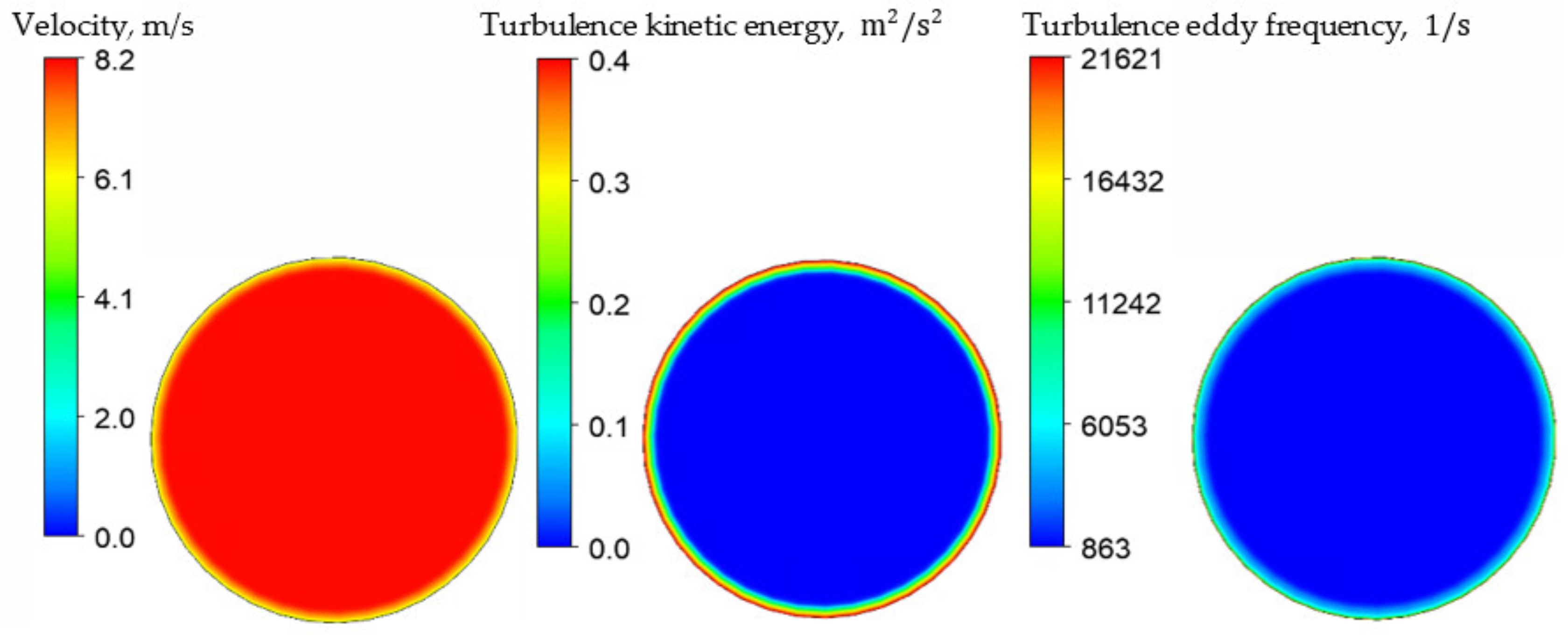
3.4. The Investigation of Turbulence Models Effects
3.5. The Error Analysis
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Zamora, M.; Henry, M.P. An FPGA Implementation of a Digital Coriolis Mass Flow Metering Drive System. IEEE Trans. Ind. Electron. 2008, 55, 2820–2831. [Google Scholar] [CrossRef]
- Patten, T.; Pawlas, G. The Effect of Swirl on Coriolis Mass Flowmeter. In Proceedings of the North Sea Flow Measurement Workshop, Lillehammer, Norway, 24–26 October 1995. [Google Scholar]
- Cheesewright, R.; Clark, C.; Bisset, D. Understanding the Experimental Response of Coriolis Massflow Meters to Flow Pulsations. Flow Meas. Instrum. 1999, 10, 207–215. [Google Scholar] [CrossRef]
- Kutin, J.; Bobovnik, G.; Hemp, J.; Bajsić, I. Velocity Profile Effects in Coriolis Mass Flowmeters: Recent Findings and Open Questions. Flow Meas. Instrum. 2006, 17, 349–358. [Google Scholar] [CrossRef]
- Weinstein, J.A.; Kassoy, D.R.; Bell, M.J. Experimental Study of Oscillatory Motion of Particles and Bubbles with Applications to Coriolis Flow Meters. Phys. Fluids 2008, 20, 103306. [Google Scholar] [CrossRef]
- Henry, M.P.; Clarke, D.W.; Archer, N.; Bowles, J.; Leahy, M.J.; Liu, R.P.; Vignos, J.; Zhou, F.B. A Self-Validating Digital Coriolis Mass-Flow Meter: An Overview. Control Eng. Pract. 2000, 8, 487–506. [Google Scholar] [CrossRef]
- Sultan, G.; Hemp, J. Modelling of the Coriolis Mass Flowmeter. J. Sound Vib. 1989, 132, 473–489. [Google Scholar] [CrossRef]
- Love, A.E.H. A Treatise on the Mathematical Theory of Elasticity, 4th ed.; Dover Publications: New York, NY, USA, 1944. [Google Scholar]
- Binulal, B.R.; Rajan, A.; Kochupillai, J. Dynamic Analysis of Coriolis Flow Meter Using Timoshenko Beam Element. Flow Meas. Instrum. 2016, 47, 100–109. [Google Scholar] [CrossRef]
- Belhadj, A.; Cheesewright, R.; Clark, C. The Simulation of Coriolis Meter Response to Pulsating Flow Using a General Purpose, F.E. Code. J. Fluids Struct. 2000, 14, 613–634. [Google Scholar] [CrossRef]
- Bobovnik, G.; Mole, N.; Kutin, J.; Štok, B.; Bajsić, I. Coupled Finite-Volume/Finite-Element Modelling of the Straight-Tube Coriolis Flowmeter. J. Fluids Struct. 2005, 20, 785–800. [Google Scholar] [CrossRef]
- Enz, S. Factors Affecting Coriolis Flowmeter Accuracy, Precision and Robustness. Ph.D. Thesis, Technical University of Denmark, Kongens Lyngby, Denmark, 2010. [Google Scholar]
- Hu, Y.-C.; Chen, Z.-Y.; Chang, P.-Z. Fluid-Structure Coupling Effects in a Dual U-Tube Coriolis Mass Flow Meter. Sensors 2021, 21, 982. [Google Scholar] [CrossRef]
- Kumar, V.; Anklin, M. Numerical Simulations of Coriolis Flow Meters for Low Reynolds Number Flows. MAPAN 2011, 26, 225–235. [Google Scholar] [CrossRef]
- Athanase, D.G. CFD Simulation and Measurement on Gas-Liquid Two Phase Flow. Ph.D. Thesis, National University of Singapore, Mechanical Department, Singapore, 2018. [Google Scholar]
- Romanov, V.A.; Beskachko, V.P. Virtual Prototyping Experience of the Coriolis Flow Meter; Springer: Cham, Switzerland; Moscow, Russia, 2018; pp. 411–420. [Google Scholar]
- Enz, S.; Thomsen, J.J. Predicting Phase Shift Effects for Vibrating Fluid-Conveying Pipes Due to Coriolis Forces and Fluid Pulsation. J. Sound Vib. 2011, 330, 5096–5113. [Google Scholar] [CrossRef]
- Bobovnik, G.; Kutin, J.; Mole, N.; Štok, B.; Bajsić, I. Numerical Analysis of Installation Effects in Coriolis Flowmeters: Single and Twin Tube Configurations. Flow Meas. Instrum. 2015, 44, 71–78. [Google Scholar] [CrossRef]
- Hua, C.; Sun, S.; Yao, H.; Zhu, H.; Xing, L. Wellhead Metering Based on Bubble and Resonance Coriolis Effect with Known Gas-Liquid Ratio. Meas. J. Int. Meas. Confed. 2020, 155, 107563. [Google Scholar] [CrossRef]
- Bobovnik, G.; Kutin, J.; Bajsić, I. Estimation of Velocity Profile Effects in the Shell-Type Coriolis Flowmeter Using CFD Simulations. Flow Meas. Instrum. 2005, 16, 365–373. [Google Scholar] [CrossRef]
- Kolhe, V.A.; Edlabadkar, R.L. Performance Evaluation of Coriolis Mass Flow Meter in Laminar Flow Regime. Flow Meas. Instrum. 2021, 77, 101837. [Google Scholar] [CrossRef]
- Yaushev, A.A.; Taranenko, P.A.; Loginovskiy, V.A. Study of the Oscillation Modes of a Coriolis Flowmeter Using a Parametric Finite Element Model, Verified by the Results of Modal Testing. Procedia Eng. 2016, 150, 336–340. [Google Scholar] [CrossRef]
- Taluja, R.; Pant, R.; Patil, P. Design and Analysis of Twin S Shape Coriolis Mass Flow Sensor; IEEE: Piscataway, NJ, USA, 2019; pp. 1–4. [Google Scholar]
- Sultan, G. Theoretical and Experimental Studies of the Coriolis Mass Flowmeter. Ph.D. Thesis, Cranfield Institute of Technology, Bedford, UK, 1990. [Google Scholar]
- Wang, S.; Clark, C.; Cheesewright, R. Virtual Coriolis Flow Meter: A Tool for Simulation and Design. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2006, 220, 817–835. [Google Scholar] [CrossRef]
- Sharma, S.; Patil, P.; Vasudev, A.; Jain, S. Performance Evaluation of an Indigenously Designed Copper (U) Tube Coriolis Mass Flow Sensors. Measurement 2010, 43, 1165–1172. [Google Scholar] [CrossRef]
- Haussmann, M. Lattice Boltzmann Methods for Turbulent Flows: Application to Coriolis Mass Flowmeter. Ph.D. Thesis, Karlsruher Institute of Technology, Karlsruhe, Germany, 2020. [Google Scholar]
- Coclite, A.; Ranaldo, S.; Pascazio, G.; de Tullio, M.D. A Lattice Boltzmann Dynamic-Immersed Boundary Scheme for the Transport of Deformable Inertial Capsules in Low-Re Flows. Comput. Math. Appl. 2020, 80, 2860–2876. [Google Scholar] [CrossRef]
- He, T. Partitioned Coupling Strategies for Fluid-Structure Interaction with Large Displacement: Explicit, Implicit and Semi-Implicit Schemes. Wind Struct. 2015, 20, 423–448. [Google Scholar] [CrossRef]
- Wong, K.K.L.; Thavornpattanapong, P.; Cheung, S.C.P.; Tu, J. Numerical Stability of Partitioned Approach in Fluid-Structure Interaction for a Deformable Thin-Walled Vessel. Comput. Math. Methods Med. 2013, 2013, 638519. [Google Scholar] [CrossRef]
- Kassiotis, C.; Ibrahimbegovic, A.; Niekamp, R.; Matthies, H.G. Nonlinear Fluid–Structure Interaction Problem. Part I: Implicit Partitioned Algorithm, Nonlinear Stability Proof and Validation Examples. Comput. Mech. 2010, 47, 305–323. [Google Scholar] [CrossRef]
- Heil, M. An Efficient Solver for the Fully Coupled Solution of Large-Displacement Fluid–Structure Interaction Problems. Comput. Methods Appl. Mech. Eng. 2004, 193, 1–23. [Google Scholar] [CrossRef]
- ANSYS, Inc. Ansys Academic Research Mechanical. Release 19.0, Theory Reference. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/prod_page.html?pn=Mechanical%20Application&pid=MechanicalApplication&lang=en&prodver=21.2/ (accessed on 19 September 2021).
- Gravemeier, V.; Wall, W.A.; Ramm, E. Numerical Solution of the Incompressible Navier-Stokes Equations by a Three-Level Finite Element Method. In Computational Fluid and Solid Mechanics 2003; Bathe, K.J., Ed.; Elsevier: Amsterdam, The Netherlands, 2003; pp. 915–918. [Google Scholar] [CrossRef]
- Gysling, D.L. An Aeroelastic Model of Coriolis Mass and Density Meters Operating on Aerated Mixtures. Flow Meas. Instrum. 2007, 18, 69–77. [Google Scholar] [CrossRef]
- Ruoff, J.; Hodapp, M.; Kück, H. Finite Element Modelling of Coriolis Mass Flowmeters with Arbitrary Pipe Geometry and Unsteady Flow Conditions. Flow Meas. Instrum. 2014, 37, 119–126. [Google Scholar] [CrossRef]
- Vijiapurapu, S.; Cui, J. Performance of Turbulence Models for Flows through Rough Pipes. Appl. Math. Model. 2010, 34, 1458–1466. [Google Scholar] [CrossRef]
- Schmitt, F.G. About Boussinesq’s Turbulent Viscosity Hypothesis: Historical Remarks and a Direct Evaluation of Its Validity. Comptes Rendus Mec. 2007, 335, 617–627. [Google Scholar] [CrossRef]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Cheah, S.C.; Iacovides, H.; Jackson, D.C.; Ji, H.; Launder, B.E. LDA Investigation of the Flow Development through Rotating U-Ducts. J. Turbomach. 1996, 118, 590–596. [Google Scholar] [CrossRef]
- Bhaskar, K.U.; Murthy, Y.R.; Raju, M.R.; Tiwari, S.; Srivastava, J.K.; Ramakrishnan, N. CFD Simulation and Experimental Validation Studies on Hydrocyclone. Miner. Eng. 2007, 20, 60–71. [Google Scholar] [CrossRef]
- Luo, J.; Razinsky, E.H. Analysis of Turbulent Flow in 180 Deg Turning Ducts with and without Guide Vanes. J. Turbomach. 2009, 131, 21011. [Google Scholar] [CrossRef]
- Zhang, P.; Roberts, R.M.; Bénard, A. Computational Guidelines and an Empirical Model for Particle Deposition in Curved Pipes Using an Eulerian-Lagrangian Approach. J. Aerosol Sci. 2012, 53, 1–20. [Google Scholar] [CrossRef]
- Maduta, R.; Jakirlic, S. An Eddy-Resolving Reynolds Stress Transport Model for Unsteady Flow Computations. In Notes on Numerical Fluid Mechanics and Multidisciplinary Design; Springer: Berlin/Heidelberg, Germany, 2012; Volume 117, pp. 77–89. [Google Scholar] [CrossRef]
- Gerolymos, G.A.; Vallet, I. Reynolds-Stress Model Prediction of 3-D Duct Flows. Flow Turbul. Combust. 2016, 96, 45–93. [Google Scholar] [CrossRef][Green Version]
- Smirnov, P.E.; Menter, F.R. Sensitization of the SST Turbulence Model to Rotation and Curvature by Applying the Spalart–Shur Correction Term. J. Turbomach. 2009, 131, 41010. [Google Scholar] [CrossRef]
- ANSYS, Inc. Ansys CFX, 2019R1, CFX-Solver Theory Guide. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/corp/v212/en/cfx_thry/cfx_thry.html (accessed on 19 November 2021).
- Sureshkumar, R. Numerical Solution of Ordinary Differential Equations. Available online: https://ocw.mit.edu/courses/aeronautics-and-astronautics/16-90-computational-methods-in-aerospace-engineering-spring-2014/numerical-integration-of-ordinary-differential-equations/ (accessed on 19 November 2021).
- Roache, P.J. Perspective: A Method for Uniform Reporting of Grid Refinement Studies. J. Fluids Eng. 1994, 116, 405–413. [Google Scholar] [CrossRef]
- Hemp, J. Calculation of the Sensitivity of a Straight Tube Coriolis Mass Flowmeter with Free Ends. Flow Meas. Instrum. 2002, 12, 411–420. [Google Scholar] [CrossRef]
- Wang, T.; Baker, R. Coriolis Flowmeters: A Review of Developments over the Past 20 Years, and an Assessment of the State of the Art and Likely Future Directions. Flow Meas. Instrum. 2014, 40, 99–123. [Google Scholar] [CrossRef]
- Luo, J.; Lakshminarayana, B. Prediction of Strongly Curved Turbulent Duct Flows with Reynolds Stress Model. AIAA J. 1997, 35, 91–98. [Google Scholar] [CrossRef]
- Chang, P. Separation of Flow; Pergamon: Oxford, UK, 1970. [Google Scholar]
- AZoM. Stainless Steel—Grade 316 (UNS S31600). Available online: https://www.azom.com/properties.aspx?ArticleID=863 (accessed on 19 November 2021).
- ASTM. Standard Specification for Chromium and Chromium-Nickel Stainless Steel Plate, Sheet, and Strip for Pressure Vessels and for General Applications; ASTM A240/A240M-20a; ASTM International: West Conshohocken, PA, USA, 2020. [Google Scholar]
- Huang, L. An Improved Phase Difference Detection Method for a Coriolis Flowmeter. Measurement 2021, 172, 108862. [Google Scholar] [CrossRef]



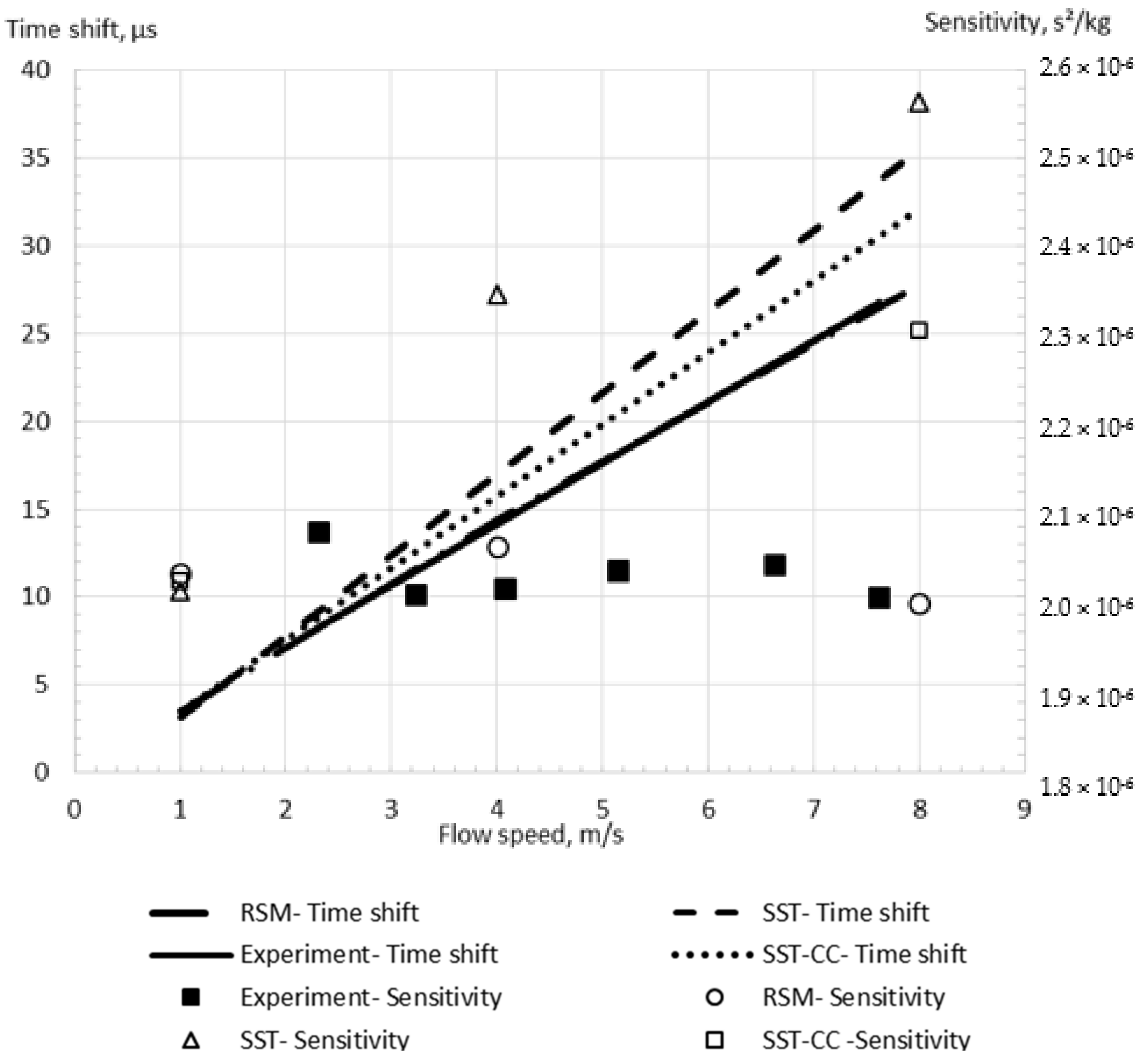
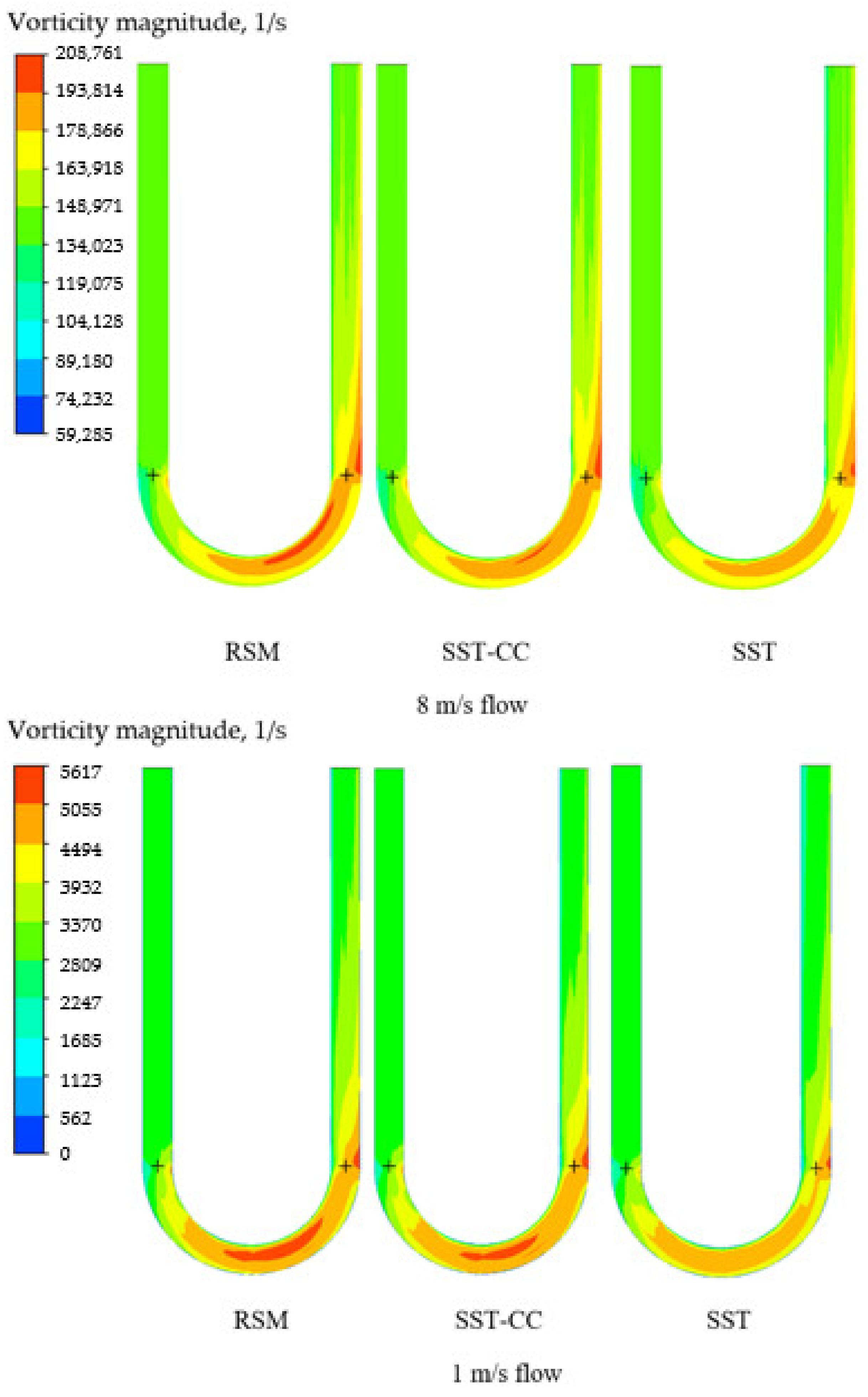
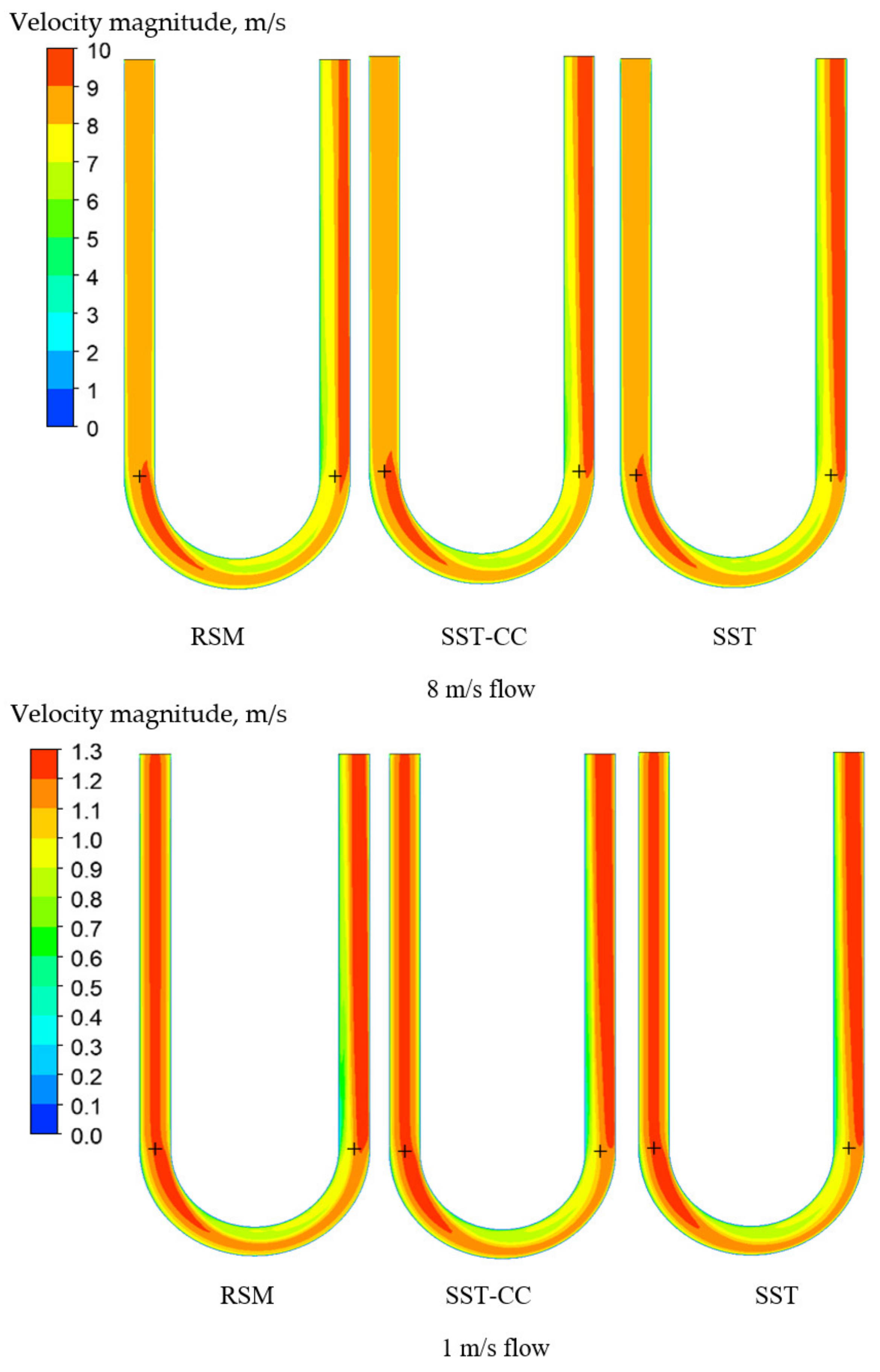

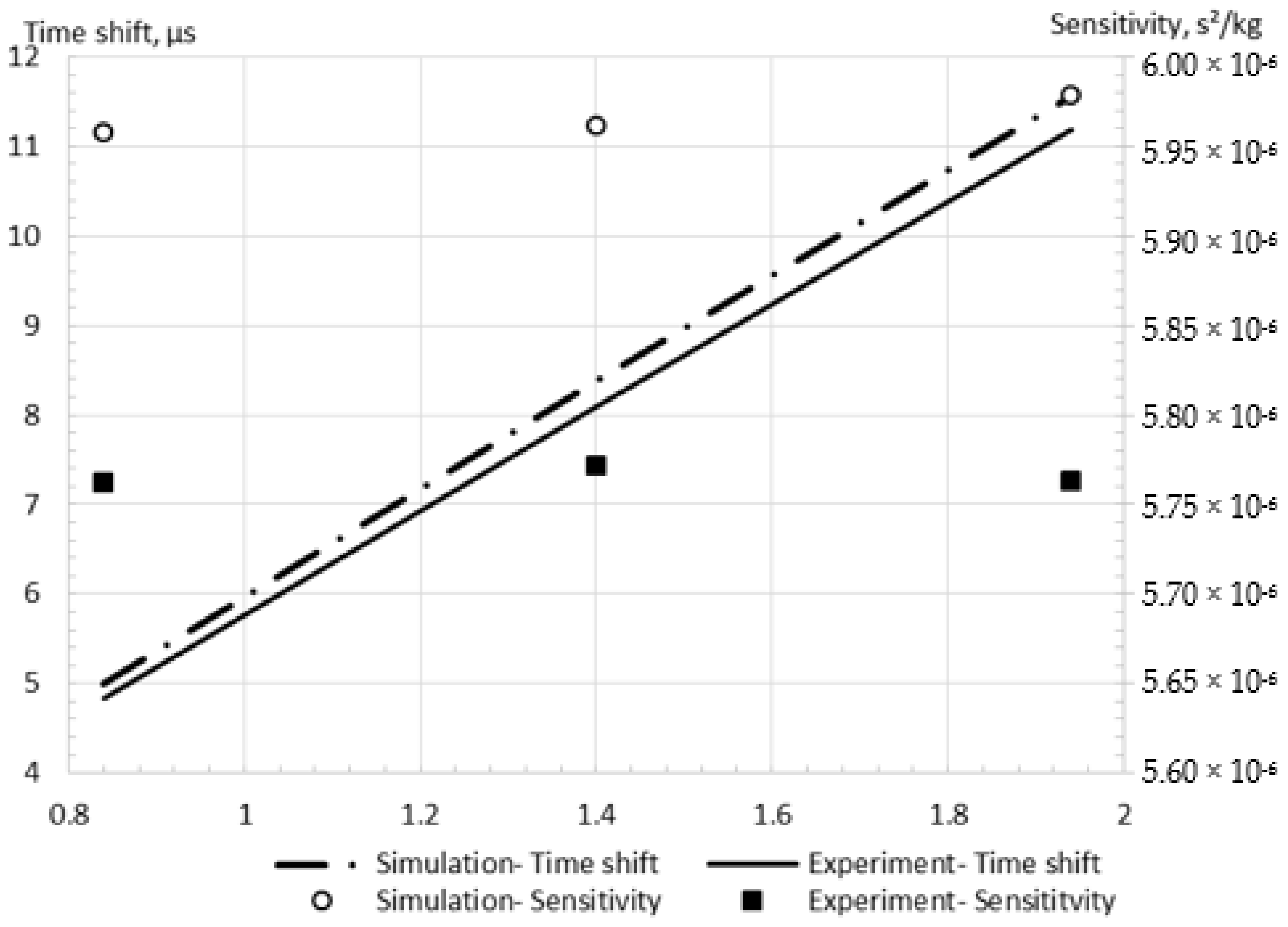
| Flowmeter Part | Property | Value |
|---|---|---|
| Tube | Shear modulus (GN/m2) | 80 |
| Density (kg/m3) | 8027 | |
| Young’s modulus (kg/m2) | 208 | |
| Fluid | Density (kg/m3) | 1000 |
| Number of Elements | Time Shift, ∆t, μs | Relative Difference, % | Grid Convergence Index, % |
|---|---|---|---|
| 168,000 | 37.7 | ||
| 352,000 | 34.7 | 8 | 9 |
| 712,000 | 35.3 | 2 | 4 |
| Time Step (s) | Time Shift, ∆t (μs) | Relative Difference of Time Shift (%) | |
|---|---|---|---|
| 2 × 10−3 | 38.8 | 11.80 | 2.78 × 10−6 |
| 1 × 10−3 | 34.7 | 2.96 | 2.49 × 10−6 |
| 5 × 10−4 | 33.7 | 2.42 × 10−6 |
| Sources | Error (%) | ||
|---|---|---|---|
| Simulations | Variation of tube material properties | Young’s modulus | ±3.80 |
| Density | ±1.25 | ||
| Shear modulus | ±5.13 | ||
| Numerical error | Linearity fitting | ±0.10 | |
| Discretization | ±2.00 | ||
| Time measurement | ±0.01 | ||
| Experiments | Reference meter | ±0.26 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shavrina, E.; Nguyen, V.-T.; Yan, Z.; Khoo, B.C. Fluid-Solid Interaction Simulation Methodology for Coriolis Flowmeter Operation Analysis. Sensors 2021, 21, 8105. https://doi.org/10.3390/s21238105
Shavrina E, Nguyen V-T, Yan Z, Khoo BC. Fluid-Solid Interaction Simulation Methodology for Coriolis Flowmeter Operation Analysis. Sensors. 2021; 21(23):8105. https://doi.org/10.3390/s21238105
Chicago/Turabian StyleShavrina, Evgeniia, Vinh-Tan Nguyen, Zeng Yan, and Boo Cheong Khoo. 2021. "Fluid-Solid Interaction Simulation Methodology for Coriolis Flowmeter Operation Analysis" Sensors 21, no. 23: 8105. https://doi.org/10.3390/s21238105
APA StyleShavrina, E., Nguyen, V.-T., Yan, Z., & Khoo, B. C. (2021). Fluid-Solid Interaction Simulation Methodology for Coriolis Flowmeter Operation Analysis. Sensors, 21(23), 8105. https://doi.org/10.3390/s21238105






