Model-Independent Lens Distortion Correction Based on Sub-Pixel Phase Encoding
Abstract
:1. Introduction
2. Methods
2.1. Basic Model of Lens Distortion
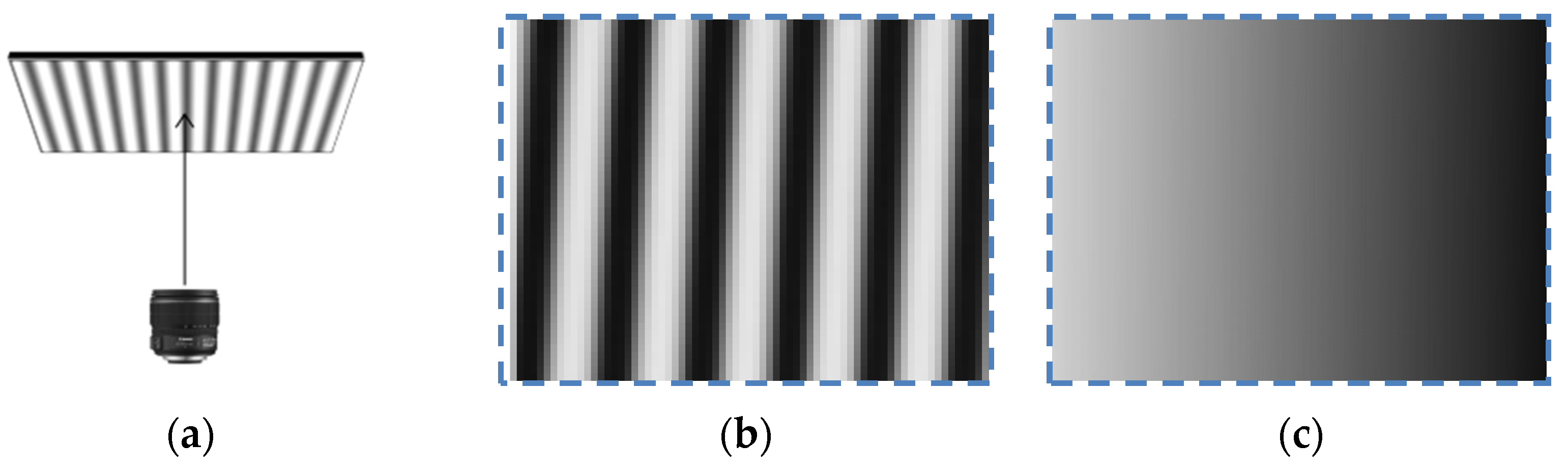
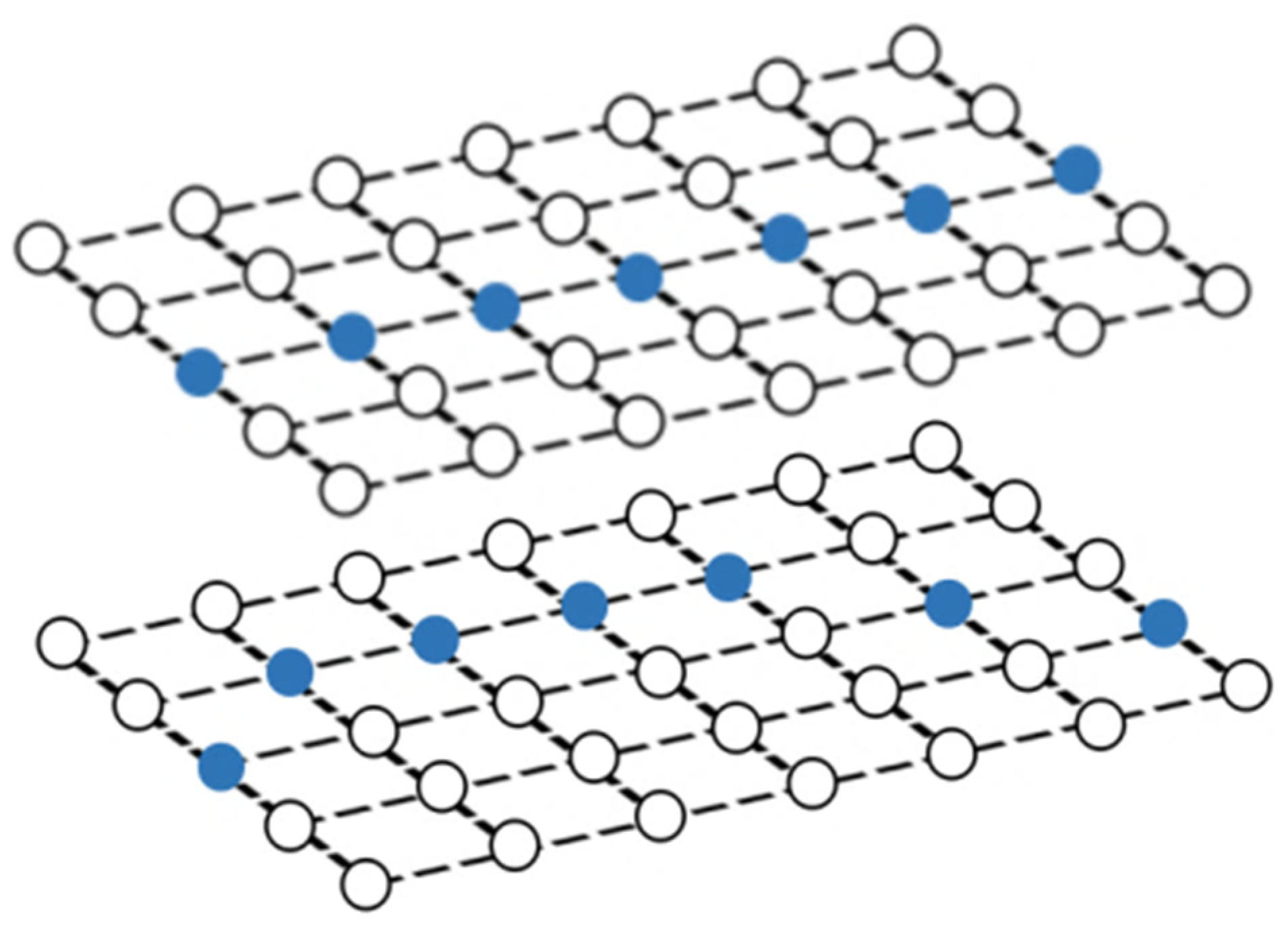
2.2. Phase Encoding and Isophase Detection
2.3. Obtaining Parameters and Correction
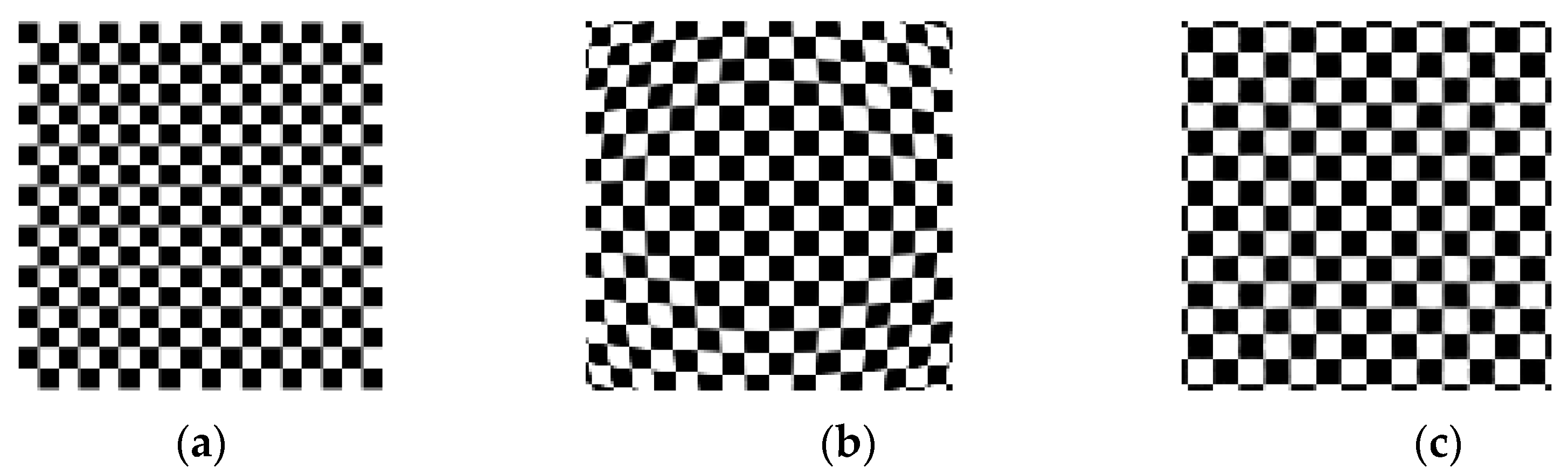
2.4. Quantitative Evaluation
2.5. Numerical Simulation
3. Experimental Results
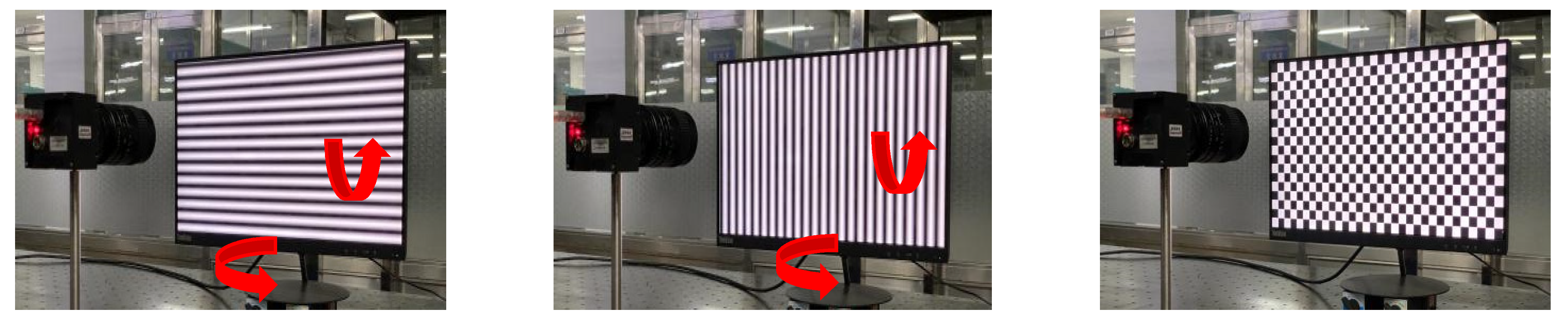
3.1. Experiments
3.2. Comparative Tests
3.3. The Impact of Parallelism and Distance on Accuracy
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Collins, T.; Bartoli, A. Planar Structure-from-Motion with Affine Camera Models: Closed-Form Solutions, Ambiguities and Degeneracy Analysis. IEEE Trans. Pattern Anal. Mach. Intell. 2016, 39, 1237–1255. [Google Scholar] [CrossRef] [PubMed]
- Guan, H.; Smith, W.A.P. Structure-From-Motion in Spherical Video Using the von Mises-Fisher Distribution. IEEE Trans. Image Process. 2017, 26, 711–723. [Google Scholar] [CrossRef] [PubMed]
- Lu, Z.; Cai, L. Camera calibration method with focus-related intrinsic parameters based on the thin-lens model. Opt. Express 2020, 28, 20858–20878. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Q.; Liu, J. Rapid nonlinear distortion correction of aerial optical zoom lens system. Acta Opt. Sin. 2015, 35, 144–154. [Google Scholar]
- Ahmed, M.; Farag, A. Non-metric calibration of camera lens distortion: Differential methods and robust estimation. IEEE Trans. Image Process 2005, 14, 1215–1230. [Google Scholar] [CrossRef] [PubMed]
- Cai, B.; Wang, Y.; Wu, J.; Wang, M.; Li, F.; Ma, M.; Chen, X.; Wang, K. An effective method for camera calibration in defocus scene with circular gratings. Opt. Lasers Eng. 2019, 114, 44–49. [Google Scholar] [CrossRef]
- Hao, S.; Han, X.; Zhang, Y.; Xu, L. Low-light enhancement based on an improved simplified Retinex model via fast illumination map refinement. Pattern Anal. Appl. 2020, 24, 1–12. [Google Scholar] [CrossRef]
- Weng, J.; Zhou, W.; Ma, S.; Qi, P.; Zhong, J. Model-Free Lens Distortion Correction Based on Phase Analysis of Fringe-Patterns. Sensors 2020, 21, 209. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z. A flexible new technique for camera calibration. Pattern Anal. Mach. Intell. IEEE Trans. 2000, 22, 1330–1334. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z. Camera calibration with one-dimensional objects. IEEE T Pattern Anal. 2004, 26, 892–899. [Google Scholar] [CrossRef] [PubMed]
- Rong, J.; Huang, S.; Shang, Z.; Ying, X. Radial Lens Distortion Correction Using Convolutional Neural Networks Trained with Synthesized Images. In Proceedings of the Asian Conference on Computer Vision, Taipei, Taiwan, 20–24 November 2016; Springer: Cham, Switzerland, 2016; pp. 35–49. [Google Scholar]
- Yin, X.; Wang, X.; Yu, J.; Zhang, M.; Fua, P.; Tao, D. FishEyeRecNet: A Multi-context Collaborative Deep Network for Fisheye Image Rectification. In Proceedings of the European Conference on Computer Vision, Munich, Germany, 8–14 September 2018; pp. 475–490. [Google Scholar]
- Xue, Z.; Xue, N.; Xia, G.-S.; Shen, W. Learning to Calibrate Straight Lines for Fisheye Image Rectification. In Proceedings of the 2019 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR), Long Beach, CA, USA, 15–20 June 2019; pp. 1643–1651. [Google Scholar]
- Liao, K.; Lin, C.; Zhao, Y.; Xu, M. Model-Free Distortion Rectification Framework Bridged by Distortion Distribution Map. IEEE Trans. Image Process. 2020, 29, 3707–3718. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Zhang, B.; Sander, P.V.; Liao, J. Blind Geometric Distortion Correction on Images Through Deep Learning. In Proceedings of the 2019 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR), Long Beach, CA, USA, 15–20 June 2019; pp. 4850–4859. [Google Scholar]
- Thirthala, S.; Pollefeys, M. Radial Multifocal Tensors. Int. J. Comput. Vis. 2012, 96, 195–211. [Google Scholar] [CrossRef]
- Xu, Y.; Gao, F.; Ren, H.; Zhang, Z.; Jiang, X. An Iterative Distortion Compensation Algorithm for Camera Calibration Based on Phase Target. Sensors 2017, 17, 1188. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Takeda, M.; Mutoh, K. Fourier transform profilometry for the automatic measurement of 3-D object shapes. Appl. Opt. 1983, 22, 3977–3982. [Google Scholar] [CrossRef]
- Weng, J.; Zhong, J.; Hu, C.; Zhong, J. Digital reconstruction based on angular spectrum diffraction with the ridge of wavelet transform in holographic phase-contrast microscopy. Opt. Express 2008, 16, 21971–21981. [Google Scholar] [CrossRef]
- Liu, D.; Liu, X.; Wang, M. Camera Self-Calibration with Lens Distortion from a Single Image. Photogramm. Eng. Remote Sens. 2016, 82, 325–334. [Google Scholar] [CrossRef]
- Yoneyama, S.; Kitagawa, A.; Kitamura, K.; Kikuta, H. In-Plane Displacement Measurement Using Digital Image Correlation with Lens Distortion Correction. JSME Int. J. Ser. A 2006, 49, 458–467. [Google Scholar] [CrossRef] [Green Version]
- Yoneyama, S.; Kikuta, H.; Kitagawa, A.; Kitamura, K. Lens distortion correction for digital image correlation by measuring rigid body displacement. Opt. Eng. 2006, 45, 023602. [Google Scholar] [CrossRef]











| Method | Results (Partial Enlargement) | RMS | Time Consuming | Description |
|---|---|---|---|---|
| Zhang |  | 1.28 pixels | 1.1 s | The accuracy is related to the quality and position of the input calibration images. |
| Tsai |  | 1.83 pixels | 2.9 s | It is not possible to calibrate all external parameters through a plane, and non-linear calculations may make the results unstable. Only consider radial distortion. |
| Thirthala |  | 3.81 pixels | 1.9 s | The position of the distortion center affects the correction result. |
| The proposed method |  | 0.39 pixels | 2.2 s | The position of the calibration targets is fixed. Simple operation and high precision. |
| Distance | 30 cm | 35 cm | 40 cm | 45 cm | 50 cm |
| RMS | 0.40 pixels | 0.39 pixels | 0.44 pixels | 0.47 pixels | 0.55 pixels |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, P.; Wang, S.; Wang, W.; Ye, Q.; Ye, S. Model-Independent Lens Distortion Correction Based on Sub-Pixel Phase Encoding. Sensors 2021, 21, 7465. https://doi.org/10.3390/s21227465
Xiong P, Wang S, Wang W, Ye Q, Ye S. Model-Independent Lens Distortion Correction Based on Sub-Pixel Phase Encoding. Sensors. 2021; 21(22):7465. https://doi.org/10.3390/s21227465
Chicago/Turabian StyleXiong, Pengbo, Shaokai Wang, Weibo Wang, Qixin Ye, and Shujiao Ye. 2021. "Model-Independent Lens Distortion Correction Based on Sub-Pixel Phase Encoding" Sensors 21, no. 22: 7465. https://doi.org/10.3390/s21227465
APA StyleXiong, P., Wang, S., Wang, W., Ye, Q., & Ye, S. (2021). Model-Independent Lens Distortion Correction Based on Sub-Pixel Phase Encoding. Sensors, 21(22), 7465. https://doi.org/10.3390/s21227465






