Advanced Structural Health Monitoring Method by Integrated Isogeometric Analysis and Distributed Fiber Optic Sensing
Abstract
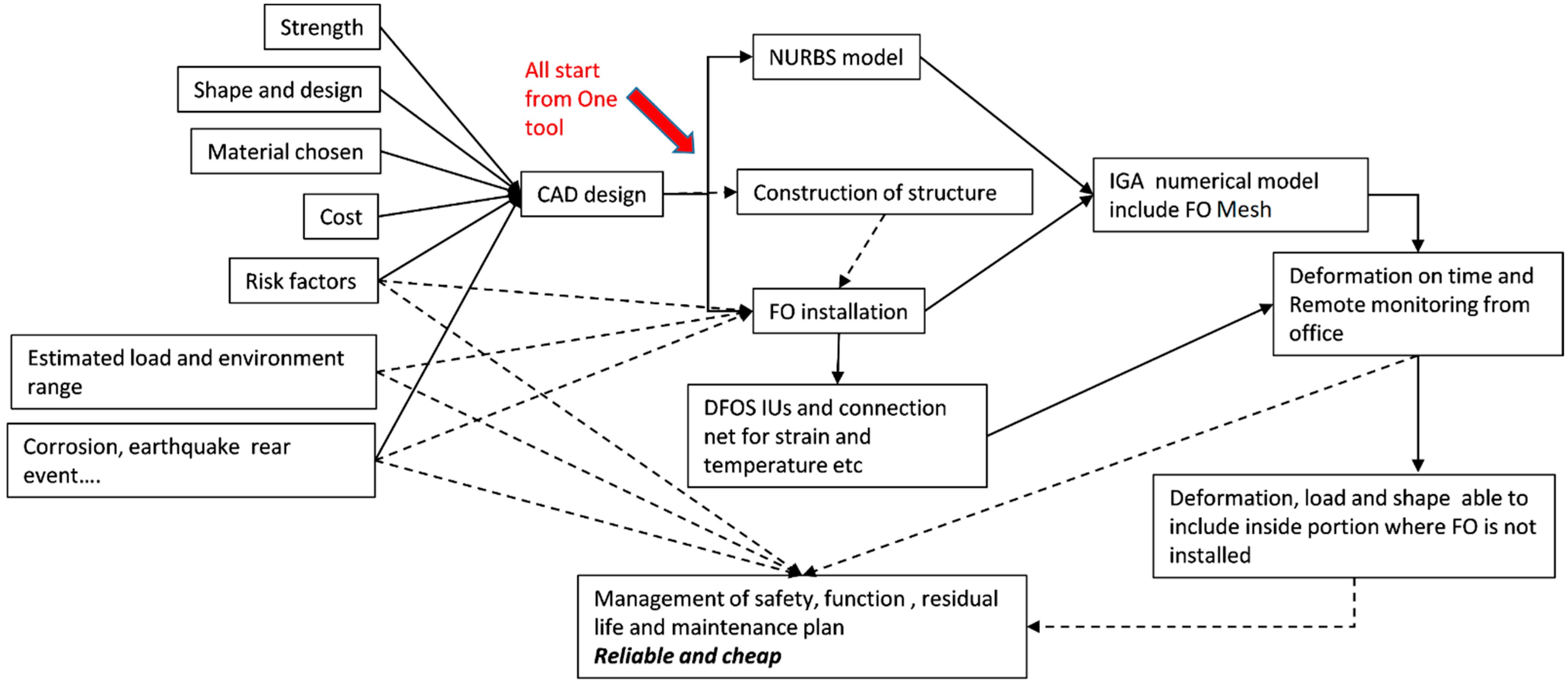
:1. Introduction
- Numerical analysis is dominated by FEM, but it does not utilize the CAD model data. Creation of a FEM model needs manual preprocessing jobs, which translates to high costs for general applications in engineering;
- Spatial resolution, accuracy, robustness and installation costs of onsite sensors need to be considered for practical usage. Using multiple discrete sensors such as strain gauges to cover a wide area requires complex wiring and data acquisition instruments with limited scalability.
Distributed Fiber Optical Sensing: State of the Art
2. Formulation of Integrated IGA and DFOS
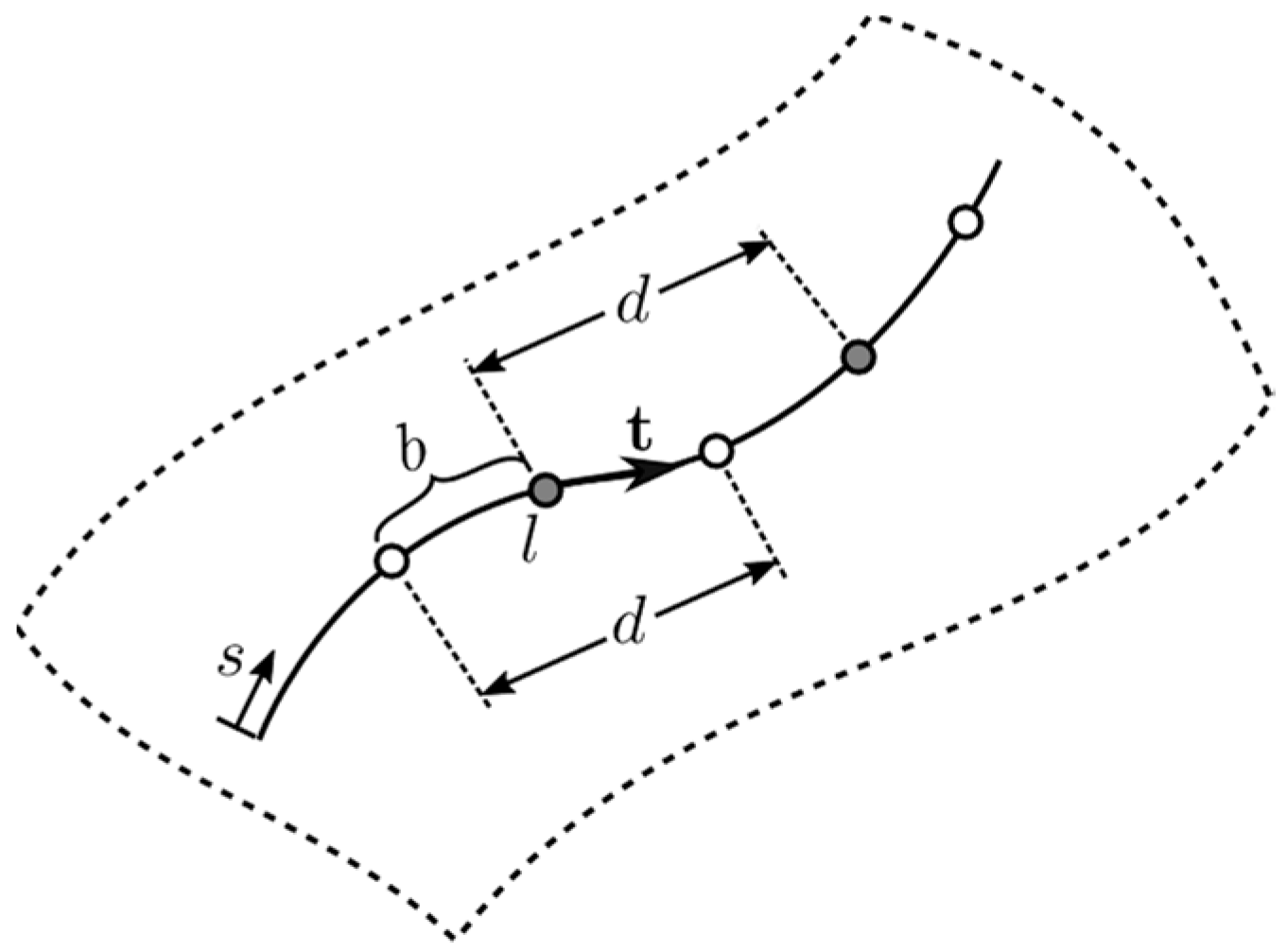
2.1. Coordinate Systems
2.2. B-Spline and NURBS Basis Functions
- Non-negativity;
- Partition of unity, which means basis functions add up to 1;
- Locality, which means the support or influence of a basis function extends only a limited region within the geometry;
- Convex hull, which means the geometry always lie inside the control polygon;
- Affine covariance, which means the geometry transforms in the same manner as the control points.
2.3. Isogeometric Analysis
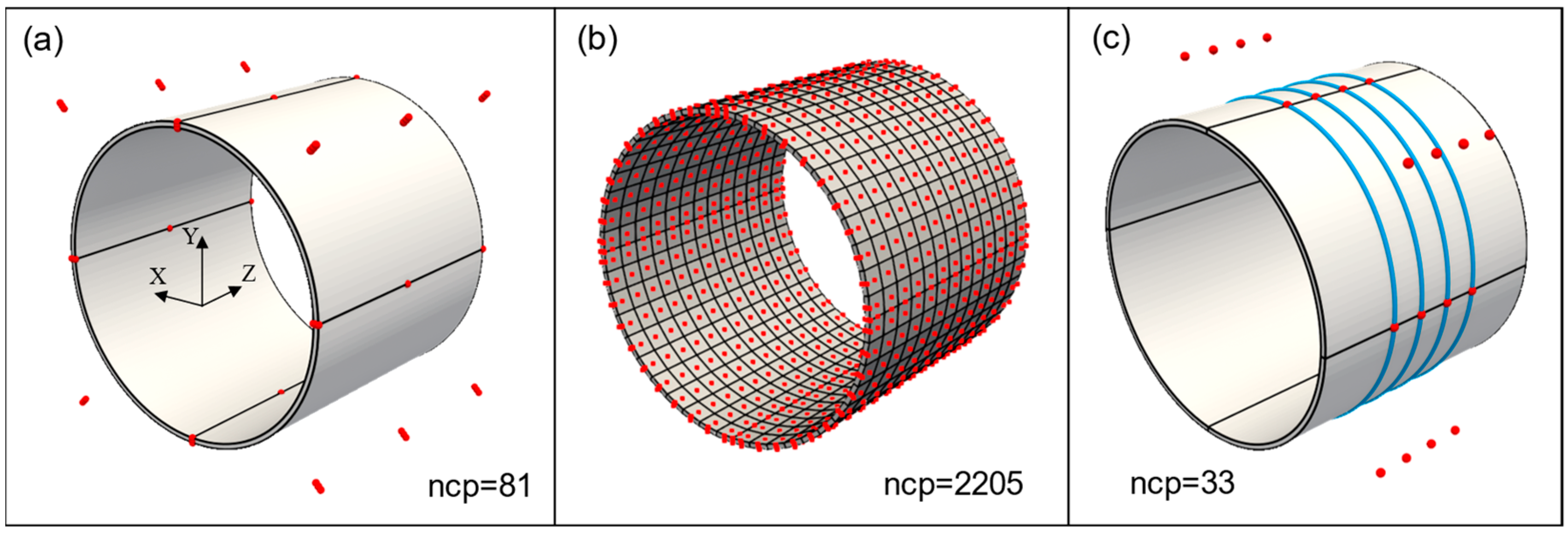
2.4. Fiber Mesh
2.5. Conversion from Strain Tensor to Fiber Strains
2.6. Merits of IGA and DFOS
3. Stress-Strain Analysis with Integrated IGA and DFOS
3.1. Experiment with a Cylindrical Pipe
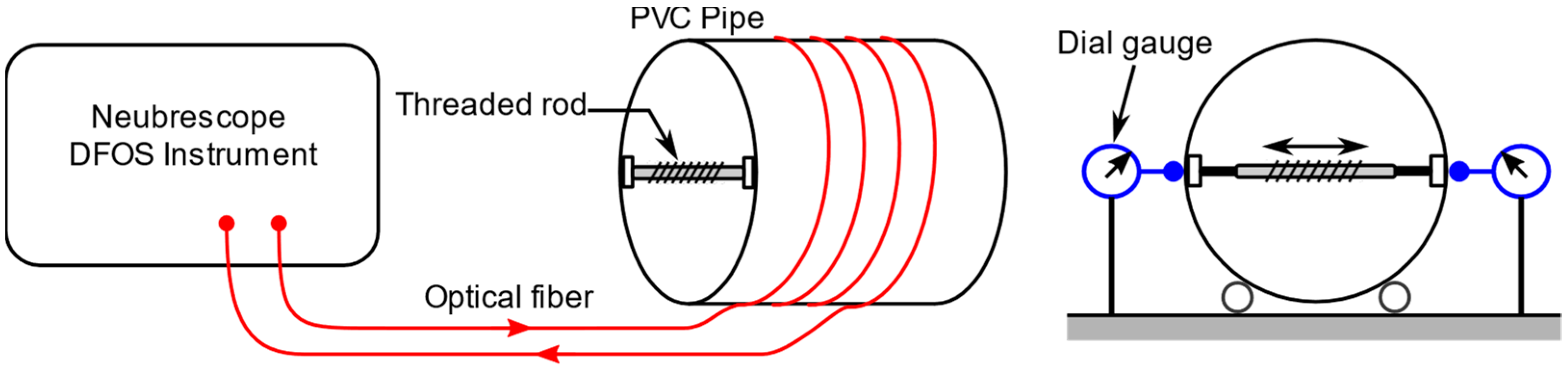
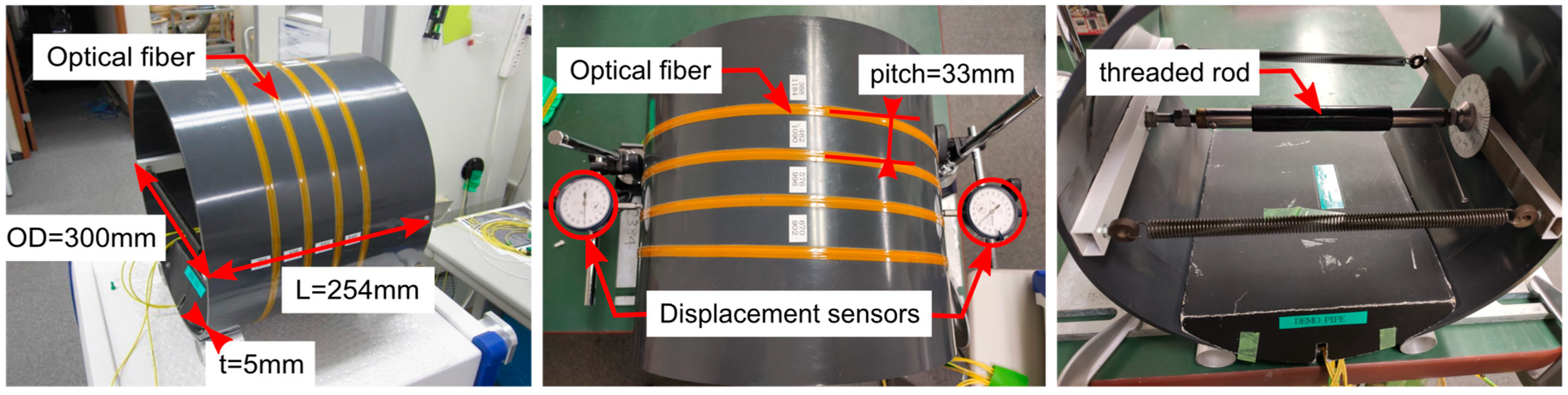
3.1.1. Experiment Setup
3.1.2. Experiment Procedure
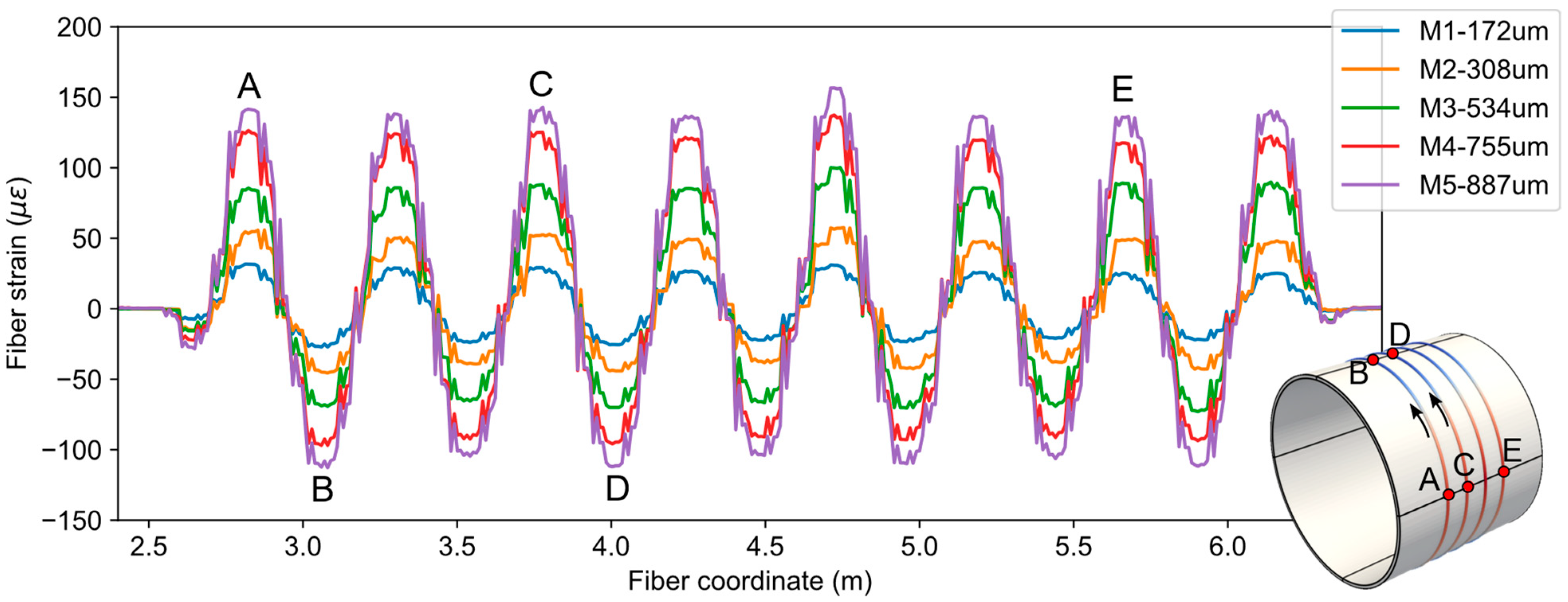
3.2. Fiber Strain Measurements
3.3. Forward IGA Using Idealized Boundary Conditions
3.3.1. Geometry and Analysis Mesh
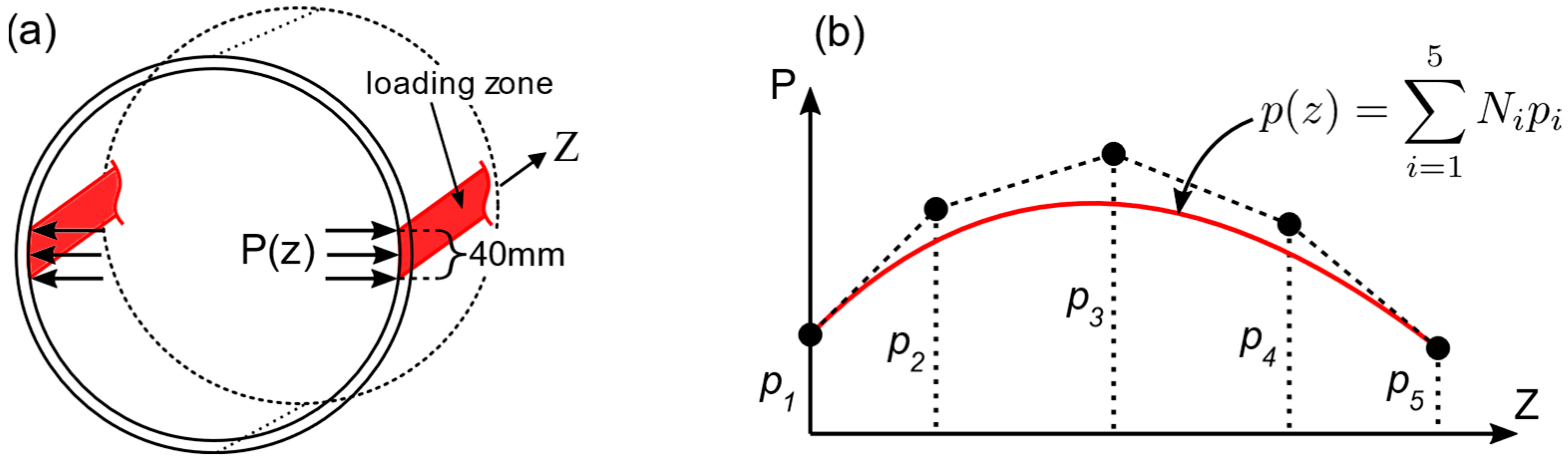
3.3.2. Boundary Conditions
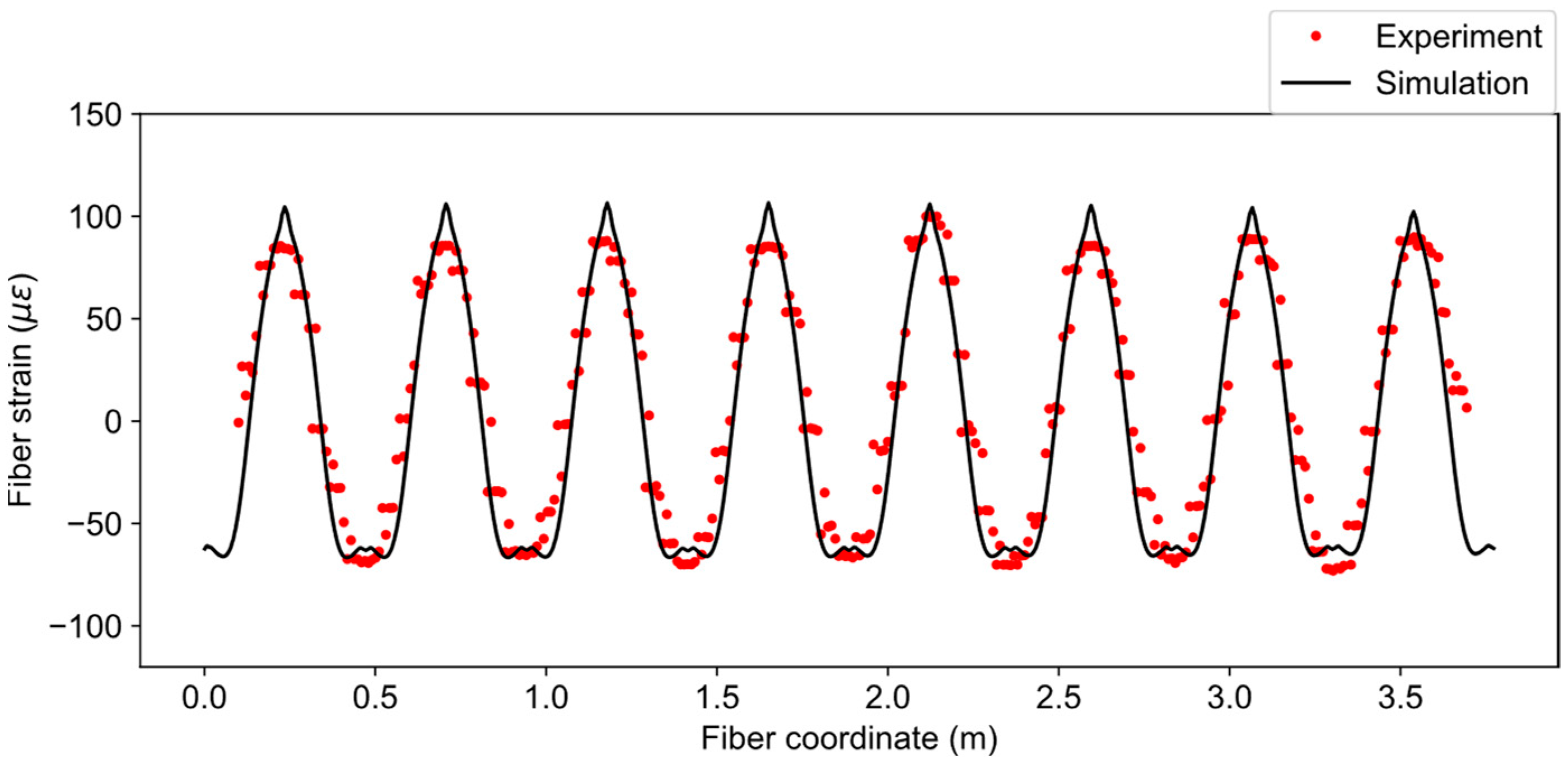
3.4. Results and Discussion
4. Estimation of Boundary Conditions Using Inverse IGA
4.1. Concept of Inverse IGA
4.2. Estimation of Boundary Conditions for the Pipe Model
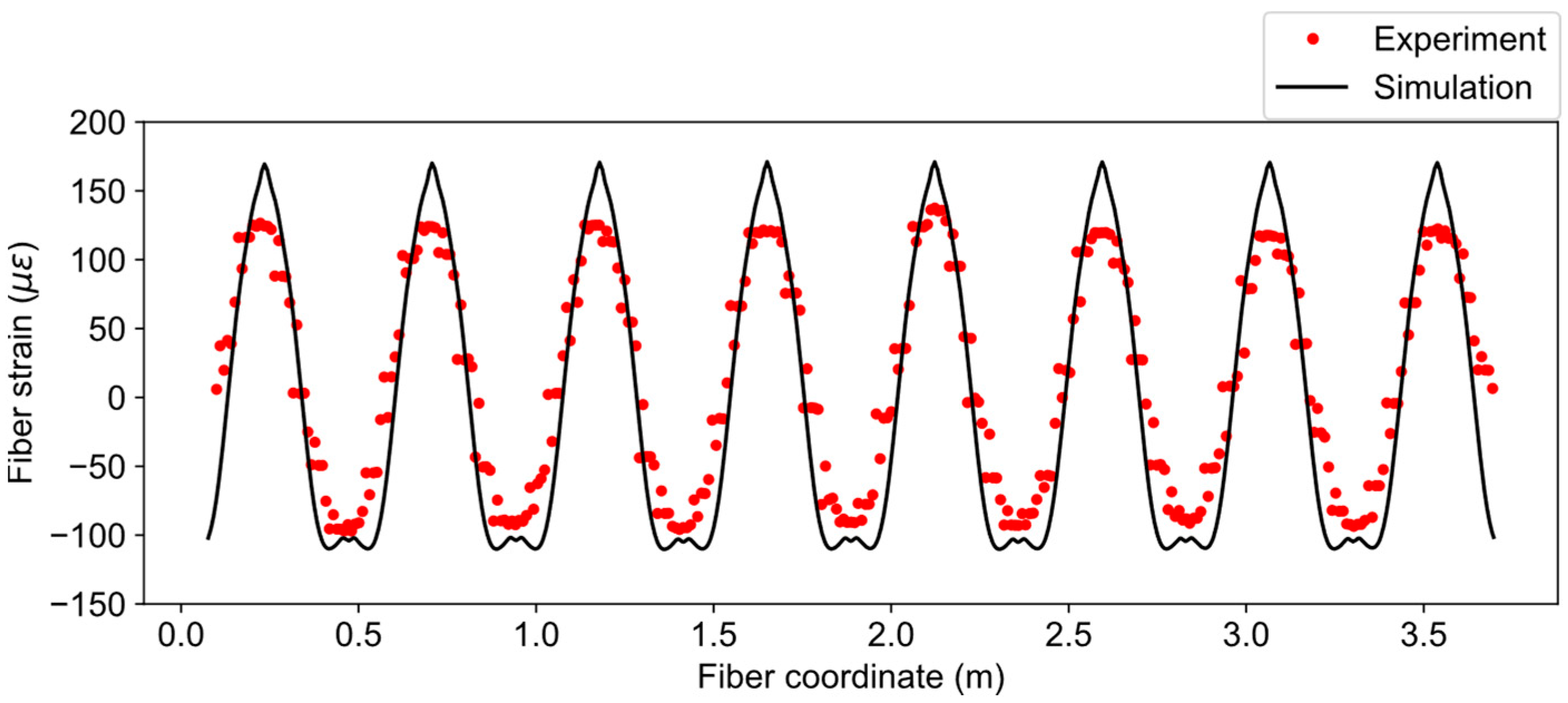
4.3. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Floris, I.; Adam, J.M.; Calderón, P.A.; Sales, S. Fiber Optic Shape Sensors: A comprehensive review. Opt. Lasers Eng. 2021, 139, 106508. [Google Scholar] [CrossRef]
- Yamauchi, Y.; Kishida, K. A Study of The Stability, Reliability, And Accuracy of Neubrescope-Based Pipe Thinning Detection System. In Proceedings of the 3rd International Conference on Structural Health Monitoring of Intelligent Infrastructure, Vancouver, BC, Canada, 13–16 November 2007. [Google Scholar]
- Kishida, K.; Li, C.H.; Nishiguchi, K.; Yamauchi, Y.; Guzik, A.; Tsuda, T. Hybrid Brillouin-Rayleigh distributed sensing system. In Proceedings of the OFS2012 22nd International Conference on Optical Fiber Sensors, Beijing, China, 14–19 October 2012; SPIE: Bellingham, WA, USA, 2012; Volume 8421, p. 84212G. [Google Scholar]
- Hotate, K. Recent achievements in BOCDA/BOCDR. In Proceedings of the IEEE Sensors, Valencia, Spain, 2–5 November 2014; pp. 142–145. [Google Scholar]
- Froggatt, M.; Moore, J. High-spatial-resolution distributed strain measurement in optical fiber with Rayleigh scatter. Appl. Opt. 1998, 37, 1735–1740. [Google Scholar] [CrossRef] [PubMed]
- Wu, Q.; Nair, S.; Shuck, M.; Van Oort, E.; Guzik, A.; Kishida, K. Advanced distributed fiber optic sensors for monitoring poor zonal isolation with hydrocarbon migration in cemented annuli. In Proceedings of the Society of Petroleum Engineers—SPE Deepwater Drilling and Completions Conference, Galveston, TX, USA, 14–15 September 2016. [Google Scholar]
- Nicolas, M.J.; Sullivan, R.W.; Richards, W.L. Fiber Bragg grating strains to obtain structural response of a carbon composite wing. In Proceedings of the ASME 2013 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, SMASIS 2013, Snowbird, UT, USA, 16–18 September 2013; Volume 2. [Google Scholar]
- Richards, L.; Parker, A.; Ko, W.; Piazza, A. Fiber Optic Wing Shape Sensing on NASA’s Ikhana UAV. In Proceedings of the NAVAIR Meeting, Edwards, CA, USA, 7 February 2008. [Google Scholar]
- Bakalyar, J.; Jutte, C. Validation Tests of Fiber Optic Strain-Based Operational Shape and Load Measurements. In Proceedings of the 53rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Honolulu, HI, USA, 23–26 April 2012; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Tan, X.; Abu-Obeidah, A.; Bao, Y.; Nassif, H.; Nasreddine, W. Measurement and visualization of strains and cracks in CFRP post-tensioned fiber reinforced concrete beams using distributed fiber optic sensors. Autom. Constr. 2021, 124, 103604. [Google Scholar] [CrossRef]
- Kishida, K.; Zhang, H.; Li, C.-H.; Guzik, A.; Suzuki, H.; Wu, Z. Diagnostic of Corrosion Based Thinning in Steam Pipelines by Means of Neubrescope High Precision Optical Fiber Sensing System. In Proceedings of the 5th International Workshop on Structural Health Monitoring, Stanford, CA, USA, 12–14 September 2005. [Google Scholar]
- Piccolo, A.; Lecieux, Y.; Delepine-Lesoille, S.; Leduc, D. Non-invasive tunnel convergence measurement based on distributed optical fiber strain sensing. Smart Mater. Struct. 2019, 28, 045008. [Google Scholar] [CrossRef] [Green Version]
- Kulpa, M.; Howiacki, T.; Wiater, A.; Siwowski, T.; Sieńko, R. Strain and displacement measurement based on distributed fibre optic sensing (DFOS) system integrated with FRP composite sandwich panel. Meas. J. Int. Meas. Confed. 2021, 175, 109099. [Google Scholar] [CrossRef]
- Sui, Y.; Cheng, X.; Wei, J. Distributed fibre optic monitoring of damaged lining in double-arch tunnel and analysis of its deformation mode. Tunn. Undergr. Space Technol. 2021, 110, 103812. [Google Scholar] [CrossRef]
- Wu, M.C.H.; Kamensky, D.; Wang, C.; Herrema, A.J.; Xu, F.; Pigazzini, M.S.; Verma, A.; Marsden, A.L.; Bazilevs, Y.; Hsu, M.C. Optimizing fluid–Structure interaction systems with immersogeometric analysis and surrogate modeling: Application to a hydraulic arresting gear. Comput. Methods Appl. Mech. Eng. 2017, 316, 668–693. [Google Scholar] [CrossRef] [Green Version]
- Tessler, A.; Spangler, J.L. A least-squares variational method for full-field reconstruction of elastic deformations in shear-deformable plates and shells. Comput. Methods Appl. Mech. Eng. 2005, 194, 327–339. [Google Scholar] [CrossRef]
- Kefal, A.; Tabrizi, I.E.; Yildiz, M.; Tessler, A. A smoothed iFEM approach for efficient shape-sensing applications: Numerical and experimental validation on composite structures. Mech. Syst. Signal Process. 2021, 152, 107486. [Google Scholar] [CrossRef]
- Bazilevs, Y.; Beirão Da Veiga, L.; Cottrell, J.A.; Hughes, T.J.R.; Sangalli, G. Isogeometric Analysis: Approximation, Stability and Error Estimates for h-refined Meshes. Math. Model. Methods Appl. Sci. 2006, 16, 1031–1090. [Google Scholar] [CrossRef]
- Cottrell, J.A.; Hughes, T.J.R.; Bazilevs, Y. Isogeometric Analysis: Toward Integration of CAD and FEA; Wiley: Hoboken, NJ, USA, 2009; ISBN 9780470748732. [Google Scholar]
- Nguyen, V.P.; Anitescu, C.; Bordas, S.P.A.; Rabczuk, T. Isogeometric analysis: An overview and computer implementation aspects. Math. Comput. Simul. 2015, 117, 89–116. [Google Scholar] [CrossRef] [Green Version]
- Casquero, H.; Wei, X.; Toshniwal, D.; Li, A.; Hughes, T.J.R.; Kiendl, J.; Zhang, Y.J. Seamless integration of design and Kirchhoff–Love shell analysis using analysis-suitable unstructured T-splines. Comput. Methods Appl. Mech. Eng. 2020, 360, 112765. [Google Scholar] [CrossRef]
- De Luycker, E.; Benson, D.J.; Belytschko, T.; Bazilevs, Y.; Hsu, M.C. X-FEM in isogeometric analysis for linear fracture mechanics. Int. J. Numer. Methods Eng. 2011, 87, 541–565. [Google Scholar] [CrossRef] [Green Version]
- Herrema, A.J.; Wiese, N.M.; Darling, C.N.; Ganapathysubramanian, B.; Krishnamurthy, A.; Hsu, M.C. A framework for parametric design optimization using isogeometric analysis. Comput. Methods Appl. Mech. Eng. 2017, 316, 944–965. [Google Scholar] [CrossRef] [Green Version]
- Benzaken, J.; Herrema, A.J.; Hsu, M.-C.; Evans, J.A. A Rapid and Efficient Isogeometric Design Space Exploration Framework with Application to Structural Mechanics. Comput. Methods Appl. Mech. Eng. 2017, 316, 1215–1256. [Google Scholar] [CrossRef] [Green Version]
- Agrawal, V.; Gautam, S.S. IGA: A Simplified Introduction and Implementation Details for Finite Element Users. J. Inst. Eng. Ser. C 2018, 100, 561–585. [Google Scholar] [CrossRef]
- Kumar, M.; Kvamsdal, T.; Johannessen, K.A. Superconvergent patch recovery and a posteriori error estimation technique in adaptive isogeometric analysis. Comput. Methods Appl. Mech. Eng. 2017, 316, 1086–1156. [Google Scholar] [CrossRef]
- Ma, N.; Takata, K.; Shimizu, N. Isogeometric Analysis and Its Application to Sheet Metal Forming Simulation (in Japanese). Plastics 2020, 3, 400–404. [Google Scholar] [CrossRef]
- Di Sante, R. Fibre Optic Sensors for Structural Health Monitoring of Aircraft Composite Structures: Recent Advances and Applications. Sensors 2015, 15, 18666–18713. [Google Scholar] [CrossRef]
- Fan, L.; Bao, Y. Review of fiber optic sensors for corrosion monitoring in reinforced concrete. Cem. Concr. Compos. 2021, 120, 104029. [Google Scholar] [CrossRef]
- Xu, C.; Sharif Khodaei, Z. Shape Sensing with Rayleigh Backscattering Fibre Optic Sensor. Sensors 2020, 20, 4040. [Google Scholar] [CrossRef]
- Horiguchi, T.; Shimizu, K.; Kurashima, T.; Tateda, M.; Koyamada, Y. Development of a Distributed Sensing Technique Using Brillouin Scattering. J. Lightwave Technol. 1995, 13, 1296–1302. [Google Scholar] [CrossRef]
- Hotate, K.; Tanaka, M. Distributed fiber brillouin strain sensing with 1-cm spatial resolution by correlation-based continuous-wave technique. IEEE Photonics Technol. Lett. 2002, 14, 179–181. [Google Scholar] [CrossRef]
- Piegl, L.; Tiller, W. The NURBS Book; Monographs in Visual Communications; Springer: Berlin/Heidelberg, Germany, 1995; ISBN 978-3-642-97387-1. [Google Scholar]
- Rogers, D.F. An Introduction to NURBS: With Historical Perspective; Morgan Kaufmann Publishers: Burlington, MA, USA, 2001; ISBN 9781558606692. [Google Scholar]
- Hughes, T.J.R.; Cottrell, J.A.; Bazilevs, Y. Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput. Methods Appl. Mech. Eng. 2005, 194, 4135–4195. [Google Scholar] [CrossRef] [Green Version]
- Kefal, A.; Oterkus, E. Isogeometric iFEM Analysis of Thin Shell Structures. Sensors 2020, 20, 2685. [Google Scholar] [CrossRef]








| IGA | Classical FEM | DFOS | Strain Gauge |
|---|---|---|---|
| Can use CAD model directly | Cannot use CAD model directly | Distributed measurement data | Discrete sensors for multiple locations |
| Exact geometry | Approximate geometry | High spatial resolution | Single point |
| Same basis functions for the geometry, fiber model and solution fields | Different basis functions (need data conversion between models) | Long range (~25 km) in a single fiber | Require multiplexers |
| High efficiency in terms of total DOF in the system | Low efficiency in terms of total DOF in system | Remote, 24/7 monitoring | |
| continuous across element boundaries | continuous across element boundaries | Stable in harsh environments |
| Material Type | Polyvinylchloride (PVC) |
|---|---|
| Young’s modulus (nominal) | 2.8 GPa |
| Poisson’s ratio (nominal) | 0.38 |
| Optical Technology | TW-COTDR |
|---|---|
| Sampling interval | 1 cm |
| Spatial resolution | 2 cm |
| Averaging count | 216 |
| Repeatability |
| Initial Loading | Optimized Loading | |
|---|---|---|
| RMS fiber strain error | 13.421 με (7.318%) | 5.863 με (3.197%) |
| Max fiber strain error | 30.539 με (16.652%) | 16.245 με (8.858%) |
| Displacement error | - | 3.914% * |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aung, T.L.; Ma, N.; Kishida, K.; Guzik, A. Advanced Structural Health Monitoring Method by Integrated Isogeometric Analysis and Distributed Fiber Optic Sensing. Sensors 2021, 21, 5794. https://doi.org/10.3390/s21175794
Aung TL, Ma N, Kishida K, Guzik A. Advanced Structural Health Monitoring Method by Integrated Isogeometric Analysis and Distributed Fiber Optic Sensing. Sensors. 2021; 21(17):5794. https://doi.org/10.3390/s21175794
Chicago/Turabian StyleAung, Thein Lin, Ninshu Ma, Kinzo Kishida, and Artur Guzik. 2021. "Advanced Structural Health Monitoring Method by Integrated Isogeometric Analysis and Distributed Fiber Optic Sensing" Sensors 21, no. 17: 5794. https://doi.org/10.3390/s21175794
APA StyleAung, T. L., Ma, N., Kishida, K., & Guzik, A. (2021). Advanced Structural Health Monitoring Method by Integrated Isogeometric Analysis and Distributed Fiber Optic Sensing. Sensors, 21(17), 5794. https://doi.org/10.3390/s21175794






