Three-dimensional Magnetic Induction Tomography: Improved Performance for the Center Regions inside a Low Conductive and Voluminous Body
Abstract
:1. Introduction
2. Materials and Methods
2.1. Characteristics of Limiting Noise
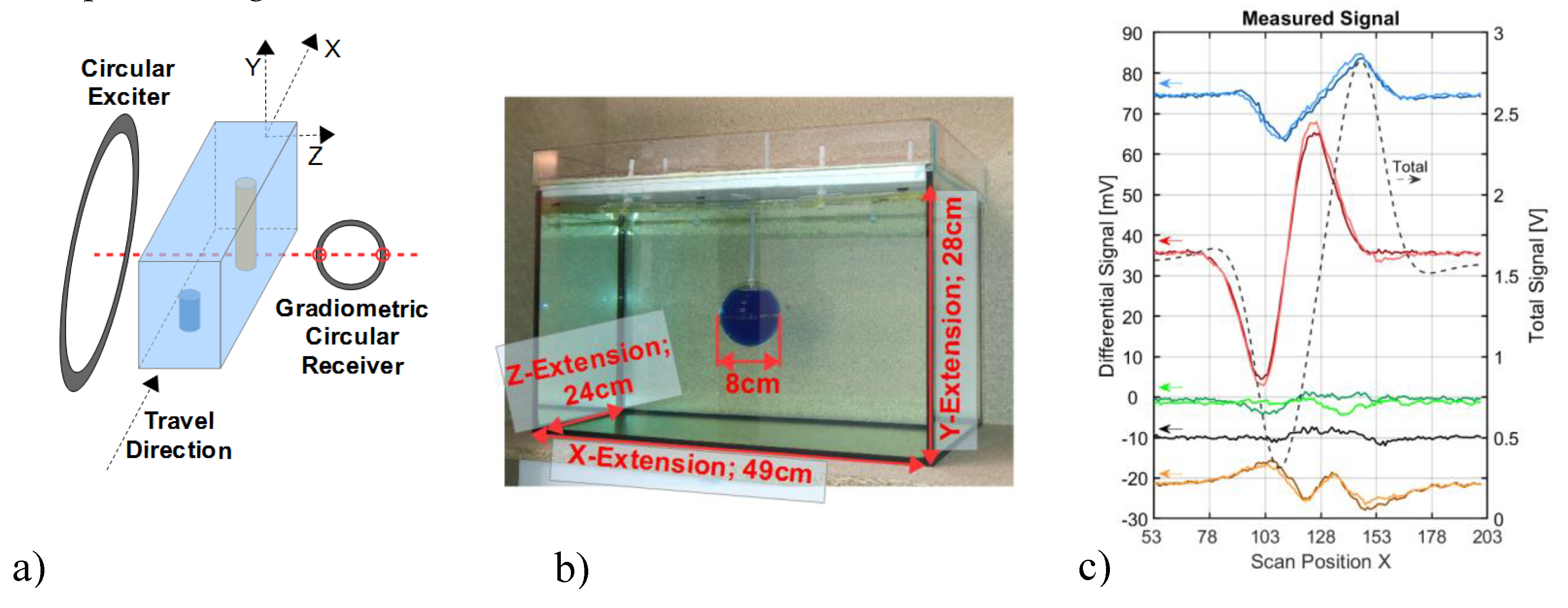
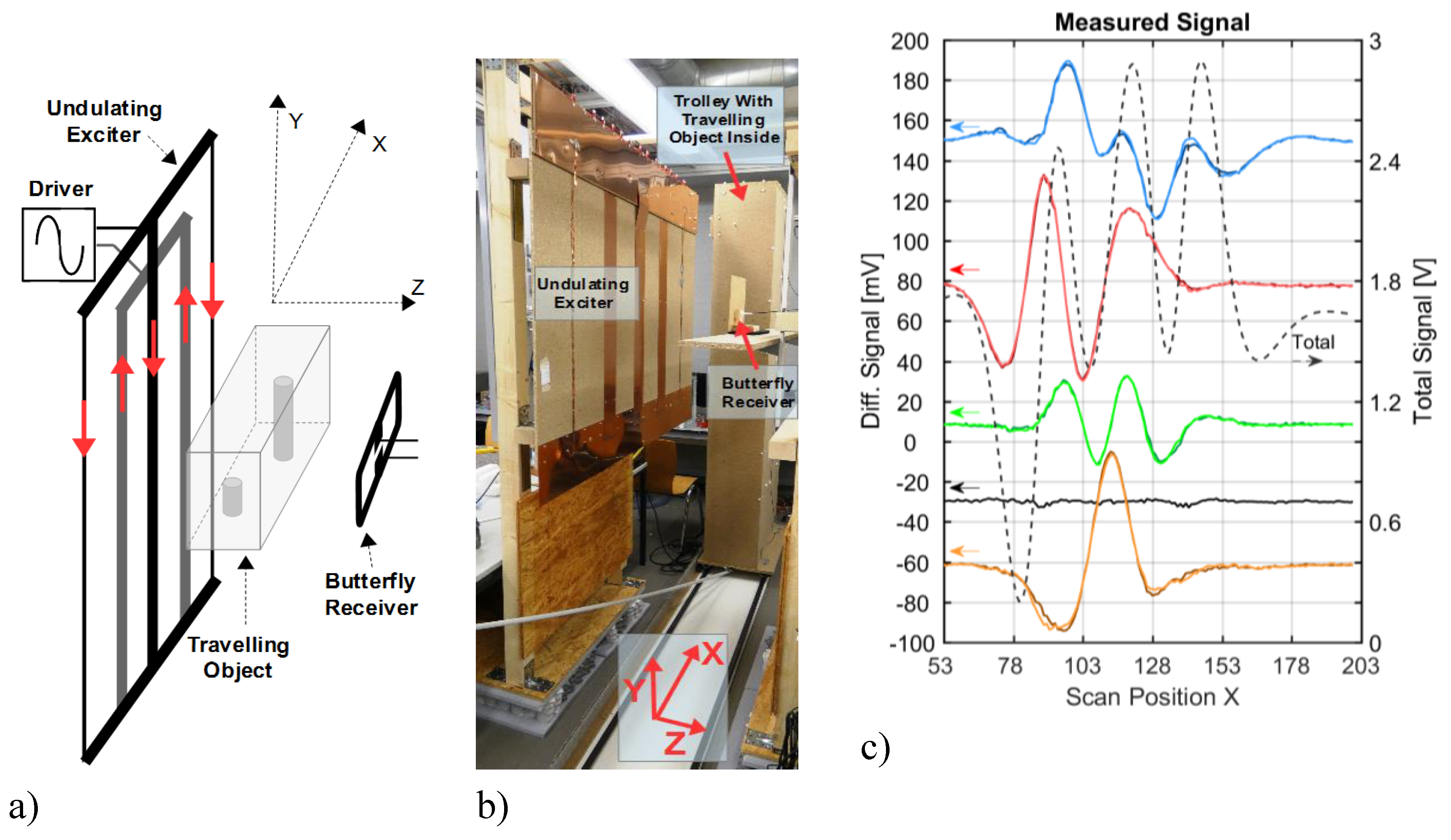
2.2. Three Different Measurement Configurations
2.3. Mathematical Signal Calculation
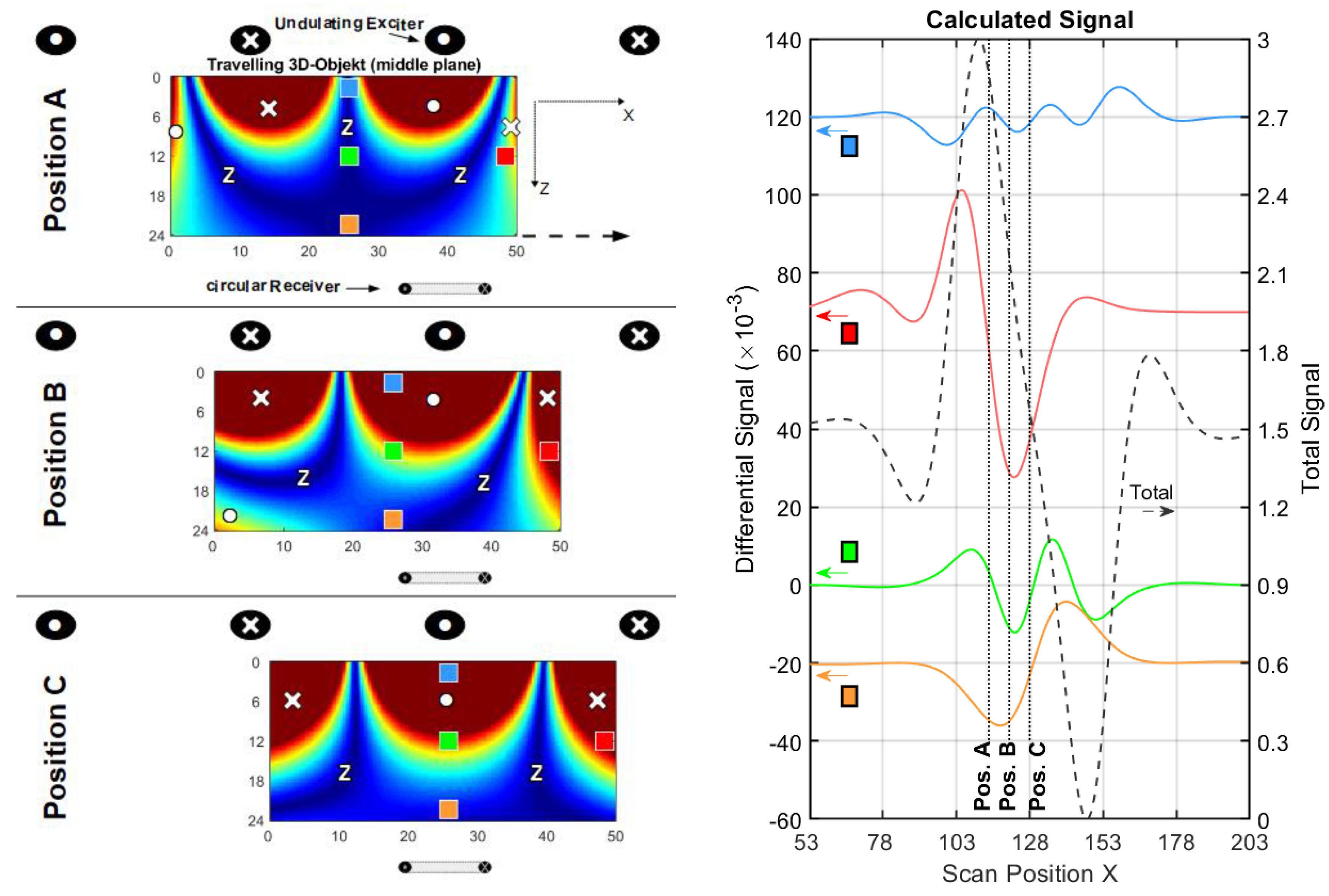
2.3.1. Special Case-Undulating Exciter
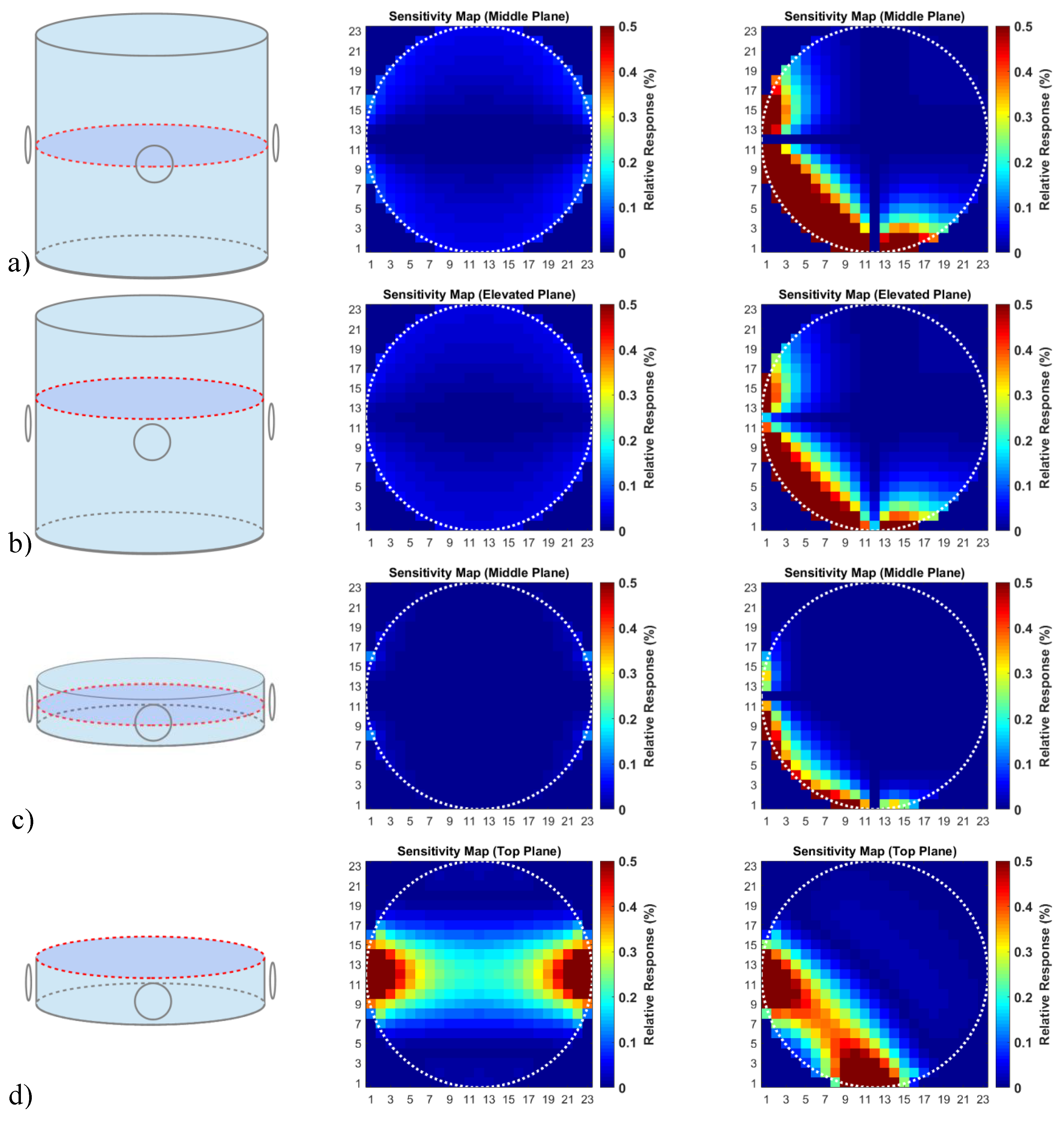
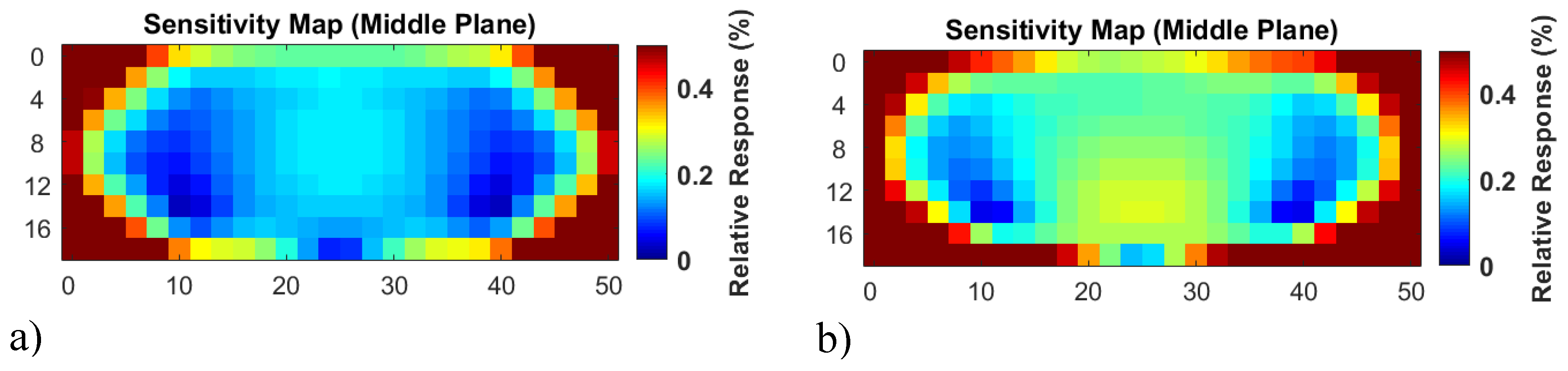
2.4. Differential Signal, Sensitivity Matrix, and Sensitivity Map
2.5. Iterative Image Reconstruction
- An “unknown” body with an inhomogeneous conductivity distribution is measured (here, calculated via the forward problem) and results in the “real” signal . Noise is added to the computed signal with the SNR being set to 50 dB; thus, it is 10 dB lower than the practical SNR, shown in Figure 2.
- An estimated body with the same outer contour, but with a homogenous conductivity distribution is calculated, and results in .
- For subsequent and iteratively corrected body estimations, the total signal difference () should be minimized (i.e., minimizing the root mean square [RMS] of ), ideally down to the noise level. Therefore, the homogenous conductivity distribution inside the estimated body is locally increased or decreased by small amounts to maintain the stability of the iterative procedure within certain limits (e.g., the conductivity cannot become negative, and it also cannot exceed specific values for biological tissue), based on the inverted and regularized sensitivity matrix (Jacobian ) and the differential signal [21].
- The corrected leads to a new , which in turn leads to a generally decreased RMS of the new differential signal . A new must be calculated for the modified conductivity distribution, and further correction of the estimated body occurs with the current .
- The overall procedure is repeated until no further decrease of can be obtained. Then, the last modified conductivity distribution should approach the unknown conductivity distribution of the real body, within the general limits of the ill-posed MIT principle.
3. Analysis of Three Different Exciter Setups and Their Effect on the Eddy Current Distribution and the Obtained Sensitivity
3.1. Eddy Current Distribution in a Travelling Conductive Sheet (2D Object)
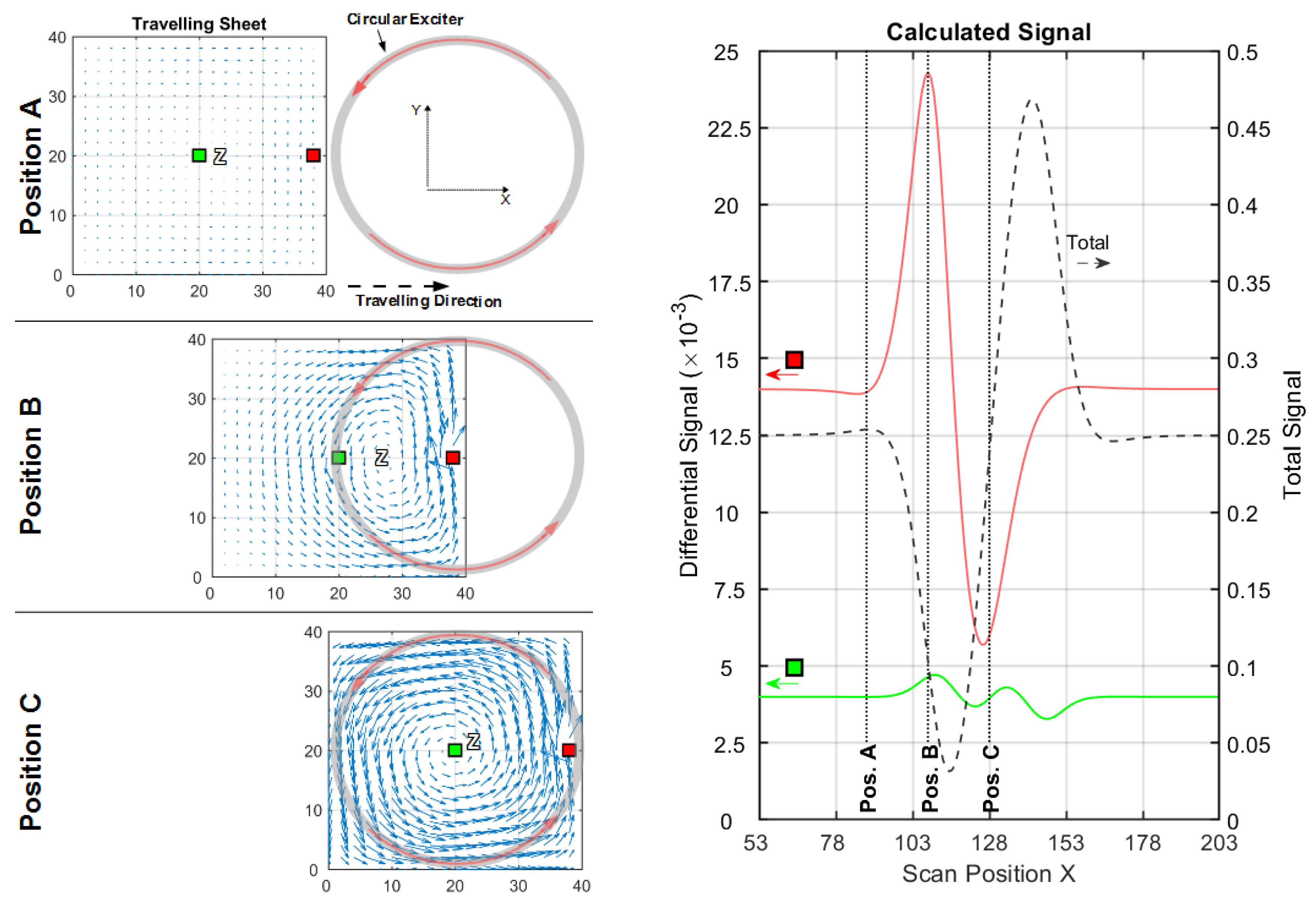
3.1.1. Conductive Sheet in a Circular Coil Setup
3.1.2. Conductive Sheet in a Vertical Wire Setup
- When tracing the current values of the O-mode (Figure 5, position C) along the midline (x-direction) at 20 cm, the result would be similar to half a period of a sinusoidal signal. The O-mode corresponds to a spatial frequency in the x-direction, and the lateral size of the sheet equals half a period of this spatial frequency.
- When tracing the current values of the Φ-mode (Figure 7, position C) along the x-direction, the result would be similar to a full period of a sinusoidal signal, i.e., a doubled spatial frequency in the x-direction with respect to the O-mode.
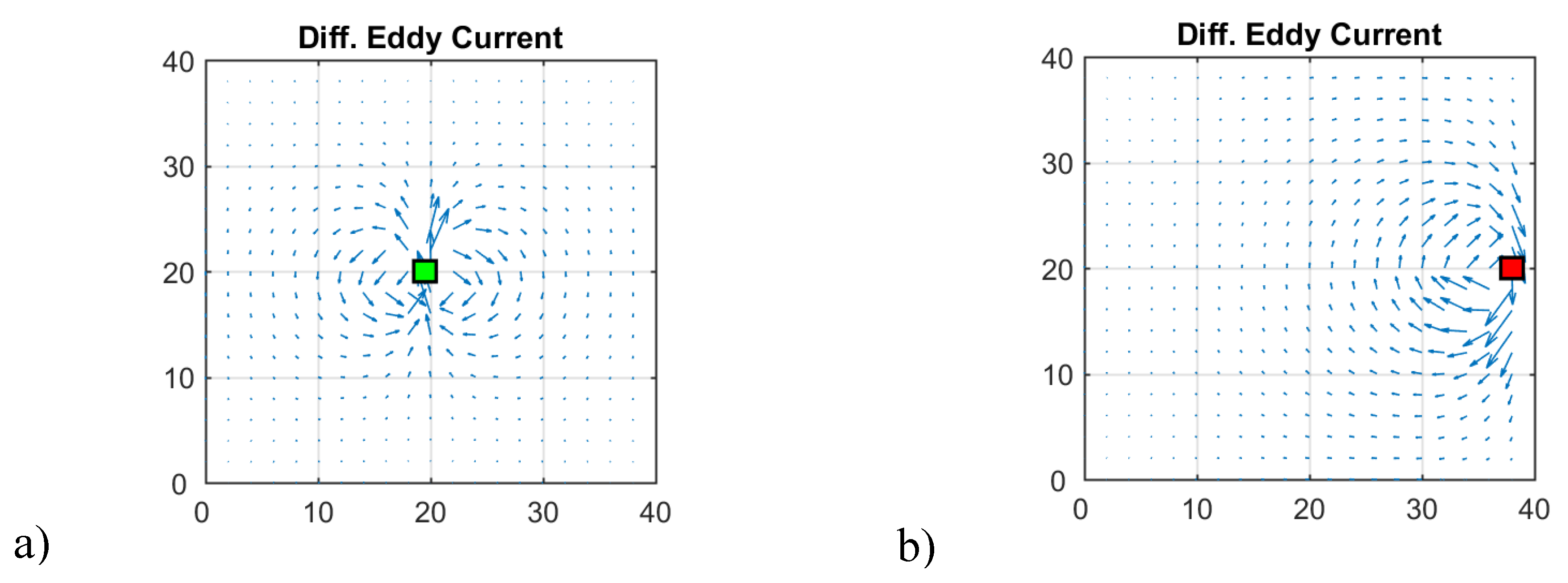
3.1.3. Conductive Sheet in a Undulator Exciter Setup
3.1.4. Intermediate Discussion (2D)
3.2. Eddy Current Distribution in a Travelling Conductive Volume (3D Object)
3.2.1. 3D Object in a Circular Coil Setup
3.2.2. 3D Object in a Vertical Wire Setup
3.2.3. 3D Object in an Undulator Setup
3.2.4. Intermediate Discussion (3D)
3.3. Experimental Verification
4. Three-dimensional Reconstruction of Low Conducting and Voluminous Bodies
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A: Vector Potential of an Infinite Undulator in the X-y-plane
Appendix B: Vector Potential of a Linear Current Thread and a Current Loop
Appendix C: Field of a Geometrically Limited Undulator
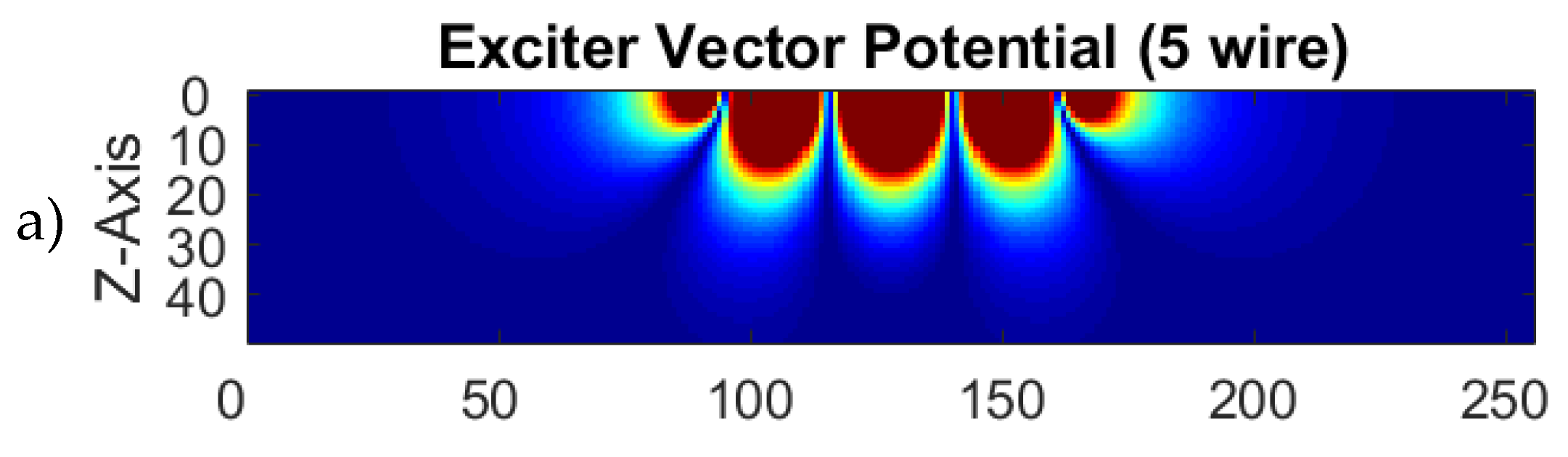

Appendix D: Shortcut for the Analysis of the Forward Problem

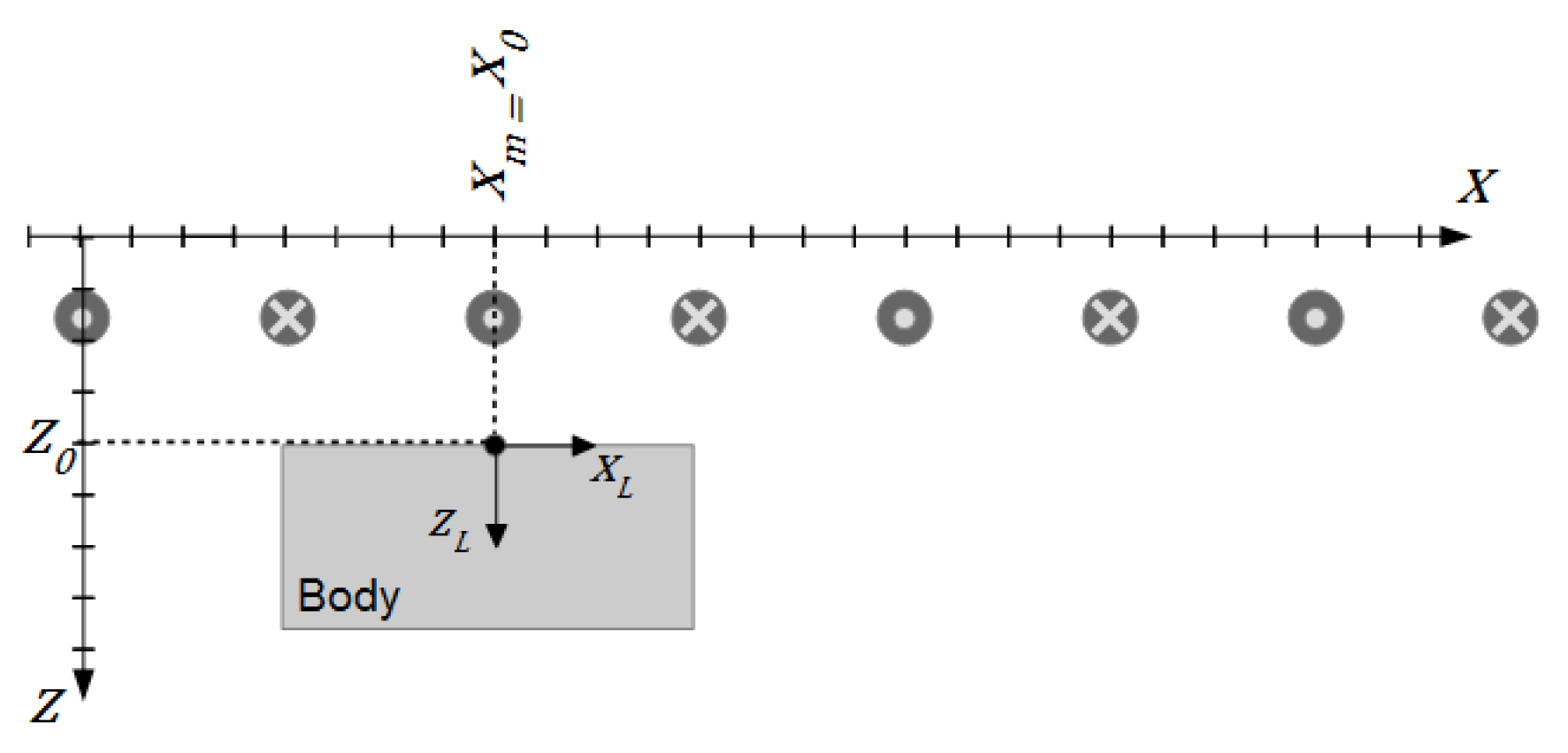
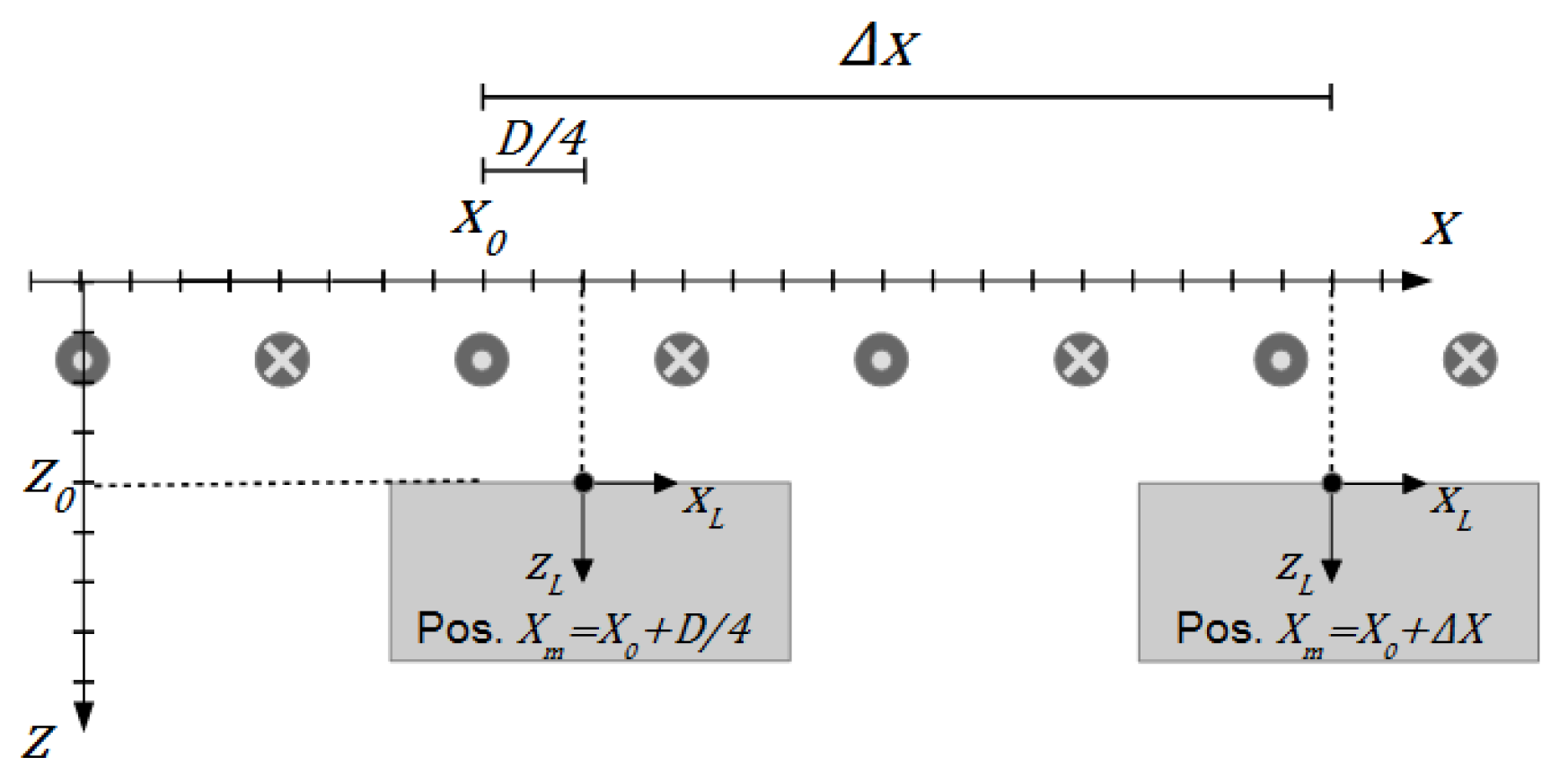
Appendix E: Applicability of the Support of the Undulator for more Arbitrarily Shaped Bodies

Appendix F: Conductive Sheet in a Circular Exciter Setup with a Parallel Receiver

| Total Signal (max. Amplitude) | Edge Diff. Signal (Max. Amplitude) | Center Diff. Signal (Max. Amplitude) | Edge Sensitivity 1 | Center Sensitivity 1 (CAS) |
|---|---|---|---|---|
Appendix G: Conductive Sheet in a Undulator Exciter Setup with a Circular Receiver
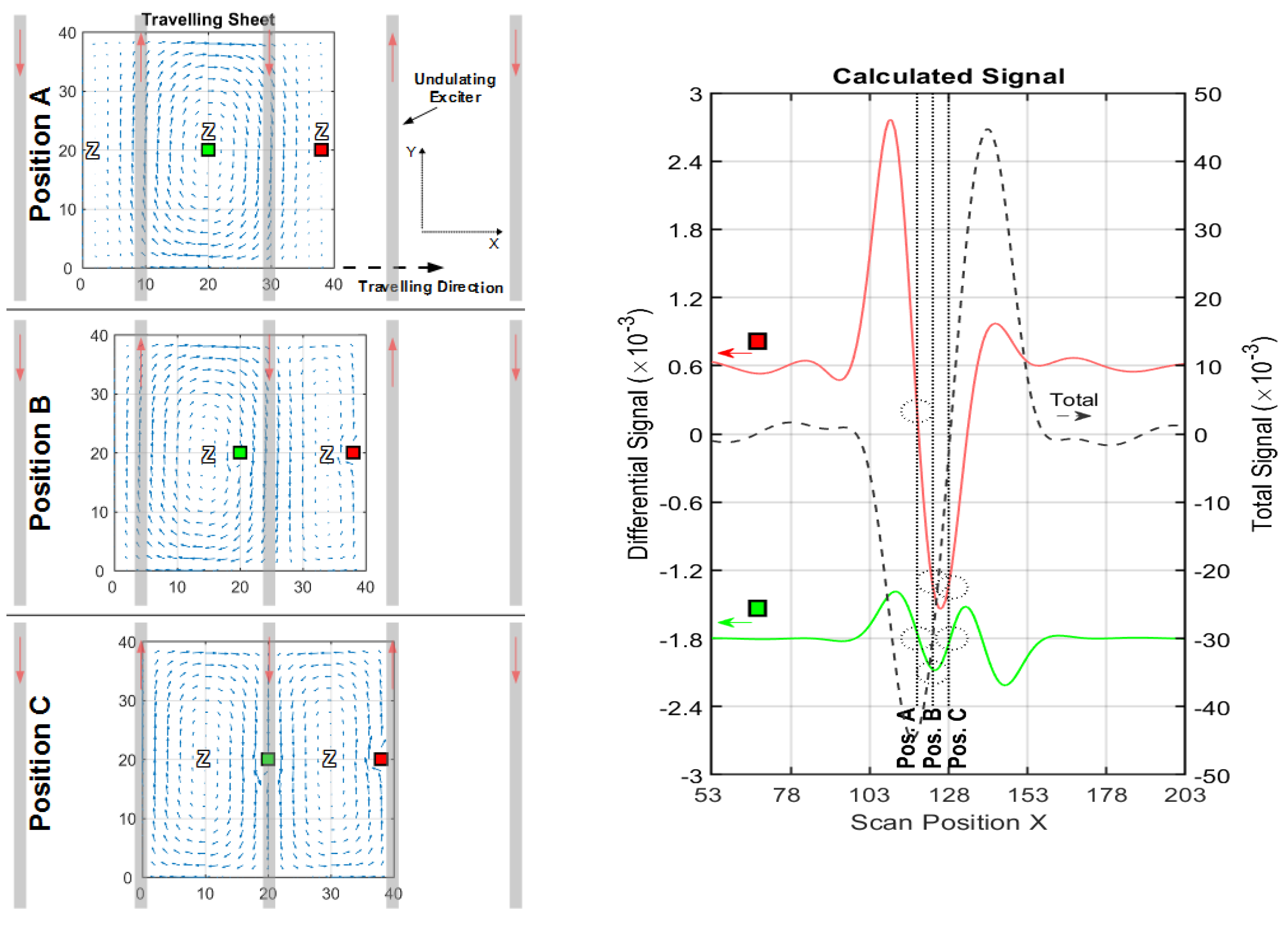
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
Appendix H: Conductive Volume in a Undulator Exciter Setup with a Circular Receiver
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
References
- Wei, H.; Soleimani, M. Electromagnetic tomography for medical and industrial applications: Challenges and opportunities [Point of View]. Proc. IEEE 2013, 101, 559–565. [Google Scholar] [CrossRef]
- Wei, H.-Y.; Soleimani, M. Hardware and software design for a National Instrument-based magnetic induction tomography system for prospective biomedical applications. Physiol. Meas. 2012, 33, 863–879. [Google Scholar] [CrossRef] [PubMed]
- Scharfetter, H.; Rauchenzauner, S.; Merwa, R.; Biró, O.; Hollaus, K. Planar gradiometer for magnetic induction tomography (MIT): Theoretical and experimental sensitivity maps for a low-contrast phantom. Physiol. Meas. 2004, 25, 325–333. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.; Soleimani, M. Magnetic induction tomography methods and applications: A review. Meas. Sci. Technol. 2017, 28, 072001. [Google Scholar] [CrossRef] [Green Version]
- Wei, H.-Y.; Soleimani, M. Two-phase low conductivity flow imaging using magnetic induction tomography. Prog. Electromagn. Res. 2012, 131, 99–115. [Google Scholar] [CrossRef] [Green Version]
- Merwa, R.; Brunner, P.; Missner, A.; Hollaus, K.; Scharfetter, H. Solution of the inverse problem of magnetic induction tomography (MIT) with multiple objects: Analysis of detectability and statistical properties with respect to the reconstructed conducting region. Physiol. Meas. 2006, 27, S249–S259. [Google Scholar] [CrossRef]
- Vauhkonen, M.; Hamsch, M.; Igney, C.H. A measurement system and image reconstruction in magnetic induction tomography. Physiol. Meas. 2008, 29, S445–S454. [Google Scholar] [CrossRef]
- Scharfetter, H.; Hollaus, K.; Rosell-Ferrer, J.; Merwa, R. Single-step 3-D image reconstruction in magnetic induction tomography: Theoretical limits of spatial resolution and contrast to noise ratio. Ann. Biomed. Eng. 2006, 34, 1786–1798. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z.; Luo, H.; He, W.; He, C.; Song, X.; Zahng, Z. A multi-channel magnetic induction tomography measurement system for human brain model imaging. Physiol. Meas. 2009, 30, S175–S186. [Google Scholar] [CrossRef]
- Wei, H.-Y.; Ma, L.; Soleimani, M. Volumetric magnetic induction tomography. Meas. Sci. Technol. 2012, 23, 055401. [Google Scholar] [CrossRef]
- Igney, C.H.; Watson, S.; Williams, R.J.; Griffiths, H.; Dossel, O. Design and performance of a planar-array MIT system with normal sensor alignment. Physiol. Meas. 2005, 26, S263. [Google Scholar] [CrossRef] [PubMed]
- Klein, M.; Rueter, D. A large and quick induction field scanner for examining the interior of extended objects or humans. Prog. Electromagn. Res. B 2017, 78, 155–173. [Google Scholar] [CrossRef] [Green Version]
- Dekdouk, B.; Yin, W.; Ktistis, C.; Armitage, D.W.; Peyton, A.J. A method to solve the forward problem in magnetic induction tomography based on the weakly coupled field approximation. IEEE Trans. Biomed. Eng. 2010, 57, 914–921. [Google Scholar] [CrossRef] [PubMed]
- Armitage, D.W.; LeVeen, H.H.; Pethig, R. Radiofrequency-induced hyperthermia: Computer simulation of specific absorption rate distributions using realistic anatomical models. Phys. Med. Biol. 1983, 28, 31–42. [Google Scholar] [CrossRef] [PubMed]
- Marmugi, L.; Deans, C.; Renzoni, F. Electromagnetic induction imaging with atomic magnetometers: Unlocking the low-conductivity regime. Appl. Phys. Lett. 2019, 115, 083503. [Google Scholar] [CrossRef]
- Bottomley, P.A.; Andrew, E.R. RF magnetic field penetration, phase shift and power dissipation in biological tissue: Implications for NMR imaging. Phys. Med. Biol. 1978, 23, 630–643. [Google Scholar] [CrossRef]
- Gürsoy, D.; Scharfetter, H. Reconstruction artefacts in magnetic induction tomography due to patient’s movement during data acquisition. Physiol. Meas. 2009, 30, S165–S174. [Google Scholar] [CrossRef]
- Ma, L.; Wei, H.-Y.; Soleimani, M. Planar magnetic induction tomography for 3D near subsurface imaging. Prog. Electromagn. Res. 2013, 138, 65–82. [Google Scholar] [CrossRef] [Green Version]
- Morris, A.; Griffiths, H.; Gough, W. A numerical model for magnetic induction tomographic measurements in biological tissues. Physiol. Meas. 2001, 22, 113–119. [Google Scholar] [CrossRef]
- Ktistis, C.; Armitage, D.W.; Peyton, A.J. Calculation of the forward problem for absolute image reconstruction in MIT. Physiol. Meas. 2008, 29, S455–S464. [Google Scholar] [CrossRef]
- Watson, S.; Igney, C.H.; Dössel, O.; Williams, R.J.; Griffiths, H. A comparison of sensors for minimizing the primary signal in planar-array magnetic induction tomography. Physiol. Meas. 2005, 26, S319–S331. [Google Scholar] [CrossRef] [PubMed]
- Zolgharni, M.; Ledger, P.D.; Armitage, D.W.; Holder, D.S.; Griffiths, H. Imaging cerebral haemorrhage with magnetic induction tomography: Numerical modelling. Physiol. Meas. 2009, 30, S187–S200. [Google Scholar] [CrossRef] [PubMed]
- Lei, J.; Liu, S.; Li, Z.; Schlaberg, H.I.; Sun, M. An image reconstruction algorithm based on new objective functional for electrical capacitance tomography. Meas. Sci. Technol. 2008, 19, 015505. [Google Scholar] [CrossRef]
- Yang, W.Q.; Peng, L. Image reconstruction algorithms for electrical capacitance tomography. Meas. Sci. Technol. 2003, 14, R1–R13. [Google Scholar] [CrossRef]
- Ma, L.; Banasiak, R.; Soleimani, M. Magnetic induction tomography with high performance GPU implementation. Prog. Electromagn. Res. B 2016, 65, 49–63. [Google Scholar] [CrossRef] [Green Version]
- Good, R.H. Elliptic integrals, the forgotten functions. Eur. J. Phys. 2001, 22, 119–126. [Google Scholar] [CrossRef]










| Total Signal (max. Amplitude) | Edge Diff. Signal (Max. Amplitude) | Center Diff. Signal (Max. Amplitude) | Edge Sensitivity 1 | Center Sensitivity 1 (CAS) |
|---|---|---|---|---|
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
| Total Signal (max. amplitude) | Edge Diff. Signal (max. amplitude) | Center Diff. Signal (max. amplitude) | Edge Sensitivity 1 | CAS 1 |
|---|---|---|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klein, M.; Erni, D.; Rueter, D. Three-dimensional Magnetic Induction Tomography: Improved Performance for the Center Regions inside a Low Conductive and Voluminous Body. Sensors 2020, 20, 1306. https://doi.org/10.3390/s20051306
Klein M, Erni D, Rueter D. Three-dimensional Magnetic Induction Tomography: Improved Performance for the Center Regions inside a Low Conductive and Voluminous Body. Sensors. 2020; 20(5):1306. https://doi.org/10.3390/s20051306
Chicago/Turabian StyleKlein, Martin, Daniel Erni, and Dirk Rueter. 2020. "Three-dimensional Magnetic Induction Tomography: Improved Performance for the Center Regions inside a Low Conductive and Voluminous Body" Sensors 20, no. 5: 1306. https://doi.org/10.3390/s20051306
APA StyleKlein, M., Erni, D., & Rueter, D. (2020). Three-dimensional Magnetic Induction Tomography: Improved Performance for the Center Regions inside a Low Conductive and Voluminous Body. Sensors, 20(5), 1306. https://doi.org/10.3390/s20051306





