Non-Contact Damage Detection under Operational Conditions with Multipoint Laservibrometry
Abstract
1. Introduction
2. Methods
2.1. Damage Index
- Step1: Measure the velocities at the measurement points on the intact and damaged structure by the MPV. These are the time response vectors and .
- Step 2: Calculate the spectral density matrices and of the time response vectors and . Use Equation (4) to calculate the singular value spectrum of the intact structure , the damaged structure , and their corresponding singular vectors and .
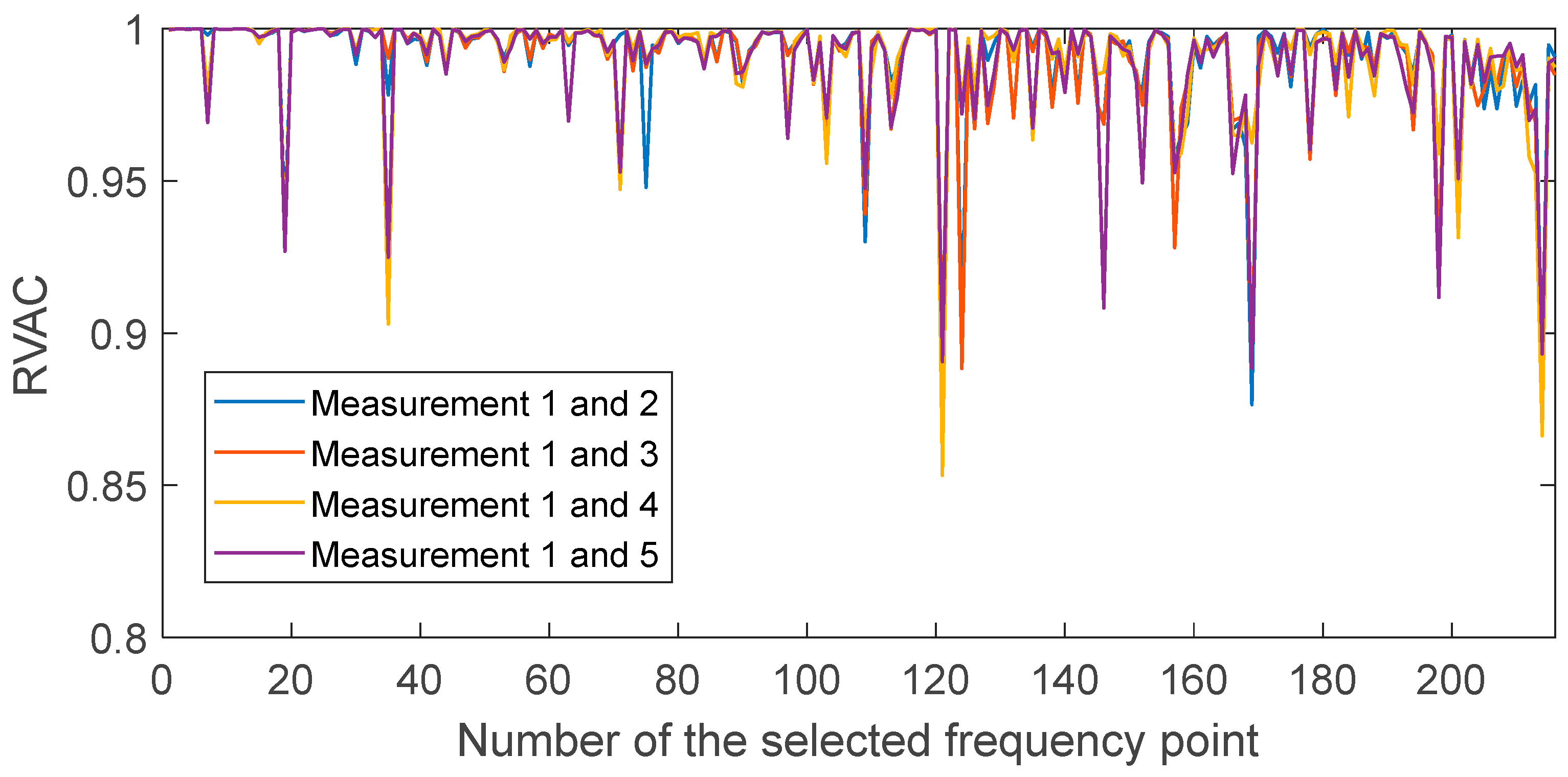
- Step 3: Find the peaks in the singular value spectrum and their corresponding frequency points . Then, calculate the RVAC values at each frequency point by using and instead of and in Equation (1):
- Step 4: Calculate the DRQ value by using Equation (3).
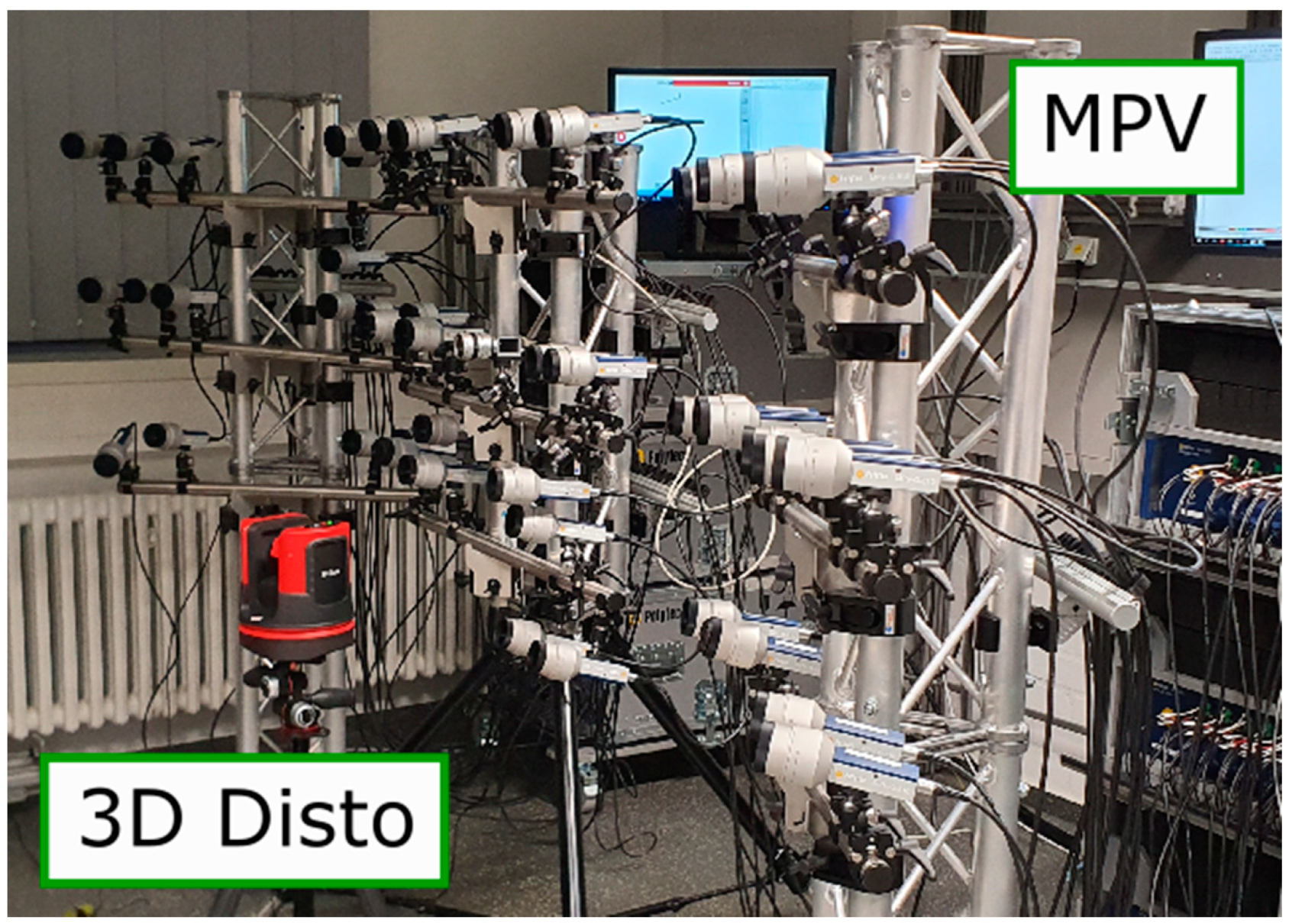
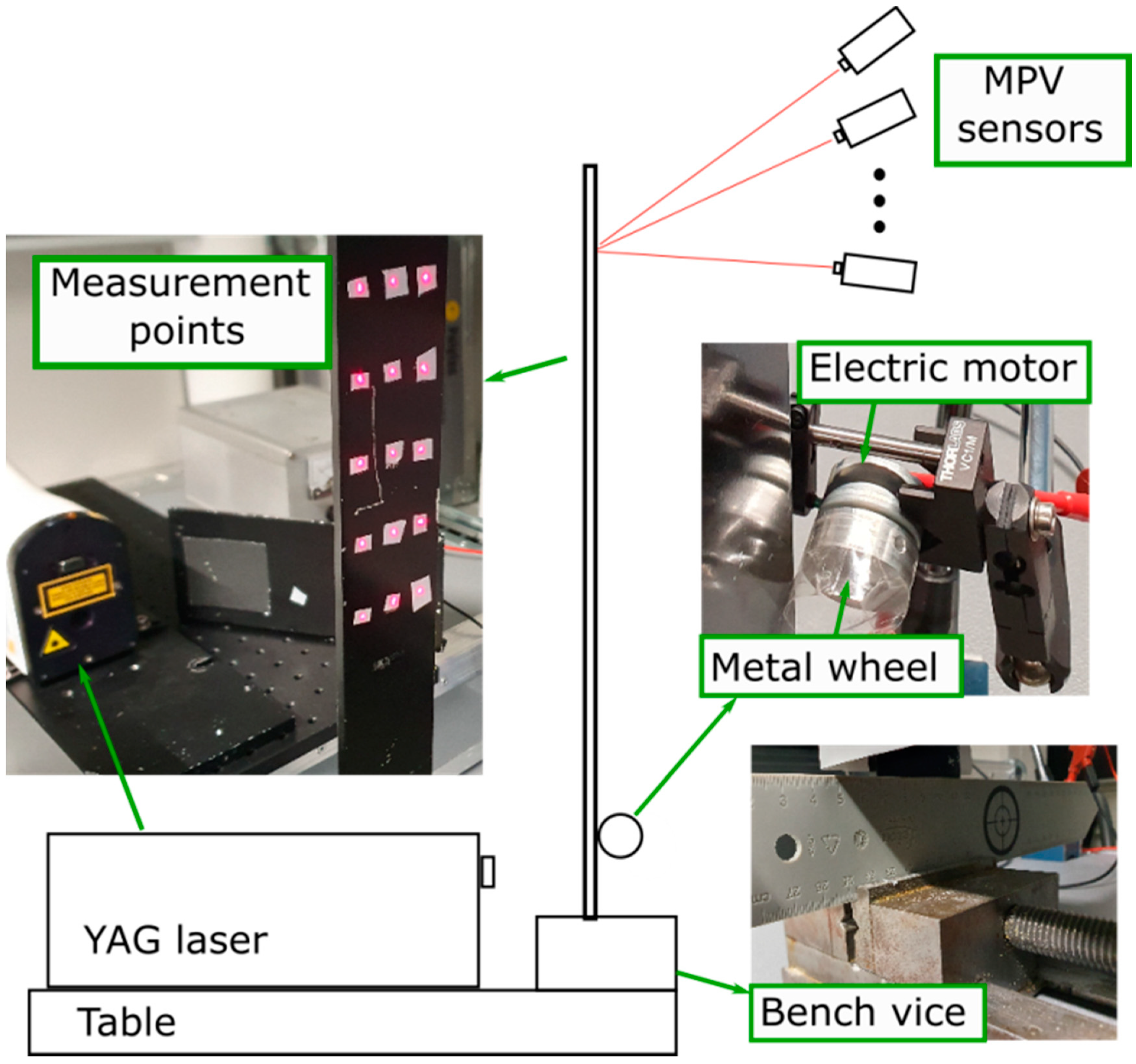
2.2. Measurement Setup
2.3. Experiment
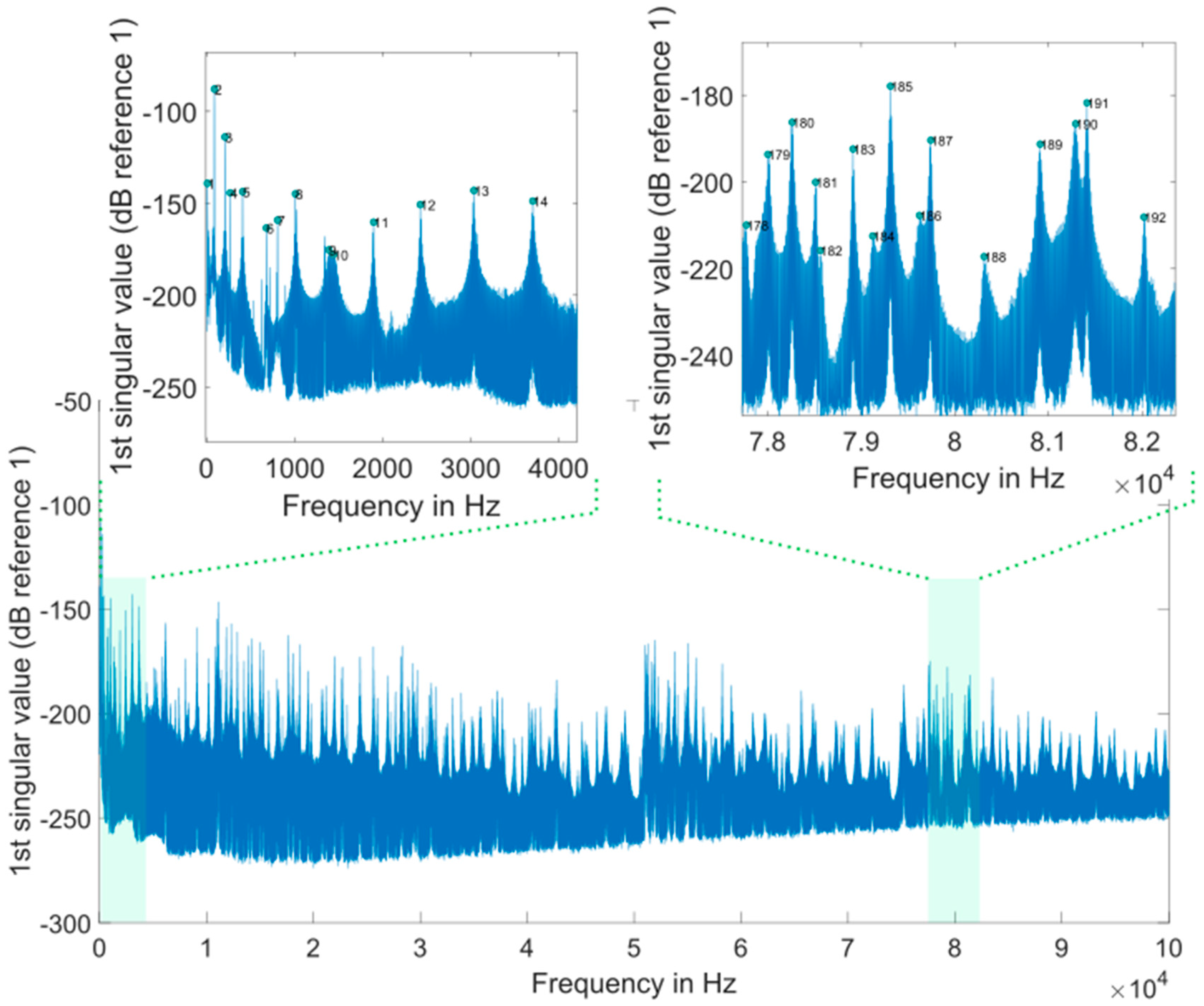
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Doebling, S.W.; Farrar, C.R. Summary Review of Vibration-Based Damage Identification Methods. Shock Vib. Digest 1998, 30, 91–105. [Google Scholar] [CrossRef]
- Carden, E.P.; Fanning, P. Vibration Based Condition Monitoring: A Review. Struct. Health Monit. 2004, 3, 355–377. [Google Scholar] [CrossRef]
- Fan, W.; Qiao, P. Vibration-based Damage Identification Methods: A Review and Comparative Study. Struct. Health Monit. 2011, 10, 83–111. [Google Scholar] [CrossRef]
- Vanlanduit, S.; Parloo, E.; Cauberghe, B.; Guillaume, P.; Verboven, P. A robust singular value decomposition for damage detection under changing operating conditions and structural uncertainties. J. Sound Vib. 2005, 284, 1033–1050. [Google Scholar] [CrossRef]
- Cao, S.; Ouyang, H. Robust structural damage detection and localization based on joint approximate diagonalization technique in frequency domain. Smart Mate. Struct. 2016, 26, 015005. [Google Scholar] [CrossRef]
- Cao, S.; Ouyang, H. Robust multi-damage localisation using common eigenvector analysis and covariance matrix changes. Mech. Syst. Sig. Process. 2018, 111, 663–677. [Google Scholar] [CrossRef]
- Serridge, M.; Licht, T. Piezoelectric Accelerometer and Vibration Preamplifier Handbook, 1st ed.; K. Larsen & Son A/S: Glostrup, Denmark, 1987. [Google Scholar]
- Elies, S. Performance Analysis of Commercial Accelerometers: A Parameter Review. Sens. Transducers 2015, 193, 179–190. [Google Scholar]
- Cao, X.; Tarigan, B.; Rembe, C.; Schwarze, H. Vibrationsbasierende White-Etching-Cracks-Detektion an Wälzlagern. tm-Tech. Mess. 2018, 85, 443–453. [Google Scholar] [CrossRef]
- Scruby, C.; Drain, L. Laser Ultrasonics Techniques and Applications, 1st ed.; CRC Press: New York, NY, USA, 1990. [Google Scholar]
- Vanlanduit, S.; Guillaume, P.; Schoukens, J.; Parloo, E. Linear and nonlinear damage detection using a scanning laser vibrometer. Shock Vib. 2002, 9, 43–56. [Google Scholar] [CrossRef]
- Rothberg, S.J.; Allen, M.S.; Castellini, P.; Di Maio, D.; Dirckx, J.J.J.; Ewins, D.J.; Halkon, B.J.; Muyshondt, P.; Paone, N.; Ryan, T.; et al. An international review of laser Doppler vibrometry: Making light work of vibration measurement. Opt. Lasers Eng. 2017, 99, 11–22. [Google Scholar] [CrossRef]
- Castellini, P.; Martarelli, M.; Tomasini, E.P. Laser Doppler Vibrometry: Development of advanced solutions answering to technology’s needs. Mech. Syst. Sig. Process. 2006, 20, 1265–1285. [Google Scholar] [CrossRef]
- Dräbenstedt, A.; Cao, X.; Polom, U.; Pätzold, F.; Zeller, T.; Hecker, P.; Seyfried, V.; Rembe, C. Mobile seismic exploration. AIP Conf. Proc. 2016, 1740, 030001. [Google Scholar]
- Kowarsch, R.; Rigele, T.; Rembe, C. Laser-Doppler vibrometer microscope with variable heterodyne carrier. J. Phys: Conf. Ser. 2018, 1149, 012016. [Google Scholar] [CrossRef]
- Solodov, I.; Rahammer, M.; Gulnizkij, N. Highly-sensitive and frequency-selective imaging of defects via local defect resonance. In Proceedings of the 11th European Conference on Non-Destructive Testing, Prague, Czech Republic, 6–11 October 2014. [Google Scholar]
- Solodov, I.; KREUTZBRUCK, M. Resonant Defects: A New Approach to Highly-Sensitive Ultrasound-Activated NDT Techniques. In Proceedings of the 19th World Conference on Non-Destructive Testing, Munich, Germany, 13–17 June 2016. [Google Scholar]
- Haist, T.; Lingel, C.; Osten, W.; Winter, M.; Giesen, M.; Ritter, F.; Sandfort, K.; Rembe, C.; Bendel, K. Multipoint vibrometry with dynamic and static holograms. Rev. Sci. Instrum. 2013, 84, 121701. [Google Scholar] [CrossRef] [PubMed]
- MacPherson, W.N.; Reeves, M.; Towers, D.P.; Moore, A.J.; Jones, J.D.C.; Dale, M.; Edwards, C. Multipoint laser vibrometer for modal analysis. Appl. Opt. 2007, 46, 3126–3132. [Google Scholar] [CrossRef]
- Fu, Y.; Guo, M.; Phua, P.B. Acousto-optical signal processing; Interferometry; Nondestructive testing; Vibration analysis; Detector arrays; Laser beams; Laser scattering; Laser sources; Optical components; Optical design. Appl. Opt. 2011, 50, 1280–1288. [Google Scholar] [CrossRef]
- Kajiwara, I.; Hosoya, N. Vibration testing based on impulse response excited by laser ablation. J. Sound Vib. 2011, 330, 5045–5057. [Google Scholar] [CrossRef]
- Hosoya, N.; Kajiwara, I.; Hosokawa, T. Vibration testing based on impulse response excited by pulsed-laser ablation: Measurement of frequency response function with detection-free input. J. Sound Vib. 2012, 331, 1355–1365. [Google Scholar] [CrossRef]
- Philp, W.R.; Booth, D.J.; Perry, N.D. Single pulse laser excitation of structural vibration using power densities below the surface ablation threshold. J. Sound Vib. 1995, 185, 643–654. [Google Scholar] [CrossRef]
- Castellini, P.; Revel, G.M.; Scalise, L.; De Andrade, R.M. Experimental and numerical investigation on structural effects of laser pulses for modal parameter measurement. Opt. Lasers Eng. 1999, 32, 565–581. [Google Scholar] [CrossRef]
- Brincker, R.; Zhang, L.; Andersen, P. Modal identification of output-only systems using frequency domain decomposition. Smart Mater. Struct. 2001, 10, 441–445. [Google Scholar] [CrossRef]
- Maia, N.M.M.; Almeida, R.A.B.; Urgueira, A.P.V.; Sampaio, R.P.C. Damage detection and quantification using transmissibility. Mech. Syst. Sig. Process. 2011, 25, 2475–2483. [Google Scholar] [CrossRef]
- Sampaio, R.P.C.; Maia, N.M.M. Strategies for an efficient indicator of structural damage. Mech. Syst. Sig. Process. 2009, 23, 1855–1869. [Google Scholar] [CrossRef]
- Allemang, R.J. The modal assurance criterion—twenty years of use and abuse. Sound Vib. 2003, 37, 14–23. [Google Scholar]
- Mottershead, J.E.; Friswell, M.I. Model Updating In Structural Dynamics: A Survey. J. Sound Vib. 1993, 167, 347–375. [Google Scholar] [CrossRef]
- Arora, V.; Singh, S.P.; Kundra, T.K. Finite element model updating with damping identification. J. Sound Vib. 2009, 324, 1111–1123. [Google Scholar] [CrossRef]
- Jull, M.Ø.Ø.; Amador, S.D.R.; Skafte, A.; Hansen, J.B.; Aenlle, M.L.; Brincker, R. One-Step FE Model Updating Using Local Correspondence and Mode Shape Orthogonality. Shock Vib. 2019, 2019, 12. [Google Scholar] [CrossRef]
- Cao, X.; Backhaus, S.G.; Scheidl, R.; Rembe, C. Numerical and experimental analysis of structure-borne sound transmission in coupled systems. AIP Conf. Proc. 2016, 1740, 070004. [Google Scholar]
- Brincker, R.; Ventura, C.E. Introduction to Operational Modal Analysis, 1st ed.; WILEY: Chichester, UK, 2015. [Google Scholar]
- Rembe, C.; Wörtge, M.; Dräbenstedt, A.; Braun, T. Optisches Interferometer und Vibrometer mit solch einem optischen Interferometer. German. Patent DE102013209833A1, 27 November 2014. [Google Scholar]
- O’Malley, P.; Woods, T.; Judge, J.; Vignola, J. Five-axis scanning laser vibrometry for three-dimensional measurements of non-planar surfaces. Meas. Sci. Technol. 2009, 20, 115901. [Google Scholar] [CrossRef]
- Kim, D.; Song, H.; Khalil, H.; Lee, J.; Wang, S.; Park, K. 3-D Vibration Measurement Using a Single Laser Scanning Vibrometer by Moving to Three Different Locations. IEEE Trans. Instrume. Meas. 2014, 63, 2028–2033. [Google Scholar] [CrossRef]
- Briggs, C.H. Laserscanner oder Lasernachführungsgerät mit einem Projektor. German Patent DE112011102995B4, 19 May 2016. [Google Scholar]
- Watkins, D.S. Matrix Computations, 2nd ed.; WILEY-INTERSCIENCE: New York, NY, USA, 2002. [Google Scholar]
- Khalil, H.; Kim, D.; Nam, J.; Park, K. Accuracy and noise analyses of 3D vibration measurements using laser Doppler vibrometer. Measurement 2016, 94, 883–892. [Google Scholar] [CrossRef]
- Bendel, K.; Fischer, M.; Schuessler, M. Vibrational analysis of power tools using a novel three-dimensional scanning vibrometer. Vibrational analysis of power tools using a novel three-dimensional scanning vibrometer. Proc. SPIE 2004, 5503. [Google Scholar] [CrossRef]
- Jailin, C. Full field modal measurement with a single standard camera. Opt. Lasers Eng. 2018, 107, 265–272. [Google Scholar] [CrossRef]
- Cremer, H.; Heckl, M.; Petersson, B.A.T. Structure-Borne Sound, 3rd ed.; Springer: Berlin, Germany, 2005. [Google Scholar]
- Blevins, R.D. Formulas for Dynamics, Acoustics and Vibration, 1st ed.; John Wiley & Sons Ltd.: West Sussex, UK, 2016. [Google Scholar]
- Cao, S.; Ouyang, H.; Cheng, L. Baseline-free adaptive damage localization of plate-type structures by using robust PCA and Gaussian smoothing. Mech. Syst. Sig. Process. 2019, 122, 232–246. [Google Scholar] [CrossRef]
- Dessi, D.; Camerlengo, G. Damage identification techniques via modal curvature analysis: Overview and comparison. Mech. Syst. Sig. Process. 2015, 52–53, 181–205. [Google Scholar] [CrossRef]
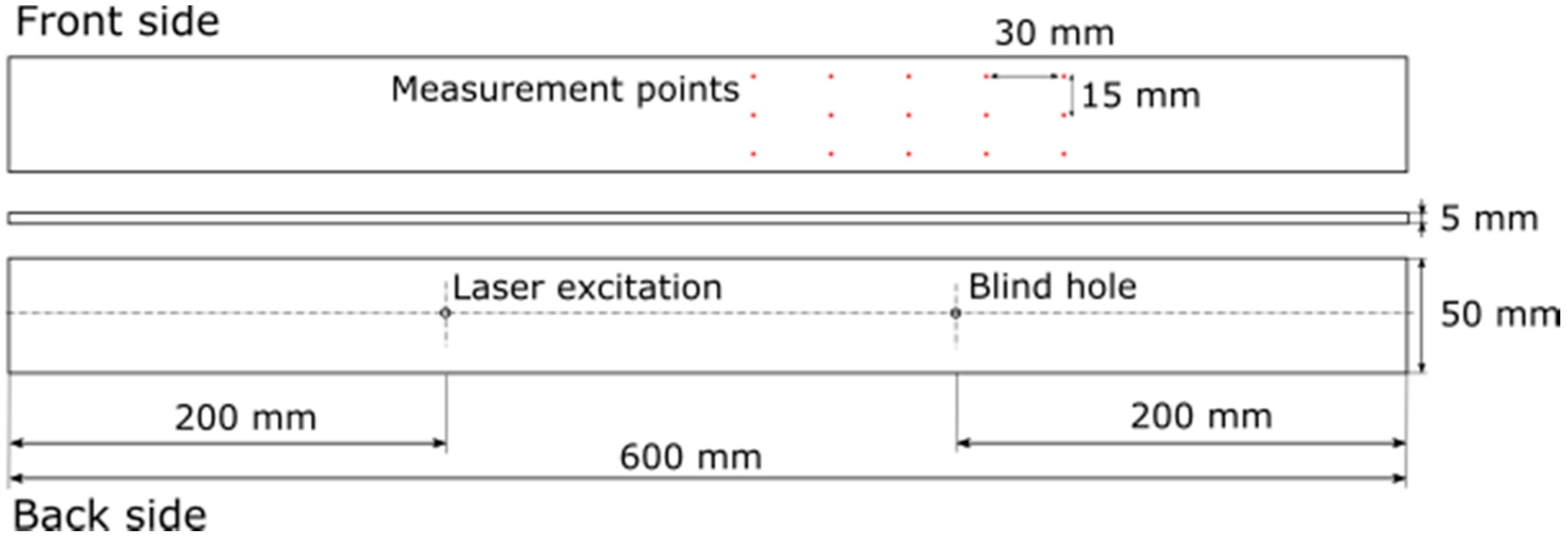





| Damage Level 1 | Damage Level 2 | Damage Level 3 | |
|---|---|---|---|
| Diameter of the blind hole in mm | 0 | 4.30 | 4.30 |
| Depth in mm | 0 | 2.66 | 4.09 |
| Measurements 1 and 2 | Measurements 1 and 3 | Measurements 1 and 4 | Measurements 1 and 5 |
|---|---|---|---|
| 0.9904 | 0.9896 | 0.9905 | 0.9894 |
| Frequency Range in kHz | 0–35 | 35–70 | 70–100 |
| Number of Resonance Frequency Point | 1–70 | 71–120 | 121–143 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, X.; Rembe, C. Non-Contact Damage Detection under Operational Conditions with Multipoint Laservibrometry. Sensors 2020, 20, 732. https://doi.org/10.3390/s20030732
Cao X, Rembe C. Non-Contact Damage Detection under Operational Conditions with Multipoint Laservibrometry. Sensors. 2020; 20(3):732. https://doi.org/10.3390/s20030732
Chicago/Turabian StyleCao, Xiaodong, and Christian Rembe. 2020. "Non-Contact Damage Detection under Operational Conditions with Multipoint Laservibrometry" Sensors 20, no. 3: 732. https://doi.org/10.3390/s20030732
APA StyleCao, X., & Rembe, C. (2020). Non-Contact Damage Detection under Operational Conditions with Multipoint Laservibrometry. Sensors, 20(3), 732. https://doi.org/10.3390/s20030732




