A Novel Piecewise Frequency Control Strategy Based on Fractional-Order Filter for Coordinating Vibration Isolation and Positioning of Supporting System
Abstract
:1. Introduction
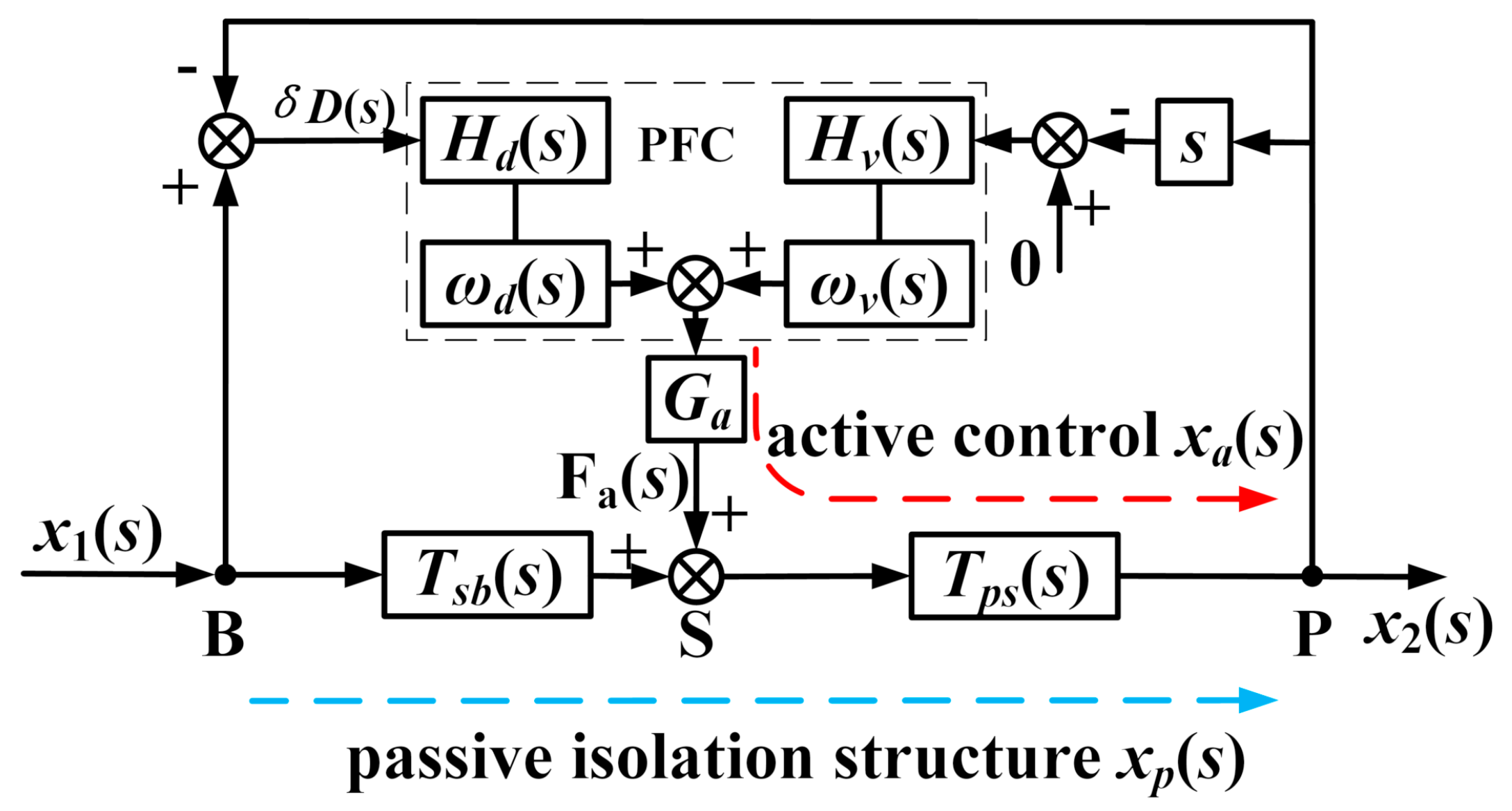
2. Description of PFC Strategy for VIP
3. Effects of AVI and Positioning Controls
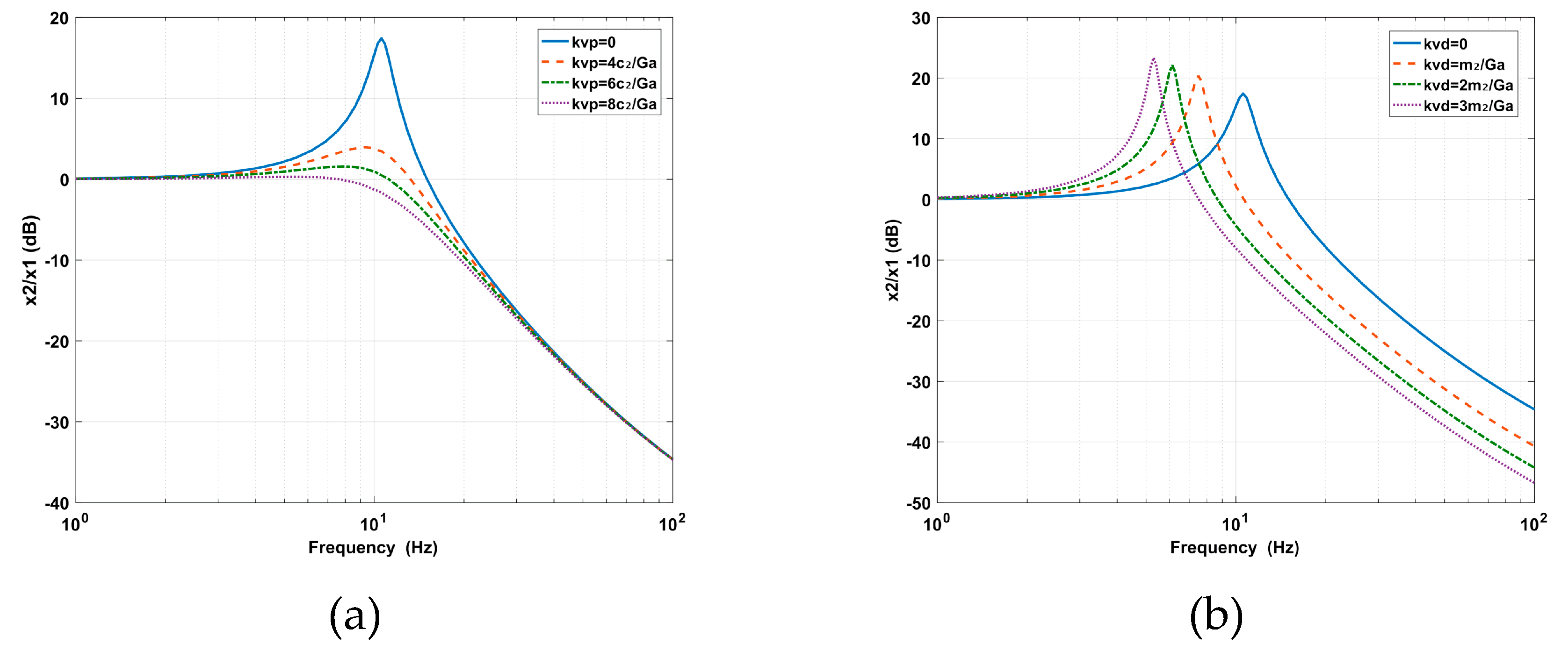
3.1. Effect of AVF on AVI
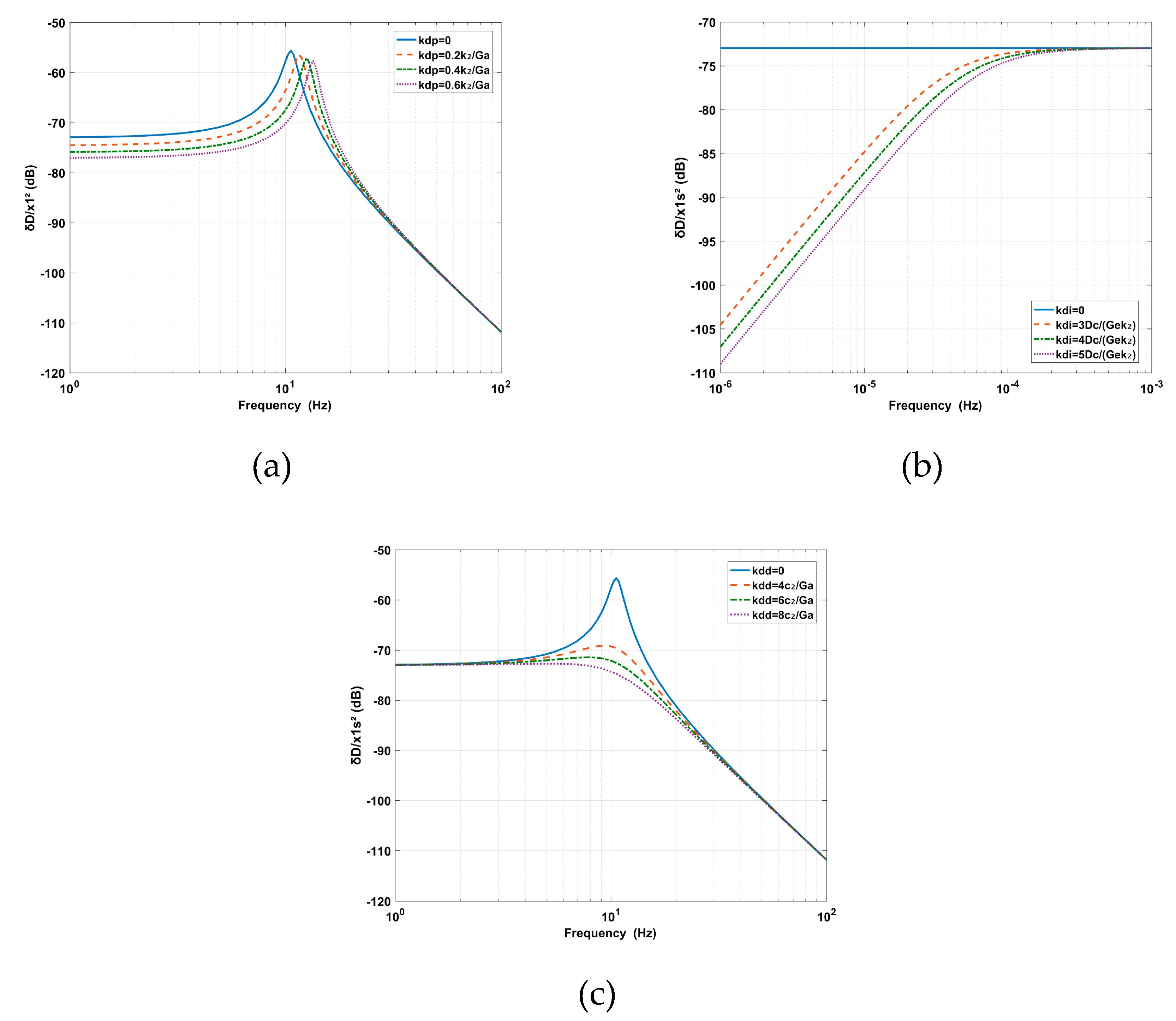
3.2. Effect of RPF on Positioning
3.3. Interaction of AVI and Positioning
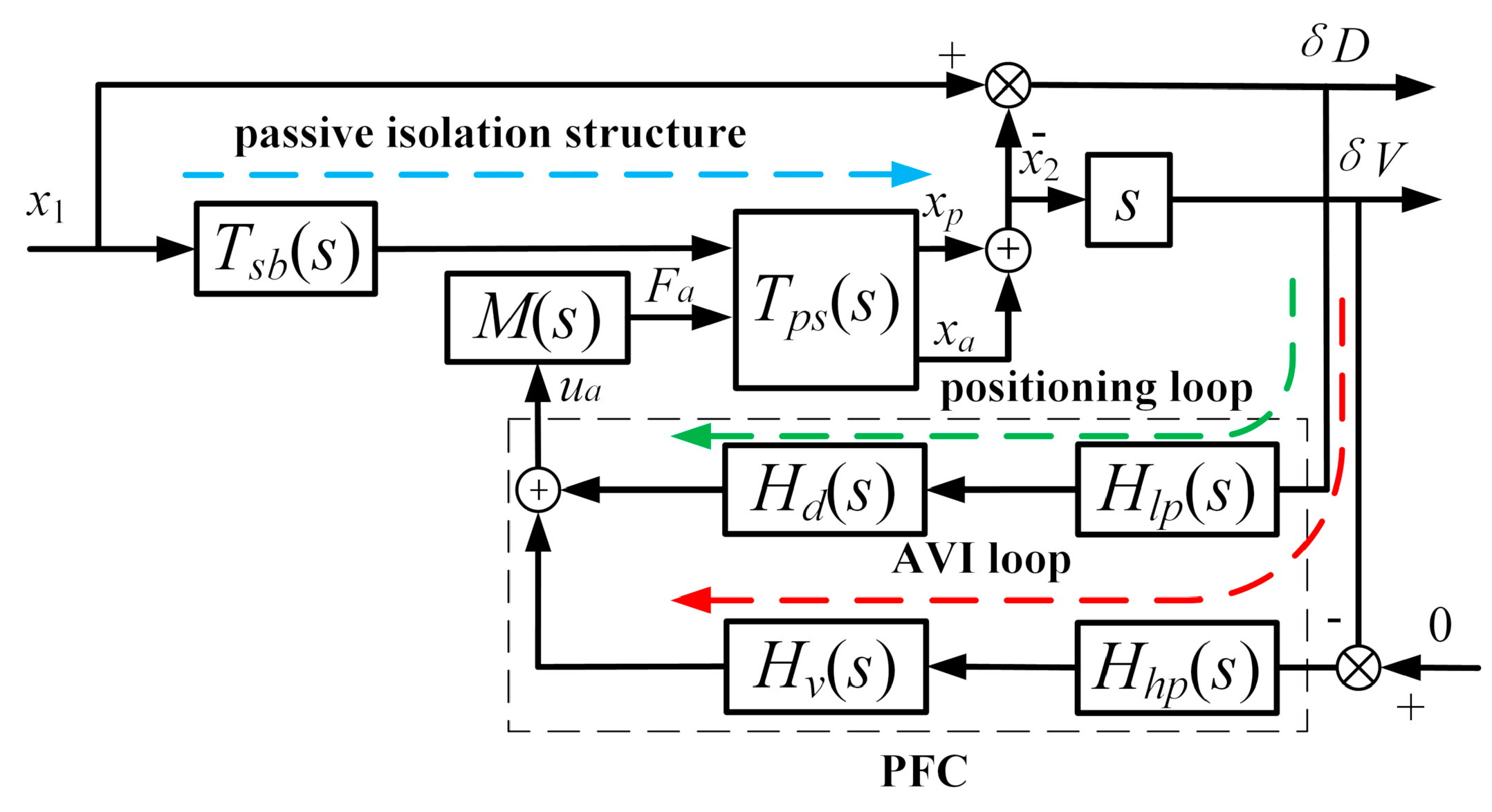
4. Analysis and Optimization of PFC
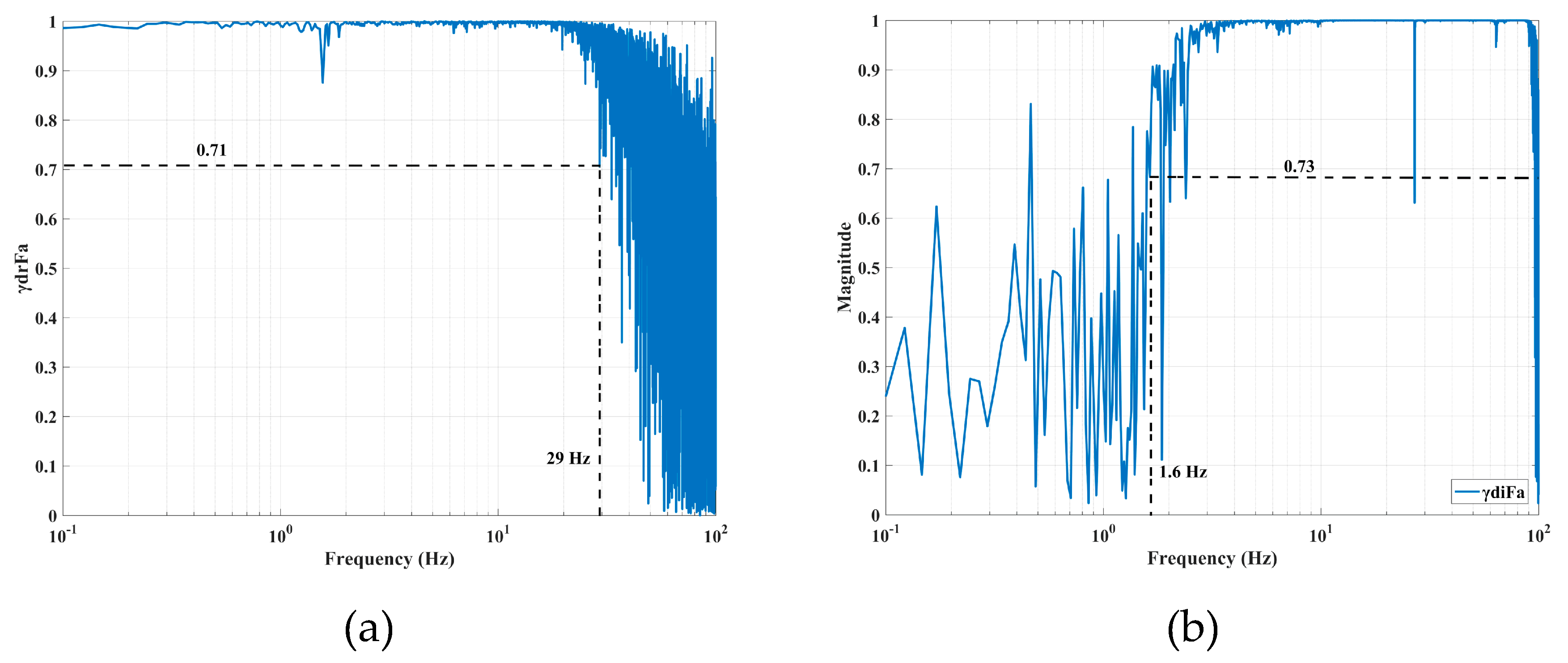
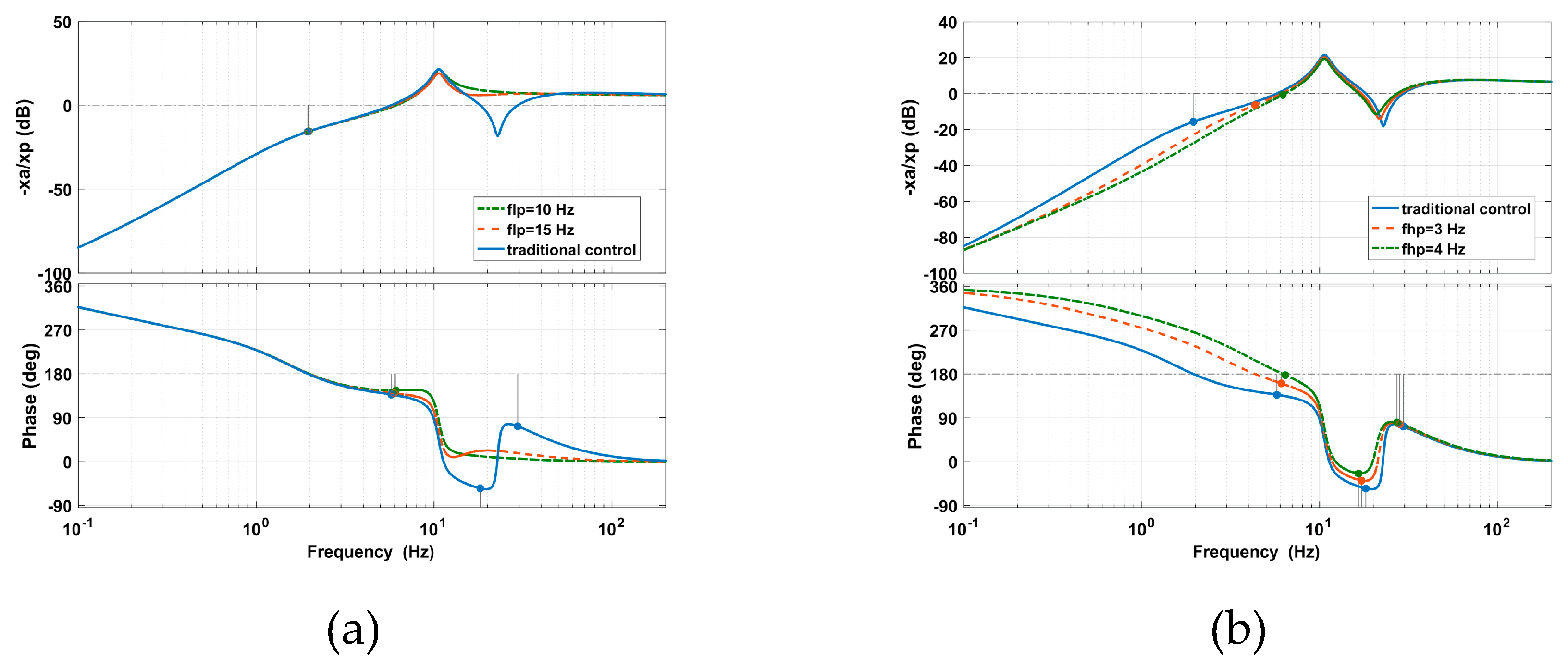
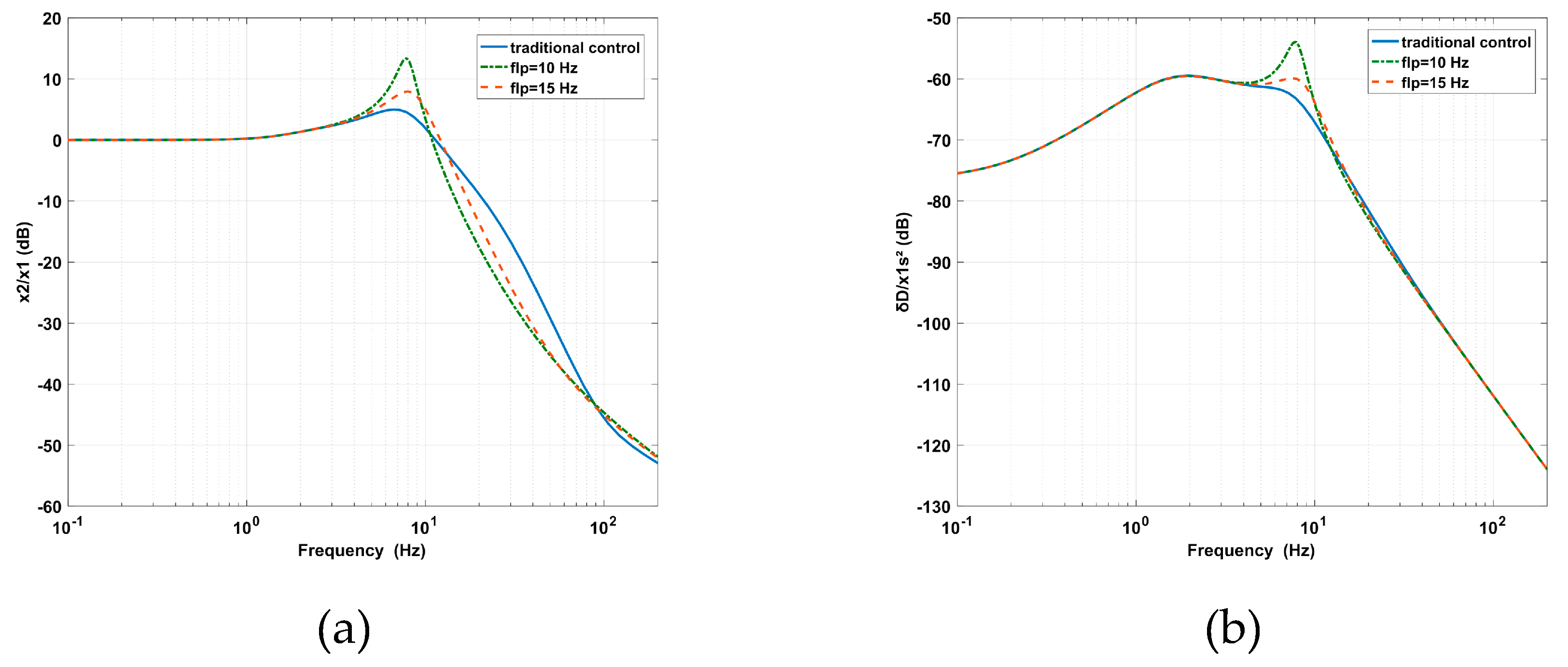
4.1. PFC Based on IOFs
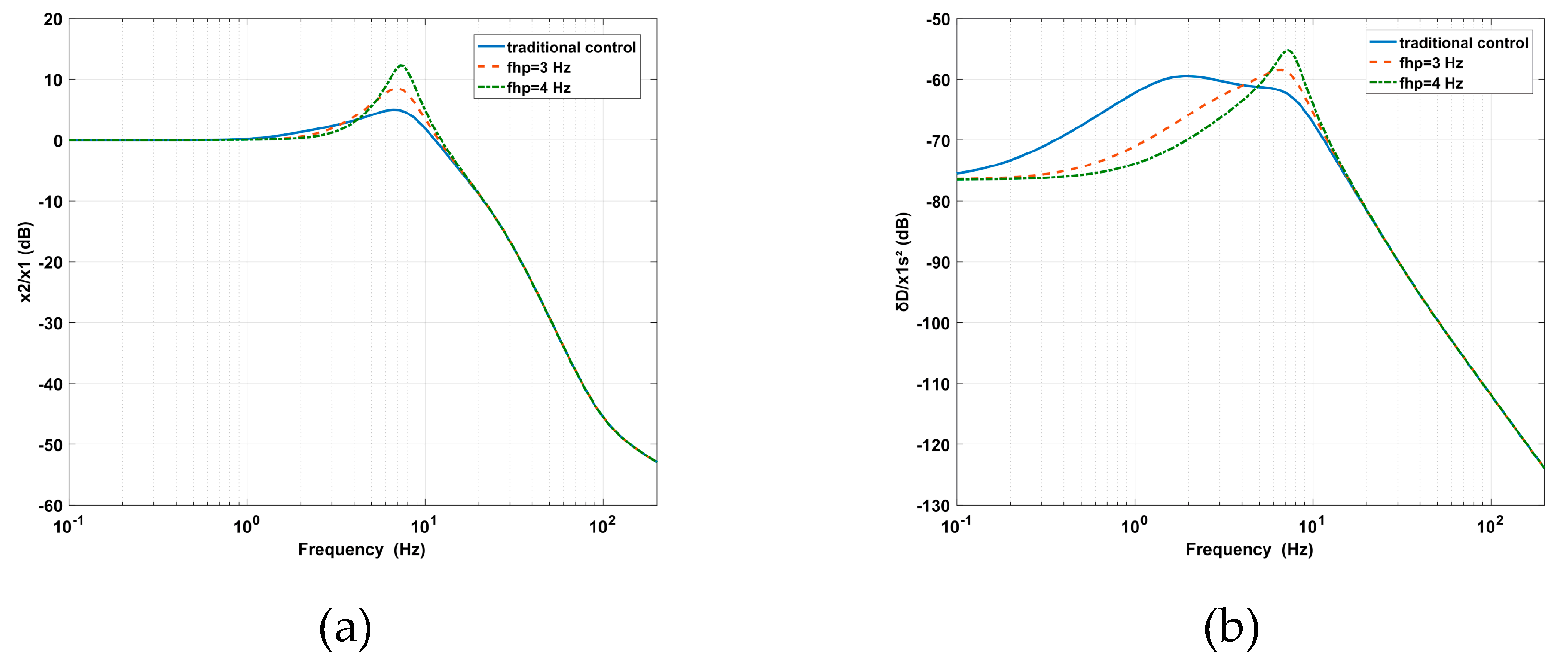
4.2. PFC Based on FOFs
- (1)
- Phase margin = PM ≥ 40°;
- (2)
- Gain margin = GM ≥ 15 dB;
- (3)
- Vibration transmissibility: max() ≤ 5 dB and the amplitude crossover frequency is close to 10 Hz;
- (4)
- Positioning transmissibility: max() ≤ −75 dB when the frequency is smaller than 2 Hz, otherwise max () ≤ −60 dB.
5. Experimental Verification
5.1. Experimental Setup
5.2. Experimental Results
5.3. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ito, S.; Schitter, G. Comparison and classification of high-precision actuators based on stiffness influencing vibration isolation. IEEE-Asme Trans. Mech. 2015, 21, 1169–1178. [Google Scholar] [CrossRef]
- Butler, H. Position control in lithographic equipment. IEEE Contr. Syst. Mag. 2011, 31, 28–47. [Google Scholar] [CrossRef]
- Kienholz, D.A. Active alignment and vibration control system for a large airborne optical system. In Proceedings of the SPIE-The International Society for Optical Engineering, Newport Beach, CA, USA, 6–8 March 2000; pp. 464–472. [Google Scholar] [CrossRef]
- Heertjes, M.; de Graaff, K.; van der Toorn, J.G. Active vibration isolation of metrology frames; a modal decoupled control design. J. Vib. Acoust. 2005, 127, 223–233. [Google Scholar] [CrossRef]
- Tjepkema, D.; Dijk, J.V.; Soemers, H.M.J.R. Sensor fusion for active vibration isolation in precision equipment. J. Sound. Vib. 2012, 331, 735–749. [Google Scholar] [CrossRef]
- Gong, Z.P.; Ding, L.; Yue, H.H.; Gao, H.B.; Liu, R.Q.; Deng, Z.Q.; Lu, Y.F. System integration and control design of a maglev platform for space vibration isolation. J. Vib. Control 2019, 25, 1720–1736. [Google Scholar] [CrossRef]
- Ma, K.; Ghasemi-Nejhad, M.N. Frequency-weighted adaptive control for simultaneous precision positioning and vibration suppression of smart structures. Smart Mater Struct. 2004, 13, 1143–1154. [Google Scholar] [CrossRef]
- van Dijk, J. Mechatronic design of hard-mount concepts for precision equipment. In Motion and Vibration Control; Springer: Dordrecht, The Netherlands, 2009; pp. 315–324. [Google Scholar]
- Pu, H.Y.; Li, J.M.; Wang, M.; Huang, Y.N.; Sun, Y. Optimum design of an eddy current damper considering the magnetic congregate effect. J. Phys. D Appl. Phys. 2019, 53, 115002. [Google Scholar]
- Pu, H.Y.; Yuan, S.J.; Peng, Y.; Meng, K.; Zhao, J.L.; Xie, R.Q.; Huang, Y.N.; Sun, Y.; Yang, Y.; Xie, S.R.; et al. Multi-layer electromagnetic spring with tunable negative stiffness for semi-active vibration isolation. Mech. Syst. Signal Pr. 2019, 121, 942–960. [Google Scholar]
- Hanieh, A.A. Active isolation and damping of vibrations via Stewart platform. Ph.D. Thesis, Universit’e Libre de Bruxelles, Brussels, Belgium, 2003. [Google Scholar]
- van der Poel, G.W. An exploration of active hard mount vibration isolation for precision equipment. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2010. [Google Scholar]
- Liao, F.H.; Li, X.P.; Yuan, Z.Y. Self-tuning of position feedback and velocity feedback of active vibration isolation system with 6 dofs. Mechanika 2012, 17, 630–636. [Google Scholar] [CrossRef]
- Beijen, M.A.; Heertjes, M.F.; Butler, H.; Steinbuch, M. Practical tuning guide to mixed feedback and feedforward control of soft-mounted vibration isolators. IFAC-PapersOnLine 2016, 49, 163–169. [Google Scholar] [CrossRef]
- Zhang, Y.K.; Dong, W.B.; Liu, W.; Li, Z.F.; Lv, S.M.; Sang, X.R.; Yang, Y. Verification of the microgravity active vibration isolation system based on parabolic flight. Microgravity Sci. Technol. 2017, 29, 415–426. [Google Scholar] [CrossRef]
- Jackson, M.; Kim, Y.; Whorton, M. Design and Analysis of the g-LIMIT Baseline Vibration Isolation Control System. In Proceedings of the AiAA Guidance, Navigation, & Control Conference & Exhibit, Monterey, CA, USA, 5–8 August 2002; p. 5019. [Google Scholar] [CrossRef]
- Yang, X.L.; Wu, H.T.; Chen, B.; Kang, S.Z.; Cheng, S.L. Dynamic modeling and decoupled control of a flexible stewart platform for vibration isolation. J. Sound Vib. 2019, 439, 398–412. [Google Scholar] [CrossRef]
- Yun, Y.; Li, Y. Modeling and control analysis of a 3-pupu dual compliant parallel manipulator for micro positioning and active vibration isolation. J. Dyn. Syst-T. Asme. 2012, 134, 021001. [Google Scholar] [CrossRef]
- Zhu, W.H.; Tryggvason, B.; Piedboeuf, J.C. On active acceleration control of vibration isolation systems. Control Eng. Pract. 2006, 14, 863–873. [Google Scholar] [CrossRef]
- Matichard, F.; Lantz, B.; Mason, K.; Mittleman, R.; Abbott, B.; Abbott, S.; Allwine, E.; Barnum, S.; Birch, J.; Biscans, S.; et al. Advanced LIGO two-stage twelve-axis vibration isolation and positioning platform. Part 1: Design and production overview. Precis. Eng. 2015, 40, 273–286. [Google Scholar]
- Thayer, D.; Campbell, M.; Flotow, A.V.; Vagners, J. Six-axis vibration isolation system using soft actuators and multiple sensors. J. Spacecr. Rocket. 2012, 39, 206–212. [Google Scholar] [CrossRef]
- Coopmans, C.; Jensen, A.M.; Chen, Y.Q. Fractional-order complementary filters for small unmanned aerial system navigation. J. Intell. Robot Syst. 2014, 73, 429–453. [Google Scholar] [CrossRef]
- Luo, Y.; Zhang, T.; Zhou, L.; Chen, Y.Q. Pre-filtering and head-dependent adaptive feed-forward compensation for translation vibration in hard-disc-drive. Mechatronics 2015, 27, 13–19. [Google Scholar] [CrossRef]
- Charef, M.; Charef, A. Fractional order controller based on the fractionalization of PID controller. In Proceedings of the 2017 5th International Conference on Electrical Engineering-Boumerdes (ICEE-B), Boumerdes, Algeria, 29–31 October 2017; pp. 1–5. [Google Scholar] [CrossRef]
- Zheng, W.J.; Luo, Y.; Wang, X.H.; Pi, Y.G.; Chen, Y.Q. Fractional order piλdμ controller design for satisfying time and frequency domain specifications simultaneously. ISA Trans. 2017, 68, 212–222. [Google Scholar] [CrossRef]
- Fleming, A.J. Nanopositioning system with force feedback for high-performance tracking and vibration control. IEEE-Asme. T. Mech. 2010, 15, 433–447. [Google Scholar] [CrossRef] [Green Version]
- Skogestad, S.; Postlethwaite, I. Multivariable Feedback Control: Analysis and Design; John Wiley & Sons: New York, NY, USA, 2001. [Google Scholar]
- Soltan, A.; Radwan, A.G.; Soliman, A.M. Fractional order filter with two fractional elements of dependent orders. Microelectron. J. 2012, 43, 818–827. [Google Scholar] [CrossRef]
- Radwan, A.G.; Elwakil, A.S.; Soliman, A.M. On the generalization of second-order filters to the fractional-order domain. J. Circuit Syst. Comp. 2009, 18, 361–386. [Google Scholar] [CrossRef]
- Ma, C.; Hori, Y. Fractional-order control: Theory and applications in motion control [past and present]. IEEE Ind. Electron. Mag. 2007, 1, 6–16. [Google Scholar] [CrossRef]
- Oustaloup, A.; Levron, F.; Mathieu, B.; Nanot, F.M. Frequency-band complex noninteger differentiator: Characterization and synthesis. IEEE Trans. Circuits Syst. I 2002, 47, 25–39. [Google Scholar] [CrossRef]







| Symbol | Property | Value |
|---|---|---|
| m1 | Mass of payload | 2 kg |
| m2 | Mass of base | 2.2 kg |
| k2 | Stiffness constant | 9.59 KN/m |
| c2 | Damping coefficient | 20 Ns/m |
| f | Inherent frequency | 10.5 Hz |
| Ga | Voice coil motor (VCM) gain | 3.17 N/V |
| Ge | Eddy-current sensor gain | 0.421 mm/V |
| Cut-Off Frequency | Gain Margin and Phase Crossover Frequency | Phase Margin and Gain Crossover Frequency |
|---|---|---|
| flp = 10 Hz & fhp = 1.6 Hz | 15.5 dB @ 1.98 Hz | 34.2° @ 6.11 Hz |
| flp = 15 Hz & fhp = 1.6 Hz | 15.6 dB @ 1.96 Hz | 40.3° @ 5.96 Hz |
| flp = 29 Hz & fhp = 1.6 Hz (Traditional control) | 15.7 dB @ 1.95 Hz | 42.6° @ 5.74 Hz 125° @ 18.2 Hz 108° @ 29.5 Hz |
| flp = 29 Hz & fhp = 3 Hz | 6.42 dB @ 4.33 Hz | 19.5° @ 6.1 Hz 141° @ 17.2 Hz 103° @ 28.2 Hz |
| flp = 29 Hz & fhp = 4 Hz | 0.734 dB @ 6.21 Hz | 2.33° @ 6.41 Hz 156° @ 16.5 Hz 99.6° @ 27.2 Hz |
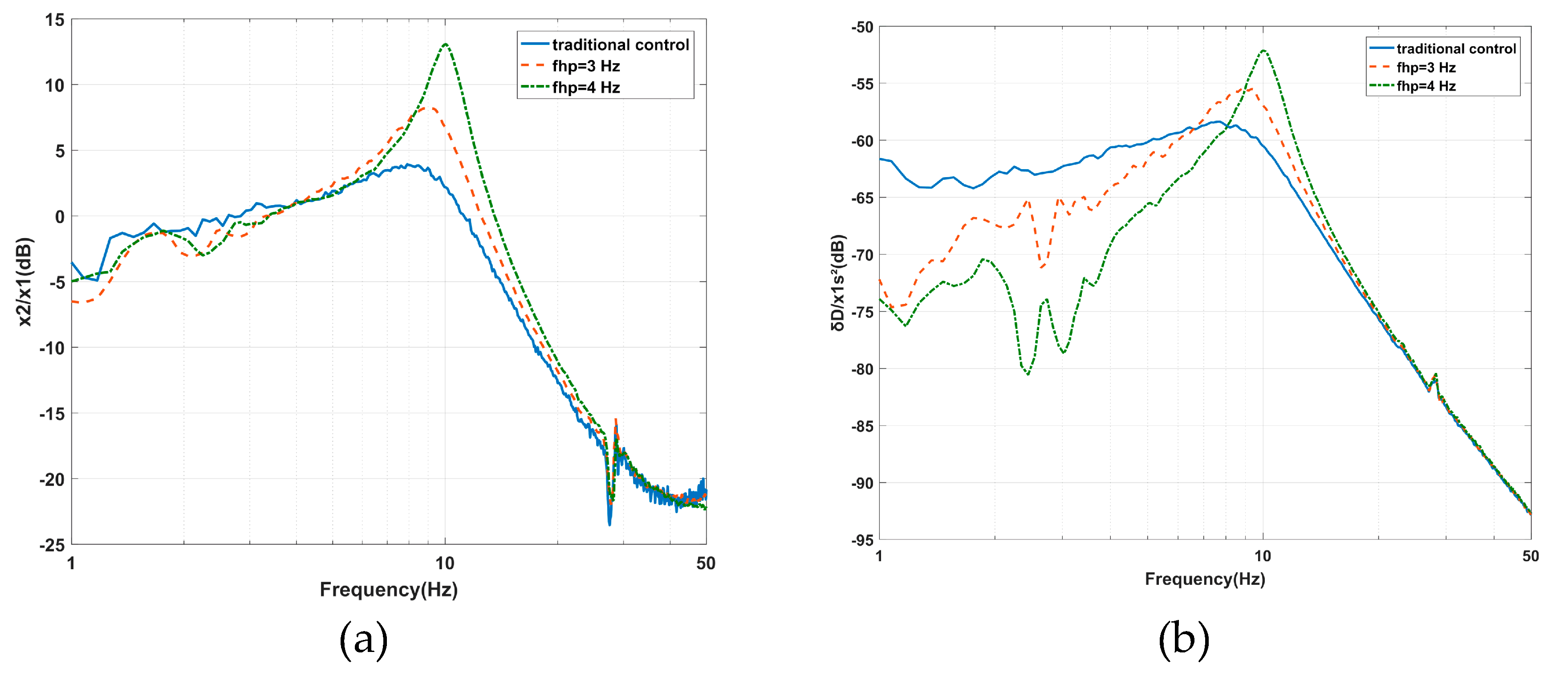
| Cut-Off Frequency | AVI Performance | Positioning Performance |
|---|---|---|
| flp = 10 Hz & fhp = 1.6 Hz | Maximum value: 10.84 dB @ 7.03 Hz Cross-frequency: 9.57 Hz | Maximum value: −48.7 dB @ 7.22 Hz |
| flp = 15 Hz & fhp = 1.6 Hz | Maximum value: 8.89 dB @ 7.52 Hz Cross-frequency: 10.16 Hz | Maximum value: −51.41 dB @ 7.52 Hz |
| flp = 29 Hz & fhp = 1.6 Hz (Traditional control) | Maximum value: 3.93 dB @ 7.91 Hz Cross-frequency: 11.23 Hz | Maximum value: −58.37 dB @ 7.72 Hz |
| flp = 29 Hz & fhp = 3 Hz | Maximum value: 8.98 dB @ 8.24 Hz Cross-frequency: 12.5 Hz | Maximum value: −55.36 dB @ 8.89 Hz |
| flp = 29 Hz & fhp = 4 Hz | Maximum value: 10.06 dB @ 13.07 Hz Cross-frequency: 13.57 Hz | Maximum value: −52.15 dB @ 10.06 Hz |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tao, Y.; Jiang, W.; Han, B.; Li, X.; Luo, Y.; Zeng, L. A Novel Piecewise Frequency Control Strategy Based on Fractional-Order Filter for Coordinating Vibration Isolation and Positioning of Supporting System. Sensors 2020, 20, 5307. https://doi.org/10.3390/s20185307
Tao Y, Jiang W, Han B, Li X, Luo Y, Zeng L. A Novel Piecewise Frequency Control Strategy Based on Fractional-Order Filter for Coordinating Vibration Isolation and Positioning of Supporting System. Sensors. 2020; 20(18):5307. https://doi.org/10.3390/s20185307
Chicago/Turabian StyleTao, Yeying, Wei Jiang, Bin Han, Xiaoqing Li, Ying Luo, and Lizhan Zeng. 2020. "A Novel Piecewise Frequency Control Strategy Based on Fractional-Order Filter for Coordinating Vibration Isolation and Positioning of Supporting System" Sensors 20, no. 18: 5307. https://doi.org/10.3390/s20185307
APA StyleTao, Y., Jiang, W., Han, B., Li, X., Luo, Y., & Zeng, L. (2020). A Novel Piecewise Frequency Control Strategy Based on Fractional-Order Filter for Coordinating Vibration Isolation and Positioning of Supporting System. Sensors, 20(18), 5307. https://doi.org/10.3390/s20185307





