1. Introduction
Carbon Fiber Reinforced Polymers (CFRP) composites have a wide range of applications in the automobile and aerospace industries due to their superior mechanical properties combined with reduced density and good resistance to corrosion and fatigue. They are commonly used at critical areas of engineering structures due to their high specific strength and stiffness [
1]. Since composite laminates are often used in load-carrying structures, an important issue is to evaluate the allowable design values of composite structures which considers various defects and damages [
2,
3].
Fiber reinforced polymer materials can be manufactured by several processes such as liquid molding, compression molding, resin infusion and injection molding. All these processes belong to autoclave manufacturing methods, and each of them produces different manufacturing fiber, waviness defects being one of the most relevant [
4,
5,
6]. The waviness is produced due to the axial compression of the fibers by the non-uniform pressure distribution between films and has a predominant effect on mechanical properties such as tensile, compressive, flexural and fatigue strength, being one of the most studied defects in the literature [
7,
8,
9,
10,
11].
The use of these materials in the manufacturing of large structures, such as aircrafts, requires mechanical joining of the parts using rivets or bolts. To generate the holes for these joints, the most widely used machining process is drilling [
12,
13,
14]. However, due to the low machinability of the CFRP materials caused by the abrasive character of the fiber reinforcement, the workpiece is susceptible to experience drilling induced damage, delamination being the prevalent damage [
15,
16,
17]. The life in service of the component can be affected by this problem [
18,
19,
20,
21] and is still a challenge for the aerospace industry to reduce the induced damage during machining [
14,
22,
23].
To characterize the behavior of the composite, the open-hole tension (OHT) and open-hole compression (OHC) tests are often used. During the last decades, relevant works focused on understanding the damage mechanism of laminates subjected to longitudinal loads. In the quasi-static OHT studies, different sizes and lay-ups were analyzed. Three distinctive failure modes are commonly found in the specimens: brittle failure, pull-out, and delamination. Delamination is the main failure affecting the in-plane strength, failure mechanism and hole size effect [
24,
25,
26,
27,
28]. For the OHC tests, failure was initiated as matrix cracking, but increasing the load, fiber kinking and delamination take dominant roles, especially at the edges of the hole. When damage reached a critical condition, the laminate fails catastrophically [
29,
30,
31,
32,
33]. The open hole geometry is also used in fatigue studies [
34,
35,
36,
37,
38], where it is proved that longitudinal strain, stiffness and surface temperature can provide valuable information regarding damage progression, fatigue life, and also for predicting notched fatigue performance.
Non-destructive methods are an effective tool to detect and control the damage evolution before failure caused by manufacturing defects or fatigue loading. To monitor the damage state in polymer matrix laminated structures, many characterization techniques are available nowadays, but the most widely used techniques are ultrasonic C-scan [
36,
39], X-ray radiography [
33,
40,
41,
42], acoustic emission [
43,
44], ultrasonic testing [
45,
46], infrared thermography (IRT) [
47,
48,
49] and Digital Image Correlation (DIC) [
26,
37,
50,
51]. The non-destructive techniques also help us to develop and validate damage progression models used to predict the damage occurring prior to failure [
27,
28,
52]. In this work, we use only IRT and DIC techniques.
During cycle loading of composite structures, material temperature varies as a function of material kinematics. The heating of the CFRP composite must be controlled because heat can affect the mechanical behavior and instigate chemical changes to the polymer matrix. Infrared thermography allows for measuring the surface temperature variation of the emitting body. This method can be applied in a passive or active mode: the first is generally applied on materials, which experience a different temperature than the surrounding materials; the second needs an external stimulus to induce a surface temperature variation, which can be heat or a mechanical source [
53]. The evaluation and growth of the damage is possible in active mode under fatigue testing [
46,
47,
54] as the evaluation of fatigue strength/durability/limit [
55,
56,
57]. Despite the versatility of this methodology to be applied over different types and geometries [
37,
52,
58,
59,
60], not many studies have focused on the open hole thick multi-directional laminates.
The Digital Image Correlation (DIC) is one of the optical methods applied to obtain accurate displacement and strain distributions on the surface of materials. By this method, 2D and 3D displacement fields could be obtained, but two or more cameras are required in the 3D case [
61]. DIC is applied to different problems in composites, such as 3D woven carbon/epoxy composite to characterize the strain field distribution during quasi-static tests [
37] or to locate the fracture initiation in the GFRP tensile tests [
62]. It has been also applied to fatigue studies, for example, to determine the interlaminar shear stress distributions and their variations with the number of cycles for carbon/epoxy composites [
51] or to measure deformations on the pin surface for low cycle fatigue loads [
63].
Despite the extensive literature, damage mechanisms and microscopic damage characteristics of open-hole thick laminates are still not fully described. In this study, the behavior of a thick CFRP laminate used in the aircraft industry is investigated. The purpose of this paper is to discuss damage detection and progression using non-destructive techniques during quasi-static and fatigue tests in specimens with stress concentrators. Among the existing techniques, two are investigated in this study: Infrared Thermography and Digital Image Correlation. Post-fatigue damaged samples were also analyzed using an optical microscope. Finally, the experimental tests are numerically simulated using the finite element method (FEM) in conjunction with a progressive damage analysis.
2. Materials and Experimental Setup
The material studied in this work is currently used in industry and is part of multiple structural elements of commercial aircraft. Experimental tests were performed on CFRP specimens cut from quasi-isotropic laminate plates made of 54 unidirectional carbon/epoxy plies stacked with different orientations (0°, 45°, −45°, 90°). The total thickness of the laminate is 9.7 mm and the resin content is 34%.
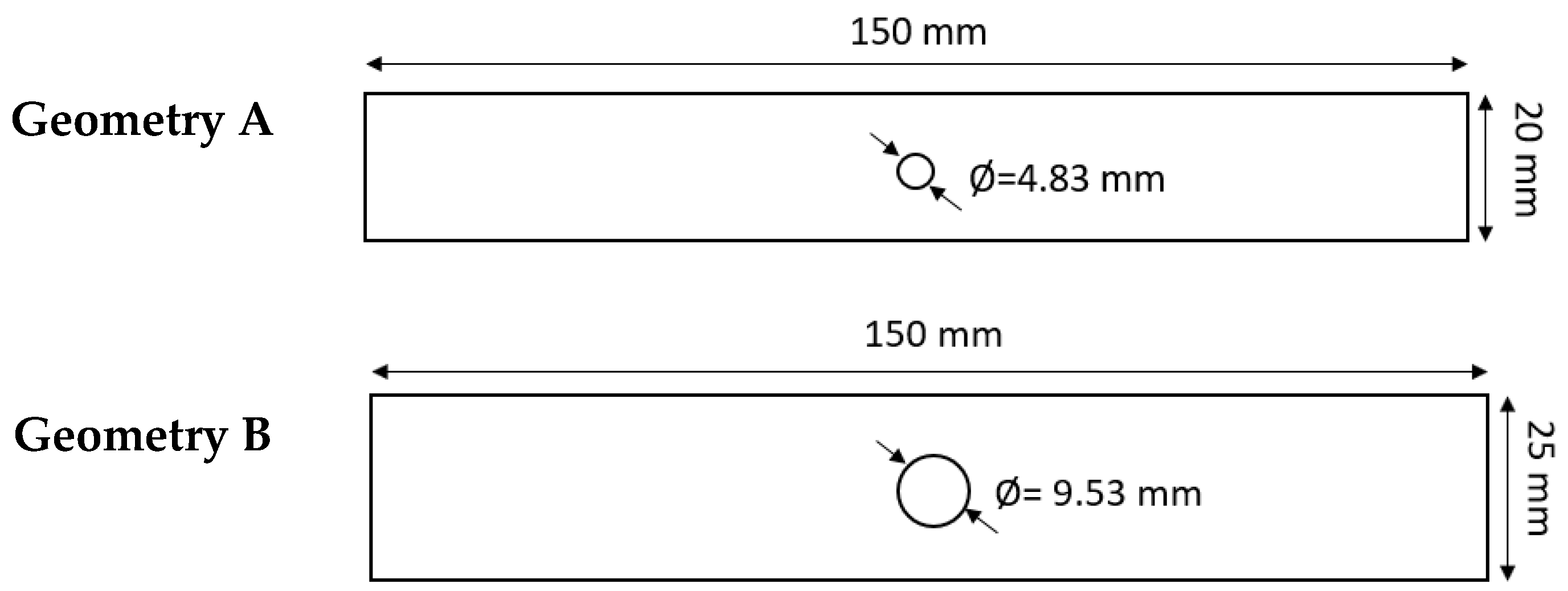
Two different geometries are used in the study, depicted in
Figure 1. Samples of these geometries were prepared for both the longitudinal and transverse direction of the original laminate plate material. The hole and the external perimeter were cut using an abrasive waterjet cutting technique.
Two non-destructive techniques were applied to analyze the mechanical behavior of these specimens under different load conditions: infrared thermography method for the fatigue study and digital image correlation method for the tensile test study. A hydraulic testing machine, INSTRON 8801, with a load cell of 100 kN, was used for both the static and fatigue tests. The next sections explain the set-up for each case.
2.1. Infrared-Monitored Fatigue Tests
Infrared thermography (IRT) is a non-destructive technique (NDT) widely used in the mechanical and structural fields for contactless measurement of the surface temperature distribution of an object. This technique reveals internal defects and material inhomogeneity of components, especially in the aeronautical field, because it allows quick inspections of large areas.
Based on thermoelastic theory, the temperature of a material changes when the material changes volume due to mechanical work. The temperature variation can be related to the stress change in an adiabatic environment using Equation (1), where Δ
T is the surface temperature variations of the sample during a fatigue cycle; T is room temperature;
is the density;
is the specific heat capacity at constant pressure; α
11 and α
22 are the surface coefficients of thermal expansion in 1 and 2 directions; Δσ
1 and Δσ
2 are the amplitudes of the principal stresses at the surface [
64]. All these parameters can be found in the literature [
65,
66].
Usually, the loading process can be treated as adiabatic if the fatigue loading frequency is higher than 5 Hz. Therefore, the temperature variation during a cyclic load is related to the stress change. The development of the stresses during the fatigue loading can be considered as an indicator of damage growth [
67].
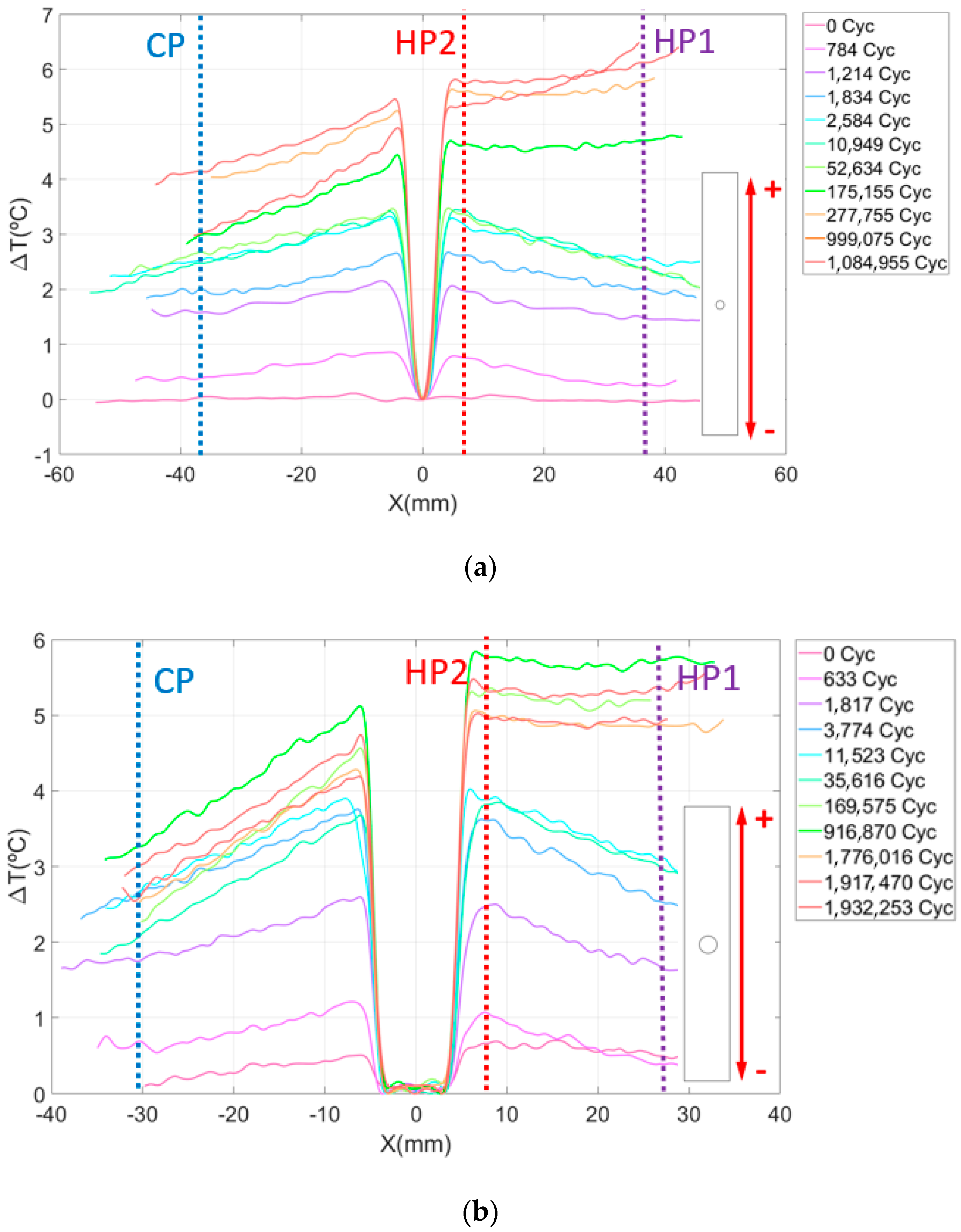
For this analysis, all tests were conducted at room temperature. During fatigue testing, infrared images of the samples surfaces were acquired using an IR camera (Testo 882, Titisee-Neustadt, Germany) to measure the variation of the specimen surface temperature. This camera is shown in
Figure 2. The IR camera pixel resolution of 320 × 240 and the temperature sensitivity of 50 mK was sufficient to monitor the temperature variation accurately.
This part of the study was carried out using specimens oriented in longitudinal and transversal orientations of the original laminate plate material. The fatigue tests were performed under load control mode with a σ
max = 60 kN, a σ
min = 0 kN and a frequency of 10 Hz to minimize non-adiabatic effect and reduce testing time [
64]. The limit of cycles was established at 2 million.
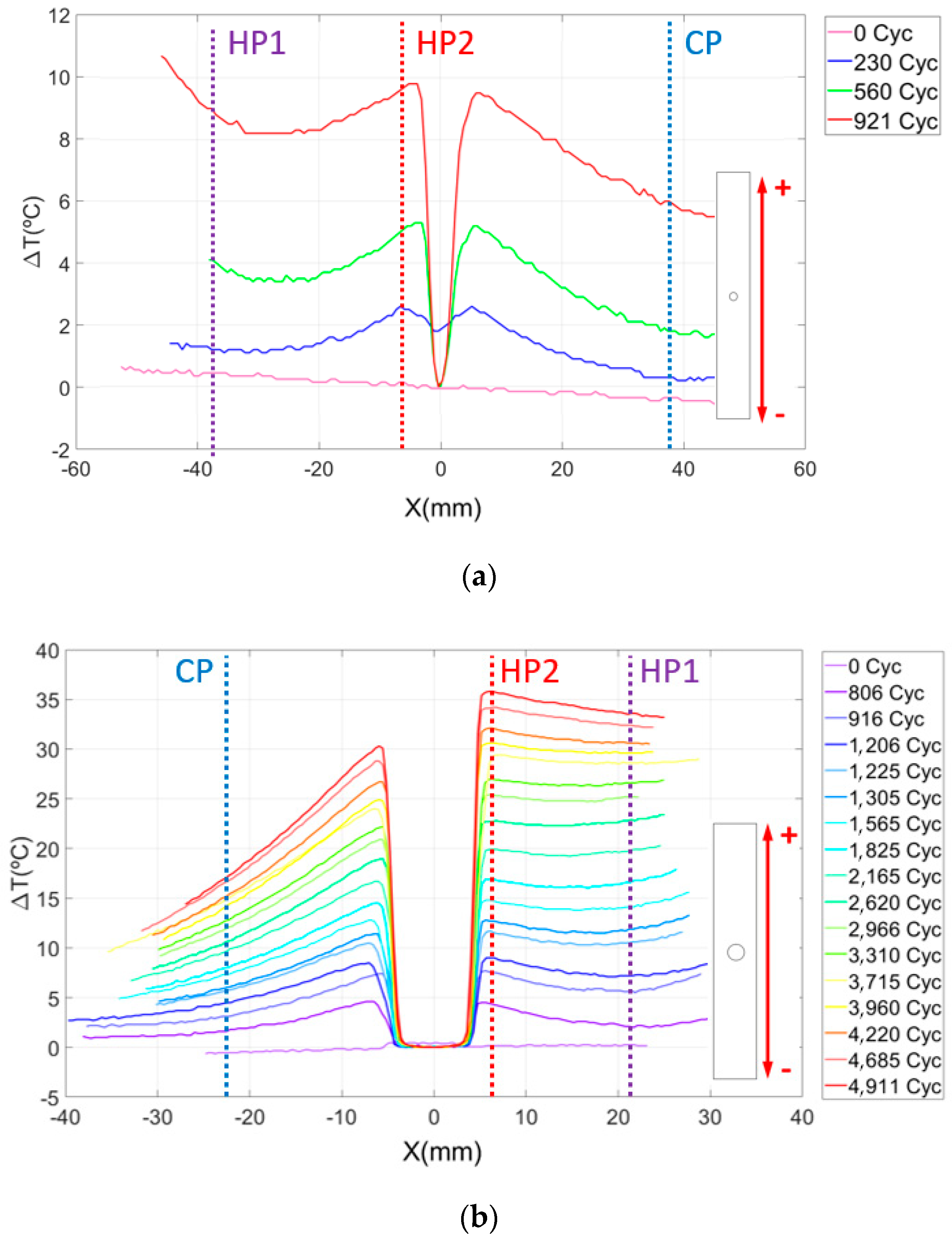
Figure 3 shows an example of the temperature variation measurement in a longitudinal specimen during fatigue testing with the geometry A.
2.2. Digital Image Correlation Monitored Quasi-Static Tests
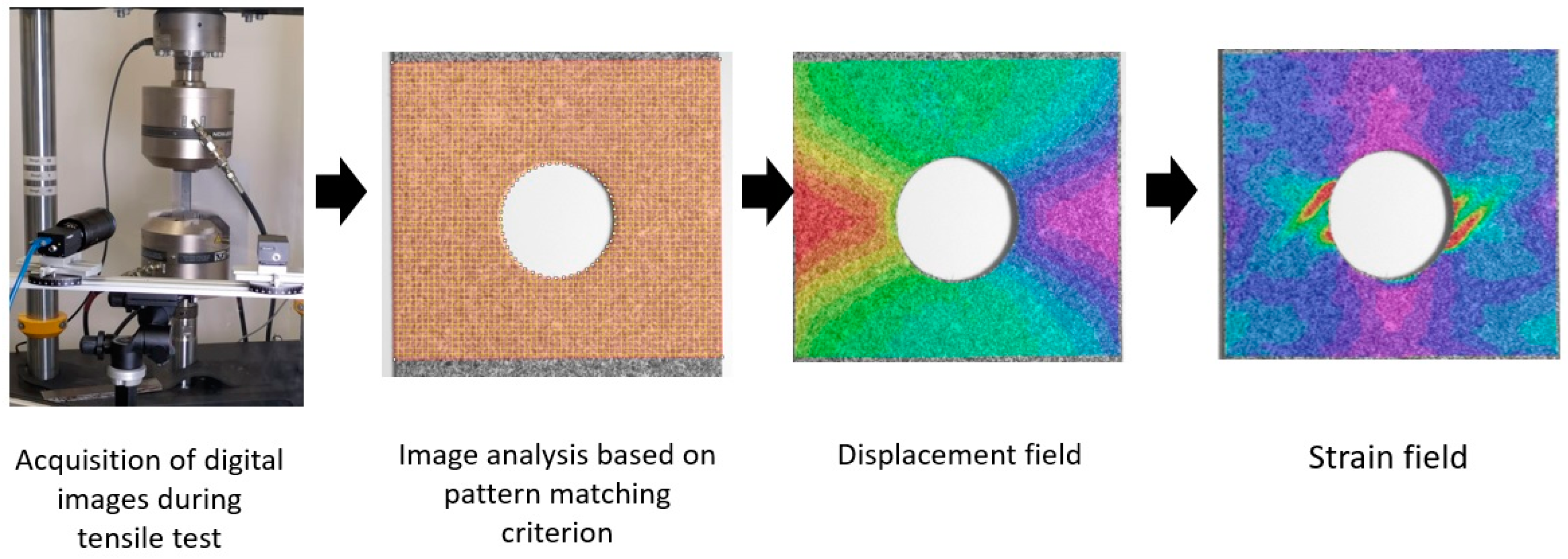
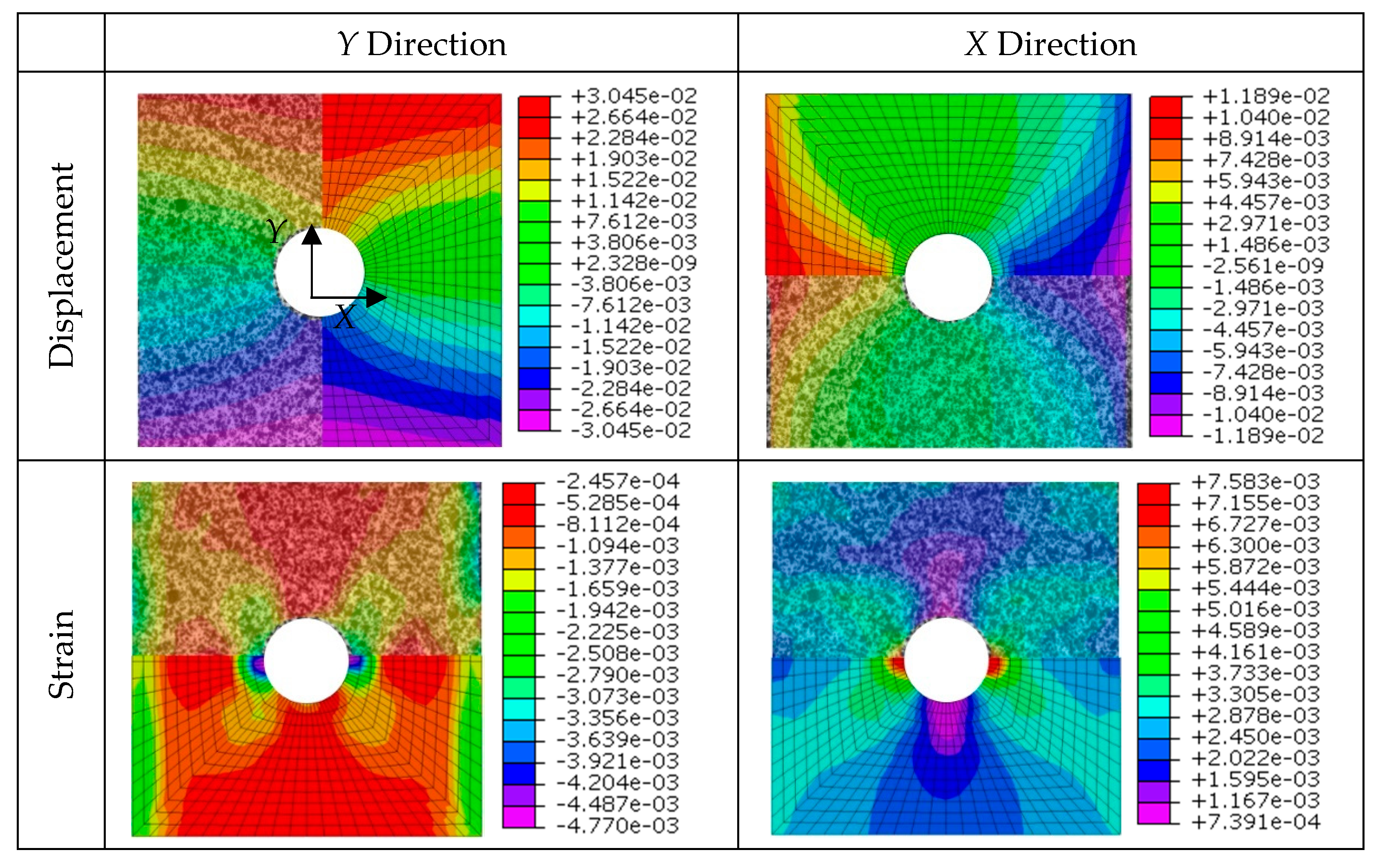
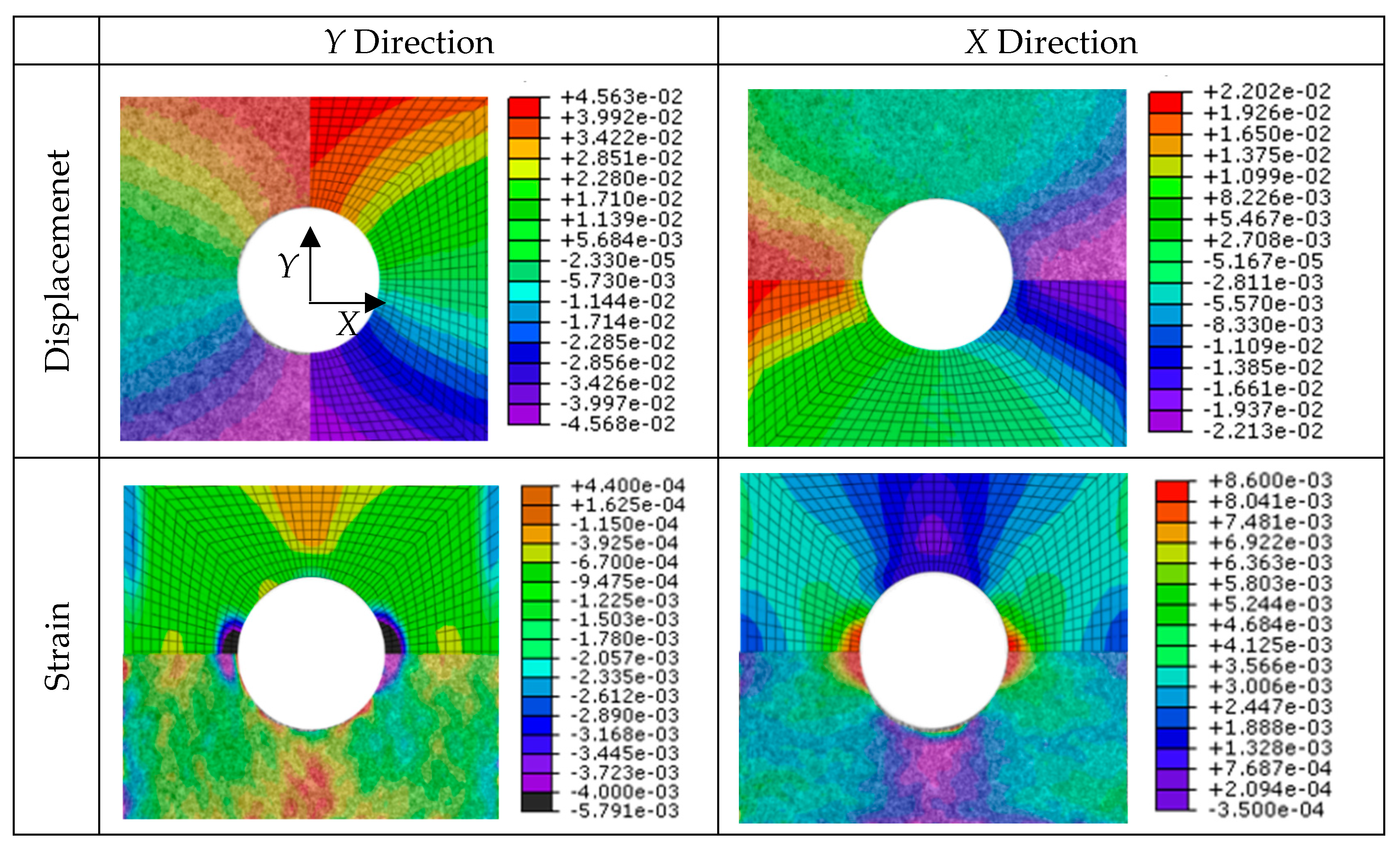
The Digital Image Correlation technique (DIC) was used to obtain the strain field on the up layer of the laminate during quasi-static tests. This non-destructive methodology is an optical displacement measure technique that employs image pattern tracking for accurate 2D or 3D surface deformation measurements during testing. In this work, 2D tracking was used. The DIC technique divides the region of interest (ROI) in squared faces to track their displacement based on an image pattern matching criterion and compares each deformed image with either the reference or the previous one. A ROI that covered the whole area around the hole of the specimen was defined. A scheme of the DIC technique application process is shown in
Figure 4. As can be seen, the DIC technique enables the detection of strain concentration zones in the analyzed structures, and it is also possible to identify the regions where cracking is initiated.
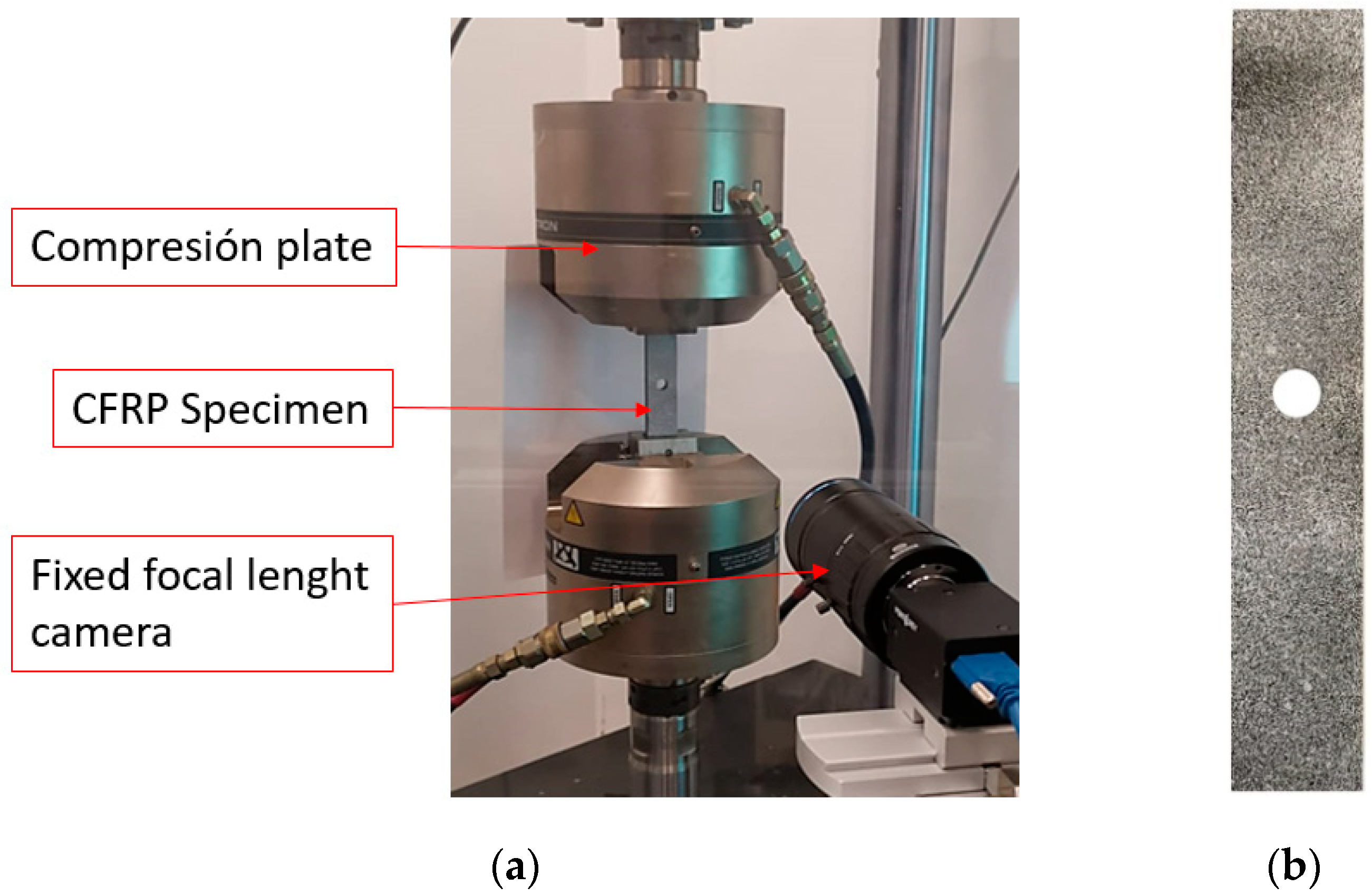
Images were taken with a high resolution fixed focal lens (HF7518V-2, Myutron, Tokyo, Japan) and extension rings of 10 mm (focal length of 65 mm). In order to apply DIC, the upper layer surface of the specimen was speckled using randomly distributed black and white spray paints to increase contrast, as shown in
Figure 5b. Moreover, the quality of the pattern was inspected using VIC-2D Digital Image Correlation software (v.6.0.2 Correlated Solutions Inc., Irmo, SC, USA) to verify optimum speckle pattern on the surface of the specimens. A perpendicular relative position between camera and specimen was ensured to acquire images and to avoid out-of-plane displacements during testing, as shown in
Figure 5a. All tests were performed under displacement control mode with quasi-static conditions, and an applied displacement rate of 2 mm/min following the D5766/D5766M standard [
68]. The force-displacement response data were registered. No pre-load was applied, and all tests were continued until the global failure of the specimens.
A facet size (the grid which divides the ROI) of 23 pixels and a step size (the spacing between control points) of 5 pixels were defined for tracking the speckle pattern during the mechanical test. A zero-normalized squared difference (ZNSSD) pattern-matching criterion was used to perform displacement correlation. Reference measurements were taken in a single image mode to enable DIC calibration. The results obtained were calibration deviation = 0.008 pixels (limit value: 0.011 pixels) and scale deviation = 0.16 µm (limit value: 0.22 µm).
The images acquired at the test were analyzed with the abovementioned software to estimate the surface displacement and engineering strain fields. Macro damages such as cracking were also examined from the obtained strain maps.
5. Conclusions
This work was concerned with non-destructive techniques to characterize the influence of the fiber waviness defect and the stress concentrator in a thick CFRP material representative of those used in the aircraft industry.
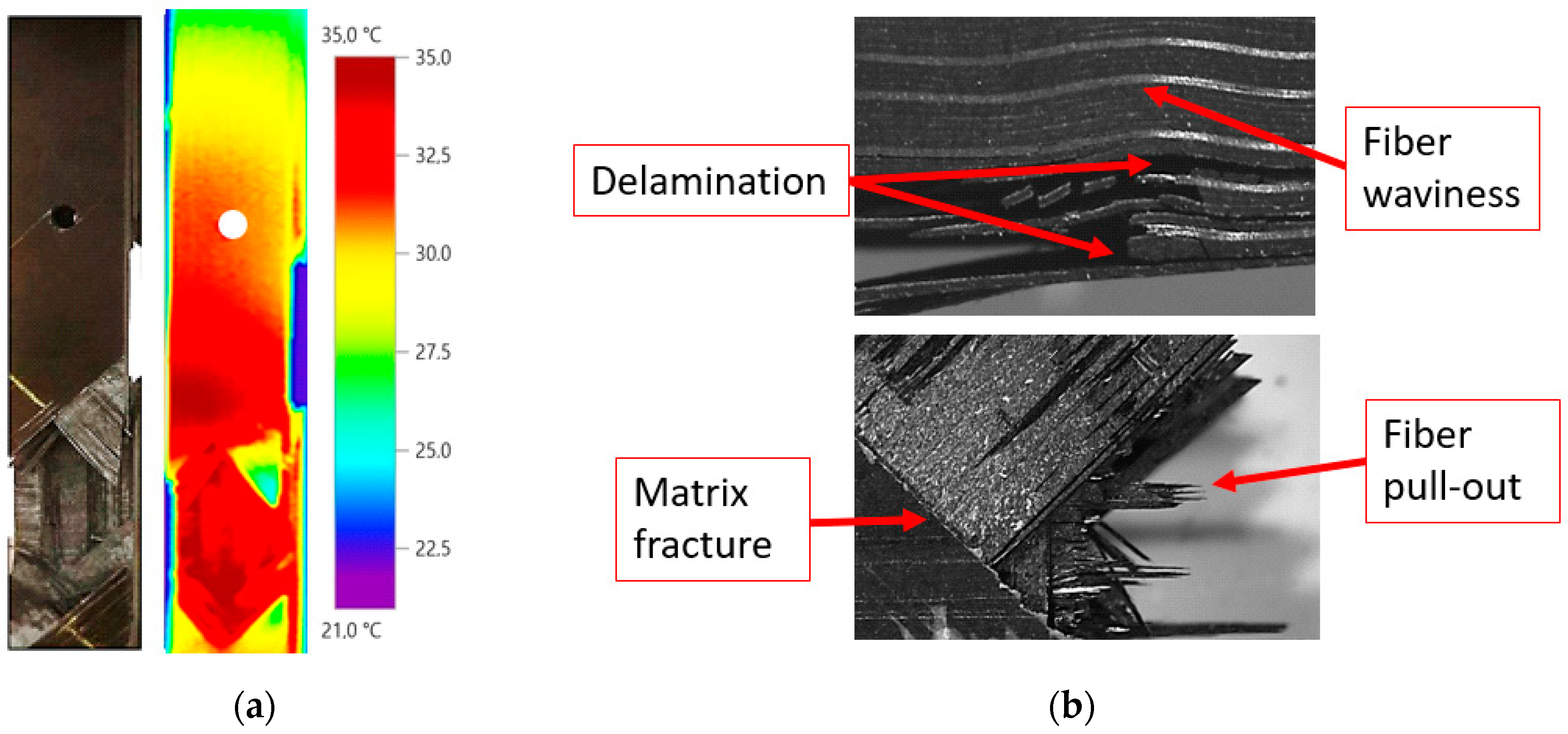
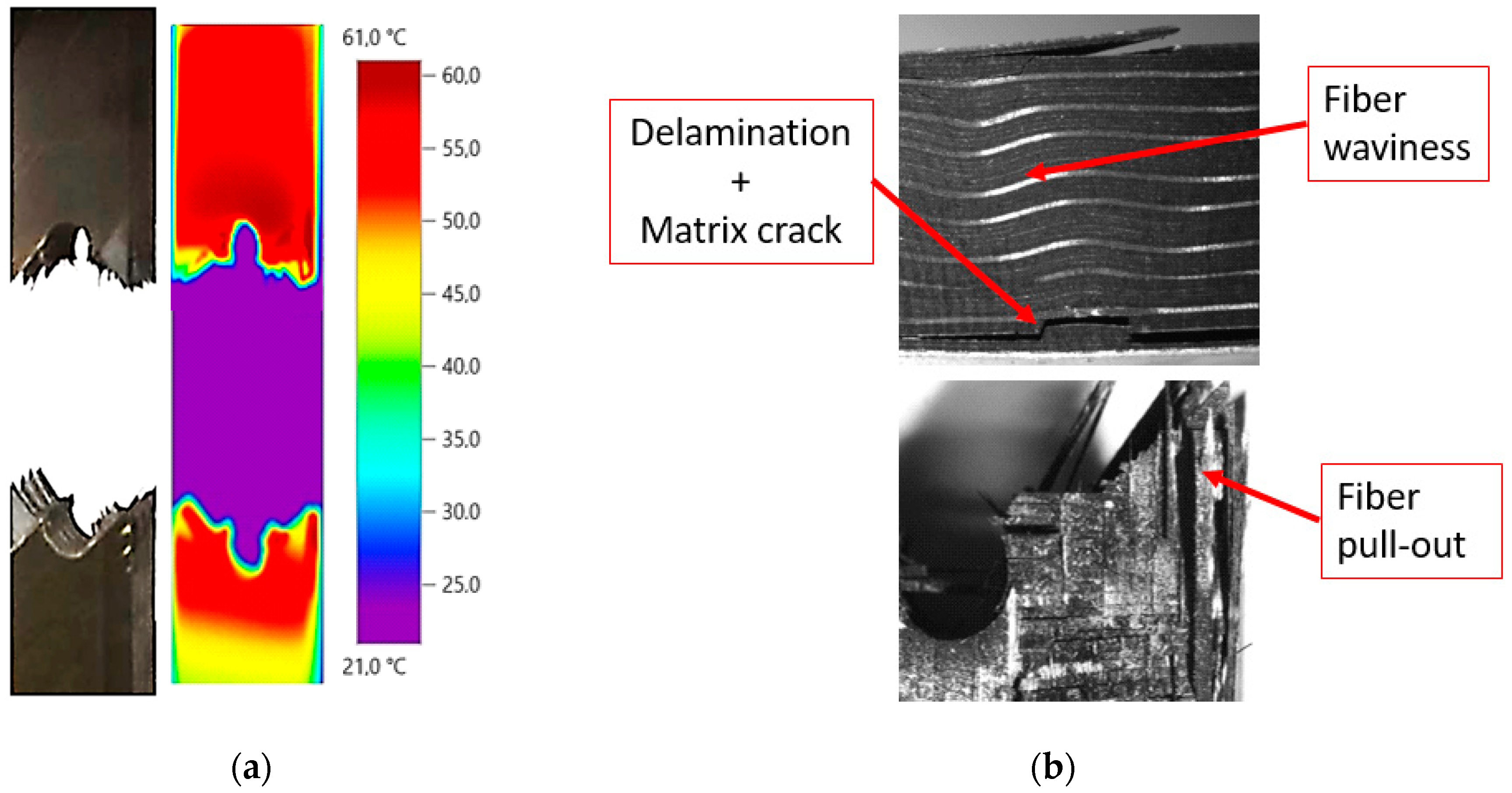
In the first place, an infrared camera captured the surface temperature distribution during fatigue loading testing. The specimens were cut in the two main perpendicular directions of the laminate. Based on the temperature gradient distribution maps, it was observed that the fiber waviness generated during the manufacturing process can produce a more severe damage in the material than the damage generated by the open hole. The influence of the waviness defect became more relevant when the load applied was close to the limit load. The temperature (and consequently the damage) grew more rapidly in this area of the specimen than around the hole. Further investigation in the testing of multidirectional composites with both stress concentrators (fiber waviness and open hole) can be beneficial, including changes in the test frequency. The combination of both phenomena was also observed in specimens with more plies oriented in the load direction, whose fatigue testing exceeded 2,000,000 cycles. For those cases, surface temperature was reduced by 84–89% at the hottest points of the material, which means that the damage generated by the waviness can be reduced as a function of the number of plies aligned with the load.
In the second place, the digital image correlation technique (DIC) was used to obtain the strain field in the surface material during quasi-static tensile test. The upper surface layer of the specimen was speckled using randomly distributed black and white spray paints to increase contrast and a camera was used to acquire images during testing. Results revealed that the strain distribution around the hole is able to predict the crack initiation and progression in the external plies of the CFRP laminate, establishing a work-load limit for the life in service of the material. This information can be useful to avoid the catastrophic failure of a structure.
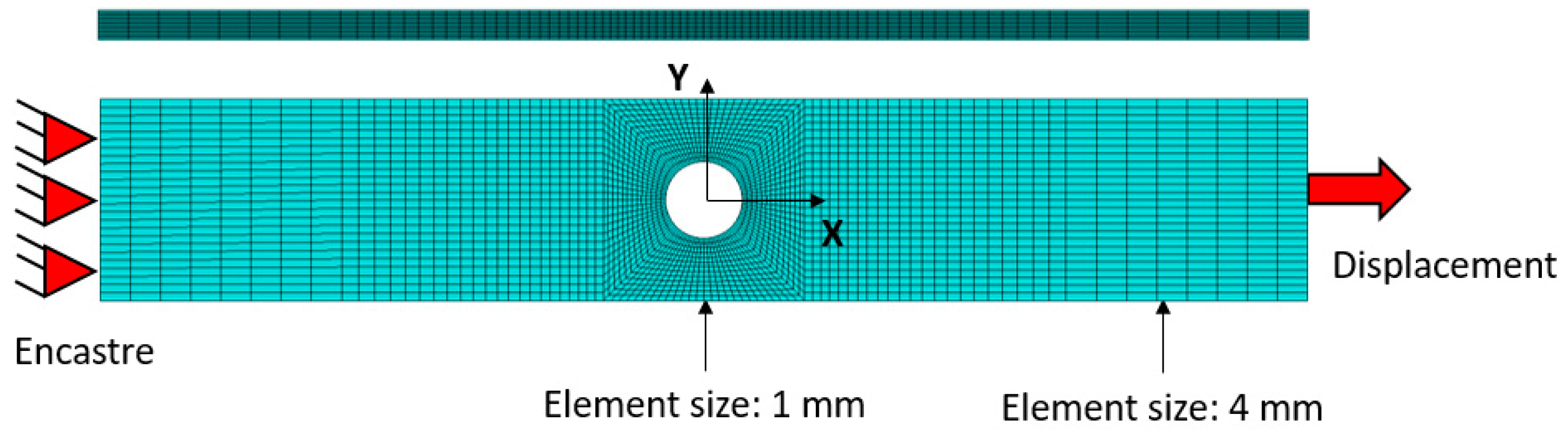
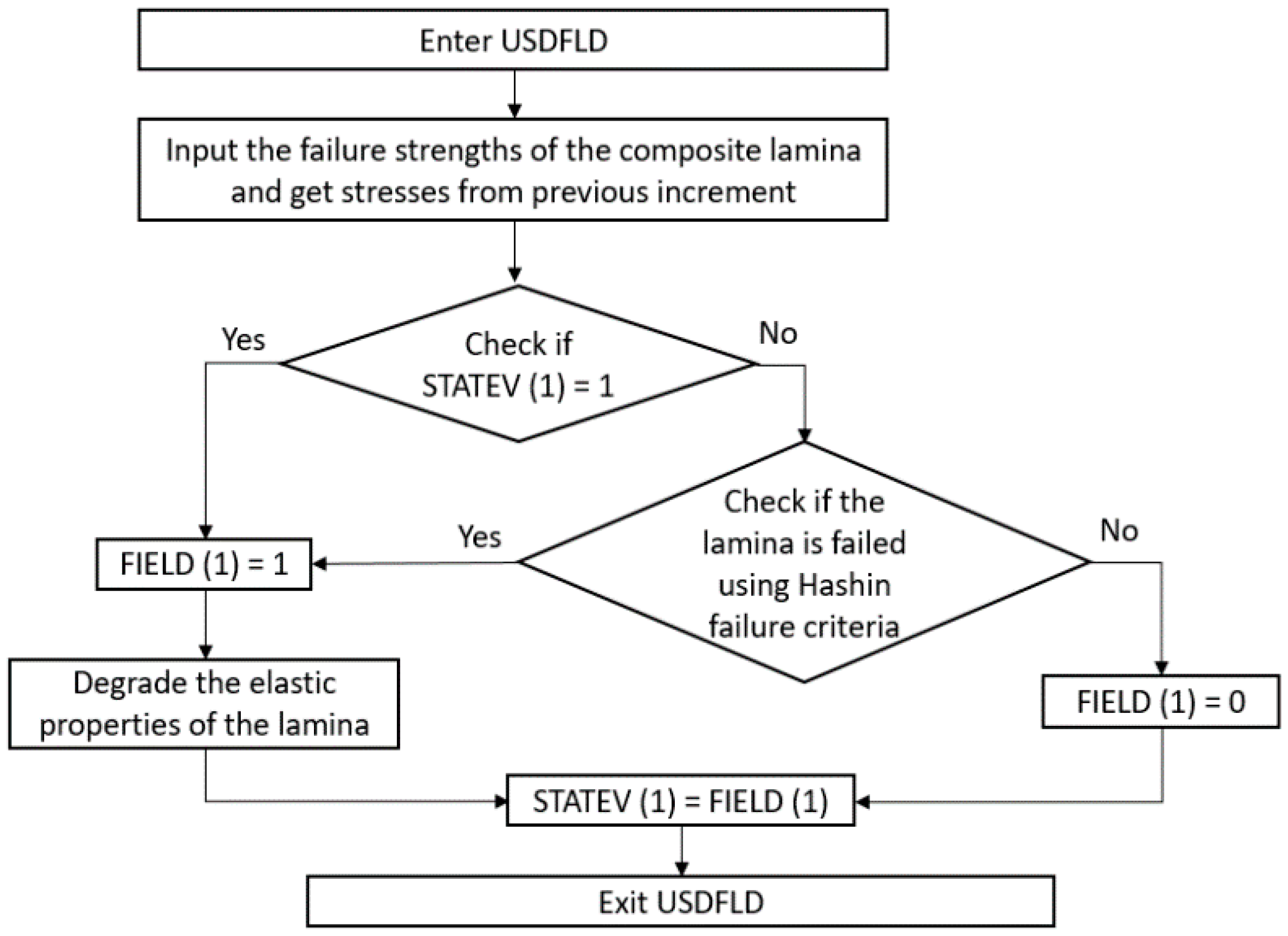
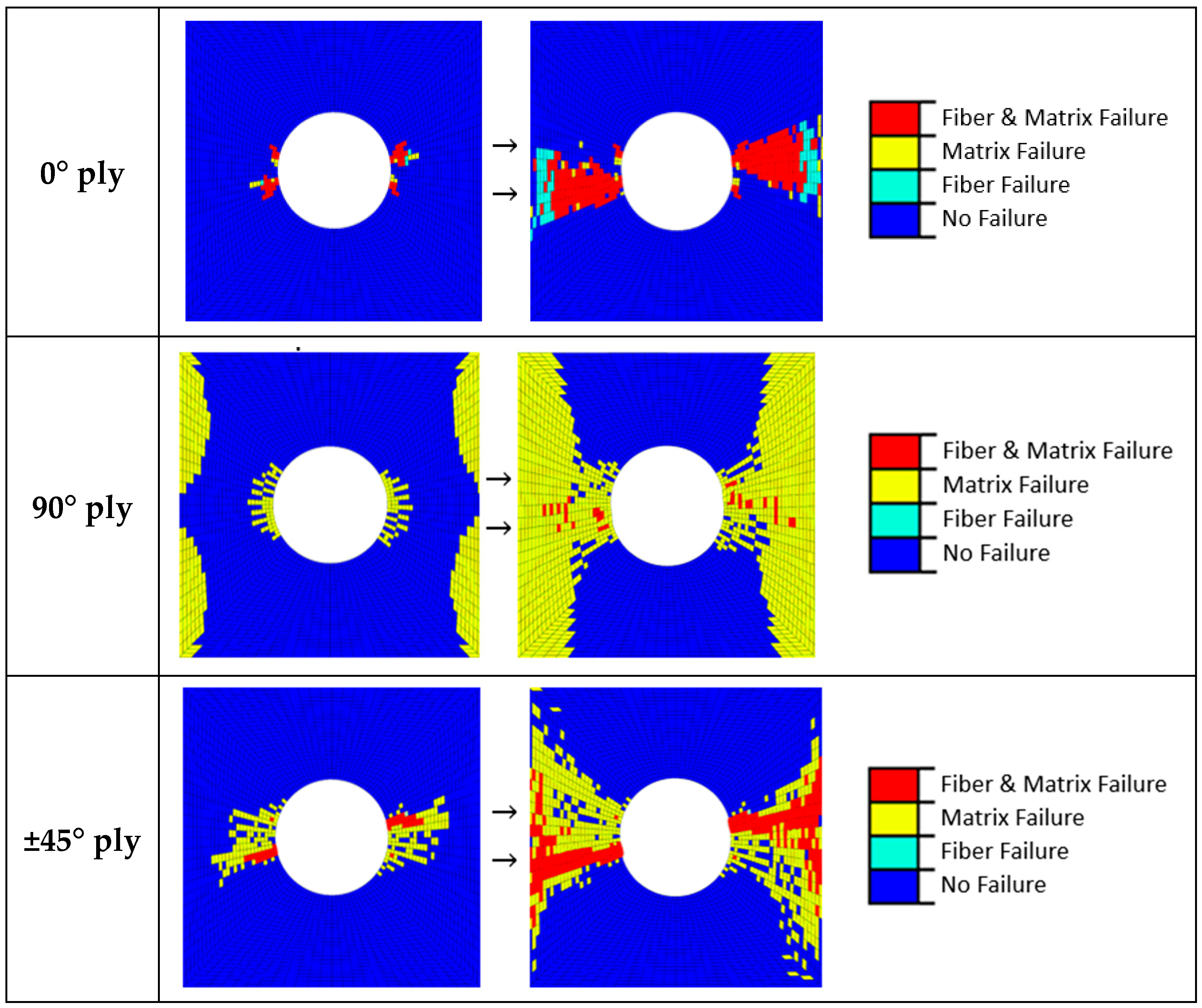
Finally, the experimental quasi-static tests were numerically simulated using the finite element method. Failure was implemented using Hashin criterion, which differentiates between matrix and fiber failure modes. Results showed good agreement between the numerical and DIC results. The model was also used to predict the damage sequence in the thick laminate. It was observed that, initially, damage starts to propagate from the hole to the free edge of the specimen in terms of matrix cracking and delamination bounded by ±45 plies. Finally, the catastrophic failure occurs with a high dispersion matrix crack damage in the 90° plies.