Direction-of-Arrival Estimation Methods in Interferometric Echo Sounding
Abstract
1. Introduction
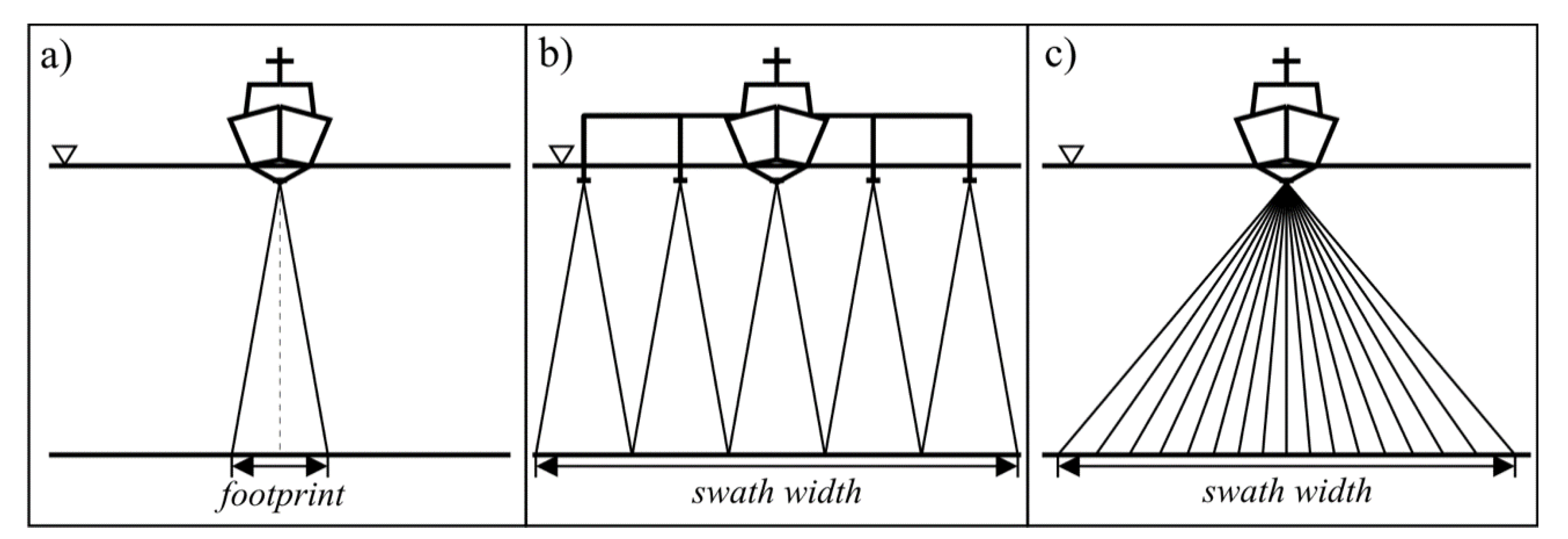
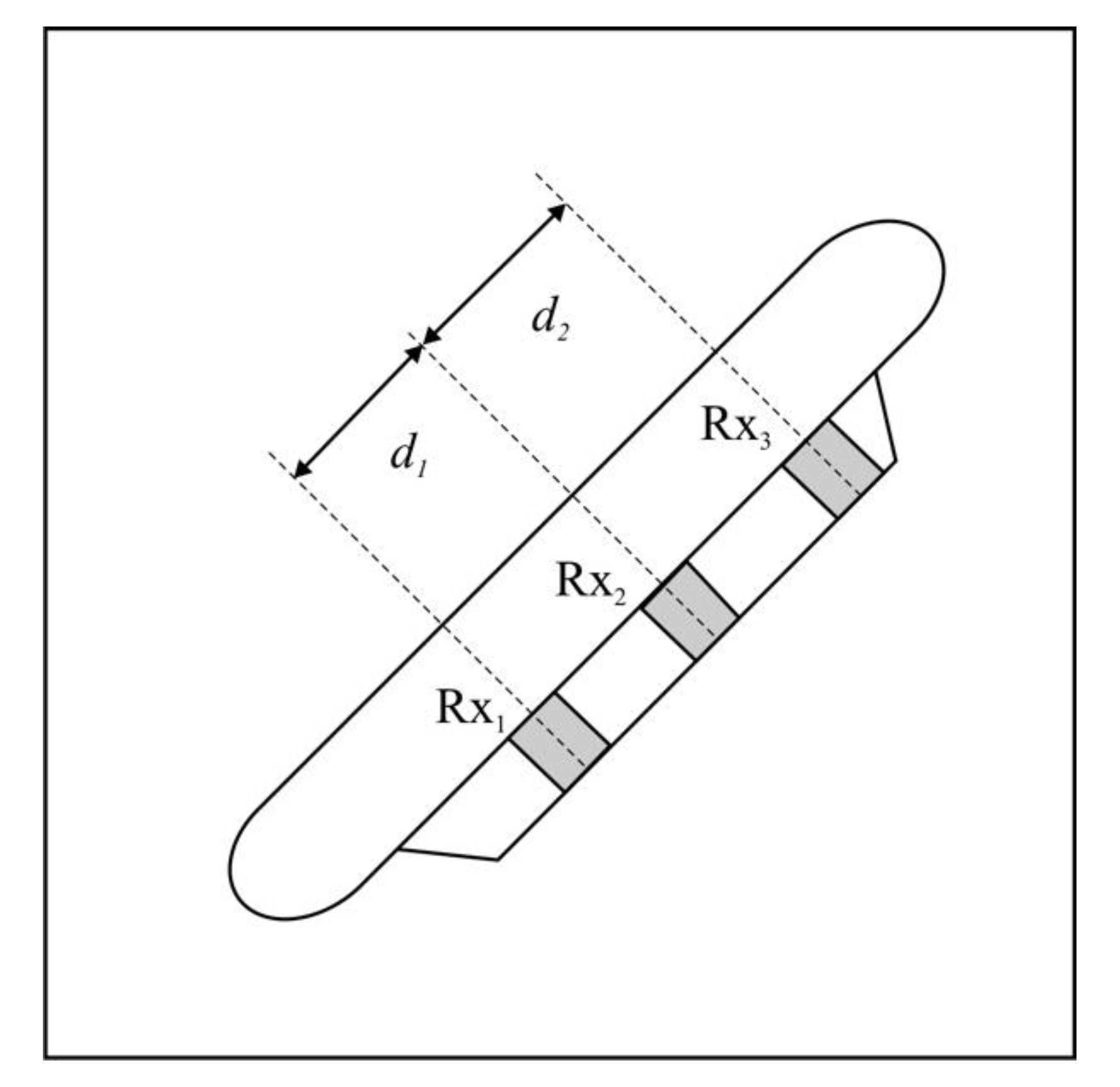
- The multi-channel echo sounder (MCES).
- The multi-beam echo sounder (MBES).
- The interferometric echo sounder (IES).
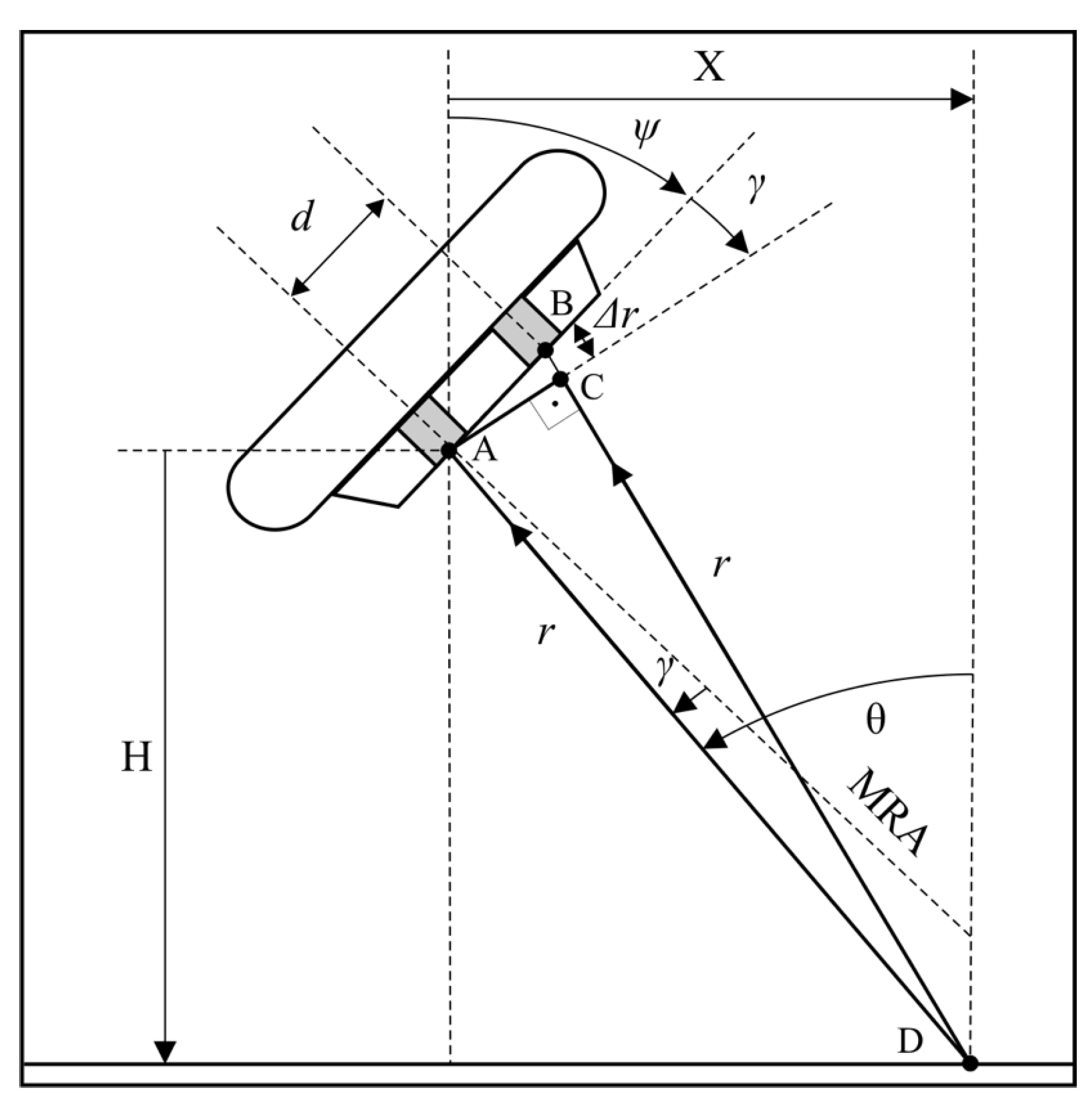
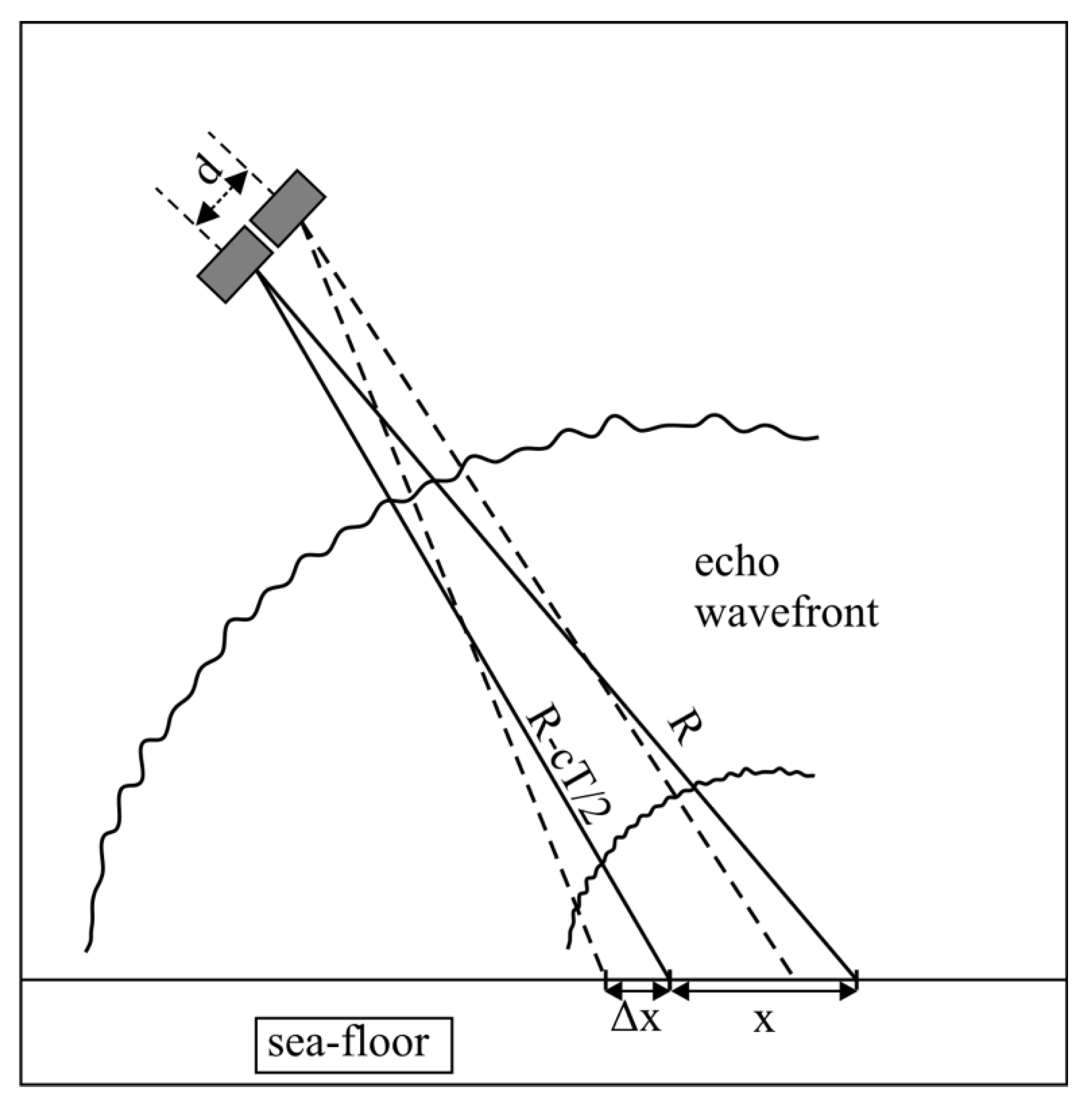
2. Classical Interferometry
3. Differential Interferometry
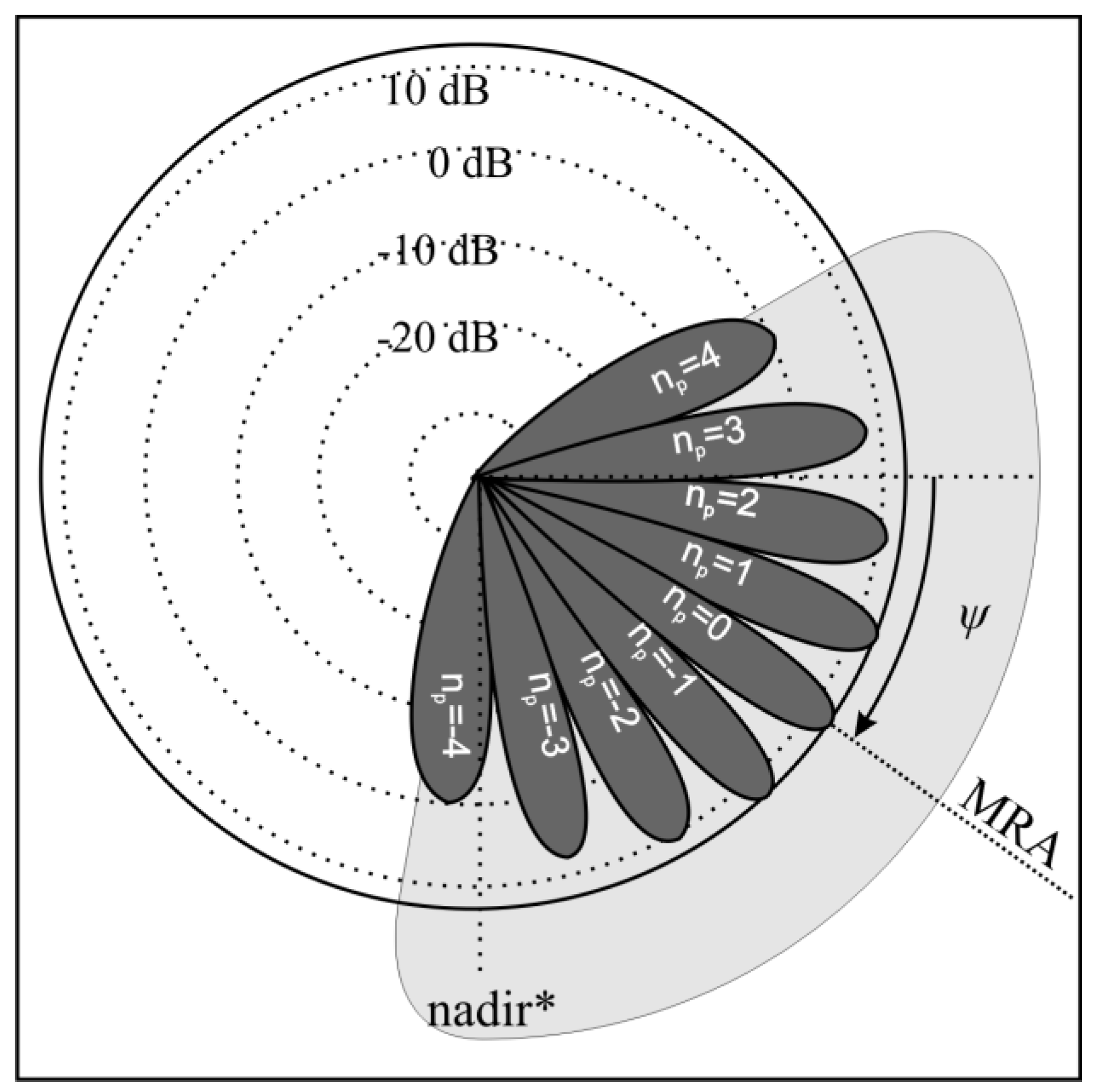
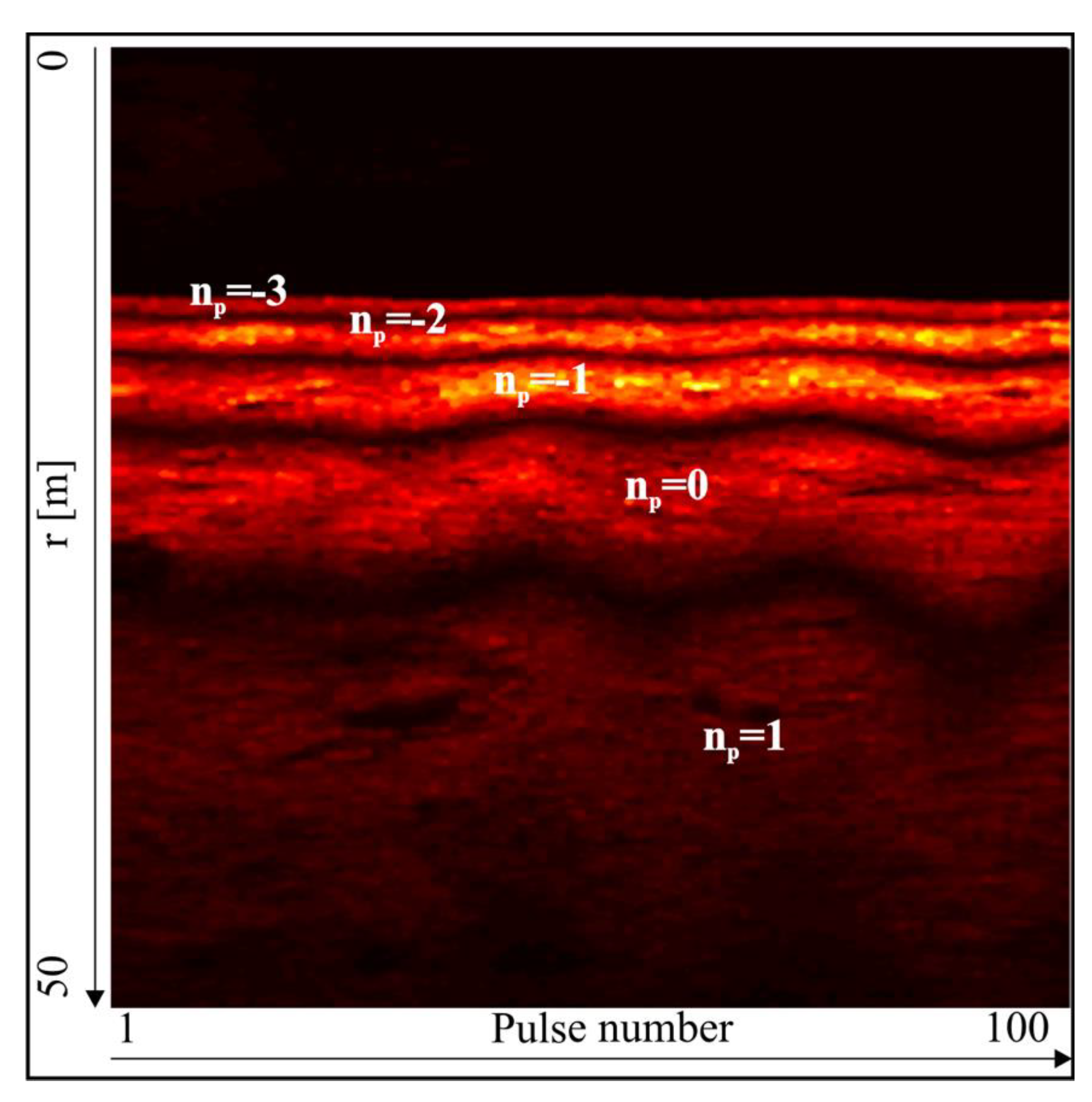
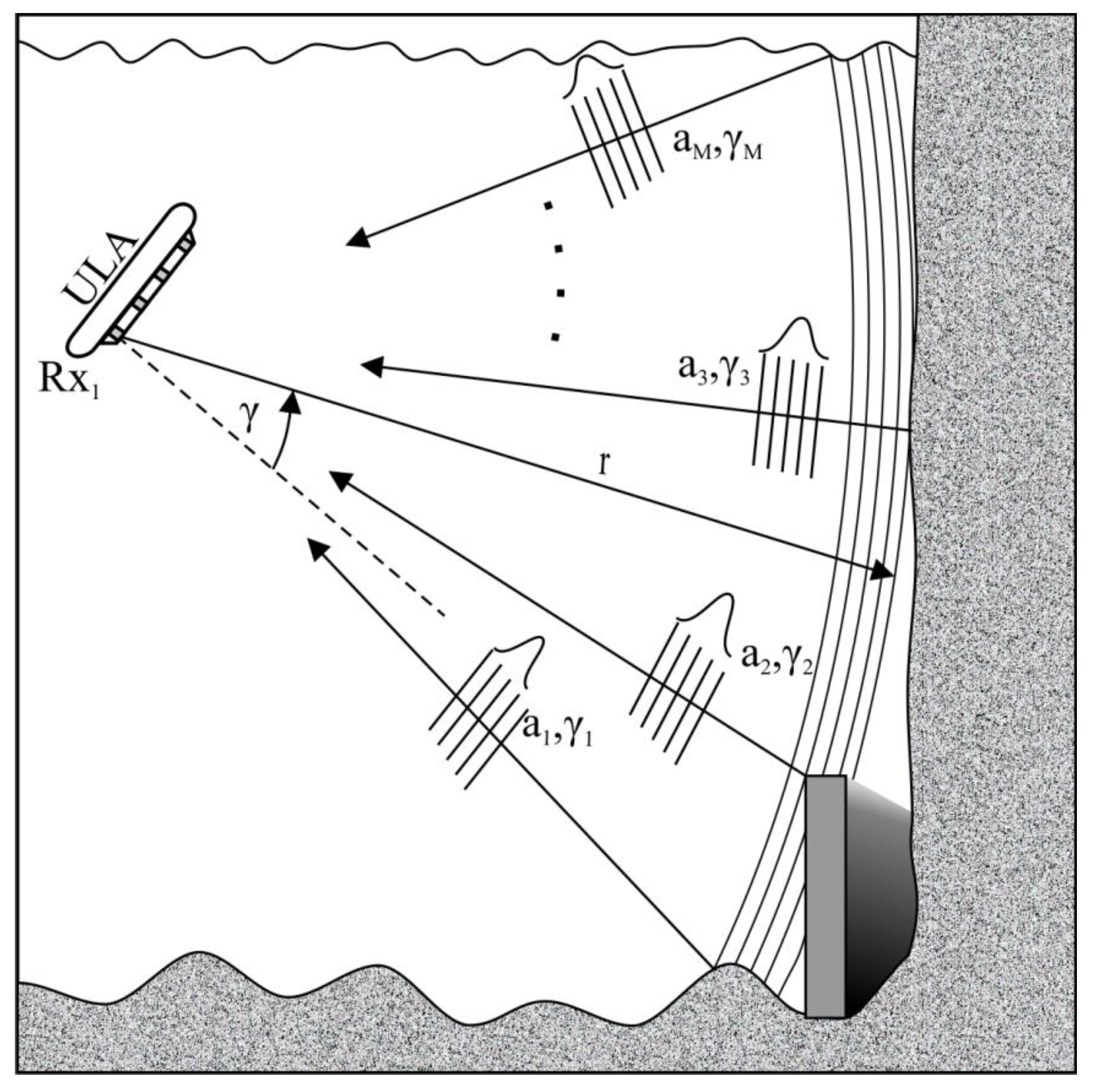
4. Multi-Phase Difference Interferometry
- 1
- A linear -element equispaced array (uniform linear array—ULA) is used to measure transmitted signal echoes propagating in the same plane as the array.
- 2.
- At each instant in time, exactly -independent, coplanar plane waves are incident on the receiving array.
- 3.
- The acoustic backscatter is narrowband.
- 4.
- The receiving array element output signals are in a steady state across the entire array.
5. Sources of Errors
6. Current Research Review
- Improvement in DOA accuracy.
- The application of subarray processing.
- The application of interferometric bathymetry to synthetic aperture sonar (SAS).
- Total least-squares.
- Modified Prony.
- Root-MUSIC.
- ESPRIT-TLS.
- Matrix Pencil.
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ainslie, M.A. Principles of Sonar Performance Modeling; Springer: Berlin/Heidelberg, Germany, 2010; p. 4. [Google Scholar] [CrossRef]
- Cloet, R.L.; Hurst, S.L.; Edwards, C.R.; Phillips, P.S.; Duncan, A.J. A Sideways-looking Towed Depth-measuring System. J. Navig. 1982, 35, 411–420. [Google Scholar] [CrossRef]
- Stubbs, A.R.; McCartney, B.S.; Legg, J.G. Telesounding, a method of wide swathe depth measurement. Int. Hydrogr. Rev. 1974, L, 23–59. [Google Scholar]
- US Army Corps of Engineers. Hydrographic Surveying-Engineer Manual; US Army Corps of Engineers: Washington, DC, USA, 2013. [Google Scholar]
- International Hydrographic Organization. Manual on Hydrography–Publication C-13, 1st ed.; International Hydrographic Bureau: Monaco, 2005; pp. 119–120. [Google Scholar]
- International Hydrographic Organization. IHO Standards for Hydrographic Surveys-Special Publication 44, 5th ed.; International Hydrographic Bureau: Monaco, 2008; p. 15. [Google Scholar]
- Hiller, T.; Brisson, L.N.; Wright, S. Measuring Bathymetric Uncertainty of the EdgeTech 4600 Sonar. In Proceedings of the Hydro12-Taking care of the sea, Rotterdam, The Netherlands, 13–15 November 2012. [Google Scholar] [CrossRef]
- Hariharan, P. Basics of Interferometry, 2nd ed.; Academic Press: San Diego, CA, USA, 2007; pp. 13–14. [Google Scholar]
- Cloet, R.L.; Edwards, C.R. The bathyscan precision swathe sounder. In Proceedings of the Oceans ’86, Washington, DC, USA, 23–25 September 1986; pp. 153–162. [Google Scholar] [CrossRef]
- Liu, Z.J.; Naar, D.F. Swath Bathymetry Processing of GLORI-B and SeaBeam 2000. Mar. Geophys. Res. 1997, 19, 407–419. [Google Scholar] [CrossRef]
- Matsumoto, H. Characteristics of SeaMARC II phase data. IEEE J. Ocean. Eng. 1990, 15, 350–360. [Google Scholar] [CrossRef]
- Pryor, D.E. Theory and test of bathymetric side scan sonar. In Proceedings of the Oceans’88, Baltimore, MD, USA, 31 October–2 November 1988; pp. 379–384. [Google Scholar] [CrossRef]
- Sun, J.; Tyce, R.C. Performance Limitations on Travel Time and Phase Data Extraction for Bathymetry Side-scan Sonars. In Proceedings of the Oceans’93, Victoria, BC, Canada, 18–21 October 1993; pp. II-71–II-76. [Google Scholar] [CrossRef]
- Denbigh, P.N. Stereoscopic visualization and contour mapping of the sea bed using a bathymetric sidescan sonar (BASS). Radio Electron. Eng. 1983, 53, 301–307. [Google Scholar] [CrossRef]
- Lesnikowski, N. A decade of isophase swath bathymetr. In Proceedings of the Oceans’99, Seattle, WA, USA, 13–16 September 1999; pp. 901–903. [Google Scholar] [CrossRef]
- de Moustier, C. State of the art in swath bathymetry survey systems. Int. Hydrogr. Rev. 1988, LXV, 25–54. [Google Scholar]
- Lurton, X. Swath bathymetry using phase difference: Theoretical analysis of acoustical measurement precision. IEEE J. Ocean. Eng. 2000, 25, 351–363. [Google Scholar] [CrossRef]
- Jin, G.; Tang, D. Uncertainties of differential phase estimation associated with interferometric sonars. IEEE J. Ocean. Eng. 1996, 21, 53–64. [Google Scholar] [CrossRef]
- Wilby, A.D. The advantages, challenges and practical implementation of an interferometric swath bathymetry system. In Proceedings of the Oceans’99, Seattle, WA, USA, 13–16 September 1999; pp. 23–29. [Google Scholar]
- Llort-Pujol, G.; Sintes, C.; Chonavel, T.; Morrison, A.T.; Daniel, S. Advanced Interferometric Techniques for high resolution bathymetry. Mar. Technol. Soc. J. 2012, 46, 9–31. [Google Scholar] [CrossRef]
- Cervenka, P.; de Moustier, C. Postprocessing and corrections of bathymetry derived from sidescan sonar systems: Application with SeaMARC II. IEEE J. Ocean. Eng. 1994, 19, 619–629. [Google Scholar] [CrossRef]
- Kraeutner, P.H. Small Aperture Acoustic Imaging Using Model Based Array Signal Processing. Ph. D. Thesis, Simon Fraser University, Burnaby, BC, Canada, 1998. [Google Scholar]
- Kraeutner, P.H.; Bird, J.S. Principal components array processing for swath acoustic mapping. In Proceedings of the Oceans’97, Halifax, NS, Canada, 6–9 October 1997; pp. 1246–1254. [Google Scholar] [CrossRef]
- Kraeutner, P.H.; Bird, J.S. Beyond interferometry, resolving multiple angles-of-arrival in swath bathymetric imaging. In Proceedings of the Oceans’99, Seattle, WA, USA, 13–16 September 1999; pp. 37–45. [Google Scholar] [CrossRef]
- Kraeutner, P.H.; Bird, J.S.; Charbonneau, B.; Bishop, D.; Hegg, F. Multi-angle swath bathymetry sidescan quantitative performance analysis. In Proceedings of the Oceans’02, Biloxi, MI, USA, 29–31 October 2002; pp. 2253–2263. [Google Scholar] [CrossRef]
- Tufts, D.W.; Kumaresan, R. Estimation of frequencies of multiple sinusoids: Making linear prediction perform like maximum likelihood. Proc. IEEE 1982, 70, 975–990. [Google Scholar] [CrossRef]
- Grall, P.; Marszal, J. Depth determination accuracy of the modified Prony method in a swath mapping application. In Proceedings of the IEEE 2018 Joint Conference–Acoustics, Ustka, Poland, 11–14 September 2018; pp. 74–79. [Google Scholar] [CrossRef]
- Denbigh, P.N. Swath Bathymetry: Principles of Operation and an Analysis of Errors. IEEE J. Ocean. Eng. 1989, 14, 289–298. [Google Scholar] [CrossRef]
- Sintes, C.; Llort-Pujol, G.; Guériot, D. Coherent Probabilistic Error Model for Interferometric Sidescan Sonars. IEEE J. Ocean. Eng. 2010, 35, 412–423. [Google Scholar] [CrossRef]
- Bird, J.S.; Mullins, G.K. Analysis of Swath Bathymetry Sonar Accuracy. IEEE J. Ocean. Eng. 2005, 30, 372–390. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, H.; Zhou, T.; Liu, X.; Ren, C. An Improved Method for Unwrapping Phase Difference in Bathymetry. In Proceedings of the 2010 IEEE International Conference on Information and Automation, Harbin, China, 20–23 June 2010; pp. 1071–1075. [Google Scholar] [CrossRef]
- Sintes, C.; Llort-Pujol, G.; Le Caillec, L.-M. Vernier interferometer performance analysis. In Proceedings of the OCEANS’11 MTS, Waikoloa, HI, USA, 19–22 September 2011. [Google Scholar] [CrossRef]
- Sintes, C.; Foote, K.G.; Llort-Pujol, G. Relationships among Vernier-method and other direction-of-arrival estimators. In Proceedings of the OCEANS 2015, Genoa, Italy, 18–21 May 2015. [Google Scholar] [CrossRef]
- Mironov, A.S.; Fomina, E.S. Processing Hydroacoustic Signals in Systems for Sonar Surveying. In Proceedings of the 2018 International Multi-Conference on Industrial Engineering and Modern Technologies, Vladivostok, Russia, 3–4 October 2018. [Google Scholar] [CrossRef]
- Singh, J.S.; Ioana, C.; Geen, M.; Mars, J. Wideband signal processing techniques for Interferometric Sonars. Oeans’18 Mts/IEEE 2018, 36, 1540–1550. [Google Scholar] [CrossRef]
- Singh, J.S.; Ioana, C.; Geen, M.; Mars, J. Interferometric Measurements with Wideband Signal Processing Techniques. In Proceedings of the Oceans’19 MTS/IEEE, Marseille, France, 17–20 June 2019. [Google Scholar] [CrossRef]
- Llort-Pujol, G.; Sintes, C. Interferometric angle estimation for bathymetry performance analysis. In Proceedings of the Oceans’11, Santander, Spain, 6–9 June 2011. [Google Scholar] [CrossRef]
- Sintes, C.; Llort-Pujol, G. Empirical Interferometric Phase Variance Formulas for Bathymetric Applications. IEEE Trans. Geosci. Remote Sens. 2012, 50, 2079–2097. [Google Scholar] [CrossRef]
- Rao, B.D.; Arun, K.S. Model based processing of signals: A state space approach. Proc. IEEE 1992, 80, 283–309. [Google Scholar] [CrossRef]
- van der Veen, A.-J.; Deprettere, E.F.; Swindlehurst, A.L. Subspace-based signal analysis using singular value decomposition. Proc. IEEE 1993, 81, 1277–1308. [Google Scholar] [CrossRef]
- Hua, Y.; Sarkar, T.K. Matrix pencil method for estimating parameters of exponentially damped/undamped sinusoids in noise. IEEE Trans. Acoust. SpeechSignal Process. 1990, 38, 814–824. [Google Scholar] [CrossRef]
- Rahman, M.A.; Yu, K.B. Total least squares approach for frequency estimation using linear prediction. IEEE Trans. Acoust. SpeechSignal Process. 1987, ASSP-35, 1440–1454. [Google Scholar] [CrossRef]
- Wax, M.; Kaliath, T. Detection of signals by information theoretic criteria. IEEE Trans. Acoust. Speech Signal Process. 1985, 33, 387–392. [Google Scholar] [CrossRef]
- Grall, P.; Marszal, J. Theoretical analysis of a new approach to order determination for a modified Prony method in swath mapping application. Hydroacoustics 2017, 20, 63–74. [Google Scholar]
- Grall, P. The accuracy of a new approach to order determination for a modified Prony method in swath mapping application. Hydroacoustics 2017, 20, 51–62. [Google Scholar]
- Holt, J.N.; Antill, R.J. Determining the number of terms in a Prony algorithm exponential fit. Math. Biosci. 1997, 36, 319–323. [Google Scholar] [CrossRef]
- Li, Z.; Li, H.; Zhou, T.; Yuan, Y. Multiple sub-array beamspace CAATI algorithm for multibeam bathymetry system. J. Mar. Sci. Appl. 2007, 6, 47–52. [Google Scholar] [CrossRef]
- Zhou, T.; Li, H.-S.; Zhu, Z.-D.; Yuan, Y.-Y. Application of modified multiple subarrays detection method to multibeam bathymetry system. J. Mar. Sci. Appl. 2005, 4, 39–43. [Google Scholar] [CrossRef]
- Zhou, T.; Shen, J.; Zhu, J.; Zhang, W.; Peng, D. Beamforming interferometry bathymetry method based on multi-coprime sensor array. Int. J. Distrib. Sens. Netw. 2019, 15. [Google Scholar] [CrossRef]
- Doan, S.V.; Tran, T.C.; Nguyen, V.C. DOA Estimation of Underwater Acoustic Signals Using Non-uniform Linear Arrays. In Proceedings of the 14th EAI International Conference, INISCOM, Da Nang, Vietnam, 27–28 August 2018; pp. 103–110. [Google Scholar] [CrossRef]
- Vincent, P.; Maussang, V.; Lurton, X.; Sintès, C.; Garello, R. Bathymetry Degradation Causes for Frequency Modulated Multibeam Echo Sounders. In Proceedings of the 2012 Oceans, Hampton Roads, VA, USA, 14–19 October 2012. [Google Scholar] [CrossRef]
- Wang, L.; Bellettini, A.; Hollett, R.; Tesei, A.; Pinto, M.; Chapman, S.; Gade, K. InSAS’00: Interferometric SAS and INS aided SAS imaging. In Proceedings of the Oceans’01, Honolulu, HI, USA, USA, 5–8 November 2001; pp. 1–9. [Google Scholar] [CrossRef]
- Sæbø, T.O. Seafloor Depth Estimation by Means of Interferometric Synthetic Aperture Sonar. Ph.D. Thesis, University of Tromsø, Tromsø, Norway, 2010. [Google Scholar]
- Sæbø, T.O.; Synnes, S.A.V.; Hansen, R.E. Wideband Interferometry in Synthetic Aperture Sonar. IEEE Trans. Geosci. Remote Sens. 2013, 51, 4450–4459. [Google Scholar] [CrossRef]
- Giardina, P.E. Interferometric Synthetic Aperture Sonar Signal Processing for Autonomous Underwater Vehicles Operating Shallow Water. Ph.D. Thesis, University of New Orleans Theses and Dissertations, New Orleans, LA, USA, 2012; p. 1553. [Google Scholar]


| No. | System Name (Prod. Year) | Freq. [kHz] 1 | No. of Receive Elements | d | Beam Width [deg. × deg.] 3 |
|---|---|---|---|---|---|
| 1 | Telesounding (1974) | 250 | 1 2 2 | 33/60 λ 33 λ | 1 × 50 |
| 2 | Bathyscan (1982) | 300 | 2 | 10 λ, 11 λ | 1 × 25 |
| 3 | TOPO-SSS (1982) | 160 | 2 | 1.9 λ | 2 × 45 |
| 4 | SeaMARC II (1983) | 11, 12 | 2 | 0.5 λ | 2 × 55 |
| 5 | SeaMARC/S (1985) | 150 | 3 | λ | 2 × 45 |
| 6 | SeaMARC/R (1989) | 11, 12 | 2 | 0.5 λ | 2 × 55 |
| 7 | SYSTEM120 (1989) | 120 | 3 | λ | 2 × 50 |
| 8 | SeaMARC TAMU (1990) | 11, 12 | 3 | 0.45 λ | 2 × 65 |
| 9 | SYSTEM09 (1990) | 9, 10 | 2 | 0.8 λ | 2.5 × 65 |
| 10 | GLORI-B (1992) | 6.8, 6.3 | 2 | 0.7 λ | 2.7 × 35 |
| 11 | Deepscan (1999) | 60, 120 | 3 | 0.8 λ | 1.5 × 50 |
| No. | Manufacturer, System Name | Freq. [kHz]1 | No. of Receive Elements | Beam Width [deg. × deg.] | |
|---|---|---|---|---|---|
| 1 | Klein, HydroChart 3500/5000 | 455 | 4/5 | 0.4 × 120 | |
| 2 | ITER Systems, Bathyswath-2 | 117 234 468 | 4 | 0.85 0.55 0.55 | × 140 |
| 3 | Kongsberg, GeoswathPlus | 125 250 500 | 4 | 0.85 0.55 0.55 | × 140 |
| 4 | Teledyne, Benthos C3D 2 | 200 | 6 | 1 × 100 | |
| 5 | Edgetech, 4600 | 230 550 | 8 | 0.64 0.5 | × 100 |
| 6 | Edgetech, 6205/6205s | 230 550 | 10 | 0.7 0.5 | × 100 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grall, P.; Kochanska, I.; Marszal, J. Direction-of-Arrival Estimation Methods in Interferometric Echo Sounding. Sensors 2020, 20, 3556. https://doi.org/10.3390/s20123556
Grall P, Kochanska I, Marszal J. Direction-of-Arrival Estimation Methods in Interferometric Echo Sounding. Sensors. 2020; 20(12):3556. https://doi.org/10.3390/s20123556
Chicago/Turabian StyleGrall, Piotr, Iwona Kochanska, and Jacek Marszal. 2020. "Direction-of-Arrival Estimation Methods in Interferometric Echo Sounding" Sensors 20, no. 12: 3556. https://doi.org/10.3390/s20123556
APA StyleGrall, P., Kochanska, I., & Marszal, J. (2020). Direction-of-Arrival Estimation Methods in Interferometric Echo Sounding. Sensors, 20(12), 3556. https://doi.org/10.3390/s20123556





