Simulation and Test of a Contactless Voltage Measurement Method for Overhead Lines Based on Reconstruction of Integral Node Parameters
Abstract
1. Introduction
2. Measurement System and Algorithm Model
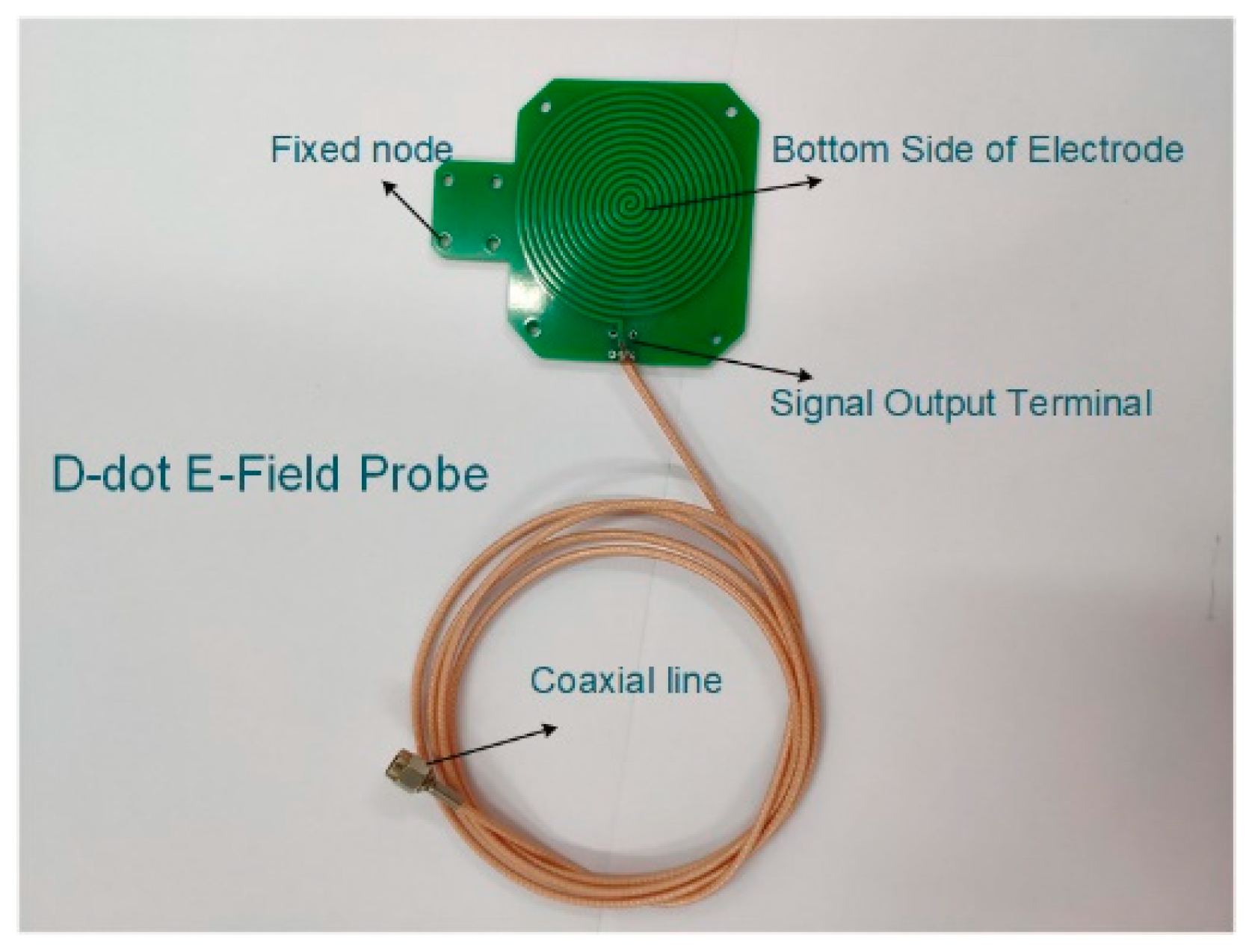
2.1. Detection Terminal
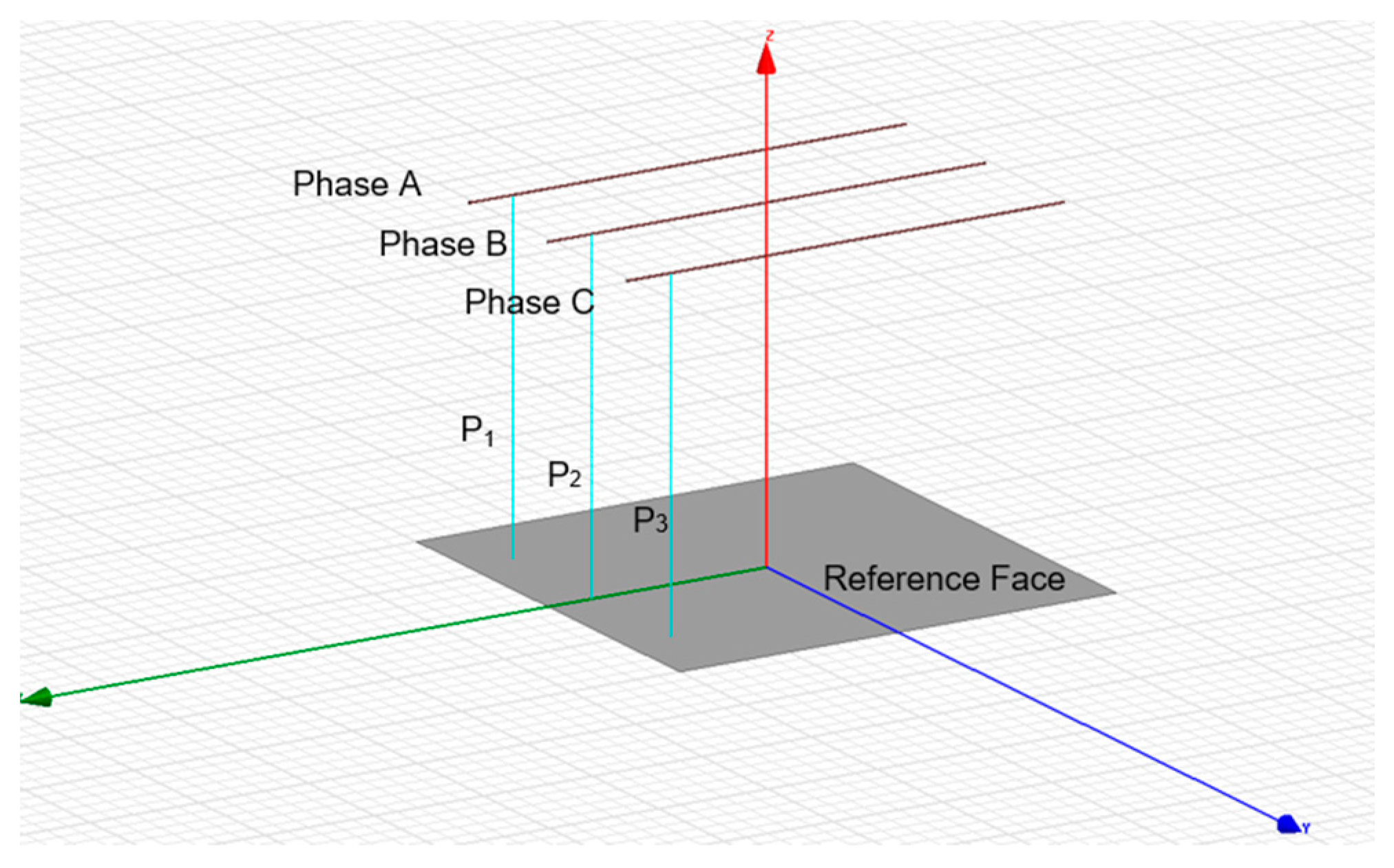
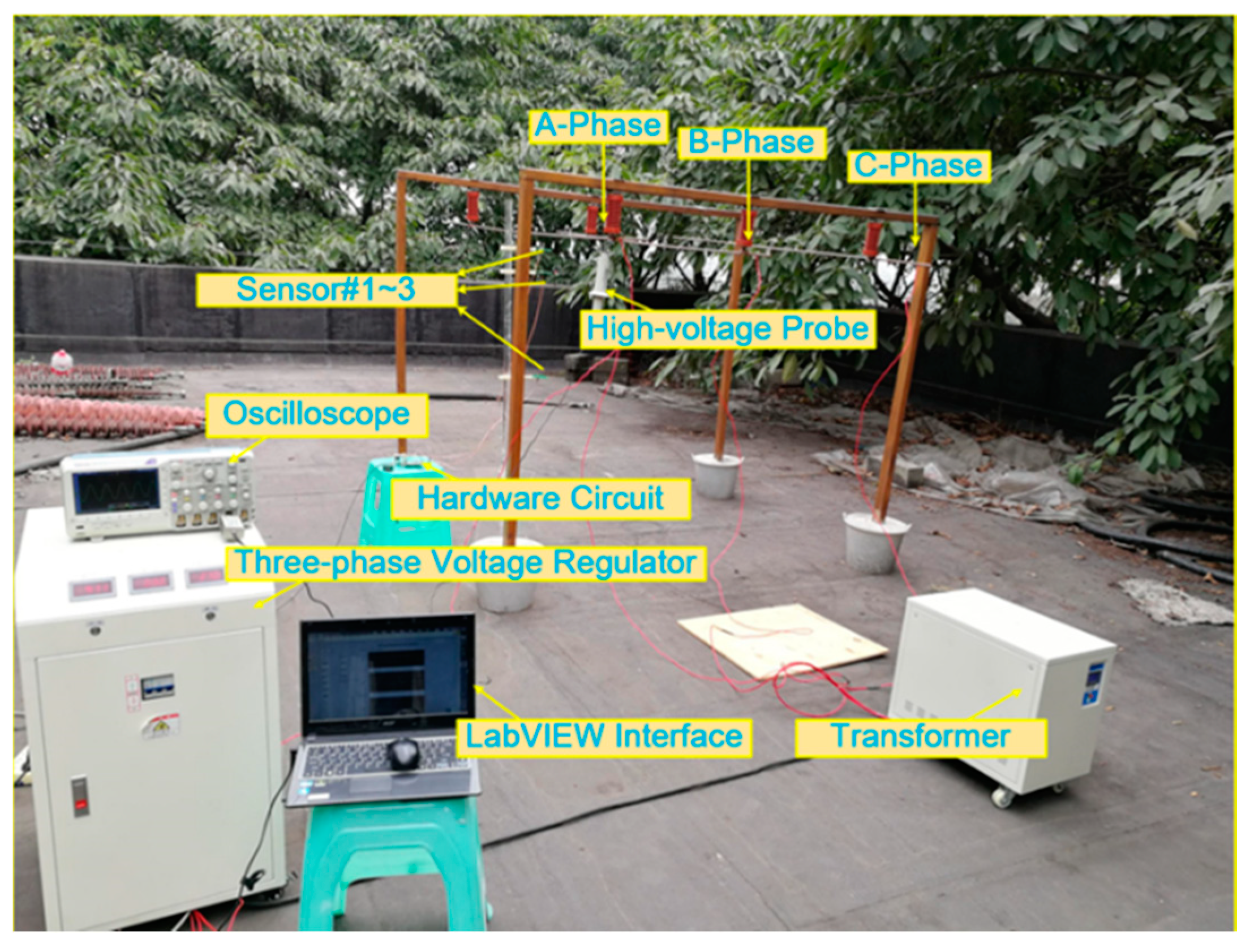
2.2. Construction of the Voltage Measurement System
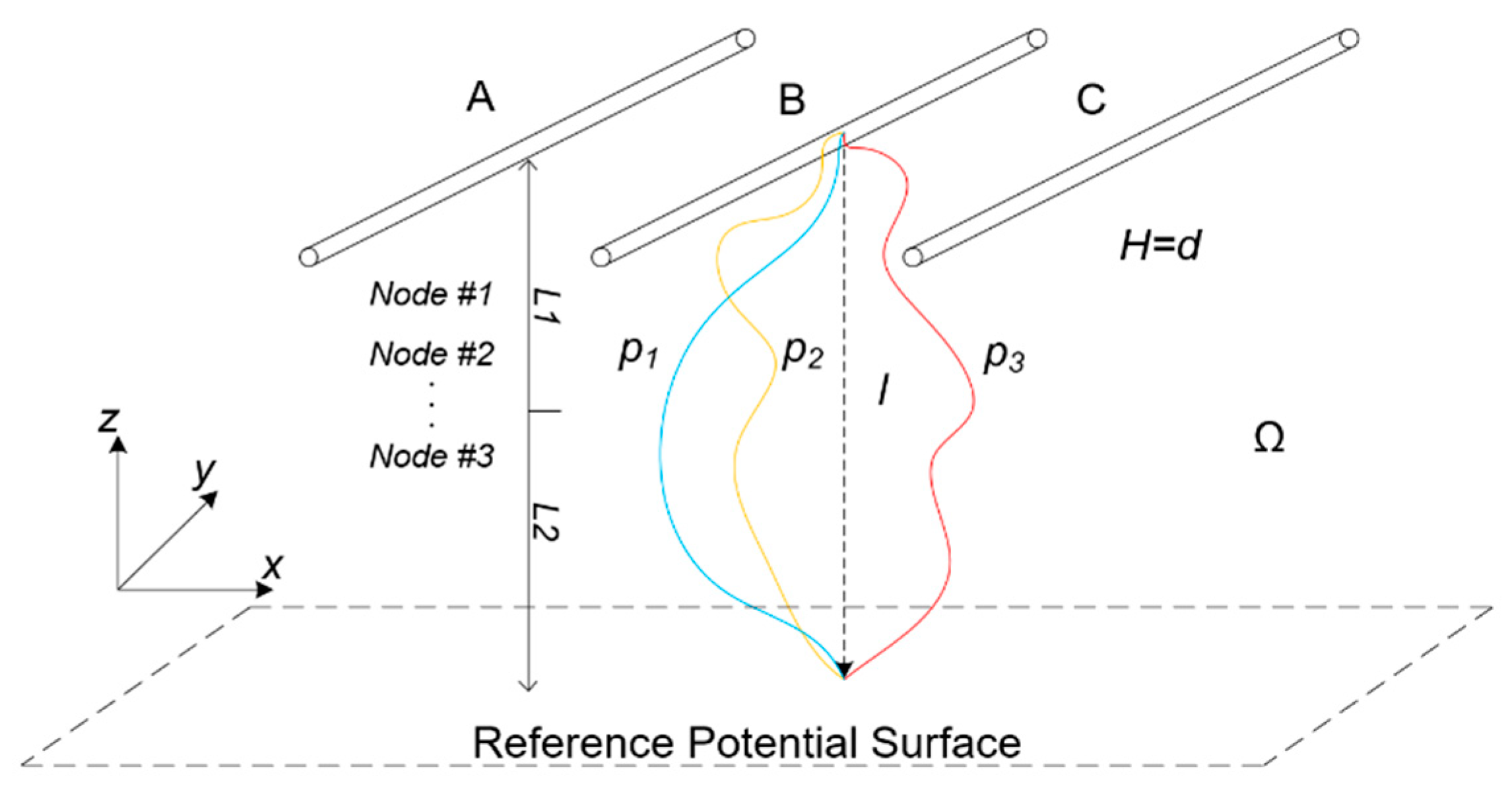
2.3. Node Parameters Reconstruction Algorithm Model
2.3.1. Gauss–Chebyshev Algorithm
2.3.2. Algorithm Model of Node Parameters Reconstruction
3. Simulation Calculation and Test
3.1. Judgment of Reconstruction Factors
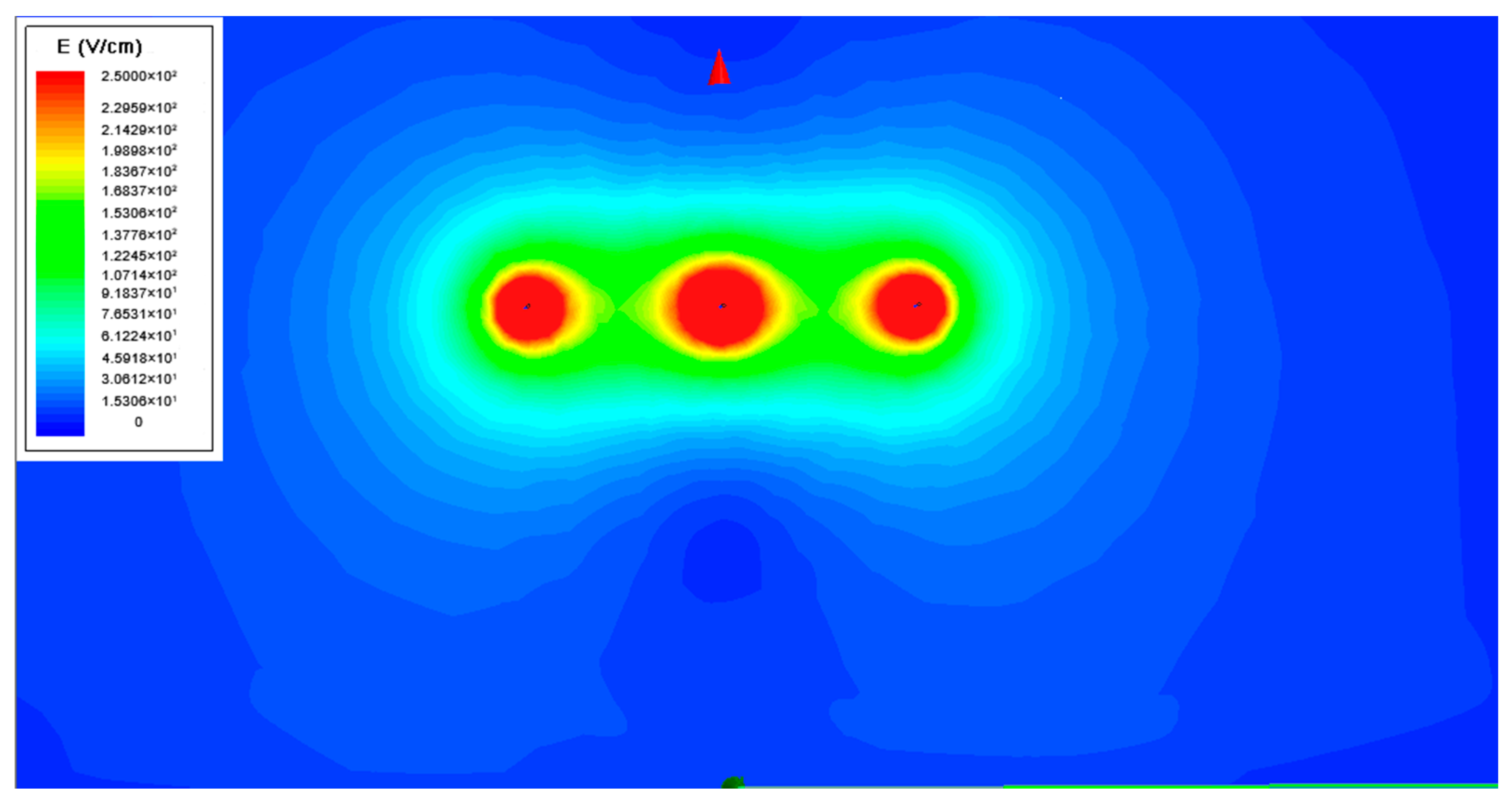
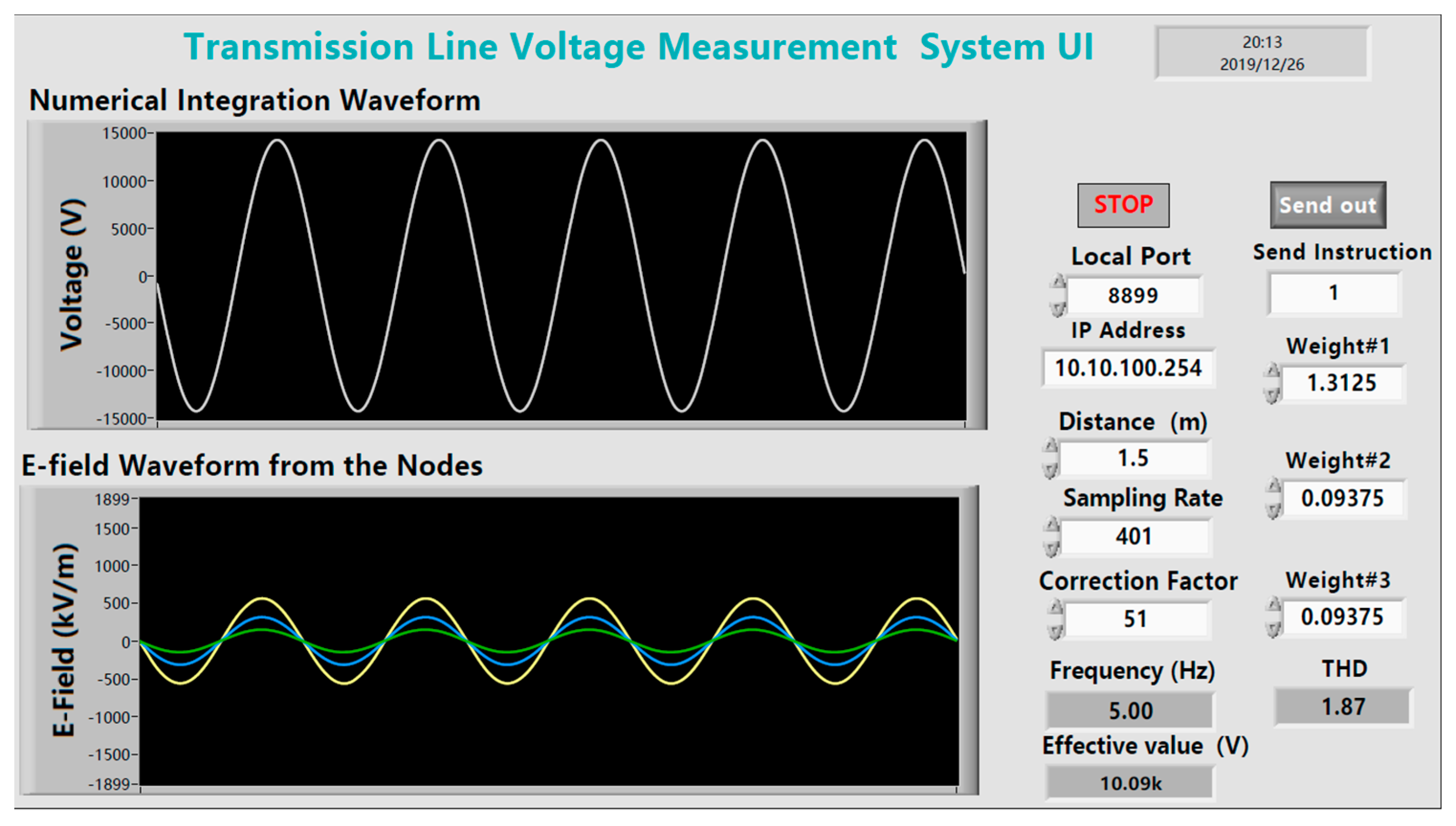
3.2. Simulation Test
4. Experiment and Discussion
- (1)
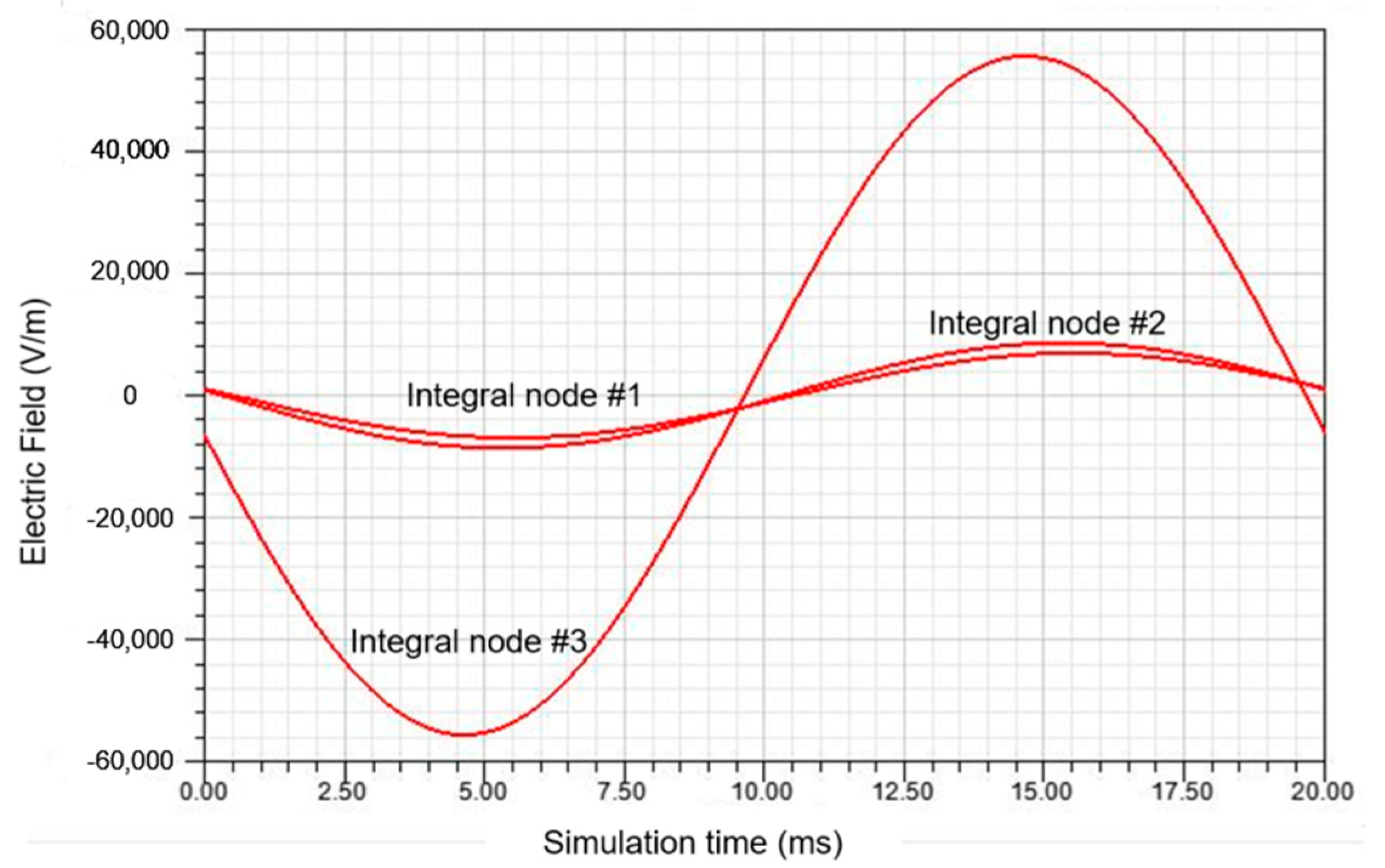
- The parallel distributed D-dot E-field sensor has good output characteristics, and the phase deviation of the E-field signal of each node is less than 1.8°, which ensures the reliability of the E-field parameters at the position of the reconstructed node.
- (2)
- In the node parameter reconstruction simulation calculation model, the weights and the position parameters of the integral nodes are determined by the voltage and height of overhead lines, so the integral algorithm model can match with the overhead line parameters well.
- (3)
- Similar to the simulation test results, the deviation of the voltage measurement system after reconstruction is less than 0.4% in the voltage range of 10–20 kV, which is nearly 10 times smaller than the deviation of the system before the node reconstruction.
- (4)
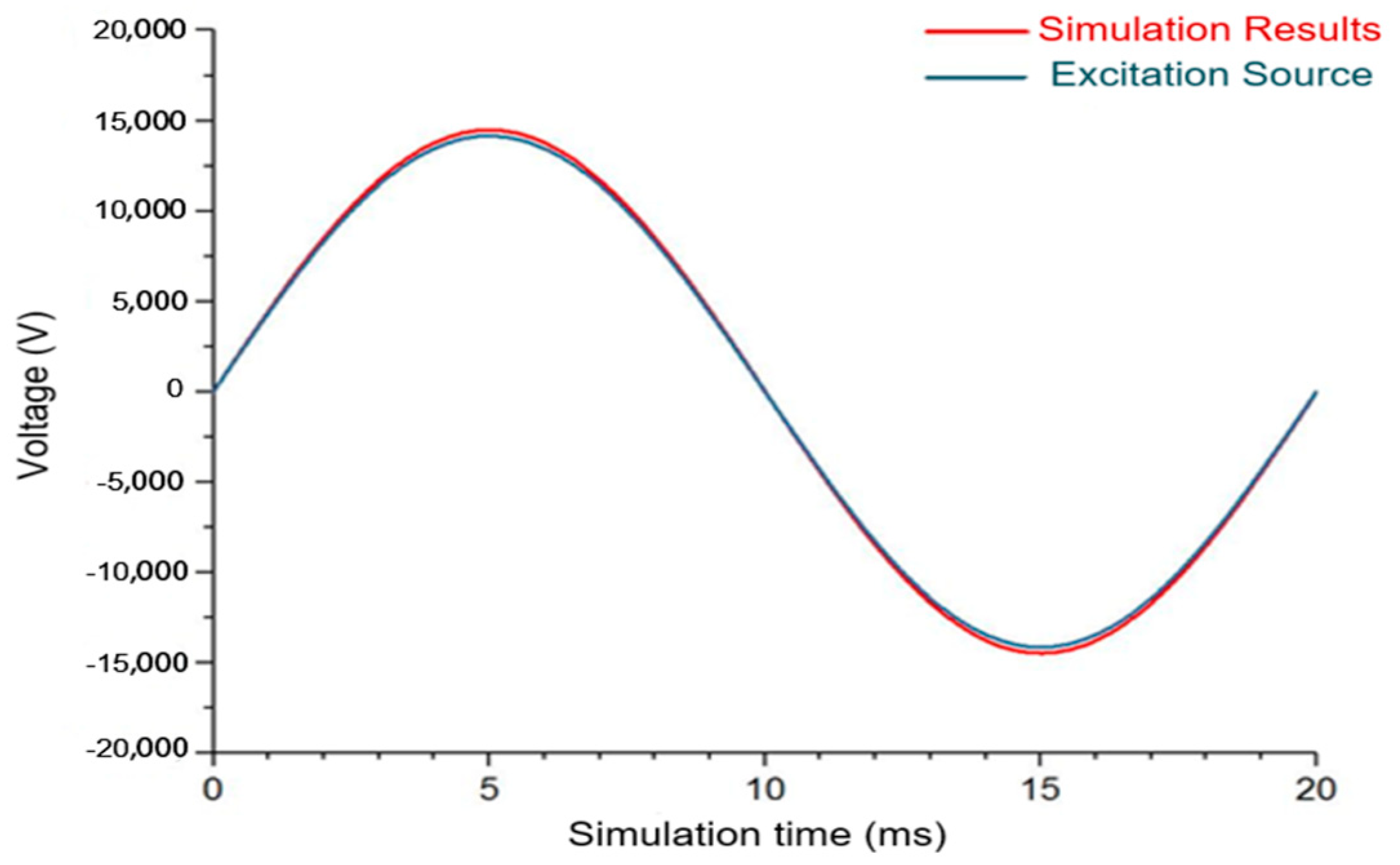
- The E-field waveform, the simulated calculation waveform, and the platform measurement waveform have good consistency, which proves that the reconstructed measurement system is featured by good anti-interference performance.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bakar, A.H.A.; Rahim, N.A.; Zambri, M.K.M. Analysis of lightning-caused ferroresonance in Capacitor Voltage Transformer (CVT). Int. J. Electr. Power Energy Syst. 2011, 33, 1536–1541. [Google Scholar] [CrossRef]
- Liu, X.J.; Zeng, Y.; An, B.P.; Zhang, X.L. Research on Characteristics of ECVT for Power Quality Detection and Optimum Design of Its Parameter. Energies 2019, 12, 2416. [Google Scholar] [CrossRef]
- Meng, Z.; Li, H.; Zhang, C.; Chen, M.; Chen, Q. Research on the reliability of capacitor voltage transformers calibration results. Measurement 2019, 146, 770–779. [Google Scholar] [CrossRef]
- Wang, D.; Li, P.; Wen, Y. Design and modeling of magnetically driven electric-field sensor for non-contact DC voltage measurement in electric power systems. Rev. Sci. Instrum. 2016, 87, 105001. [Google Scholar] [CrossRef] [PubMed]
- Xiao, D.; Liu, H.; Zhou, Q.; Xie, Y.; Ma, Q. Influence and Correction from the Human Body on the Measurement of a Power-Frequency Electric Field Sensor. Sensors 2016, 16, 859. [Google Scholar] [CrossRef]
- Mckenzie, G.; Record, P. Non-contact voltage measurement using electronically varying capacitance. Electron. Lett. 2010, 46, 214. [Google Scholar] [CrossRef]
- Zhou, Q.; He, W.; Xiao, D.; Li, S.; Zhou, K. Study and Experiment on Non-Contact Voltage Sensor Suitable for Three-Phase Transmission Line. Sensors 2016, 16, 40. [Google Scholar] [CrossRef]
- Li, Z.; Yuan, H.; Cui, Y.; Ding, Z.; Zhao, L. Measurement of Distorted Power-Frequency Electric Field with Integrated Optical Sensor. IEEE Trans. Instrum. Meas. 2018, 68, 1132–1139. [Google Scholar] [CrossRef]
- Firth, J.; Brodzeli, Z.; Ciobotaru, M.; Phung, T.; Ladouceur, F.; Silvestri, L. Accurate optical measurement of high voltage waveform using novel optical liquid crystal-based sensor. Sens. Actuators A Phys. 2017, 268, 164–172. [Google Scholar] [CrossRef]
- Lee, D.J.; Lee, Y.S.; Kwon, J.Y.; Jung, J.K. Simple optical transformer system for intense electric field and voltage sensing. IEICE Electron. Express 2015, 12, 20141090. [Google Scholar] [CrossRef]
- Pan, F.; Xiao, X.; Xu, Y.; Ren, S.Y. An Optical AC Voltage Sensor Based on the Transverse Pockels Effect. Sensors 2011, 11, 6593–6602. [Google Scholar] [CrossRef] [PubMed]
- Li, C.S.; Zeng, Z.; He, X.L. Optical voltage sensor using bismuth silicate crystal grown by hydrothermal method. J. Optoelectron. Laser 2014, 2, 239–245. [Google Scholar]
- Zhang, G.G.; Li, W.F.; Qi, L.; Liu, J.C.; Song, Z.X.; Wang, J.H. Design of Wideband GHz Electric Field Sensor Integrated with Optical Fiber Transmission Link for Electromagnetic Pulse Signal Measurement. Sensors 2018, 18, 3167. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Wang, J.; Wei, G.; Yang, Y. Design and Simulation Test of an Open D-Dot Voltage Sensor. Sensors 2015, 15, 23640–23652. [Google Scholar] [CrossRef]
- Wang, K.; Duan, Y.; Shi, L.; Qiu, S. Laboratory Calibration of D-dot Sensor Based on System Identification Method. Sensors 2019, 19, 3255. [Google Scholar] [CrossRef]
- Xiao, D.; Ma, Q.; Xie, Y.; Zheng, Q.; Zhang, Z. A Power-Frequency Electric Field Sensor for Portable Measurement. Sensors 2018, 18, 1053. [Google Scholar] [CrossRef]
- Hu, X.; Wang, J.; Wei, G.; Deng, X. Decomposition of Composite Electric Field in a Three-Phase D-Dot Voltage Transducer Measuring System. Sensors 2016, 16, 1683. [Google Scholar] [CrossRef]
- Wang, J.; Fan, Y.; Zhu, L.; Bai, Y. Design and research of a three-phase voltage sensor based on D-dot principle. IEEJ Trans. Electr. Electron. Eng. 2017, 12, 55–61. [Google Scholar] [CrossRef]
- Tao, Y.; Wang, J.; Xu, Z. Design, simulation and test of a double differential D-dot overvoltage sensor. Int. J. Appl. Electromagn. Mech. 2018, 58, 431–449. [Google Scholar] [CrossRef]
- Zhao, P.; Wang, J.; Wang, Q.; Xiao, Q.; Zhang, R.; Ou, S.; Tao, Y. Simulation, Design, and Test of a Dual-Differential D-Dot Overvoltage Sensor Based on the Field-Circuit Coupling Method. Sensor 2019, 19, 3413. [Google Scholar] [CrossRef]
- Huiskamp, T.; Beckers, F.J.C.M.; Heesch, E.J.M.V.; Pemen, A.J.M. B-Dot and D-Dot Sensors for (Sub)Nanosecond High-Voltage and High-Current Pulse Measurements. IEEE Sens. J. 2016, 16, 3792–3801. [Google Scholar] [CrossRef]
- Hu, G.H.; Li, P.J.; Liu, X.D.; Zhao, Y. Inverse Source Problems in Electrodynamics. Inverse Probl. Imaging 2018, 12, 1411–1428. [Google Scholar] [CrossRef]
- Zhang, Z.; Lei, J.; Xie, X.; Zhao, H.; Zhong, J.; Xiao, D. Optimization algorithm of inverse problem for a power-frequency electric field. Int. Trans. Electr. Energy Syst. 2015, 25, 89–98. [Google Scholar] [CrossRef]
- Wei, S.; Zhang, L.; Gao, W.; Cao, Z. Non-contact Voltage Measurement Based on Electric-Field Effect. Procedia Eng. 2011, 15, 1973–1977. [Google Scholar] [CrossRef]
- Chavez, P.P.; Jaeger, N.A.F.; Rahmatian, F. Accurate Voltage Measurement by the Quadrature Method. IEEE Power Eng. Rev. 2002, 22, 59. [Google Scholar] [CrossRef]
- Rahmatian, F.; Chavez, P.P.; Jaeger, N.A.F. 230 kV Optical Voltage Transducers Using Multiple Electric Field Sensors. IEEE Trans. Power Deliv. 2002, 17, 417–422. [Google Scholar] [CrossRef]
- Si, D.; Wang, J.; Wei, G.; Yan, X. Method and Experimental Study of Voltage Measurement Based on Electric Field Integral with Gauss-Legendre Algorithm. IEEE Trans. Instrum. Meas. 2019. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, Y.; Li, W.; Zeng, X.; Tang, J.; Wang, Y.; Deng, X. Research on Transmission Line Voltage Measurement Method of D-Dot Sensor Based on Gaussian Integral. Sensors 2018, 18, 2455. [Google Scholar] [CrossRef]
- Wang, J.; Li, X.; Ou, S.; Zhang, R. Research on Transmission Line Voltage Measurement Method Based on Improved Gaussian Integral. IEEE Access 2019, 7, 73711–73718. [Google Scholar] [CrossRef]
- Dehghan, M.; Masjed-Jamei, M.; Eslahchi, M.R. On numerical improvement of the Second Kind of Gauss-Chebyshev quadrature rules. Appl. Math. Comput. 2005, 168, 431–446. [Google Scholar] [CrossRef]
- Galybin, A.N. Integral equations and Gauss-Chebyshev quadrature for planar rectangular cracks. Int. J. Mech. Sci. 2018, 146, 272–279. [Google Scholar] [CrossRef]
- Wang, J.; Gao, L. Research on the Algorithm and Test of Transmission Line Voltage Measurement Based on Electric Field Integral Method. IEEE Access 2018, 6, 72766–72773. [Google Scholar] [CrossRef]


| n | An | t1/x1 | t2/x2 | t3/x3 | t4/x4 | t5/x5 |
|---|---|---|---|---|---|---|
| 2 | 1.5708 | 0.7071/0.8535d | −0.7071/0.1465d | |||
| 3 | 1.0472 | 0.8660/0.9330d | 0/0.5000d | −0.8660/0.0670d | ||
| 4 | 0.785 | 0.9239/0.9620d | 0.3827/0.6914d | −0.3827/0.3087d | −0.9239/0.0381d | |
| 5 | 0.628 | 0.9755/0.9878d | 0.5878/0.7939d | 0/0.5000d | −0.5878/0.2061d | −0.9755/0.0245d |
| m | A1j/A2j | x11/x21 | x12/x22 | x13/x23 | x14/x24 |
|---|---|---|---|---|---|
| 1 | 0.8750d/0.1250d | 0.4375d/0.9375d | |||
| 2 | 0.4375d/0.0625d | 0.1849d/0.9014d | 0.6901d/0.9736d | ||
| 3 | 0.2917d/0.0417d | 0.1281d/0.8933d | 0.4375d/0.9375d | 0.7469d/0.9817d | |
| 4 | 0.2188d/0.0313d | 0.0898d/0.8878d | 0.3554d/0.9258d | 0.5196d/0.9492d | 0.7852d/0.9872d |
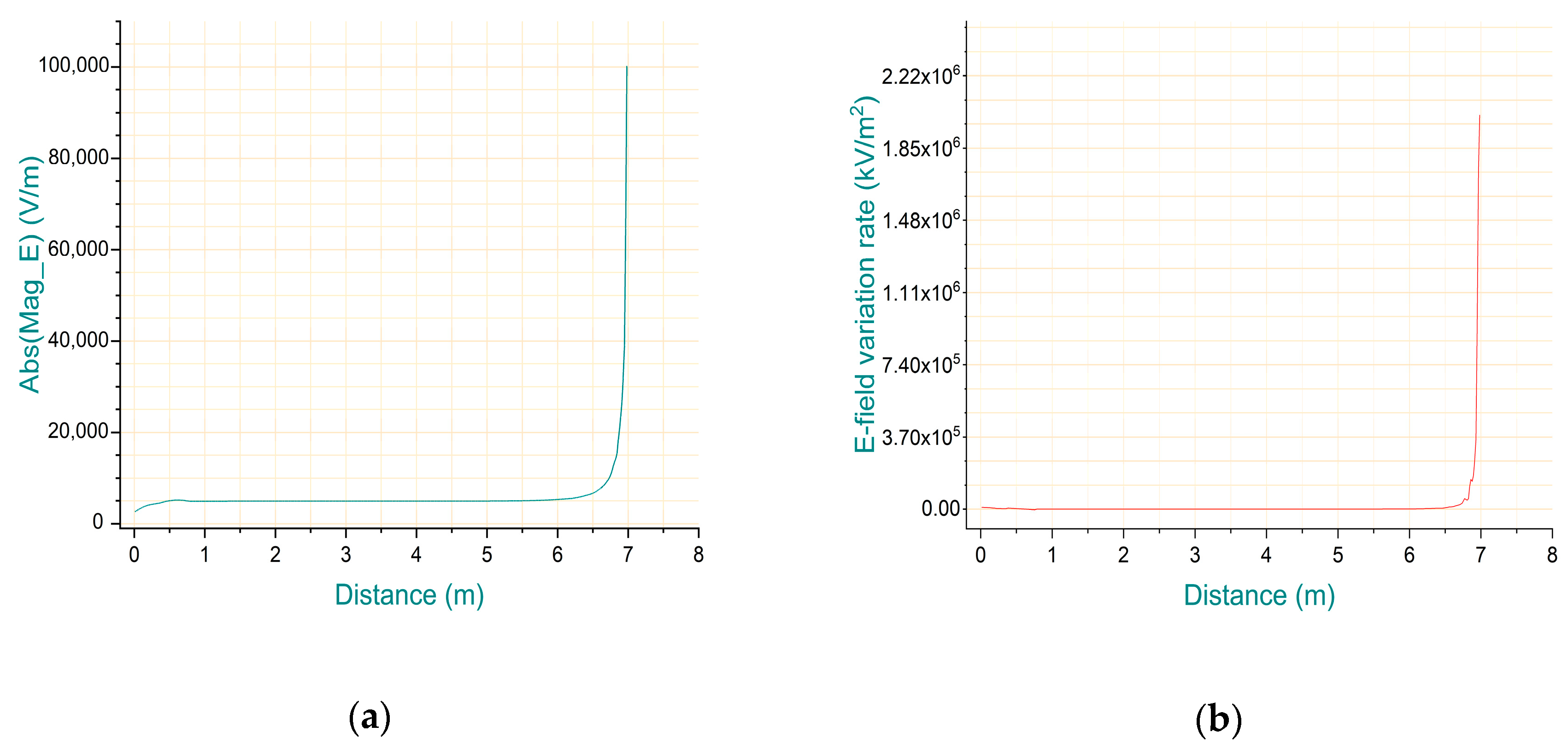
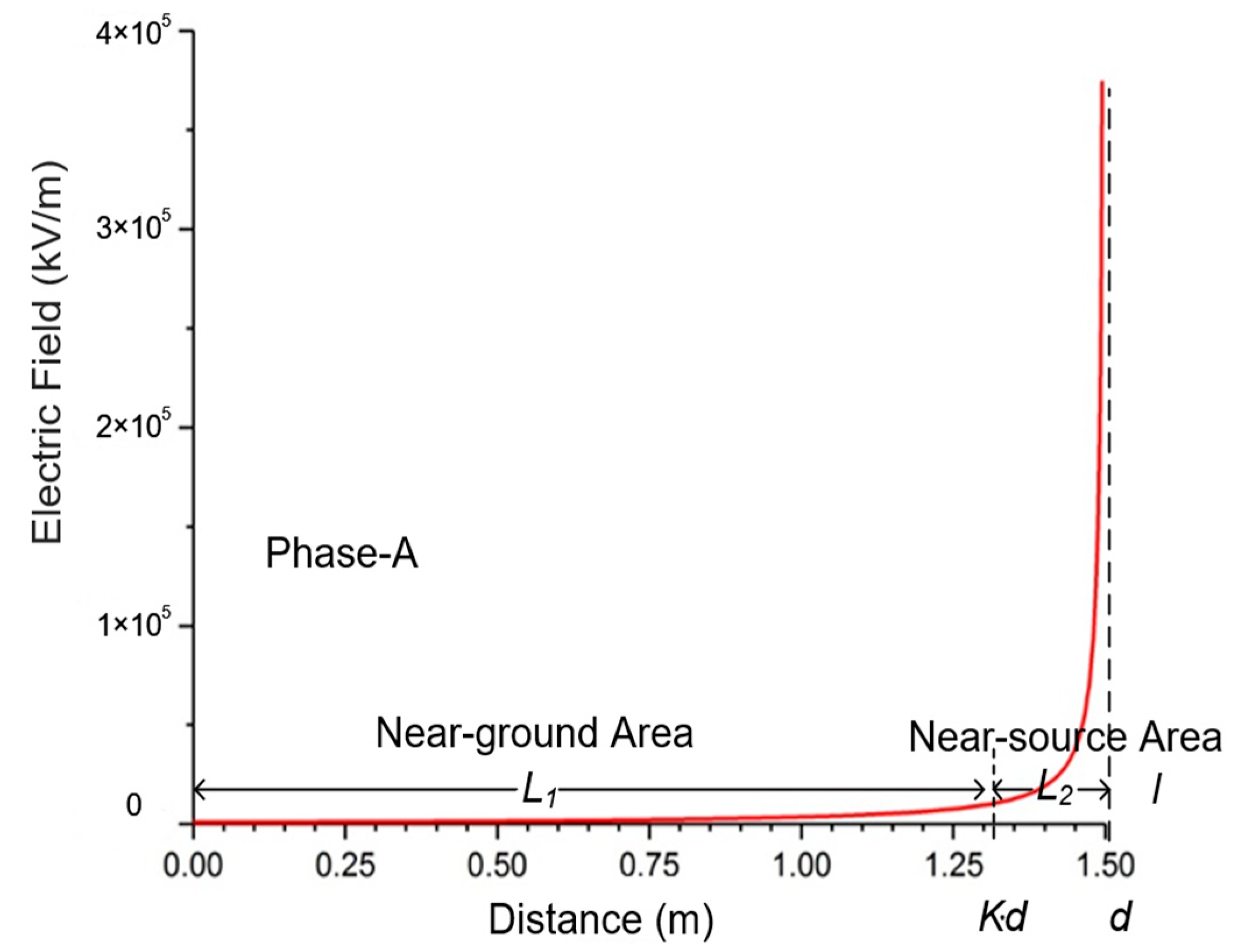
| Voltage Level VL (kV) | Lead Height d (m) | Partition Position (m) | E-Field Values upon the Partition Sites (V/m) | E-Field Variation Rate upon the Partition Sites (kV/m2) | Reconstruction Factor k |
|---|---|---|---|---|---|
| 10 | 6.5 | 5.785 | 1672.044 | 2.131 | 0.890 |
| 35 | 7.0 | 6.175 | 5481.911 | 2.358 | 0.884 |
| 66 | 7.0 | 6.035 | 9955.669 | 2.753 | 0.862 |
| 110 | 7.0 | 5.982 | 16,429.093 | 2.224 | 0.854 |
| Interval | Node No. | Position (m) | Weights |
|---|---|---|---|
| L1 | 1 | 0.656 | 1.3125 |
| L2 | 2 | 1.352 | 0.09375 |
| 3 | 1.460 | 0.09375 |
| Vr (kV) | Vc (kV) | ε% | Vn (kV) | ε% |
|---|---|---|---|---|
| 10.1 | 9.74 | 3.564 | 10.06 | 0.396 |
| 11.9 | 11.55 | 2.941 | 11.86 | 0.337 |
| 14.1 | 13.49 | 4.326 | 14.05 | 0.355 |
| 16.0 | 15.45 | 3.438 | 15.94 | 0.375 |
| 18.1 | 17.73 | 2.044 | 18.03 | 0.387 |
| 20.2 | 19.6 | 2.970 | 20.13 | 0.347 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Yan, X.; Zhong, L.; Zhu, X. Simulation and Test of a Contactless Voltage Measurement Method for Overhead Lines Based on Reconstruction of Integral Node Parameters. Sensors 2020, 20, 246. https://doi.org/10.3390/s20010246
Wang J, Yan X, Zhong L, Zhu X. Simulation and Test of a Contactless Voltage Measurement Method for Overhead Lines Based on Reconstruction of Integral Node Parameters. Sensors. 2020; 20(1):246. https://doi.org/10.3390/s20010246
Chicago/Turabian StyleWang, Jingang, Xiaojun Yan, Lu Zhong, and Xiaobao Zhu. 2020. "Simulation and Test of a Contactless Voltage Measurement Method for Overhead Lines Based on Reconstruction of Integral Node Parameters" Sensors 20, no. 1: 246. https://doi.org/10.3390/s20010246
APA StyleWang, J., Yan, X., Zhong, L., & Zhu, X. (2020). Simulation and Test of a Contactless Voltage Measurement Method for Overhead Lines Based on Reconstruction of Integral Node Parameters. Sensors, 20(1), 246. https://doi.org/10.3390/s20010246







