An Inverse Approach of Damage Identification Using Lamb Wave Tomography
Abstract
:1. Introduction
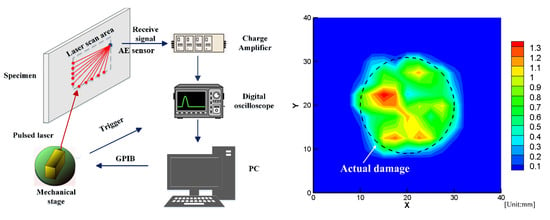
2. Experimental Scheme
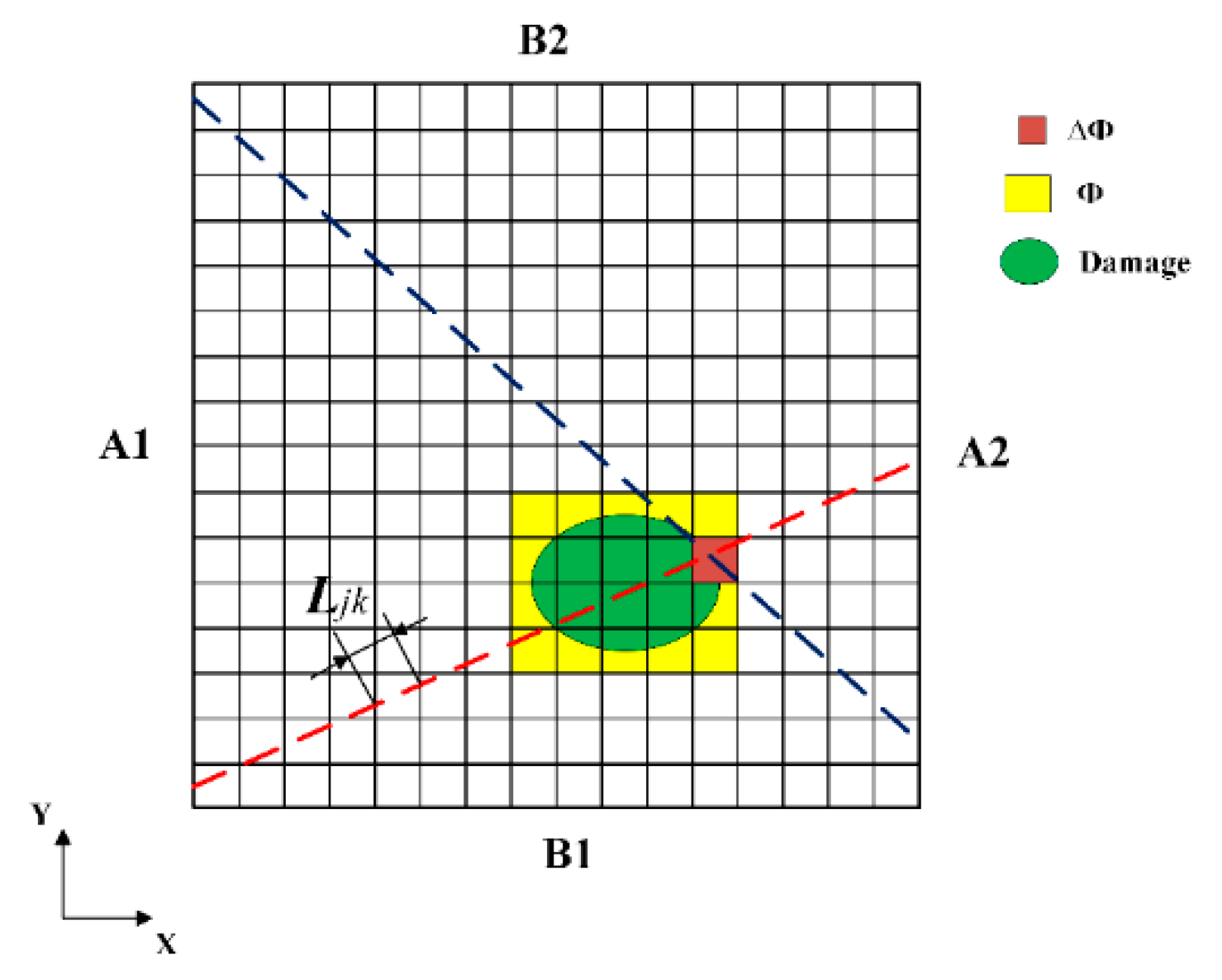
3. Reconstruction Algorithm
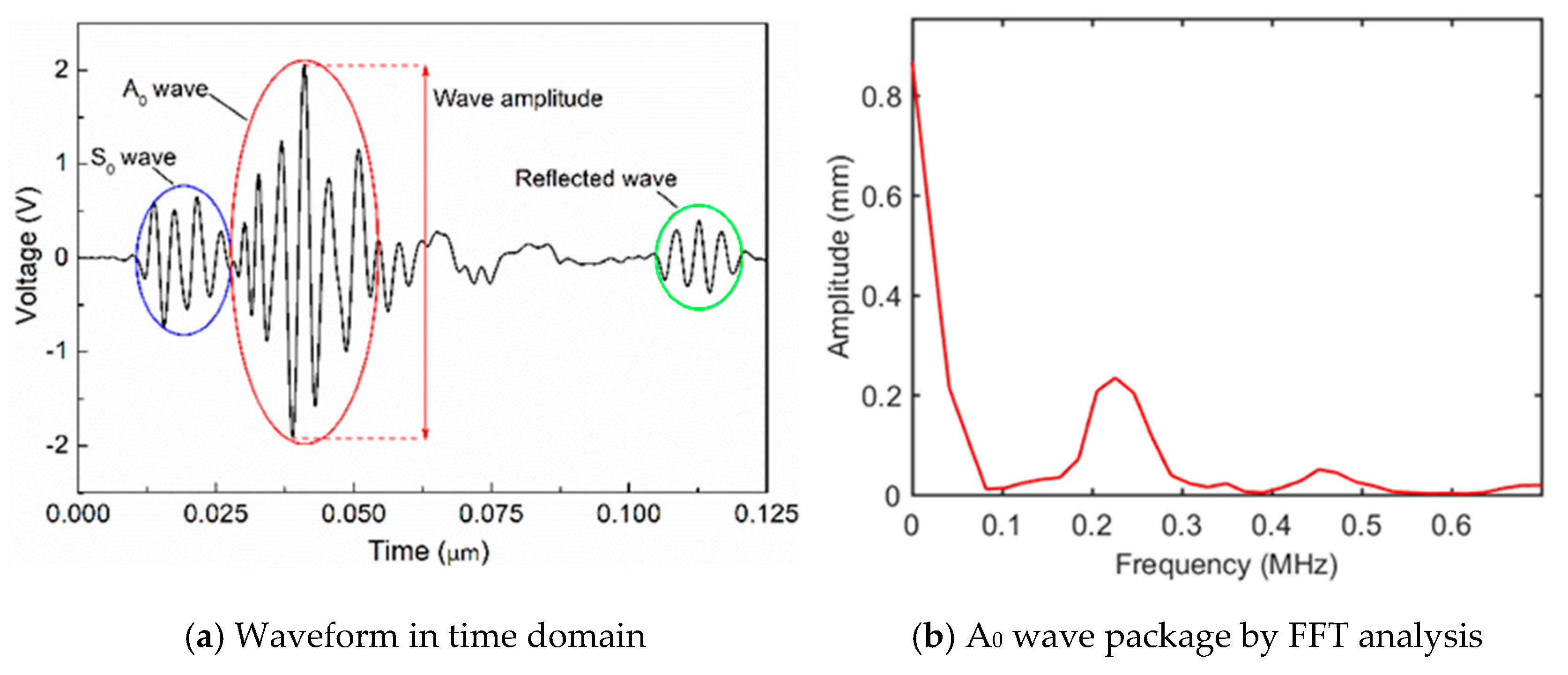
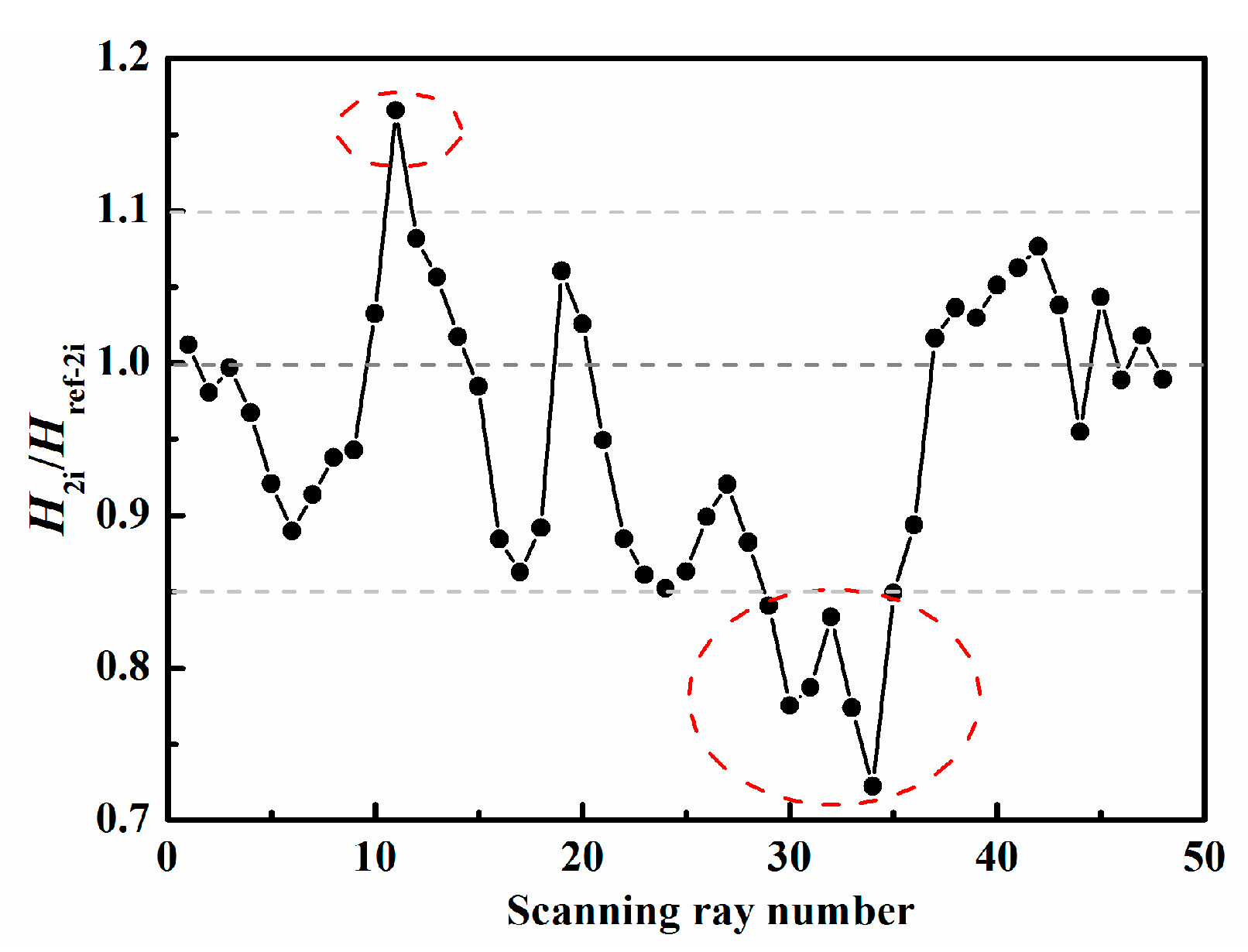
4. Results and Discussion
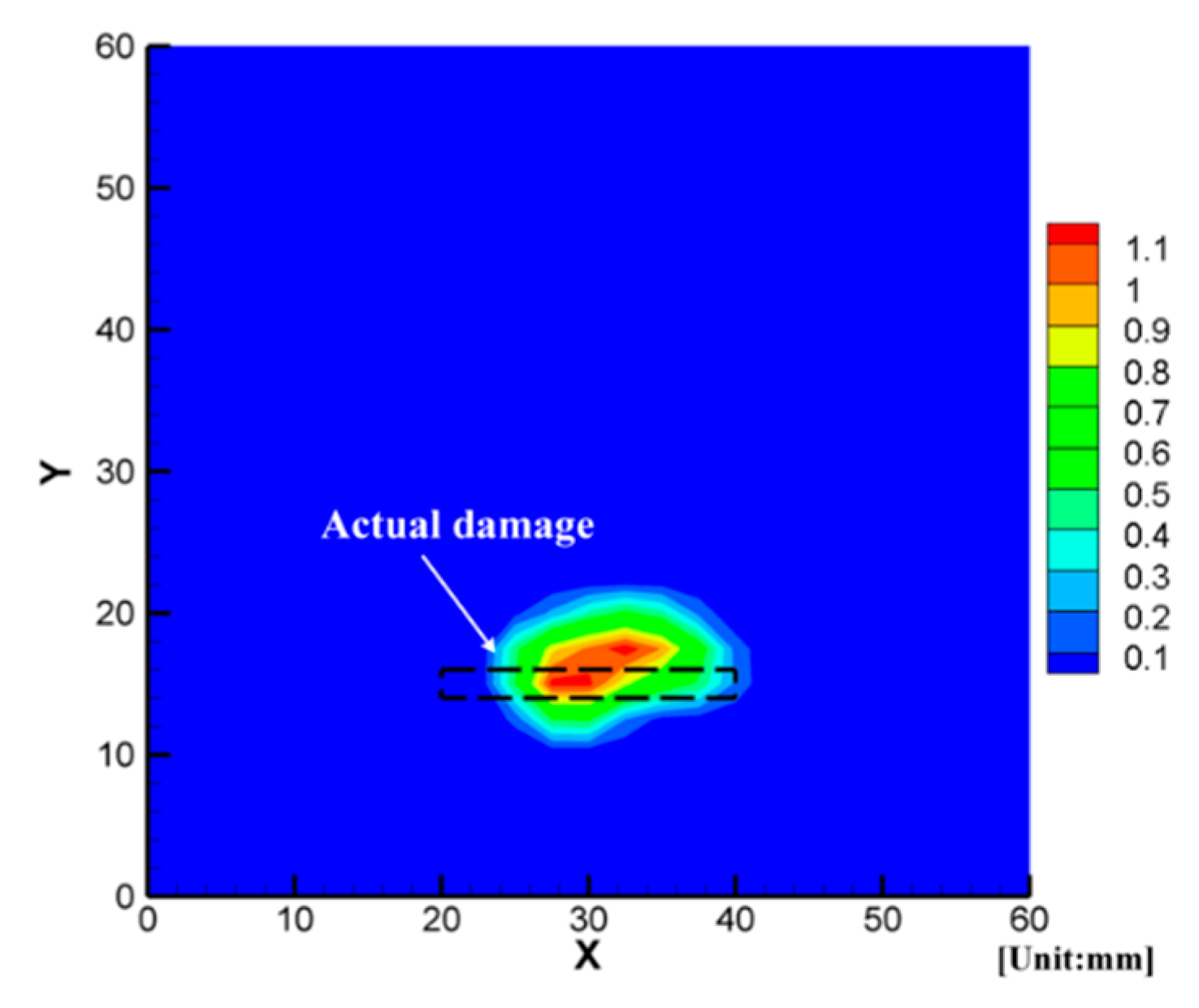
4.1. An Aluminum Plate with a Non-Penetrating Notch
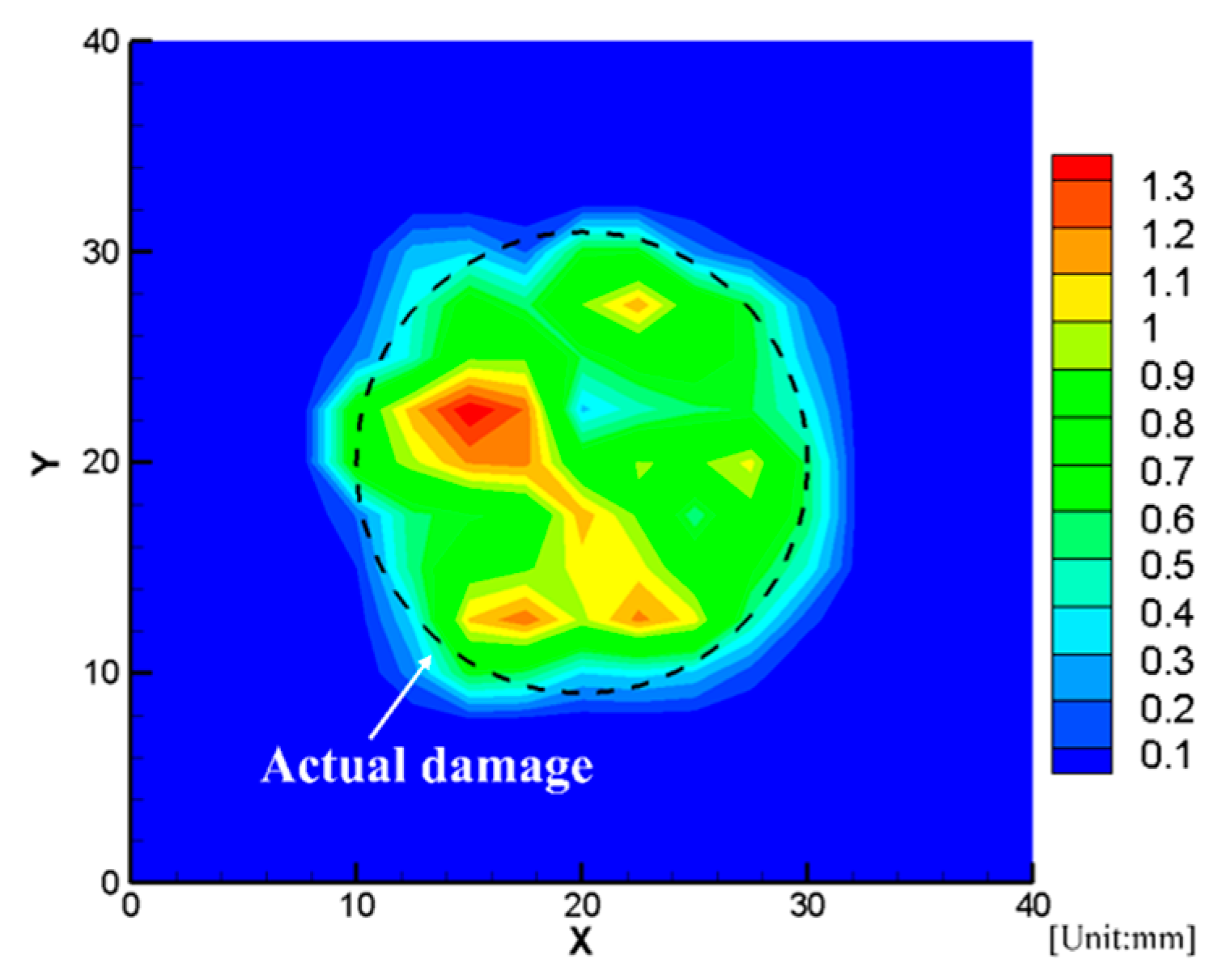
4.2. A CFRP Laminated Plate with Impact-Induced Internal Delamination
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Tiwari, K.A.; Raisutis, R.; Samaitis, V. Hybrid signal processing technique to improve the defect estimation in ultrasonic non-destructive testing of composite structures. Sensors 2017, 17, 2858. [Google Scholar] [CrossRef]
- Tiwari, K.A.; Raisutis, R. Identification and characterization of defects in glass fiber reinforced plastic by refining the guided Lamb waves. Materials 2018, 11, 1173. [Google Scholar] [CrossRef] [PubMed]
- Zeng, L.; Huang, L.; Lin, J. Damage imaging of composite structures using multipath scattering Lamb waves. Compos. Struct. 2019, 216, 331–339. [Google Scholar] [CrossRef]
- Eremin, A.; Golub, M.; Glushkov, E.; Glushkova, N. Identification of delamination based on the Lamb wave scattering resonance frequencies. NDT E Int. 2019, 103, 145–153. [Google Scholar] [CrossRef]
- Grangeat, P. Tomography; Wiley: New York, NY, USA, 2013. [Google Scholar]
- Nathan, B.; Vladimir, Y. Electromagnetic and Acoustic Wave Tomography: Direct and Inverse Problems in Practical Applications; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Jansen, D.P.; Hutchins, D.A. Lamb wave tomography. In Proceedings of the IEEE Ultrasonics Symposium, Honolulu, HI, USA, 4–7 December 1990; pp. 1017–1020. [Google Scholar]
- Su, Z.; Lin, Y. Identification of Damage using Lamb Waves: From Fundamentals to Applications; Springer: London, UK, 2009. [Google Scholar]
- Hutchins, D.; Jansen, D.; Edwards, C. Lamb-wave tomography using non-contact transduction. Ultrasonics 1993, 31, 97–103. [Google Scholar] [CrossRef]
- Jansen, D.; Hutchins, D.; Mottram, J. Lamb wave tomography of advanced composite laminates containing damage. Ultrasonics 1994, 32, 83–90. [Google Scholar] [CrossRef]
- McKeon, J.C.P.; Hinders, M.K. Parallel projection and crosshole Lamb wave contact scanning tomography. J. Acoust. Soc. Am. 1999, 106, 2568–2577. [Google Scholar] [CrossRef]
- Malyarenko, E.V.; Hinders, M.K. Fan beam and double crosshole Lamb wave tomography for mapping flaws in aging aircraft structures. J. Acoust. Soc. Am. 2000, 108, 1631–1639. [Google Scholar] [CrossRef]
- Leonard, K.R.; Malyarenko, E.V.; Hinders, M.K. Ultrasonic Lamb wave tomography. Inverse Probl. 2002, 18, 1795–1808. [Google Scholar] [CrossRef]
- Hou, J.; Leonard, K.R.; Hinders, M.K. Automatic multi-mode Lamb wave arrival time extraction for improved tomographic reconstruction. Inverse Probl. 2004, 20, 1873–1888. [Google Scholar] [CrossRef]
- Leonard, K.R.; Hinders, M.K. Lamb wave tomography of pipe-like structures. Ultrasonics 2005, 43, 574–583. [Google Scholar] [CrossRef]
- Huang, S.; Zhang, Y.; Wang, S.; Zhao, W.; Jiang, X. Multi-mode electromagnetic ultrasonic Lamb wave tomography imaging for variable-depth defects in metal plates. Sensors 2016, 16, 628. [Google Scholar] [CrossRef]
- Chen, L.; Xiao, Q.; Liang, W.; Hong, J.; Zou, X. A time-of-flight revising approach to improve the image quality of Lamb wave tomography for the detection of defects in composite panels. Sci. Eng. Compos. Mater. 2018, 25, 587–592. [Google Scholar] [CrossRef]
- Seher, M.; Huthwaite, P.; Lowe, M.J.S. Experimental studies of the inspection of areas with restricted access using A0 Lamb wave tomography. IEEE Trans. Ultrason. Ferroelectr. Freq. 2016, 63, 1455–1467. [Google Scholar] [CrossRef]
- Zhao, J.; Miao, X.; Li, F.; Li, H. Probabilistic diagnostic algorithm-based damage detection for plates with non-uniform sections using the improved weight function. J. Vib. Eng. Technol. 2018, 6, 249–260. [Google Scholar] [CrossRef]
- Malyarenko, E.V.; Hinders, M.K. Ultrasonic Lamb wave diffraction tomography. Ultrasonics 2001, 39, 269–281. [Google Scholar] [CrossRef]
- Belanger, P.; Cawley, P.; Simonetti, F. Guided wave diffraction tomography within the born approximation. IEEE Trans. Ultrason. Ferroelectr. Freq. 2010, 57, 1405–1418. [Google Scholar] [CrossRef]
- Ng, C.-T. A two-stage approach for quantitative damage imaging in metallic plates using Lamb waves. Earthq. Struct. 2015, 8, 821–841. [Google Scholar] [CrossRef]
- Chan, E.; Rose, L.R.F.; Wang, C.H. An extended diffraction tomography method for quantifying structural damage using numerical Green’s functions. Ultrasonics 2015, 59, 1–13. [Google Scholar] [CrossRef]
- Ren, Y.; Qiu, L.; Yuan, S.; Su, Z.Q. A diagnostic imaging approach for online characterization of multi-impact in aircraft composite structures based on a scanning spatial-wavenumber filter of guided wave. Mech. Syst. Signal Process. 2017, 90, 44–63. [Google Scholar] [CrossRef]
- Tant, K.M.M.; Galetti, E.; Mulholland, A.J.; Curtis, A.; Gachagan, A. A transdimensional Bayesian approach to ultrasonic travel-time tomography for non-destructive testing. Inverse Probl. 2018, 34, 095002. [Google Scholar] [CrossRef]
- Qiu, L.; Yuan, S.; Zhang, X.; Wang, Y. A time reversal focusing based impact imaging method and its evaluation on complex composite structures. Smart Mater. Struct. 2011, 20, 105014. [Google Scholar] [CrossRef]
- Morii, M.; Hu, N.; Fukunaga, H.; Li, J.H.; Liu, Y.L.; Atobe, S.; Alamusi; Qiu, J.H. A new inverse algorithm for tomographic reconstruction of damage images using Lamb waves. Comput. Mater. Con. 2011, 26, 37–66. [Google Scholar]
- Hu, B.; Hu, N.; Li, L.; Li, W.; Tang, S.; Li, Y.; Peng, X.; Homma, A.; Liu, Y.; Wu, L.; et al. Tomographic reconstruction of damage images in hollow cylinders using Lamb waves. Ultrasonics 2014, 54, 2015–2023. [Google Scholar] [CrossRef]
- Zhou, C.; Su, Z.Q.; Cheng, L. Probability-based diagnostic imaging using hybrid features extracted from ultrasonic Lamb wave signals. Smart Mater. Struct. 2011, 20, 125005. [Google Scholar] [CrossRef]
- Stark, H.; Woods, J.; Paul, I.; Hingorani, R. Direct Fourier reconstruction in computer tomography. IEEE Trans. Acoust. Speech Signal Process. 1981, 29, 237–245. [Google Scholar] [CrossRef]
- Zhuang, T.; Leng, S.; Nett, E.B. Fan-beam and cone-beam image reconstruction via filtering the back-projection image of differentiated projection data. Phys. Med. Boil. 2004, 49, 5489–5503. [Google Scholar] [CrossRef]
- Cebeiro, J.; Morvidone, M.; Nguyen, M.K. Back-projection inversion of a conical radon transform. Inverse Probl. Sci. Eng. 2016, 24, 328–352. [Google Scholar] [CrossRef]
- Gordon, R.; Bender, R.; Herman, G.T. Algebraic Reconstruction Techniques (ART) for three-dimensional electron microscopy and X-ray photography. J. Theor. Biol. 1970, 29, 471–481. [Google Scholar] [CrossRef]
- Gilbert, P. Iterative methods for the three-dimensional reconstruction of an object from projections. J. Theor. Biol. 1972, 36, 105–117. [Google Scholar] [CrossRef]
- Rosalie, C.; Chan, A.; Chiu, W.K.; Galea, S.C.; Rose, F.; Rajic, N. Structural health monitoring of composite structures using stress wave methods. Compos. Struct. 2005, 67, 157–166. [Google Scholar] [CrossRef]
- Kazantsev, D.; Pickalov, V. New iterative reconstruction methods for fan-beam tomography. Inverse Probl. Sci. Eng. 2018, 26, 773–791. [Google Scholar] [CrossRef]
- Zhao, Z.; Chang, F.; Li, B. Ultrasonic CT reconstruction based on improved maximum likelihood expectation maximization algorithm. J. Electron. Meas. Instrum. 2016, 30, 1327–1332. [Google Scholar]
- Zhang, F.; Zhang, Q.; Cui, X.; Dong, C.; Liu, Y.; Liu, Y.; Sun, W.; Gui, Z. Low-dose computed tomography reconstruction algorithm based on patch similarity and maximum likelihood expectation maximization. J. Comput. Appl. 2014, 9, 2716–2719. [Google Scholar]


 : Laser position,
: Laser position,  : Sensor).
: Sensor).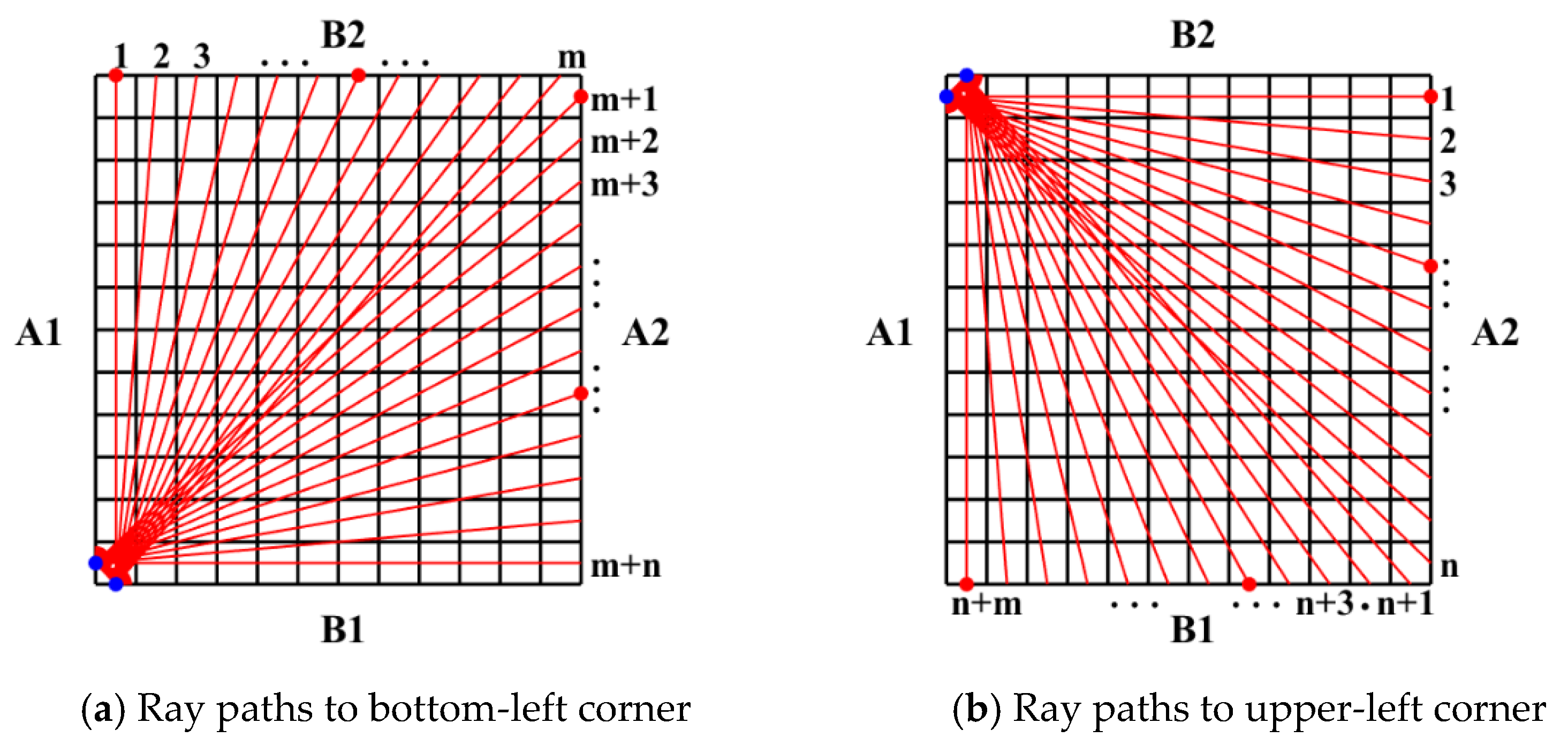







| Young’s Modulus E (Gpa) | Poisson’s Ratio v | ρ (kg/m3) |
|---|---|---|
| 70 | 0.35 | 2700 |
| E1 (Gpa) | E2 (Gpa) | v12 | v23 | G12 (Gpa) | ρ (kg/m3) |
|---|---|---|---|---|---|
| 135 | 10.5 | 0.25 | 0.4 | 5.5 | 1489 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Zhou, S.; Ning, H.; Yan, C.; Hu, N. An Inverse Approach of Damage Identification Using Lamb Wave Tomography. Sensors 2019, 19, 2180. https://doi.org/10.3390/s19092180
Liu Y, Zhou S, Ning H, Yan C, Hu N. An Inverse Approach of Damage Identification Using Lamb Wave Tomography. Sensors. 2019; 19(9):2180. https://doi.org/10.3390/s19092180
Chicago/Turabian StyleLiu, Yaolu, Shijie Zhou, Huiming Ning, Cheng Yan, and Ning Hu. 2019. "An Inverse Approach of Damage Identification Using Lamb Wave Tomography" Sensors 19, no. 9: 2180. https://doi.org/10.3390/s19092180
APA StyleLiu, Y., Zhou, S., Ning, H., Yan, C., & Hu, N. (2019). An Inverse Approach of Damage Identification Using Lamb Wave Tomography. Sensors, 19(9), 2180. https://doi.org/10.3390/s19092180