Online Monitoring of Moisture Diffusion in Carbon Fiber Composites Using Miniaturized Flexible Material Integrated Sensors
Abstract
1. Introduction
2. Materials and Methods
2.1. Sensor Fabrication
2.2. Composite Fabrication
2.3. Moisture Measurements
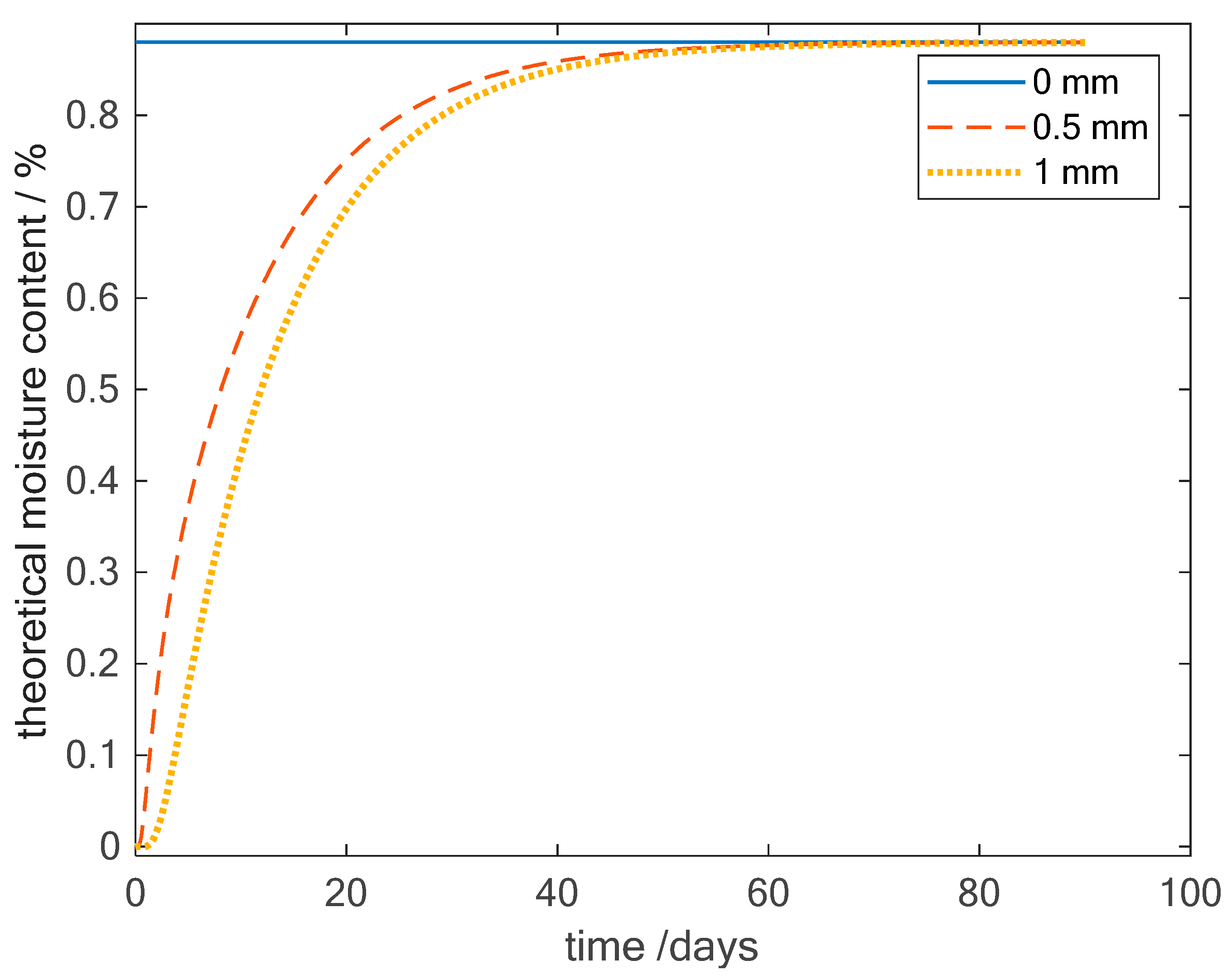
2.4. Theory of Moisture Diffusion
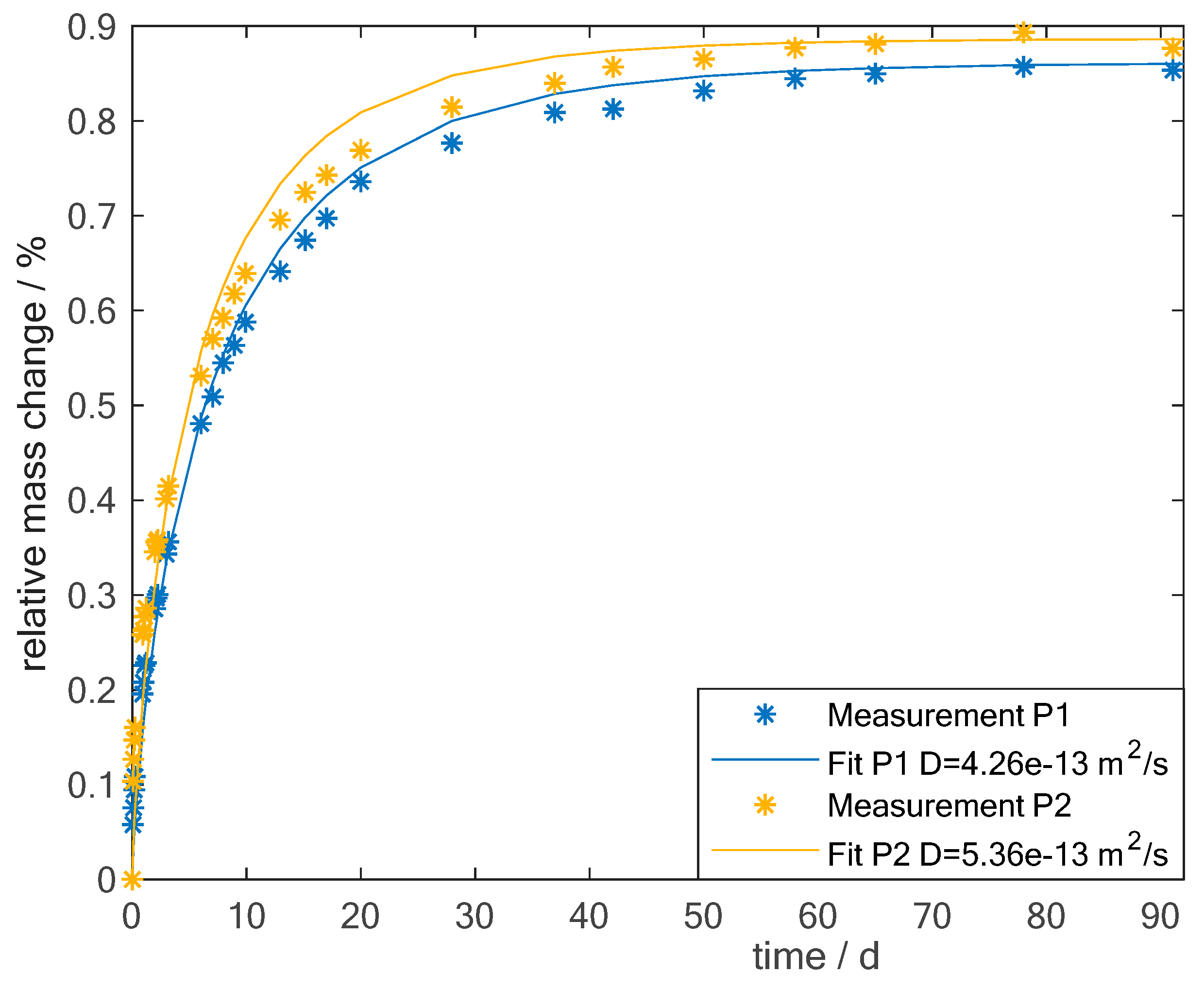
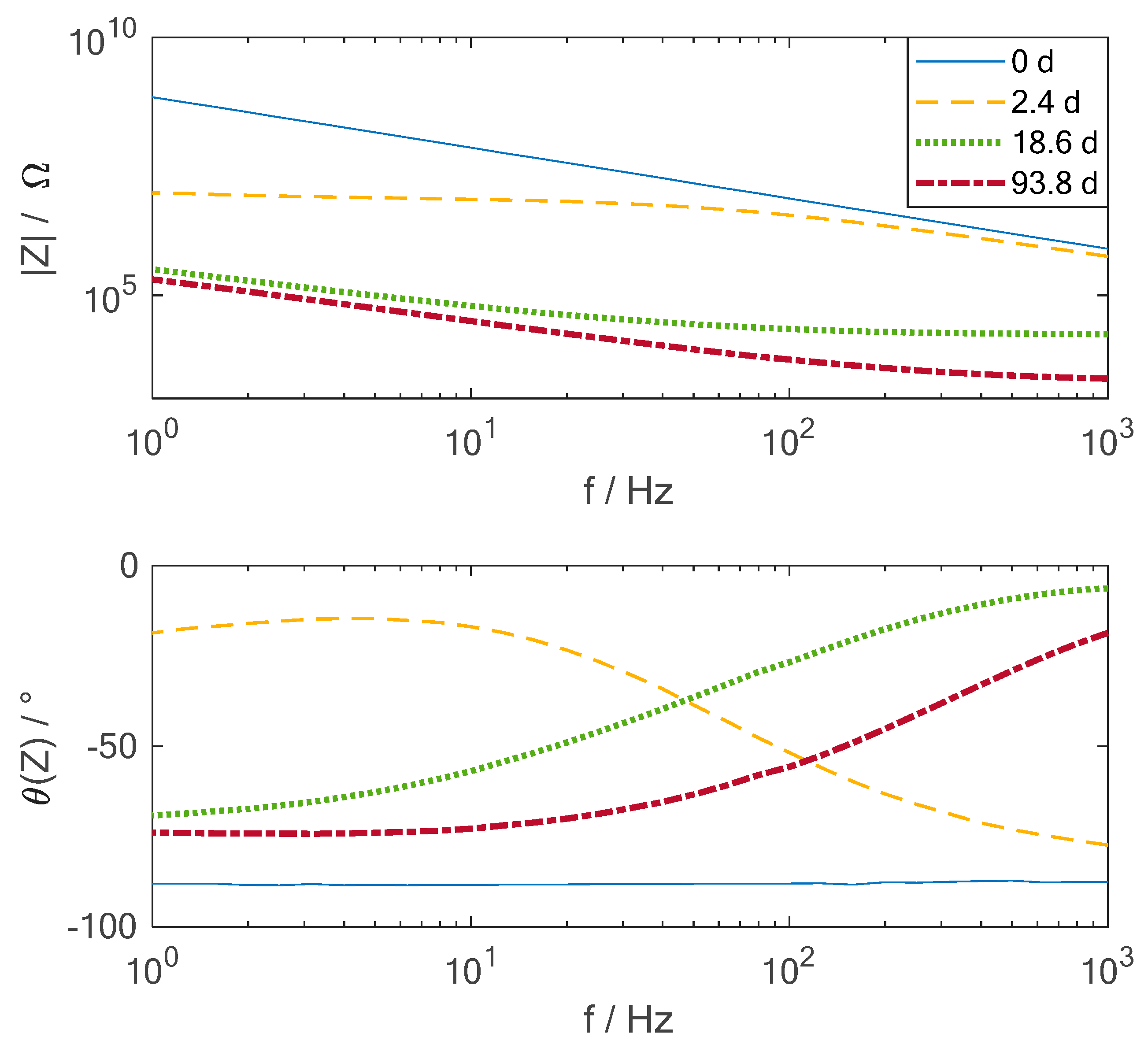
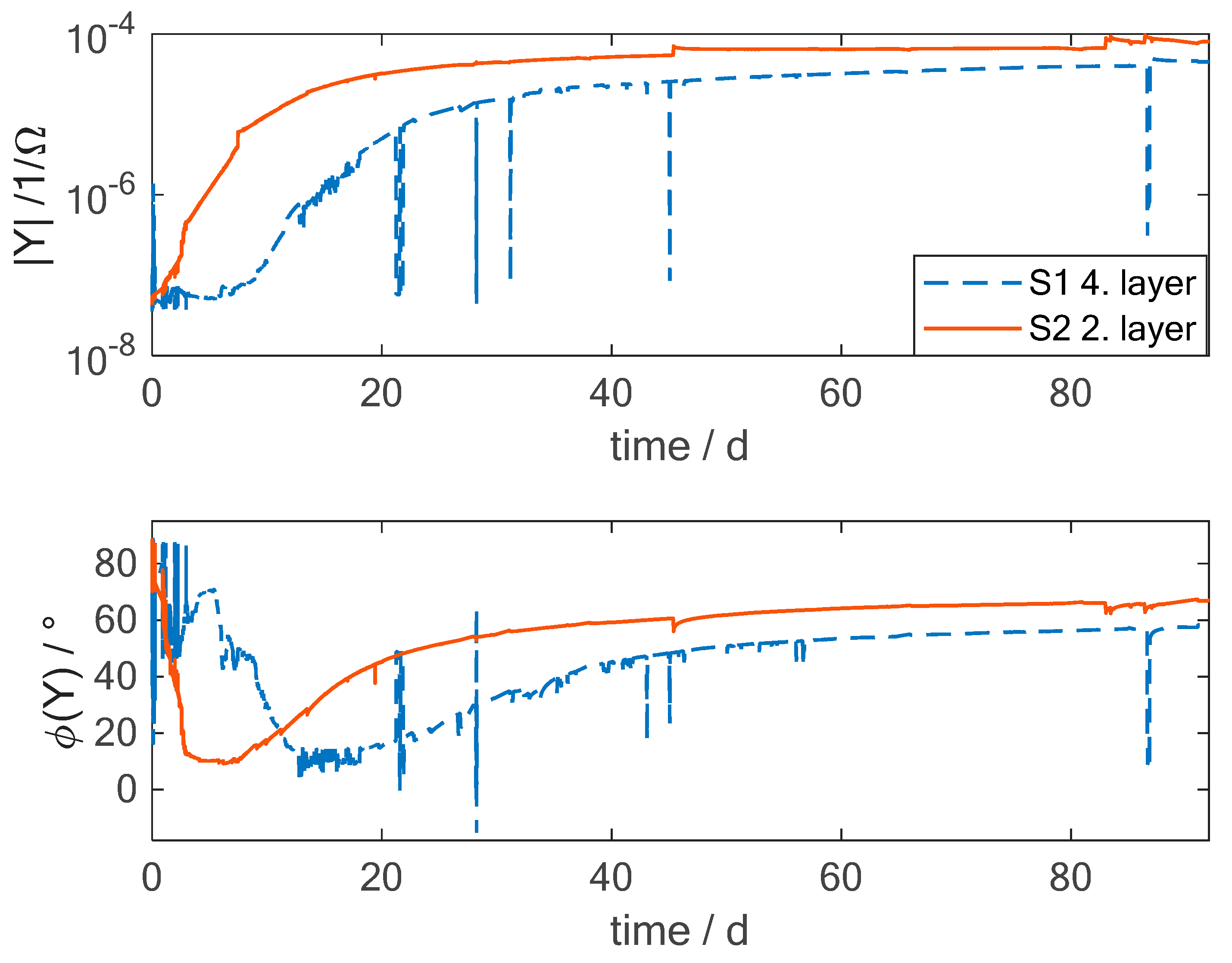
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Almeida, J.H.S.; Souza, S.D.B.; Botelho, E.C.; Amico, S.C. Carbon fiber-reinforced epoxy filament-wound composite laminates exposed to hygrothermal conditioning. J. Mater. Sci. 2016, 51, 1–12. [Google Scholar] [CrossRef]
- Zafar, A.; Bertocco, F.; Schjodt-Thomsen, J.; Rauhe, J.C. Investigation of the long term effects of moisture on carbon fibre and epoxy matrix composites. Compos. Sci. 2012, 72, 656–666. [Google Scholar] [CrossRef]
- Meng, M.; Rizvi, M.J.; Le, H.R.; Grove, S.M. Multi-scale modelling of moisture diffusion coupled with stress distribution in CFRP laminated composites. Compos. Struct. 2016, 138, 295–304. [Google Scholar] [CrossRef]
- Gibson, R.F. Principles of Composite Material Mechanics, 4th ed.; Mechanical Engineering; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9781498720694. [Google Scholar]
- Tual, N.; Carrere, N.; Davies, P.; Bonnemains, T.; Lolive, E. Characterization of sea water ageing effects on mechanical properties of carbon/epoxy composites for tidal turbine blades. Compos. Part A 2015, 78, 380–389. [Google Scholar] [CrossRef]
- Ryan, J.M.; Adams, R.; Brown, S.G.R. Moisture Ingress Effect on Properties of CFRP. In Proceedings of the 17th International Conference on Composite Materials (ICCM 17), Edinburgh, UK, 27–31 July 2009. [Google Scholar]
- Meng, M.; Rizvi, M.J.; Grove, S.M.; Le, H.R. Effects of hygrothermal stress on the failure of CFRP composites. Compos. Struct. 2015, 133, 1024–1035. [Google Scholar] [CrossRef]
- Diamanti, K.; Soutis, C. Structural health monitoring techniques for aircraft composite structures. Prog. Aerosp. Sci. 2010, 46, 342–352. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Wan, B.; Cai, G.; Qu, Y. Effect of specimen thicknesses on water absorption and flexural strength of CFRP laminates subjected to water or alkaline solution immersion. Constr. Build. Mater. 2019, 208, 314–325. [Google Scholar] [CrossRef]
- De Parscau du Plessix, B.; Jacquemin, F.; Lefébure, P.; Le Corre, S. Characterization and modeling of the polymerization-dependent moisture absorption behavior of an epoxy-carbon fiber-reinforced composite material. J. Compos. Mater. 2016, 50, 2495–2505. [Google Scholar] [CrossRef]
- Schürmann, H. Konstruieren mit Faser-Kunststoff-Verbunden, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007; ISBN 9783540721895. [Google Scholar]
- Krauklis, A.E.; Gagani, A.I.; Echtermeyer, A.T. Near-infrared spectroscopic method for monitoring water content in epoxy resins and fiber-reinforced composites. Materials 2018, 11, 586. [Google Scholar] [CrossRef]
- Cinquin, M.; Castaings, M.; Hosten, B.; Brassier, P.; Pérès, P. Monitoring of the moisture content in carbon-epoxy plates using Lamb waves. NDT E Int. 2005, 38, 37–44. [Google Scholar] [CrossRef]
- Salas, M.; Focke, O.; Herrmann, A.S.; Lang, W. Wireless actuation of piezo-elements for the structural health monitoring of carbon-fiber-reinforced-polymers. Mechatronics 2016, 34, 128–136. [Google Scholar] [CrossRef]
- Salas, M.; Focke, O.; Herrmann, A.S.; Lang, W. Low-frequency Inductive Power Transmission for Piezo-Wafer-Active-Sensors in the Structural Health Monitoring of Carbon-Fiber-Reinforced-Polymer. Procedia Technol. 2014, 15, 649–658. [Google Scholar] [CrossRef][Green Version]
- Davis, G.D.; Rich, M.J.; Drzal, L.T. Drzal Monitoring Moisture Uptake and Delamination in CFRP-Reinforced Concrete Structures with Electrochemical Impedance Sensors. J. Nondestruct. Eval. 2004, 23, 1–9. [Google Scholar] [CrossRef]
- Shimamura, Y.; Urabe, T.; Todoroki, A.; Kobayashi, H. Electrical impedance change method for moisture absorption monitoring of CFRP. Adv. Compos. Mater. 2004, 13, 297–310. [Google Scholar] [CrossRef]
- Salas, M.; Hübner, M.; Borysov, M.; Koerdt, M.; Rennoch, M.; Herrmann, A.S.; Lang, W. Measuring material moisture in fiber reinforced polymers by integrated Sensors. IEEE Sens. J. 2018, 18, 3836–3843. [Google Scholar] [CrossRef]
- Kahali Moghaddam, M.; Boll, D.; Lang, W. Embedding rigid and flexible inlays in carbon fiber reinforced plastics. In Proceedings of the 2014 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Besacon, France, 8–11 July 2014. [Google Scholar]
- Kahali Moghaddam, M.; Breede, A.; Chaloupka, A.; Bödecker, A.; Habben, C.; Meyer, E.M.; Brauner, C.; Lang, W. Design, fabrication and embedding of microscale interdigital sensors for real-time cure monitoring during composite manufacturing. Sens. Actuators A Phys. 2016, 243, 123–133. [Google Scholar] [CrossRef]
- Hübner, M.; Lang, W. Online monitoring of composites with a miniaturized flexible combined dielectric and temperature sensor. Proceedings 2017, 1, 627. [Google Scholar] [CrossRef]
- Hübner, M.; Dumstorff, G.; Mogaddam, M.K.; Plagemann, P.; Lang, W. Online monitoring of the curing of adhesives with a miniaturised interdigital sensor using impedance spectroscopy. J. Adhes. Sci. Technol. 2018, 32, 772–786. [Google Scholar] [CrossRef]
- Dumstorff, G.; Paul, S.; Lang, W. Integration without disruption: The basic challenge of sensor integration. IEEE Sens. J. 2014, 14, 2102–2111. [Google Scholar] [CrossRef]
- Ali, S.; Hassan, A.; Hassan, G.; Bae, J.; Lee, C.H. All-printed humidity sensor based on gmethyl-red/methyl-red composite with high sensitivity. Carbon 2016, 105, 23–32. [Google Scholar] [CrossRef]
- Imawan, C.; Bai, T.; Budiawanti, S. A capacitive-type humidity sensor using polymer electrolytes of PVA-LiCl thick films. In Proceedings of the 2016 International Seminar on Sensors, Instrumentation, Measurement and Metrology (ISSIMM 2016), Malang, Indonesia, 10–11 August 2016; pp. 48–52. [Google Scholar] [CrossRef]
- Oprea, A.; Bârsan, N.; Weimar, U.; Bauersfeld, M.L.; Ebling, D.; Wöllenstein, J. Capacitive humidity sensors on flexible RFID labels. Sens. Actuators Microsyst. 2007, 132, 2039–2042. [Google Scholar] [CrossRef]
- Zhang, T.; He, Y.; Wang, R.; Geng, W.; Wang, L.; Niu, L.; Li, X. Analysis of dc and ac properties of humidity sensor based on polypyrrole materials. Sens. Actuators B Chem. 2008, 131, 687–691. [Google Scholar] [CrossRef]
- Fürjes, P.; Kovács, A.; Dücso, C.; Ádám, M.; Müller, B.; Mescheder, U. Porous silicon-based humidity sensor with interdigital electrodes and internal heaters. Sens. Actuators B Chem. 2003, 95, 140–144. [Google Scholar] [CrossRef]
- Qu, W.; Meyer, J.U. Thick-film humidity sensor based on porous MnWO4material. Meas. Sci. Technol. 1997, 8, 593–600. [Google Scholar] [CrossRef]
- Martin, J.; Shetty, K.; Reimann, N.; Neukirchner, S.; Fügmann, U. Material-integrated composite humidity sensors for condition monitoring of fiber-reinforced plastics. Technol. Lightweight Struct. 2017, 1, 128–137. [Google Scholar]
- Hübner, M.; Koerdt, M.; Hardi, E.; Herrmann, A.S.; Lang, W. Embedding miniaturized flexible sensors for online monitoring of fibre composite production. In Proceedings of the ECCM18, Athens, Greece, 24–28 June 2018. [Google Scholar]
- Shen, C.-H.; Springer, G.S. Moisture absorption and desorption of composite materials. J. Compos. Mater. 1976, 10, 2–20. [Google Scholar] [CrossRef]
- Choi, H.S.; Ahn, K.J.; Nam, J.; Chun, H.J. Hygroscopic aspects of epoxy/carbon fiber composite laminates in aircraft environments. Compos. Part A 2001, 32, 709–720. [Google Scholar] [CrossRef]
- Canal, L.P.; Michaud, V. Micro-scale modeling of water diffusion in adhesive composite joints. Compos. Struct. 2014, 111, 340–348. [Google Scholar] [CrossRef]

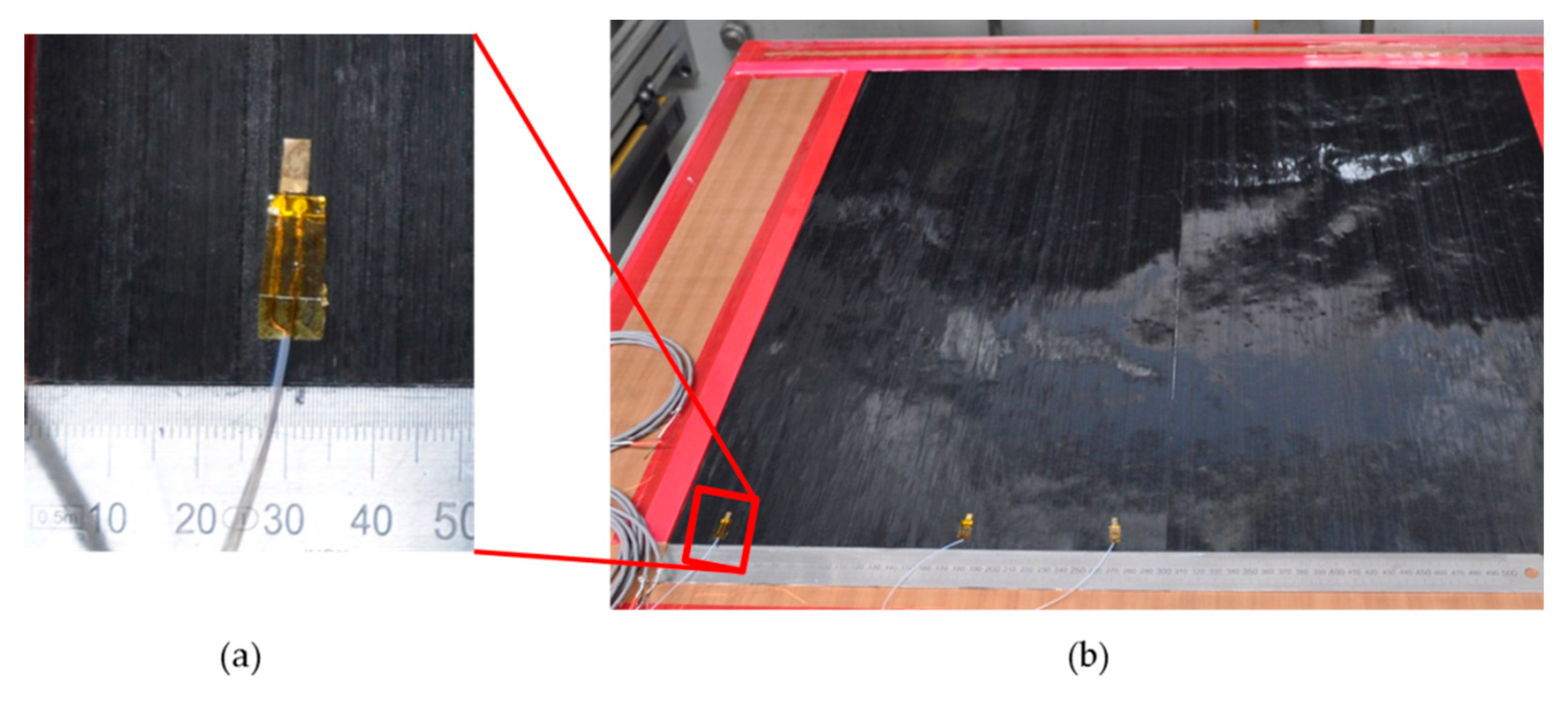
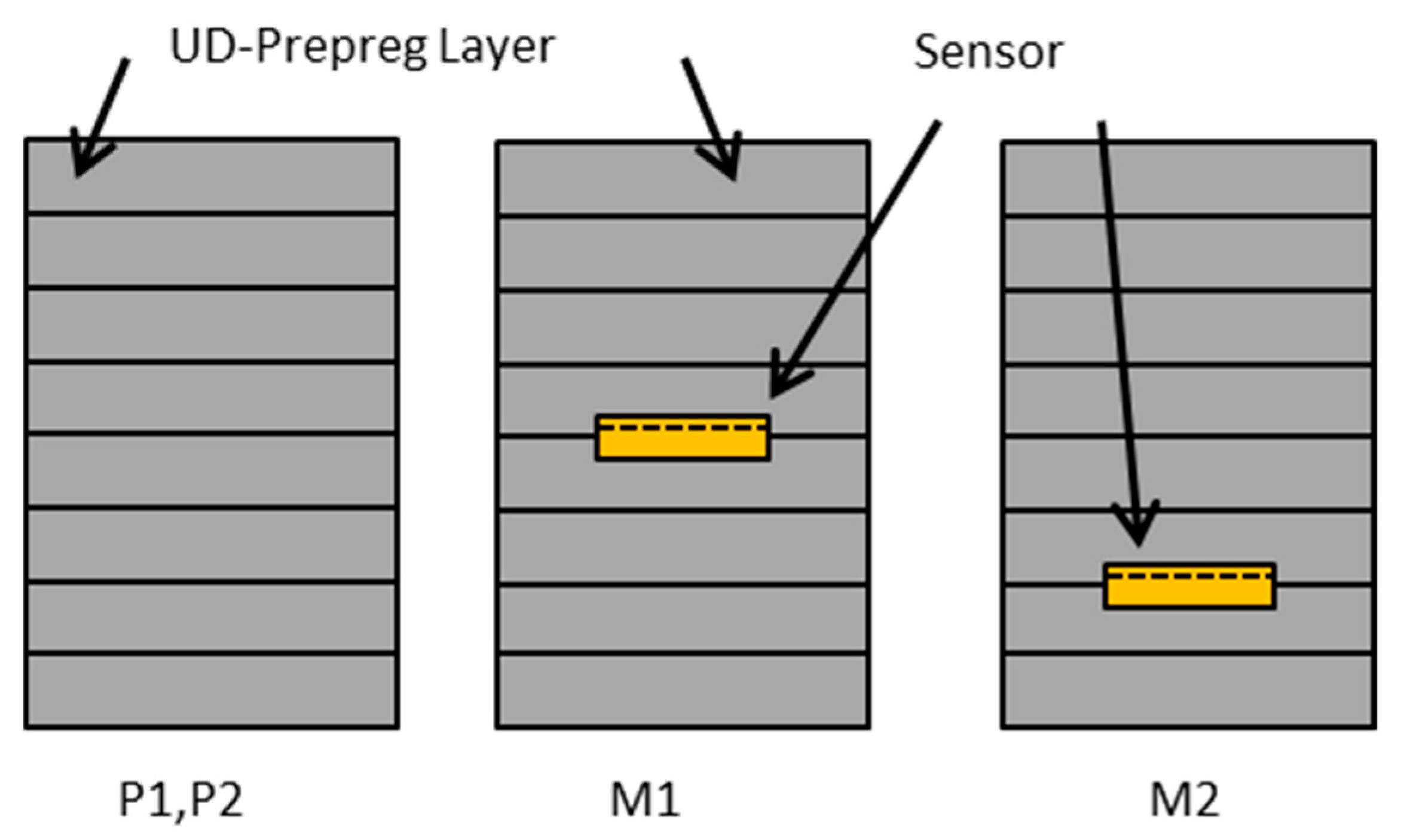

| Sensor | N | L | w | s |
|---|---|---|---|---|
| S1 | 300 | 3000 mm | 10 µm | 10 µm |
| S2 | 150 | 6000 mm | 10 µm | 10 µm |
| Sample | b | d | Sensor |
|---|---|---|---|
| P1 | 20 mm | 100 mm | no sensor |
| P2 | 20 mm | 100 mm | no sensor |
| M1 | 61 mm | 100 mm | S1 between layer 4–5 |
| M2 | 35 mm | 110 mm | S2 between layer 2–3 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hübner, M.; Lepke, D.; Hardi, E.; Koerdt, M.; Herrmann, A.S.; Lang, W. Online Monitoring of Moisture Diffusion in Carbon Fiber Composites Using Miniaturized Flexible Material Integrated Sensors. Sensors 2019, 19, 1748. https://doi.org/10.3390/s19081748
Hübner M, Lepke D, Hardi E, Koerdt M, Herrmann AS, Lang W. Online Monitoring of Moisture Diffusion in Carbon Fiber Composites Using Miniaturized Flexible Material Integrated Sensors. Sensors. 2019; 19(8):1748. https://doi.org/10.3390/s19081748
Chicago/Turabian StyleHübner, Martina, Dennis Lepke, Elisabeth Hardi, Michael Koerdt, Axel S. Herrmann, and Walter Lang. 2019. "Online Monitoring of Moisture Diffusion in Carbon Fiber Composites Using Miniaturized Flexible Material Integrated Sensors" Sensors 19, no. 8: 1748. https://doi.org/10.3390/s19081748
APA StyleHübner, M., Lepke, D., Hardi, E., Koerdt, M., Herrmann, A. S., & Lang, W. (2019). Online Monitoring of Moisture Diffusion in Carbon Fiber Composites Using Miniaturized Flexible Material Integrated Sensors. Sensors, 19(8), 1748. https://doi.org/10.3390/s19081748





