Dynamic Model Updating for Bridge Structures Using the Kriging Model and PSO Algorithm Ensemble with Higher Vibration Modes
Abstract
1. Introduction
2. Higher Mode Identification
3. Kriging Model
4. Particle Swarm Optimization
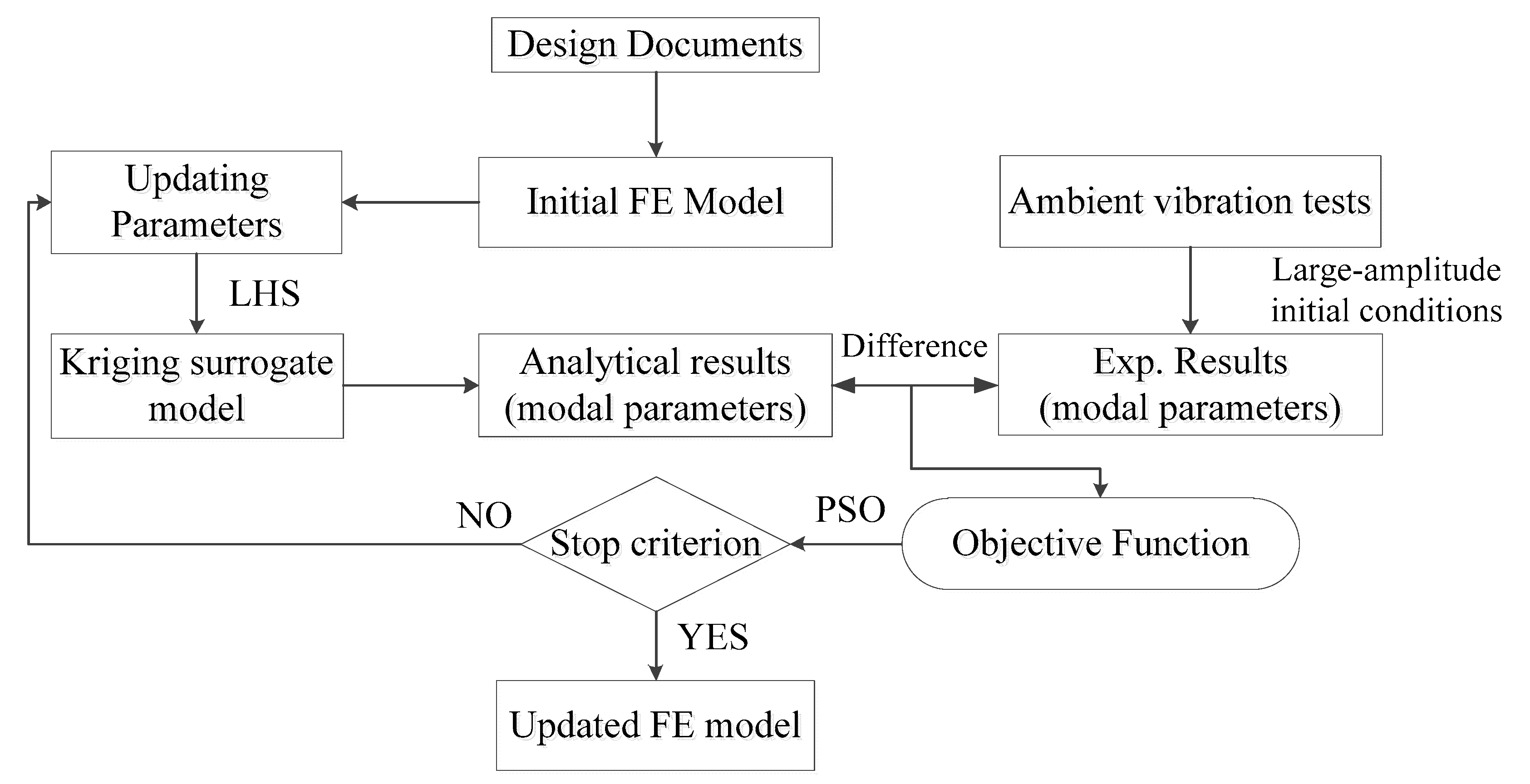
5. Model Updating with Kriging Model
6. Model Updating Verification for Jalón Viaduct
6.1. Bridge Description and Summary of OMA
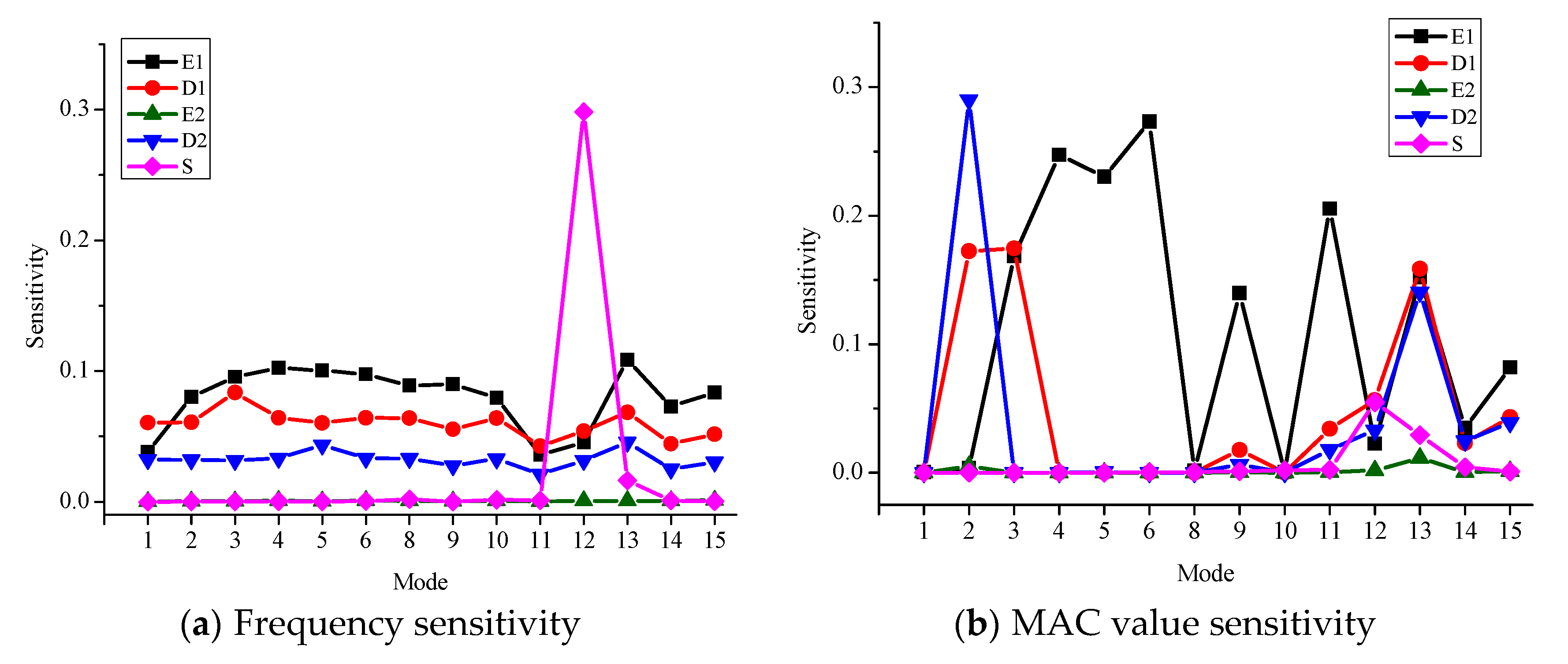
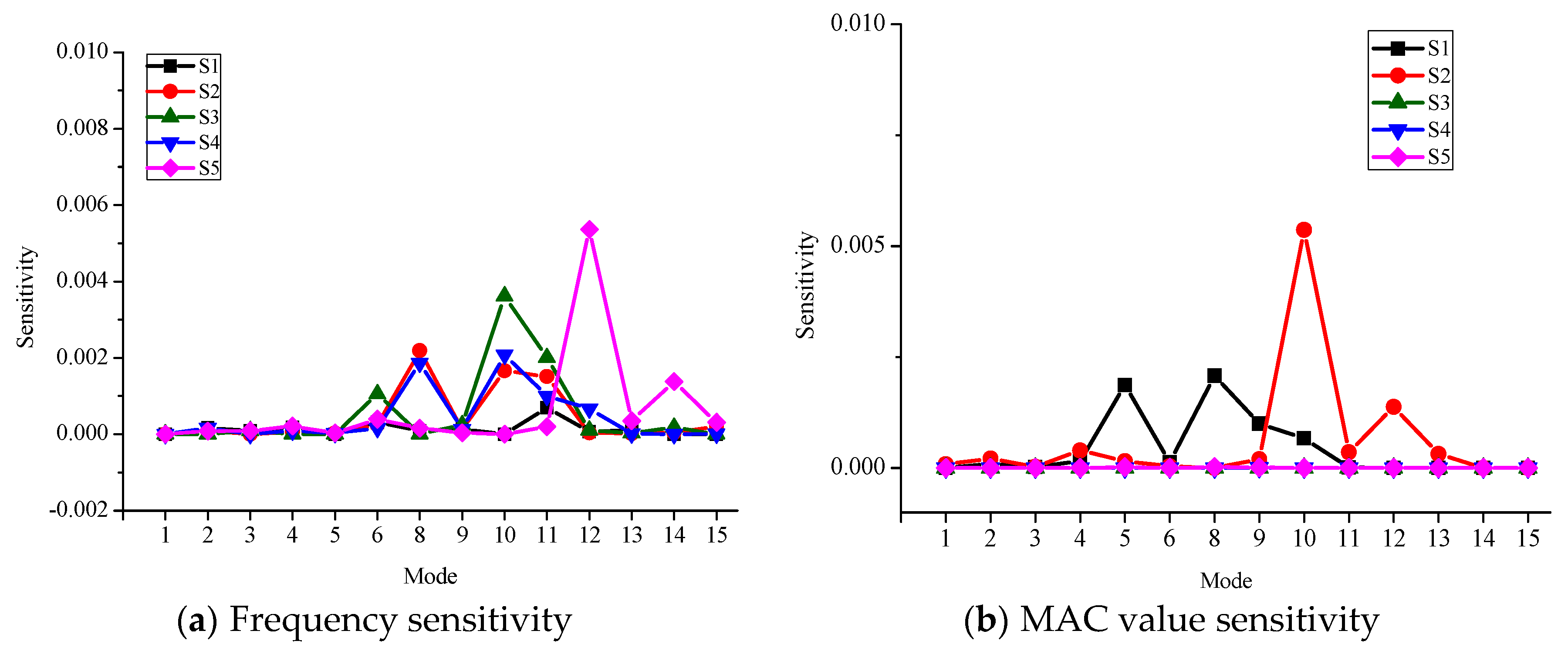
6.2. Updating Parameters Selection
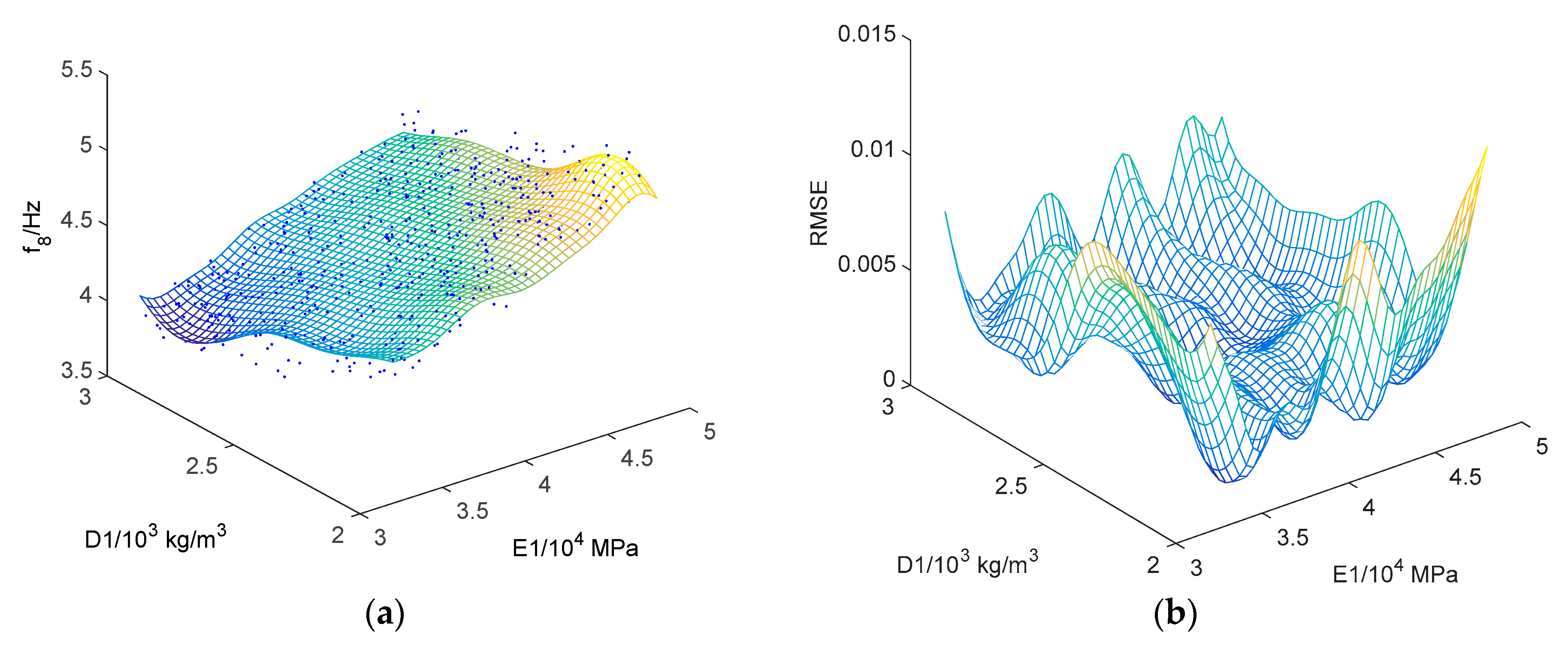
6.3. Kriging Model Establishment
6.4. Model Updating
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hsieh, K.H.; Halling, M.W.; Barr, P.J. Overview of vibrational structural health monitoring with representative case studies. J. Bridge Eng. 2006, 11, 707–715. [Google Scholar] [CrossRef]
- Ko, J.M.; Ni, Y.Q. Technology developments in structural health monitoring of large-scale bridges. Eng. Struct. 2005, 27, 1715–1725. [Google Scholar] [CrossRef]
- Li, H.; Ou, J.P. Structural health monitoring: from sensing technology stepping to health diagnosis. Proced. Eng. 2011, 15, 753–760. [Google Scholar]
- Lynch, J.P. An overview of wireless structural health monitoring for civil structures. Philos. Trans. R. Soc. A 2007, 365, 345–372. [Google Scholar] [CrossRef] [PubMed]
- Brownjohn, J.M. Structural health monitoring of civil infrastructure. Philos. Trans. R. Soc. A 2007, 365, 589–622. [Google Scholar] [CrossRef] [PubMed]
- Soyoz, S.; Feng, M.Q. Long-Term Monitoring and Identification of Bridge Structural Parameters. Comput. Aided Civ. Inf. 2009, 24, 82–92. [Google Scholar] [CrossRef]
- Mottershead, J.E.; Link, M.; Friswell, M.I. The sensitivity method in finite element model updating: A tutorial. Mech. Syst. Signal Pr. 2011, 24, 2275–2296. [Google Scholar] [CrossRef]
- Khodaparast, H.H.; Mottershead, J.E.; Friswell, M.I. Perturbation methods for the estimation of parameter variability in stochastic model updating. Mech. Syst. Signal Pr. 2008, 22, 1751–1773. [Google Scholar] [CrossRef]
- Feng, D.; Feng, M.Q. Model updating of railway bridge using in situ dynamic displacement measurement under trainloads. J. Bridge Eng. 2015, 20, 04015019. [Google Scholar] [CrossRef]
- Jaishi, B.; Ren, W.X. Finite element model updating based on eigenvalue and strain energy residuals using multiobjective optimisation technique. Mech. Syst. Signal Pr. 2007, 21, 2295–2317. [Google Scholar] [CrossRef]
- Jaishi, B. Finite Element Model Updating of Civil Engineering Structures under Operational Conditions. Ph.D. Thesis, Fuzhou University, Fuzhou, China, 2005. [Google Scholar]
- Sehgal, S.; Kumar, H. Structural dynamic model updating techniques: A state of the art review. Arch. Comput. Methods Eng. 2015, 23, 515–533. [Google Scholar] [CrossRef]
- Bakir, P.G.; Reynders, E.; De Roeck, G. Sensitivity-based finite element model updating using constrained optimization with a trust region algorithm. J. Sound Vib. 2007, 305, 211–225. [Google Scholar] [CrossRef]
- Weng, S.; Xia, Y.; Zhou, X.Q.; Xu, Y.L.; Zhu, H.P. Inverse substructure method for model updating of structures. J. Sound Vib. 2012, 331, 5449–5468. [Google Scholar] [CrossRef]
- Reynders, E.; De Roeck, G. Reference-based combined deterministic–stochastic subspace identification for experimental and operational modal analysis. Mech. Syst. Signal Pr. 2008, 22, 617–637. [Google Scholar] [CrossRef]
- Farrar, C.R.; Duffey, T.A.; Cornwell, P.J.; Doebling, S.W. Excitation methods for bridge structures. In Proceedings of the 17th International Modal Analysis Conference, Kissimmee, FL, USA, 8–11 February 1999. [Google Scholar]
- Brownjohn, J.M.W.; Xia, P.Q.; Hao, H.; Xia, Y. Civil structure condition assessment by FE model updating: methodology and case studies. Finite Elem. Anal. Des. 2001, 37, 761–775. [Google Scholar] [CrossRef]
- Moaveni, B.; Conte, J.P.; Hemez, F.M. Uncertainty and Sensitivity Analysis of Damage Identification Results Obtained Using Finite Element Model Updating. Comput. Aided Civ. Inf. 2009, 24, 320–334. [Google Scholar] [CrossRef]
- Marwala, T. Finite element model updating using response surface method. In Proceedings of the 45th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics & Materials Conference, Palm Springs, CA, USA, 19–22 April 2004; pp. 5165–5173. [Google Scholar]
- Deng, L.; Cai, C.S. Bridge model updating using response surface method and genetic algorithm. J. Bridge Eng. 2010, 15, 553–564. [Google Scholar] [CrossRef]
- Shan, D.; Li, Q.; Khan, I.; Zhou, X. A novel finite element model updating method based on substructure and response surface model. Eng. Struct. 2015, 103, 147–156. [Google Scholar] [CrossRef]
- Ren, W.X.; Chen, H.B. Finite element model updating in structural dynamics by using the response surface method. Eng. Struct. 2010, 32, 2455–2465. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, D.J.; Ma, J.; Li, Y. Performance comparison of two meta-model for the application to finite element model updating of structures. J. Harbin Inst. Tech. 2014, 21, 68–78. [Google Scholar]
- Gano, S.E.; Renaud, J.E.; Martin, J.D.; Simpson, T.W. Update strategies for kriging models used in variable fidelity optimization. Struct. Multidisc. Optim. 2006, 32, 287–298. [Google Scholar] [CrossRef]
- Wang, J.T.; Wang, C.J.; Zhao, J.P. Frequency response function-based model updating using Kriging model. Mech. Syst. Signal Pr. 2017, 87, 218–228. [Google Scholar] [CrossRef]
- Khodaparast, H.H.; Mottershead, J.E.; Badcock, K.J. Interval model updating with irreducible uncertainty using the Kriging predictor. Mech. Syst. Signal Pr. 2011, 25, 1204–1226. [Google Scholar] [CrossRef]
- Yu, D.J.; Ren, W.X. EMD-based stochastic subspace identification of structures from operational vibration measurements. Eng. Struct. 2005, 27, 1741–1751. [Google Scholar] [CrossRef]
- Qin, S.Q.; Reynders, E.; He, L.Q.; Bui, T.T.; De Roeck, G. Effects of initial conditions in operational modal analysis. Struct. Control Health Monit. 2014, 21, 557–573. [Google Scholar] [CrossRef]
- Magalhães, F.; Cunha, Á.; Caetano, E.; Brincker, R. Damping estimation using free decays and ambient vibration tests. Mech. Syst. Signal Pr. 2010, 24, 1274–1290. [Google Scholar] [CrossRef]
- Magalhães, F.; Caetano, E.; Cunha, Á.; Flamand, O.; Grillaud, G. Ambient and free vibration tests of the Millau Viaduct: Evaluation of alternative processing strategies. Eng. Struct. 2012, 45, 372–384. [Google Scholar] [CrossRef]
- Van Overschee, P.; Moor, B.L. Subspace Identification for the Linear Systems: Theory-Implementation-Applications; Kluwer academic publishers: Dordrecht, the Netherlands, 1996; ISBN 978-1-4613-0465-4. [Google Scholar]
- Zhang, J.; Au, F.T.K. Calibration of initial cable forces in cable-stayed bridge based on Kriging approach. Finite Elem. Anal. Des. 2014, 92, 80–92. [Google Scholar] [CrossRef]
- Qin, S.Q.; Zhou, Y.L.; Cao, H.Y. Wahab, M.A. Model updating in complex bridge structures using Kriging model ensemble with Genetic Algorithm. KSCE J. Civil Eng. 2017, 1–12. [Google Scholar] [CrossRef]
- Fang, S.E.; Perera, R. Damage identification by response surface based model updating using D-optimal design. Mech. Syst. Signal Pr. 2011, 25, 717–733. [Google Scholar] [CrossRef]
- Mckay, M.D. Latin Hypercube Sampling as a Tool in Uncertainty Analysis of Computer Models. In Proceedings of the 24th Winter Simulation, Arlington, VA, USA, 13–16 December 1992; pp. 557–564. [Google Scholar]
- Shabbir, F.; Omenzetter, P. Particle Swarm Optimization with Sequential Niche Technique for Dynamic Finite Element Model Updating. Comput. Aided Civ. Inf. 2015, 30, 359–375. [Google Scholar] [CrossRef]
- Sciammarella, P.F.; Lamonaca, F.; Scuro, C.; Carnì, D.L.; Olivito, R. Synchronization of IoT layers for Structural Health Monitoring. In Proceedings of the Metrology for Industry 4.0 & IoT, Brescia, Italy, 16–18 April 2018. [Google Scholar]
- Sciammarella, P.F.; Lamonaca, F.; Scuro, C.; Olivito, R.; Carnì, D.L. Internet of Things for Structural Health Monitoring. In Proceedings of the Metrology for Industry 4.0 & IoT, Brescia, Italy, 16–18 April 2018. [Google Scholar]
- Dan, D.; Chen, Y.; Xu, B. A PSO driven intelligent model updating and parameter identification scheme for cable-damper system. Shock Vib. 2015, 2015, 1–14. [Google Scholar] [CrossRef]

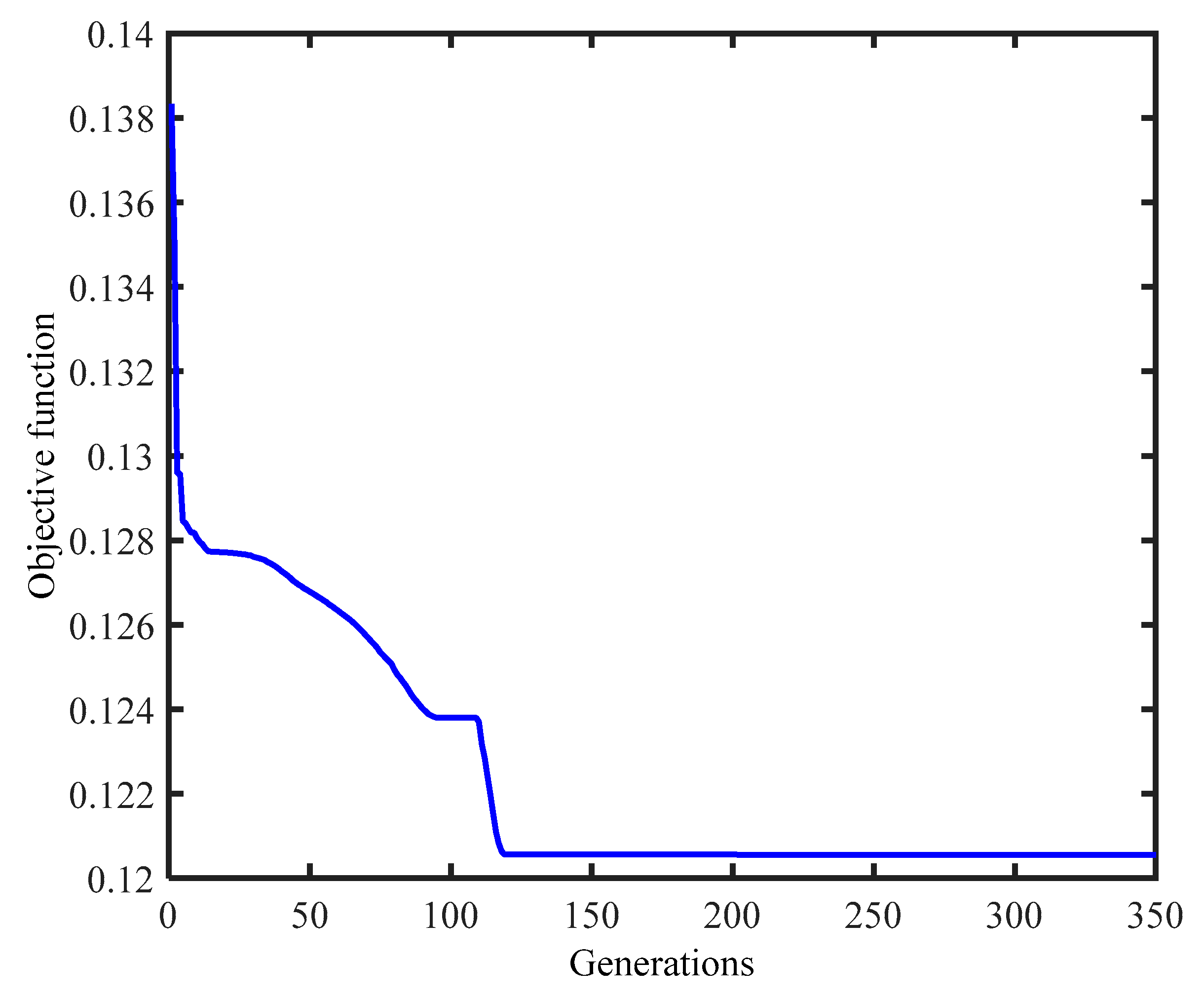

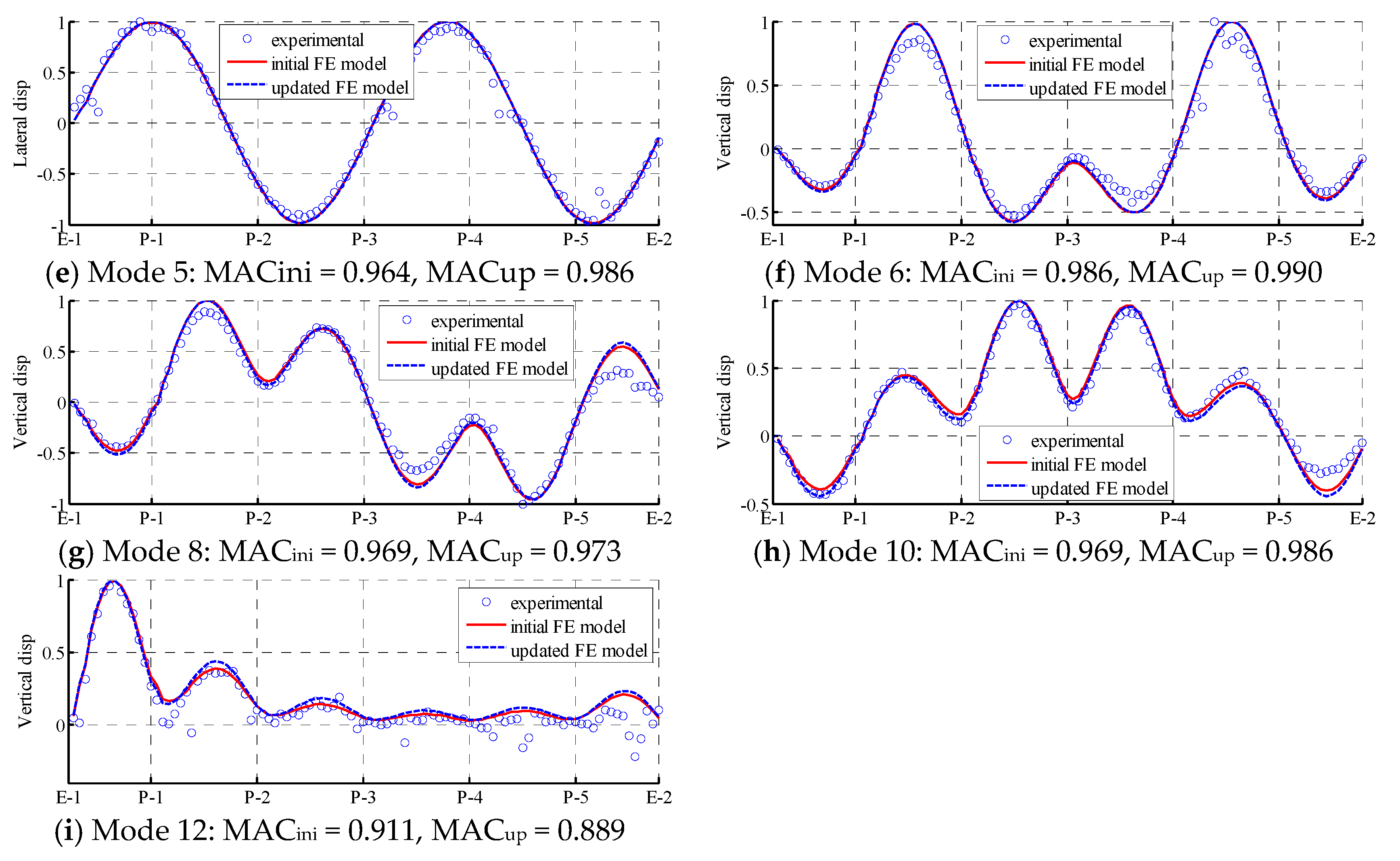

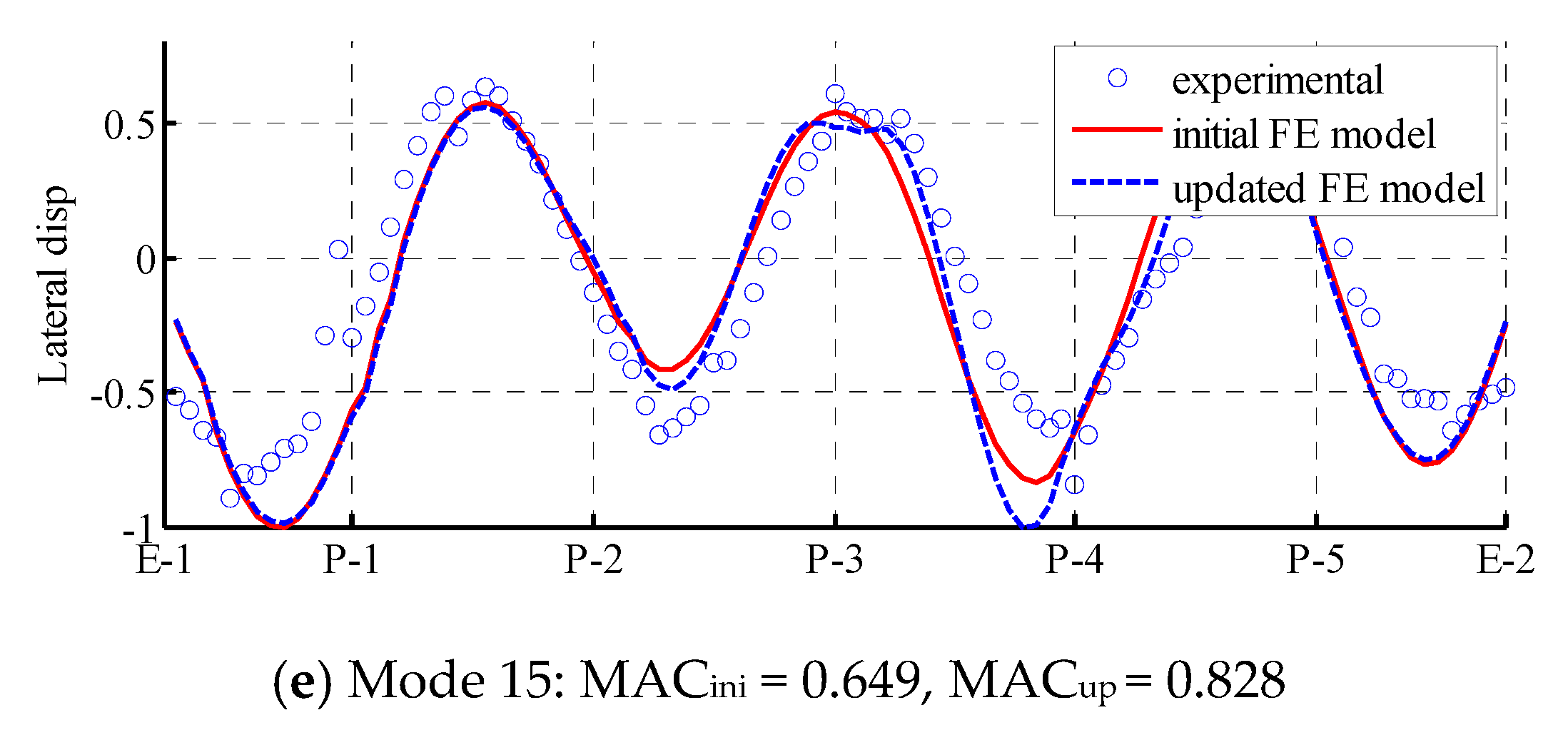
| Mode | Type | Initial FE Model | Ambient Vibration | Free-Ambient Vibration | ||||
|---|---|---|---|---|---|---|---|---|
| fa,ini (Hz) | fea (Hz) | (%) | fef | (%) | MPC | MAC | ||
| 1 | 1st↔ | 0.601 | 0.648 | 0.75 | 0.648 | 0.69 | 0.94 | 0.973 |
| 2 | 2nd↔ | 1.182 | 1.238 | 0.81 | 1.219 | 1.34 | 0.96 | 0.977 |
| 3 | 3rd↔ | 2.201 | 2.230 | 1.00 | 2.186 | 1.53 | 0.85 | 0.926 |
| 4 | 1st↕ | 3.264 | 3.279 | 0.42 | 3.267 | 0.40 | 0.96 | 0.985 |
| 5 | 4th↔ | 3.595 | 3.536 | 1.37 | 3.493 | 0.70 | 0.93 | 0.964 |
| 6 | 2nd↕ | 3.788 | 3.758 | 0.54 | 3.783 | 0.61 | 0.98 | 0.986 |
| 8 | 4th↕ | 4.421 | 4.471 | 0.66 | 4.452 | 0.48 | 0.98 | 0.969 |
| 10 | 5th↕ | 5.015 | 5.056 | 0.57 | 5.045 | 0.62 | 0.97 | 0.969 |
| 12 | 6th↕ | 5.996 | 6.036 | 0.69 | 6.027 | 0.70 | 0.91 | 0.911 |
| Mode | Type | Initial FE Model | Free-Ambient Vibration | |||
|---|---|---|---|---|---|---|
| fa,ini (Hz) | fef | (%) | MPC | MAC | ||
| 9 | 5th↔ | 5.211 | 4.917 | 0.74 | 0.94 | 0.958 |
| 11 | 1st↺ | 5.717 | 5.309 | 0.73 | 0.85 | 0.865 |
| 13 | 6th↔ | 7.554 | 7.455 | 0.70 | 0.87 | 0.499 |
| 14 | 2nd↺ | 9.290 | 8.420 | 0.96 | 0.83 | 0.701 |
| 15 | 7th↔ | 10.180 | 9.329 | 0.96 | 0.79 | 0.649 |
| Updating Parameters | Initial Value | Updated Value | Difference (%) |
|---|---|---|---|
| E1 (104 MPa) | 4.00 | 3.85 | −3.8 |
| D1 (103 kg/m3) | 2.50 | 2.36 | −5.6 |
| E2 (102 MPa) | 2.80 | 2.42 | −13.6 |
| D2 (103 kg/m3) | 1.70 | 1.86 | 9.4 |
| S1 (105 kN/m) | 2.00 | 3.29 | 64.5 |
| S5 (105 kN/m) | 2.00 | 3.43 | 71.5 |
| Mode | Mode Type | Experimental (Free–Ambient) | Initial FE Model | Updated FE Model | ||||
|---|---|---|---|---|---|---|---|---|
| fef (Hz) | fa,ini (Hz) | Difference (%) | MAC | fa,up (Hz) | Difference (%) | MAC | ||
| 1 | 1st↔ | 0.648 | 0.601 | 7.25 | 0.973 | 0.634 | 2.18 | 0.978 |
| 2 | 2nd↔ | 1.219 | 1.182 | 3.04 | 0.977 | 1.196 | 1.92 | 0.983 |
| 3 | 3rd↔ | 2.186 | 2.201 | −0.69 | 0.926 | 2.191 | −0.24 | 0.962 |
| 4 | 1st↕ | 3.267 | 3.264 | 0.09 | 0.985 | 3.234 | 1.00 | 0.996 |
| 5 | 4th↔ | 3.493 | 3.595 | −2.92 | 0.964 | 3.558 | −1.85 | 0.986 |
| 6 | 2nd↕ | 3.783 | 3.788 | −0.13 | 0.986 | 3.757 | 0.70 | 0.990 |
| 8 | 4th↕ | 4.452 | 4.421 | 0.70 | 0.969 | 4.461 | −0.20 | 0.973 |
| 9 | 5th↔ | 4.917 | 5.211 | −5.98 | 0.958 | 5.127 | −4.28 | 0.989 |
| 10 | 5th↕ | 5.045 | 5.015 | 0.59 | 0.969 | 5.047 | −0.04 | 0.986 |
| 11 | 1st↺ | 5.309 | 5.717 | −7.69 | 0.865 | 5.372 | −1.18 | 0.929 |
| 12 | 6th↕ | 6.027 | 5.966 | 1.01 | 0.911 | 5.966 | 1.02 | 0.889 |
| 13 | 6th↔ | 7.455 | 7.554 | −1.33 | 0.499 | 7.497 | −0.56 | 0.585 |
| 14 | 2nd↺ | 8.420 | 9.290 | −10.33 | 0.701 | 9.043 | −7.40 | 0.791 |
| 15 | 7th↔ | 9.329 | 10.180 | −9.12 | 0.649 | 10.121 | −8.49 | 0.828 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, S.; Zhang, Y.; Zhou, Y.-L.; Kang, J. Dynamic Model Updating for Bridge Structures Using the Kriging Model and PSO Algorithm Ensemble with Higher Vibration Modes. Sensors 2018, 18, 1879. https://doi.org/10.3390/s18061879
Qin S, Zhang Y, Zhou Y-L, Kang J. Dynamic Model Updating for Bridge Structures Using the Kriging Model and PSO Algorithm Ensemble with Higher Vibration Modes. Sensors. 2018; 18(6):1879. https://doi.org/10.3390/s18061879
Chicago/Turabian StyleQin, Shiqiang, Yazhou Zhang, Yun-Lai Zhou, and Juntao Kang. 2018. "Dynamic Model Updating for Bridge Structures Using the Kriging Model and PSO Algorithm Ensemble with Higher Vibration Modes" Sensors 18, no. 6: 1879. https://doi.org/10.3390/s18061879
APA StyleQin, S., Zhang, Y., Zhou, Y.-L., & Kang, J. (2018). Dynamic Model Updating for Bridge Structures Using the Kriging Model and PSO Algorithm Ensemble with Higher Vibration Modes. Sensors, 18(6), 1879. https://doi.org/10.3390/s18061879






