Dynamics of a Predator–Prey Model with the Effect of Oscillation of Immigration of the Prey
Abstract
1. Introduction
2. Model and Boundedness without Oscillation of the Immigration
3. Theoretical Analysis of the Model without Immigration
3.1. Kolmogorov Analysis
- (1)
- If the number of predators increases and the number of prey is fixed, then the prey and predator growth rate decrease. The factors that express these conditions are represented as follows:
- (2)
- The model includes the environment carrying capacity. The following condition of model (1) is attained:
- (3)
- The model has a minimum prey value, even in the case of a small predator population. The following condition of model (1) is attained:
- (4)
- The predators coexist with the prey if the following condition is satisfied:
3.2. Existence of Equilibrium Points
- (i)
- When , then . Then, it has always without any condition.
- (ii)
- (i)
- When , then . It is clear that under the following condition:
- (ii)
3.3. Local and Global Stability Analysis
4. Model with Oscillation of Immigration
5. Numerical Simulations
6. Discussion and Conclusions
- Without consideration the oscillation of the immigration of the prey, the dynamic behavior of the system is in steady state coexistence. This coincides with the theoretical analysis of Proposition 2 and Theorem 6.
- However, when investigating the oscillation of immigration of the prey, the dynamic behavior of the system tends to exhibit stable fluctuations which increase because of the increase in the immigration parameter and the oscillation parameter that exists within the immigration.
- The likelihood of coexistence of the system increases as the value of immigration parameter increases. In addition to, when neglecting the oscillation parameter i.e., , the likelihood of coexistence of the system increases more.
- Without consideration the oscillation of immigration of the prey, the dynamic behavior of the system is in fluctuated coexistence. This coincides with the theoretical analysis in Proposition 1 and the Corollary.
- However, when investigating the oscillation of the immigration of the prey, the dynamic behavior of the system tends to exhibit stable fluctuations which increase because of the increase in the immigration parameter and the oscillation parameter that exists within the immigration.
- The likelihood of the coexistence of the system increases as the value of the immigration parameter increases. In addition, when neglecting the oscillation parameter, i.e., , the likelihood of coexistence of the system increases more.
- The dynamic of the system tends to exhibit a more stable coexistence as the immigration parameter increases.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hadeler, K.P.; Freedman, H.I. Predator-prey population with parasite infection. J. Math. Biol. 1989, 27, 609–631. [Google Scholar] [CrossRef] [PubMed]
- Kuang, Y.; Beretta, E. Global qualitative analysis of a ratio-dependent predator-prey system. J. Math. Biol. 1998, 36, 389–406. [Google Scholar] [CrossRef]
- Murray, J.D. Mathematical Biology; Springer: New York, NY, USA, 2002; Volume 2. [Google Scholar]
- Hsu, S.-B.; Hwang, T.-W.; Kuang, Y. A ratio-dependent food chain model and its applications to biological control. Math. Biosci. 2003, 181, 55–83. [Google Scholar] [CrossRef]
- Alebraheem, J.; Abu-Hassan, Y. Dynamics of a two predator-one prey system. Comput. Appl. Math. 2014, 33, 767–780. [Google Scholar] [CrossRef]
- Al-Moqbali, M.K.A.; Al-Salti, N.S.; Elmojtaba, I.M. Prey–Predator Models with Variable Carrying Capacity. Mathematics 2018, 6, 102. [Google Scholar] [CrossRef]
- Lotka, A.J. Elements of Physical Biology; Williams & Wilkins: Baltimore, MD, USA, 1925. [Google Scholar]
- Volterra, V. Variazione e fluttuazini del numero d’individui in specie animali conviventi. Mem R Accad. Naz Lincei 1926, 2, 31–113. [Google Scholar]
- Kuang, Y. Basic properties of mathematical population models. J. Biomath. 2002, 17, 129–142. [Google Scholar]
- Rockwood, L.L. Introduction to Population Ecology; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Solomon, M.E. The natural control of animal populations. J. Anim. Ecol. 1949, 18, 1–35. [Google Scholar] [CrossRef]
- Holling, C.S. Some Characteristics of Simple Types of Predation and Parasitism. Can. Entomol. 1959, 91, 385–398. [Google Scholar] [CrossRef]
- Crowley, P.H.; Martin, E.K. Functional responses and interference within and between year classes of a dragonfly population. J. North. Am. Benth Soc. 1989, 8, 211–221. [Google Scholar] [CrossRef]
- Upadhyay, R.K.; Naj, R.K. Dynamics of a three species food chain model with Crowley-Martin type functional response. Chaos Solit. Fractals. 2009, 42, 1337–1346. [Google Scholar] [CrossRef]
- Ali, N.; Jazar, M. Global dynamics of a modified Leslie–Gower predator–prey model with Crowley–Martin functional responses. J. Appl. Math. Comput. 2013, 43, 271–293. [Google Scholar] [CrossRef]
- Shi, X.Y.; Zhou, X.Y.; Song, X.Y. Analysis of a stage-structured predator–prey model with Crowley–Martin function. J. Appl. Math. Comput. 2011, 36, 459–472. [Google Scholar] [CrossRef]
- Meng, X.Y.; Huo, H.F.; Xiang, H.; Yin, Q. Stability in a predator-prey model with Crowley-Martin function and stage structure for prey. Appl. Math. Comput. 2014, 232, 810–819. [Google Scholar] [CrossRef]
- Maiti, A.; Dubey, B.; Chakraborty, A. Global analysis of a delayed stage structure prey–predator model with Crowley–Martin type functional response. Math. Comput. Simul. 2019, 162, 58–84. [Google Scholar] [CrossRef]
- Alebraheem, J.; Abu-Hassan, Y. Simulation of complex dynamical behaviour in prey predator model. In Proceedings of the 2012 International Conference on Statistics in Science, Business and Engineering, Langkawi, Malaysia, 10–12 September 2012. [Google Scholar] [CrossRef]
- Mortoja Golam, S.K.; Panja, P.; Mondal, S.K. Dynamics of a predator-prey model with nonlinear incidence rate, Crowley-Martin type functional response and disease in prey population. Ecol. Gen. Genom. 2019, 10, 100035. [Google Scholar] [CrossRef]
- Ren, J.; Yu, L.; Siegmund, S. Bifurcations and chaos in a discrete predator-prey model with Crowley-Martin functional response. Nonlinear Dyn. 2017, 90, 19–41. [Google Scholar] [CrossRef]
- Liu, W.; Cai, D. Bifurcation, chaos analysis and control in a discrete-time predator–prey system. Adv. Differ. Equ. 2019, 2019, 11. [Google Scholar] [CrossRef]
- Liu, X.-Q.; Zhong, S.-M.; Tian, B.-D.; Zheng, F.-X. Asymptotic properties of a stochastic predator-prey model with Crowley-Martin functional response. Appl. Math. Comput. 2013, 43, 479–490. [Google Scholar] [CrossRef]
- Xu, C.; Ren, G.; Yu, Y. Extinction Analysis of Stochastic Predator–Prey System with Stage Structure and Crowley–Martin Functional Response. Entropy 2019, 21, 252. [Google Scholar] [CrossRef]
- Mukherjee, D. The effect of refuge and immigration in a predator–prey system in the presence of a competitor for the prey. Nonlinear Anal. Real World Appl. 2016, 31, 277–287. [Google Scholar] [CrossRef]
- Tahara, T.; Gavina, M.K.A.; Kawano, T.; Tubay, J.M.; Rabajante, J.F.; Ito, H.; Morita, S.; Ichinos, G.; Okabe, T.; Togashi, T.; et al. Asymptotic stability of a modified Lotka-Volterra model with small immigrations. Sci. Rep. 2018, 8, 7029. [Google Scholar] [CrossRef] [PubMed]
- Upadhyay, R.K.; Iyengar, S. Effect of seasonality on the dynamics of 2and 3 species prey-predator systems. Nonlinear Anal. Real World Appl. 2007, 6, 509–530. [Google Scholar] [CrossRef]
- Gakkhar, S.; Naji, R.K. Seasonally perturbed prey-predator system with predator-dependent functional response. Chaos Solitons Fractals 2003, 18, 1075–1083. [Google Scholar] [CrossRef]
- Alebraheem, J. Fluctuations in interactions of prey predator systems. Sci. Int. 2016, 28, 2357–2362. [Google Scholar]
- Freedman, I. Deterministic Mathematical Models in Population Ecology; Marcel Dekker, Inc.: New York, NY, USA, 1980. [Google Scholar]
- Sigmund, K. Kolmogorov and Population Dynamics, Kolmogorovs Heritage in Mathematics; Springer: Berlin/Heidelberg, Germany, 2007; pp. 177–186. [Google Scholar]
- Dubey, B.; Upadhyay, R. Persistence and extinction of one-prey and two-predator system. Nonlinear Anal. 2004, 9, 307–329. [Google Scholar] [CrossRef]
- Huo, H.F.; Ma, Z.P.; Liu, C.Y. Persistence and stability for a generalized leslie-gower model with stage structure and dispersal. Abstr. Appl. Anal. 2009, 2009, 135843. [Google Scholar] [CrossRef]
- Kar, T.; Batabyal, A. Persistence and stability of a two prey one predator system. Int. J. Eng. Sci. Technol. 2010, 2, 174–190. [Google Scholar] [CrossRef]
- Tian, X.; Xu, R. Global dynamics of a predator-prey system with holling type II functional response. Nonlinear Anal. Model. Control. 2011, 16, 242–253. [Google Scholar] [CrossRef]
- Alebraheem, J.; Abu-Hasan, Y. The Effects of Capture Efficiency on the Coexistence of a Predator in a Two Predators-One Prey Model. J. Appl. Sci. 2011, 11, 3717–3724. [Google Scholar] [CrossRef]
- Alebraheem, J.; Abu-Hasan, Y. Persistence of Predators in a Two Predators-One Prey Model with Non-Periodic Solution. Appl. Math. Sci. 2012, 6, 943–956. [Google Scholar]
- Alebraheem, J. Relationship between the Paradox of Enrichment and the Dynamics of Persistence and Extinction in Prey-Predator Systems. Symmetry 2018, 10, 532. [Google Scholar] [CrossRef]
- Tunç, C.; Tunç, O. Qualitative analysis for a variable delay system of differential equations of second order. J. Taibah Univ. Sci. 2019, 13, 468–477. [Google Scholar] [CrossRef]
- Shah, K.; Tunç, C. Existence theory and stability analysis to a system of boundary value problem. J. Taibah Univ. Sci. 2017, 11, 1330–1342. [Google Scholar] [CrossRef]
- Brown, J.H.; Kodric-Brown, A. Turnover rates in insular biogeography: Effect of immigration on extinction. Ecology 1977, 58, 445–449. [Google Scholar] [CrossRef]
- Richards, C.M. Inbreeding depression and genetic rescue in a plant metapopulation. Am. Nat. 2000, 155, 383–394. [Google Scholar] [CrossRef] [PubMed]
- Eriksson, A.; Elías-Wolff, F.; Mehlig, B.; Manica, A. The emergence of the rescue effect from explicit within-and between-patch dynamics in a metapopulation. Proc. R. Soc. B 2014, 281, 20133127. [Google Scholar] [CrossRef]
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).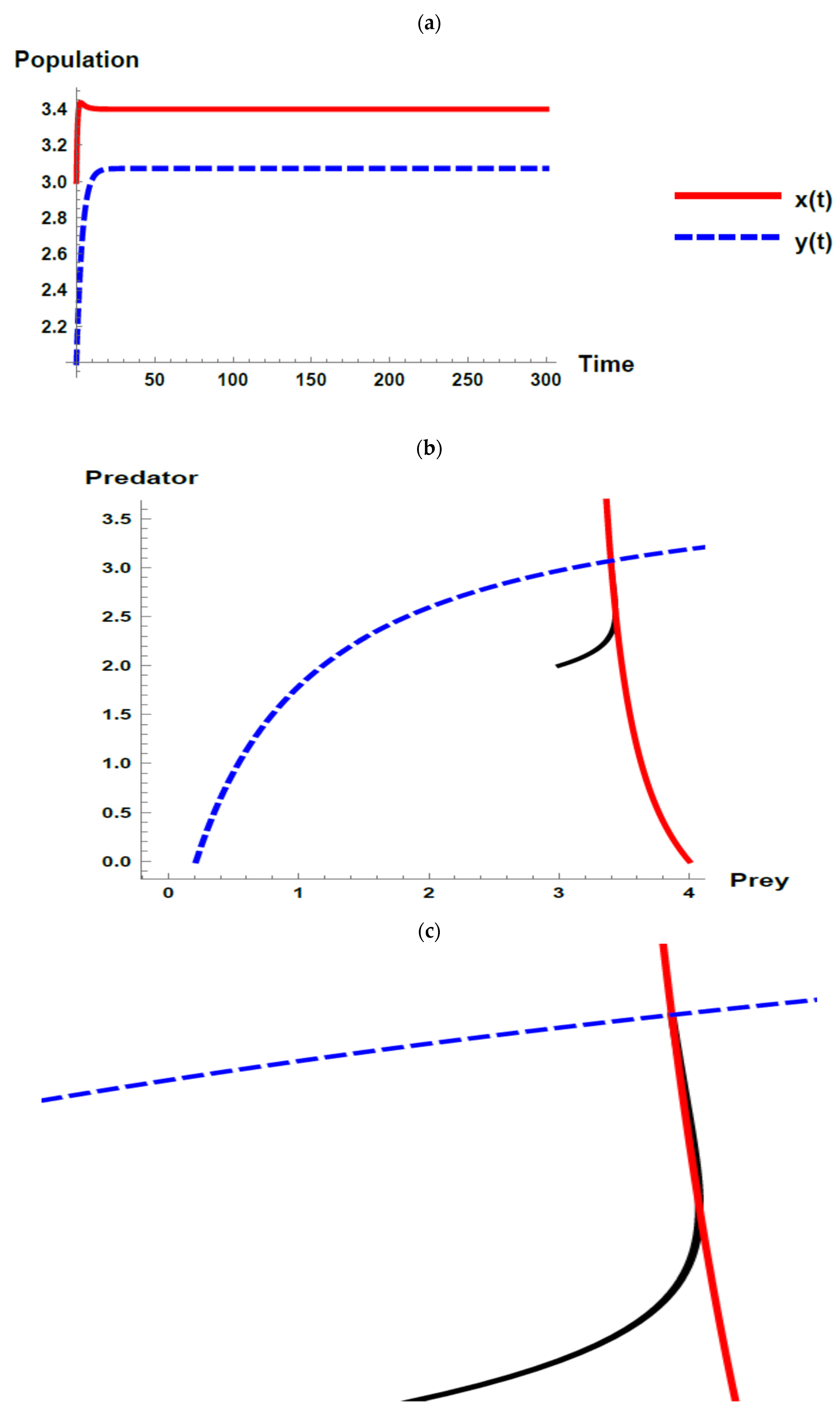
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).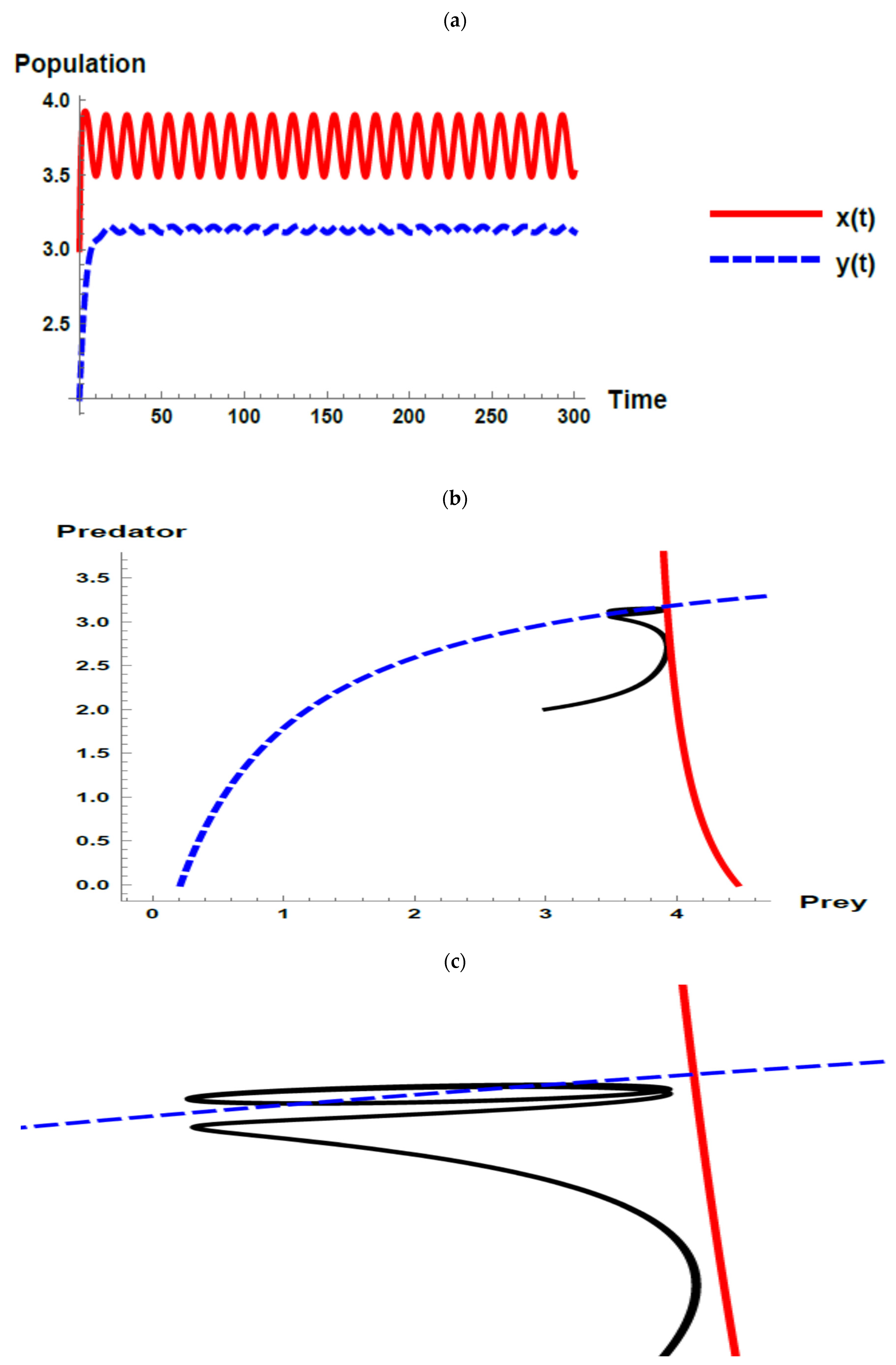
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).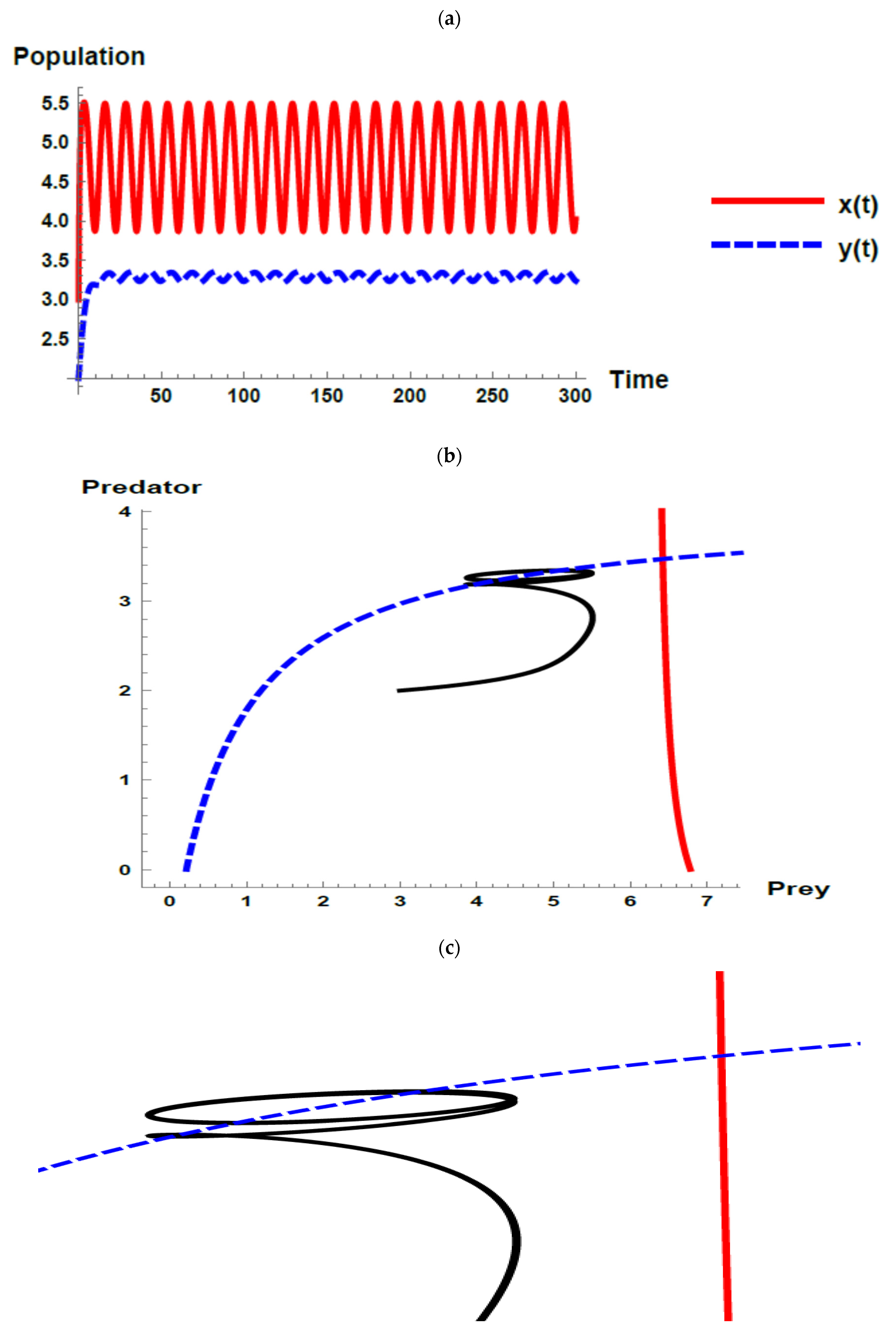
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).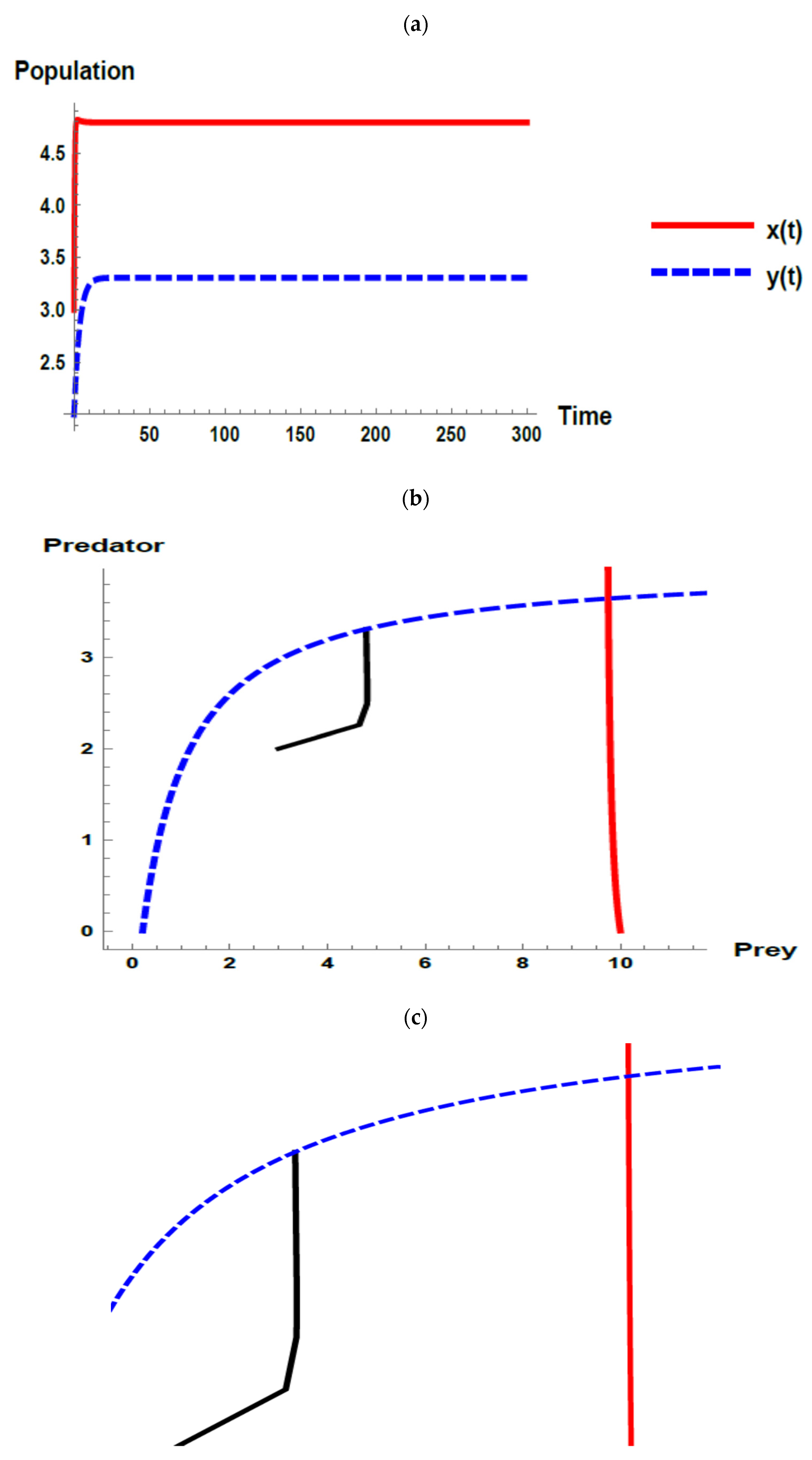
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).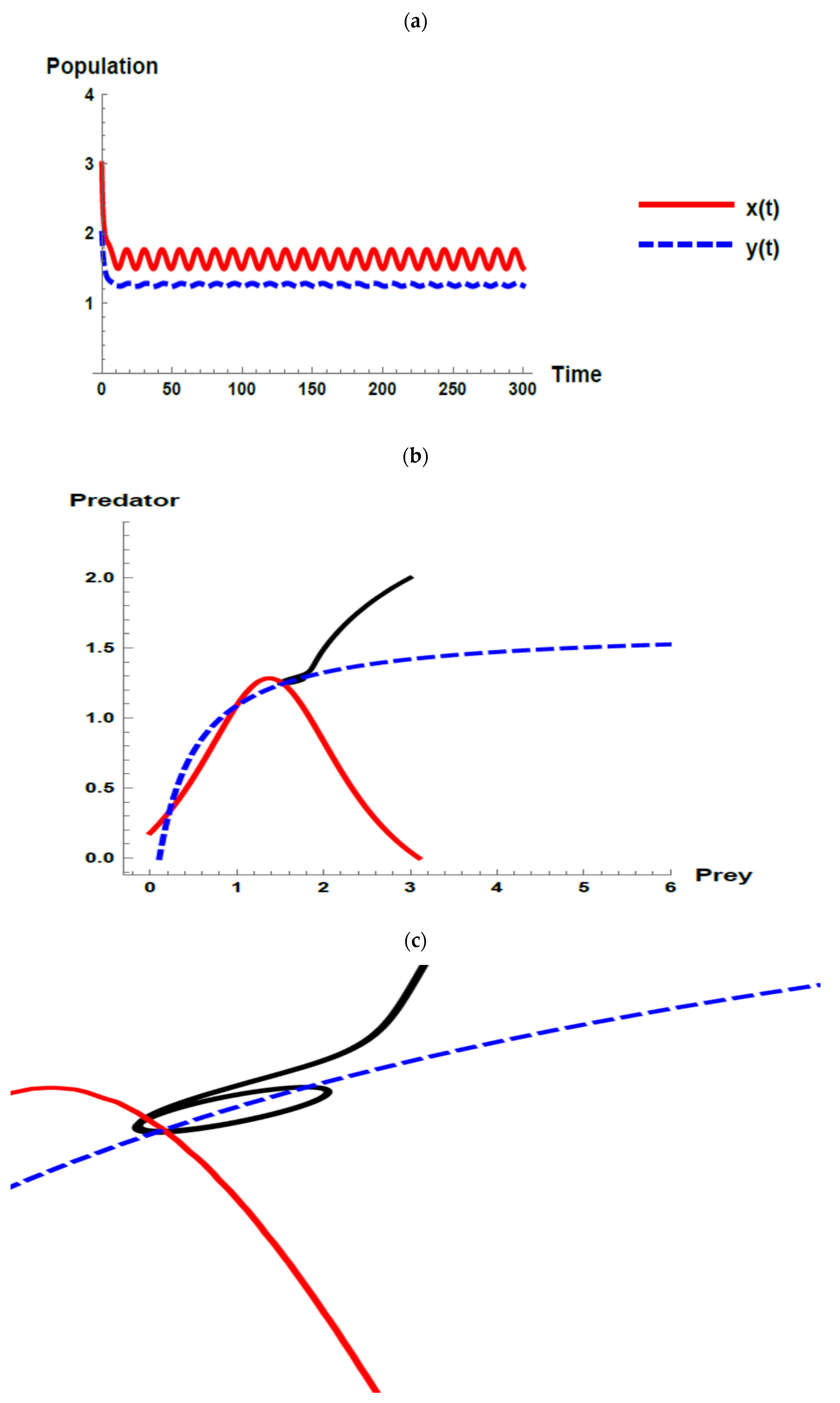
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).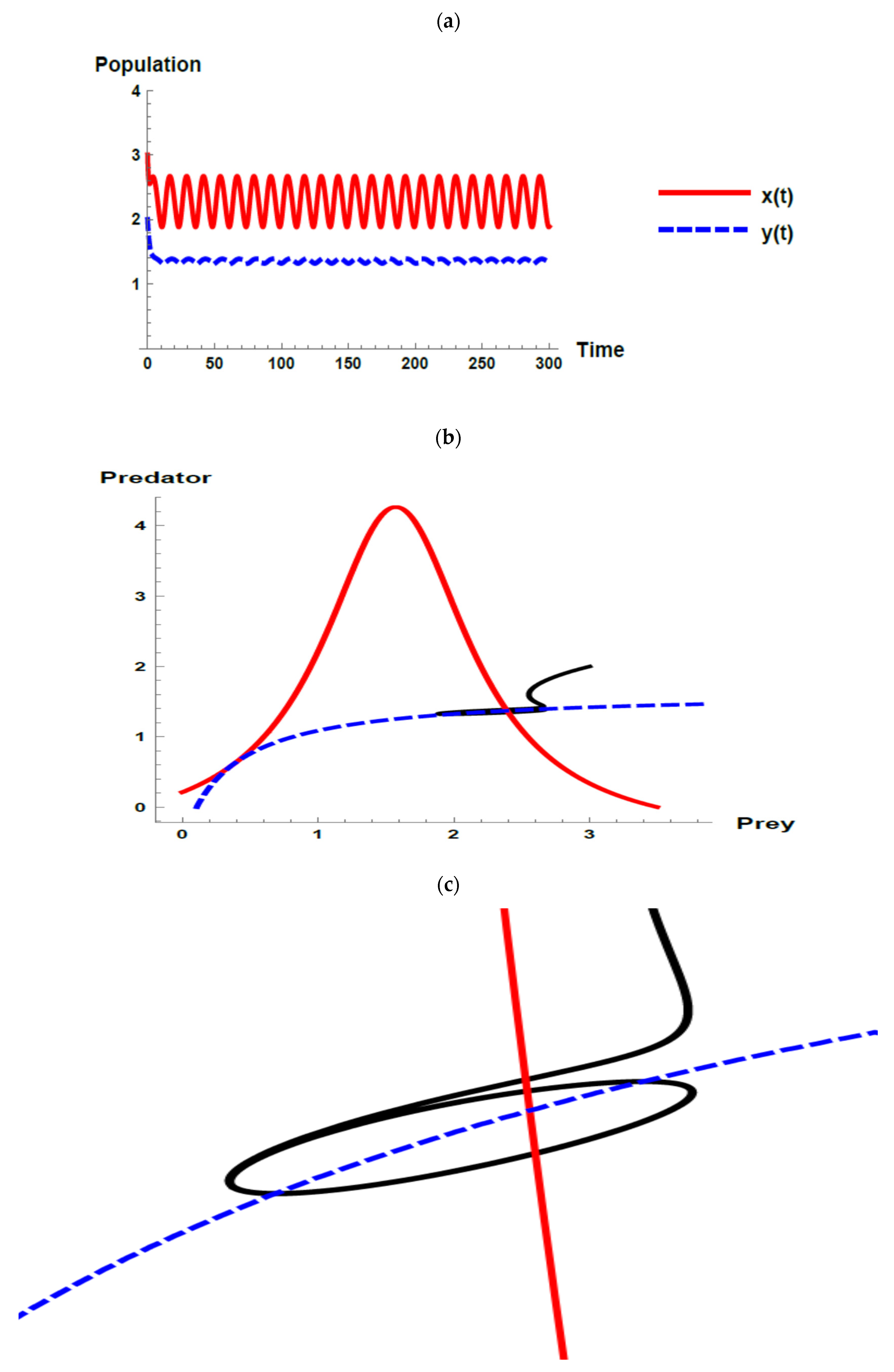
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).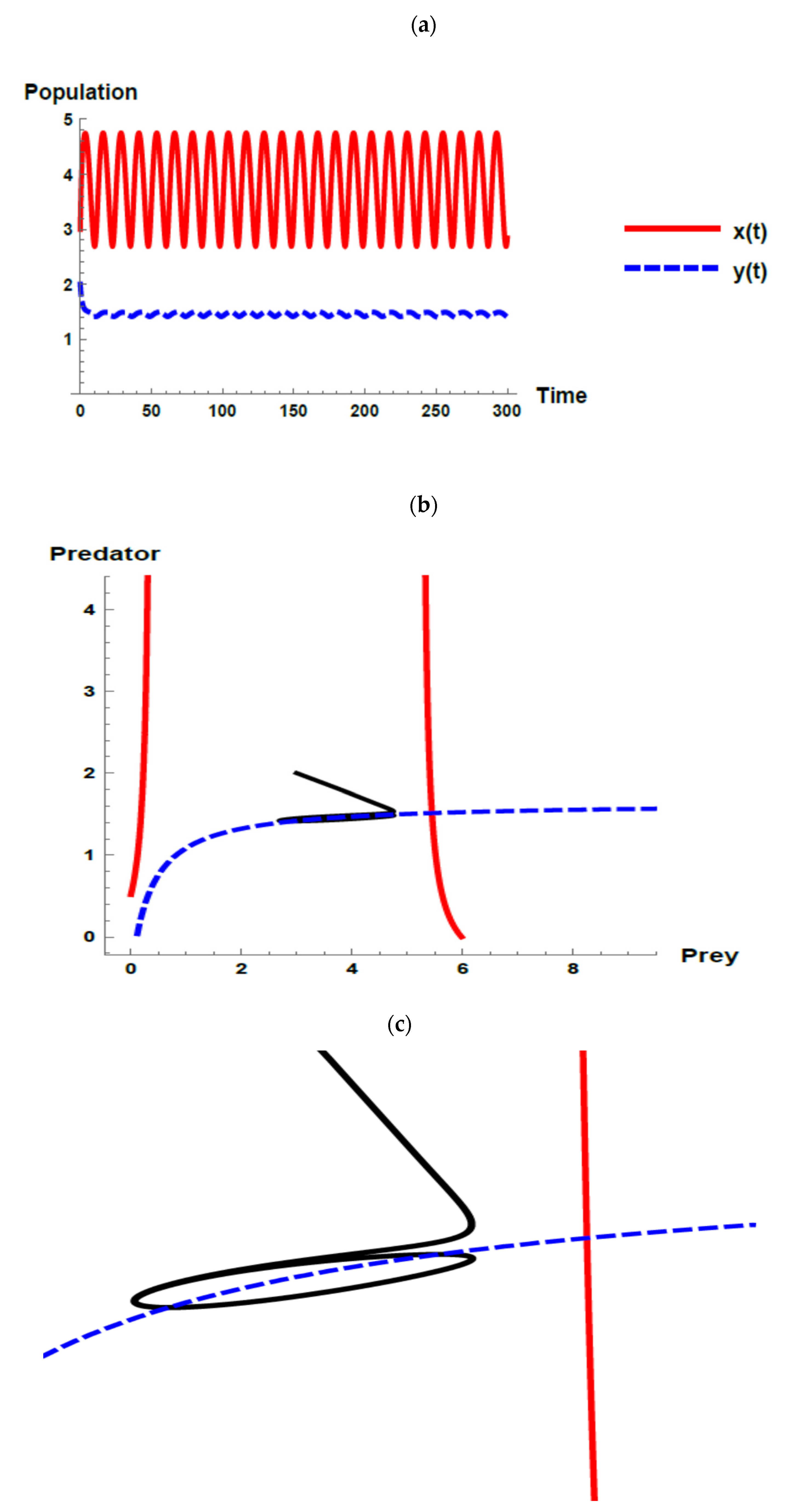
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
 ) and predator (
) and predator ( ) populations and phase plane trajectories; (c) cross-sectional picture of (b).
) populations and phase plane trajectories; (c) cross-sectional picture of (b).
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alebraheem, J. Dynamics of a Predator–Prey Model with the Effect of Oscillation of Immigration of the Prey. Diversity 2021, 13, 23. https://doi.org/10.3390/d13010023
Alebraheem J. Dynamics of a Predator–Prey Model with the Effect of Oscillation of Immigration of the Prey. Diversity. 2021; 13(1):23. https://doi.org/10.3390/d13010023
Chicago/Turabian StyleAlebraheem, Jawdat. 2021. "Dynamics of a Predator–Prey Model with the Effect of Oscillation of Immigration of the Prey" Diversity 13, no. 1: 23. https://doi.org/10.3390/d13010023
APA StyleAlebraheem, J. (2021). Dynamics of a Predator–Prey Model with the Effect of Oscillation of Immigration of the Prey. Diversity, 13(1), 23. https://doi.org/10.3390/d13010023




