Next Article in Journal
Modulation of p75NTR on Mesenchymal Stem Cells Increases Their Vascular Protection in Retinal Ischemia-Reperfusion Mouse Model
Next Article in Special Issue
Unveiling the N-Terminal Homodimerization of BCL11B by Hybrid Solvent Replica-Exchange Simulations
Previous Article in Journal
Potentiometric Study of Carbon Nanotube/Surfactant Interactions by Ion-Selective Electrodes. Driving Forces in the Adsorption and Dispersion Processes
Previous Article in Special Issue
Downhill, Ultrafast and Fast Folding Proteins Revised
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
IJMS
Volume 22
Issue 2
10.3390/ijms22020828
ijms-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Review

Double Mutant Cycles as a Tool to Address Folding, Binding, and Allostery

by
Livia Pagano
1,
Angelo Toto
1,
Francesca Malagrinò
1,
Lorenzo Visconti
1,
Per Jemth
2,* and
Stefano Gianni
1,*
1
Istituto Pasteur—Fondazione Cenci Bolognetti, Dipartimento di Scienze Biochimiche ‘A. Rossi Fanelli’ and Istituto di Biologia e Patologia Molecolari del CNR, Sapienza Università di Roma, 00185 Rome, Italy
2
Department of Medical Biochemistry and Microbiology, Uppsala University, SE-75123 Uppsala, Sweden
*
Authors to whom correspondence should be addressed.
Int. J. Mol. Sci. 2021, 22(2), 828; https://doi.org/10.3390/ijms22020828
Submission received: 31 December 2020 / Revised: 13 January 2021 / Accepted: 13 January 2021 / Published: 15 January 2021
(This article belongs to the Special Issue Protein Folding and Misfolding ---- Structure and Functions)
Browse Figures
Versions Notes

Abstract

:
Quantitative measurement of intramolecular and intermolecular interactions in protein structure is an elusive task, not easy to address experimentally. The phenomenon denoted ‘energetic coupling’ describes short- and long-range interactions between two residues in a protein system. A powerful method to identify and quantitatively characterize long-range interactions and allosteric networks in proteins or protein–ligand complexes is called double-mutant cycles analysis. In this review we describe the thermodynamic principles and basic equations that underlie the double mutant cycle methodology, its fields of application and latest employments, and caveats and pitfalls that the experimentalists must consider. In particular, we show how double mutant cycles can be a powerful tool to investigate allosteric mechanisms in protein binding reactions as well as elusive states in protein folding pathways.

1. Introduction

A rigorous description of any physical system demands the detailed characterization of its structural elements, as well as the quantitative investigation of its energetic components. In the case of proteins, whilst an arsenal of different experimental techniques has been developed to address the structural features of these molecules [1,2,3], the balance of forces regulating protein architecture, folding and function is much more difficult to address experimentally. In fact, such an aim is complicated by the very small changes in energy between all the different populated conformational states and their rapid rates of inter-conversion [4]. Furthermore, because protein sequences include a combination of hydrophobic (non-polar) and hydrophilic (polar) regions, the transient interactions of these regions with water molecules result in a very complex scenario and a quantitative prediction of all contributing Gibbs free energies is still elusive.
How can we approach this problem? The so-called ‘double mutant cycles’ approach is a powerful method to measure the strength of molecular contacts between interacting side-chains [5,6,7,8,9]. This technique is generally based on the synergy between site-directed mutagenesis and quantitative measurement of biophysical properties of a protein system. Here we provide a review focused on this experimental methodology. In particular, we recapitulate the key principles of double mutant cycle analysis, exemplifying some possible applications and we discuss the method in light of published work. Furthermore, we emphasize the key caveats and pitfalls associated with double mutant cycle.

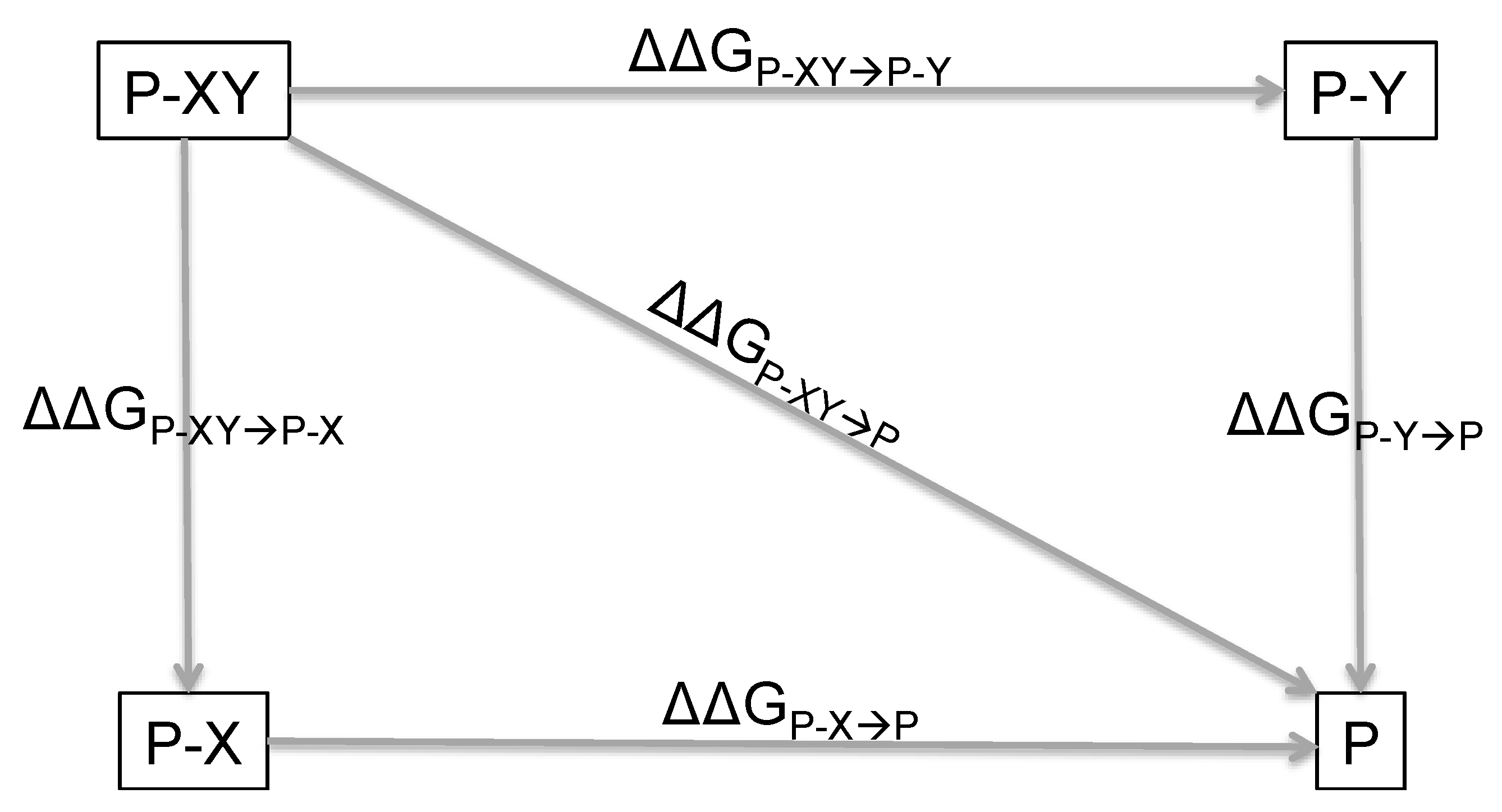
2. Principles of Double Mutant Cycles and Basic Equations

In his seminal analysis of the theory of heredity [10], William Bateson observed that, for several genetic features, the concept of ‘dominant’ and ‘recessive’, previously introduced by Mendel, was inadequate. In fact, in some cases, it was clear that the effect of a mutation in given gene was severely influenced by one or more other genes. In these cases, the term ‘epistasis’ was suggested, reflecting the condition whereby the phenotypic manifestation of a mutation is dependent on the genetic background in which it appears [11,12]. Analogously, ever since the early days of protein engineering, it was clear that some features of amino-acid side chains in regulating protein function were dependent on other side chains. In fact, only two years after the purification of the very first site-directed mutant, performed on the tyrosyl-tRNA synthetase in 1982 [13], it was observed on the same enzyme that the role of two residues in the active site was affected by the conformational change introduced by a threonine to proline mutation in a distal position, thereby introducing the concept of ‘coupling’ [14].
It is of critical importance to define quantitatively the extent of coupling between two interacting amino acids as well as to introduce a feasible methodology to measure it, the double mutant cycle [5,6,7,8,9]. In principle, a double mutant cycle assumes that when a perturbation is introduced in two non-interacting residues, X and Y, the effect of each single perturbation is additive in a double mutant where both perturbations are present. Thus, the change in free energy upon mutation of X, associated with any structural or functional behavior of a given protein, may be expressed as ΔΔGP-XY→P-Y. Analogously, the change in free energy associated with the residue Y will be equal to ΔΔGP-XY→P-X. If the mutations are independent of each other, then a double mutant of X and Y will display as:
ΔΔGP-XY→P = ΔΔGP-XY→P-Y + ΔΔGP-XY→P-X
Conversely, a non-zero value of:
ΔΔΔGXY = ΔΔGP-XY→P − ΔΔGP-XY→P-Y − ΔΔGP-XY→P-X
would correspond to the free energy of interaction between the X and Y and would therefore correspond to the coupling free energy of X and Y with respect to the probed structural or functional property of the protein. The ΔΔΔGXY measures therefore the energetic strength of the interaction between positions X and Y and, when its value is different from zero, it represents the interaction (or coupling) energy between the two residues. A scheme summarizing the basic principles and equations of double mutant cycles is reported in Figure 1.

3. The Double Mutant Cycle: Strengths, Caveats, and Pitfalls

When introducing an experimental technique, it is of particular importance to highlight its major advantages, as well as the caveats and pitfalls. Thus, what is the advantage to perform double mutant cycles as compared to single site mutagenesis? When mutating an amino acid side-chain into another, the change in free energy upon mutation ΔGN-N′ is in fact the sum of different components [15]: (i) The change in free energy of covalent bonds, ΔGcov; (ii) that arising from the changes in noncovalent bonds located at the site of mutation, ΔGnoncov; (iii) any additional free energy changes related to a reorganization of the protein, ΔGreorg; and (iv) changes in solvation energy, ΔGsolv. When performing folding and/or binding experiments, the ΔGcov is conserved in all states and, therefore, it cancels out in the analysis. On the other hand, ΔGnoncov is generally assumed to be the dominant contributor to ΔGN-N′. However, the contribution of the four ΔG values may differ for different states. For example, the values of ΔGreorg and ΔGsolv may be different in native and denatured states for a folding reaction, such that ΔGN-N’-ΔGD-D′ will report not only on the energetics at the mutation site ΔGnoncov. This effect will complicate the analysis of the experimental data. A similar situation may occur for bound and free states for a bimolecular interaction.
To exemplify this scenario, let us consider the binding between a protein and a ligand:
PN + L = PNL
When and if a site-directed mutation is introduced in PN, the folding stability of the protein could be affected, such that its native state is destabilized. Thus, by considering the scheme:
PD = PN + L = PNL
In the latter case, binding is considered in conjunction with a large conformational change, such as the (un)folding of the protein. In this case, it is clear that the apparent KD would be affected by the presence of a linked equilibrium, such that:
K D a p p = K D 1 1 + 1 K D − N
Thus, if the mutation affects the stability of the native state such that a significant population of the protein is shifted towards the denatured state, the apparent binding constant is also affected; even in cases in which the mutation would not perturb the binding interface at all. In those cases, ΔGreorg and ΔGsolv are dominant over the value of ΔGnoncov, and any analysis of the local effect of the mutation is prevented.
By considering these premises, it is clear that the double mutant cycle, which relies on calculating the difference between the effects on the double mutant over the two single variants, is extremely powerful. In fact, even in cases in which the mutation results in structural shifts of the protein, as exemplified above, those found in the single mutants are usually present in the double mutant and, therefore, their energetic effects in the double mutant cycle are cancelled out [16,17,18]. These considerations reinforce the importance of this method as a tool to investigate directly the energetics of interaction between two residues.
Due to the complexity of site directed mutagenesis, it is important to stress that conservative mutations should be used to probe binding or folding [19]. Values of ΔGreorg and ΔGsolv for conservative mutations are more likely to be lower than the value of ΔGnoncov, which, as briefly discussed above, tends to simplify the analysis. Additionally, since the coupling free energy arises from the difference between the value obtained for the double mutant minus the sum of the values obtained from the single mutants, it might be difficult to calculate a reliable value of ΔΔΔGXY when the effect of one mutation is very large such that limitations in the biophysical method result in a large experimental error. For example, isothermal titration calorimetry usually works best in the range 100 nM–1 μM and mutations resulting in a 100-fold destabilization will lead to a large experimental error.
Over and above the theoretical basis of this type of analysis, there are also some practical issues that demand additional consideration. In fact, it is a good advice to choose the mutations properly. Since the double mutant cycle technique is aimed at measuring the free energy of interactions between two residues, it is recommended to keep the two terms ΔGreorg and ΔGsolv as low as possible. Consequently, since mutations of large hydrophobic side chains may lead to very large ΔGreorg and ΔGsolv, these are not well suited for double mutant cycles. Thus, in analogy to the Φ value analysis in protein folding studies [19], it is recommended to mutate hydrophobic side chains, introducing small side chain deletions and without altering the stereochemistry (i.e., Ile→Val→Ala→Gly; Leu→Ala→Gly; Thr→Ser; Phe→Ala→Gly). Additionally, analogously to what is normally suggested in the case of the Φ value analysis in protein folding, a reliable dataset is generally based on a large number of site-directed mutants, allowing to probe pairwise residue interactions encompassing a relevant fraction of the protein structure.
After the mutations are introduced, it is critical to measure the changes in free energy accurately. This task may be achieved by employing the standard spectroscopic methodologies that are classically used in affinity and/or stability measurements for proteins. These techniques may span from equilibrium titration with spectroscopic techniques such as absorbance, fluorescence, circular dichroism, etc., to other methods, such as calorimetry.

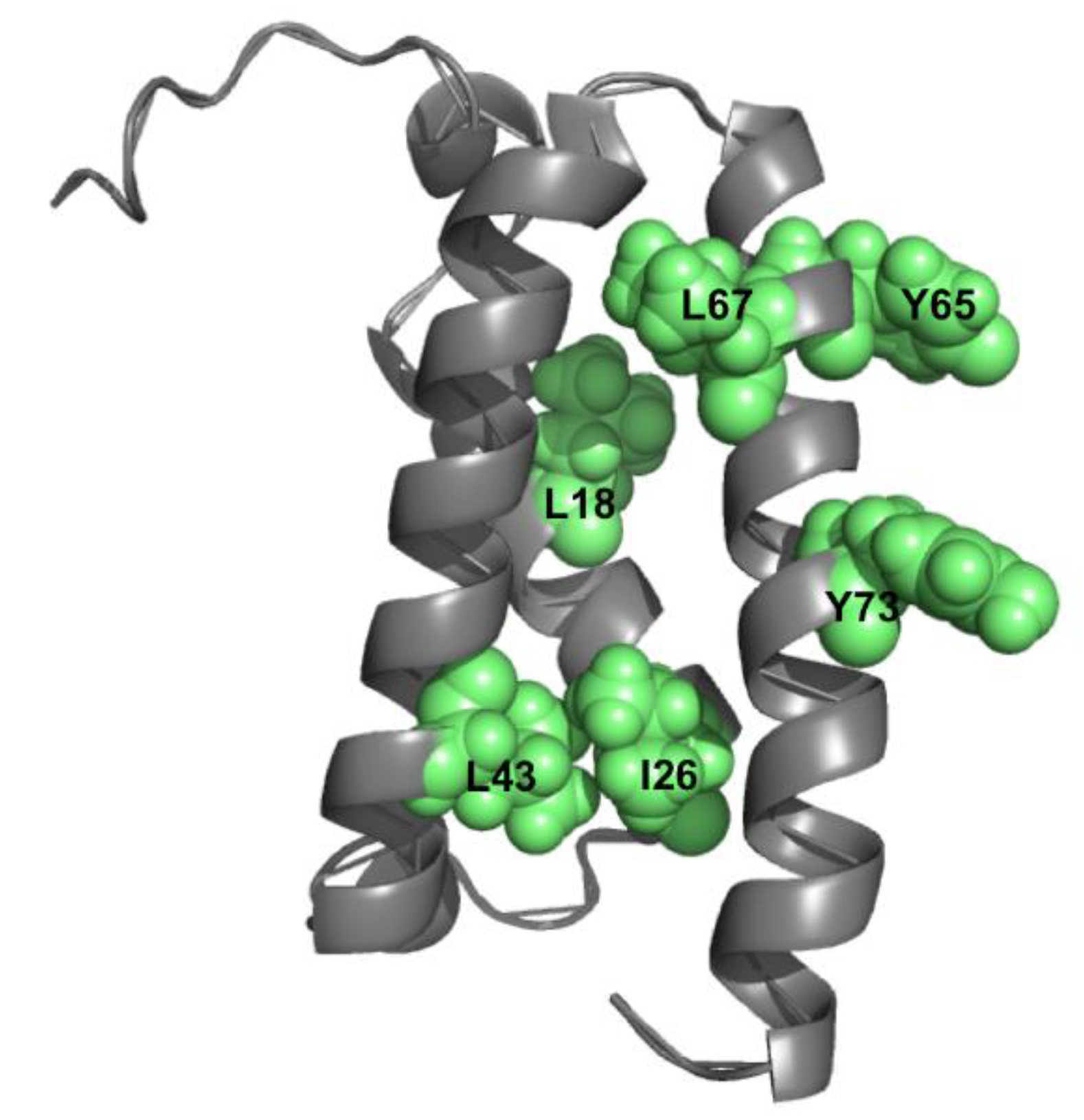
4. Double Mutant Cycles to Understand Intramolecular Interactions

The invention of double mutant cycles was associated with the quantification of intramolecular interactions in proteins. In their pioneering work on protein engineering Winter, Fersht, and co-workers observed that, in the case of a tyrosyl-tRNA synthetase, a variant in which a threonine residue was mutated into proline increased the affinity of the enzyme for ATP [13]. By making mutants of the proline-containing enzyme at two other positions directly involved in ATP binding, it was possible to show that the presence of the proline improves the strength of one of these contacts. On the basis of these observations, it was proposed that the propagation of a structural change in an enzyme induced by mutation could be explored by the use of further mutations, thereby introducing the double mutant cycle methodology as a unique tool for the investigation of intramolecular interactions in proteins.
Following this original work, several protein systems of known structure have been subjected to double mutant cycles [6]. In these cases, the method represents a powerful tool to unveil the details of distinct interactions in protein stability and function. In this context, it is worth to highlight the employment of double mutant cycles to clarify the role of surface and buried salt bridges in protein stability, as well as charge-aromatic interactions. Importantly, the cycles have also been used in more complex approaches with three interacting residues, implying the design of multidimensional cycles. Barnase [20,21,22], staphylococcal nuclease [23,24], and lambda repressor [25,26] are three examples of proteins subjected to double mutant cycle analysis.
Over and above the cases in which double mutant cycles have been employed to address intramolecular interactions in known structures, it is important to note that, in some context, the approach can be used to investigate elusive states that are difficult to characterize experimentally. The KIX domain is a globular domain that is a part of a large coactivator protein called CBP [27]. The three-dimensional structure of KIX consists of three α-helices and two short 310− helices (Figure 2). A detailed characterization of its folding pathway revealed that, whilst the protein appears to fold in a simple two-state manner, its denatured state retains a considerable amount of structure [28], as probed by the analysis of the so-called m values, a set of parameters related to the change in accessible surface area upon (un)folding [29]. To address the structural details of the residual structure in the denatured state of KIX, double mutant cycles were performed [28]. In particular, by focusing primarily on the single variants that had a major effect on the folding m values, a series of double mutants were produced and characterized. Interestingly, the folding characterization of these mutants allowed mapping the presence of distinct non-native interactions in the denatured state, as well as in measuring their strength in terms of ΔΔΔG. A similar analysis of structure in the denatured state was also performed on the C-terminal domain of nucleophosmin by conducting folding and unfolding experiments on several site-directed variants at different experimental conditions [30].

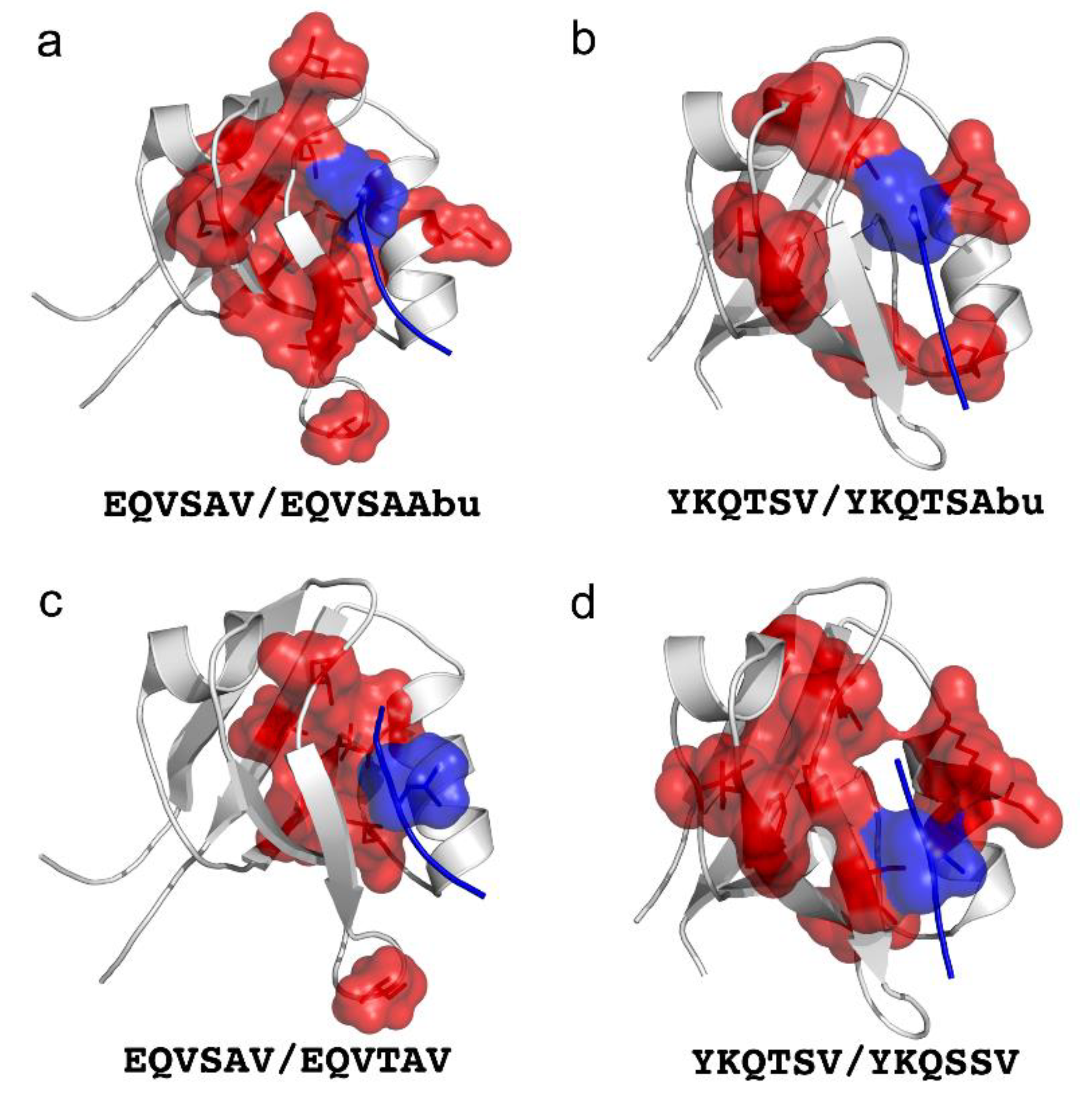
5. Protein Binding and Allostery

Ever since the pioneering work of Kendrew and Perutz, it became clear that a deep understanding of proteins’ structure and function would depend on the rigorous description of their dynamic properties. In fact, only the identification of the R and T quaternary states of haemoglobin provided a structural framework to understand its cooperative and allosteric behavior, pinpointing the role of residues ‘other’ (from the Greek allo-) than those in the binding site in regulating function [31]. In this context, when studying the binding of two molecules, it is clear that double mutant cycles may provide a straightforward method to analyze binding and allostery [16,32]. When studying a number of i mutants in the A protein, binding to j mutants of the B protein, it is possible to address i × j double mutant cycles and define their interactions quantitatively. In practical terms, in those cases, it would be required to perform the experiments: (i) on the mutant of protein A versus the wild-type form of the protein B; (ii) on the wild-type of protein A versus the mutant form of the protein B; (iii) on all combinations of the mutant variants of both proteins. If the changes in free energies are not additive, the probed residues are energetically coupled.
The interaction between barnase and barstar represents a paradigm example of the use of double mutant cycles to understand protein-protein binding. In this case, several site-directed mutants were analyzed providing a detailed description of the energetics of such interactions [18,33]. In particular, it was found that the coupling free energy generally depends on the distance between the probed residues. Whilst residues closer than 7 Å tend to interact co-operatively, at greater distances the changes in free energy upon mutations become additive. However, it was found that the interaction network between functional residues is not always trivial [34,35,36,37], such that they can be directly deduced from the crystal structure. For example, the salt-bridge between Lys27 in barnase and Asp39 of barstar was found to be very strong, while that between Glu76 of barstar and Arg59 of barnase is relatively weak, showing the importance of the local environment for binding [38], as well as the need to complement the structural data with biophysical experiments.
The double mutant cycle approach has been subsequently employed on other protein systems, with the specific aim to quantify the interaction networks between interacting protein systems. Relevant examples include coiled-coil XGCN4-p1 [39], as well as characterization of the allosteric network of different PDZ [40,41,42,43] and SH3 domains [44]. In all these cases, it was possible to identify energetic coupling between residues that were not necessarily in direct contact in the three-dimensional structure of the respective protein complexes, confirming the concept that allosteric networks tend to follow intricate pathways that demand a careful experimental investigation.
Out of the examples highlighted above, it is particularly interesting to discuss some of the conclusions that have been drawn on PDZ domains [40,41,42,43]. In fact, the experimental data suggested that, in the majority of cases, a positive coupling energy for the Val0 to Abu substitution was observed in both PDZ2 from PTP-BL and PDZ3 from PSD95 (Figure 3), although the absolute values were variable. Thus, the effect of the mutations in the protein on binding was more pronounced when the wild-type peptide was used, as compared to that in which a methyl group was removed from position 0. This intriguing finding suggested that the entire PDZ structure could been under selective pressure to optimize the binding of its physiological ligand. Sequence variation in the protein domain is therefore not neutral to peptide binding, strongly indicating that selectivity by the domain is not solely determined by the subset of residues directly involved in ligand binding. Importantly, this finding appears to support a scenario whereby the cross-talk between binding sites and remote residues may be used to fine tune target selectivity, and possibly to decrease the cross-reactivity between homologous PDZ domains.
The complex nature of the functions of proteins often demands their structure to be composed of multiple domains. In these cases, it is very frequent that function and binding may depend on the interdomain architecture and dynamics, such that the individual domains in isolation display different features compared to when they are present in multidomain constructs. While this concept is widely accepted, our detailed knowledge about the effects of supertertiary structure in folding and function is still limited. In this context, double mutant cycles proved very useful. An extensive double mutant cycle analysis of a PDZ domain, both in isolation and in the context of a supramodule comprising the PDZ domain, an SH3 and a GK domain, showed that allosteric networks are highly sensitive to the supertertiary structure [45]. In particular, it was found that the presence of the SH3–GK tandem resulted in strong coupling from the bound peptide ligand to the β1β2 loop, β2β3 loop, and α3 helix that was not observed with the single PDZ3 domain. These findings prompted the authors to reinforce the importance of extending double mutant cycle experiments to multidomain systems.

6. Double Mutant Cycles In Silico

The double mutant cycle methodology generally relies on the design, production and characterization of protein mutant variants. Nevertheless, an interesting application of this method is an in silico analysis only. Horovitz and co-workers, who investigated the interaction between two cysteine residues, namely Cys137 and Cys518, in the Escherichia coli chaperonin GroEL, first introduced this approach [46]. By performing a multiple sequence alignment on proteins belonging to the Hsp60 family, they found that naturally-occurring variations at positions 137 and 518 were likely to be coordinated. An experimental validation of this interaction further substantiated this analysis and suggested that the study of the co-variance of residues at different positions may represent a valuable method to detect interactions in protein systems.
In a seminal study published in Science, Lockless and Ranganathan extended the approach to a larger scale [47]. By using the PDZ domain family as a model system, the authors investigated systematically the evolutionary coupling between the different positions of the domain. The principle of the method is that a statistical coupling between two sites, i and j, may be defined as the degree to which amino acid frequencies at site i change in response to a perturbation of frequencies at another site, j. Then, by focusing on residue His76, which is in an important position in defining the specificity of PDZ domains, a set of energetically coupled positions for the binding site was identified, including unexpected long-range interactions. Whilst extremely interesting, however, this study was subsequently reassessed and it was found that the energetic coupling was not a special feature of the coevolved network of residues in PDZ domains [48,49]. In addition, as mentioned, in the context of the PDZ3-SH3-GK supramodule the experimentally determined allosteric network for PDZ3 is different from that in isolation [50]. Thus, statistical coupling from sequence analysis is not necessarily a reporter of energetic coupling and allostery and must, therefore, be supported with extensive experimental data.

7. Double Mutant Cycles by Native Mass Spectrometry

A very interesting application of double mutant cycles has been recently implemented by studying the formation of protein-protein interactions via native mass spectrometry [51]. The intrinsic power of this technique lies in the ability to transfer protein complexes to the gas phase, without altering their native state [52,53,54]. Hence, by measuring a single high-resolution native mass spectrum and determining the intensities of the complexes formed by the two wild-type proteins, the complex of each wild-type protein with a mutant protein, and the complex of the two mutant proteins, it is possible to obtain the pairwise interaction energies between the two mutated residues with great precision. In fact, it can be demonstrated that native mass spectrometry both circumvents the determination of individual binding constants, which are of course prone to experimental error, and is independent of the concentration of the free unbound species [55]. Remarkably, the method may be employed directly on crude cell lysates [56], which further simplifies the experiments and interpretations. In fact, in these cases, the purification of the mutant variants may not be required, which simplifies the experimental approach consistently.
Double mutant cycles by mass spectrometry have been successfully employed to study the binding between the proteins E9 and Im2 [51] and, more recently, to understand the dimerization of SOD1 in naturally-occurring mutants associated with ALS. The authors analyzed the coupling constants by co-expressing wild-type SOD1 with ALS-causing mutations [57]. The result is a quantitative measurement of the inherent preference of these variants to form homo-dimers (mutant-mutant or wild type-wild type) rather than heterodimers (mutant-wild type). Of exceptional interest, the analysis successfully demonstrated that heterodimerization preference of SOD1 in ALS-causing mutations correlates with the reported average duration of the disease. Their findings suggest that heterodimerization of mutant variants of SOD1 is directly involved in the development of ALS and provide another clear example of how the initial events of self-assembly represent a critical point in the intimate link between protein misfolding and disease [58].

8. Conclusions

The double mutant cycles methodology represents a very powerful technique offering the tantalizing possibility to address directly the strength of pairwise interactions in proteins. As exemplified in the case of PDZ domains, the analysis of the sign of the observed energetic coupling may be used to describe protein selectivity in conserved homologous protein-protein interaction domains. Furthermore, in conjunction with other techniques, it may represent a future tool to address ambitious tasks, such as the understanding of structure of metastable states as well as the description of aggregation phenomena. With the specific aim to help the experimentalist, we have recapitulated here the key features, along with the associated caveats and pitfalls, as well as some key examples of applications.

Author Contributions

L.P., P.J., and S.G. wrote the first version of the manuscript. L.P., P.J., A.T., F.M., L.V. and S.G. discussed and revised the manuscript. L.P., P.J., A.T., F.M., L.V. and S.G. All authors have read and agreed to the published version of the manuscript.

Funding

Work partly supported by grants from Sapienza University of Rome (B52F16003410005, RP11715C34AEAC9B and RM1181641C2C24B9 to S.G), the Associazione Italiana per la Ricerca sul Cancro (individual grant, IG 2020, 24551, to S.G.), the Istituto Pasteur Italia (Teresa Ariaudo Research Project 2018, to A.T.) and the Swedish Research Council (2020-04395 to P.J.). F.M. is the recipient of a FIRC-AIRC fellowship.

Conflicts of Interest

The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript or in the decision to publish the results.

References

  1. Yip, K.M.; Fischer, N.; Paknia, E.; Chari, A.; Stark, H. Atomic-resolution protein structure determination by cryo-EM. Nature 2020, 587, 157–161. [Google Scholar] [CrossRef] [PubMed]
  2. Wüthrich, K. Protein structure determination in solution by nmr spectroscopy. World Sci. Res. 1995, 5, 11–14. [Google Scholar]
  3. Dobson, C.M. Biophysical techniques in structural biology. Annu. Rev. Biochem. 2019, 88, 25–33. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  4. Fersht, A.R. Structure and Mechanism in Protein Science; Freeman: New York, NY, USA, 1999. [Google Scholar]
  5. Horovitz, A.; Fleisher, R.C.; Mondal, T. Double-mutant cycles: New directions and applications. Curr. Opin. Struct. Biol. 2019, 58, 10–17. [Google Scholar] [CrossRef]
  6. Horovitz, A. Double-mutant cycles: A powerful tool for analyzing protein structure and function. Fold. Des. 1996, 1, 121–126. [Google Scholar] [CrossRef] [Green Version]
  7. Cockroft, S.L.; Hunter, C.A. Chemical double-mutant cycles: Dissecting non-covalent interactions. Chem. Soc. Rev. 2007, 36, 172–188. [Google Scholar] [CrossRef]
  8. Sali, D.; Bycroft, M.; Fersht, A.R. Surface electrostatic interactions contribute little of stability of barnase. J. Mol. Biol. 1991, 220, 779–788. [Google Scholar]
  9. Otzen, D.E.; Fersht, A.R. Analysis of protein-protein interactions by mutagenesis: Direct versus indirect effects. Protein. Eng. 1999, 12, 41–45. [Google Scholar] [CrossRef] [Green Version]
  10. Bateson, W. Mendel’s Principles of Heredity: A Defence; Cambridge University Press: Cambridge, UK, 1902. [Google Scholar]
  11. Moore, J.H. A global view of epistasis. Nat. Genet. 2005, 37, 13–14. [Google Scholar] [CrossRef]
  12. Starr, T.N.; Thornton, J.W. Epistasis in protein evolution. Protein Sci. 2016, 25, 1204–1218. [Google Scholar] [CrossRef] [Green Version]
  13. Winter, G.; Fersht, A.R.; Wilkinson, A.J.; Zoller, M.; Smith, M. Redesigning enzyme structure by site-directed mutagenesis: Tyrosyl tRNA synthetase and ATP binding. Nature 1982, 299, 756–758. [Google Scholar] [CrossRef] [PubMed]
  14. Carter, P.J.; Winter, G.; Wilkinson, A.J.; Fersht, A.R. The use of double mutants to detect structural changes in the active site of the tyrosyl-tRNA synthetase (Bacillus stearothermophilus). Cell 1984, 38, 835–840. [Google Scholar] [CrossRef]
  15. Fersht, A.R.; Sato, S. Φ-Value analysis and the nature of protein-folding transition states. Proc. Natl. Acad. Sci. USA 2004, 101, 7976–7981. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  16. Horovitz, A.; Fersht, A.R. Strategy for analysing the co-operativity of intramolecular interactions in peptides and proteins. J. Mol. Biol. 1990, 214, 613–617. [Google Scholar] [CrossRef]
  17. Fersht, A.R.; Matouschek, A.; Serrano, L. The folding of an enzyme. I. Theory of protein engineering analysis of stability and pathway of protein folding. J. Mol. Biol. 1992, 224, 771–782. [Google Scholar] [CrossRef]
  18. Serrano, L.; Horovitz, A.; Avron, B.; Bycroft, M.; Fersht, A.R. Estimating the contribution of engineered surface electrostatic interactions to protein stability by using double-mutant cycles. Biochemistry 1990, 29, 9343–9352. [Google Scholar] [CrossRef]
  19. Sato, S.; Religa, T.L.; Fersht, A.R. Φ-Analysis of the folding of the b domain of protein a using multiple optical probes. J. Mol. Biol. 2006, 360, 850–864. [Google Scholar] [CrossRef] [PubMed]
  20. Serrano, L.; Bycroft, M.; Fersht, A.R. Aromatic-aromatic interactions and protein stability: Investigation by double-mutant cycles. J. Mol. Biol. 1991, 218, 465–475. [Google Scholar] [CrossRef]
  21. Horovitz, A.; Serrano, L.; Avron, B.; Bycroft, M.; Fersht, A.R. Strength and co-operativity of contributions of surface salt bridges to protein stability. J. Mol. Biol. 1990, 216, 1031–1044. [Google Scholar] [CrossRef]
  22. Vaughan, C.K.; Harryson, P.; Buckle, A.M.; Fersht, A.R. A structural double-mutant cycle: Estimating the strength of a buried salt bridge in barnase. Acta Crystallogr. D 2002, 58, 591–600. [Google Scholar] [CrossRef] [Green Version]
  23. Green, S.M.; Shortle, D. Patterns of nonadditivity between pairs of stability mutations in staphylococcal nuclease. Biochemistry 1993, 32, 10131–10139. [Google Scholar] [CrossRef] [PubMed]
  24. Chen, J.; Stites, W.E. Energetics of side chain packing in staphylococcal nuclease assessed by systematic double mutant cycles. Biochemistry 2001, 40, 14004–14011. [Google Scholar] [CrossRef] [PubMed]
  25. Marqusee, S.; Sauer, R.T. Contributions of a hydrogen bond/salt bridge network to the stability of secondary and tertiary structure in lambda repressor. Protein Sci. 1994, 3, 2217–2225. [Google Scholar] [CrossRef] [PubMed]
  26. Myers, J.K.; Oas, T.G. Contribution of a buried hydrogen bond to λ repressor folding kinetics. Biochemistry 1999, 38, 6761–6768. [Google Scholar] [CrossRef] [PubMed]
  27. Thakur, J.K.; Yadav, A.; Yadav, G. Molecular recognition by the KIX domain and its role in gene regulation. Nucleic Acids Res. 2014, 42, 2112–2125. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  28. Troilo, F.; Bonetti, D.; Toto, A.; Visconti, L.; Brunori, M.; Longhi, S.; Gianni, S. The folding pathway of the KIX domain. J. Am. Chem. Soc. 2017, 12, 1683–1690. [Google Scholar] [CrossRef]
  29. Myers, J.K.; Pace, C.N.; Scholtz, J.M. Denaturant m values and heat capacity changes: Relation to changes in accessible surface areas of protein unfolding. Protein Sci. 1995, 4, 2138–2148. [Google Scholar] [CrossRef] [Green Version]
  30. Scaloni, F.; Gianni, S.; Federici, L.; Falini, B.; Brunori, M. Folding mechanism of the C-terminal domain of nucleophosmin: Residual structure in the denatured state and its pathophysiological significance. Fed. Proc. 2009, 23, 2360–2365. [Google Scholar] [CrossRef]
  31. Perutz, M.F.; Kendrew, J.C.; Watson, H.C. Structure and function of haemoglobin: II. Some relations between polypeptide chain configuration and amino acid sequence. J. Mol. Biol. 1965, 13, 669–678. [Google Scholar] [CrossRef]
  32. Goodey, N.M.; Benkovic, S.J. Allosteric regulation and catalysis emerge via a common route. Nat. Chem. Biol. 2008, 4, 474–482. [Google Scholar] [CrossRef]
  33. Schreiber, G.; Fersht, A.R. Rapid, electrostatically assisted association of proteins. Nat. Struct. Biol. 1996, 3, 427–431. [Google Scholar] [CrossRef] [PubMed]
  34. Ming, D.; Chen, R.; Huang, H. Amino-acid network clique analysis of protein mutation non-additive effects: A case study of lysozme. Int. J. Mol. Sci. 2018, 19, 1427. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  35. Rajasekaran, N.; Sekhar, A.; Naganathan, A.N. A universal pattern in the percolation and dissipation of protein structural perturbations. J. Phys. Chem. Lett. 2017, 8, 4779–4784. [Google Scholar] [CrossRef] [PubMed]
  36. Horovitz, A. Non-additivity in protein-protein interactions. J. Mol. Biol. 1987, 196, 733–735. [Google Scholar] [CrossRef]
  37. Nussinov, R.; Tsai, C.-J. Allostery without a conformational change? Revisiting the paradigm. Curr. Opin. Struct. Biol. 2015, 30, 17–24. [Google Scholar] [CrossRef]
  38. Schreiber, G.; Fersht, A.R. Energetics of protein-protein interactions: Analysis of the Barnase-barstar interface by single mutations and double mutant cycles. J. Mol. Biol. 1995, 248, 478–486. [Google Scholar] [CrossRef]
  39. Ibarra-Molero, B.; Zitzewitz, J.A.; Matthews, C.R. Salt-bridges can stabilize but do not accelerate the folding of the homodimeric coiled-coil peptide GCN4-p1. J. Mol. Biol. 2004, 336, 989–996. [Google Scholar] [CrossRef]
  40. Jemth, P.; Gianni, S. PDZ domains:  Folding and binding. Biochemistry 2007, 46, 8701–8708. [Google Scholar] [CrossRef]
  41. Gianni, S.; Haq, S.R.; Montemiglio, L.C.; Jürgens, M.C.; Engström, Å.; Chi, C.N.; Brunori, M.; Jemth, P. Sequence-specific long range networks in PSD-95/Discs Large/ZO-1 (PDZ) domains tune their binding selectivity. J. Biol. Chem. 2011, 286, 27167–27175. [Google Scholar] [CrossRef] [Green Version]
  42. Hultqvist, G.; Haq, S.R.; Punekar, A.S.; Chi, C.N.; Engström, Å.; Bach, A.; Strømgaard, K.; Selmer, M.; Gianni, S.; Jemth, P. Energetic pathway sampling in a protein interaction domain. Structure 2013, 21, 1193–1202. [Google Scholar] [CrossRef] [Green Version]
  43. Eildal, J.N.N.; Hultqvist, G.; Balle, T.; Stuhr-Hansen, N.; Padrah, S.; Gianni, S.; Strømgaard, K.; Jemth, P. Probing the role of backbone hydrogen bonds in protein-peptide interactions by amide-to-ester mutations. J. Am. Chem. Soc. 2013, 135, 12998–13007. [Google Scholar] [CrossRef] [PubMed]
  44. Malagrinò, F.; Troilo, F.; Bonetti, D.; Toto, A.; Gianni, S. Mapping the allosteric network within a SH3 domain. Sci. Rep. 2019, 9, 8279. [Google Scholar] [CrossRef] [PubMed]
  45. Laursen, L.; Kliche, J.; Gianni, S.; Jemth, P. Supertertiary protein structure affects an allosteric network. Proc. Natl. Acad. Sci. USA 2020, 117, 24294–24304. [Google Scholar] [CrossRef] [PubMed]
  46. Horovitz, A.; Bochkareva, E.S.; Yifrach, O.; Girshovich, A.S. Prediction of an inter-residue interaction in the chaperonin groel from multiple sequence alignment is confirmed by double-mutant cycle analysis. J. Mol. Biol. 1994, 238, 133–138. [Google Scholar] [CrossRef]
  47. Lockless, S.W.; Ranganathan, R. Evolutionarily conserved pathways of energetic connectivity in protein families. Science 1999, 286, 295–299. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  48. Chi, C.N.; Elfstrom, L.; Shi, Y.; Snall, T.; Engstrom, A.; Jemth, P. Reassessing a sparse energetic network within a single protein domain. Proc. Natl. Acad. Sci. USA 2008, 105, 4679–4684. [Google Scholar] [CrossRef] [Green Version]
  49. Fodor, A.A.; Aldrich, R.W. On evolutionary conservation of thermodynamic coupling in proteins. J. Biol. Chem. 2004, 279, 19046–19050. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  50. Gautier, C.; Troilo, F.; Cordier, F.; Malagrinò, F.; Toto, A.; Visconti, L.; Zhu, Y.; Brunori, M.; Wolff, N.; Gianni, S. Hidden kinetic traps in multidomain folding highlight the presence of a misfolded but functionally competent intermediate. Proc. Natl. Acad. Sci. USA 2020, 117, 19963–19969. [Google Scholar] [CrossRef]
  51. Sokolovski, M.; Cveticanin, J.; Hayoun, D.; Korobko, I.; Sharon, M.; Horovitz, A. Measuring inter-protein pairwise interaction energies from a single native mass spectrum by double-mutant cycle analysis. Nat. Commun. 2017, 8, 212. [Google Scholar] [CrossRef] [Green Version]
  52. Chandler, S.A.; Benesch, J.L. Mass spectrometry beyond the native state. Curr. Opin. Chem. Biol. 2018, 42, 130–137. [Google Scholar] [CrossRef]
  53. Mehmood, S.; Allison, T.M.; Robinson, C.V. Mass spectrometry of protein complexes: From origins to applications. Annu. Rev. Phys. Chem. 2015, 66, 453–474. [Google Scholar] [CrossRef] [PubMed]
  54. Lössl, P.; van de Waterbeemd, M.; Heck, A.J. The diverse and expanding role of mass spectrometry in structural and molecular biology. EMBO Rep. 2016, 35, 2634–2657. [Google Scholar] [CrossRef] [PubMed]
  55. Sharon, M.; Horovitz, A. Probing allosteric mechanisms using native mass spectrometry. Curr. Opin. Struct. Biol. 2015, 34, 7–16. [Google Scholar] [CrossRef] [Green Version]
  56. Cveticanin, J.; Netzer, R.; Arkind, G.; Fleishman, S.J.; Horovitz, A.; Sharon, M. Estimating interprotein pairwise interaction energies in cell lysates from a single native mass spectrum. Anal. Chem. 2018, 90, 10090–10094. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  57. Cveticanin, J.; Mondal, T.; Meiering, E.M.; Sharon, M.; Horovitz, A. Insight into the autosomal-dominant inheritance pattern of SOD1-associated ALS from native mass spectrometry. J. Mol. Biol. 2020, 432, 5995–6002. [Google Scholar] [CrossRef] [PubMed]
  58. Chiti, F.; Dobson, C.M. Protein misfolding, amyloid formation, and human disease: A summary of progress over the last decade. Annu. Rev. Biochem. 2017, 86, 27–68. [Google Scholar] [CrossRef]
Ijms 22 00828 g001 550
Figure 1. Schematic representation of a double mutant cycle. P-XY is the wild-type protein with the two residues X and Y. P-X and P-Y are the variants where Y and X are mutated, respectively, and P is the corresponding double mutant. The change in free energy upon mutation of X (or Y) is equal to ΔΔGP-XY→P-Y (or ΔΔGP-XY→P-X) and ΔΔGP-XY→P is the change in free energy of the double mutant.
Figure 1. Schematic representation of a double mutant cycle. P-XY is the wild-type protein with the two residues X and Y. P-X and P-Y are the variants where Y and X are mutated, respectively, and P is the corresponding double mutant. The change in free energy upon mutation of X (or Y) is equal to ΔΔGP-XY→P-Y (or ΔΔGP-XY→P-X) and ΔΔGP-XY→P is the change in free energy of the double mutant.
Ijms 22 00828 g001
Ijms 22 00828 g002 550
Figure 2. Structural distribution of residues L18, I26, L43, Y65, L67, and Y73 (green spheres) in the KIX domain (PDB 2AGH). The analysis of folding m values, in combination with double mutant cycle analysis, allowed to characterize, from a thermodynamic perspective (i.e., measuring ΔΔΔG values), the role of these residues in stabilizing non-native interactions in the denatured state of KIX. With the exception of I26 and L43 that are in direct contact, residues displaying significant coupling free energies are located in different regions of the domain (see [28] for details).
Figure 2. Structural distribution of residues L18, I26, L43, Y65, L67, and Y73 (green spheres) in the KIX domain (PDB 2AGH). The analysis of folding m values, in combination with double mutant cycle analysis, allowed to characterize, from a thermodynamic perspective (i.e., measuring ΔΔΔG values), the role of these residues in stabilizing non-native interactions in the denatured state of KIX. With the exception of I26 and L43 that are in direct contact, residues displaying significant coupling free energies are located in different regions of the domain (see [28] for details).
Ijms 22 00828 g002
Ijms 22 00828 g003 550
Figure 3. Graphic representation of the different allosteric networks of PDZ domain-ligand complexes of protein tyrosine phosphatase Basophil-like PDZ2 (a and c panels) and PSD-95 PDZ3 (b and d panels) with their natural ligand (showed below each panel). All the mutated residues are represented as spheres both in the ligand (blue) and in the PDZ domain (red). The peptide ligand mutations Val→Abu (deletion of a γ-methyl group from Val), Ser→Thr or Thr→Ser were chosen because they interact with the binding pocket of PDZ domains.
Figure 3. Graphic representation of the different allosteric networks of PDZ domain-ligand complexes of protein tyrosine phosphatase Basophil-like PDZ2 (a and c panels) and PSD-95 PDZ3 (b and d panels) with their natural ligand (showed below each panel). All the mutated residues are represented as spheres both in the ligand (blue) and in the PDZ domain (red). The peptide ligand mutations Val→Abu (deletion of a γ-methyl group from Val), Ser→Thr or Thr→Ser were chosen because they interact with the binding pocket of PDZ domains.
Ijms 22 00828 g003
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Pagano, L.; Toto, A.; Malagrinò, F.; Visconti, L.; Jemth, P.; Gianni, S. Double Mutant Cycles as a Tool to Address Folding, Binding, and Allostery. Int. J. Mol. Sci. 2021, 22, 828. https://doi.org/10.3390/ijms22020828

AMA Style

Pagano L, Toto A, Malagrinò F, Visconti L, Jemth P, Gianni S. Double Mutant Cycles as a Tool to Address Folding, Binding, and Allostery. International Journal of Molecular Sciences. 2021; 22(2):828. https://doi.org/10.3390/ijms22020828

Chicago/Turabian Style

Pagano, Livia, Angelo Toto, Francesca Malagrinò, Lorenzo Visconti, Per Jemth, and Stefano Gianni. 2021. "Double Mutant Cycles as a Tool to Address Folding, Binding, and Allostery" International Journal of Molecular Sciences 22, no. 2: 828. https://doi.org/10.3390/ijms22020828

APA Style

Pagano, L., Toto, A., Malagrinò, F., Visconti, L., Jemth, P., & Gianni, S. (2021). Double Mutant Cycles as a Tool to Address Folding, Binding, and Allostery. International Journal of Molecular Sciences, 22(2), 828. https://doi.org/10.3390/ijms22020828

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop