Serological Number for Characterization of Circulating Antibodies
Abstract
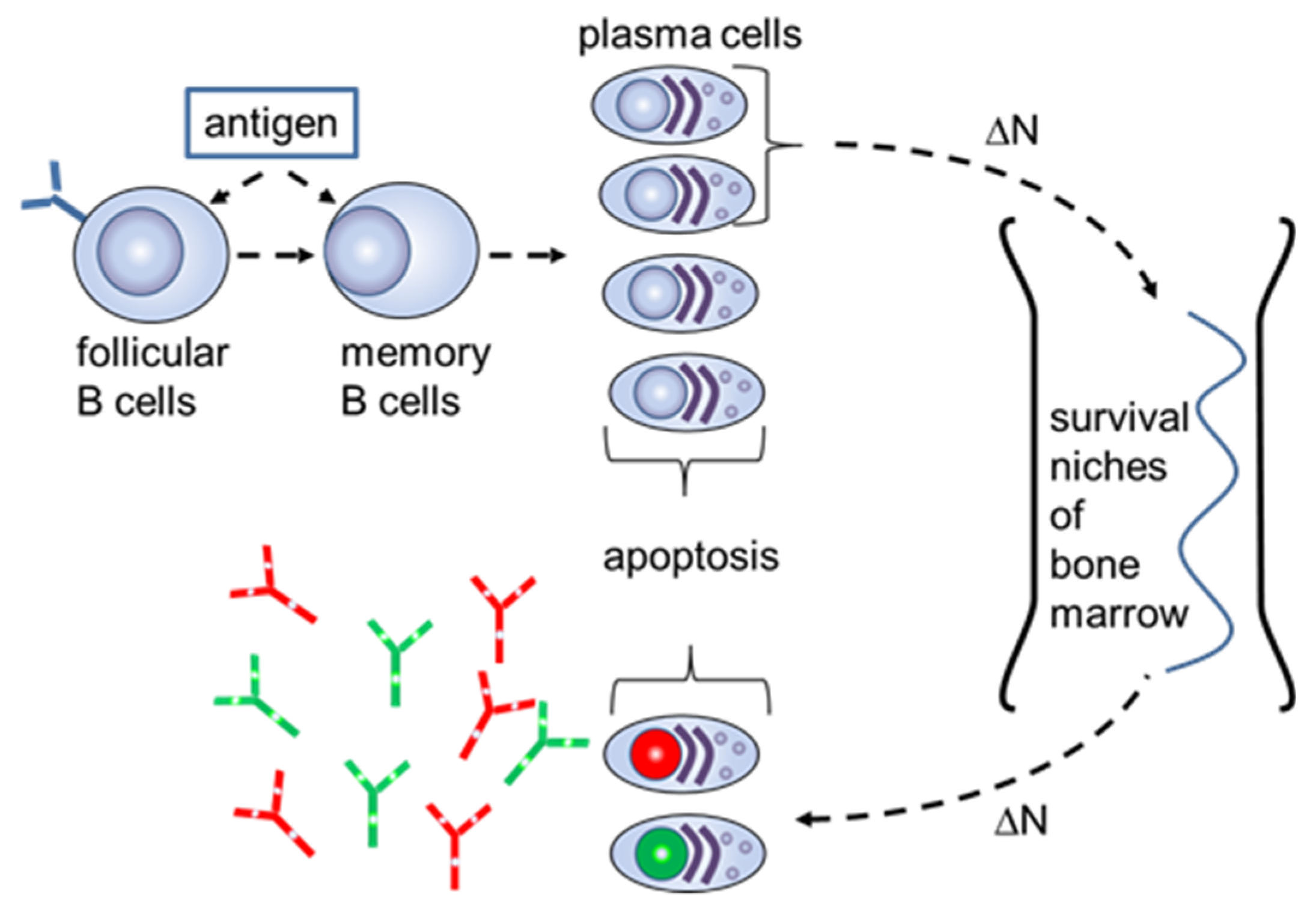
1. Introduction
2. Results
2.1. Analytical Estimation of the S-Number of Different Binding Motifs
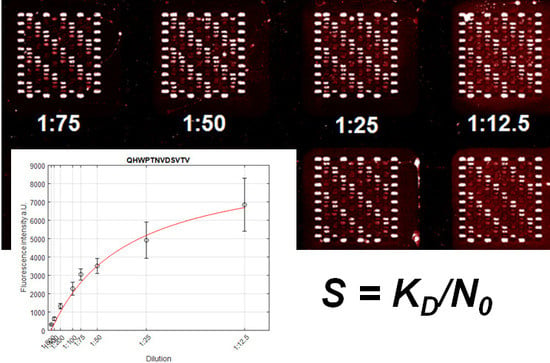
2.2. Dilution Measurements of the S-Number
3. Discussion
4. Materials and Methods
4.1. Equation for Measurement of the S-Number in Array Format
4.2. Methods to Measure the S-number
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Andrews, S.F.; Kaur, K.; Pauli, N.T.; Huang, M.; Huang, Y.P.; Wilson, P.C. High Preexisting Serological Antibody Levels Correlate with Diversification of the Influenza Vaccine Response. J. Virol. 2015, 89, 3308–3317. [Google Scholar] [CrossRef] [PubMed]
- Weiser, A.A.; Or-Guil, M.; Tapia, V.; Leichsenring, A.; Schuchhardt, J.; Frommel, C.; Volkmer-Engert, R. SPOT synthesis: Reliability of array-based measurement of peptide binding affinity. Anal. Biochem. 2005, 342, 300–311. [Google Scholar] [CrossRef] [PubMed]
- Greiff, V.; Redestig, H.; Luck, J.; Bruni, N.; Valai, A.; Hartmann, S.; Rausch, S.; Schuchhardt, J.; Or-Guil, M. A minimal model of peptide binding predicts ensemble properties of serum antibodies. BMC Genom. 2012, 13, 79. [Google Scholar] [CrossRef] [PubMed]
- Legutki, J.B.; Zhao, Z.G.; Greving, M.; Woodbury, N.; Johnston, S.A.; Stafford, P. Scalable high-density peptide arrays for comprehensive health monitoring. Nat. Commun. 2014, 5, 4785. [Google Scholar] [CrossRef] [PubMed]
- Ryvkin, A.; Ashkenazy, H.; Smelyanski, L.; Kaplan, G.; Penn, O.; Weiss-Ottolenghi, Y.; Privman, E.; Ngam, P.B.; Woodward, J.E.; May, G.D.; et al. Deep Panning: Steps towards probing the IgOme. PLoS ONE 2012, 7, e41469. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.Y.; Hu, Q.; Liu, S.; Tallo, L.J.; Sadzewicz, L.; Schettine, C.A.; Nikiforov, M.; Klyushnenkova, E.N.; Ionov, Y. Serum Antibody Repertoire Profiling Using in Silico Antigen Screen. PLoS ONE 2013, 8, e67181. [Google Scholar] [CrossRef] [PubMed]
- Christiansen, A.; Kringelum, J.V.; Hansen, C.S.; Bogh, K.L.; Sullivan, E.; Patel, J.; Rigby, N.M.; Eiwegger, T.; Szepfalusi, Z.; de Masi, F.; et al. High-throughput sequencing enhanced phage display enables the identification of patient-specific epitope motifs in serum. Sci. Rep. 2015, 5, 12913. [Google Scholar] [CrossRef] [PubMed]
- Weber, L.K.; Palermo, A.; Kugler, J.; Armant, O.; Isse, A.; Rentschler, S.; Jaenisch, T.; Hubbuch, J.; Dubel, S.; Nesterov-Mueller, A.; et al. Single amino acid fingerprinting of the human antibody repertoire with high density peptide arrays. J. Immunol. Methods 2017, 443, 45–54. [Google Scholar] [CrossRef] [PubMed]
- OShannessy, D.J.; Winzor, D.J. Interpretation of deviations from pseudo-first-order kinetic behavior in the characterization of ligand binding by biosensor technology. Anal. Biochem. 1996, 236, 275–283. [Google Scholar] [CrossRef] [PubMed]
- Radbruch, A.; Muehlinghaus, G.; Luger, E.O.; Inamine, A.; Smith, K.G.C.; Dorner, T.; Hiepe, F. Competence and competition: The challenge of becoming a long-lived plasma cell. Nat. Rev. Immunol. 2006, 6, 741–750. [Google Scholar] [CrossRef] [PubMed]
- Hofer, T.; Muehlinghaus, G.; Moser, K.; Yoshida, T.; Mei, H.E.; Hebel, K.; Hauser, A.; Hoyer, B.; Luger, E.O.; Dorner, T.; et al. Adaptation of humoral memory. Immunol. Rev. 2006, 211, 295–302. [Google Scholar] [CrossRef] [PubMed]
- Brodin, P.; Jojic, V.; Gao, T.X.; Bhattacharya, S.; Angel, C.J.L.; Furman, D.; Shen-Orr, S.; Dekker, C.L.; Swan, G.E.; Butte, A.J.; et al. Variation in the Human Immune System Is Largely Driven by Non-Heritable Influences. Cell 2015, 160, 37–47. [Google Scholar] [CrossRef] [PubMed]
- Sasaki, S.; He, X.S.; Holmes, T.H.; Dekker, C.L.; Kemble, G.W.; Arvin, A.M.; Greenberg, H.B. Influence of Prior Influenza Vaccination on Antibody and B-Cell Responses. PLoS ONE 2008, 3, e2975. [Google Scholar] [CrossRef] [PubMed]
- Monto, A.S.; Malosh, R.E.; Petrie, J.G.; Martin, E.T. The Doctrine of Original Antigenic Sin: Separating Good from Evil. J. Infect. Dis. 2017, 215, 1782–1788. [Google Scholar] [CrossRef] [PubMed]
- Cobey, S.; Hensley, S.E. Immune history and influenza virus susceptibility. Curr. Opin. Virol. 2017, 22, 105–111. [Google Scholar] [CrossRef]
- Lee, J.; Boutz, D.R.; Chromikova, V.; Joyce, M.G.; Vollmers, C.; Leung, K.; Horton, A.P.; DeKosky, B.J.; Lee, C.H.; Lavinder, J.J.; et al. Molecular-level analysis of the serum antibody repertoire in young adults before and after seasonal influenza vaccination. Nat. Med. 2016, 22, 1456–1464. [Google Scholar] [CrossRef]
- Jones, R.B.; Gordus, A.; Krall, J.A.; MacBeath, G. A quantitative protein interaction network for the ErbB receptors using protein microarrays. Nature 2006, 439, 168–174. [Google Scholar] [CrossRef] [PubMed]
- Wegner, G.J.; Lee, H.J.; Corn, R.M. Characterization and optimization of peptide arrays for the study of epitope-antibody interactions using surface plasmon resonance imaging. Anal. Chem. 2002, 74, 5161–5168. [Google Scholar] [CrossRef]
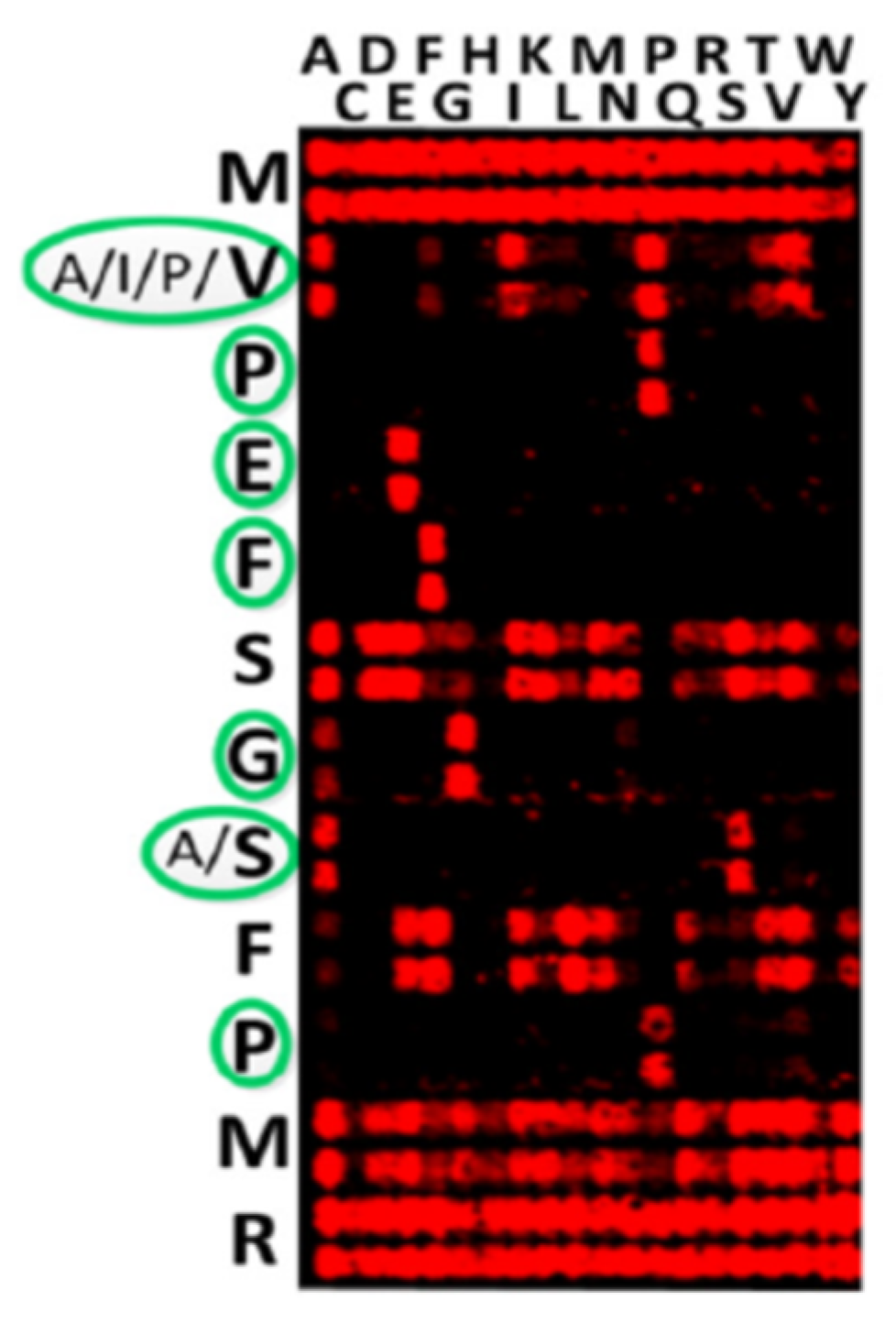
| N | Amino Acid Sequence | Characteristics of the Motif | S-number | Standard Deviation in % |
|---|---|---|---|---|
| 1 | GGQVRSIHSGPT | heterogeneous motif | 0.00267 | 37 |
| 2 | KEVPALTAVETGAT | LXAXETX motif group, poliovirus motif | 0.00386 | 31 |
| 3 | MVPEFSGSFPMR | Staphylococcus aureus motif | 0.00620 | 56 |
| 4 | LIADLNAESTSR | heterogeneous motif | 0.00775 | 22 |
| 5 | VLSSTAIKVDSV | heterogeneous motif | 0.00865 | 49 |
| 6 | VMSVNASTTAAN | heterogeneous motif | 0.01183 | 41 |
| 7 | QMKAWFPQTTYD | KXXFPQXT motif | 0.01219 | 48 |
| 8 | LRPNAVQTDTLA | heterogeneous motif | 0.01302 | 39 |
| 9 | SWVLTATETGSS | LXAXETX motif group, poliovirus motif | 0.01427 | 34 |
| 10 | NPVEDYLDYSVI | NPVEXXX motif | 0.01490 | 60 |
| 11 | ETKSDDMLLSNV | heterogeneous motif | 0.01537 | 31 |
| 12 | AKIRMFLDTDYK | heterogeneous motif | 0.01841 | 58 |
| 13 | VDTINLPQNTIQ | heterogeneous motif | 0.02059 | 49 |
| 14 | TALDAVSTGFSW | heterogeneous motif | 0.02597 | 42 |
| 15 | QHWPTNVDSVTV | heterogeneous motif | 0.04362 | 24 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palermo, A.; Nesterov-Mueller, A. Serological Number for Characterization of Circulating Antibodies. Int. J. Mol. Sci. 2019, 20, 604. https://doi.org/10.3390/ijms20030604
Palermo A, Nesterov-Mueller A. Serological Number for Characterization of Circulating Antibodies. International Journal of Molecular Sciences. 2019; 20(3):604. https://doi.org/10.3390/ijms20030604
Chicago/Turabian StylePalermo, Andrea, and Alexander Nesterov-Mueller. 2019. "Serological Number for Characterization of Circulating Antibodies" International Journal of Molecular Sciences 20, no. 3: 604. https://doi.org/10.3390/ijms20030604
APA StylePalermo, A., & Nesterov-Mueller, A. (2019). Serological Number for Characterization of Circulating Antibodies. International Journal of Molecular Sciences, 20(3), 604. https://doi.org/10.3390/ijms20030604