Accuracy and Precision of the Receptorial Responsiveness Method (RRM) in the Quantification of A1 Adenosine Receptor Agonists
Abstract
1. Introduction
2. Results
2.1. Response to Adenosine
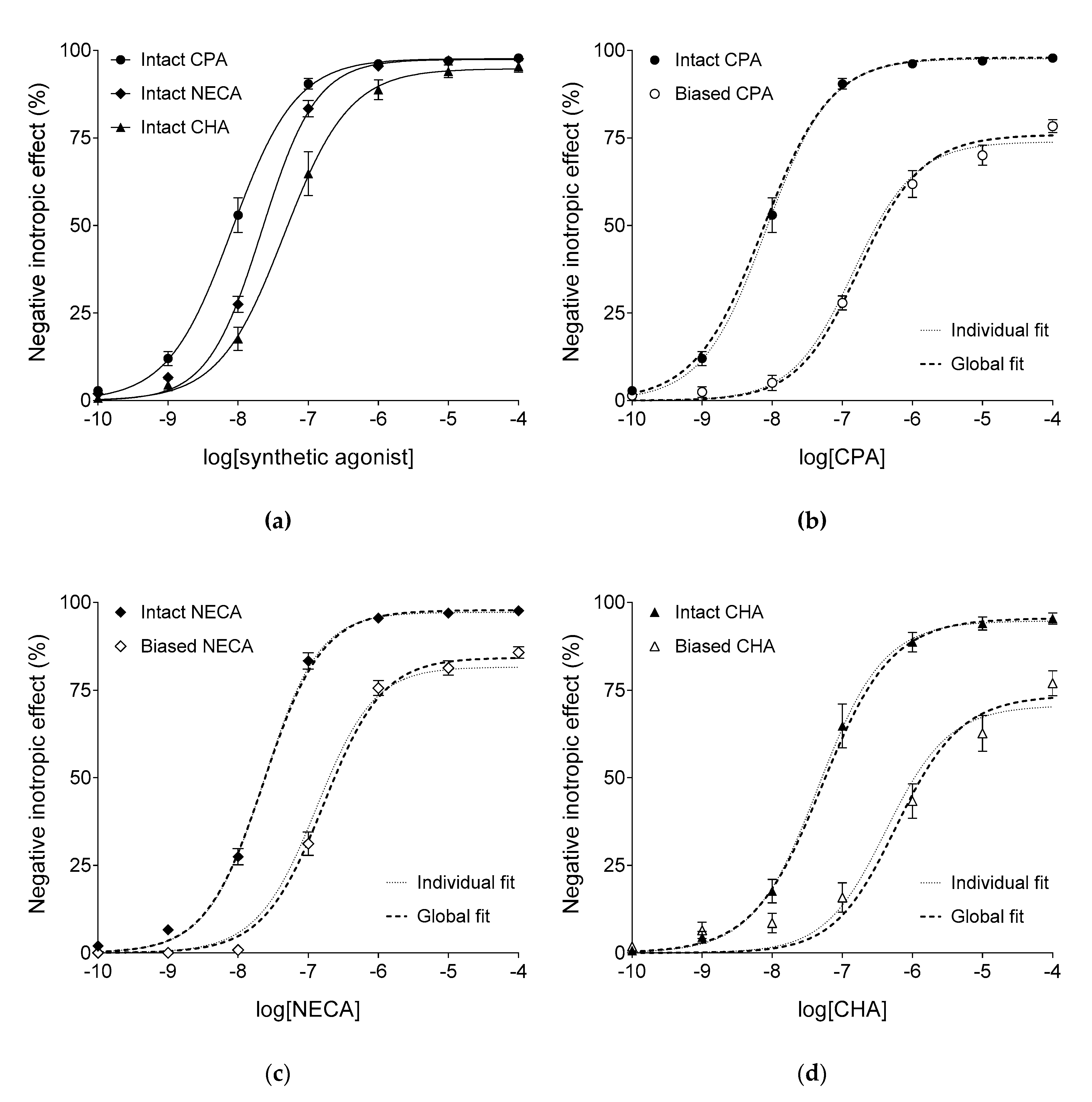
2.2. Responses to Synthetic A1 Adenosine Receptor Agonists
2.3. Curve Fitting to the Biased Responses Given to Synthetic A1 Adenosine Receptor Agonists
2.4. Curve Fitting to the Intact Responses Given to Synthetic A1 Adenosine Receptor Agonists
3. Discussion
4. Materials and Methods
4.1. Materials
4.2. Animals, Preparations and Protocols
4.3. Empirical Characterization of the E/c Curves
4.4. Assessment of the “Biasing” Concentration
4.5. Fitting Settings
4.6. Data Analysis
Author Contributions
Funding
Conflicts of Interest
References
- Rang, H.P. The receptor concept: pharmacology’s big idea. Br. J. Pharmacol. 2006, 147, S9–S16. [Google Scholar] [CrossRef] [PubMed]
- Colquhoun, D. The quantitative analysis of drug-receptor interactions: A short history. Trends Pharmacol. Sci. 2006, 27, 149–157. [Google Scholar] [CrossRef] [PubMed]
- Giraldo, J.; Vivas, N.M.; Vila, E.; Badia, A. Assessing the (a)symmetry of concentration-effect curves: Empirical versus mechanistic models. Pharmacol. Ther. 2002, 95, 21–45. [Google Scholar] [CrossRef]
- Gesztelyi, R.; Zsuga, J.; Kemeny-Beke, A.; Varga, B.; Juhasz, B.; Tosaki, A. The Hill equation and the origin of quantitative pharmacology. Arch. Hist. Exact Sci. 2012, 66, 427–438. [Google Scholar] [CrossRef]
- Roche, D.; Gil, D.; Giraldo, J. Mathematical modeling of G protein-coupled receptor function: What can we learn from empirical and mechanistic models? Adv. Exp. Med. Biol. 2014, 796, 159–181. [Google Scholar] [CrossRef]
- Kenakin, T.P. A Pharmacology Primer: Techniques for More Effective and Strategic Drug Discovery; Academic Press: London, UK, 2018. [Google Scholar]
- Hill, A.V. The possible effects of the aggregation of the molecules of haemoglobin on its dissociation curves. J. Physiol. (Lond.) 1910, 40, 4–7. [Google Scholar]
- Goutelle, S.; Maurin, M.; Rougier, F.; Barbaut, X.; Bourguignon, L.; Ducher, M.; Maire, P. The Hill equation: A review of its capabilities in pharmacological modelling. Fundam. Clin. Pharmacol. 2008, 22, 633–648. [Google Scholar] [CrossRef]
- Black, J.W.; Leff, P. Operational models of pharmacological agonism. Proc. R. Soc. Lond. B Biol. Sci. 1983, 220, 141–162. [Google Scholar] [CrossRef]
- Buchwald, P. A Receptor Model with Binding Affinity, Activation Efficacy, and Signal Amplification Parameters for Complex Fractional Response Versus Occupancy Data. Front. Pharmacol. 2019, 10, 605. [Google Scholar] [CrossRef]
- Gesztelyi, R.; Zsuga, J.; Juhász, B.; Dér, P.; Vecsernyés, M.; Szentmiklósi, A.J. Concentration estimation via curve fitting: Quantification of negative inotropic agents by using a simple mathematical method in guinea pig atria. Bull. Math. Biol. 2004, 66, 1439–1453. [Google Scholar] [CrossRef]
- Karsai, D.; Zsuga, J.; Juhász, B.; Dér, P.; Szentmiklósi, A.J.; Tósaki, A.; Gesztelyi, R. Effect of nucleoside transport blockade on the interstitial adenosine level characterized by a novel method in guinea pig atria. J. Cardiovasc. Pharmacol. 2006, 47, 103–109. [Google Scholar] [CrossRef] [PubMed]
- Karsai, D.; Gesztelyi, R.; Zsuga, J.; Jakab, A.; Szendrei, L.; Juhasz, B.; Bak, I.; Szabo, G.; Lekli, I.; Vecsernyes, M.; et al. Influence of hyperthyroidism on the effect of adenosine transport blockade assessed by a novel method in guinea pig atria. Cell Biochem. Biophys. 2007, 47, 45–52. [Google Scholar] [CrossRef]
- Grenczer, M.; Pinter, A.; Zsuga, J.; Kemeny-Beke, A.; Juhasz, B.; Szodoray, P.; Tosaki, A.; Gesztelyi, R. The influence of affinity, efficacy and slope factor on the estimates obtained by the receptorial responsiveness method (RRM): A computer simulation study. Can. J. Physiol. Pharmacol. 2010, 88, 1061–1073. [Google Scholar] [CrossRef] [PubMed]
- Grenczer, M.; Zsuga, J.; Majoros, L.; Pinter, A.; Kemeny-Beke, A.; Juhasz, B.; Tosaki, A.; Gesztelyi, R. Effect of asymmetry of concentration-response curves on the results obtained by the receptorial responsiveness method (RRM): An in silico study. Can. J. Physiol. Pharmacol. 2010, 88, 1074–1083. [Google Scholar] [CrossRef] [PubMed]
- Fredholm, B.B.; IJzerman, A.P.; Jacobson, K.A.; Klotz, K.N.; Linden, J. International Union of Pharmacology. XXV. Nomenclature and classification of adenosine receptors. Pharmacol. Rev. 2001, 53, 527–552. [Google Scholar] [PubMed]
- Mundell, S.; Kelly, E. Adenosine receptor desensitization and trafficking. Biochim. Biophys. Acta 2011, 1808, 1319–1328. [Google Scholar] [CrossRef]
- Motulsky, H.J.; Christopoulos, A. Fitting Models to Biological Data Using Linear and Nonlinear Regression. A Practical Guide to Curve Fitting; GraphPad Software Inc.: San Diego, CA, USA, 2004. [Google Scholar]
- Prism 8 Curve Fitting Guide; GraphPad Software Inc.: La Jolla, CA, USA; Available online: https://www.graphpad.com/guides/prism/8/curve-fitting/index.htm (accessed on 24 September 2019).
- McDonald, J.H. Handbook of Biological Statistics; Sparky House Publishing: Baltimore, MD, USA, 2009. [Google Scholar]
- Wilbur, S.L.; Marchlinski, F.E. Adenosine as an antiarrhythmic agent. Am. J. Cardiol. 1997, 79, 30–37. [Google Scholar] [CrossRef] [PubMed]
- Endoh, M. Dual inhibition of myocardial function through muscarinic and adenosine receptors in the mammalian heart. J. Appl. Cardiol. 1987, 2, 213–230. [Google Scholar]
- Kurachi, Y.; Nakajima, T.; Sugimoto, T. On the mechanism of activation of muscarinic K+ channels by adenosine in isolated atrial cells: Involvement of GTP-binding proteins. Pflugers Arch. 1986, 407, 264–274. [Google Scholar] [CrossRef] [PubMed]
- Endoh, M. Muscarinic regulation of Ca2+ signaling in mammalian atrial and ventricular myocardium. Eur. J. Pharmacol. 1999, 375, 177–196. [Google Scholar] [CrossRef] [PubMed]
- Belardinelli, L.; Shryock, J.C.; Song, Y.; Wang, D.; Srinivas, M. Ionic basis of the electrophysiological actions of adenosine on cardiomyocytes. FASEB J. 1995, 9, 359–365. [Google Scholar] [CrossRef] [PubMed]
- Pavan, B.; IJzerman, A.P. Processing of adenosine receptor agonists in rat and human whole blood. Biochem. Pharmacol. 1998, 56, 1625–1632. [Google Scholar] [CrossRef]
- Deb, P.K.; Deka, S.; Borah, P.; Abed, S.N.; Klotz, K.N. Medicinal Chemistry and Therapeutic Potential of Agonists, Antagonists and Allosteric Modulators of A1 Adenosine Receptor: Current Status and Perspectives. Curr. Pharm. Des. 2019, 25, 2697–2715. [Google Scholar] [CrossRef] [PubMed]
- Burnstock, G.; Fredholm, B.B.; North, R.A.; Verkhratsky, A. The birth and postnatal development of purinergic signalling. Acta Physiol. (Oxf) 2010, 199, 93–147. [Google Scholar] [CrossRef]
- Kiss, Z.; Pak, K.; Zsuga, J.; Juhasz, B.; Varga, B.; Szentmiklosi, A.J.; Haines, D.D.; Tosaki, A.; Gesztelyi, R. The guinea pig atrial A1 adenosine receptor reserve for the direct negative inotropic effect of adenosine. Gen. Physiol. Biophys. 2013, 32, 325–335. [Google Scholar] [CrossRef]
- Pak, K.; Papp, C.; Galajda, Z.; Szerafin, T.; Varga, B.; Juhasz, B.; Haines, D.; Szentmiklosi, A.J.; Tosaki, A.; Gesztelyi, R. Approximation of A1 adenosine receptor reserve appertaining to the direct negative inotropic effect of adenosine in hyperthyroid guinea pig left atria. Gen. Physiol. Biophys. 2014, 33, 177–188. [Google Scholar] [CrossRef]
- Erdei, T.; Szabo, A.M.; Lampe, N.; Szabo, K.; Kiss, R.; Zsuga, J.; Papp, C.; Pinter, A.; Szentmiklosi, A.J.; Szilvassy, Z.; et al. FSCPX, a Chemical Widely Used as an Irreversible A1 Adenosine Receptor Antagonist, Modifies the Effect of NBTI, a Nucleoside Transport Inhibitor, by Reducing the Interstitial Adenosine Level in the Guinea Pig Atrium. Molecules 2018, 23, 2186. [Google Scholar] [CrossRef]
- Kemeny-Beke, A.; Jakab, A.; Zsuga, J.; Vecsernyes, M.; Karsai, D.; Pasztor, F.; Grenczer, M.; Szentmiklosi, A.J.; Berta, A.; Gesztelyi, R. Adenosine deaminase inhibition enhances the inotropic response mediated by A1 adenosine receptor in hyperthyroid guinea pig atrium. Pharmacol. Res. 2007, 56, 124–131. [Google Scholar] [CrossRef]
- Gesztelyi, R.; Kiss, Z.; Wachal, Z.; Juhasz, B.; Bombicz, M.; Csepanyi, E.; Pak, K.; Zsuga, J.; Papp, C.; Galajda, Z.; et al. The surmountable effect of FSCPX, an irreversible A(1) adenosine receptor antagonist, on the negative inotropic action of A(1) adenosine receptor full agonists in isolated guinea pig left atria. Arch. Pharm. Res. 2013, 36, 293–305. [Google Scholar] [CrossRef]
- Fenouillet, E.; Mottola, G.; Kipson, N.; Paganelli, F.; Guieu, R.; Ruf, J. Adenosine Receptor Profiling Reveals an Association between the Presence of Spare Receptors and Cardiovascular Disorders. Int. J. Mol. Sci. 2019, 20, 5964. [Google Scholar] [CrossRef]
- Zsuga, J.; Erdei, T.; Szabó, K.; Lampe, N.; Papp, C.; Pinter, A.; Szentmiklosi, A.J.; Juhasz, B.; Szilvássy, Z.; Gesztelyi, R. Methodical Challenges and a Possible Resolution in the Assessment of Receptor Reserve for Adenosine, an Agonist with Short Half-Life. Molecules 2017, 22, 839. [Google Scholar] [CrossRef] [PubMed]
- Szabo, A.M.; Erdei, T.; Viczjan, G.; Kiss, R.; Zsuga, J.; Papp, C.; Pinter, A.; Juhasz, B.; Szilvassy, Z.; Gesztelyi, R. An Advanced in Silico Modelling of the Interaction between FSCPX, an Irreversible A1 Adenosine Receptor Antagonist, and NBTI, a Nucleoside Transport Inhibitor, in the Guinea Pig Atrium. Molecules 2019, 24, 2207. [Google Scholar] [CrossRef] [PubMed]

| Ordinary Fit | Robust Fit | |
|---|---|---|
| No weighting | Individual fit | Individual fit |
| Global fit | Global fit | |
| Weighting by 1/Y2 | Individual fit | |
| Global fit | ||
| Weighting by 1/SD2 | Individual fit | |
| Global fit |
| Intact CPA (n = 6) | Intact NECA (n = 7) | Intact CHA (n = 6) | |
|---|---|---|---|
| Emax (%) | 97.4 ± 0.8 | 97.5 ± 0.5 ns | 94.8 ± 1.7 ns, ns |
| logEC50 | −8.1 ± 0.09 | −7.66 ± 0.05 ** | −7.33 ± 0.11 ###, + |
| n | 1.02 ± 0.05 | 1.14 ± 0.07 ns | 0.98 ± 0.03 ns, ns |
| Individual, Ordinary, ø | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.88 | −19,107 | −6.87 | −8.92 | −6.45 | −52,574 |
| 95% CI | −6.93 to −6.83 | very wide | −6.92 to −6.83 | ? to −8.32 | −6.55 to −6.36 | very wide |
| cx (nM) | 131.4 | 0 | 133.6 | 1.2 | 352.7 | 0 |
| Individual, Robust, ø | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.9 | −19,107 | −6.88 | −8.64 | −6.47 | −5257 |
| cx (nM) | 125.9 | 0 | 131.4 | 2.3 | 335.8 | 0 |
| Global, Ordinary, ø | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.84 | −7201 | −6.77 | −35,907 | −6.39 | −9,892,707,770 |
| 95% CI | very wide | very wide | very wide | very wide | very wide | very wide |
| cx (nM) | 145.9 | 0 | 170 | 0 | 403.7 | 0 |
| Global, Robust, ø | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.85 | −7201 | −6.82 | −35,907 | −6.36 | −9,892,707,770 |
| cx (nM) | 142.6 | 0 | 153.3 | 0 | 439 | 0 |
| Individual, Ordinary, 1/Y2 | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −8.21 | −19107 | −6.36 | −8.51 | −317,820,174,071 | −52,574 |
| 95% CI | ? to −8.163 | very wide | ? to −6.34 | −8.89 to −8.2 | very wide | very wide |
| cx (nM) | 6.2 | 0 | 438.5 | 3.1 | 0 | 0 |
| Global, Ordinary, 1/Y2 | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −366.5 | −7201 | −6.146 | −35,907 | - | - |
| 95% CI | very wide | very wide | very wide | very wide | ||
| cx (nM) | 0 | 0 | 714.8 | 0 | ||
| Individual, Ordinary, 1/SD2 | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.9 | −42,781 | - | −8.66 | −6.5 | −56,039 |
| 95% CI | −6.99 to −6.82 | very wide | ? to −7.96 | −6.77 to −6.26 | very wide | |
| cx (nM) | 125.7 | 0 | 2.2 | 318.6 | 0 | |
| Global, Ordinary, 1/SD2 | Biased CPA | Intact CPA | Biased NECA | Intact NECA | Biased CHA | Intact CHA |
| logcx | −6.87 | −1353 | - | - | −8.26 | −22.51 |
| 95% CI | very wide | very wide | very wide | very wide | ||
| cx (nM) | 136.5 | 0 | 554.1 | 3.11 × 10−14 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szabo, A.M.; Viczjan, G.; Erdei, T.; Simon, I.; Kiss, R.; Szentmiklosi, A.J.; Juhasz, B.; Papp, C.; Zsuga, J.; Pinter, A.; et al. Accuracy and Precision of the Receptorial Responsiveness Method (RRM) in the Quantification of A1 Adenosine Receptor Agonists. Int. J. Mol. Sci. 2019, 20, 6264. https://doi.org/10.3390/ijms20246264
Szabo AM, Viczjan G, Erdei T, Simon I, Kiss R, Szentmiklosi AJ, Juhasz B, Papp C, Zsuga J, Pinter A, et al. Accuracy and Precision of the Receptorial Responsiveness Method (RRM) in the Quantification of A1 Adenosine Receptor Agonists. International Journal of Molecular Sciences. 2019; 20(24):6264. https://doi.org/10.3390/ijms20246264
Chicago/Turabian StyleSzabo, Adrienn Monika, Gabor Viczjan, Tamas Erdei, Ildiko Simon, Rita Kiss, Andras Jozsef Szentmiklosi, Bela Juhasz, Csaba Papp, Judit Zsuga, Akos Pinter, and et al. 2019. "Accuracy and Precision of the Receptorial Responsiveness Method (RRM) in the Quantification of A1 Adenosine Receptor Agonists" International Journal of Molecular Sciences 20, no. 24: 6264. https://doi.org/10.3390/ijms20246264
APA StyleSzabo, A. M., Viczjan, G., Erdei, T., Simon, I., Kiss, R., Szentmiklosi, A. J., Juhasz, B., Papp, C., Zsuga, J., Pinter, A., Szilvassy, Z., & Gesztelyi, R. (2019). Accuracy and Precision of the Receptorial Responsiveness Method (RRM) in the Quantification of A1 Adenosine Receptor Agonists. International Journal of Molecular Sciences, 20(24), 6264. https://doi.org/10.3390/ijms20246264







