Study on the Mechanism of Lipid Peroxidation Induced by Carbonate Radicals
Abstract
1. Introduction
2. Results
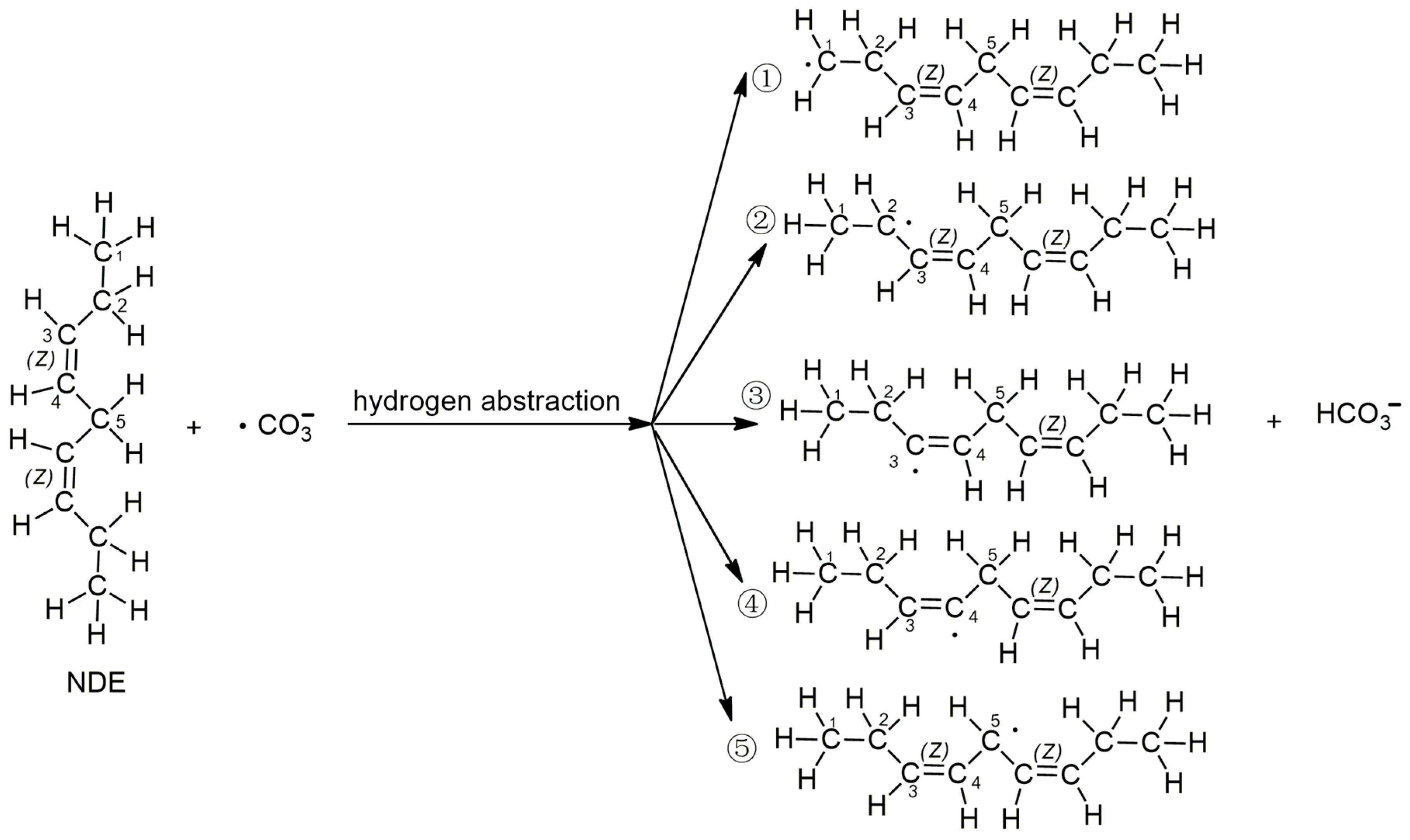
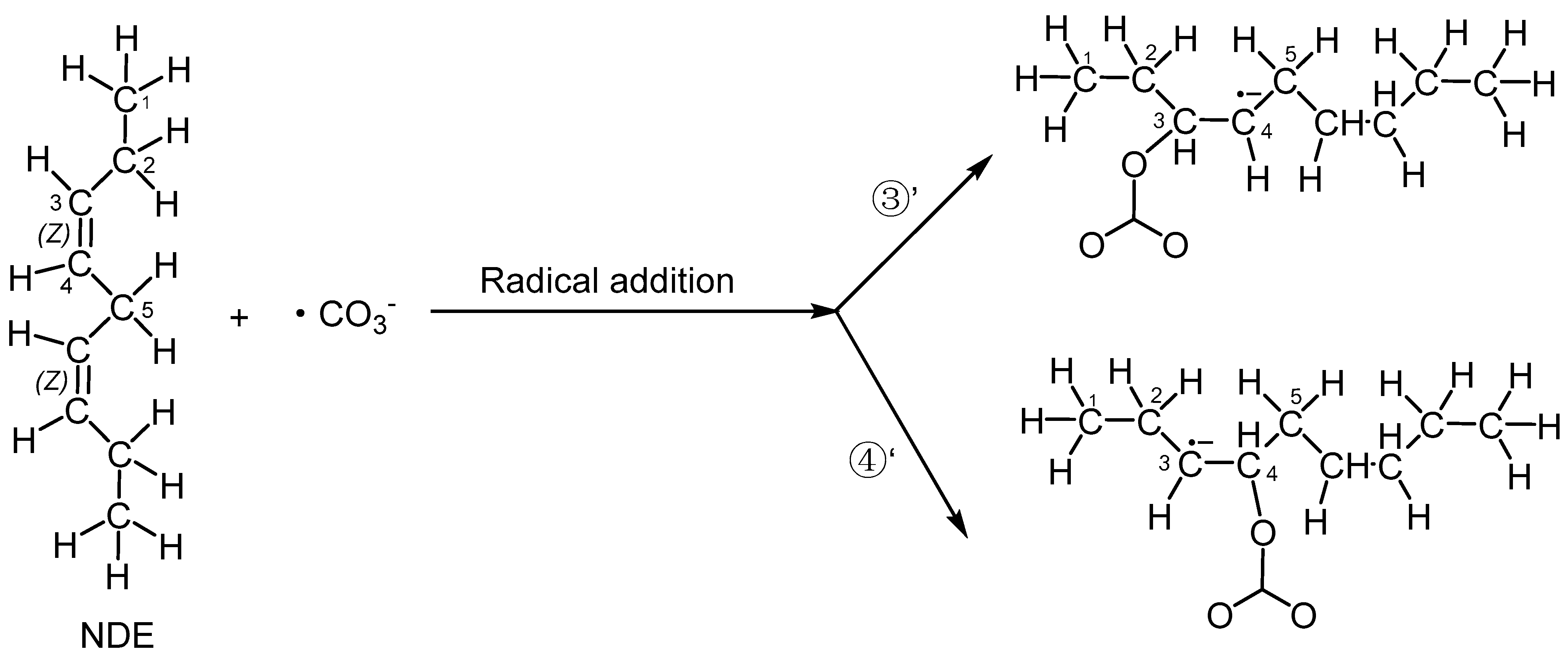
2.1. Reaction of Carbonate Free Radical with Polyunsaturated Fatty Acids
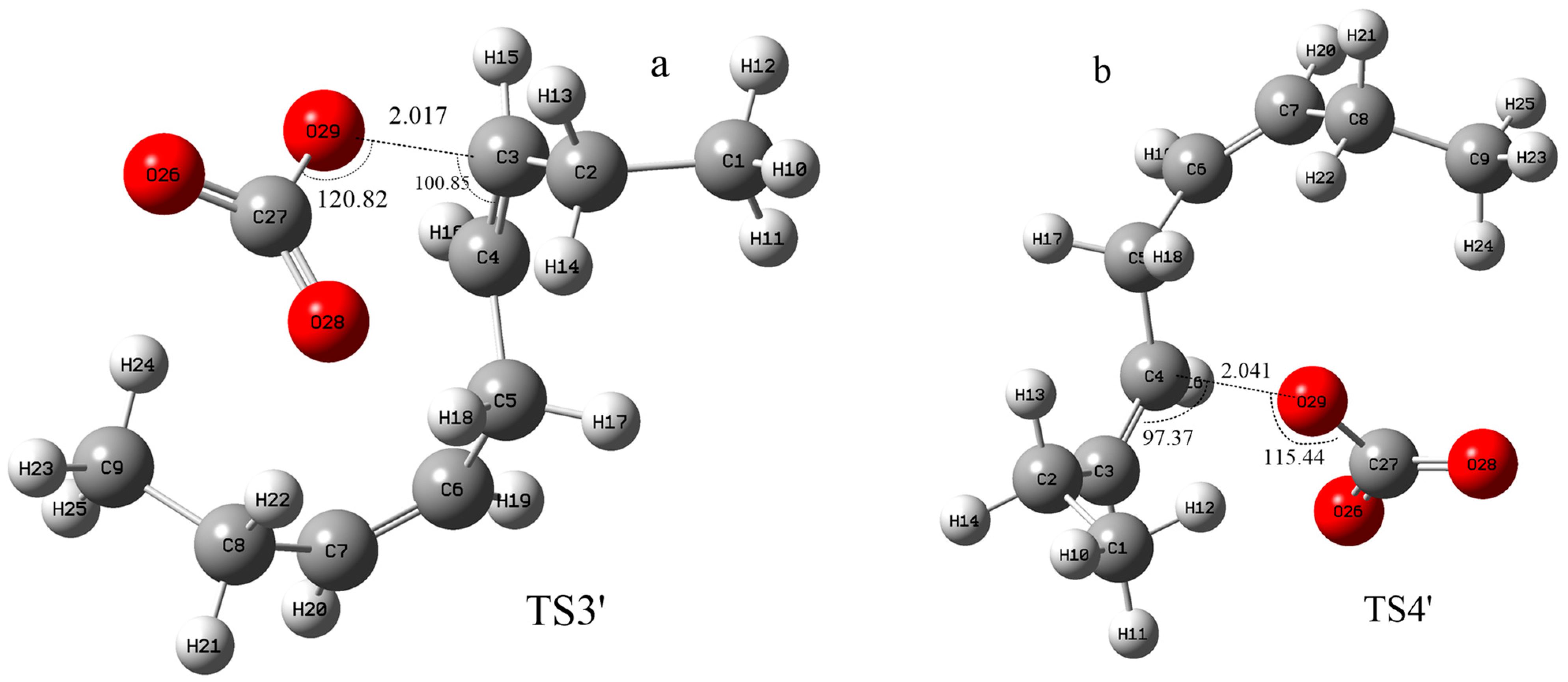
2.2. The Potential Energy Surfaces of the Reactions between Carbonate Radicals and Different Positions in NDE
2.3. Electron Spin Density Analysis
2.4. The Reaction Rate Constant
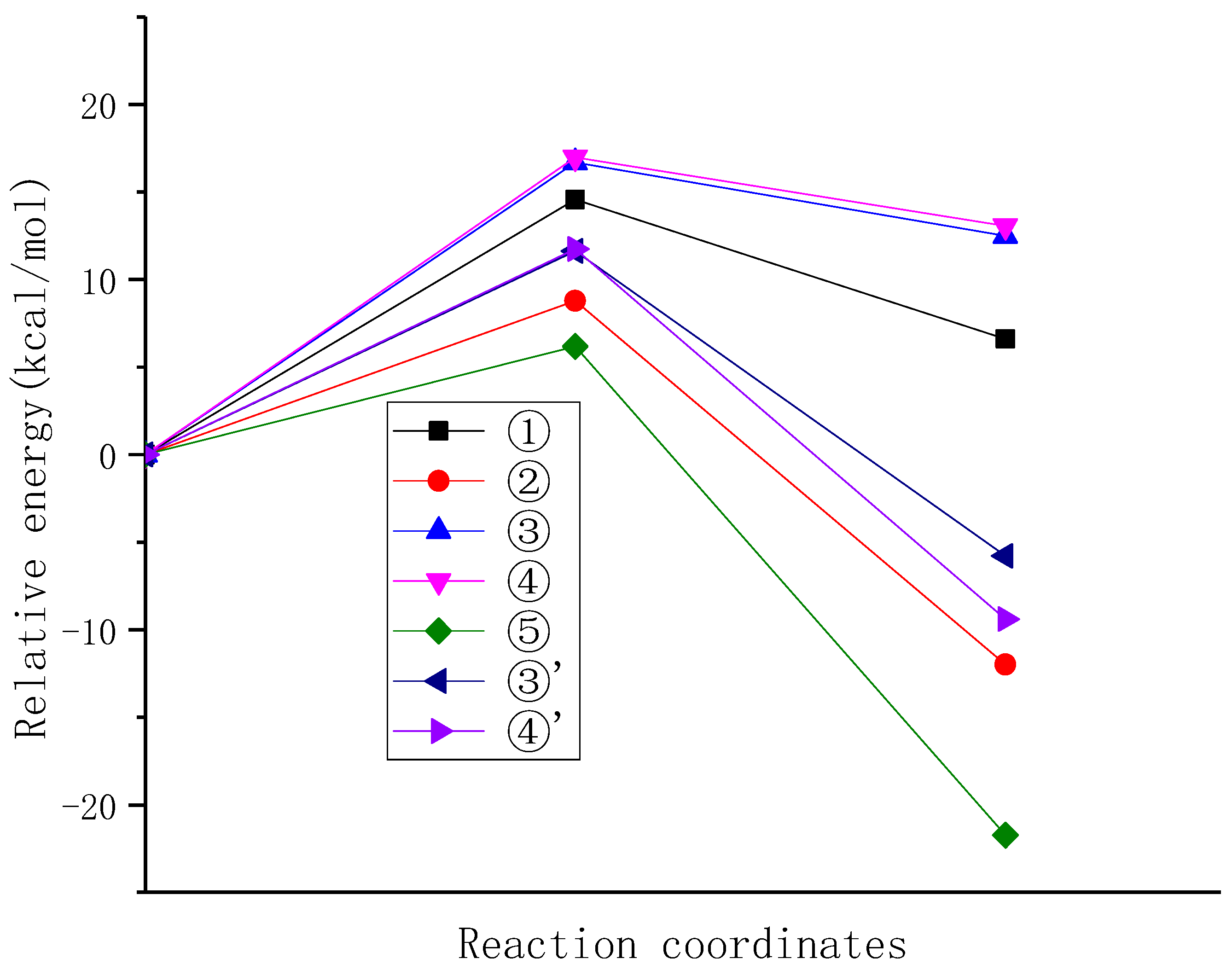
2.5. The Energy Change in the Reactions
3. Discussion
4. Computational Methods
4.1. Quantum Chemistry Computation
4.2. Rate Constant Computation
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Juan, C.A.; Pérez de la Lastra, J.M.; Plou, F.J.; Pérez-Lebeña, E. The Chemistry of Reactive Oxygen Species (ROS) Revisited: Outlining Their Role in Biological Macromolecules (DNA, Lipids and Proteins) and Induced Pathologies. Int. J. Mol. Sci. 2021, 22, 4642. [Google Scholar] [CrossRef]
- Sharma, P.; Jha, A.B.; Dubey, R.S.; Pessarakli, M. Reactive Oxygen Species, Oxidative Damage, and Antioxidative Defense Mechanism in Plants under Stressful Conditions. J. Bot. 2012, 2012, 217037. [Google Scholar] [CrossRef]
- Pizzino, G.; Irrera, N.; Cucinotta, M.; Pallio, G.; Mannino, F.; Arcoraci, V.; Squadrito, F.; Altavilla, D.; Bitto, A. Oxidative Stress: Harms and Benefits for Human Health. Oxidative Med. Cell. Longev. 2017, 2017, 8416763. [Google Scholar] [CrossRef]
- Dubois-Deruy, E.; Peugnet, V.; Turkieh, A.; Pinet, F. Oxidative Stress in Cardiovascular Diseases. Antioxidants 2020, 9, 864. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Guo, C.; Kong, J. Oxidative stress in neurodegenerative diseases. Neural Regen. Res. 2012, 7, 376–385. [Google Scholar] [CrossRef]
- Anik, M.I.; Mahmud, N.; Masud, A.A.; Khan, M.I.; Islam, M.N.; Uddin, S.; Hossain, M.K. Role of Reactive Oxygen Species in Aging and Age-Related Diseases: A Review. ACS Appl. Bio Mater. 2022, 5, 4028–4054. [Google Scholar] [CrossRef]
- Auten, R.L.; Davis, J.M. Oxygen Toxicity and Reactive Oxygen Species: The Devil Is in the Details. Pediatr. Res. 2009, 66, 121–127. [Google Scholar] [CrossRef] [PubMed]
- Gaschler, M.M.; Stockwell, B.R. Lipid peroxidation in cell death. Biochem. Biophys. Res. Commun. 2017, 482, 419–425. [Google Scholar] [CrossRef] [PubMed]
- Yaman, S.O.; Ayhanci, A. Lipid Peroxidation; IntechOpen: London, UK, 2021; pp. 1–11. [Google Scholar]
- Collin, F. Chemical Basis of Reactive Oxygen Species Reactivity and Involvement in Neurodegenerative Diseases. Int. J. Mol. Sci. 2019, 20, 2407. [Google Scholar] [CrossRef]
- Halliwell, B. Reactive oxygen species in living systems: Source, biochemistry, and role in human disease. Am. J. Med. 1991, 91, S14–S22. [Google Scholar] [CrossRef]
- Sharifi-Rad, M.; Anil Kumar, N.V.; Zucca, P.; Varoni, E.M.; Dini, L.; Panzarini, E.; Rajkovic, J.; Tsouh Fokou, P.V.; Azzini, E.; Peluso, I.; et al. Lifestyle, oxidative stress, and antioxidants: Back and forth in the pathophysiology of chronic diseases. Front. Physiol. 2020, 11, 694. [Google Scholar] [CrossRef]
- Lobo, V.; Patil, A.; Phatak, A.; Chandra, N. Free radicals, antioxidants and functional foods: Impact on human health. Pharmacogn. Rev. 2010, 4, 118. [Google Scholar] [CrossRef]
- Halliwell, B.; Adhikary, A.; Dingfelder, M.; Dizdaroglu, M. Hydroxyl radical is a significant player in oxidative DNA damage in vivo. Chem. Soc. Rev. 2021, 50, 8355–8360. [Google Scholar] [CrossRef] [PubMed]
- Galano, A.; Tan, D.X.; Reiter, R.J. Melatonin as a natural ally against oxidative stress: A physicochemical examination. J. Pineal Res. 2011, 51, 1–16. [Google Scholar] [CrossRef]
- Rice-Evans, C.A.; Burdon, R.H. Normal biochemical processes and pathological states. Free. Radic. Damage Its Control 1994, 28, 131. [Google Scholar]
- Illés, E.; Mizrahi, A.; Marks, V.; Meyerstein, D. Carbonate-radical-anions, and not hydroxyl radicals, are the products of the Fenton reaction in neutral solutions containing bicarbonate. Free Radic. Biol. Med. 2019, 131, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Lan, X.; Dai, Y.; Jing, W.; Meng, X.; Liu, F.; Wang, S.; He, A.; Li, N. DFT investigation on the carbonate radical formation in the system containing carbon dioxide and hydroxyl free radical. J. Mol. Graph. Model. 2022, 114, 108182. [Google Scholar] [CrossRef] [PubMed]
- Patra, S.G.; Mizrahi, A.; Meyerstein, D. The Role of Carbonate in Catalytic Oxidations. Acc. Chem. Res. 2020, 53, 2189–2200. [Google Scholar] [CrossRef]
- Rayaroth, M.P.; Boczkaj, G.; Aubry, O.; Aravind, U.K.; Aravindakumar, C.T. Advanced Oxidation Processes for Degradation of Water Pollutants—Ambivalent Impact of Carbonate Species: A Review. Water 2023, 15, 1615. [Google Scholar] [CrossRef]
- Meli, R.; Nauser, T.; Latal, P.; Koppenol, W.H. Reaction of peroxynitrite with carbon dioxide: Intermediates and determination of the yield of CO3•− and NO2•. J. Biol. Inorg. Chem. JBIC A Publ. Soc. Biol. Inorg. Chem. 2002, 7, 31–36. [Google Scholar] [CrossRef]
- Medinas, D.B.; Cerchiaro, G.; Trindade, D.F.; Augusto, O. The carbonate radical and related oxidants derived from bicarbonate buffer. IUBMB Life 2007, 59, 255–262. [Google Scholar] [CrossRef]
- Hardeland, R. The Underrated Carbonate Radical (CO3•−)—Detoxification and Reduced Formation by Melatonin. Biomed. J. Sci. Tech. Res. 2017, 1, 634–637. [Google Scholar] [CrossRef]
- Lee, Y.A.; Yun, B.H.; Kim, S.K.; Margolin, Y.; Dedon, P.C.; Geacintov, N.E.; Shafirovich, V. Mechanisms of oxidation of guanine in DNA by carbonate radical anion, a decomposition product of nitrosoperoxycarbonate. Chem. Eur. J. 2007, 13, 4571–4581. [Google Scholar] [CrossRef]
- Joffe, A.; Geacintov, N.E.; Shafirovich, V. DNA lesions derived from the site selective oxidation of guanine by carbonate radical anions. Chem. Res. Toxicol. 2003, 16, 1528–1538. [Google Scholar] [CrossRef]
- Augusto, O.; Bonini, M.G.; Amanso, A.M.; Linares, E.; Santos, C.C.; De Menezes, S.L. Nitrogen dioxide and carbonate radical anion: Two emerging radicals in biology. Free. Radic. Biol. Med. 2002, 32, 841–859. [Google Scholar] [CrossRef] [PubMed]
- Ramirez, D.C.; Gomez-Mejiba, S.E.; Corbett, J.T.; Deterding, L.J.; Tomer, K.B.; Mason, R.P. Cu, Zn-superoxide dismutase-driven free radical modifications: Copper-and carbonate radical anion-initiated protein radical chemistry. Biochem. J. 2009, 417, 341–353. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Gebicka, L.; Didik, J.; Gebicki, J.L. Reactions of heme proteins with carbonate radical anion. Res. Chem. Intermed. 2009, 35, 401–409. [Google Scholar] [CrossRef]
- Bühl, M.; DaBell, P.; Manley, D.W.; McCaughan, R.P.; Walton, J.C. Bicarbonate and alkyl carbonate radicals: Structural integrity and reactions with lipid components. J. Am. Chem. Soc. 2015, 137, 16153–16162. [Google Scholar] [CrossRef] [PubMed]
- Cadet, J.; Davies, K.J. Oxidative DNA damage & repair: An introduction. Free. Radic. Biol. Med. 2017, 107, 2–12. [Google Scholar] [PubMed]
- Martemucci, G.; Costagliola, C.; Mariano, M.; D’andrea, L.; Napolitano, P.; D’Alessandro, A.G. Free radical properties, source and targets, antioxidant consumption and health. Oxygen 2022, 2, 48–78. [Google Scholar] [CrossRef]
- Andreu, I.; Neshchadin, D.; Rico, E.; Griesser, M.; Samadi, A.; Morera, I.M.; Gescheidt, G.; Miranda, M.A. Probing lipid peroxidation by using linoleic acid and benzophenone. Chem. Eur. J. 2011, 17, 10089–10096. [Google Scholar] [CrossRef]
- Guo, J.-J.; Hsieh, H.-Y.; Hu, C.-H. Chain-breaking activity of carotenes in lipid peroxidation: A theoretical study. J. Phys. Chem. B 2009, 113, 15699–15708. [Google Scholar] [CrossRef]
- Tejero, I.; González-Lafont, À.; Lluch, J.M.; Eriksson, L.A. Theoretical modeling of hydroxyl-radical-induced lipid peroxidation reactions. J. Phys. Chem. B 2007, 111, 5684–5693. [Google Scholar] [CrossRef]
- Chatgilialoglu, C.; Ferreri, C.; Guerra, M.; Samadi, A.; Bowry, V.W. The Reaction of Thiyl Radical with Methyl Linoleate: Completing the Picture. J. Am. Chem. Soc. 2017, 139, 4704–4714. [Google Scholar] [CrossRef]
- Nah, T.; Kessler, S.H.; Daumit, K.E.; Kroll, J.H.; Leone, S.R.; Wilson, K.R. OH-initiated oxidation of sub-micron unsaturated fatty acid particles. Phys. Chem. Chem. Phys. 2013, 15, 18649–18663. [Google Scholar] [CrossRef] [PubMed]
- Szori, M.; Abou-Abdo, T.; Fittschen, C.; Csizmadia, I.G.; Viskolcz, B. Allylic hydrogen abstraction II. H-abstraction from 1, 4 type polyalkenes as a model for free radical trapping by polyunsaturated fatty acids (PUFAs). Phys. Chem. Chem. Phys. 2007, 9, 1931–1940. [Google Scholar] [CrossRef] [PubMed]
- Szori, M.; Fittschen, C.; Csizmadia, I.G.; Viskolcz, B. Allylic H-Abstraction Mechanism: The Potential Energy Surface of the Reaction of Propene with OH Radical. J. Chem. Theory Comput. 2006, 2, 1575–1586. [Google Scholar] [CrossRef]
- Lu, T.; Chen, F. Multiwfn: A multifunctional wavefunction analyzer. J. Comput. Chem. 2012, 33, 580–592. [Google Scholar] [CrossRef]
- Dhaouadi, Z.; Nsangou, M.; Garrab, N.; Anouar, E.; Marakchi, K.; Lahmar, S. DFT study of the reaction of quercetin with ·O2− and ·OH radicals. J. Mol. Struct. THEOCHEM 2009, 904, 35–42. [Google Scholar] [CrossRef]
- Szori, M.; Csizmadia, I.G.; Viskolcz, B. Nonenzymatic Pathway of PUFA Oxidation. A First-Principles Study of the Reactions of OH Radical with 1,4-Pentadiene and Arachidonic Acid. J. Chem. Theory Comput. 2008, 4, 1472–1479. [Google Scholar] [CrossRef]
- Tawada, Y.; Tsuneda, T.; Yanagisawa, S.; Yanai, T.; Hirao, K. A long-range-corrected time-dependent density functional theory. J. Chem. Phys. 2004, 120, 8425–8433. [Google Scholar] [CrossRef]
- Kontogianni, V.G.; Gerothanassis, I.P. Analytical and structural tools of lipid hydroperoxides: Present state and future perspectives. Molecules 2022, 27, 2139. [Google Scholar] [CrossRef] [PubMed]
- Yang, W.S.; Kim, K.J.; Gaschler, M.M.; Patel, M.; Shchepinov, M.S.; Stockwell, B.R. Peroxidation of polyunsaturated fatty acids by lipoxygenases drives ferroptosis. Proc. Natl. Acad. Sci. USA 2016, 113, E4966–E4975. [Google Scholar] [CrossRef] [PubMed]
- Epperlein, M.M.; Nourooz-Zadeh, J.; Jayasena, S.D.; Hothersall, J.S.; Noronha-Dutra, A.; Neild, G.H. Nature and biological significance of free radicals generated during bicarbonate hemodialysis. J. Am. Soc. Nephrol. 1998, 9, 457–463. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09; Gaussian Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Yanai, T.; Tew, D.P.; Handy, N.C. A new hybrid exchange–correlation functional using the Coulomb-attenuating method (CAM-B3LYP). Chem. Phys. Lett. 2004, 393, 51–57. [Google Scholar] [CrossRef]
- Marenich, A.V.; Cramer, C.J.; Truhlar, D.G. Universal Solvation Model Based on Solute Electron Density and on a Continuum Model of the Solvent Defined by the Bulk Dielectric Constant and Atomic Surface Tensions. J. Phys. Chem. B 2009, 113, 6378–6396. [Google Scholar] [CrossRef] [PubMed]
- Miller, W.H. Quantum mechanical transition state theory and a new semiclassical model for reaction rate constants. J. Chem. Phys. 1974, 61, 1823–1834. [Google Scholar] [CrossRef]
- Canneaux, S.; Bohr, F.; Henon, E. KiSThelP: A program to predict thermodynamic properties and rate constants from quantum chemistry results. J. Comput. Chem. 2013, 35, 82–93. [Google Scholar] [CrossRef] [PubMed]
- Gilson, M.K.; Liu, T.; Baitaluk, M.; Nicola, G.; Hwang, L.; Chong, J. BindingDB in 2015: A public database for medicinal chemistry, computational chemistry and systems pharmacology. Nucleic Acids Res. 2016, 44, D1045–D1053. [Google Scholar] [CrossRef]
- Liu, Y.P.; Lu, D.H.; Gonzalez-Lafont, A.; Truhlar, D.G.; Garrett, B.C. Direct dynamics calculation of the kinetic isotope effect for an organic hydrogen-transfer reaction, including corner-cutting tunneling in 21 dimensions. J. Am. Chem. Soc. 1993, 115, 7806–7817. [Google Scholar] [CrossRef]
- Dennington, R.; Keith, T.; Millam, J. GaussView 5.0; Gaussian Inc.: Wallingford, CT, USA, 2008. [Google Scholar]


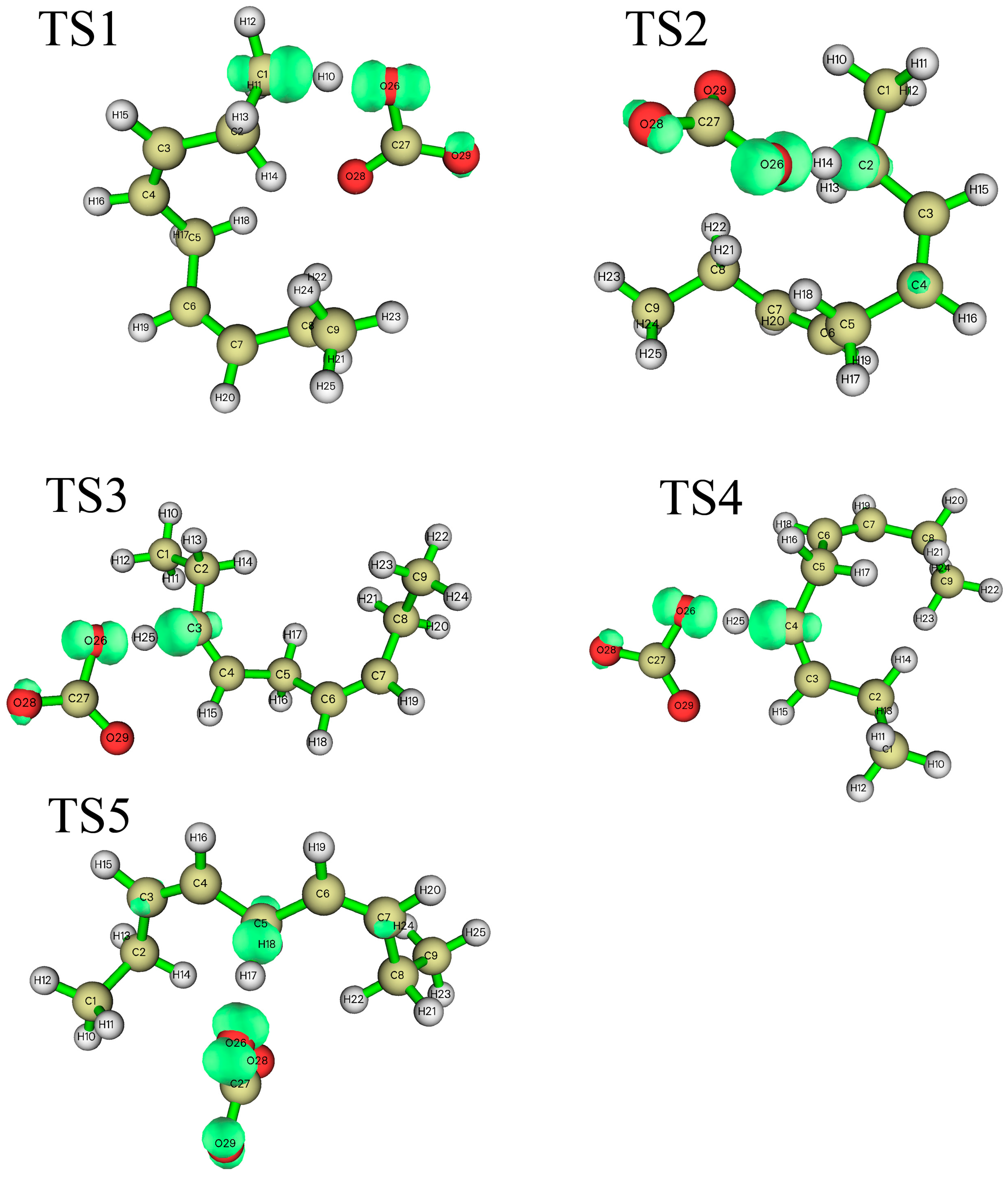
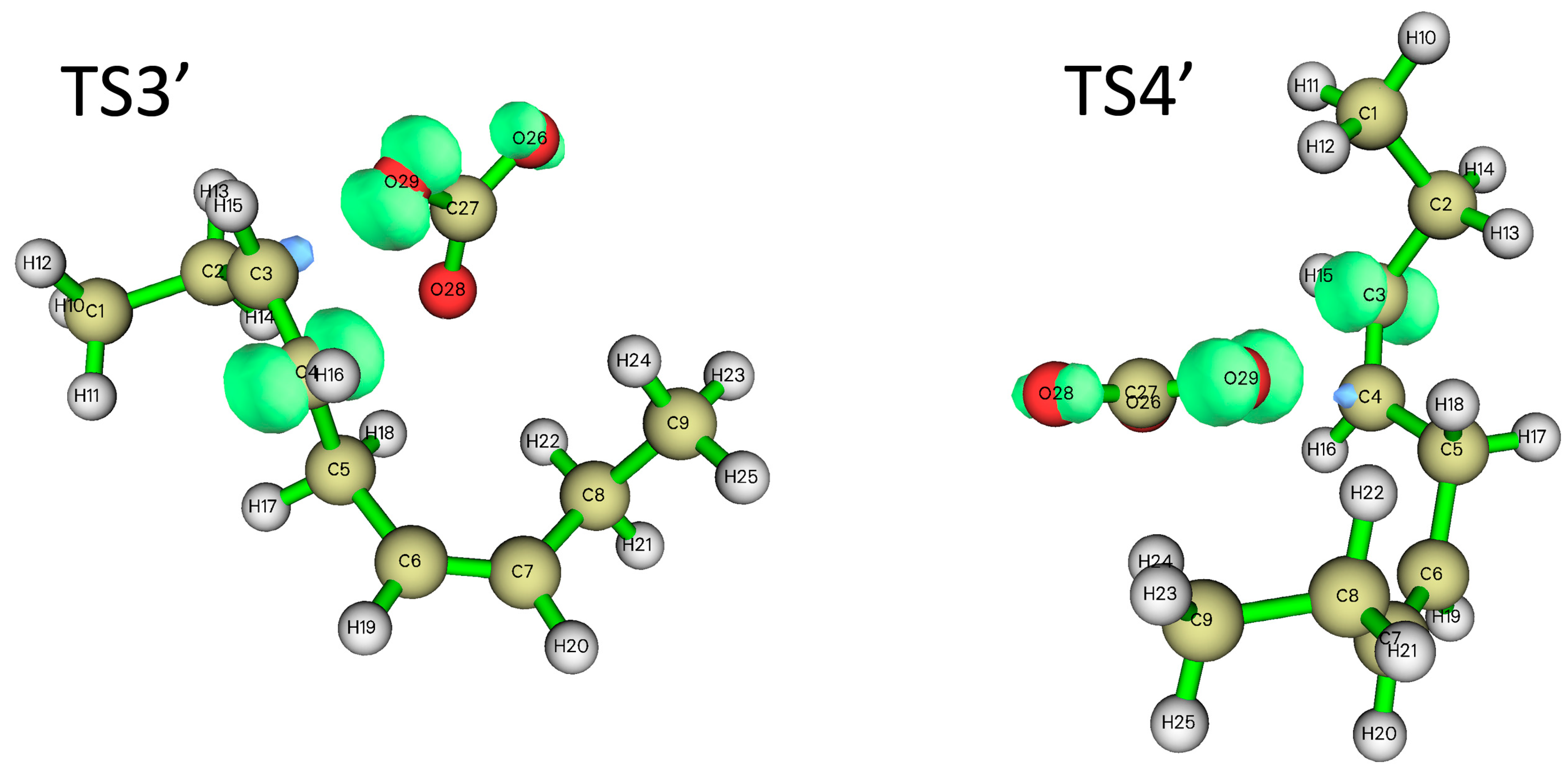
| Reaction | FTS (cm−1) | κ | QA | QB | QTS | ΔE (kcal/mol) | k (s−1/(mole/cm3)) |
|---|---|---|---|---|---|---|---|
| ① | −1994.99 | 4.871 | 6.38 × 1012 | 9.18 × 1017 | 2.39 × 1021 | 14.573 | 1.042 × 10−26 |
| ② | −1781.46 | 4.087 | - | - | 1.88 × 1021 | 8.786 | 1.208 × 10−22 |
| ③ | −2036.75 | 5.504 | - | - | 9.95 × 1021 | 16.688 | 1.263 × 10−27 |
| ④ | −2041.48 | 5.054 | - | - | 8.85 × 1021 | 16.975 | 6.934 × 10−28 |
| ⑤ | −1565.33 | 3.383 | - | - | 1.22 × 1021 | 6.186 | 5.232 × 10−21 |
| ③′ | −455.59 | 1.202 | - | - | 8.9 × 1020 | 11.632 | 1.375 × 10−25 |
| ④′ | −449.13 | 1.196 | - | - | 8.37 × 1020 | 11.740 | 1.078 × 10−25 |
| Reaction | (kcal/mol) | (kcal/mol) | (kcal/mol) |
|---|---|---|---|
| ① | 6.354 | 6.354 | 6.685 |
| ② | −12.518 | −12.518 | −10.535 |
| ③ | 11.995 | 11.995 | 13.311 |
| ④ | 12.568 | 12.568 | 13.735 |
| ⑤ | −22.327 | −22.327 | −20.656 |
| ③′ | −6.143 | −6.735 | 7.823 |
| ④′ | −9.614 | −10.206 | 4.046 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, H.; Xiong, S.-F.; Dong, L.-L.; Dai, Z.-T. Study on the Mechanism of Lipid Peroxidation Induced by Carbonate Radicals. Molecules 2024, 29, 1125. https://doi.org/10.3390/molecules29051125
Cao H, Xiong S-F, Dong L-L, Dai Z-T. Study on the Mechanism of Lipid Peroxidation Induced by Carbonate Radicals. Molecules. 2024; 29(5):1125. https://doi.org/10.3390/molecules29051125
Chicago/Turabian StyleCao, Heng, Sheng-Feng Xiong, Li-Long Dong, and Zhou-Tong Dai. 2024. "Study on the Mechanism of Lipid Peroxidation Induced by Carbonate Radicals" Molecules 29, no. 5: 1125. https://doi.org/10.3390/molecules29051125
APA StyleCao, H., Xiong, S.-F., Dong, L.-L., & Dai, Z.-T. (2024). Study on the Mechanism of Lipid Peroxidation Induced by Carbonate Radicals. Molecules, 29(5), 1125. https://doi.org/10.3390/molecules29051125





