Reversible Surface Energy Storage in Molecular-Scale Porous Materials
Abstract
1. Introduction
2. Results and Discussion
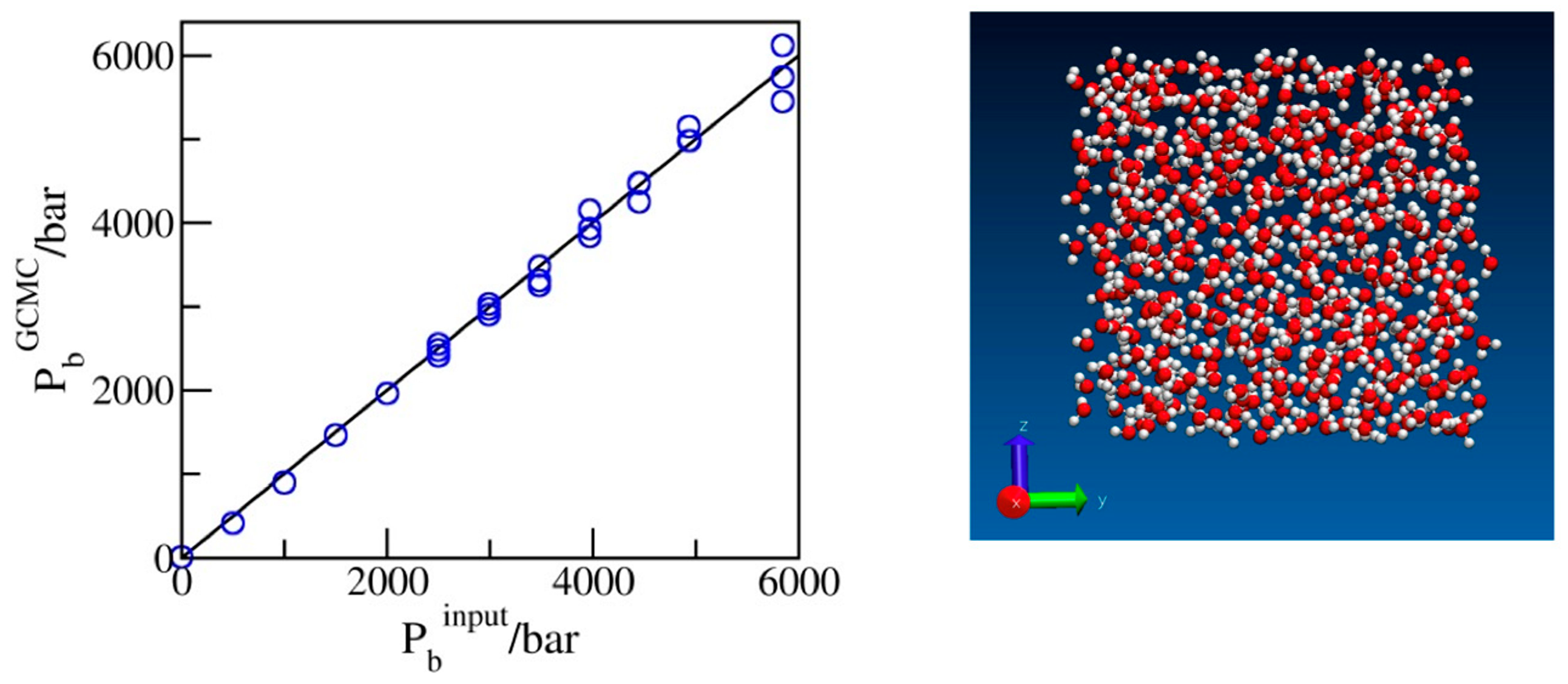
2.1. Benchmarking
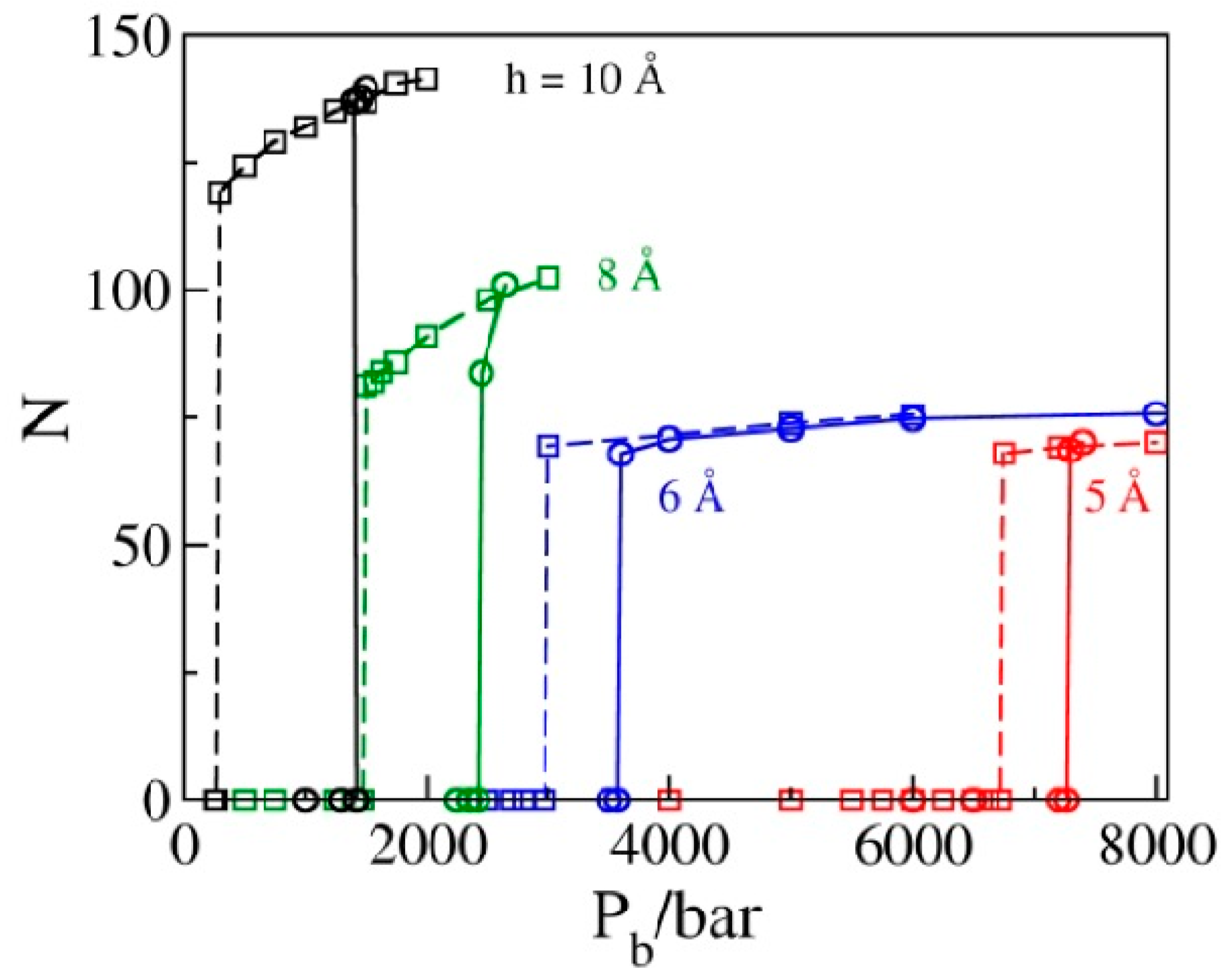
2.2. Intrusion/Expulsion Cycling
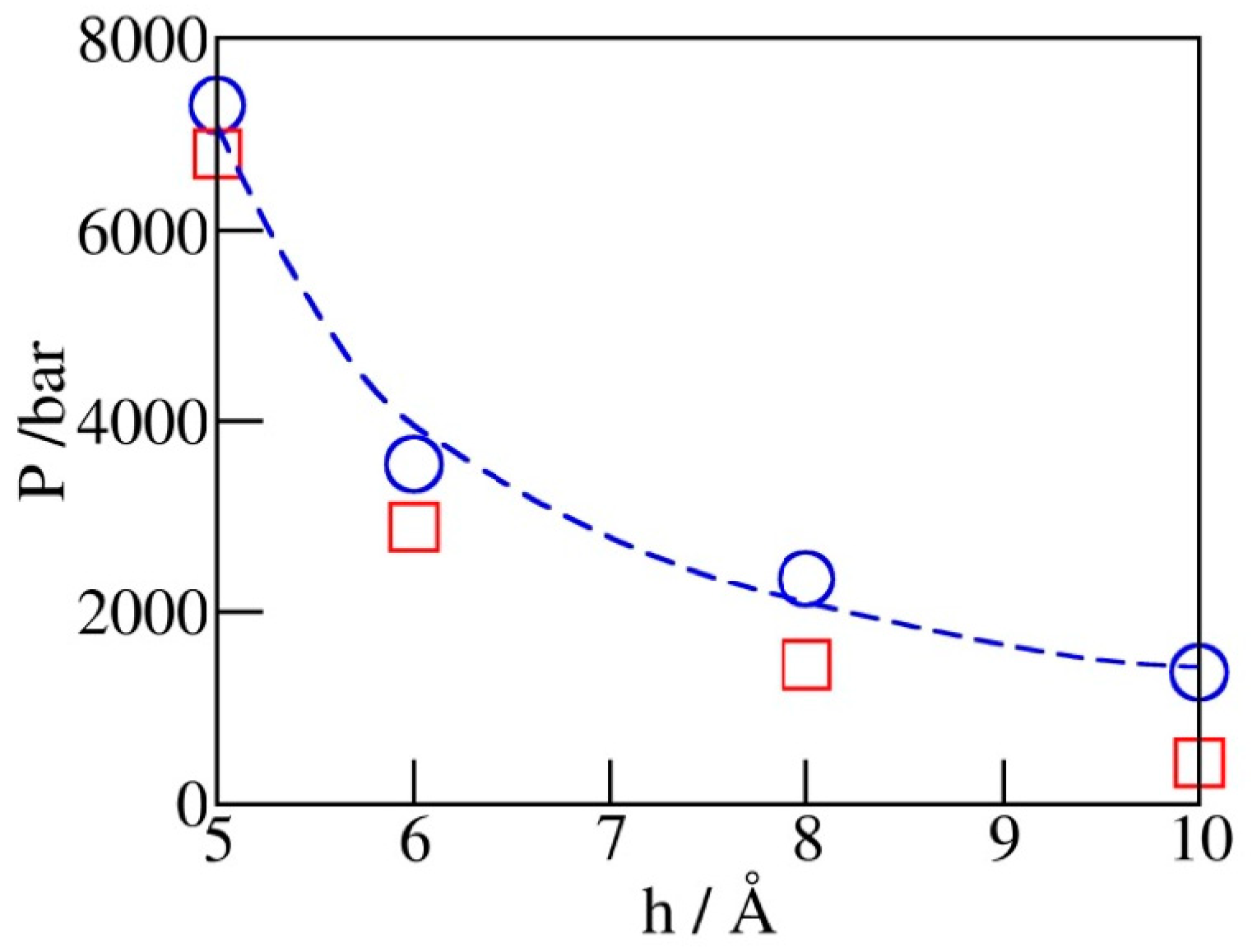
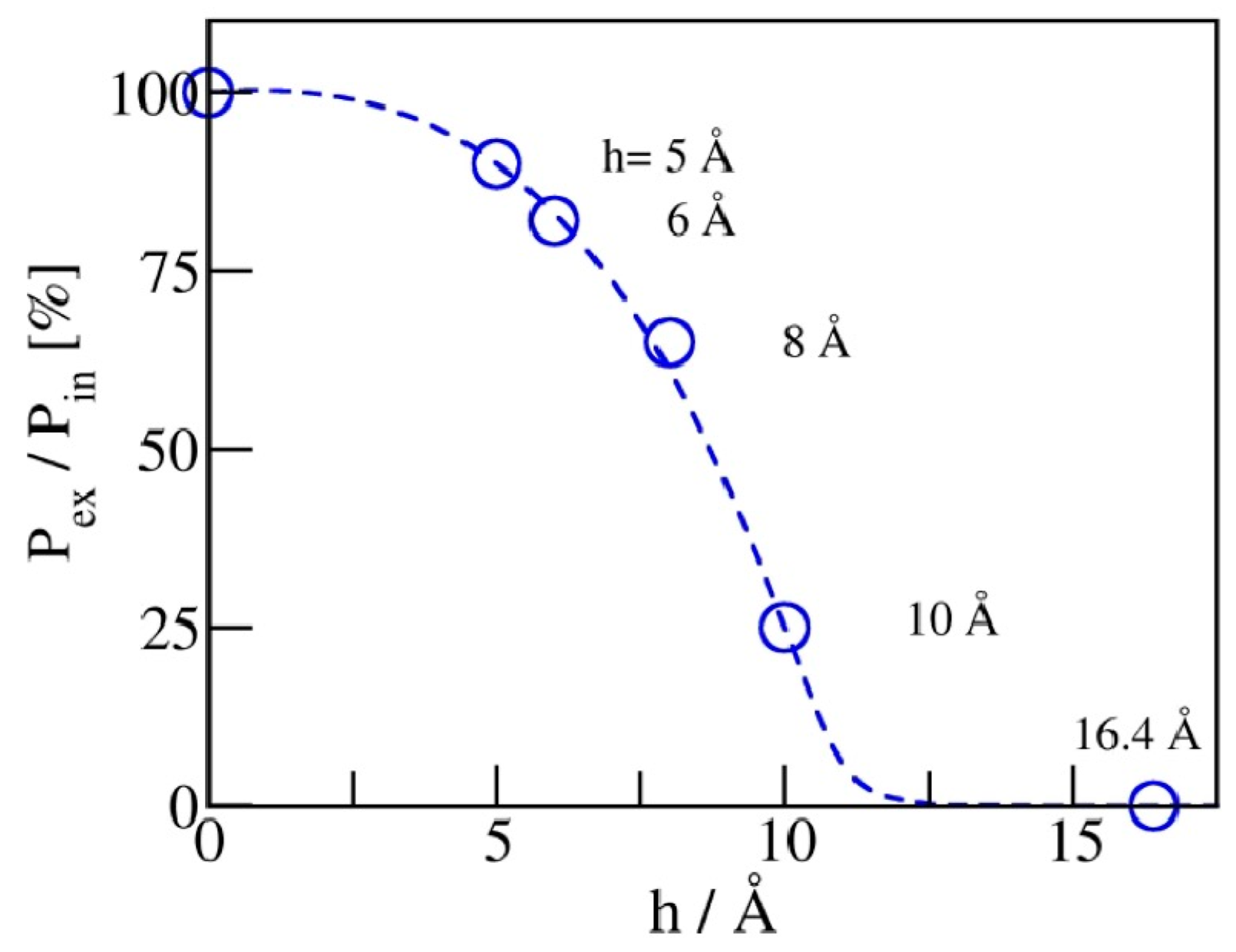
2.3. Phase Transition Pressures
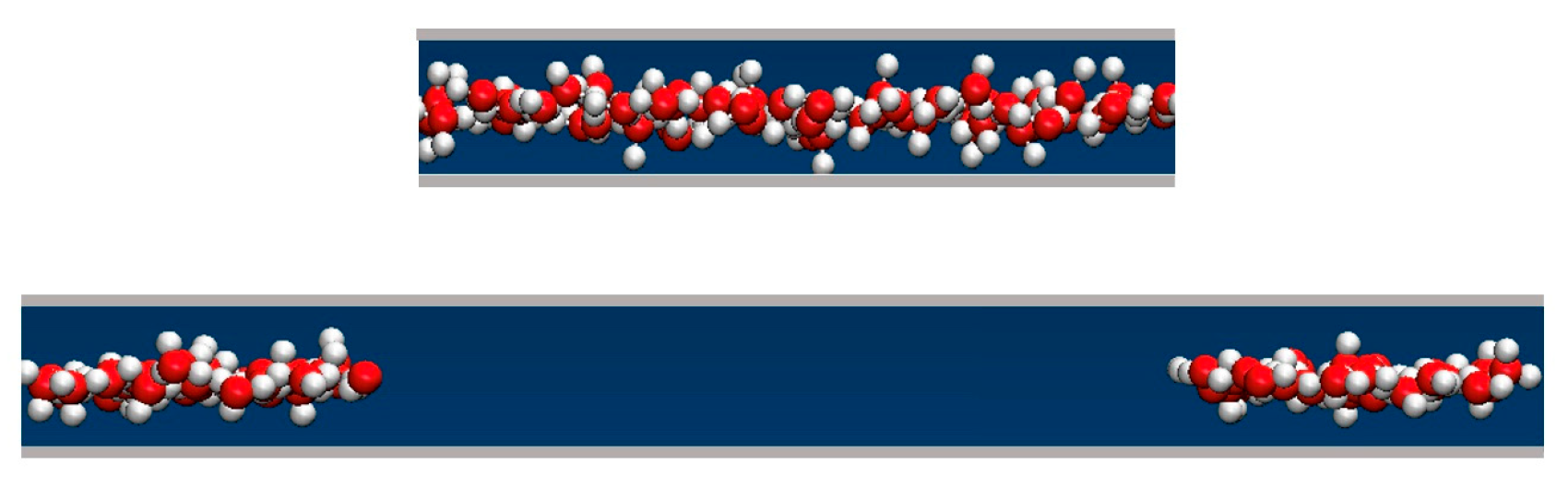
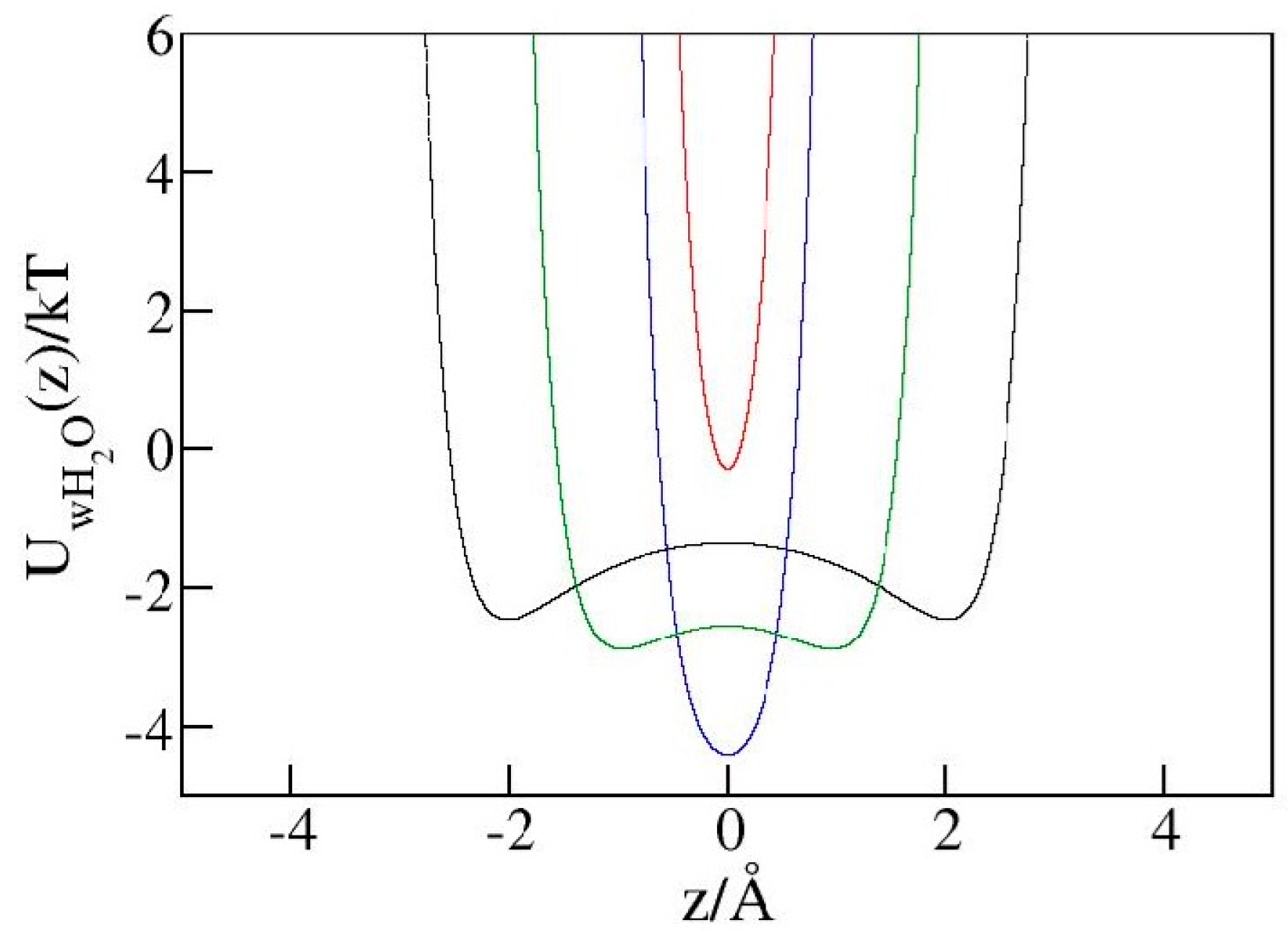
2.4. Liquid Structure in Narrow Pores
2.5. Lyophilic Absorbent
2.6. Limiting Energy Density
2.7. Polydispersity
3. Models and Methods
3.1. Models
3.2. Simulation Methods
4. Concluding Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Grosu, Y.; Mierzwa, M.; Eroshenko, V.A.; Pawlus, S.; Chorazewski, M.; Nedelec, J.M.; Grolier, J.P.E. Mechanical, Thermal, and Electrical Energy Storage in a Single Working Body: Electrification and Thermal Effects upon Pressure-Induced Water Intrusion Extrusion in Nanoporous Solids. ACS Appl. Mater. Interfaces 2017, 9, 7044–7049. [Google Scholar] [CrossRef] [PubMed]
- Ryzhikov, A.; Khay, I.; Nouali, H.; Daou, T.J.; Patarin, J. Drastic change of the intrusion-extrusion behavior of electrolyte solutions in pure silica (star)BEA-type zeolite. Phys. Chem. Chem. Phys. 2014, 16, 17893–17899. [Google Scholar] [CrossRef] [PubMed]
- Ryzhikov, A.; Khay, I.; Nouali, H.; Daou, T.J.; Patarin, J. High pressure intrusion–extrusion of electrolyte solutions in aluminosilicate FAU and BEA-type zeolites. Micropor. Mesopor. Mater. 2016, 221, 1–7. [Google Scholar] [CrossRef]
- Han, A.; Qiao, Y. A volume-memory liquid. Appl. Phys. Lett. 2007, 91, 173123. [Google Scholar] [CrossRef]
- Saada, M.A.; Rigolet, S.; Paillaud, J.-L.; Bats, N.; Soulard, M.; Patarin, J. Investigation of the Energetic Performance of Pure Silica ITQ-4 (IFR) Zeolite under High Pressure Water Intrusion. J. Phys. Chem. C 2010, 114, 11650–11658. [Google Scholar] [CrossRef]
- Le Donne, A.; Tinti, A.; Amayuelas, E.; Kashyap, H.K.; Camisasca, G.; Remsing, R.C.; Roth, R.; Grosu, Y.; Meloni, S. Intrusion and extrusion of liquids in highly confining media: Bridging fundamental research to applications. Adv. Phys. X 2022, 7, 2052353. [Google Scholar] [CrossRef]
- Tinti, A.; Giacomello, A.; Grosu, Y.; Casciola, C.M. Intrusion and extrusion of water in hydrophobic nanopores. Proc. Natl. Acad. Sci. USA 2017, 114, E10266–E10273. [Google Scholar] [CrossRef]
- Confalonieri, G.; Daou, T.J.; Nouali, H.; Arletti, R.; Ryzhikov, A. Energetic Performance of Pure Silica Zeolites under High-Pressure Intrusion of LiCl Aqueous Solutions: An Overview. Molecules 2020, 25, 2145. [Google Scholar] [CrossRef]
- Fraux, G.; Coudert, F.X.; Boutin, A.; Fuchs, A.H. Forced intrusion of water and aqueous solutions in microporous materials: From fundamental thermodynamics to energy storage devices. Chem. Soc. Rev. 2017, 46, 7421–7437. [Google Scholar] [CrossRef]
- Han, A.J.; Lu, W.Y.; Kim, T.; Chen, X.; Qiao, Y. Influence of anions on liquid infiltration and defiltration in a zeolite Y. Phys. Rev. E 2008, 78, 4. [Google Scholar] [CrossRef] [PubMed]
- Eroshenko, V.A.; Regis, R.C.; Soulard, M.; Patarin, J. Energetics: A new field of applications for hydrophobic zeolites. J. Am. Chem. Soc. 2001, 123, 8129–8130. [Google Scholar] [CrossRef] [PubMed]
- Eroshenko, V.A.; Regis, R.C.; Soulard, M.; Patarin, J. The heterogeneous systems ‘water-hydrophobic zeolites’: New molecular springs. Compt. Rend. Phys. 2002, 3, 111–119. [Google Scholar] [CrossRef]
- Soulard, M.; Patarin, J.; Eroshenko, V.A.; Regis, R. Molecular spring or bumper: A new application for hydrophobic zeolitic materials. In Studies in Surface Science and Catalysis; Elsevier: Amsterdam, The Netherlands, 2004; Volume 154, pp. 1830–1837. [Google Scholar]
- Xu, R.; Pang, W.; Yu, J.; Huo, Q.; Chen, J. Chemistry of Zeolites and Related Porous Materials Synthesis and Structure; John Wiley & Sons Asia: Hoboken, NJ, USA, 2007. [Google Scholar]
- Confalonieri, G.; Ryzhikov, A.; Arletti, R.; Quartieri, S.; Vezzalini, G.; Isaac, C.; Paillaud, J.-L.; Nouali, H.; Daou, T.J. Structural interpretation of the energetic performances of a pure silica LTA-type zeolite. Phys. Chem. Chem. Phys. 2020, 22, 5178–5187. [Google Scholar] [CrossRef] [PubMed]
- Cambiaso, S.R.F.; Tinti, A.; Bochicchio, D.; Grosu, Y.; Rossi, G.; Giacomello, A. Grafting heterogeneities rule water intrusion and extrusion in nanopores. arXiv 2023, arXiv:2305.15250. [Google Scholar]
- Grosu, Y.; Li, M.; Peng, Y.L.; Luo, D.; Li, D.; Faik, A.; Nedelec, J.M.; Grolier, J.P. A Highly Stable Nonhysteretic {Cu-2(tebpz) MOF plus water} Molecular Spring. Chem. Phys. Chem. 2016, 17, 3359–3364. [Google Scholar] [CrossRef]
- Leung, K.; Luzar, A. Dynamics of capillary evaporation. II. Free energy barriers. J. Chem. Phys. 2000, 113, 5845–5852. [Google Scholar] [CrossRef]
- Leung, K.; Luzar, A.; Bratko, D. Dynamics of capillary drying in water. Phys. Rev. Lett. 2003, 90, 065502. [Google Scholar] [CrossRef]
- Sharma, S.; Debenedetti, P.G. Free Energy Barriers to Evaporation of Water in Hydrophobic Confinement. J. Phys. Chem. B 2012, 116, 13282–13289. [Google Scholar] [CrossRef]
- Gao, Y.; Li, M.Z.; Zhang, Y.; Lu, W.Y.; Xu, B.X. Spontaneous outflow efficiency of confined liquid in hydrophobic nanopores. Proc. Natl. Acad. Sci. USA 2020, 117, 25246–25253. [Google Scholar] [CrossRef]
- Yaminsky, V.V.; Ohnishi, S.; Ninham, B. Long-range hydrophobic forces due to capillary bridging. In Handbook of Surfaces and Interfaces of Materials; Nalwa, H.S., Ed.; Academic Press: New York, NY, USA, 2001; Volume 4, pp. 131–227. [Google Scholar]
- Luzar, A. Activation barrier scaling for the spontaneous evaporation of confined water. J. Phys. Chem. B 2004, 108, 19859–19866. [Google Scholar] [CrossRef]
- Ghasemi, M.; Ramsheh, S.M.; Sharma, S. Quantitative Assessment of Thermodynamic Theory in Elucidating the Behavior of Water under Hydrophobic Confinement. J. Phys. Chem. B 2018, 122, 12087–12096. [Google Scholar] [CrossRef] [PubMed]
- Tinti, A.; Giacomello, A.; Meloni, S.; Casciola, C.M. Classical nucleation of vapor between hydrophobic plates. J. Chem. Phys. 2023, 158, 134708. [Google Scholar] [CrossRef] [PubMed]
- Guillemot, L.; Biben, T.; Galarneau, A.; Vigier, G.; Charlaix, E. Activated drying in hydrophobic nanopores and the line tension of water. Proc. Natl. Acad. Sci. USA 2012, 109, 19557–19562. [Google Scholar] [CrossRef] [PubMed]
- Bey, R.; Coasne, B.; Picard, C. Probing the concept of line tension down to the nanoscale. J. Chem. Phys. 2020, 152, 094707. [Google Scholar] [CrossRef] [PubMed]
- Bratko, D.; Curtis, R.A.; Blanch, H.W.; Prausnitz, J.M. Interaction between hydrophobic surfaces with metastable intervening liquid. J. Chem. Phys. 2001, 115, 3873–3877. [Google Scholar] [CrossRef]
- Giovambattista, N.; Rossky, P.J.; Debenedetti, P.G. Effect of pressure on the phase behavior and structure of water confined between nanoscale hydrophobic and hydrophilic plates. Phys. Rev. E 2006, 73, 041604. [Google Scholar] [CrossRef]
- Moucka, F.; Bratko, D.; Luzar, A. Electrolyte pore/solution partitioning by expanded grand canonical ensemble Monte Carlo simulation. J. Chem. Phys. 2015, 142, 124705. [Google Scholar] [CrossRef]
- Zamfir, S.G.M.F.; Bratko, D. High-Pressure Infiltration–Expulsion of Aqueous NaCl in Planar Hydrophobic Nanopores. J. Phys. Chem. C 2020, 124, 23433–23445. [Google Scholar] [CrossRef]
- Ronchi, L.; Ryzhikov, A.; Nouali, H.; Daou, T.J.; Patarin, J. Energetic performances of FER-type zeolite in the presence of electrolyte solutions under high pressure. Energy 2017, 130, 29–37. [Google Scholar] [CrossRef]
- Michelin-Jamois, M.; Picard, C.; Vigier, G.; Charlaix, E. Giant Osmotic Pressure in the Forced Wetting of Hydrophobic Nanopores. Phys. Rev. Lett. 2015, 115, 036101. [Google Scholar] [CrossRef]
- Teplukhin, A.V. Thermodynamic and Structural Characteristics of SPC/E Water at 290 K under High Pressure. J. Struct. Chem. 2019, 2019, 1590–1598. [Google Scholar] [CrossRef]
- Lum, K.; Chandler, D.; Weeks, J.D. Hydrophobicity at small and large length scales. J. Phys. Chem. B 1999, 103, 4570–4577. [Google Scholar] [CrossRef]
- Paulo, G.; Gubbiotti, A.; Giacomello, A. An atomistically informed multiscale approach to the intrusion and extrusion of water in hydrophobic nanopores. J. Chem. Phys. 2023, 158, 204707. [Google Scholar] [CrossRef]
- Liu, J.C.; Monson, P.A. Does water condense in carbon pores? Langmuir 2005, 21, 10219–10225. [Google Scholar] [CrossRef] [PubMed]
- Tinti, A.; Camisasca, G.; Giacomello, A. Structure and dynamics of water confined in cylindrical nanopores with varying hydrophobicity. Phil. Trans. Roy. Soc. A 2021, 379, 20200403. [Google Scholar] [CrossRef]
- Deroche, I.; Daou, T.J.; Picard, C.; Coasne, B. Reminiscent capillarity in subnanopores. Nat. Commun. 2019, 10, 4642. [Google Scholar] [CrossRef] [PubMed]
- Ronchi, L.; Ryzhikov, A.; Nouali, H.; Daou, T.J.; Patarin, J. Energetic Performances of Pure-Silica DDR Zeolite by High-Pressure Intrusion-Extrusion of Electrolyte Aqueous Solutions: A Shock-Absorber with Huge Absorbed Energy. J. Phys. Chem. C 2018, 122, 2726–2733. [Google Scholar] [CrossRef]
- Li, R.Y.Z.; Xia, G. Effect of inter-pore interference on liquid evaporation rates from nanopores by direct simulation Monte Carlo. Phys. Fluids 2023, 35, 032015. [Google Scholar] [CrossRef]
- Vanzo, D.; Luzar, A.; Bratko, D. Pressure-sensitive conversions between Cassie and Wenzel wetting states on a nanocorrugated surface. Appl. Phys. A 2022, 128, 323. [Google Scholar] [CrossRef]
- Mehrani, R.; Sharma, S. Stability of Water Confined between Supported Self-Assembled Monolayers. J. Phys. Chem. B 2022, 126, 5110–5116. [Google Scholar] [CrossRef]
- Altabet, Y.E.; Debenedetti, P.G. The role of material flexibility on the drying transition of water between hydrophobic objects: A thermodynamic analysis. J. Chem. Phys. 2014, 141, 18C531. [Google Scholar] [CrossRef]
- Altabet, Y.E.; Haji-Akbari, A.; Debenedetti, P.G. Effect of material flexibility on the thermodynamics and kinetics of hydrophobically induced evaporation of water. Proc. Natl. Acad. Sci. USA 2017, 114, E2548–E2555. [Google Scholar] [CrossRef] [PubMed]
- Bratko, D.; Daub, C.D.; Leung, K.; Luzar, A. Effect of field direction on electrowetting in a nanopore. J. Am. Chem. Soc. 2007, 129, 2504–2510. [Google Scholar] [CrossRef] [PubMed]
- Ritchie, J.A.; Yazdi, J.S.; Bratko, D.; Luzar, A. Metastable Sessile Nanodroplets on Nanopatterned Surfaces. J. Phys. Chem. C 2012, 116, 8634–8641. [Google Scholar] [CrossRef]
- Daub, C.D.; Wang, J.; Kudesia, S.; Bratko, D.; Luzar, A. The influence of molecular-scale roughness on the surface spreading of an aqueous nanodrop. Faraday Discuss. 2010, 146, 67–77. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The Missing Term In Effective Pair Potentials. J. Phys. Chem. B 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Lee, S.H.; Rossky, P.J. A Comparison of the Structure and Dynamics of Liquid Water at Hydrophobic and Hydrophilic Surfaces—A Molecular-Dynamics Simulation Study. J. Chem. Phys. 1994, 100, 3334–3345. [Google Scholar] [CrossRef]
- Shelley, J.C.; Patey, G.N. Boundary condition effects in simulations of water confined between planar walls. Mol. Phys. 1996, 88, 385–398. [Google Scholar] [CrossRef]
- Bratko, D.; Jonsson, B.; Wennerstrom, H. Electrical Double-Layer Interactions with Image Charges. Chem. Phys. Lett. 1986, 128, 449–454. [Google Scholar] [CrossRef]
- Gloor, G.J.; Jackson, G.; Blas, F.J.; de Miguel, E. Test-area simulation method for the direct determination of the interfacial tension of systems with continuous or discontinuous potentials. J. Chem. Phys. 2005, 123, 134703. [Google Scholar] [CrossRef] [PubMed]
- Moucka, F.; Bratko, D.; Luzar, A. Salt and Water Uptake in Nanoconfinement under Applied Electric Field: An Open Ensemble Monte Carlo Study. J. Phys. Chem. C 2015, 119, 20416–20425. [Google Scholar] [CrossRef]
- Moucka, F.; Zamfir, S.; Bratko, D.; Luzar, A. Molecular polarizability in open ensemble simulations of aqueous nanoconfinements under electric field. J. Chem. Phys. 2019, 150, 164702. [Google Scholar] [CrossRef]
- Vanzo, D.; Bratko, D.; Luzar, A. Wettability of pristine and alkyl-functionalized graphane. J. Chem. Phys. 2012, 137, 034707. [Google Scholar] [CrossRef]
- Bratko, D.; Chakraborty, A.K.; Shakhnovich, E.I. Frozen phases of random heteropolymers in disordered media. Phys. Rev. Lett. 1996, 76, 1844–1847. [Google Scholar] [CrossRef] [PubMed]
- Bratko, D.; Striolo, A.; Wu, J.Z.; Blanch, H.W.; Prausnitz, J.M. Orientation-averaged pair potentials between dipolar proteins or colloids. J. Phys. Chem. B 2002, 106, 2714–2720. [Google Scholar] [CrossRef]
- Jaffe, R.L.; Gonnet, P.; Werder, T.; Walther, J.H.; Koumoutsakos, P. Water-carbon interactions—2: Calibration of potentials using contact angle data for different interaction models. Mol. Simul. 2004, 30, 205–216. [Google Scholar] [CrossRef]
- Yeh, I.C.; Berkowitz, M.L. Ewald summation for systems with slab geometry. J. Chem. Phys. 1999, 111, 3155–3162. [Google Scholar] [CrossRef]
- Frenkel, D.; Smit, B. Understanding Molecular Simulation, from Algorithms to Applications; Academic: San Diego, CA, USA, 2002. [Google Scholar]
- Adams, D.J. Chemical potential of hard-sphere fluid by Monte Carlo methods. Mol. Phys. 1974, 28, 1241–1252. [Google Scholar] [CrossRef]
- Adams, D.J. Grand Canonical Monte Carlo for Lennard Jones fluid. Mol. Phys. 1975, 29, 307–311. [Google Scholar] [CrossRef]
- Moucka, F.; Nezbeda, I.; Smith, W.R. Chemical Potentials, Activity Coefficients, and Solubility in Aqueous NaCl Solutions: Prediction by Polarizable Force Fields. J. Chem. Theory Comput. 2015, 11, 1756–1764. [Google Scholar] [CrossRef]
- Adams, L.H. Equilibrium in binary systems under pressure. I. An experimental and thermodynamic investigation of the system, NaC1-H2O, at 25°. J. Am. Chem. Soc. 1931, 53, 3769–3813. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bratko, D. Reversible Surface Energy Storage in Molecular-Scale Porous Materials. Molecules 2024, 29, 664. https://doi.org/10.3390/molecules29030664
Bratko D. Reversible Surface Energy Storage in Molecular-Scale Porous Materials. Molecules. 2024; 29(3):664. https://doi.org/10.3390/molecules29030664
Chicago/Turabian StyleBratko, Dusan. 2024. "Reversible Surface Energy Storage in Molecular-Scale Porous Materials" Molecules 29, no. 3: 664. https://doi.org/10.3390/molecules29030664
APA StyleBratko, D. (2024). Reversible Surface Energy Storage in Molecular-Scale Porous Materials. Molecules, 29(3), 664. https://doi.org/10.3390/molecules29030664





