Next Article in Journal
Free Radical Production Induced by Nitroimidazole Compounds Lead to Cell Death in Leishmania infantum Amastigotes
Next Article in Special Issue
Revolutionizing Molecular Design for Innovative Therapeutic Applications through Artificial Intelligence
Previous Article in Journal
Heteroleptic Complexes of Ruthenium Nitrosyl with Pyridine and Bypiridine—Synthesis and Photoisomerization
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 29
Issue 17
10.3390/molecules29174040
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Structural Insights into Endostatin–Heparan Sulfate Interactions Using Modeling Approaches

by
Urszula Uciechowska-Kaczmarzyk
1,
Martin Frank
2,
Sergey A. Samsonov
1 and
Martyna Maszota-Zieleniak
1,*
1
Laboratory of Molecular Modeling, Department of Theoretical Chemistry, Faculty of Chemistry, University of Gdansk, Wita Stwosza 63, 80-308 Gdańsk, Poland
2
Biognos AB, P.O. Box 8963, 40274 Göteborg, Sweden
*
Author to whom correspondence should be addressed.
Molecules 2024, 29(17), 4040; https://doi.org/10.3390/molecules29174040
Submission received: 19 July 2024 / Revised: 19 August 2024 / Accepted: 22 August 2024 / Published: 26 August 2024
(This article belongs to the Special Issue Computational Insights into Protein Engineering and Molecular Design)
Browse Figures
Review Reports Versions Notes

Abstract

:
Glycosaminoglycans (GAGs) play a key role in a variety of biological processes in the extracellular matrix (ECM) via interactions with their protein targets. Due to their high flexibility, periodicity and electrostatics-driven interactions, GAG-containing complexes are very challenging to characterize both experimentally and in silico. In this study, we, for the first time, systematically analyzed the interactions of endostatin, a proteolytic fragment of collagen XVIII known to be anti-angiogenic and anti-tumoral, with heparin (HP) and representative heparan sulfate (HS) oligosaccharides of various lengths, sequences and sulfation patterns. We first used conventional molecular docking and a docking approach based on a repulsive scaling–replica exchange molecular dynamics technique, as well as unbiased molecular dynamic simulations, to obtain dynamically stable GAG binding poses. Then, the corresponding free energies of binding were calculated and the amino acid residues that contribute the most to GAG binding were identified. We also investigated the potential influence of Zn2+ on endostatin–HP complexes using computational approaches. These data provide new atomistic details of the molecular mechanism of HP’s binding to endostatin, which will contribute to a better understanding of its interplay with proteoglycans at the cell surface and in the extracellular matrix.

1. Introduction

Glycosaminoglycans (GAGs), a class of long linear anionic polysaccharides with disaccharide repetitive units [1], play important roles in many crucial biological processes through their interactions with numerous protein partners [2,3,4]. GAGs are known to interact with intracellular, secreted or membrane-bound proteins and thereby regulate cell proliferation, migration, differentiation, angiogenesis, axon guidance, responses to central nervous system (CNS) injury, virus attachment and entry, and immune response [5,6,7]. Therefore, a detailed structural understanding of protein–GAG interactions is important for deciphering the biological mechanisms they are involved in and for designing new therapeutic strategies for diseases such as cancer and autoimmune disorders [3]. Despite the importance of protein–GAG interactions, a limited number (less than 100) of experimental 3D structures of protein–GAG complexes are available [8,9]. Most of them represent complexes with heparin (HP) with a degree of polymerization (dp) ranging from dp2 to dp7 [8,10,11]. This relatively low number of available experimental structures is due to the structural complexity, conformational heterogeneity and flexibility of GAGs and to the difficulty of obtaining oligosaccharides of a defined length and sulfation pattern in sufficient amounts for structural studies [6]. Furthermore, experimental techniques are often not sufficient for studying the interactions occurring at an atomistic level in detail, whereas their combination with computational approaches has proved to be useful for the detailed characterization of structure–function relationships in protein–GAG complexes [12,13,14,15]. This study focuses on a GAG-binding protein, endostatin, which is an anti-angiogenic proteolytic fragment of collagen XVIII [16] that interacts with several proteins regulating angiogenesis at the surface of endothelial cells including VEGFR1 and VEGFR2 [17]. Recombinant endostatin has been tested in clinical studies and significantly improves the survival of patients with late-stage non-small-cell lung carcinoma. Clinical data have also been collected on patients with other cancers treated by endostatin [18]. Endostatin’s binding to heparan sulfate (HS) is enhanced in presence of Zn2+, and its antiproliferative effect on endothelial cells is stimulated by fibroblast growth factor-2 (FGF2) [19]. Mutations of amino acid residues that coordinate Zn2+ lead to the decrease of endostatin anti-tumor activity [20], but not its anti-proliferative effect on endothelial cells, stimulated by vascular endothelial growth factor (VEGF) [21], or its anti-angiogenic activity in a model of angiogenesis induced by VEGF [19]. The regulation of endostatin activities by Zn2+ appears to be context-dependent and warrants further investigation since Zn2+ itself is able to bind HP [22]. Zinc-dependent dimers have been observed in crystals of human endostatin [23], but attempts to co-crystallize murine endostatin with an HP dp4 failed [24]. The crystal structures of both human and murine endostatin are available, and two HP binding sites have been identified by mutagenesis [25] and predicted by molecular docking [19].
In this study, we aimed to improve our understanding of the molecular recognition of HP and HS by endostatin in its monomeric form. For this, we applied several computational approaches including conventional molecular docking and a novel effective docking approach based on a repulsive scaling replica exchange molecular dynamics technique [26] that is particularly efficient for GAGs [27] and allows for the docking of long GAG molecules. The obtained binding poses were further analyzed using molecular dynamics (MD) approaches. We analyzed the impact of individual endostatin residues on HP/HS binding in terms of their free energy contributions to GAG binding depending on the GAG type, length and sulfation pattern. Furthermore, we in silico investigated the effect of Zn2+ on the formation of endostatin–HP complexes. The effect of mutation of several amino acid residues of endostatin was computationally predicted to contribute to HP/HS binding to these GAGs. Our results provide new insights into the structural properties of endostatin–GAG complexes. They could also be used to fine-tune the affinity of endostatin to HP/HS oligosaccharides of various lengths and sequences and so to modulate the biological activities of endostatin mediated by HP/HS, which could be potentially an attractive therapeutic target.

2. Results

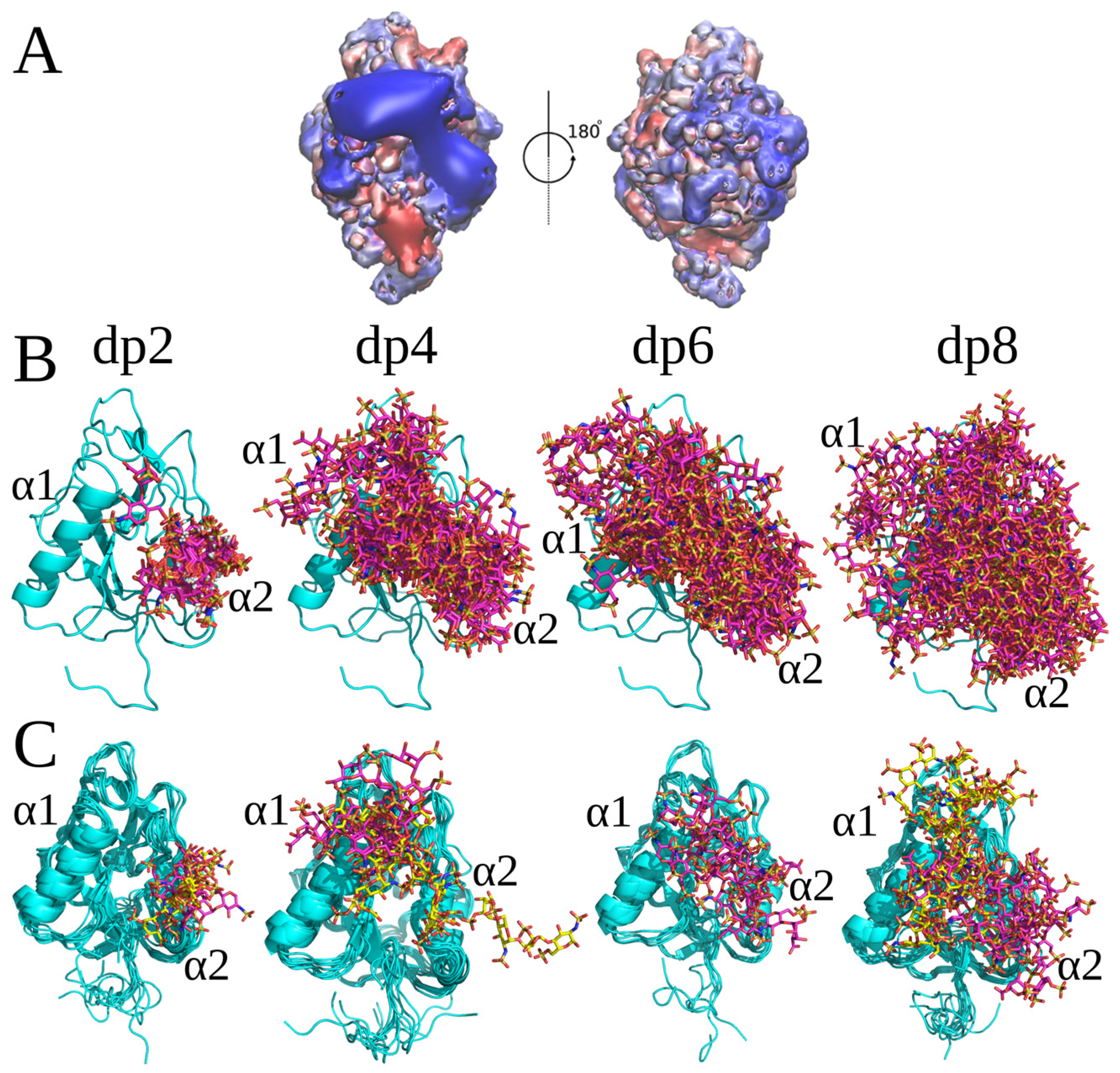
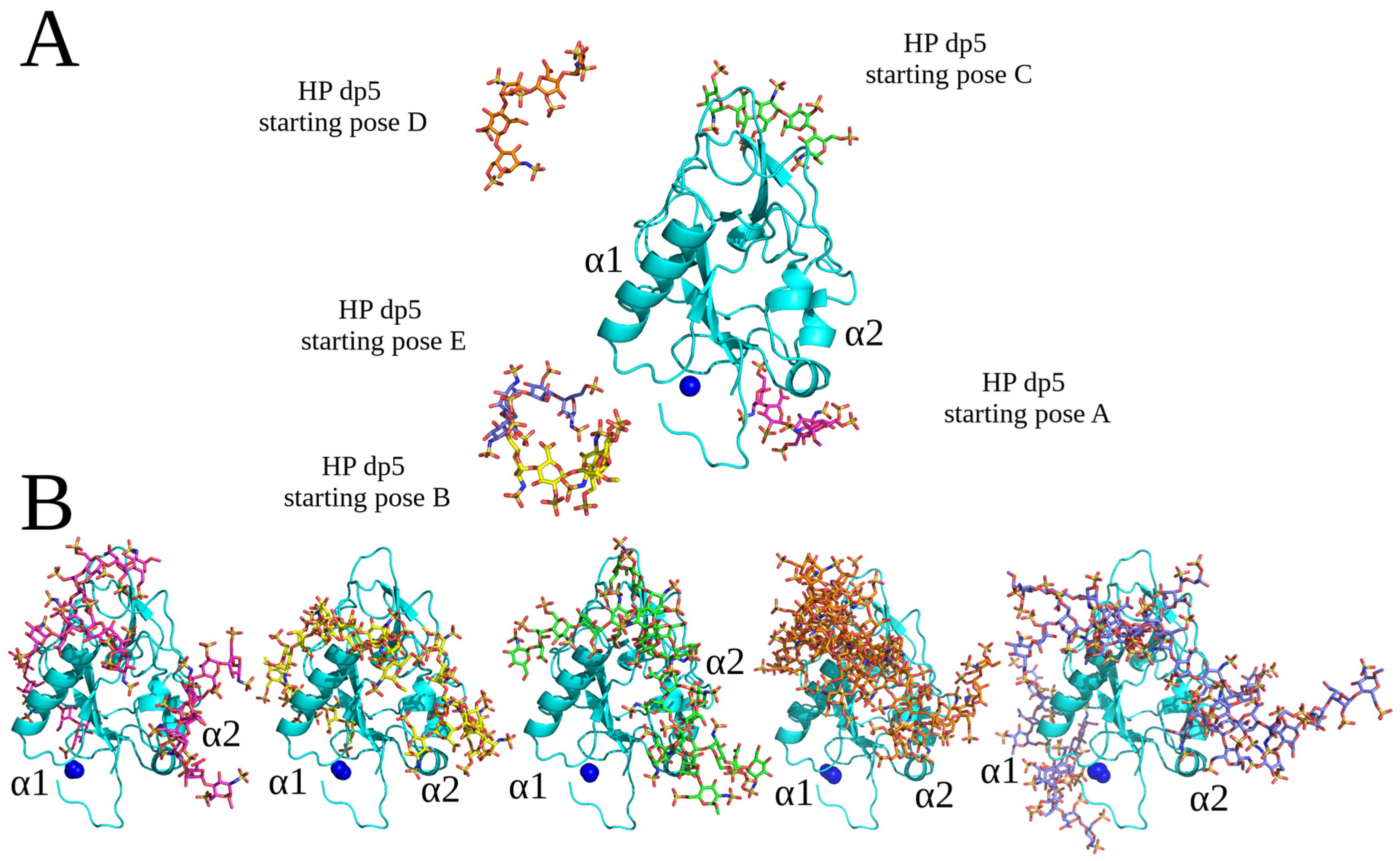
2.1. Conventional Molecular Docking and MD Simulations

Autodock 3 (AD3) molecular docking runs followed by MD simulations were used to predict and analyze the recognition of different GAGs by endostatin in its monomeric form. The length of the docked GAGs was varied from dp2 to dp8, aiming to define the core GAG binding site and to understand the dependence of binding on the GAG length. Since there are in total 48 possible different combinations of HP/HS dp2 periodic units [9], we selected several of the most representative combinations, including HP and four HS variants, for this analysis. Although a systematic screening study of all 48 combinations would be interesting to perform, this was, however, beyond the scope of this work due to the absence of available reference experimental data. The dp8 was used as the longest GAG due to the limitation of the AD3’s performance quality by the number of the ligand’s degrees of freedom [28]. All GAGs docked to the region of the protein corresponding to the extent of its positive electrostatic potential (Figure 1A). For HP structures with a 1C4 ring puckering conformation of IdoA(2S) (dp2, dp4, dp6 and dp8), two overlapping clusters of docking solutions were observed. They were all located in the region close to or between the two α-helices (α1, α2), which is the most positively charged area of endostatin (Figure 1). The docking solutions for dp2 and dp4 were close to the α2 helix (Figure 1B). The free energy of binding (ΔG) is more favorable for longer HP oligosaccharides. The residues that contributed most favorably to ΔG were Arg47, Arg53, Arg62, Arg63 and Arg66 for HP dp2 and Arg27 and Arg139 for HP dp4 (Table 1, Figure S1). All docking solutions for 1C4 HP dp6 and dp8 were located between the α1 and α2 helices (Figure 1), with a major contribution of Arg62, Arg63, Arg66, Arg24 and Arg27 (Table 1, Figure S1).
All docked HP with a 2S0 ring puckering conformation of IdoA(2S) bound at least partially similarly to HP, with a 1C4 conformation for IdoA(2S) (Figure 2). The only significant differences were observed for the binding poses of the HP dp4 and dp6. For HP dp4, the structures were aligned with the α1 helix and directed towards the N-terminal loop of endostatin (Figure 2), whereas they were located near α1 helix for the 1C4 HP dp4 (Figure 1). For HP dp6, in case of a 2S0 ring puckering conformation, an additional second most populated cluster was obtained, in which the structures can be seen as an elongation of the ones in the most populated cluster presented for oligosaccharides with both ring puckering conformations. The absence of similar differences for HP dp2 can be explained by the fact that dp4 may be the minimal length which adopts the same binding pose as longer polysaccharides. On the other hand, dp6 and dp8 seem to be long enough to interact with the α1 and α2 helices and N-terminal part of the protein at the same time. This is in agreement with our previous studies on the important role of IdoA(2S) ring conformational flexibility in HP for docking and MD-based free energy calculations in protein–GAG systems [29]. In addition to the different binding modes obtained for the HP molecules with different IdoA(2S) ring conformations, the corresponding free energies of binding, which were used as a measure of the stability of the obtained complexes, were also different (Table 1), suggesting a potential multipose binding characteristic for other protein–GAG systems of these short oligosaccharides [30,31,32] and a potential dependence of their affinity on the IdoA(2A) ring pucker.
The docking solutions obtained for desulfated HS dp2, dp4, dp6 and dp8 formed clusters that overlapped so that the corresponding binding poses of longer oligosaccharides represented the elongation of shorter ones (Figure S2). The free energy of binding for the desulfated HS was significantly less favorable than that for HP, confirming the importance of electrostatic interactions established by sulfate groups (Table 1, Figure S3). The binding pose of the desulfated HS dp2 was closer to the α1 helix compared to HP 1C4 and 2S0 dp2 (Figure S4). The desulfated HS dp4 has a binding pose similar to that of HP 1C4 dp4, with both interacting with the α2 helix and being directed to the N-terminal loop of endostatin (Figure S5). For desulfated HS dp6 and dp8, a higher similarity of their poses can be observed in comparison to both HP 1C4 and HP 2S0 (Figure 3), suggesting a more defined binding pose.
Docking predictions for HS dp8 with the repeating units GlcNS(6S)-GlcA, GlcNS-GlcA and GlcNS-IdoA(2S) and with IdoA(2S) in 1C4 and 2S0 ring conformations yielded two clusters (Figure 4A–D). Docking solutions corresponded to similar binding affinities and were located between the α1 and α2 helix. The binding affinity of HS dp8 with the GlcNS(6S)-GlcA unit, GlcNS-GlcA and GlcNS-IdoA(2S) 2S0 yielded on average ΔG ~ −50 kcal/mol, whereas the HS dp8 with the GlcNS-IdoA(2S) 1C4 unit resulted in ΔG ~ −60 kcal/mol (Table 1). This might be due to the stronger contribution of the Arg24, Arg27 and Arg139 residues to the binding (Figure S6). For HS (GlcNS-IdoA(2S))4, depending on the Ido(2S) ring pucker conformation, the second cluster was localized essentially differently.
To summarize the results obtained for HS oligosaccharides with the conventional docking approach, it was observed that not only the net sulfation degree but the particular sulfation pattern and IdoA(2S) can affect the affinity of these molecular complexes. At the same time, the performed analysis does not allow us to distinguish a particular binding pose but rather a co-existence of binding poses corresponding to a similar binding free energy (multipose binding).
Since Zn2+ binding is critical for endostatin to maintain a stable native structure [33] and is required for its biological activities, we also conducted molecular docking followed by an MD analysis of HP and several HS dp8 ligands using endostatin’s structure and including a Zn2+ ion (PDB ID: 1BNL) to analyze if the effect of the Zn2+ is also detectable with our computational approach (Figure S7). The reason to use dp8 in this part of the study was that while it is the biggest size of GAG that AD3 still can effectively deal with [28], it was shown to bind endostatin in a previous experimental study [19]. All the docked poses were found in the previously described binding sites predicted in the absence of Zn2+. In case of HP, the binding affinity obtained in our calculations increased in the presence of a Zn2+ ion (Table 1 and Table 2), while HP was bound closer to the α1 helix when the ion was present (Figure 5). Although there was no direct contact between HP dp8 and the ion observed, the more favorable binding energy could be attributed to a long-range electrostatics effect. The solutions obtained for HS with the repeating units GlcNS(6S)-GlcA and GlcNS-GlcA also formed clusters similar to those obtained in absence of Zn2+. In addition, the cluster located near the N-terminus of endostatin and Zn2+ was found for both HS oligosaccharides. For HS, this comprised GlcNS-IdoA(2S) disaccharide units with a 1C4 conformation; the structures within the cluster were further extended into the binding region than for the same dp6 with a 2S0 conformation (Figure S7). The presence of Zn2+ did not significantly affect the binding free energies in the complexes of endostatin with HS dp8 in contrast to with HP dp8 (Table 1 and Table 2, Figure S8).

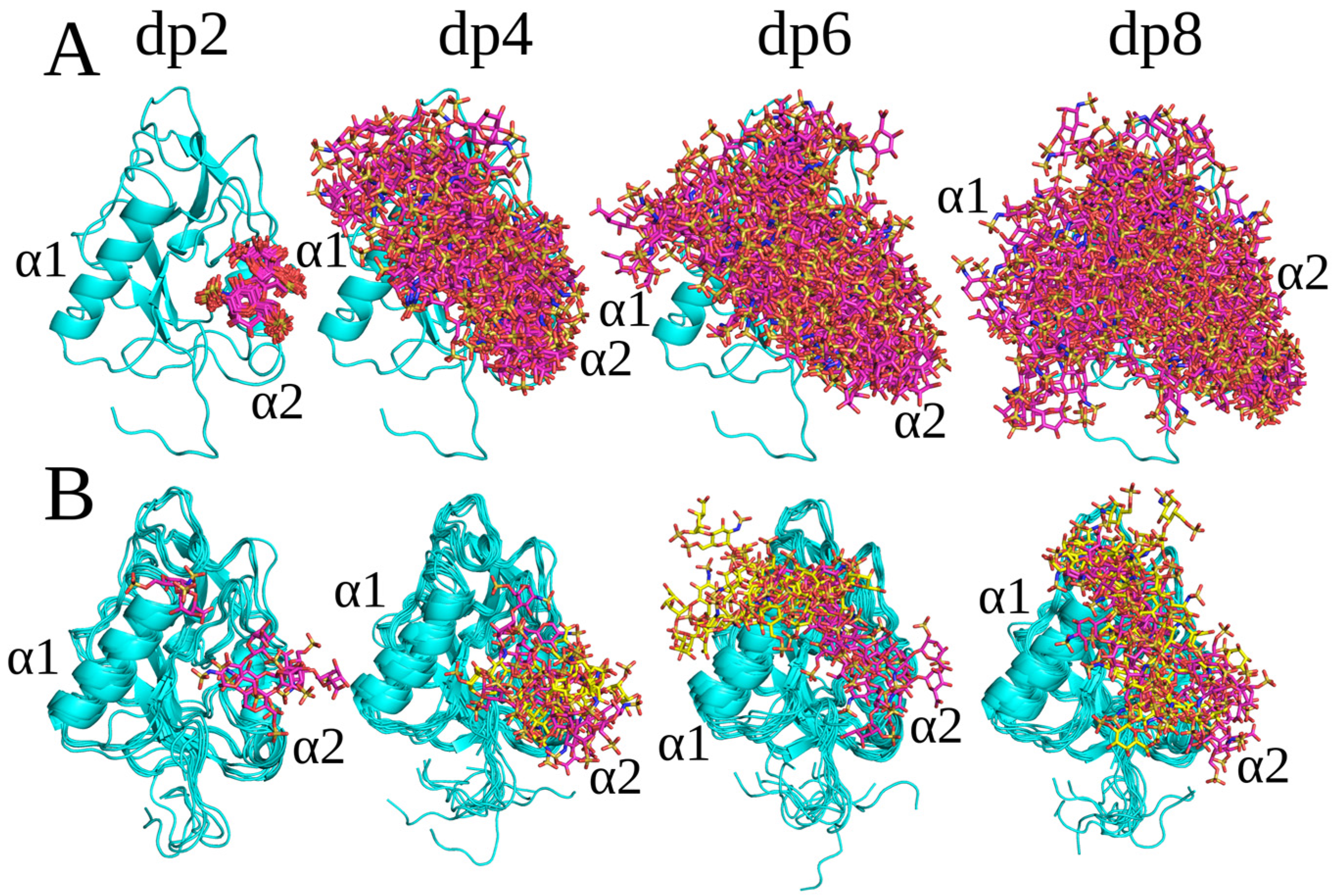
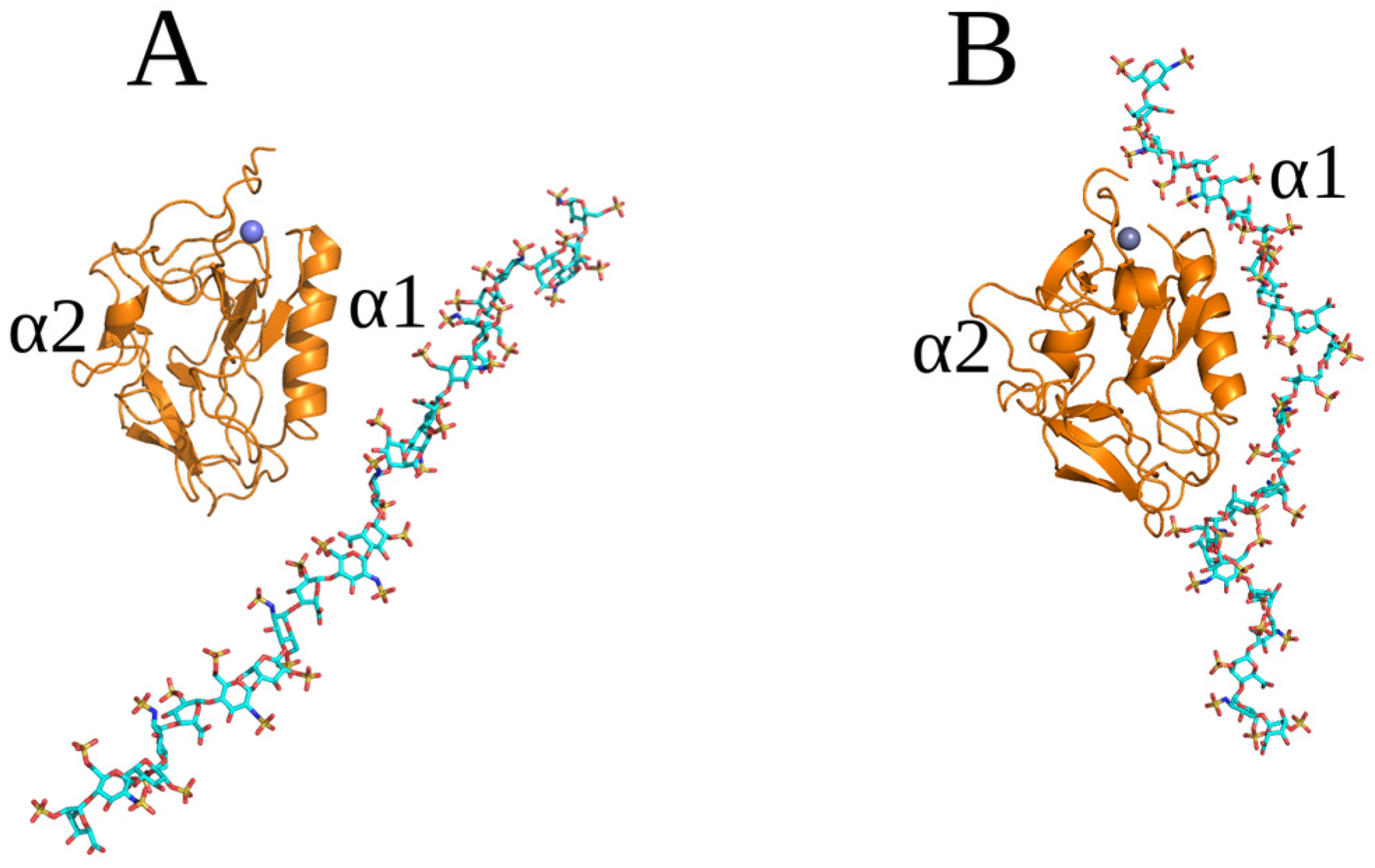
2.2. RS-REMD Analysis

As we stated before, oligosaccharide longer than dp8 are too long to expect a reliable performance of conventional docking approaches [34]. To check the interactions between endostatin and longer oligosaccharides, we performed RS-REMD simulations for the endostatin–HP dp24 complex. This length was chosen with the aim of being able to cover all the positive patches on the protein surface at once, while the HP sequence was used for this proof-of-concept analysis since this sequence is usually the most representative in the PDB and can be clearly defined in computational protein–GAG analyses. The convergence time of this complex during the RS-REMD simulation was around 5 ns. Then, the HP remained in the same binding site for the next 55 ns and was aligned to the axis of the protein and located close to the α2 helix, which is very similar to the results obtained for shorter GAGs (Figure 6).
The trajectory obtained from RS-REMD simulation was analyzed using the MM-GBSA approach. According to our earlier studies [27], the most favorable values of electrostatic energy in vacuo obtained from an MM-GBSA analysis correspond to the most likely structures in protein–GAG complexes. Therefore, a refinement procedure was performed for the twenty structures with the lowest values of this binding free energy component. The starting structures and the structures obtained as a result of the refinement are presented in Figures S9–S28.
The 20 endostatin–HP dp24 complex structures were sorted and numbered from the most (1) to the least (20) electrostatically favorable. In 15 out of the 20 refined structures, the oligosaccharide adopted a binding pose, in which it was extended and parallel to the axis of the protein, with visible contacts between the HP dp24 and the N-terminal loop of the endostatin (Figures S9–S28), which is similar to the results from AD3. However, in complexes 2, 3, 4, 7 and 18, HP dp24 bound mainly to the central part of the protein. The MM-GBSA analysis identified complexes 2 and 17 as the most energetically favorable (Table 3). Although they represented essentially different structures, in both of them HP dp24 was in contact with the N-terminus of endostatin (Figure 7).
The analysis of the averaged decomposed binding energy of the endostatin amino acid residues presented in Table 4 did not suggest that the residues located within the α1 and α2 helices of the protein are mainly responsible for the stabilization of the complex structure with HP dp24, as they were in the case of the shorter HP/HS oligosaccharides. The Arg27 and Arg139 residues had the lowest ΔG values (−12.9 and −11.2 kcal/mol, respectively) (Table 4), which is consistent with previous reports [19]. Other favorable binding contributions were found mostly for Arg24, and two other residues previously predicted to contribute to HP binding [19], Arg53 and Arg63. The decomposition analysis was performed in detail for the two most energetically favorable complexes (Table S1). The amino acids Arg24, Arg27, Arg63 and Arg64, which positively contributed to the binding of HS, are located within the Walker A motif of endostatin (Figure 8), which consists of the RX(2-3)R sequence interacting with ATP [35]. This suggests that HS could modulate the ATPase activity of endostatin, in as much as HP has been reported to bind to ATPases [36,37].
For both best-scoring binding poses, an analysis of the hydrogen bonds formed between endostatin and HP dp24 atoms was performed using CAT software [38] (Tables S2 and S3). The largest population of hydrogen bonds involved the Arg27 and Arg53 amino acid residues. Additionally, for pose 1, the Arg63 residue was involved, while for pose 2 the Arg24 and Arg139 residues were the hydrogen bond donors with the largest population. These results are consistent with the results obtained by the per residue decomposition analysis. The high number of the multiple stabilizing hydrogen bonds between the protein and HP dp24, as well as the electrostatic contribution, suggest that there is rather low specificity in GAGs’ recognition by endostatin.
An analysis of the of HP dp24 ring puckering conformation of the best-scoring binding poses was also performed. The MD trajectories obtained in the refinement procedures were analyzed using CAT software [38]. Figure S29 shows the conformational changes occurring during MD for individual HP dp24 rings for the two best-scoring complexes. For both complexes, there was a 1C4 ring puckering conformation of IdoA(2S) clearly dominating for most of the rings during the entire MD simulation time, with the exception of the IdoA(2S) in the fifth position in the sequence, when counted from the non-reducing end, for which boat/twist conformations were also present. The dominance of the 1C4 ring puckering conformation was previously suggested by both experimental and theoretical studies [39]. The boat/twist conformation was more populated for the highest scoring complex, where it was observed for most of the analyzed frames. This may be explained by the fact that this oligosaccharide residue strongly interacts with Arg63, which potentially favors the boat/twist conformation.

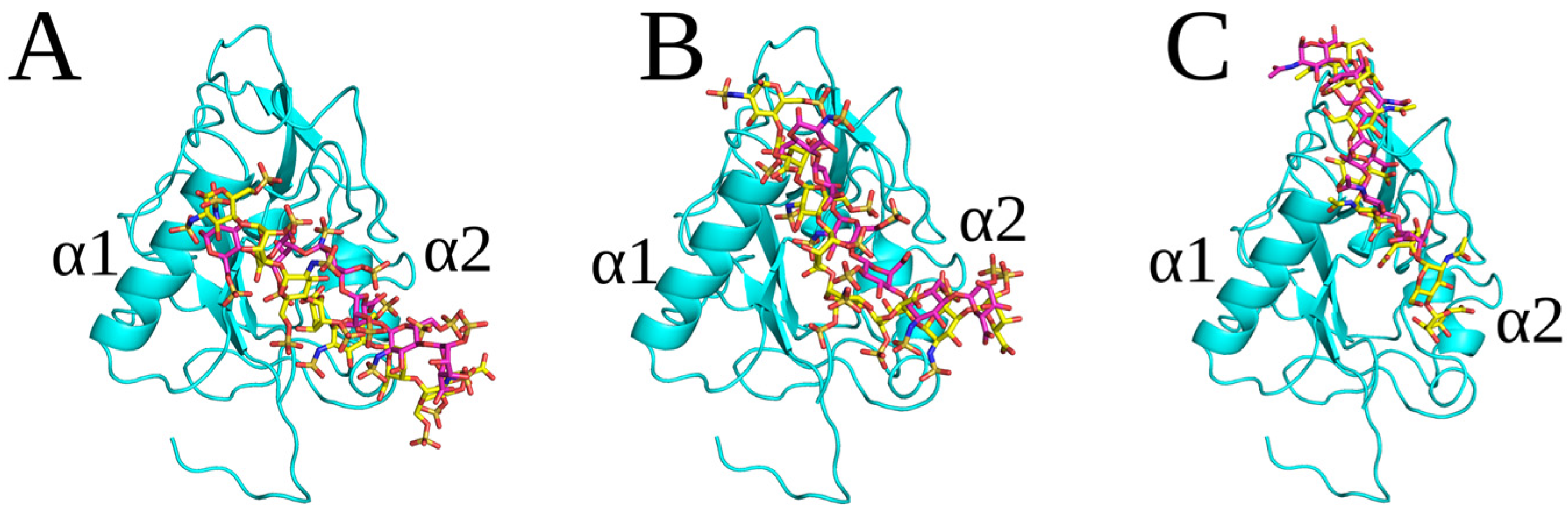
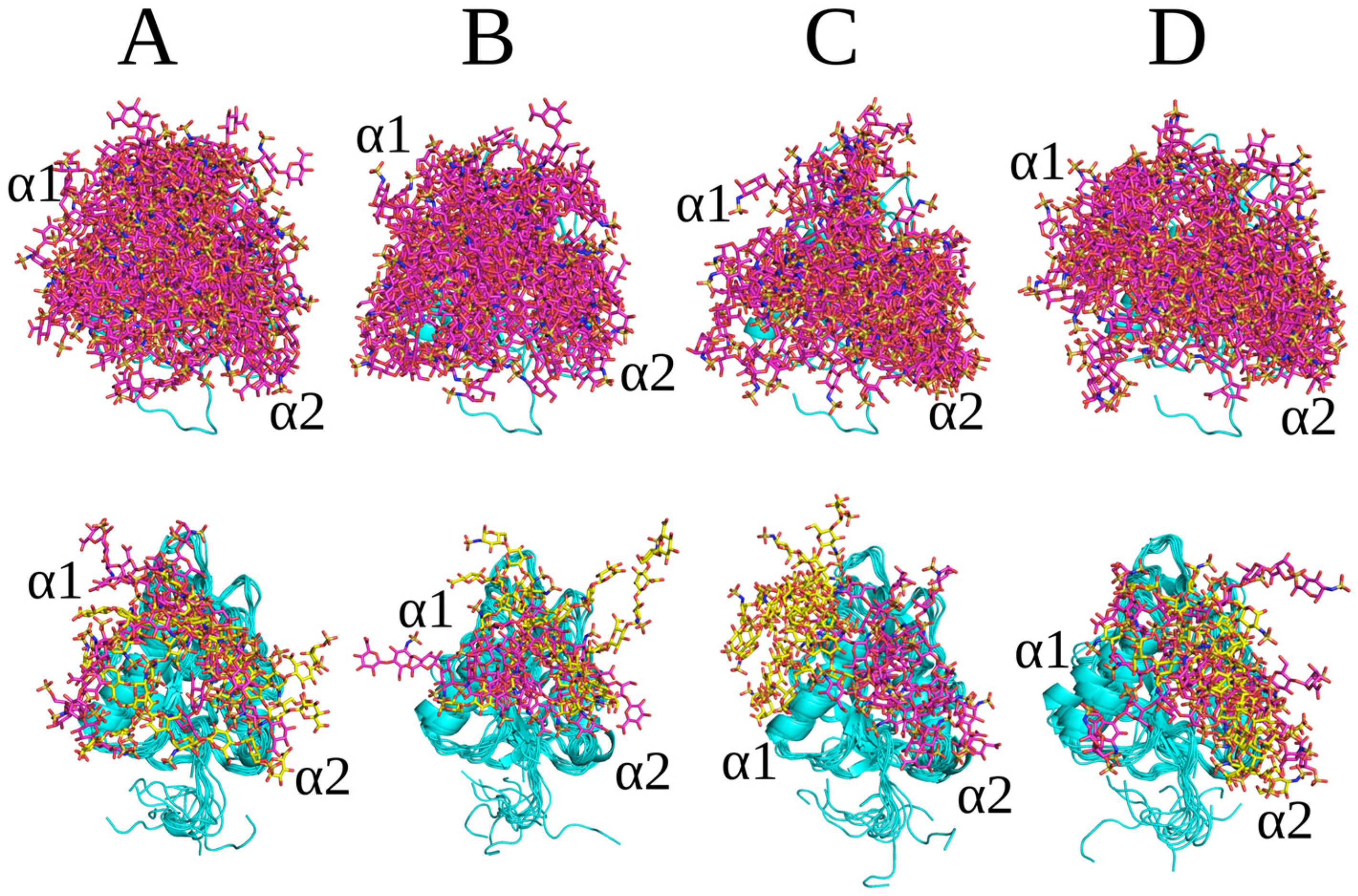
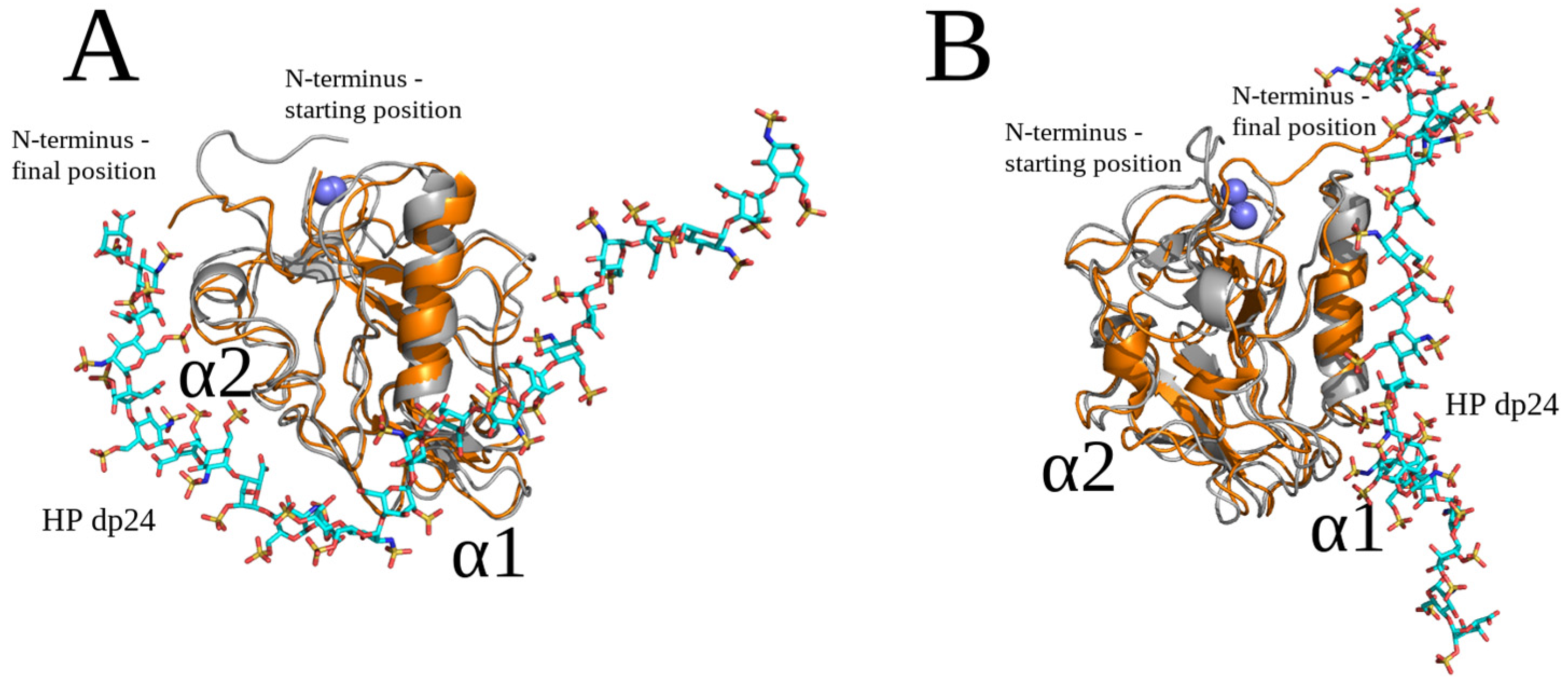
2.3. Unbiased MD Simulation Analysis

Unbiased MD simulations with different versions of the force field parameters in comparison to AMBER MD simulations were performed in NAMD in order to complement the findings obtained with AMBER. The protocol parameters were optimized for the unbiased MD simulations in NAMD independently of the ones in the AMBER MD simulations. The studied molecular system consists of endostatin and HP dp5 (Figure 9). The use of an HP probe of this particular length, different by a single saccharide unit from the dp4 and dp6 used in conventional docking, was supposed to provide even more heterogeneity in the conditions in the performed simulations to allow for more general and less approach-biased conclusions about the structural properties of the analyzed complexes. In total, 35 MD simulations were performed, starting from five different spatial positions (A-E) of HP dp5 (Figure 9A). All the obtained structures of the endostatin–HP dp5 complexes (Figure 9B) represented a combination of binding poses with a most commonly obtained one that was located near or between the α1 and α2 helix, which is in agreement with the results described in the previous sections (see Figure 1 and Figure 2 for the most representative cluster, cluster 1). Therefore, despite the structural heterogeneity of the obtained binding poses of HP oligosaccharides and the differences in the protocols used in the conventional docking and unbiased MD simulations, one common representative cluster of HP binding poses was calculated by both approaches.
Individual simulations covered timescale of 0.5 μs; however, some were extended to 1 μs (Figure 10). As can be seen from the accumulated atom–atom contact trajectory, the binding of HP dp5 typically occurred in less than 50 ns. The full-residue atom–atom contact trajectory provided a detailed view of the dynamics of the interaction of HP dp5 with endostatin during unbiased MD simulations. The binding modes sampled were quite diverse; however, certain binding motives did re-occur independently of the starting structure. Interactions occurred mainly between HP dp5 and the Arg24, Arg27, Arg53, Arg63 and Arg139 residues. Although the interactions obtained by different replicas of the MD were reproducible, the ligand still could have become stuck in ‘artificial sites’. It should be noted that the accumulated timescale (20 μs) sampled here exceeds the timescales typically sampled in MD simulations reported in the scientific literature.

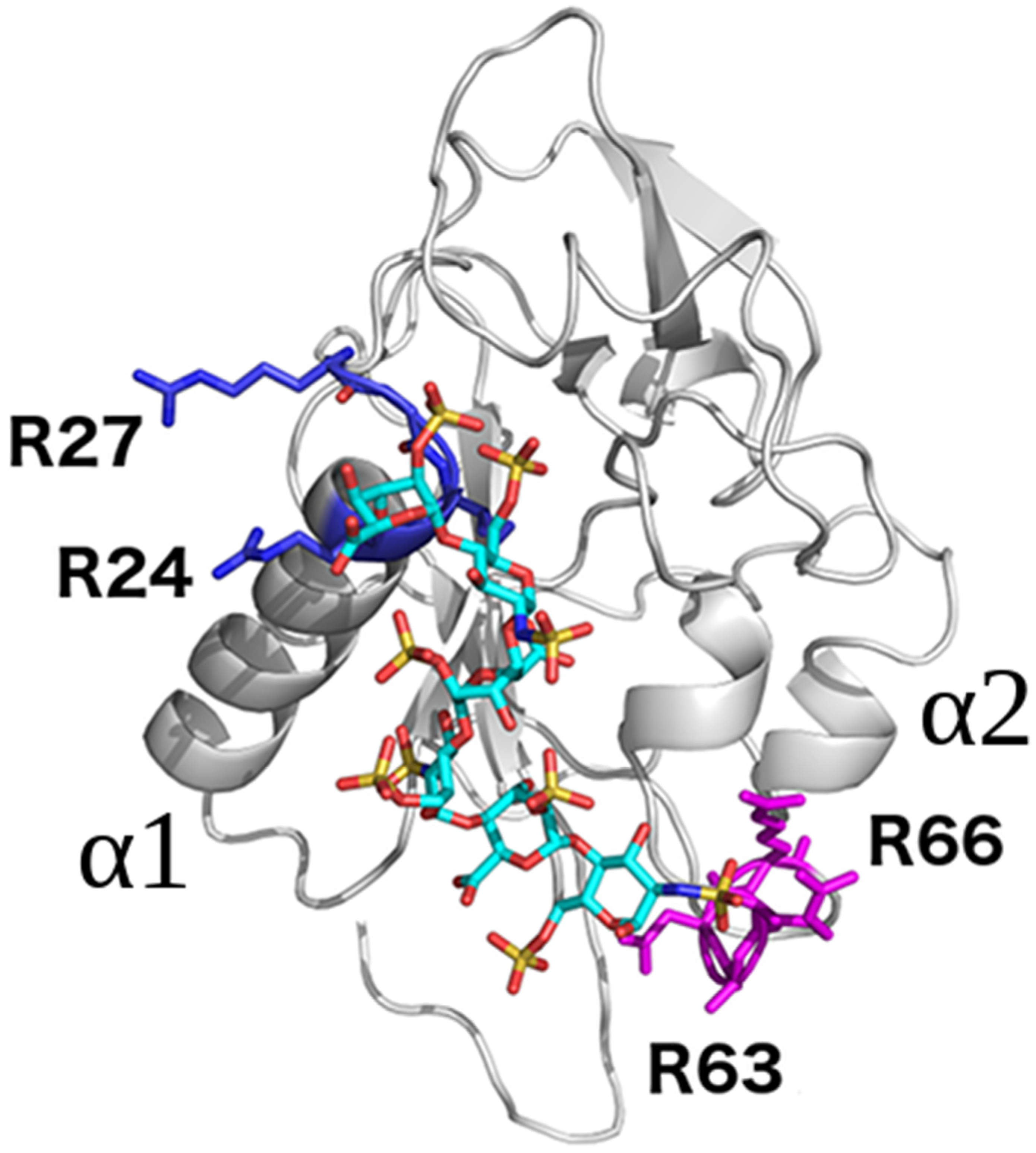
2.4. Endostatin Mutations

The aspartate residues of endostatin located close to the predicted GAG binding region unfavorably contributed to the binding of HP and HS oligosaccharides. Therefore, we performed single mutations of Asp30, Asp56 and Asp65; a double mutation of Asp30 and Asp56; and a triple mutation of Asp30, Asp56 and Asp65 to arginine to determine whether these mutations could improve the binding of endostatin to HP and affect the binding pose. The mutations significantly affected the MM-GBSA free energy, rendering it more favorable, from −53.8 to −64.1, −79.3 and −81.6 kcal/mol for the single D65R, D30R and D56R mutant, respectively (Table 5). For the double and triple mutants, the energy was −107.2 and −111.4 kcal/mol, respectively. Asp30 and Asp56 might thus contribute more unfavorably to the binding to HP and HS oligosaccharides than Asp65 in the wild-type protein.

3. Discussion

In this work, a systematic and rigorous computational analysis of endostatin–HP/HS complexes was performed to obtain novel insights into their structural and energetic properties depending on the GAG length, sulfation pattern and the presence of Zn2+. Several complementary computational approaches were applied to this system. For short GAGs (dp2-dp8), Arg24, Arg27, Arg62, Arg63, Arg66 and Arg139 are the residues which contribute the most to the stabilityof the complex. For a longer ligand, HP dp24, an RS-REMD simulation with an endostatin monomer as a receptor identified two representative binding poses with the most favorable binding energies. For the first pose, the most favorable amino acid residues are located close to the N-terminal loop (His1, Asp4) and the α2 helix (Arg62, Arg63 and Arg66). The His1 residue interacts with HP dp24 and this interaction potentially induces substantial conformational changes in the endostatin N-terminus. For the second pose, Arg residues from the α2 helix are mainly involved in the complex’s stabilization (Arg24, Arg27, Arg53, Arg63 and Arg139). Several of these residues belong to the Walker A motif and so potentially could compete with ATP for binding to this endostatin region, affecting its catalytic activity. This is in agreement with the fact that the plasma membrane Ca2+-ATPase is inhibited by HP [37]. Our results show that different HP/HS oligosaccharides are docked to similar binding sites on the endostatin surface independently of the docking method applied. GAG poses are mainly located in the region between the two α-helices (α1, α2), which is in agreement with the electrostatic potential calculations data obtained by the PBSA approach. The MM-GBSA analysis shows that the binding of desulfated HS dp2-dp8 is significantly less favorable than the binding of sulfated HP dp2-dp8, suggesting the key role of electrostatic interactions in this system. The influence of three endostatin residues unfavorably contributing to GAG binding was further analyzed. MD studies of endostatin mutants complexed with HP showed that the D30R and D56R mutations render the binding energy more favorable. We also performed a comprehensive analysis of HP and HS dp8 structures using an endostatin structure including a Zn2+ ion. The presence of Zn2+ essentially affected the binding free energies in the complexes, rendering them comparable to the ones seen in the absence of Zn2+ for HP dp8, but not for HS dp8, while for HS this yielded an additional docking pose located near the N-terminus of endostatin and Zn2+.
Our in silico approaches allowed us to improve our understanding of the molecular mechanisms underlying endostatin’s binding to HP and HS to generate models of several endostatin–HP/HS complexes and to identify the specific amino acid residues stabilizing these complexes. The results obtained using various theoretical and experimental techniques are consistent with each other and with previous experimental data [19,25].

4. Materials and Methods

4.1. Endostatin’s Structure

The crystal structure of human endostatin (PDB ID: 1BNL 2.9 Å) was used for the molecular docking and MD simulations. The amino acid residues of endostatin were numbered starting with the first residue of endostatin (His1572 in the full-length collagen XVIII α1 chain according to the UNIPROT numeration). Mutated structures were produced by the Chimera UCSF program [40].

4.2. Electrostatic Potential Calculations

Electrostatic potential isosurfaces were calculated using the PBSA program from AmberTools 20 [41], with a grid spacing of 1 Å.

4.3. Conventional Molecular Docking

The conventional docking of the flexible ligands HP/HS dp2, dp4, dp6 and dp8 to the endostatin receptor was performed with Autodock 3 (AD3) [42], with a grid spacing of 0.375 Å. The following ligands were used: 1. HP (disaccharide unit GlcNS(6S)-IdoA(2S) in both IdoA(2S) 1C4 and 2S0 ring puckering conformations); 2. desulfated HS (disaccharide unit GlcNAc-IdoA); 3. HS (disaccharide unit GlcNS(6S)-GlcA); 4. HS (disaccharide unit GlcNS-GlcA); 5. HS (disaccharide unit GlcNS-IdoA(2S) in both IdoA(2S) 1C4 and 2S0 ring puckering conformations). The 1C4 and 2S0 ring puckering conformations for all IdoA(2S) residues within the same molecule were defined by the dehidral angles (C1, C2, C3, C4) and (C1, O5, C5, C4), with their values corresponding to the X-ray structure of HP (PDB ID: 1HPN), where the HP molecule is available in both conformations [43]. For the desulfated HS, only IdoA 1C4 was considered since it is energetically a more favorable pucker conformation [39]. The Lamarckian genetic algorithm was used, with an initial population size of 300, 105 generations, 9995 × 105 energy evaluations and 103 independent runs. The fifty top docking solutions were clustered by the DBSCAN algorithm [44], with a neighborhood search radius of 3.0–3.2 Å and a minimal number of cluster members of three. The cluster numbering used throughout the manuscript corresponds to their size ranking, with cluster 1 to be the most populated, cluster 2 the second populated, etc. In the clustering procedure, the distance between two structures was defined as the root mean square of their atomic distances while pairing up the nearest atoms of the same type, which takes into account the fact that GAGs are made up of periodic units [45]. The docking studies of endostatin with Zn2+ were performed using the parameters available from the AMBER 16 software package [41].

4.4. Molecular Dynamics and MM-GBSA Calculations

MD simulations of endostatin in a complex with HP and HS oligosaccharides were carried out in AMBER 16 [41]. A complex was solvated in a truncated octahedron TIP3P water periodic box with a 10 Å distance from solute atoms in each direction to the box wall and neutralized by Na+ or Cl− counterions. The ff99SBonlysc [46] and GLYCAM06 force field [47] parameters were used for the endostatin and GAGs, respectively. MD simulations were preceded by two energy minimization steps: 1500 cycles of the steepest descent and 1000 cycles of a conjugate gradient with harmonic force restraints of 10 kcal/(mol·Å2) on solute atoms, and then 3000 cycles of the steepest descent and 3000 cycles of a conjugate gradient without restraints. The system was then heated from 0 to 300 K for 10 ps with harmonic force restraints of 10 kcal/(mol·Å2) on the solute atoms, and a 100 ps MD equilibration was run at 300 K and 105 Pa in NTP without restraints. A cut-off of 8 Å was applied to treat non-bonded interactions, and the Particle Mesh Ewald method was applied to treat long-range electrostatic interactions. Following the equilibration procedure, 25 ns of productive MD runs were performed within periodic boundary conditions in NTP. 25 ns is of a sufficient length for the MD simulation of stable protein–GAG complexes [11]. To furthermore increase the sampling, three random initial structures from each cluster were simulated, which resulted in 75 ns of simulations for each cluster. The results from each of three simulations were independently analyzed. The energetic post-processing of the trajectories and per residue energy decomposition were carried out using MM-GBSA with igb = 2 for the protein–GAG complexes for all the frames obtained from the productive MD run. We did not consider explicitly entropic contributions to the binding, since taking into account entropy would increase the overall uncertainty in the calculated binding energies [48,49]. Therefore, the calculated free energies should be understood as enthalpies, which also implicitly partially account for the entropic component of the solvent in the implicit model. These trajectories were analyzed using the VMD program for visualization [50].

4.5. Repulsive Scaling–Replica Exchange Molecular Dynamics (RS-REMD)

4.5.1. Molecular Dynamics Simulation

For molecular docking using the RS-REMD protocol introduced by Siebenmorgen et al., we applied protocols from [26] with minor modifications for protein–GAG systems [27]. The ff14SBonlysc force field parameters for endostatin [46] and the GLYCAM06 [47] for HP dp24 were used. The ligand was placed far from the N-terminus of the protein. The implicit solvent with the model igb = 8 implemented in the original approach of Siebenmorgen et al. [26] and an infinite cut-off for non-bonded interactions was used. There were 3000 steps of minimization, followed by heating to 300 K for 10 ns with a Langevin thermostat. The harmonic restraint of 0.05 kcal/(mol·Å2) was applied to all heavy atoms of the protein and Zn2+, as was the positional restraint of 1.0 kcal/(mol·Å2) between the center of mass (COM) of the receptor and the ligand in the production run. The 16 replicas were used, with different Lennard-Jones (LJ) parameters for atomic pairs of receptor and ligand molecules. Two parameters: d (adjusting the effective van der Waals radius, equivalent to the LJ sigma parameter) and e (changing the LJ potential well depth, equivalent to the LJ epsilon parameter) were assigned to 0.00 Å, 0.01 Å, 0.02 Å, 0.04 Å, 0.08 Å, 0.12 Å, 0.16 Å, 0.20 Å, 0.24 Å, 0.28 Å, 0.32 Å, 0.38 Å, 0.44 Å, 0.50 Å, 0.58 Å, 0.68 Å and 1.00, 0.99, 0.98, 0.97, 0.96, 0.94, 0.92, 0.90, 0.88, 0.86, 0.84, 0.82, 0.80, 0.78, 0.76, 0.74, respectively. The total duration of the production run was 60 ns per replica. The details on the protocols used by this particular approach, as well as an analysis of its prediction power for docking GAGs, are described in our previous studies [27,51,52], as well as in the original publication by Siebenmorgen et al. [26].

4.5.2. Binding Free Energy Calculations/Scoring

The binding free energy was calculated using the MM-GBSA method with the model igb = 2 [53] for all 16 replicas.

4.5.3. Refinement

The structures from the RS-REMD step were refined for the 20 frames with the lowest in vacuo electrostatic energy obtained from the MM-GBSA analysis. Unrestrained MD simulations 40 ns in length were performed as described above (see Molecular Dynamics and MM-GBSA Calculations Section).

4.5.4. MD Data Analysis

The RS-REMD and refinement trajectories were analyzed using the CPPTRAJ program in AMBER Tools 17 [41]. In addition, the Conformational Analysis Tools (CAT) program [38] was used for the hydrogen bond analysis, as well as for the rings’ puckering conformation analysis. The trajectories were visualized in the VMD 1.9.3 program [50], and PyMOL 1.2r3pre [54] was used for the production of figures.

4.6. Unbiased MD Simulation

Starting structures were built using the graphical interface of YASARA [55]. HP dp5 (GlcNS(6S)-IdoA(2S)-GlcNS(6S)-IdoA(2S)-GlcNS(6S) with IdoA(2S) in a 1C4 conformation) was positioned in the simulation box (63 Å × 63 Å × 63 Å) at a distance larger than 10 Å from the nearest protein atom. The molecular system was solvated in 0.9% NaCl solution (0.15 M) and simulations were performed at 310 K using periodic boundary conditions and the AMBER14 (ff14sb) force field [46]. GLYCAM06 [47] parameters were used for the GAGs. The box size was rescaled dynamically to maintain a water density of 0.996 g/mL. Simulations were performed using YASARA with GPU acceleration in “fast mode” (4 fs time step) [56] on “standard computing boxes” equipped with one 12-core i9 CPU and NVIDIA GeForce GTX 1080 Ti, reaching a sampling performance of about 300 ns/day. Multiple replicas were sampled, reaching accumulated timescales of about 20 μs. These simulations added up to more than 60 computing days. Snapshots were recorded every 25 ps. The stability of the complexes was monitored based on an atom–atom contact analysis (contact distance cut-off = 4 Å). CAT [38] was used for the analysis of trajectory data, general data processing and the generation of scientific plots. PyMOL [54] was used to generate molecular graphics.

Supplementary Materials

The following supporting information can be downloaded at https://www.mdpi.com/article/10.3390/molecules29174040/s1, Figure S1: Per-residue free energy decomposition of the last nanosecond of the productive MD run for HP 1C4 dp2 (A), dp4 (B), dp6 (C) and dp8 (D). In blue and red are the amino acid residues showing the most favorable and unfavorable effect on binding energy, respectively. Figure S2: deHP dp2, dp4, dp6 and dp8’s top 50 docking poses (endostatin—blue color, cartoon; HP—pink color, licorice) (A); structures of the complexes obtained from the last frame in MD simulations (endostatin—blue color, cartoon; HP: cluster 1—pink color, cluster 2—yellow color; licorice) (B). Figure S3: Per-residue free energy decomposition of the last nanosecond of the productive MD run for desulfated HS dp2 (A), dp4 (B), dp6 (C) and dp8 (D). In blue and red are the amino acid residues showing the most favorable and unfavorable effect on binding energy, respectively. Figure S4: superimposition of the conformations that scored best in terms of MM-GBSA for HP; 1C4 dp2 in cyan, HP 2S0 dp2 in magenta and desulfated HS dp2 in yellow. Figure S5: superimposition of the conformations that scored best in terms of MM-GBSA for HP 1C4 dp4 in green and desulfated HS dp4 in yellow. Figure S6: Per-residue free energy decomposition of the last nanosecond of the productive MD run for HS with the periodic units GlcNS(6S)-GlcA (A), GlcNS-GlcA (B), GlcNS-IdoA(2S) 1C4 (C) and GlcNS-IdoA(2S) 2S0 dp6 (D). In blue and red are amino acid residues showing the most favorable and unfavorable effect on binding energy, respectively. Figure S7: Upper panel—HS’s top 50 docking poses; lower panel—structures of the complexes obtained from the last frame in MD simulations with the repeating units (A) GlcNS(6S)-GlcA, (B) GlcNS-GlcA, (C) GlcNS-IdoA(2S) 1C4 and (D) GlcNS-IdoA(2S) 2S0 dp6 (in stick representation, magenta—cluster 1, yellow cluster 2, green cluster 3) with endostatin bound to Zn2+ (rendered as a dark blue sphere) in cartoon. Figure S8: Per-residue free energy decomposition of the last nanosecond of the productive MD run for the complex of endostatin bound to Zn2+ with HS dp8, with the repeating units GlcNS(6S)-GlcA (A), GlcNS-GlcA (B) GlcNS-IdoA(2S) 2S0 (C) and GlcNS-IdoA(2S) 1C4 (D). In blue and red are amino acid residues showing the most favorable and unfavorable effect on binding energy, respectively. Figure S9: Structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S10: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S11: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S12: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S13: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S14: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S15: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S16: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S17: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S18: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S19: Structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S20: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S21: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S22: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S23: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S24: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S25: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S26: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S27: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Figure S28: structure of endostatin–HP dp24 complexes after RS-REMD (left) and refinement procedure (right): proteins are presented in orange, cartoon representation; Zn2+ in violet, sphere representation; HP dp24 in cyan, licorice representation. Table S1: the per-residue decomposition analysis of the highest scoring models (pose 1 and pose 2) of the endostatin–HP dp24 complexes after the refinement procedure. Table S2: Hydrogen bond analysis of the highest scoring pose 1 of the endostatin–HP dp24 complex in the refinement procedure. Figure S29: ring puckering analysis of HP dp24 during the refinement of the highest scoring endostatin–HP dp24 pose 1 (A) and pose 2 complexes (B). Table S3: Hydrogen bond analysis for the highest score pose 2 of the endostatin-HP dp24 complex in the refinement procedure.

Author Contributions

Conceptualization, S.A.S.; methodology, S.A.S., M.F.; software, S.A.S., M.F.; validation, S.A.S., M.F.; formal analysis, U.U.-K., M.F., M.M.-Z.; investigation, U.U.-K., M.F., M.M.-Z.; resources, S.A.S., M.F.; data curation, U.U.-K., M.F., M.M.-Z.; writing—original draft preparation, U.U.-K., M.M.-Z.; writing—review and editing, S.A.S.; visualization, M.M.-Z., M.F.; supervision, S.A.S.; project administration, S.A.S.; funding acquisition, S.A.S. All authors have read and agreed to the published version of the manuscript.

Funding

This work was supported by the National Science Centre of Poland (Narodowy Centrum Nauki, grant UMO-2023/49/B/ST4/00041). The computational resources were provided by the Polish Grid Infrastructure (PL-GRID, grants plggagstr3, plggagstr3gpu), the Interdisciplinary Center of Mathematical and Computer Modeling (ICM; grant GA-71-23), ZIH at TU Dresden (grant p_gag) and our cluster at the Faculty of Chemistry, University of Gdansk.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data are contained within the article and Supplementary Materials.

Acknowledgments

We acknowledge Sylvie Ricard-Blum for her engagement into the revision of this manuscript and fruitful discussion of the obtained results.

Conflicts of Interest

Author Martin Frank was employed by the company Biognos AB. The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

  1. Varki, A.; Cummings, R.D.; Esko, J.D.; Stanley, P.; Hart, G.W.; Aebi, M.; Darvill, A.G.; Kinoshita, T.; Packer, N.H.; Prestegard, J.H.; et al. Essentials of Glycobiology, 3rd ed.; Cold Spring Harbor Laboratory Press: Cold Spring Harbor, NY, USA, 2015. [Google Scholar]
  2. Vallet, S.D.; Clerc, O.; Ricard-Blum, S. Glycosaminoglycan–protein interactions: The first draft of the glycosaminoglycan Interactome. J. Histochem. Cytochem. 2021, 69, 93–104. [Google Scholar] [CrossRef] [PubMed]
  3. Varki, A. Biological roles of glycans. Glycobiology 2017, 27, 3–49. [Google Scholar] [CrossRef] [PubMed]
  4. Vallet, S.D.; Berthollier, C.; Ricard-Blum, S. The glycosaminoglycan interactome 2.0. Am. J. Physiol.-Cell Physiol. 2022, 322, C1271–C1278. [Google Scholar] [CrossRef] [PubMed]
  5. Ricard-Blum, S.; Perez, S. Glycosaminoglycan interaction networks and databases. Curr. Opin. Struct. Biol. 2022, 74, 102355. [Google Scholar] [CrossRef]
  6. Paiardi, G.; Milanesi, M.; Wade, R.C.; D’Ursi, P.; Rusnati, M. A bittersweet computational journey among glycosaminoglycans. Biomolecules 2021, 11, 739. [Google Scholar] [CrossRef]
  7. Bojarski, K.K.; Sage, J.; Lalmanach, G.; Lecaille, F.; Samsonov, S.A. In silico and in vitro mapping of specificity patterns of glycosaminoglycans towards cysteine cathepsins B, L, K, S and V. J. Mol. Graph. Model. 2022, 113, 108153. [Google Scholar] [CrossRef]
  8. Pérez, S.; Bonnardel, F.; Lisacek, F.; Imberty, A.; Ricard Blum, S.; Makshakova, O. GAG-DB, the new interface of the three-dimensional landscape of glycosaminoglycans. Biomolecules 2020, 10, 1660. [Google Scholar] [CrossRef]
  9. Clerc, O.; Mariethoz, J.; Rivet, A.; Lisacek, F.; Pérez, S.; Ricard-Blum, S. A pipeline to translate glycosaminoglycan sequences into 3D models. Application to the exploration of glycosaminoglycan conformational space. Glycobiology 2019, 29, 36–44. [Google Scholar] [CrossRef]
  10. Griffith, A.R.; Rogers, C.J.; Miller, G.M.; Abrol, R.; Hsieh-Wilson, L.C.; Goddard, W.A., III. Predicting glycosaminoglycan surface protein interactions and implications for studying axonal growth. Proc. Natl. Acad. Sci. USA 2017, 114, 13697–13702. [Google Scholar] [CrossRef]
  11. Samsonov, S.A.; Pisabarro, M.T. Computational analysis of interactions in structurally available protein–glycosaminoglycan complexes. Glycobiology 2016, 26, 850–861. [Google Scholar] [CrossRef]
  12. Künze, G.; Huster, D.; Samsonov, S.A. Investigation of the structure of regulatory proteins interacting with glycosaminoglycans by combining NMR spectroscopy and molecular modeling–the beginning of a wonderful friendship. Biol. Chem. 2021, 402, 1337–1355. [Google Scholar] [CrossRef]
  13. Hintze, V.; Samsonov, S.A.; Anselmi, M.; Moeller, S.; Becher, J.; Schnabelrauch, M.; Scharnweber, D.; Pisabarro, M.T. Sulfated glycosaminoglycans exploit the conformational plasticity of bone morphogenetic protein-2 (BMP-2) and alter the interaction profile with its receptor. Biomacromolecules 2014, 15, 3083–3092. [Google Scholar] [CrossRef] [PubMed]
  14. Hofmann, T.; Samsonov, S.A.; Pichert, A.; Lemmnitzer, K.; Schiller, J.; Huster, D.; Pisabarro, M.T.; von Bergen, M.; Kalkhof, S. Structural analysis of the interleukin-8/glycosaminoglycan interactions by amide hydrogen/deuterium exchange mass spectrometry. Methods 2015, 89, 45–53. [Google Scholar] [CrossRef] [PubMed]
  15. Perez, S.; Makshakova, O.; Angulo, J.; Bedini, E.; Bisio, A.; de Paz, J.L.; Fadda, E.; Guerrini, M.; Hricovini, M.; Hricovini, M.; et al. Glycosaminoglycans: What remains to be deciphered? JACS Au 2023, 3, 628–656. [Google Scholar] [CrossRef]
  16. Poluzzi, C.; Iozzo, R.V.; Schaefer, L. Endostatin and endorepellin: A common route of action for similar angiostatic cancer avengers. Adv. Drug Deliv. Rev. 2016, 97, 156–173. [Google Scholar] [CrossRef]
  17. Ricard-Blum, S.; Vallet, S.D. Matricryptins network with matricellular receptors at the surface of endothelial and tumor cells. Front. Pharmacol. 2016, 7, 11. [Google Scholar] [CrossRef]
  18. Li, K.; Shi, M.; Qin, S. Current status and study progress of recombinant human endostatin in cancer treatment. Oncol. Ther. 2018, 6, 21–43. [Google Scholar] [CrossRef] [PubMed]
  19. Ricard-Blum, S.; Féraud, O.; Lortat-Jacob, H.; Rencurosi, A.; Fukai, N.; Dkhissi, F.; Vittet, D.; Imberty, A.; Olsen, B.R.; van Der Rest, M. Characterization of endostatin binding to heparin and heparan sulfate by surface plasmon resonance and molecular modeling: Role of divalent cations. J. Biol. Chem. 2004, 279, 2927–2936. [Google Scholar] [CrossRef]
  20. Boehm, T.; O’Reilly, M.S.; Keough, K.; Shiloach, J.; Shapiro, R.; Folkman, J. Zinc-binding of endostatin is essential for its antiangiogenic activity. Biochem. Biophys. Res. Commun. 1998, 252, 190–194. [Google Scholar] [CrossRef]
  21. Yamaguchi, N.; Anand-Apte, B.; Lee, M.; Sasaki, T.; Fukai, N.; Shapiro, R.; Que, I.; Lowik, C.; Timpl, R.; Olsen, B.R. Endostatin inhibits VEGF-induced endothelial cell migration and tumor growth independently of zinc binding. EMBO J. 1999, 18, 4414–4423. [Google Scholar] [CrossRef]
  22. Woodhead, N.E.; Long, W.F.; Williamson, F.B. Binding of zinc ions to heparin. Analysis by equilibrium dialysis suggests the occurrence of two, entropy-driven, processes. Biochem. J. 1986, 237, 281. [Google Scholar] [CrossRef]
  23. Ding, Y.H.; Javaherian, K.; Lo, K.M.; Chopra, R.; Boehm, T.; Lanciotti, J.; Harris, B.A.; Li, Y.; Shapiro, R.; Hohenester, E.; et al. Zinc-dependent dimers observed in crystals of human endostatin. Proc. Natl. Acad. Sci. USA 1998, 95, 10443–10448. [Google Scholar] [CrossRef] [PubMed]
  24. Hohenester, E.; Sasaki, T.; Mann, K.; Timpl, R. Variable zinc coordination in endostatin. J. Mol. Biol. 2000, 297, 1–6. [Google Scholar] [CrossRef]
  25. Sasaki, T.; Larsson, H.; Kreuger, J.; Salmivirta, M.; Claesson-Welsh, L.; Lindahl, U.; Hohenester, E.; Timpl, R. Structural basis and potential role of heparin/heparan sulfate binding to the angiogenesis inhibitor endostatin. EMBO J. 1999, 18, 6240–6248. [Google Scholar] [CrossRef]
  26. Siebenmorgen, T.; Engelhard, M.; Zacharias, M. Prediction of protein–protein complexes using replica exchange with repulsive scaling. J. Comput. Chem. 2020, 41, 1436–1447. [Google Scholar] [CrossRef] [PubMed]
  27. Maszota-Zieleniak, M.; Marcisz, M.; Kogut, M.M.; Siebenmorgen, T.; Zacharias, M.; Samsonov, S.A. Evaluation of replica exchange with repulsive scaling approach for docking glycosaminoglycans. J. Comput. Chem. 2021, 42, 1040–1053. [Google Scholar] [CrossRef]
  28. Marcisz, M.; Zacharias, M.; Samsonov, S.A. Modeling protein–glycosaminoglycan complexes: Does the size matter? J. Chem. Inf. Model. 2021, 61, 4475–4485. [Google Scholar] [CrossRef] [PubMed]
  29. Samsonov, S.A.; Pisabarro, M.T. Importance of IdoA and IdoA (2S) ring conformations in computational studies of glycosaminoglycan–protein interactions. Carbohydr. Res. 2013, 381, 133–137. [Google Scholar] [CrossRef] [PubMed]
  30. Weiss, T.; Ricard-Blum, S.; Moschcovich, L.; Wineman, E.; Mesilaty, S.; Kessler, E. Binding of procollagen C-proteinase enhancer-1 (PCPE-1) to heparin/heparan sulfate: Properties and role in PCPE-1 interaction with cells. J. Biol. Chem. 2010, 285, 33867–33874. [Google Scholar] [CrossRef]
  31. Penk, A.; Baumann, L.; Huster, D.; Samsonov, S.A. NMR and molecular modeling reveal specificity of the interactions between CXCL14 and glycosaminoglycans. Glycobiology 2019, 29, 715–725. [Google Scholar] [CrossRef]
  32. Bojarski, K.K.; Samsonov, S.A. Role of Oligosaccharide Chain Polarity in Protein–Glycosaminoglycan Interactions. J. Chem. Inf. Model. 2020, 61, 455–466. [Google Scholar] [CrossRef] [PubMed]
  33. Han, Q.; Fu, Y.; Zhou, H.; He, Y.; Luo, Y. Contributions of Zn (II)-binding to the structural stability of endostatin. FEBS Lett. 2007, 581, 3027–3032. [Google Scholar] [CrossRef] [PubMed]
  34. Marcisz, M.; Maszota-Zieleniak, M.; Huard, B.; Samsonov, S.A. Advanced molecular dynamics approaches to model a tertiary complex APRIL/TACI with long glycosaminoglycans. Biomolecules 2021, 11, 1349. [Google Scholar] [CrossRef]
  35. Wang, N.; Silver, D.L.; Thiele, C.; Tall, A.R. ATP-binding cassette transporter A1 (ABCA1) functions as a cholesterol efflux regulatory protein. J. Biol. Chem. 2001, 276, 23742–23747. [Google Scholar] [CrossRef]
  36. Tersariol, I.L.; Dietrich, C.P.; Nader, H.B. Interaction of heparin with myosin ATPase: Possible involvement with the hemorrhagic activity and a correlation with antithrombin III high affinity-heparin molecules. Thromb. Res. 1992, 68, 247–258. [Google Scholar] [CrossRef]
  37. Felix, C.F.; Oliveira, V.H.; Moreira, O.C.; Mignaco, J.A.; Barrabin, H.; Scofano, H.M. Inhibition of plasma membrane Ca2+-ATPase by heparin is modulated by potassium. Int. J. Biochem. Cell Biol. 2007, 39, 586–596. [Google Scholar] [CrossRef] [PubMed]
  38. Conformational Analysis Tools. Available online: http://www.md-simulations.de/CAT/index.html (accessed on 10 January 2024).
  39. Sattelle, B.M.; Hansen, S.U.; Gardiner, J.; Almond, A. Free energy landscapes of iduronic acid and related monosaccharides. JACS 2010, 132, 13132–13134. [Google Scholar] [CrossRef]
  40. Pettersen, E.F.; Goddard, T.D.; Huang, C.C.; Couch, G.S.; Greenblatt, D.M.; Meng, E.C.; Ferrin, T.E. UCSF Chimera—A visualization system for exploratory research and analysis. J. Comput. Chem. 2004, 25, 1605–1612. [Google Scholar] [CrossRef]
  41. Case, D.A.; Ben-Shalom, I.Y.; Brozell, S.R.; Cerutti, D.S.; Cheatham, T.E., III; Cruzeiro, V.W.D.; Darden, T.A.; Duke, R.E.; Ghoreishi, D.; Gilson, M.K.; et al. AMBER16; University of California: San Francisco, CA, USA, 2018. [Google Scholar]
  42. Morris, G.M.; Goodsell, D.S.; Halliday, R.S.; Huey, R.; Hart, W.E.; Belew, R.K.; Olson, A.J. Automated docking using a Lamarckian genetic algorithm and an empirical binding free energy function. J. Comput. Chem. 1998, 19, 1639–1662. [Google Scholar] [CrossRef]
  43. Mulloy, B.; Forster, M.J.; Jones, C.; Davies, D.B. N.m.r. and molecular-modelling studies of the solution conformation of heparin. Biochem. J. 1993, 293, 849–858. [Google Scholar] [CrossRef]
  44. Ester, M.; Kriegel, H.P.; Sander, J.; Xu, X. Proceedings of the Second International Conference on Knowledge Discovery and Data Mining (KDD-96). kdd 1996, 96, 226–231. [Google Scholar]
  45. Samsonov, S.A.; Gehrcke, J.P.; Pisabarro, M.T. Flexibility and explicit solvent in molecular-dynamics-based docking of protein–glycosaminoglycan systems. J. Chem. Inf. Model. 2014, 54, 582–592. [Google Scholar] [CrossRef] [PubMed]
  46. Maier, J.A.; Martinez, C.; Kasavajhala, K.; Wickstrom, L.; Hauser, K.E.; Simmerling, C. ff14SB: Improving the accuracy of protein side chain and backbone parameters from ff99SB. Journal of chemical theory and computation 2015, 11, 3696–3713. [Google Scholar] [CrossRef]
  47. Kirschner, K.N.; Yongye, A.B.; Tschampel, S.M.; González-Outeiriño, J.; Daniels, C.R.; Foley, B.L.; Woods, R.J. GLYCAM06: A generalizable biomolecular force field. Carbohydrates. J. Comput. Chem. 2008, 29, 622–655. [Google Scholar] [CrossRef] [PubMed]
  48. Homeyer, N.; Gohlke, H. Free energy calculations by the molecular mechanics Poisson− Boltzmann surface area method. Mol. Inform. 2012, 31, 114–122. [Google Scholar] [CrossRef]
  49. Gandhi, N.S.; Mancera, R.L. Free energy calculations of glycosaminoglycan–protein interactions. Glycobiology 2009, 19, 1103–1115. [Google Scholar] [CrossRef] [PubMed]
  50. Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual molecular dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]
  51. Marcisz, M.; Gaardløs, M.; Bojarski, K.K.; Siebenmorgen, T.; Zacharias, M.; Samsonov, S.A. Explicit Solvent Repulsive Scaling Replica Exchange Molecular Dynamics (RS-REMD) in Molecular Modeling of Protein-Glycosaminoglycan Complexes. J. Comp. Chem. 2022, 43, 1633–1640. [Google Scholar] [CrossRef]
  52. Marcisz, M.; MAszota-Zieleniak, M.; Samsonov, S.A. Repulsive Scaling Replica Exchange Molecular Dynamics in Modeling Protein-Glycosaminoglycan Complexes. Proteoglycans Methods Protoc. Methods Mol. Biol. 2023, 2619, 153–167. [Google Scholar]
  53. Onufriev, A.; Case, D.A.; Bashford, D. Effective Born radii in the generalized Born approximation: The importance of being perfect. J. Comput. Chem. 2002, 23, 1297–1304. [Google Scholar] [CrossRef]
  54. The PyMOL Molecular Graphics System, Version 1.2r3pre; Schrödinger, LLC: New York, NY, USA, 2010.
  55. Krieger, E.; Vriend, G. YASARA View—Molecular graphics for all devices—From smartphones to workstations. Bioinformatics 2014, 30, 2981–2982. [Google Scholar] [CrossRef] [PubMed]
  56. Krieger, E.; Vriend, G. New ways to boost molecular dynamics simulations. J. Comput. Chem. 2015, 36, 996–1007. [Google Scholar] [CrossRef] [PubMed]
Molecules 29 04040 g001
Figure 1. (A) Endostatin electrostatic potential isosurfaces (blue, 2 kcal mol−1 e−1; red, −2 kcal mol−1 e−1) (B) HP dp2, dp4, dp6 and dp8’s top 50 docking poses (endostatin—blue color, cartoon; HP—pink color, licorice); (C) structures of the complexes obtained from the last frame of MD simulations (endostatin—blue color, cartoon; HP: cluster 1—pink color, cluster 2—yellow color; licorice).
Figure 1. (A) Endostatin electrostatic potential isosurfaces (blue, 2 kcal mol−1 e−1; red, −2 kcal mol−1 e−1) (B) HP dp2, dp4, dp6 and dp8’s top 50 docking poses (endostatin—blue color, cartoon; HP—pink color, licorice); (C) structures of the complexes obtained from the last frame of MD simulations (endostatin—blue color, cartoon; HP: cluster 1—pink color, cluster 2—yellow color; licorice).
Molecules 29 04040 g001
Molecules 29 04040 g002
Figure 2. (A) HP 2S0 dp2, dp4, dp6 and dp8’s top 50 docking poses (endostatin—blue color, cartoon; HP—pink color, licorice) and (B) the structures of the complexes obtained from the last frame of MD simulations (endostatin—blue color, cartoon; HP: cluster 1—pink color, cluster 2—yellow color, licorice).
Figure 2. (A) HP 2S0 dp2, dp4, dp6 and dp8’s top 50 docking poses (endostatin—blue color, cartoon; HP—pink color, licorice) and (B) the structures of the complexes obtained from the last frame of MD simulations (endostatin—blue color, cartoon; HP: cluster 1—pink color, cluster 2—yellow color, licorice).
Molecules 29 04040 g002
Molecules 29 04040 g003
Figure 3. Superimposition of endostatin on the most energetically favorable complex with HP and deHS dp6 and dp8: (A) HP 1C4 dp6 (magenta), dp8 (yellow); (B) HP 2S0 dp6 (magenta), dp8 (yellow); and (C) desulfated HS dp6 (magenta), dp8 (yellow).
Figure 3. Superimposition of endostatin on the most energetically favorable complex with HP and deHS dp6 and dp8: (A) HP 1C4 dp6 (magenta), dp8 (yellow); (B) HP 2S0 dp6 (magenta), dp8 (yellow); and (C) desulfated HS dp6 (magenta), dp8 (yellow).
Molecules 29 04040 g003
Molecules 29 04040 g004
Figure 4. Upper panel: HS’s top 50 docking poses; lower panel: structures of the complexes obtained from the last frame of MD simulations with the repeating units (A) GlcNS(6S)-GlcA, (B) GlcNS-GlcA, (C) GlcNS-IdoA(2S) 1C4 and (D) GlcNS-IdoA(2S) 2S0 dp8 (in licorice representation, magenta—cluster 1, yellow cluster 2), with endostatin in its cartoon representation.
Figure 4. Upper panel: HS’s top 50 docking poses; lower panel: structures of the complexes obtained from the last frame of MD simulations with the repeating units (A) GlcNS(6S)-GlcA, (B) GlcNS-GlcA, (C) GlcNS-IdoA(2S) 1C4 and (D) GlcNS-IdoA(2S) 2S0 dp8 (in licorice representation, magenta—cluster 1, yellow cluster 2), with endostatin in its cartoon representation.
Molecules 29 04040 g004
Molecules 29 04040 g005
Figure 5. Structures of the complexes obtained from the last frame of MD simulations of endostatin–HP dp8 complexes without (A) and with Zn2+ (B); the protein is depicted as a cyan cartoon; Zn2+ is a violet sphere; and HP is presented using a licorice representation (magenta—cluster 1, yellow cluster 2).
Figure 5. Structures of the complexes obtained from the last frame of MD simulations of endostatin–HP dp8 complexes without (A) and with Zn2+ (B); the protein is depicted as a cyan cartoon; Zn2+ is a violet sphere; and HP is presented using a licorice representation (magenta—cluster 1, yellow cluster 2).
Molecules 29 04040 g005
Molecules 29 04040 g006
Figure 6. Structure of the endostatin–HP dp24 complex obtained by the RS-REMD approach (endostatin: orange cartoon, HP dp24: licorice; starting position cyan, final position green). (A,B) correspond to the final frame of the RS-REMD simulation and the final frame of the following conventional MD refinement, respectively.
Figure 6. Structure of the endostatin–HP dp24 complex obtained by the RS-REMD approach (endostatin: orange cartoon, HP dp24: licorice; starting position cyan, final position green). (A,B) correspond to the final frame of the RS-REMD simulation and the final frame of the following conventional MD refinement, respectively.
Molecules 29 04040 g006
Molecules 29 04040 g007
Figure 7. Structures of the endostatin–HP dp24 complexes after the refinement procedure for the highest-scoring pose 1 (A) and pose 2 (B); proteins are in white (starting position) and orange (final position) cartoon; Zn2+ is a violet sphere; and HP dp24 is in cyan licorice.
Figure 7. Structures of the endostatin–HP dp24 complexes after the refinement procedure for the highest-scoring pose 1 (A) and pose 2 (B); proteins are in white (starting position) and orange (final position) cartoon; Zn2+ is a violet sphere; and HP dp24 is in cyan licorice.
Molecules 29 04040 g007
Molecules 29 04040 g008
Figure 8. Endostatin in complex with docked HP dp8 in ribbon and cyan stick representations, respectively. The amino acids that belong to the Walker A motif RX(2-3)R and interact with the adenine in ATP are colored in blue and magenta, respectively.
Figure 8. Endostatin in complex with docked HP dp8 in ribbon and cyan stick representations, respectively. The amino acids that belong to the Walker A motif RX(2-3)R and interact with the adenine in ATP are colored in blue and magenta, respectively.
Molecules 29 04040 g008
Molecules 29 04040 g009
Figure 9. (A) Five starting poses of HP dp5 (endostatin—blue color, cartoon; HP—pose A pink, pose B yellow, pose C green, pose D orange and pose E purple color, licorice; Zn2+—blue, sphere). (B) Structures of the complexes obtained from the last frame of unbiased MD simulations (endostatin—blue color, cartoon; HP—pose A pink, pose B yellow, pose C green, pose D orange and pose E purple color, licorice; Zn2+—blue, sphere).
Figure 9. (A) Five starting poses of HP dp5 (endostatin—blue color, cartoon; HP—pose A pink, pose B yellow, pose C green, pose D orange and pose E purple color, licorice; Zn2+—blue, sphere). (B) Structures of the complexes obtained from the last frame of unbiased MD simulations (endostatin—blue color, cartoon; HP—pose A pink, pose B yellow, pose C green, pose D orange and pose E purple color, licorice; Zn2+—blue, sphere).
Molecules 29 04040 g009
Molecules 29 04040 g010
Figure 10. Accumulated trajectory (35 MD simulations, separated by vertical lines) of the number of atom–atom contacts between HP dp5 and endostatin residues. Only amino acids that are in contact at least 1% of the simulation time are shown. Arg and Lys residues are highlighted in blue and cyan, respectively.
Figure 10. Accumulated trajectory (35 MD simulations, separated by vertical lines) of the number of atom–atom contacts between HP dp5 and endostatin residues. Only amino acids that are in contact at least 1% of the simulation time are shown. Arg and Lys residues are highlighted in blue and cyan, respectively.
Molecules 29 04040 g010
Table 1. MM-GBSA binding free energies of endostatin complexes with HP and HS oligosaccharides, obtained for the whole MD trajectory.
Table 1. MM-GBSA binding free energies of endostatin complexes with HP and HS oligosaccharides, obtained for the whole MD trajectory.
ΔG [kcal/mol]GAG
−30.8 ± 8.5HP (GlcNS(6S)-IdoA(2S)) dp2, 1C4
−45.6 ± 7.7HP (GlcNS(6S)-IdoA(2S)) dp4, 1C4
−50.3 ± 11.0HP (GlcNS(6S)-IdoA(2S)) dp6, 1C4
−53.8 ± 17.9HP (GlcNS(6S)-IdoA(2S)) dp8, 1C4
−33.6 ± 7.2HP (GlcNS(6S)-IdoA(2S)) dp2, 2S0
−31.1 ± 5.7HP (GlcNS(6S)-IdoA(2S)) dp4, 2S0
−68.5 ± 14.4HP (GlcNS(6S)-IdoA(2S)) dp6, 2S0
−70.5 ± 14.9HP (GlcNS(6S)-IdoA(2S)) dp8, 2S0
−18.8 ± 4.2desulfated HS (GlcNAc-IdoA) dp2
−25.7 ± 4.2desulfated HS (GlcNAc-IdoA) dp4
−43.4 ± 1.8desulfated HS (GlcNAc-IdoA) dp6
−48 ± 13.6desulfated HS (GlcNAc-IdoA) dp8
−48.2 ± 8.8HS (GlcNS(6S)-GlcA) dp6
−47.7 ± 10.7HS (GlcNS-GlcA) dp6
−48.0 ± 12.1HS (GlcNS-IdoA(2S)) dp6, 1C4
−56.9 ± 11.1HS (GlcNS-IdoA(2S)) dp6, 2S0
Table 2. MM-GBSA binding free energies analysis of endostatin complexes with HS dp8 in the presence of Zn2+.
Table 2. MM-GBSA binding free energies analysis of endostatin complexes with HS dp8 in the presence of Zn2+.
ΔG (kcal/mol)GAG
−62.5 ± 14.5HP (GlcNS(6S)-IdoA(2S))4 1C4
−51.8 ± 22.1(GlcNS(6S)-GlcA)4
−42.9 ± 13.3(GlcNS-GlcA)4
−49.2 ± 13.6(GlcNS-IdoA(2S))4 2S0
−51.9 ± 13.4(GlcNS-IdoA(2S))4 1C4
Table 3. MM-GBSA binding free energies of the 20 most favorable endostatin–HP dp24 complexes obtained by the RS-REMD approach after the refinement procedure.
Table 3. MM-GBSA binding free energies of the 20 most favorable endostatin–HP dp24 complexes obtained by the RS-REMD approach after the refinement procedure.
ΔG (kcal/mol)Complex (n°)
−91.01
−106.72
−65.73
−71.44
−80.65
−87.36
−92.07
−88.98
−56.49
−79.310
−101.111
−94.112
−78.813
−67.414
−99.815
−52.916
−105.117
−84.018
−65.719
−79.520
Table 4. Per-residue decomposition analysis of the 20 most favorable endostatin–HP dp24 complexes obtained by the RS-REMD approach after the refinement procedure. The ΔG values are average values for individual amino acid residues from all 20 analyzed complex structures.
Table 4. Per-residue decomposition analysis of the 20 most favorable endostatin–HP dp24 complexes obtained by the RS-REMD approach after the refinement procedure. The ΔG values are average values for individual amino acid residues from all 20 analyzed complex structures.
ΔG [kcal/mol]Amino Acid Residue
−3.9His1
−4.6Arg4
−8.0Arg24
−12.9Arg27
−5.9Arg38
−5.6Arg47
−10.8Arg53
−5.8Arg62
−7.8Arg63
−4.6Arg66
−2.8Lys75
−2.3Arg99
−2.9Lys106
−2.9Arg110
−2.4Lys117
−3.9Arg128
−4.5Arg129
−11.2Arg139
−2.4Arg156
Table 5. MM-GBSA binding free energies of endostatin mutant complexes with HP dp8.
Table 5. MM-GBSA binding free energies of endostatin mutant complexes with HP dp8.
ΔG [kcal/mol]Mutant
−79.3 ± 14.5D30R
−81.6 ± 15.5D56R
−64.1 ± 10.3D65R
−107.2 ± 12.7D30R, D56R
−111.4 ± 19.8D30R, D56R, D65R
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Uciechowska-Kaczmarzyk, U.; Frank, M.; Samsonov, S.A.; Maszota-Zieleniak, M. Structural Insights into Endostatin–Heparan Sulfate Interactions Using Modeling Approaches. Molecules 2024, 29, 4040. https://doi.org/10.3390/molecules29174040

AMA Style

Uciechowska-Kaczmarzyk U, Frank M, Samsonov SA, Maszota-Zieleniak M. Structural Insights into Endostatin–Heparan Sulfate Interactions Using Modeling Approaches. Molecules. 2024; 29(17):4040. https://doi.org/10.3390/molecules29174040

Chicago/Turabian Style

Uciechowska-Kaczmarzyk, Urszula, Martin Frank, Sergey A. Samsonov, and Martyna Maszota-Zieleniak. 2024. "Structural Insights into Endostatin–Heparan Sulfate Interactions Using Modeling Approaches" Molecules 29, no. 17: 4040. https://doi.org/10.3390/molecules29174040

APA Style

Uciechowska-Kaczmarzyk, U., Frank, M., Samsonov, S. A., & Maszota-Zieleniak, M. (2024). Structural Insights into Endostatin–Heparan Sulfate Interactions Using Modeling Approaches. Molecules, 29(17), 4040. https://doi.org/10.3390/molecules29174040

Article Metrics

Back to TopTop