Nanostructured Molecular–Network Arsenoselenides from the Border of a Glass-Forming Region: A Disproportionality Analysis Using Complementary Characterization Probes
Abstract
1. Introduction
2. Results and Discussion
2.1. Atomic-Specific Microstructure of the Examined Arsenoselenides
2.2. Atomic-Deficient Microstructure of the Examined Arsenoselenides
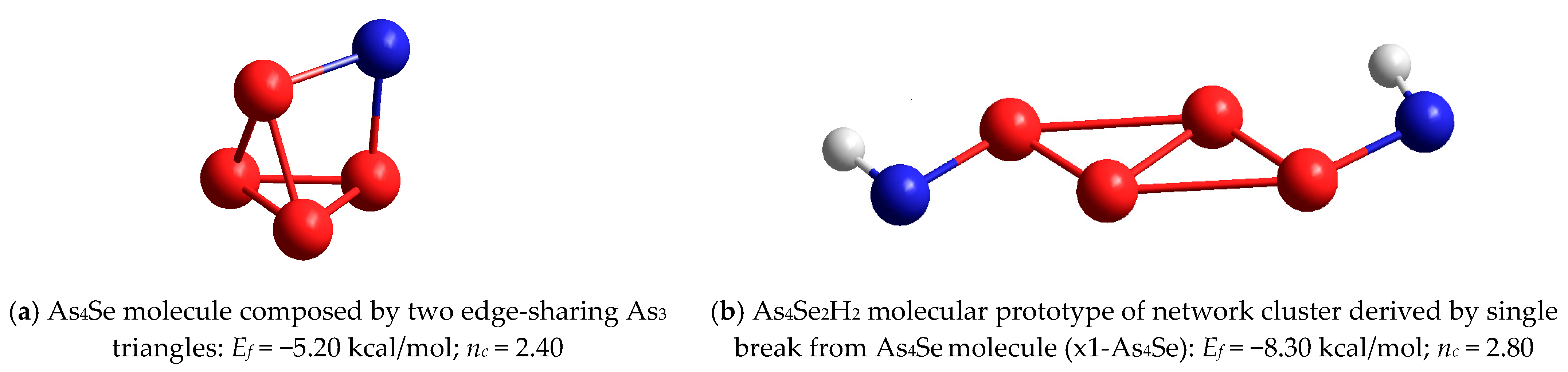
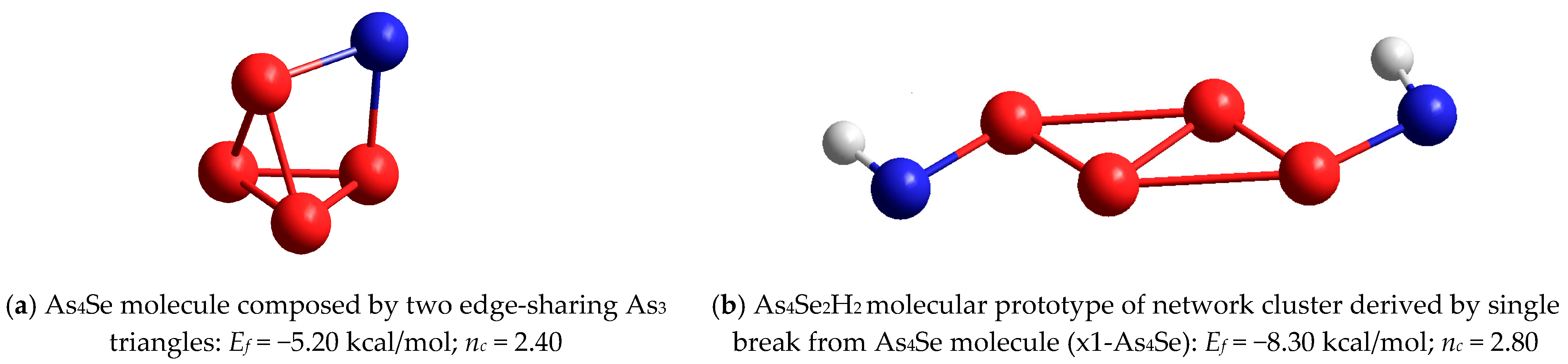
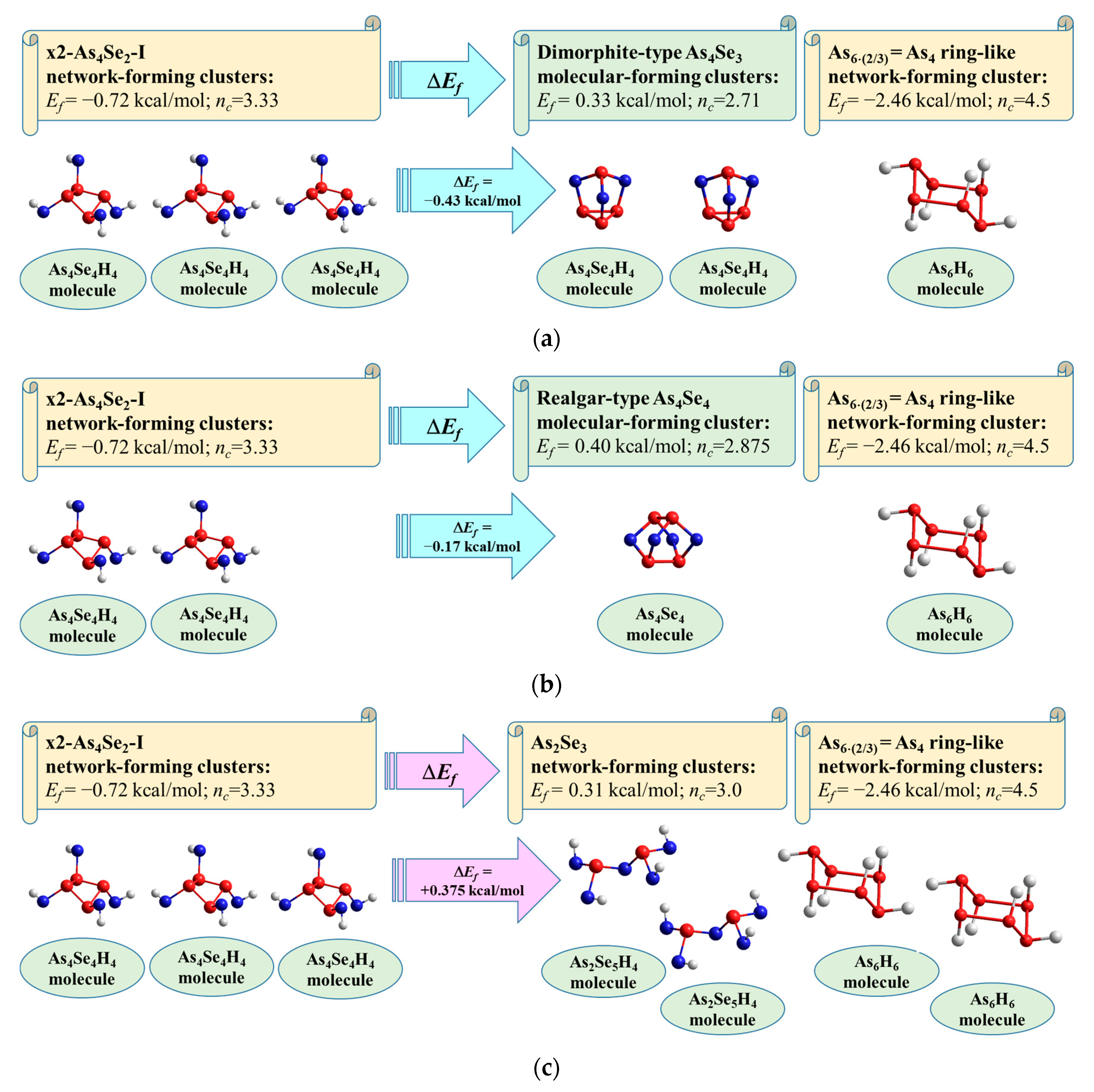
2.3. Molecular–Network Disproportionality at the Border of the Glass-Forming Region in As-Se System via Quantum–Chemical Cluster Modelling
3. Materials and Methods
3.1. Preparation, Preliminary Testing and Nanomilling of the Examined Arsenoselenides
3.2. Atomic-Specific Phase Composition and Medium-Range Structure via Xrpd Analysis
3.3. Atomic-Specific Microstructure by Micro-Rs Spectroscopy
3.4. Atomic-Deficient Microstructure by Revised PAL Analysis
3.5. Quantum–Chemical Modeling of Molecular–Network Clustering
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Feltz, A.; Aust, H.; Blayer, A. Glass formation and properties of chalcogenide systems XXVI: Permittivity and the structure of glasses AsxSe1−x and GexSe1−x. J. Non-Cryst. Solids 1983, 55, 179–190. [Google Scholar] [CrossRef]
- Feltz, A. Amorphous Inorganic Materials and Glasses; VCH: Weinheim, Germany, 1993; pp. 1–446. [Google Scholar]
- Yang, G.; Bureau, B.; Rouxel, T.; Gueguen, Y.; Gulbiten, O.; Roiland, C.; Soignard, E.; Yarger, J.L.; Troles, J.; Sangleboeuf, J.-C.; et al. Correlation between structure and physical properties of chalcogenide glasses in the AsxSe1−x system. Phys. Rev. B 2010, 82, 195206. [Google Scholar] [CrossRef]
- Adam, J.-L.; Zhang, X. Chalcogenide Glasses: Preparation, Properties and Application; Woodhead Publishing Series in Electronic and Optical Materials: Philadelphia, PA, USA; New Delhi, India, 2013; pp. 1–717. [Google Scholar]
- Cui, S.; Chahal, R.; Boussard-Plédel, C.; Nazabal, V.; Doualan, J.-L.; Troles, J.; Lucas, J.; Bureau, B. From Selenium- to Tellurium-Based Glass Optical Fibers for Infrared Spectroscopies. Molecules 2013, 18, 5373–5388. [Google Scholar] [CrossRef]
- Cui, S.; Chahal, R.; Shpotyuk, Y.; Boussard, C.; Lucas, J.; Charpentier, F.; Tariel, H.; Loreal, O.; Nazabal, V.; Sire, O.; et al. Selenide and telluride glasses for mid-infrared bio-sensing. Proc. SPIE 2014, 8938, 893805. [Google Scholar] [CrossRef]
- Eggleton, B.J. Chalcogenide photonics: Fabrication, devices and applications Introduction. Opt. Express 2010, 18, 26632–26634. [Google Scholar] [CrossRef] [PubMed]
- Eggleton, B.; Luther-Davies, B.; Richardson, K. Chalcogenide photonics. Nat. Photon 2011, 5, 141–148. [Google Scholar] [CrossRef]
- Lucas, J.; Troles, J.; Zhang, X.H.; Boussard-Pledel, C.; Poulain, P.; Bureau, B. Glasses to see beyond visible. Des verres pour voir au-delà du visible. C.R. Chimie 2018, 21, 916–922. [Google Scholar] [CrossRef]
- Zhang, X.H.; Adam, J.L.; Bureau, B. Chalcogenide Glasses. In Springer Handbook of Glass. Springer Handbooks; Musgraves, J.D., Hu, J., Calvez, L., Eds.; Springer: Cham, Switzerland, 2019; pp. 525–552. [Google Scholar] [CrossRef]
- Mehta, N. Advances in Chalcogenide Glasses (ChGs): Past, Present, and Future Applications. In Advances in Glass Research. Advances in Material Research and Technology; Ikhmayies, S.J., Ed.; Springer: Cham, Switzerland, 2023; pp. 153–168. [Google Scholar] [CrossRef]
- Stenger, F.; Mende, S.; Schwedesc, J.; Peukertd, W. Nanomilling in stirred media mills. Chem. Eng. Sci. 2005, 60, 4557–4565. [Google Scholar] [CrossRef]
- Yadav, T.P.; Yadav, R.M.; Singh, D.P. Mechanical Milling: A Top Down Approach for the Synthesis of Nanomaterials and Nanocomposites. Nanosci. Nanotechnol. 2012, 2, 22–48. [Google Scholar] [CrossRef]
- Baláž, P.; Achimovicova, M.; Baláž, M.; Billik, P.; Cherkezova-Zheleva, Z.; Manuel Criado, J.; Delogu, F.; Dutkova, E.; Gaffet, E.; Gotor, F.J.; et al. Hallmarks of mechanochemistry: From nanoparticles to technology. Chem. Soc. Rev. 2013, 42, 7571–7637. [Google Scholar] [CrossRef]
- Baláž, P.; Baláž, M.; Achimovičová, M.; Bujňáková, Z.; Dutková, E. Chalcogenide mechanochemistry in materials science: Insight into synthesis and applications (a review). J. Mater. Sci. 2017, 52, 11851–11890. [Google Scholar] [CrossRef]
- El-Eskandarany, M.S.; Al-Hazza, A.; Al-Hajji, L.A.; Ali, N.; Al-Duweesh, A.A.; Banyan, M.; Al-Ajmi, F. Mechanical Milling: A Superior Nanotechnological Tool for Fabrication of Nanocrystalline and Nanocomposite Materials. Nanomaterials 2021, 11, 2484. [Google Scholar] [CrossRef]
- Senna, M. The Optimization of Mechanochemical Processes toward Functional Nanocomposite Materials. Powders 2023, 2, 659–677. [Google Scholar] [CrossRef]
- Bonazzi, P.; Bindi, L. A crystallographic review of arsenic sulfides: Effects of chemical variations and changes induced by exposure to light. Z. Kristallogr. 2008, 223, 132–147. [Google Scholar] [CrossRef]
- Gibbs, G.V.; Wallace, A.F.; Downs, R.T.; Ross, N.L.; Cox, D.F.; Rosso, K.M. Thioarsenides: A case for long-range Lewis acid–base-directed van der Waals interactions. Phys. Chem. Minerals 2010, 38, 267–291. [Google Scholar] [CrossRef]
- Shpotyuk, Y.; Boussard-Pledel, C.; Bureau, B.; Demchenko, P.; Szlezak, J.; Cebulski, J.; Bujňáková, Z.; Baláž, P.; Shpotyuk, O. Effect of high-energy mechanical milling on the FSDP-related XRPD correlations in Se-rich glassy arsenic selenides. J. Phys. Chem. Sol. 2019, 124, 318–326. [Google Scholar] [CrossRef]
- Shpotyuk, Y.; Demchenko, P.; Bujňáková, Z.; Baláž, P.; Boussard-Pledel, C.; Bureau, B.; Shpotyuk, O. Effect of high-energy mechanical milling on the medium-range ordering in glassy As-Se. J. Am. Ceram. Soc. 2020, 103, 1631–1646. [Google Scholar] [CrossRef]
- Shpotyuk, Y.; Shpotyuk, O.; Lukáčová Bujňáková, Z.; Baáž, P.; Hyla, M.; Boussard-Pledel, C.; Bureau, B. Tailoring Se-rich glassy arsenoselenides employing the nanomilling platform. Mater. Sci. Eng. B 2024, 300, 117069. [Google Scholar] [CrossRef]
- Shpotyuk, Y.; Demchenko, P.; Shpotyuk, O.; Balitska, V.; Boussard-Pledel, C.; Bureau, B.; Lukáčová Bujňáková, Z.; Baláž, P. High-energy mechanical milling-driven reamorphization in glassy arsenic monoselenide g-AsSe: On the path tailoring special molecular-network glasses. Materials 2021, 14, 4478. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Ingram, A.; Bujnáková, Z.; Baláž, P.; Shpotyuk, Y.; Slobodzyan, D.; Wozny, M.; Boussard-Pledel, C.; Bureau, B. Free-Volume Structure of Polyvinylpyrrolidone-Capped Glassy As2Se3 Nanocomposites Prepared by Mechanical Milling. Polym. Eng. Sci. 2019, 59, 2438–2442. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Ingram, A.; Filipecki, J.; Shpotyuk, Y.; Cebulski, J.; Lukáčová Bujňáková, Z.; Baláž, P. Volumetric nanostructurization in glassy arsenoselenides driven by high-energy mechanical dry- and wet-milling. Macromol. Sympos. 2022, 405, 2100253. [Google Scholar] [CrossRef]
- Shpotyuk, Y.; Ingram, A.; Shpotyuk, O.; Demchenko, P.; Lukáčová Bujňáková, Z.; Baláž, P. Polyvinylpyrrolidone-nanosized glassy arsenoselenides characterized by complementary Positronics and XRD analysis. Appl. Nanosci. 2023, 13, 4661–4674. [Google Scholar] [CrossRef]
- Kozdras, A.; Shpotyuk, O.; Mahlovanyi, B.; Shpotyuk, Y.; Kovalskiy, A. Thermodynamic heat-transfer phenomena in nanostructured glassy substances: A comparative study on g-As5Se95 and g-As55Se45. J. Therm. Anal. Calorim. 2023, 148, 2265–2271. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Filipecki, J.; Ingram, A.; Golovchak, R.; Vakiv, M.; Klym, H.; Balitska, V.; Shpotyuk, M.; Kozdras, A. Positronics of subnanometer atomistic imperfections in solids as a high-informative structure characterization tool. Nanoscale Res. Lett. 2015, 10, 77. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Ingram, A.; Boussard-Pledel, C.; Bureau, B.; Lukáčová Bujňáková, Z.; Baláž, P.; Mahlovanyi, B.; Shpotyuk, Y. The art of Positronics in contemporary nanomaterials science: A case study of sub-nanometer scaled glassy arsenoselenides. Materials 2022, 15, 302. [Google Scholar] [CrossRef] [PubMed]
- Greaves, G.N.; Elliott, S.R.; Davis, E.A. Amorphous arsenic. Adv. Phys. 1979, 28, 49–141. [Google Scholar] [CrossRef]
- Schiferl, D.; Barret, S. The Crystal Structure of Arsenic at 4.2, 78 and 299 °K. J. Appl. Cryst. 1969, 2, 30–36. [Google Scholar] [CrossRef]
- Bastow, T.J.; Whitfied, H.J. Crystal data and nuclear quadrupole resonance spectra of tetra-arsenic triselenide. J. Chem. Soc. Dalton Trans. 1977, 10, 959–961. [Google Scholar] [CrossRef]
- Bastow, T.J.; Whitfield, H.J.J. Crystal structure of tetra-arsenic tetraselenide. Chem. Soc. Dalton Trans 1973, 17, 1739–1740. [Google Scholar] [CrossRef]
- Smail, E.J.; Sheldrick, G.M. Tetra-arsenic tetraselenide. Acta Cryst. B 1973, 29, 2014–2016. [Google Scholar] [CrossRef]
- Renninger, A.L.; Averbach, B.L. Crystalline structures of As2Se3 and As4Se4. Acta Cryst. B 1973, 29, 1583–1589. [Google Scholar] [CrossRef]
- Stergiou, A.C.; Rentzeperis, P.J. The crystal structure of arsenic selenide, As2Se3. Zeitsch. Krist 1985, 173, 185–191. [Google Scholar] [CrossRef]
- Cherin, P.; Unger, P. The Crystal Structure of Trigonal Selenium. Inorg. Chem. 1967, 6, 1589–1591. [Google Scholar] [CrossRef]
- Ballirano, P.; Maras, A. Refinement of the crystal structure of arsenolite, As2O3. Ζ. Kristalogr. 2002, 217, 177–178. [Google Scholar] [CrossRef]
- Vaipolin, A.A.; Porai-Koshits, E.A. Structural models of glasses and the structures of crystalline chalcogenides. Sov. Phys. Solid State 1963, 5, 497–500. [Google Scholar]
- Tanaka, K.; Shimakawa, K. Amorphous Chalcogenide Semiconductors and Related Materials; Springer: New York, NY, USA; Dordrecht, The Netherlands; Heidelberg, Germany; London, UK, 2011; pp. 1–242. [Google Scholar]
- Lucovsky, G. Optic modes in amorphous As2S3 and As2Se3. Phys. Rev. B 1972, 6, 1480–1489. [Google Scholar] [CrossRef]
- Pangavhane, S.D.; Nemec, P.; Wagner, T.; Janca, J.; Havel, J. Laser desorption ionization time-of-flight mass spectrometric study of binary As-Se glasses. Rapid Commun. Mass Spectrom. 2010, 24, 2000–2008. [Google Scholar] [CrossRef] [PubMed]
- Lannin, J.S. Raman scattering properties of amorphous As and Sb. Phys. Rev. B 1977, 15, 3863–3871. [Google Scholar] [CrossRef]
- Brumbach, S.B.; Rosenblatt, G.M. In-cavity laser Raman spectroscopy of vapors at elevated temperatures. As4 and As4O6. J. Chem. Phys. 1972, 56, 3110–3117. [Google Scholar] [CrossRef]
- Kovanda, V.; Vlcek, M.; Jain, H. Structure of As-Se and As-P-Se glasses studied by Raman spectroscopy. J. Non-Cryst. Solids 2003, 326–327, 88–92. [Google Scholar] [CrossRef]
- Golovchak, R.; Oelgoetz, J.; Vlcek, M.; Esposito, A.; Saiter, A.; Saiter, J.-M.; Jain, H. Complex structural rearrangements in As-Se glasses. J. Chem. Phys. 2014, 140, 054505. [Google Scholar] [CrossRef]
- Kalyva, M.; Orava, J.; Siokou, A.; Pavlista, M.; Wagner, T.; Yannopoulos, S.N. Reversible Amorphous-to-Amorphous Transitions in Chalcogenide Films: Correlating Changes in Structure and Optical Properties. Adv. Funct. Mater. 2013, 23, 2052–2059. [Google Scholar] [CrossRef]
- Properzi, L.; Santoro, M.; Minicucci, M.; Iesari, F.; Ciambezi, M.; Nataf, L.; Le Godec, Y.; Irifune, T.; Baudelet, F.; Di Cicco, A. Structural evolution mechanisms of amorphous and liquid As2Se3 at high pressures. Phys. Rev. B 2016, 93, 214205. [Google Scholar] [CrossRef]
- Hu, Y.; Liang, J.; Xia, Y.; Zhao, C.; Jiang, M.H.; Ma, J.; Tie, Z.X.; Jin, Z. 2D Arsenene and Arsenic Materials: Fundamental Properties, Preparation, and Applications. Small 2021, 18, 2104556. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Bujňáková, Z.; Baláž, P.; Ingram, A.; Shpotyuk, Y. Positron annihilation lifetime study of polyvinylpyrrolidone for nanoparticle-stabilizing pharmaceuticals. J. Pharm. Biomed. Anal. 2016, 117, 419–425. [Google Scholar] [CrossRef]
- Jensen, K.O.; Salmon, P.S.; Penfold, I.T.; Coleman, P.G. Microvoids in chalcogenide glasses studied by positron annihilation. J. Non-Cryst. Solids 1994, 170, 57–64. [Google Scholar] [CrossRef]
- Ingram, A.; Golovchak, R.; Kostrzewa, M.; Wacke, S.; Shpotyuk, M.; Shpotyuk, O. Compositional dependences of average positron lifetime in binary As-S/Se glasses. Phys. B Condens. Matter 2012, 407, 652–655. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Golovchak, R.; Ingram, A.; Boyko, V.; Shpotyuk, L. Comparative study of extended free-volume defects in As- and Ge-based glassy semiconductors: Theoretical prediction and experimental probing with PAL technique. Phys. Stat. Solidi C 2013, 10, 117–120. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Ingram, A.; Shpotyuk, M.; Filipecki, J. Prediction of free-volume-type correlations in glassy chalcogenides from positron lifetime measurements. Nucl. Instr. Meth. Phys. Res. B 2014, 338, 66–71. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Filipecki, J.; Shpotyuk, M.; Ingram, A. Free volume evolution in chalcogenide glasses as probed by PAL spectroscopy. Solid State Ionics 2014, 267, 38–43. [Google Scholar] [CrossRef]
- Vijay, Y.K.; Wate, S.; Awasthi, D.K.; Das, D.; Ghughre, S. Ion induced effects in polymers. Indian J. Eng. Mater. Sci. 2000, 7, 375–377. [Google Scholar]
- Krause-Rehberg, R.; Leipner, H. Positron Annihilation in Semiconductors: Defect Studies; Springer: Heidelberg, Germany, 1999; pp. 1–350. [Google Scholar]
- Blachnik, R.; Wickel, U. Thermal behaviour of A4B3 cage molecules (A = P, As; B = S, Se). Thermochim. Acta 1984, 81, 185–196. [Google Scholar] [CrossRef]
- Shpotyuk, O.; Hyla, M.; Shpotyuk, Y.; Lukáčová Bujňáková, Z.; Baláž, P.; Demchenko, P.; Kozdraś, A.; Boyko, V.; Kovalskiy, A. Molecular-Network Transformations in Tetra-Arsenic Triselenide Glassy Alloys Tuned within Nanomilling Platform. Molecules 2024, 29, 3245. [Google Scholar] [CrossRef] [PubMed]
- Shpotyuk, O.; Hyla, M.; Boyko, V. Structural-topological genesis of network-forming nano-clusters in chalcogenide semiconductor glasses. J. Optoelectron. Adv. Mater. 2013, 15, 1429–1437. [Google Scholar]
- Shpotyuk, O.; Hyla, M.; Boyko, V. Compositionally-dependent structural variations in glassy chalcogenides: The case of binary As-Se system. Comput. Mater. Sci. 2015, 110, 144–151. [Google Scholar] [CrossRef]
- Hyla, M.; Shpotyuk, O. Topological configurations of principal network-forming clusters in As/Ge-Se glasses. J. Optoelectron. Adv. Mater. 2017, 19, 211–217. [Google Scholar]
- Hyla, M. Compositional anomalies in Se-rich As-Se glass-forming system as revealed from positron annihilation lifetime spectroscopy and ab initio cluster modeling. Comput. Mater. Sci. 2017, 135, 165–168. [Google Scholar] [CrossRef]
- Hyla, M. Network-Forming Nanoclusters in Binary As–S/Se Glasses: From Ab Initio Quantum Chemical Modeling to Experimental Evidences. Nanoscale Res. Lett. 2017, 12, 45. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Phillips, J.C. Topology of covalent non-crystalline solids. I: Short-range order in chalcogenide alloys. J. Non-Cryst. Solids 1979, 34, 153–181. [Google Scholar] [CrossRef]
- Thorpe, M.F. Continuous deformations in random networks. J. Non-Cryst. Solids 1983, 57, 355–370. [Google Scholar] [CrossRef]
- Thorpe, M.F. Bulk and surface floppy modes. J. Non-Cryst. Solids 1995, 182, 135–142. [Google Scholar] [CrossRef]
- Whitfield, H.J. The crystal structure of tetra-arsenic trisulphide. J. Chem. Soc. A 1970, 1800–1803. [Google Scholar] [CrossRef]
- Whitfield, H.J. Crystal structure of the β-form of tetra-arsenic trisulphide. J. Chem. Soc. Dalton 1973, 17, 1737–1738. [Google Scholar] [CrossRef]
- Blachnik, R.; Hoppe, A.; Wickel, U. Die Systeme Arsen-Schwefel und Arsen-Selen und die thermodynamischen Daten ihrer Verbindungen. Z. Anorg. Allg. Chem. 1980, 468, 78–90. [Google Scholar] [CrossRef]
- Seidl, M.; Balazs, G.; Scgeer, M. The Chemistry of Yellow Arsenic. Chem. Revs. 2019, 119, 8406–8434. [Google Scholar] [CrossRef] [PubMed]
- Hart, M.; Chen, J.; Michaelides, A.; Sella, A.; Shaffer, M.S.P.; Salzmann, C.G. One-dimensional Arsenic Allotropes: Polymerization of Yellow Arsenic inside Single-wall Carbon Nanotubes. Angew. Chem. 2018, 130, 11823–11827. [Google Scholar] [CrossRef]
- Downs, R.T.; Hall-Wallace, M. The American mineralogist crystal structure database. Am. Mineral. 2003, 88, 247–250. [Google Scholar]
- Villars, P.; Cenzual, K. Pearson’s Crystal Data: Crystal Structure Database for Inorganic Compounds; Release 2014/15; ASM International: Materials Park, OH, USA, 2014. [Google Scholar]
- Elliott, S.R. Extended-range order, interstitial voids and the first sharp diffraction peak of network glasses. J. Non-Cryst. Solids 1995, 182, 40–48. [Google Scholar] [CrossRef]
- Elliott, S.R. Second sharp diffraction peak in the structure factor of binary covalent network glasses. Phys. Rev. B 1995, 51, 8599–8601. [Google Scholar] [CrossRef]
- Zeidler, A.; Salmon, P.S. Pressure-driven transformation of the ordering in amorphous network-forming materials. Phys. Rev. B 2016, 93, 214204. [Google Scholar] [CrossRef]
- Roisnel, T.; Rodriguez-Carvajal, J. WinPLOTR: A Windows tool for powder diffraction pattern analysis. Mater. Sci. Forum 2001, 118, 378–381. [Google Scholar] [CrossRef]
- Kraus, W.; Nolze, G. POWDER CELL—A program for the representation and manipulation of crystal structures and calculation of the resulting X-ray powder patterns. J. Appl. Cryst. 1996, 29, 301–303. [Google Scholar] [CrossRef]
- Feng, R.; Stachurski, Z.H.; Rodrigues, M.D.; Kluth, P.; Araujo, L.L.; Bulla, D.; Ridway, M.C. X-ray scattering from amorphous solids. J. Non-Cryst. Solids 2013, 383, 21–27. [Google Scholar] [CrossRef]
- Ehrenfest, P. On interference phenomena to be expected when Roentgen rays pass through a diatomic gas. Proc. KNAW 1915, 17, 1184–1190. [Google Scholar]
- Kansy, J. Microcomputer program for analysis of positron annihilation lifetime spectra. Nucl. Instr. Meth. Phys. Res. A 1996, 374, 235–244. [Google Scholar] [CrossRef]
- Tuomisto, F.; Makkonen, I. Defect identification in semiconductors with positron annihilation: Experiment and theory. Rev. Mod. Phys. 2013, 85, 1583–1631. [Google Scholar] [CrossRef]
- Shpotyuk, M.; Ingram, A.; Shpotyuk, O. Characterization of radiation-induced effects in amorphous arsenic sulfides by positron annihilation lifetime spectroscopy. J. Mater. Res. 2015, 30, 1422–1429. [Google Scholar] [CrossRef]
- Jean, Y.C.; Mallon, P.E.; Schrader, D.M. Principles and Application of Positron and Positronium Chemistry; World Scientific Publishing Co Pte Ltd.: Hackensack, NJ, USA; London, UK; Singapore; Hong Kong, China, 2003; pp. 1–406. [Google Scholar]
- Castelli, F.; Consolatti, G.; Marlotti, G.T. Positronium confined in nanocavities: The role of electron exchange correlations. Nanomaterials 2021, 11, 2350–2351. [Google Scholar] [CrossRef] [PubMed]
- Hehre, W.J.; Stewart, R.F.; Pople, J.A. Self-consistent molecular-orbital methods. I. Use of Gaussian expansions of slater-type atomic orbitals. J. Chem. Phys. 1969, 51, 2657–2665. [Google Scholar] [CrossRef]
- McLean, A.D.; Chandler, G.S. Contracted Gaussian basis sets for molecular calculations. I. Second row atoms, Z = 11–18. J. Chem. Phys. 1980, 72, 5639–5648. [Google Scholar] [CrossRef]
- Jackson, K. Electric fields in electronic structure calculations: Electric polarizabilities and IR and Raman spectra from first principles. Phys. Stat. Solidi B 2000, 217, 293–310. [Google Scholar] [CrossRef]
- Holomb, R.; Veres, M.; Mitsa, V. Ring-, branchy-, and cage-like AsnSm nanoclusters in the structure of amorphous semiconductors: Ab initio and Raman study. J. Optoelectron. Adv. Mater. 2009, 11, 917–923. [Google Scholar]
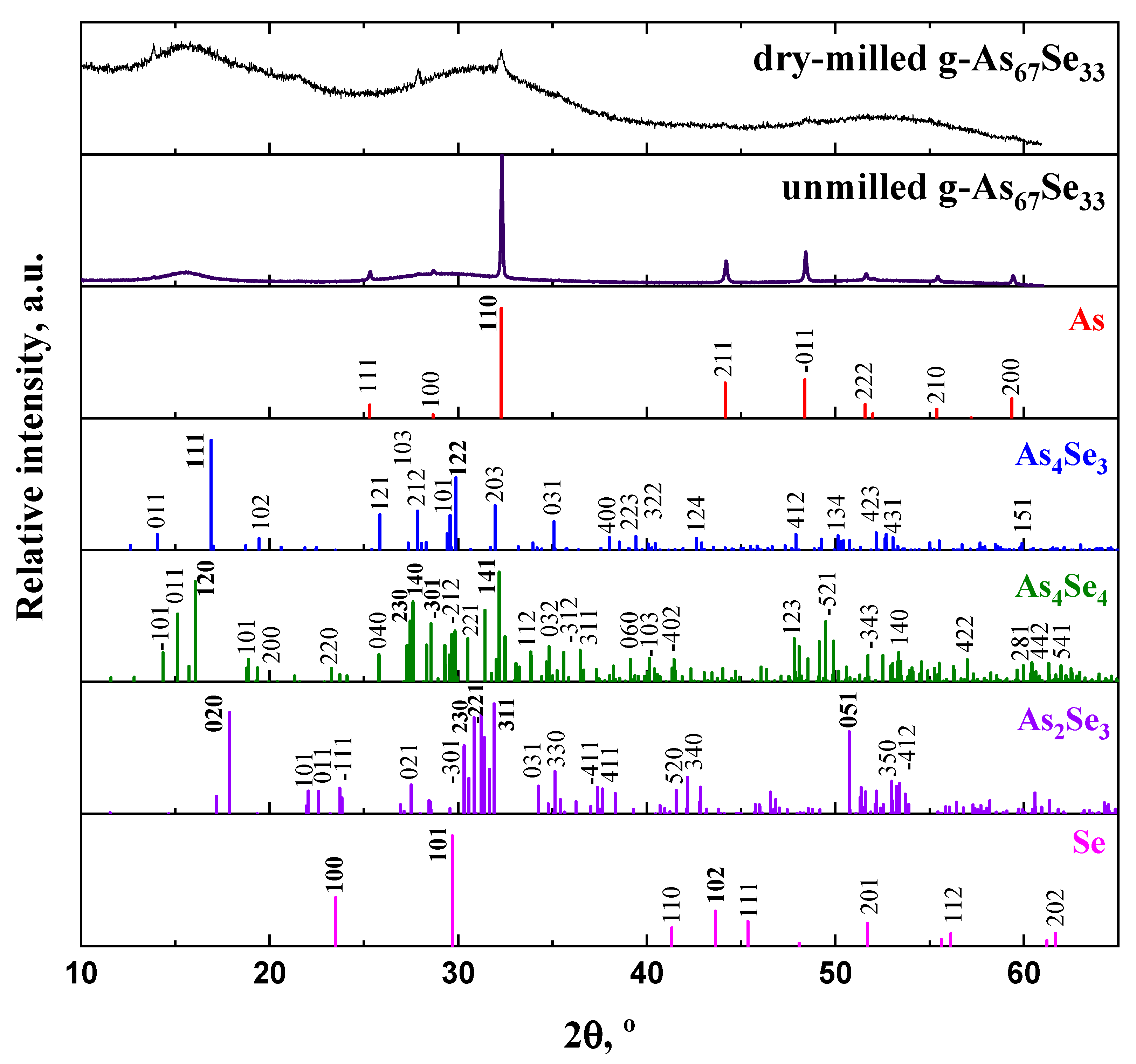
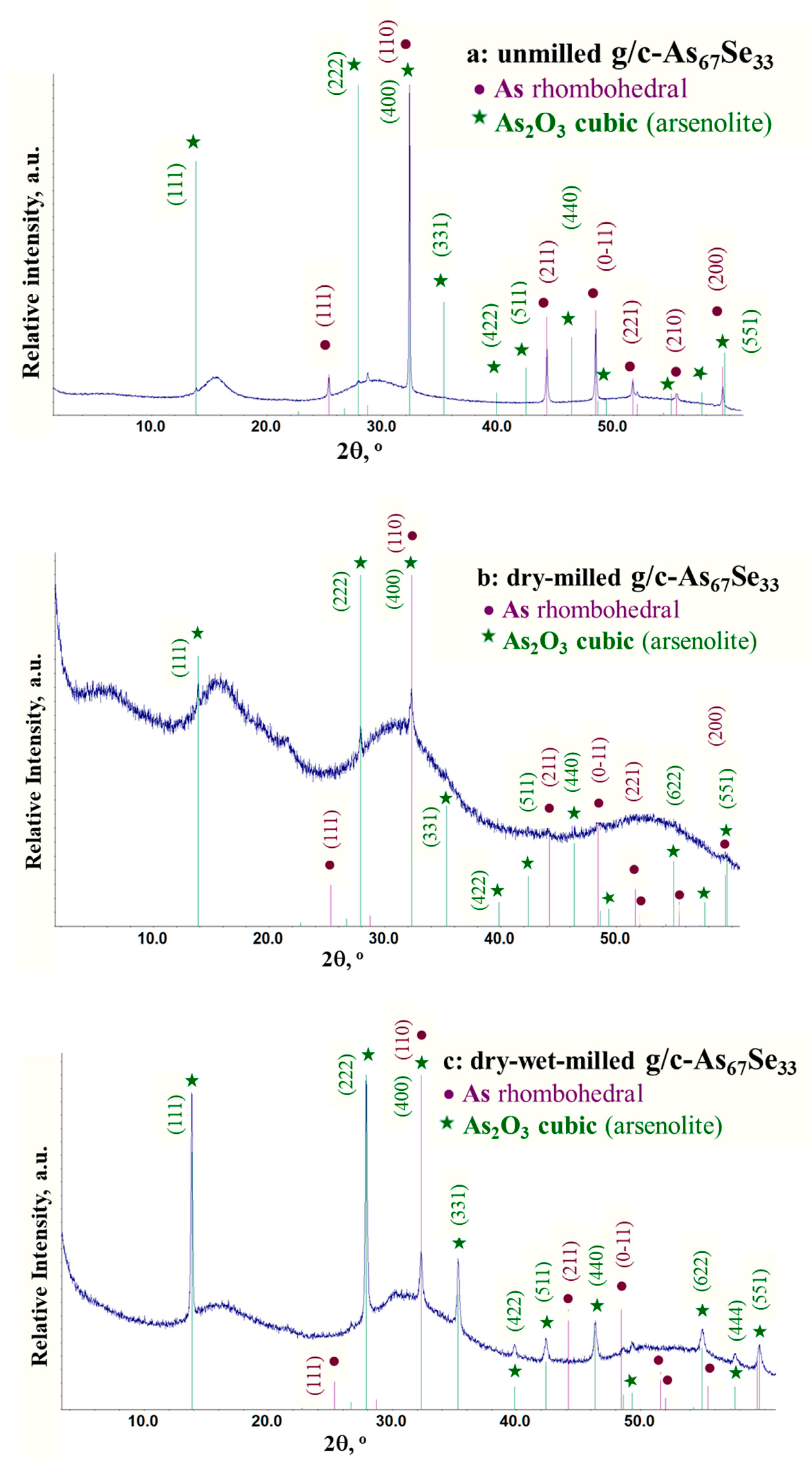
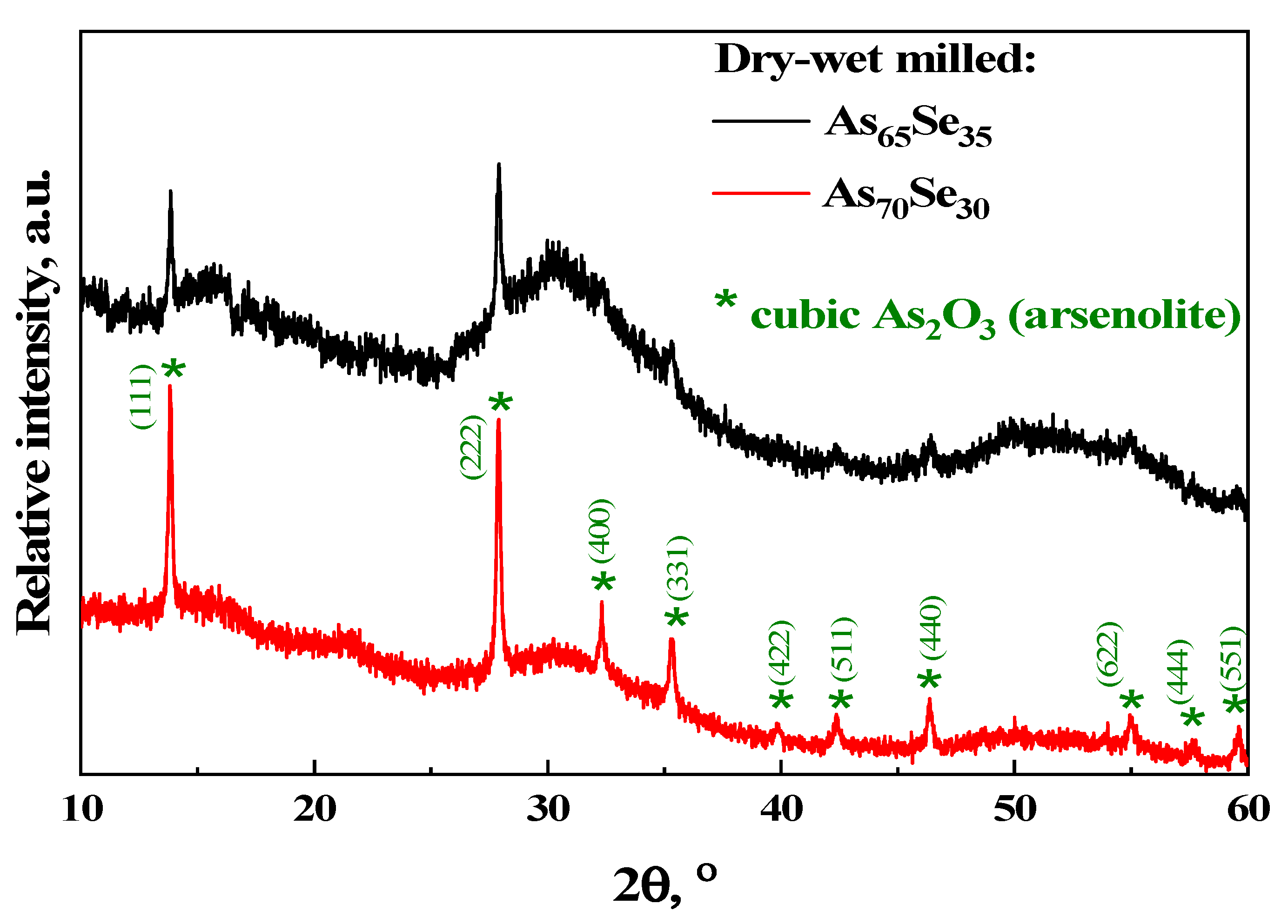


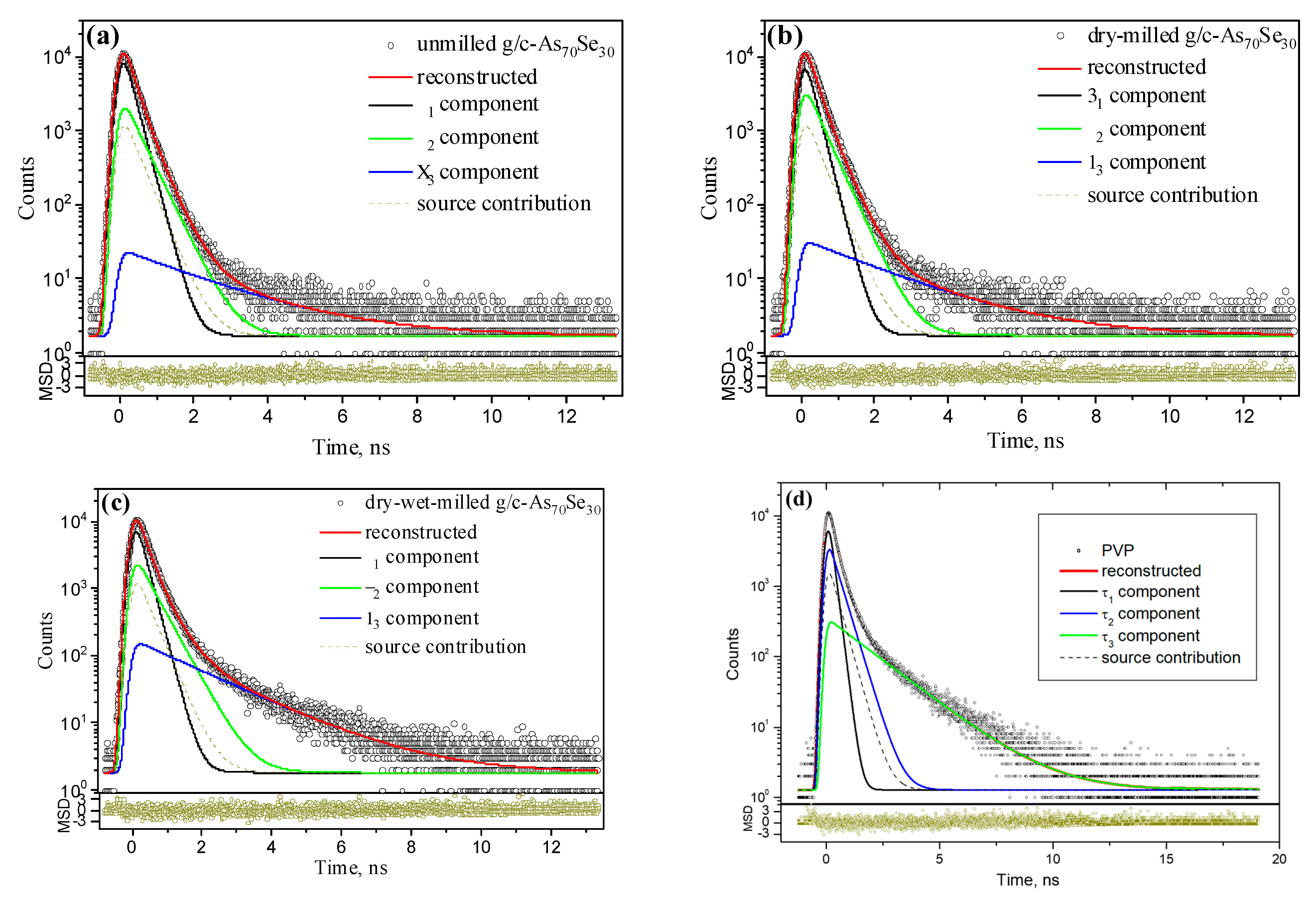
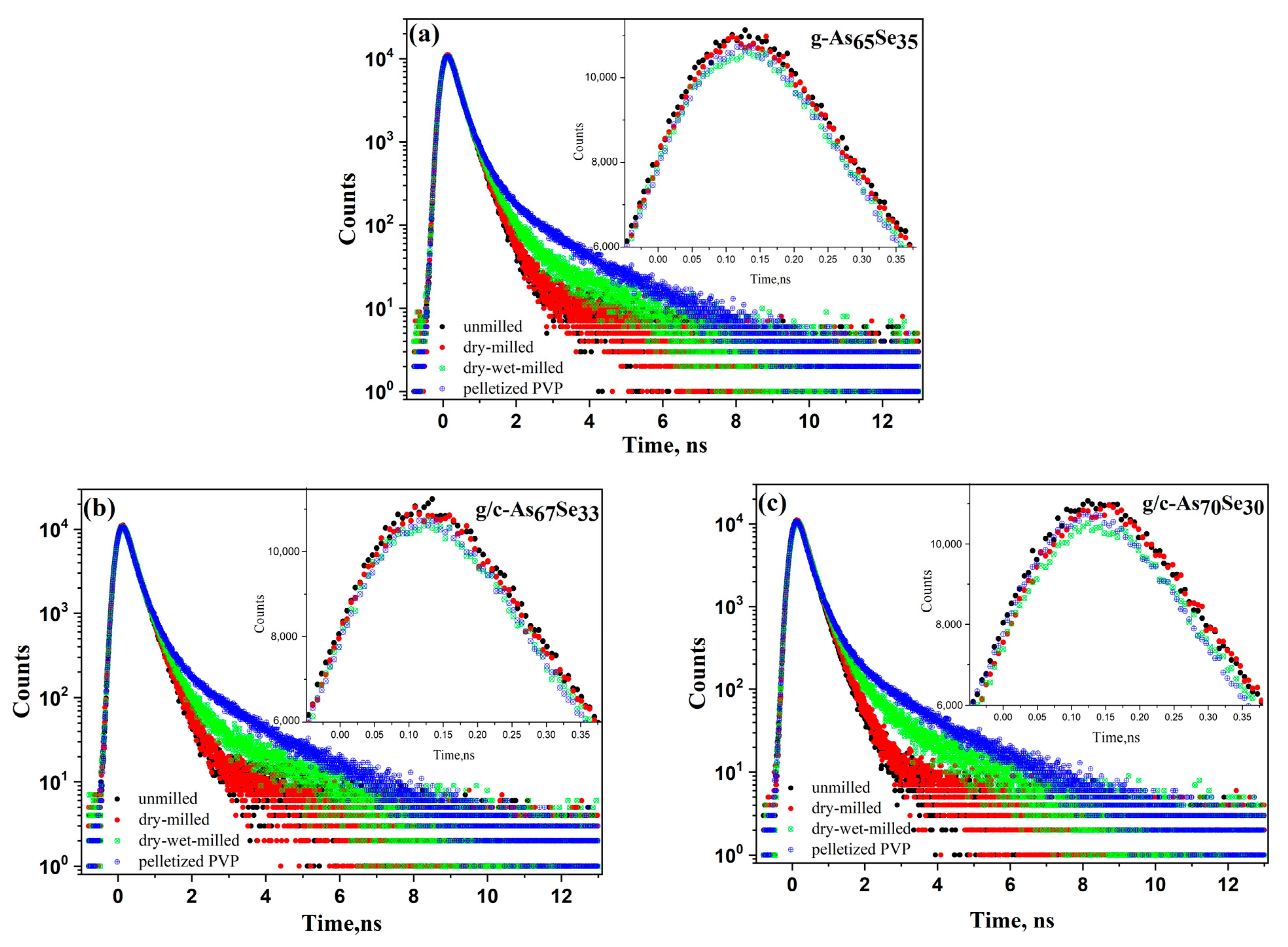

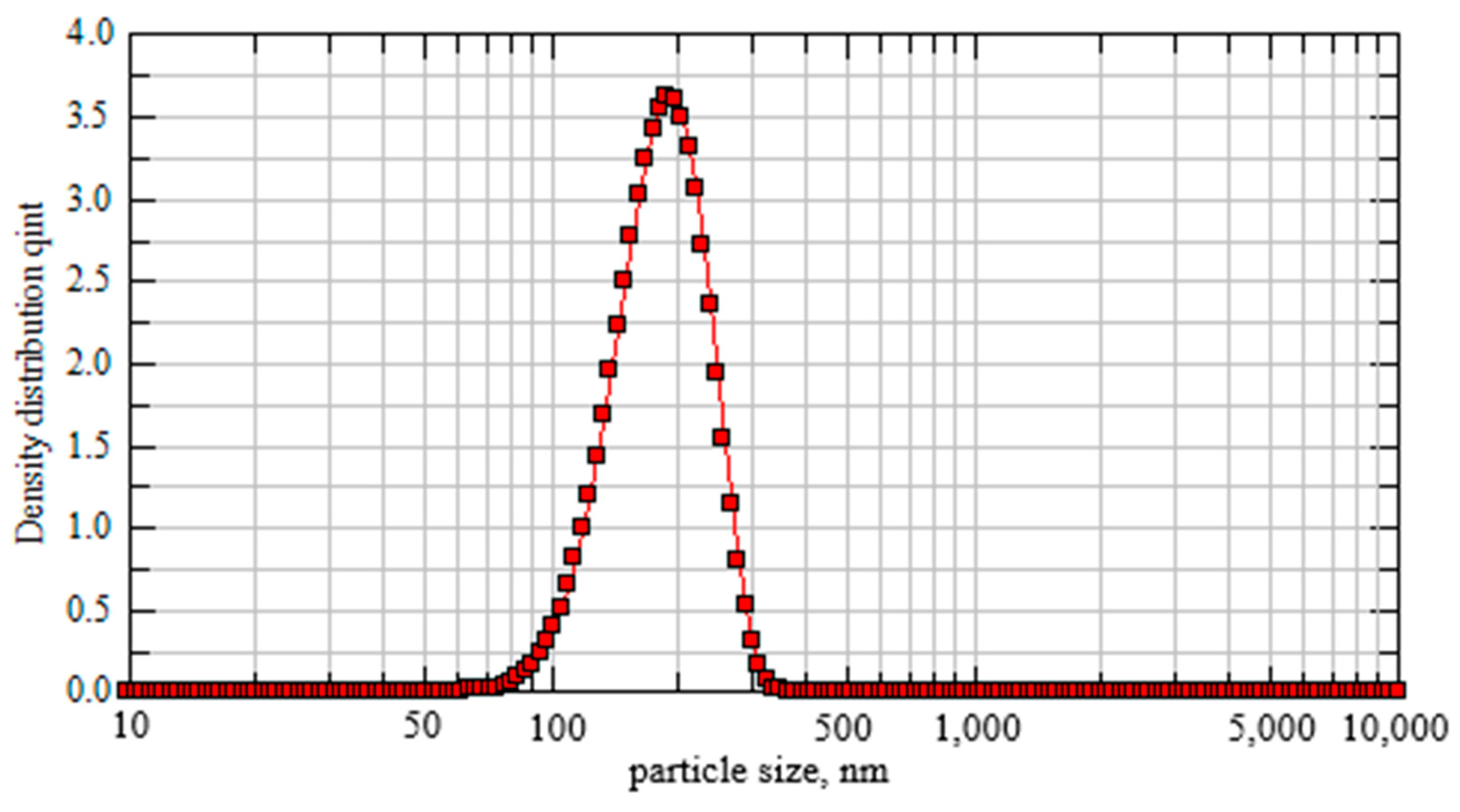
| AsxSe100−x Samples: | 2θ | FWHM | Q1 | ΔQ1 | R | L | ds | |
|---|---|---|---|---|---|---|---|---|
| Phase Composition, CN | State | °2θ | °2θ | Å−1 | Å−1 | Å | Å | Å |
| g-As65Se35, CN = 2.65 | unmilled | 15.641(5) | 2.49(1) | 1.11 | 0.18 | 5.66 | 35.52 | 6.96 |
| dry-milled | 16.170(13) | 4.42(4) | 1.15 | 0.31 | 5.48 | 19.98 | 6.74 | |
| dry–wet-milled | 15.627(42) | 6.20(12) | 1.11 | 0.44 | 5.67 | 14.25 | 6.97 | |
| g/c-As67Se33, CN = 2.67 | unmilled | 15.479(4) | 2.34(1) | 1.10 | 0.17 | 5.71 | 37.8 | 7.02 |
| dry-milled | 15.732(10) | 3.77(6) | 1.12 | 0.27 | 5.63 | 23.4 | 6.92 | |
| dry–wet-milled | 15.891(15) | 5.08(4) | 1.13 | 0.36 | 5.57 | 17.4 | 6.85 | |
| g/c-As70Se30, CN = 2.70 | unmilled | 15.318(5) | 2.33(1) | 1.09 | 0.17 | 5.78 | 37.90 | 7.11 |
| dry-milled | 15.668(4) | 4.38(5) | 1.11 | 0.31 | 5.65 | 20.17 | 6.95 | |
| dry–wet-milled | 15.605(5) | 8.33(12) | 1.11 | 0.59 | 5.67 | 10.65 | 6.97 | |
| Pelletized AsxSe100−x Samples: Phase Composition, State | [FIT-1] | τ1, ns | τ2, ns | τ3, ns | I2, a.u. | I3, a.u. | τav., ns |
|---|---|---|---|---|---|---|---|
| PVP pelletized [50] | 0.200 | 0.196 | 0.472 | 1.867 | 0.256 | 0.119 | 0.466 |
| g-As65Se35, unmilled | 0.060 | 0.207 | 0.367 | 2.089 | 0.520 | 0.015 | 0.318 |
| g-As65Se35, dry-milled | 0.040 | 0.198 | 0.371 | 2.180 | 0.560 | 0.015 | 0.325 |
| g-As65Se35, dry–wet-milled | 0.070 | 0.216 | 0.431 | 1.937 | 0.390 | 0.046 | 0.379 |
| g/c-As67Se33, unmilled | 0.090 | 0.201 | 0.362 | 2.065 | 0.550 | 0.016 | 0.318 |
| g/c-As67Se33, dry-milled | 0.050 | 0.188 | 0.362 | 2.095 | 0.600 | 0.015 | 0.323 |
| g/c-As67Se33, dry–wet-milled | 0.070 | 0.202 | 0.403 | 1.914 | 0.470 | 0.049 | 0.379 |
| g/c-As70Se30, unmilled | 0.048 | 0.218 | 0.386 | 2.019 | 0.320 | 0.011 | 0.291 |
| g/c-As70Se30, dry-milled | 0.044 | 0.218 | 0.385 | 2.120 | 0.390 | 0.013 | 0.307 |
| g/c-As70Se30, dry–wet-milled | 0.070 | 0.212 | 0.425 | 1.730 | 0.329 | 0.062 | 0.377 |
| Pelletized AsxSe100−x Samples: Phase Composition, State | Positron-Trapping Modes | Ps-Decay Modes | |||||
|---|---|---|---|---|---|---|---|
| τb, ns | κd, ns−1 | τ2−τb, ns | τ2/τb, a.u. | η, a.u. | R3, nm | fv3, % | |
| PVP pelletized [50] | 0.24 | 0.87 | 0.24 | 1.99 | 0.17 | 0.276 | 1.88 |
| g-As65Se35, unmilled | 0.27 | 1.11 | 0.10 | 1.37 | 0.23 | 0.296 | 0.30 |
| g-As65Se35, dry-milled | 0.27 | 1.35 | 0.10 | 1.38 | 0.27 | 0.304 | 0.32 |
| g-As65Se35, dry–wet-milled | 0.27 | 0.95 | 0.16 | 1.59 | 0.21 | 0.282 | 0.78 |
| g/c-As67Se33, unmilled | 0.27 | 1.22 | 0.09 | 1.35 | 0.24 | 0.293 | 0.30 |
| g/c-As67Se33, dry-milled | 0.27 | 1.56 | 0.10 | 1.36 | 0.29 | 0.297 | 0.30 |
| g/c-As67Se33, dry–wet-milled | 0.27 | 1.22 | 0.14 | 1.51 | 0.25 | 0.279 | 0.78 |
| g/c-As70Se30, unmilled | 0.25 | 0.64 | 0.13 | 1.52 | 0.21 | 0.289 | 0.14 |
| g/c-As70Se30, dry-milled | 0.26 | 0.78 | 0.12 | 1.47 | 0.17 | 0.298 | 0.25 |
| g/c-As70Se30, dry–wet-milled | 0.267 | 0.83 | 0.17 | 1.65 | 0.17 | 0.261 | 0.84 |
| Pelletized AsxSe100−x Sample | I Component | II Component | τavNP | Trapping-Conversion Modes | |||||
|---|---|---|---|---|---|---|---|---|---|
| τn | In | τint | Iint | τbNP | κdNP | τint−τbNP | τint/τbNP | ||
| ns | a.u. | ns | a.u. | ns | ns | ns−1 | ns | a.u. | |
| g-As65Se35 | 0.193 | 0.315 | 0.363 | 0.433 | 0.286 | 0.261 | 1.37 | 0.09 | 1.35 |
| g/c-As67Se33 | 0.201 | 0.359 | 0.367 | 0.418 | 0.291 | 0.266 | 1.21 | 0.10 | 1.38 |
| g/c-As70Se30 | 0.220 | 0.477 | 0.376 | 0.321 | 0.283 | 0.264 | 0.76 | 0.11 | 1.43 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shpotyuk, O.; Hyla, M.; Ingram, A.; Shpotyuk, Y.; Boyko, V.; Demchenko, P.; Wojnarowska-Nowak, R.; Lukáčová Bujňáková, Z.; Baláž, P. Nanostructured Molecular–Network Arsenoselenides from the Border of a Glass-Forming Region: A Disproportionality Analysis Using Complementary Characterization Probes. Molecules 2024, 29, 3948. https://doi.org/10.3390/molecules29163948
Shpotyuk O, Hyla M, Ingram A, Shpotyuk Y, Boyko V, Demchenko P, Wojnarowska-Nowak R, Lukáčová Bujňáková Z, Baláž P. Nanostructured Molecular–Network Arsenoselenides from the Border of a Glass-Forming Region: A Disproportionality Analysis Using Complementary Characterization Probes. Molecules. 2024; 29(16):3948. https://doi.org/10.3390/molecules29163948
Chicago/Turabian StyleShpotyuk, Oleh, Malgorzata Hyla, Adam Ingram, Yaroslav Shpotyuk, Vitaliy Boyko, Pavlo Demchenko, Renata Wojnarowska-Nowak, Zdenka Lukáčová Bujňáková, and Peter Baláž. 2024. "Nanostructured Molecular–Network Arsenoselenides from the Border of a Glass-Forming Region: A Disproportionality Analysis Using Complementary Characterization Probes" Molecules 29, no. 16: 3948. https://doi.org/10.3390/molecules29163948
APA StyleShpotyuk, O., Hyla, M., Ingram, A., Shpotyuk, Y., Boyko, V., Demchenko, P., Wojnarowska-Nowak, R., Lukáčová Bujňáková, Z., & Baláž, P. (2024). Nanostructured Molecular–Network Arsenoselenides from the Border of a Glass-Forming Region: A Disproportionality Analysis Using Complementary Characterization Probes. Molecules, 29(16), 3948. https://doi.org/10.3390/molecules29163948








