Prediction of Two-Dimensional Janus Transition-Metal Chalcogenides: Robust Ferromagnetic Semiconductor with High Curie Temperature
Abstract
1. Introduction
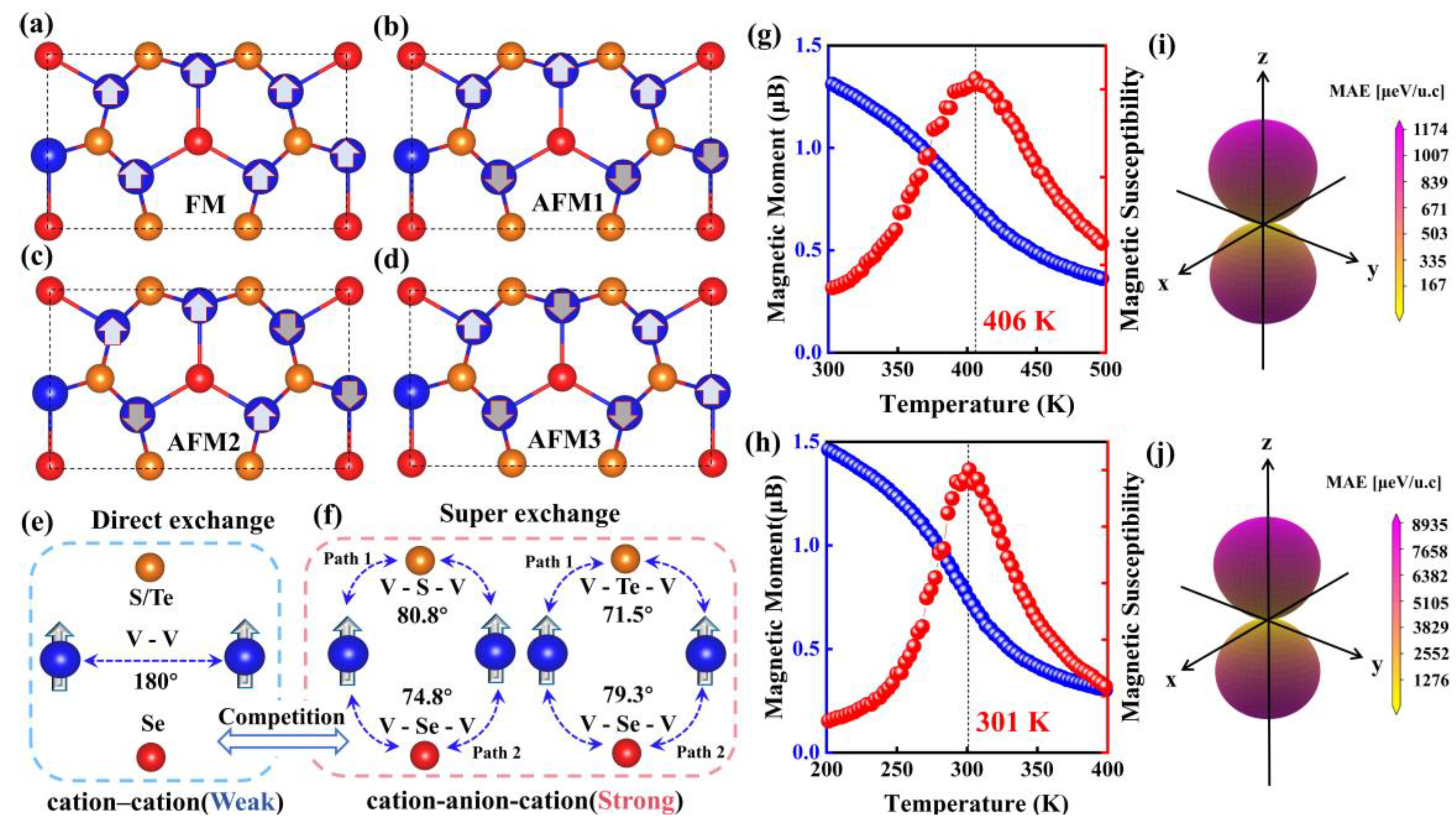
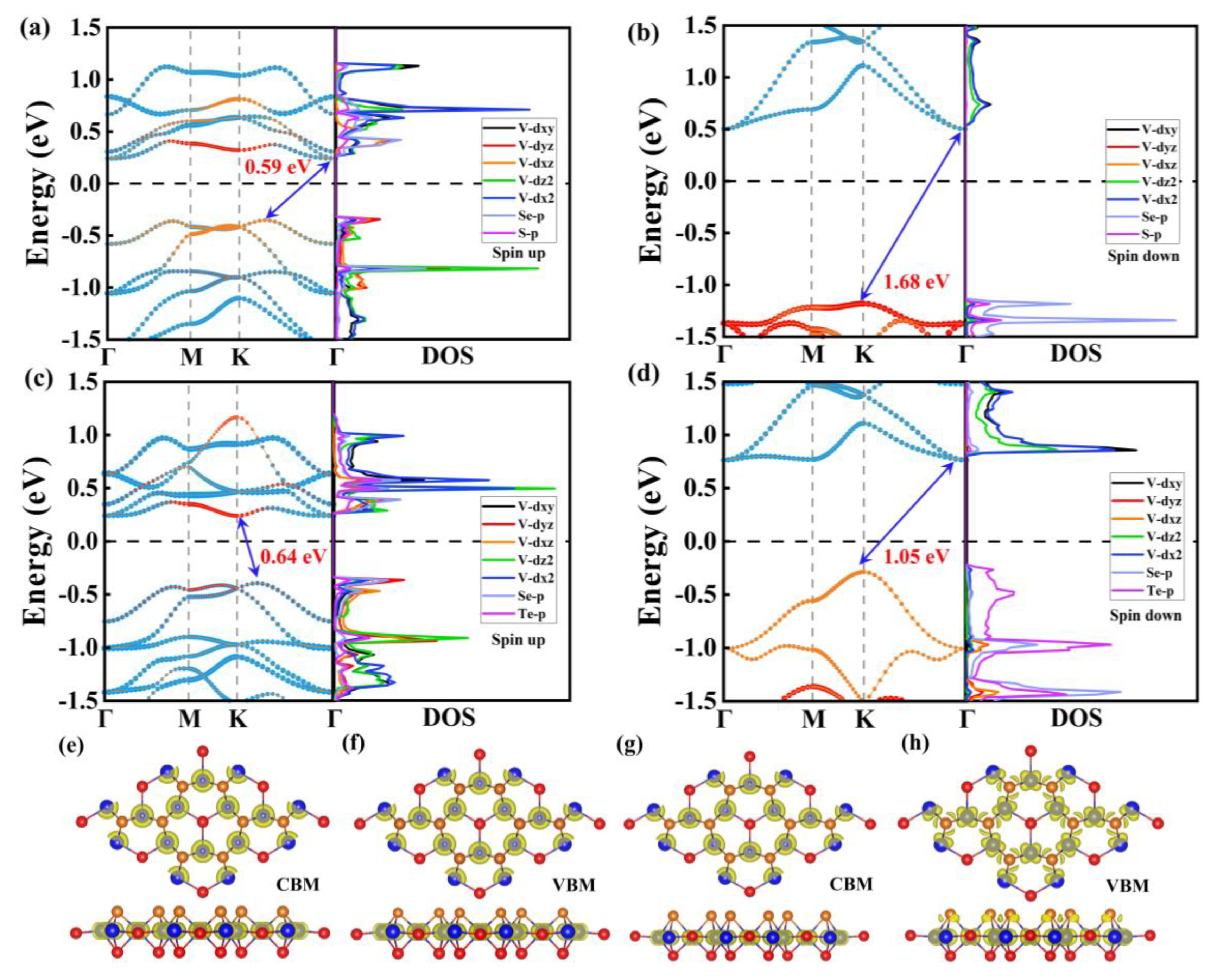
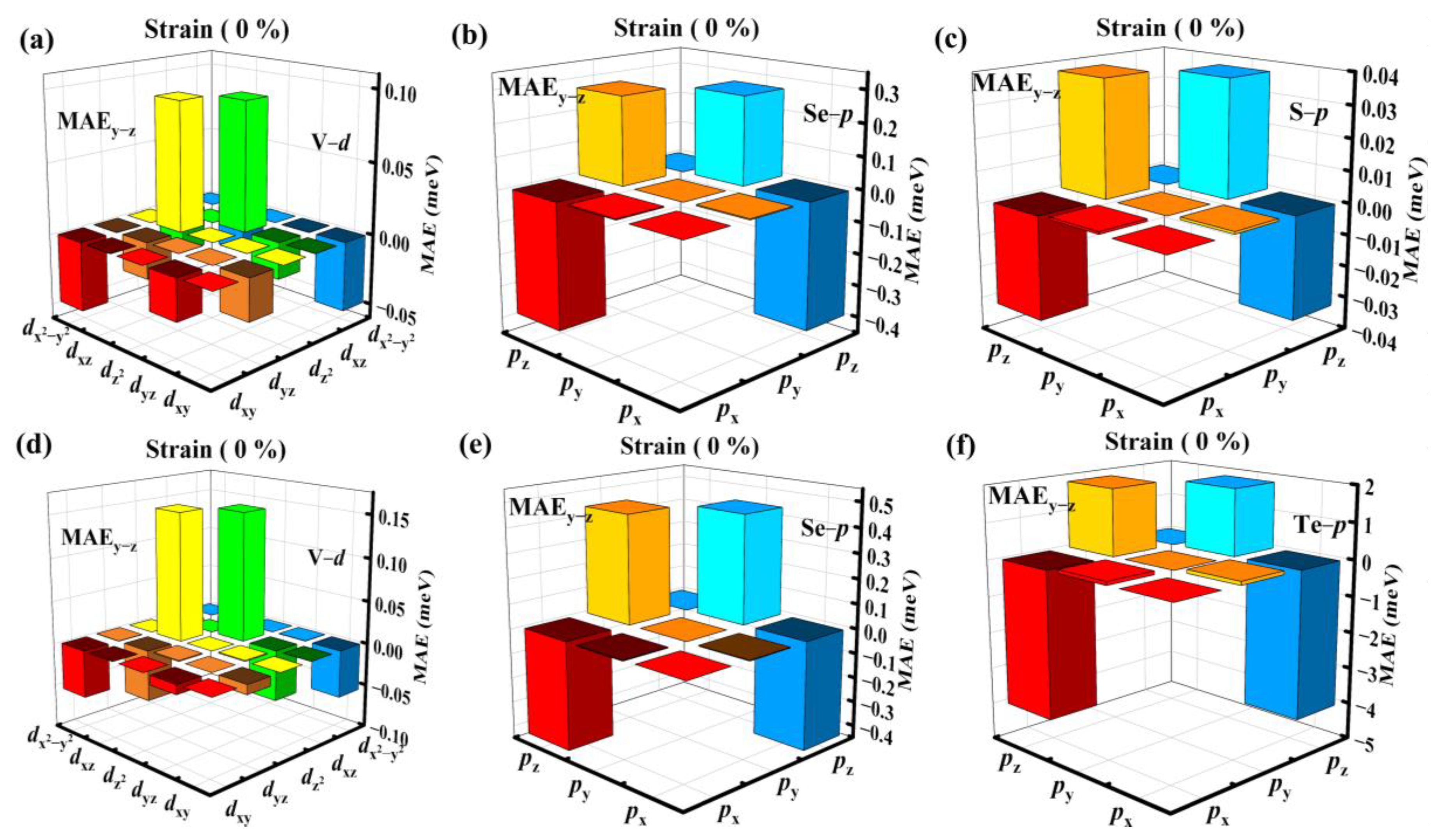
2. Results and Discussion
3. Computational Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef] [PubMed]
- Jiang, S.; Li, L.; Wang, Z.; Shan, J.; Mak, K.F. Spin tunnel field-effect transistors based on two-dimensional van der Waals heterostructures. Nat. Electron. 2019, 2, 159–163. [Google Scholar] [CrossRef]
- Lembke, D.; Bertolazzi, S.; Kis, A. Single-Layer MoS2 Electronics. Acc. Chem. Res. 2015, 48, 100–110. [Google Scholar] [CrossRef]
- Li, L.; Yu, Y.; Ye, G.J.; Ge, Q.; Ou, X.; Wu, H.; Feng, D.; Chen, X.H.; Zhang, Y. Black phosphorus field-effect transistors. Nat. Nanotechnol. 2014, 9, 372–377. [Google Scholar] [CrossRef]
- Li, X.; Wu, X. Two-dimensional monolayer designs for spintronics applications. WIREs Comput. Mol. Sci. 2016, 6, 441–455. [Google Scholar] [CrossRef]
- Liu, S.; Yin, H.; Singh, D.J.; Liu, P.-F. Ta4SiTe4: A possible one-dimensional topological insulator. Phys. Rev. B 2022, 105, 195419. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Jiang, L.; Marconcini, P.; Hossian, M.S.; Qiu, W.; Evans, R.; Macucci, M.; Skafidas, E. A tight binding and study of monolayer stanene. Sci. Rep. 2017, 7, 12069. [Google Scholar]
- Chappert, C.; Fert, A.; Van Dau, F.N. The emergence of spin electronics in data storage. Nat. Mater. 2007, 6, 813–823. [Google Scholar] [CrossRef] [PubMed]
- Boeck, J.D.; Roy, W.V.; Das, J.; Motsnyi, V.; Liu, Z.; Lagae, L.; Boeve, H.; Dessein, K.; Borghs, G. Technology and materials issues in semiconductor-based magnetoelectronics. Semicond. Sci. Technol. 2002, 17, 342. [Google Scholar] [CrossRef]
- Tomasz, D. Ferromagnetic semiconductors. Semicond. Sci. Technol. 2002, 17, 377. [Google Scholar]
- Jansen, R. Silicon spintronics. Nat. Mater. 2012, 11, 400–408. [Google Scholar] [CrossRef] [PubMed]
- Xiao, G.; Xiao, W.-Z.; Chen, Q.; Wang, L.-L. Novel two-dimensional ferromagnetic materials CrX2 (X = O, S, Se) with high Curie temperature. J. Mater. Chem. C 2022, 10, 17665–17674. [Google Scholar] [CrossRef]
- Ohno, H. Making Nonmagnetic Semiconductors Ferromagnetic. Science 1998, 281, 951–956. [Google Scholar] [CrossRef]
- Bai, Y.; Shi, R.; Wu, Y.; Wang, B.; Zhang, X. Cr2XTe4 (X = Si, Ge) monolayers: A new type of two-dimensional high-TC Ising ferromagnetic semiconductors with a large magnetic anisotropy. J. Phys. Condens. Matter 2022, 34, 384001. [Google Scholar] [CrossRef]
- Mermin, N.D.; Wagner, H. Absence of Ferromagnetism or Antiferromagnetism in One- or Two-Dimensional Isotropic Heisenberg Models. Phys. Rev. Lett. 1966, 17, 1133–1136. [Google Scholar] [CrossRef]
- Gong, C.; Li, L.; Li, Z.; Ji, H.; Stern, A.; Xia, Y.; Cao, T.; Bao, W.; Wang, C.; Wang, Y.; et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature 2017, 546, 265–269. [Google Scholar] [CrossRef]
- Huang, B.; Clark, G.; Navarro-Moratalla, E.; Klein, D.R.; Cheng, R.; Seyler, K.L.; Zhong, D.; Schmidgall, E.; McGuire, M.A.; Cobden, D.H.; et al. Layer-dependent ferromagnetism in a van der Waals crystal down to the monolayer limit. Nature 2017, 546, 270–273. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.H.; Kalantar-Zadeh, K.; Kis, A.; Coleman, J.N.; Strano, M.S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 2012, 7, 699–712. [Google Scholar] [CrossRef]
- Zhang, C.; Nie, Y.; Sanvito, S.; Du, A. First-Principles Prediction of a Room-Temperature Ferromagnetic Janus VSSe Monolayer with Piezoelectricity, Ferroelasticity, and Large Valley Polarization. Nano Lett. 2019, 19, 1366–1370. [Google Scholar] [CrossRef]
- Hu, T.; Jia, F.; Zhao, G.; Wu, J.; Stroppa, A.; Ren, W. Intrinsic and anisotropic Rashba spin splitting in Janus transition-metal dichalcogenide monolayers. Phys. Rev. B 2018, 97, 235404. [Google Scholar] [CrossRef]
- An, M.; Zhang, Y.; Chen, J.; Zhang, H.-M.; Guo, Y.; Dong, S. Tuning Magnetism in Layered Magnet VI3: A Theoretical Study. J. Phys. Chem. C 2019, 123, 30545–30550. [Google Scholar] [CrossRef]
- Tian, Y.; Gray, M.J.; Ji, H.; Cava, R.J.; Burch, K.S. Magneto-elastic coupling in a potential ferromagnetic 2D atomic crystal. 2D Mater. 2016, 3, 025035. [Google Scholar] [CrossRef]
- Lu, A.-Y.; Zhu, H.; Xiao, J.; Chuu, C.-P.; Han, Y.; Chiu, M.-H.; Cheng, C.-C.; Yang, C.-W.; Wei, K.-H.; Yang, Y.; et al. Janus monolayers of transition metal dichalcogenides. Nat. Nanotechnol. 2017, 12, 744–749. [Google Scholar] [CrossRef]
- Li, R.; Jiang, J.; Shi, X.; Mi, W.; Bai, H. Two-Dimensional Janus FeXY (X, Y = Cl, Br, and I, X ≠ Y) Monolayers: Half-Metallic Ferromagnets with Tunable Magnetic Properties under Strain. ACS Appl. Mater. Interfaces 2021, 13, 38897–38905. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Fan, Q.; Liu, Y.; Yao, G. Electrically tunable magnetism and unique intralayer charge transfer in Janus monolayer MnSSe for spintronics applications. Phys. Rev. B 2022, 105, 195410. [Google Scholar] [CrossRef]
- Sun, X.; Liu, X.; Yin, J.; Yu, J.; Li, Y.; Hang, Y.; Zhou, X.; Yu, M.; Li, J.; Tai, G.; et al. Two-Dimensional Boron Crystals: Structural Stability, Tunable Properties, Fabrications and Applications. Adv. Funct. Mater. 2017, 27, 1603300. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, B.; Guo, Y.; Zhang, Y.; Chen, Y.; Wang, J. High Curie temperature and intrinsic ferromagnetic half-metallicity in two-dimensional Cr3X4 (X = S, Se, Te) nanosheets. Nanoscale Horiz. 2019, 4, 859–866. [Google Scholar] [CrossRef]
- Anderson, P.W. New Approach to the Theory of Superexchange Interactions. Phys. Rev. 1959, 115, 2–13. [Google Scholar] [CrossRef]
- Goodenough, J.B. Theory of the Role of Covalence in the Perovskite-Type Manganites [La, M(II)]MnO3. Phys. Rev. 1955, 100, 564–573. [Google Scholar] [CrossRef]
- Li, X.; Wu, X.; Li, Z.; Yang, J.; Hou, J.G. Bipolar magnetic semiconductors: A new class of spintronics materials. Nanoscale 2012, 4, 5680–5685. [Google Scholar] [CrossRef]
- Zhao, C.-X.; Liu, J.-N.; Li, B.-Q.; Ren, D.; Chen, X.; Yu, J.; Zhang, Q. Multiscale Construction of Bifunctional Electrocatalysts for Long-Lifespan Rechargeable Zinc–Air Batteries. Adv. Funct. Mater. 2020, 30, 2003619. [Google Scholar] [CrossRef]
- Zhong, C.; Wu, W.; He, J.; Ding, G.; Liu, Y.; Li, D.; Yang, S.A.; Zhang, G. Two-dimensional honeycomb borophene oxide: Strong anisotropy and nodal loop transformation. Nanoscale 2019, 11, 2468–2475. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, X.; Zhang, Y.; Yuan, S.; Guo, Y.; Dong, S.; Wang, J. Prediction of a two-dimensional high-TC f-electron ferromagnetic semiconductor. Mater. Horiz. 2020, 7, 1623–1630. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, Y.; Ma, L.; Wu, Q.; Guo, Y.; Zhang, X.; Wang, J. MnX (X = P, As) monolayers: A new type of two-dimensional intrinsic room temperature ferromagnetic half-metallic material with large magnetic anisotropy. Nanoscale 2019, 11, 4204–4209. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Zhang, Y.; Yuan, S.; Wang, B.; Wang, J. Chromium sulfide halide monolayers: Intrinsic ferromagnetic semiconductors with large spin polarization and high carrier mobility. Nanoscale 2018, 10, 18036–18042. [Google Scholar] [CrossRef]
- Zhang, Z.; Shang, J.; Jiang, C.; Rasmita, A.; Gao, W.; Yu, T. Direct Photoluminescence Probing of Ferromagnetism in Monolayer Two-Dimensional CrBr3. Nano Lett. 2019, 19, 3138–3142. [Google Scholar] [CrossRef]
- Cai, X.; Song, T.; Wilson, N.P.; Clark, G.; He, M.; Zhang, X.; Taniguchi, T.; Watanabe, K.; Yao, W.; Xiao, D.; et al. Atomically Thin CrCl3: An In-Plane Layered Antiferromagnetic Insulator. Nano Lett. 2019, 19, 3993–3998. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Wang, L.; Maxisch, T.; Ceder, G. Oxidation energies of transition metal oxides within the GGA + U framework. Phys. Rev. B 2006, 73, 195107. [Google Scholar] [CrossRef]
- Jain, A.; Hautier, G.; Ong, S.P.; Moore, C.J.; Fischer, C.C.; Persson, K.A.; Ceder, G. Formation enthalpies by mixing GGA and GGA + U calculations. Phys. Rev. B 2011, 84, 045115. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef]
- Rezaei, N.; Alaei, M.; Akbarzadeh, H. ESpinS: A program for classical Monte-Carlo simulations of spin systems. Comput. Mater. Sci. 2022, 202, 110947. [Google Scholar] [CrossRef]
- Togo, A.; Oba, F.; Tanaka, I. First-principles calculations of the ferroelastic transition between rutile-type and CaCl2-type SiO at high pressures. Phys. Rev. B 2008, 78, 134106. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Qureshi, A.H.; Duan, Y.; Liu, Y.; Wang, Y.; Zhu, J.; Lu, J.; Guo, T.; Liu, Y.; Zhang, X. Prediction of Two-Dimensional Janus Transition-Metal Chalcogenides: Robust Ferromagnetic Semiconductor with High Curie Temperature. Molecules 2024, 29, 3915. https://doi.org/10.3390/molecules29163915
Wang Z, Qureshi AH, Duan Y, Liu Y, Wang Y, Zhu J, Lu J, Guo T, Liu Y, Zhang X. Prediction of Two-Dimensional Janus Transition-Metal Chalcogenides: Robust Ferromagnetic Semiconductor with High Curie Temperature. Molecules. 2024; 29(16):3915. https://doi.org/10.3390/molecules29163915
Chicago/Turabian StyleWang, Zijin, Ali Hamza Qureshi, Yuanyuan Duan, Yujie Liu, Yanbiao Wang, Jun Zhu, Jinlian Lu, Tianxia Guo, Yongjun Liu, and Xiuyun Zhang. 2024. "Prediction of Two-Dimensional Janus Transition-Metal Chalcogenides: Robust Ferromagnetic Semiconductor with High Curie Temperature" Molecules 29, no. 16: 3915. https://doi.org/10.3390/molecules29163915
APA StyleWang, Z., Qureshi, A. H., Duan, Y., Liu, Y., Wang, Y., Zhu, J., Lu, J., Guo, T., Liu, Y., & Zhang, X. (2024). Prediction of Two-Dimensional Janus Transition-Metal Chalcogenides: Robust Ferromagnetic Semiconductor with High Curie Temperature. Molecules, 29(16), 3915. https://doi.org/10.3390/molecules29163915





