Mixed-Mode Adsorption of l-Tryptophan on D301 Resin through Hydrophobic Interaction/Ion Exchange/Ion Exclusion: Equilibrium and Kinetics Study
Abstract
1. Introduction
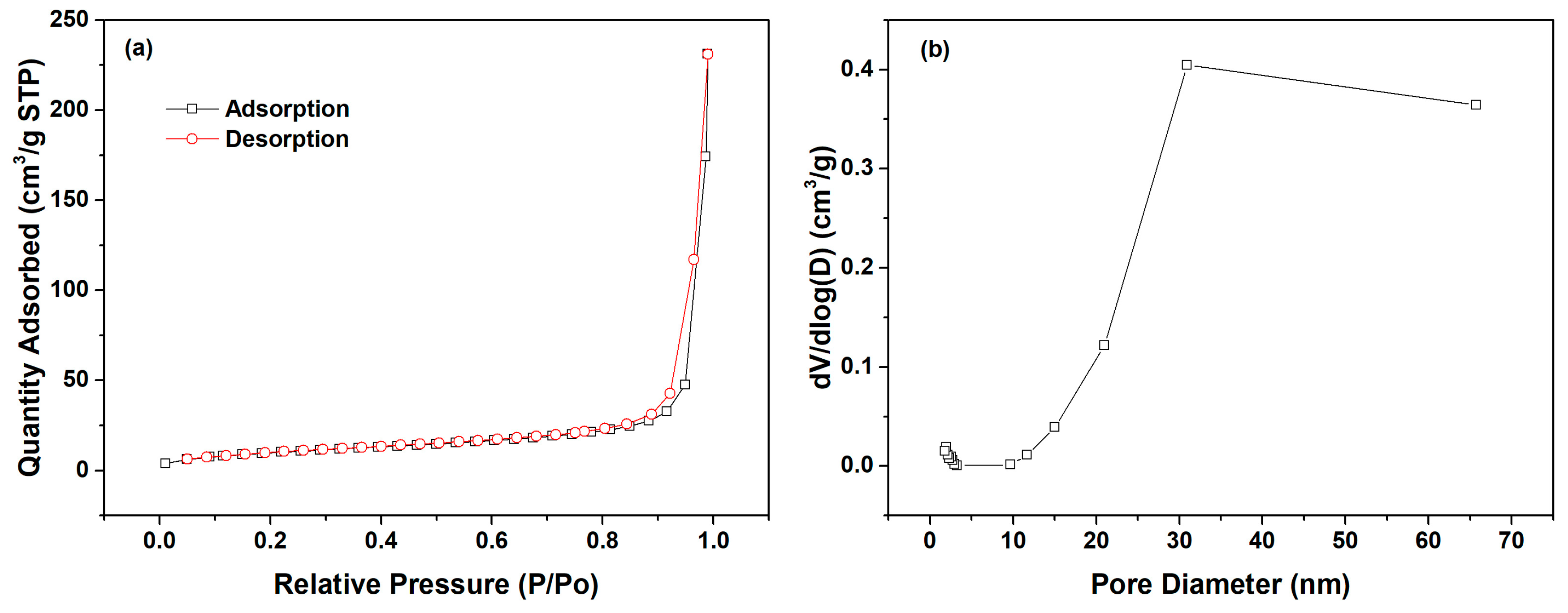
2. Results and Discussion
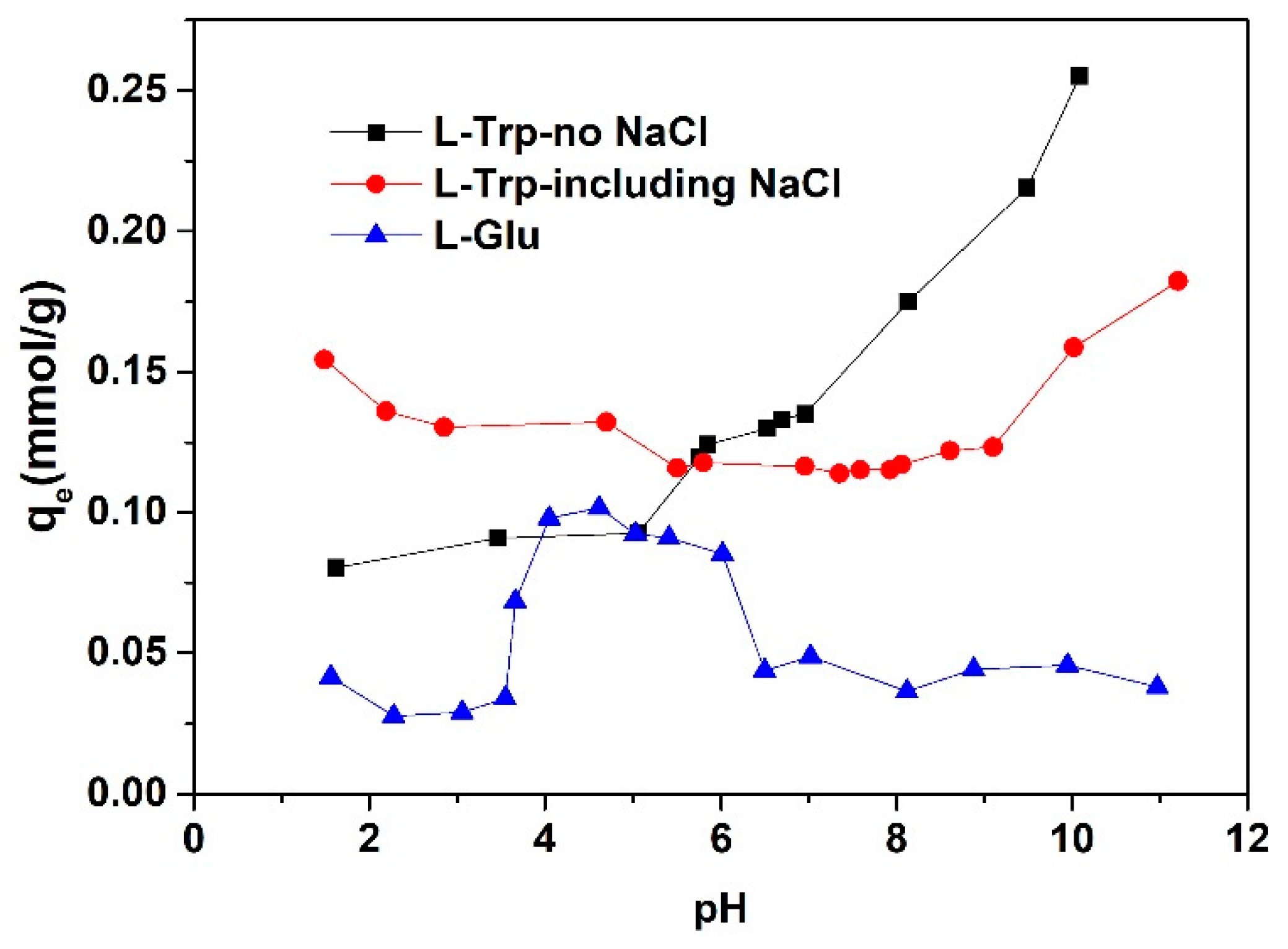
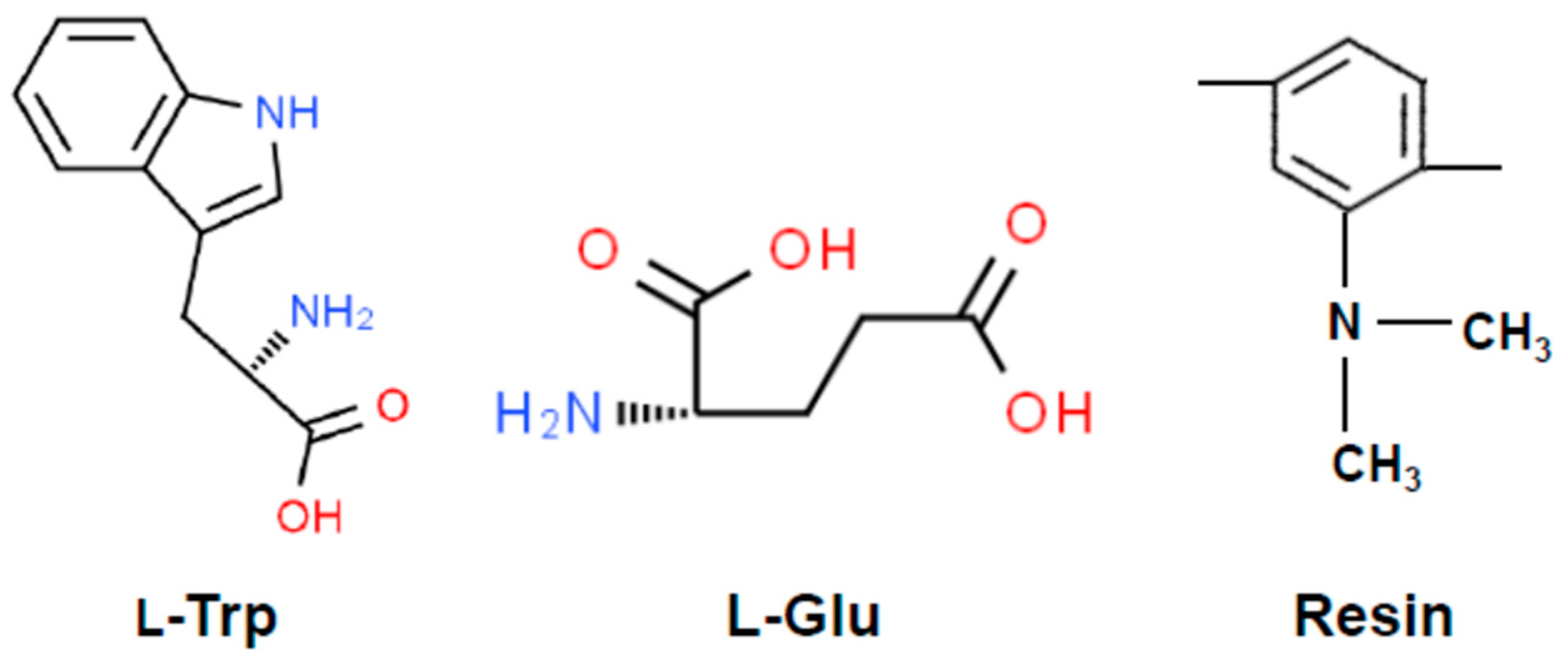
2.1. Influence of pH on Adsorption Capacity towards L-Trp and L-Glu
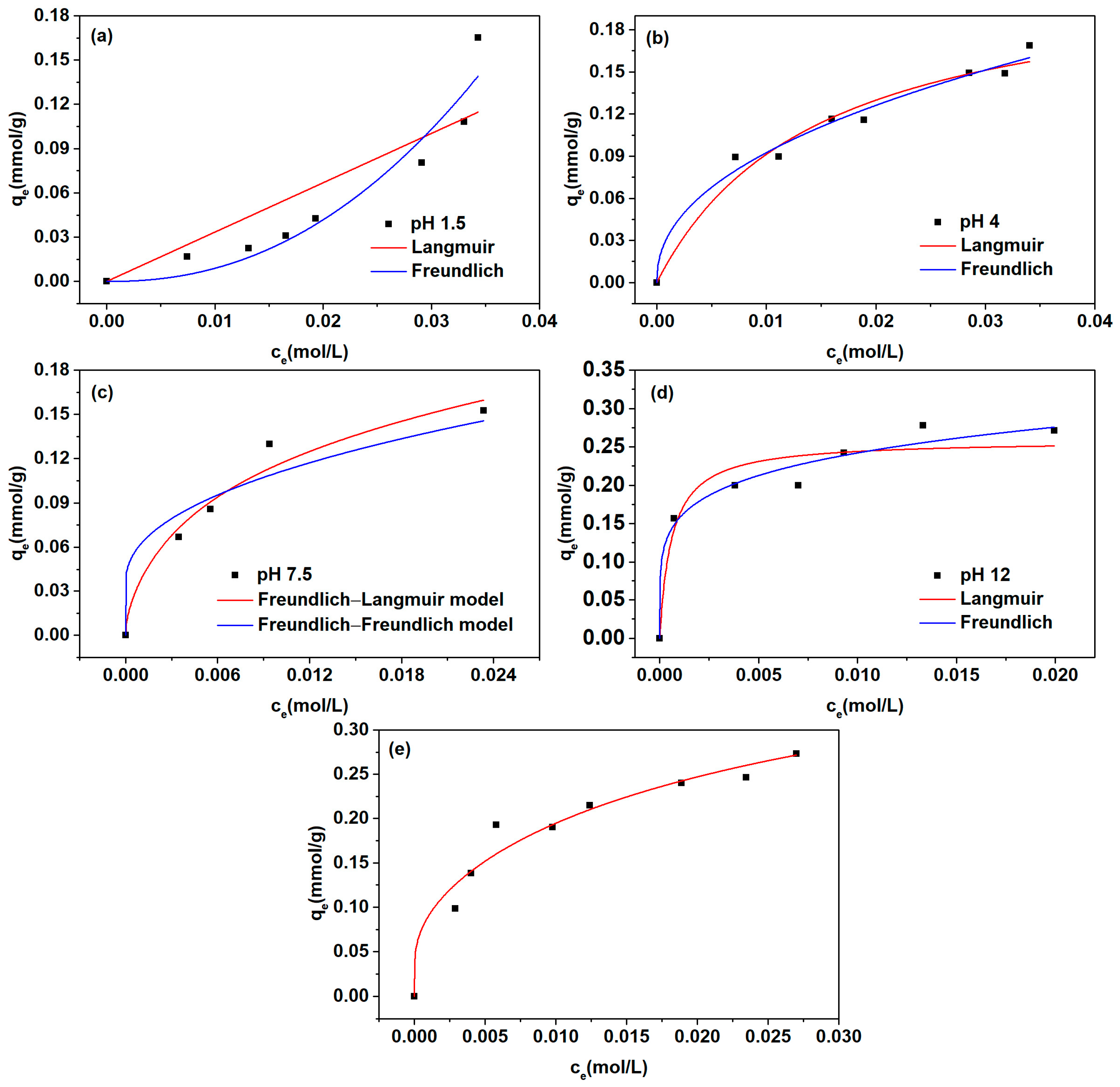
2.2. Adsorption Equilibrium Behaviors of l-Trp
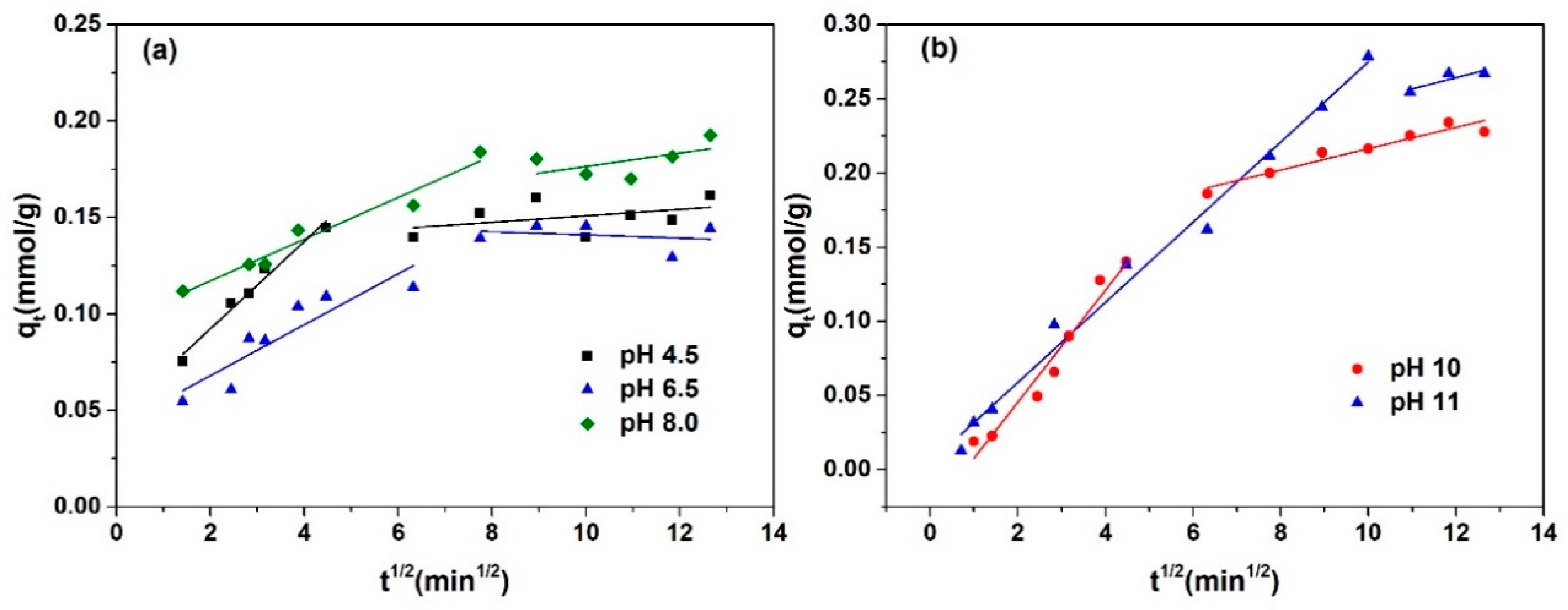
2.3. Adsorption Kinetic Behavior Investigation
3. Materials and Methods
3.1. Adsorbent
3.2. Chemicals
3.3. Influence of pH Variations on the Adsorption Amount
3.4. Adsorption Isotherm Measurement
3.5. Adsorption Kinetics Curve Determination
3.6. Analytical Method
4. Theory
4.1. Adsorption Isotherm Model
4.2. ILM
4.3. Intra-Particle Diffusion Model
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, J.; Luo, G.; Li, Z.; Li, H.; Qiu, H. A new strategy for the preparation of mixed-mode chromatographic stationary phases based on modified dialdehyde cellulose. J. Chromatogr. A 2020, 1618, 460885–460895. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Zhang, Q.; Lin, D.; Yao, S. Development and application of hydrophobic charge-induction chromatography for bioseparation. J. Chromatogr. B 2019, 1134–1135, 121850–121854. [Google Scholar] [CrossRef] [PubMed]
- Zimmermann, A.; Greco, R.; Walker, I.; Horak, J.; Cavazzini, A.; LäMmerhofer, M. Synthetic oligonucleotide separations by mixed-mode reversed-phase/weak anion-exchange liquid chromatography. J. Chromatogr. A 2014, 1354, 43–55. [Google Scholar] [CrossRef] [PubMed]
- Biba, M.; Jiang, E.; Mao, B.; Zewge, D.; Foley, J.P.; Welch, C.J. Factors influencing the separation of oligonucleotides using reversed-phase/ion-exchange mixed-mode high performance liquid chromatography columns. J. Chromatogr. A 2013, 1304, 69–77. [Google Scholar] [CrossRef] [PubMed]
- Jin, H.; Liu, Y.; Guo, Z.; Yang, F.; Wang, J.; Li, X.; Peng, X.; Liang, X. High-Performance Liquid Chromatography Separation of cis–trans Anthocyanin Isomers from Wild Lycium ruthenicum Murr. Employing a Mixed-Mode Reversed-Phase/Strong Anion-Exchange Stationary Phase. J. Agric. Food Chem. 2015, 63, 500–508. [Google Scholar] [CrossRef]
- Cai, X.; Guo, Z.; Xue, X.; Xu, J.; Zhang, X.; Liang, X. Two-dimensional liquid chromatography separation of peptides using reversed-phase/weak cation-exchange mixed-mode column in first dimension. J. Chromatogr. A 2012, 1228, 242–249. [Google Scholar] [CrossRef] [PubMed]
- Shen, G.; Zhang, F.F.; Yang, B.; Chu, C.; Liang, X. A novel amide stationary phase for hydrophilic interaction liquid chromatography and ion chromatography. Talanta 2013, 115, 129–132. [Google Scholar] [CrossRef] [PubMed]
- Qiao, L.; Wang, S.; Li, H.; Shan, Y.; Dou, A.; Shi, X.; Xu, G. A novel surface-confined glucaminium-based ionic liquid stationary phase for hydrophilic interaction/anion-exchange mixed-mode chromatography. J. Chromatogr. A 2014, 1360, 240–247. [Google Scholar] [CrossRef]
- Chester, T.L. The combination of partition, size exclusion, and hydrodynamic models in chromatography, and application to bonded phases on porous supports. J. Chromatogr. A 2020, 1620, 461011–461019. [Google Scholar] [CrossRef]
- Liu, X.; Pohl, C.; Woodruff, A.; Chen, J. Chromatographic evaluation of reversed-phase/anion-exchange/cation-exchange trimodal stationary phases prepared by electrostatically driven self-assembly process. J. Chromatogr. A 2011, 1218, 3407–3412. [Google Scholar] [CrossRef]
- Wang, W.; Qi, M.; Jia, X.; Jin, J.; Zhou, Q.; Zhang, M.; Zhou, W.; Li, A. Differential adsorption of zwitterionic PPCPs by multifunctional resins: The influence of the hydrophobicity and electrostatic potential of PPCPs. Chemosphere 2020, 241, 125023. [Google Scholar] [CrossRef] [PubMed]
- Chen, K.-H.; Chou, S.-Y.; Chang, Y.-K. Rapid purification of lysozyme by mixed-mode adsorption chromatography in stirred fluidized bed. Food Chem. 2019, 272, 619–627. [Google Scholar] [CrossRef] [PubMed]
- Wu, Q.; Lin, D.; Shi, W.; Zhang, Q.; Yao, S. A mixed-mode resin with tryptamine ligand for human serum albumin separation. J. Chromatogr. A 2016, 1431, 145–153. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Zou, X.; Zhang, Q.; Lin, D.; Yao, S. Binding mechanism of functional moieties of a mixed-mode ligand in antibody purification. Chem. Eng. J. 2020, 400, 125887–125894. [Google Scholar] [CrossRef]
- Xiong, B.; Zhu, Y.; Tian, D.; Jiang, S.; Xie, X. Flux redistribution of central carbon metabolism for efficient production of L-tryptophan in Escherichia coli. Biotechnol. Bioeng. 2021, 118, 1393–1404. [Google Scholar] [CrossRef] [PubMed]
- Jiao, P.; Zhang, X.; Li, N.; Wei, Y.; Yang, P. Cooperative adsorption of L-tryptophan and sodium ion on a hyper-cross-linked resin: Experimental studies and mathematical modeling. J. Chromatogr. A 2021, 1648, 462211–462219. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.; Jing, K.; Lu, Y. Kinetics, equilibrium and thermodynamic studies of L-tryptophan adsorption using a cation exchange resin. Chem. Eng. J. 2011, 171, 1227–1233. [Google Scholar] [CrossRef]
- Luo, W.; Wei, P.; Chen, H.; Fan, L.; Huang, L.; Huang, J.; Xu, Z.; Cen, P. Kinetics and optimization of L-tryptophan separation with ion-exchange chromatography. Korean J. Chem. Eng. 2011, 28, 1280–1285. [Google Scholar] [CrossRef]
- Tao, Z.; Du, J.; Chu, T. Potentiometric titration in studies of ion exchangers. React. Funct. Polym. 1996, 31, 17–24. [Google Scholar] [CrossRef]
- Nasiri, A.; Rajabi, S.; Hashemi, M. CuCoFe2O4@MC/AC as a new hybrid magnetic nanocomposite for metronidazole removal from wastewater: Bioassay and toxicity of effluent. Sep. Purif. Technol. 2022, 296, 121366–121382. [Google Scholar] [CrossRef]
- Si, J.; Zhang, S.; Liu, X.; Fang, K. Flower-Shaped Ni/Co MOF with the Highest Adsorption Capacity for Reactive Dyes. Langmuir 2022, 38, 6004–6012. [Google Scholar] [CrossRef] [PubMed]
- Weber, W.J.; Morris, J.C. Kinetics of adsorption on carbon from solution. J. Sanit. Eng. Div. 1963, 89, 31–60. [Google Scholar] [CrossRef]

| Langmuir Model | Freundlich Model | |||||
|---|---|---|---|---|---|---|
| qmax (mmol/g) | KL (L/mol) | R2 | KF (mmol1−1/nL1/n/g) | n | R2 | |
| l-Trp±-R+ | 0.23 | 68.08 | 0.966 | 0.73 | 2.23 | 0.981 |
| l-Trp−-R | 0.26 | 1660.50 | 0.920 | 0.57 | 5.33 | 0.971 |
| l-Trp±-R | 0.32 | 230.64 | 0.963 | 0.19 | 8.69 × 10−10 | 0.925 |
| l-Trp+-R+ | 789.10 | 4.23 × 10−3 | 0.763 | 255.87 | 0.45 | 0.918 |
| pH | 4.5 | 6.5 | 8.0 | 10.0 | 11.0 |
|---|---|---|---|---|---|
| keff (cm/min) | 6.0 × 10−3 | 7.5 × 10−3 | 7.5 × 10−3 | 3.7 × 10−3 | 2.2 × 10−3 |
| ARD% | 5.8% | 4.6% | 6.2% | 5.4% | 6.7% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Jiao, P.; Zhang, Z.; Niu, Q. Mixed-Mode Adsorption of l-Tryptophan on D301 Resin through Hydrophobic Interaction/Ion Exchange/Ion Exclusion: Equilibrium and Kinetics Study. Molecules 2024, 29, 3745. https://doi.org/10.3390/molecules29163745
Wang S, Jiao P, Zhang Z, Niu Q. Mixed-Mode Adsorption of l-Tryptophan on D301 Resin through Hydrophobic Interaction/Ion Exchange/Ion Exclusion: Equilibrium and Kinetics Study. Molecules. 2024; 29(16):3745. https://doi.org/10.3390/molecules29163745
Chicago/Turabian StyleWang, Shengping, Pengfei Jiao, Zhengtian Zhang, and Qiuhong Niu. 2024. "Mixed-Mode Adsorption of l-Tryptophan on D301 Resin through Hydrophobic Interaction/Ion Exchange/Ion Exclusion: Equilibrium and Kinetics Study" Molecules 29, no. 16: 3745. https://doi.org/10.3390/molecules29163745
APA StyleWang, S., Jiao, P., Zhang, Z., & Niu, Q. (2024). Mixed-Mode Adsorption of l-Tryptophan on D301 Resin through Hydrophobic Interaction/Ion Exchange/Ion Exclusion: Equilibrium and Kinetics Study. Molecules, 29(16), 3745. https://doi.org/10.3390/molecules29163745





