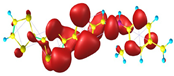
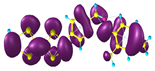
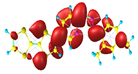
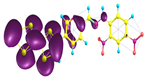
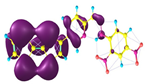
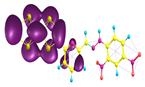
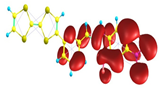
Figure 1.
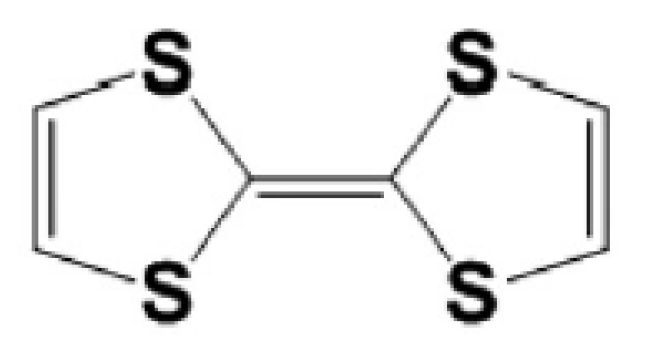
Chemical structure of the TTF moiety.
Figure 1.
Chemical structure of the TTF moiety.
Figure 2.
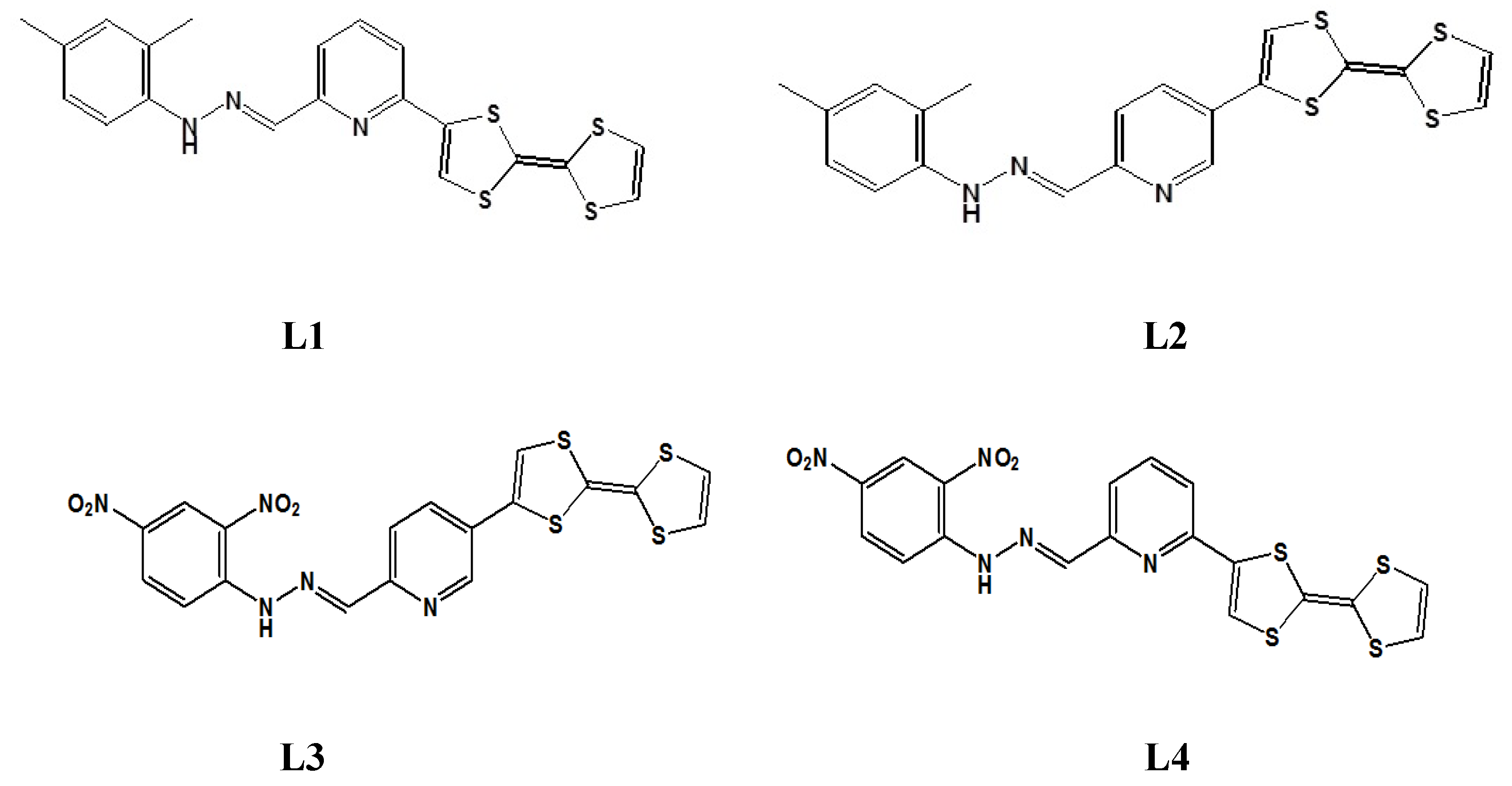
The structures of four investigated TTF-attended azine derivatives (LX) named L1, L2, L3, and L4.
Figure 2.
The structures of four investigated TTF-attended azine derivatives (LX) named L1, L2, L3, and L4.
Figure 3.
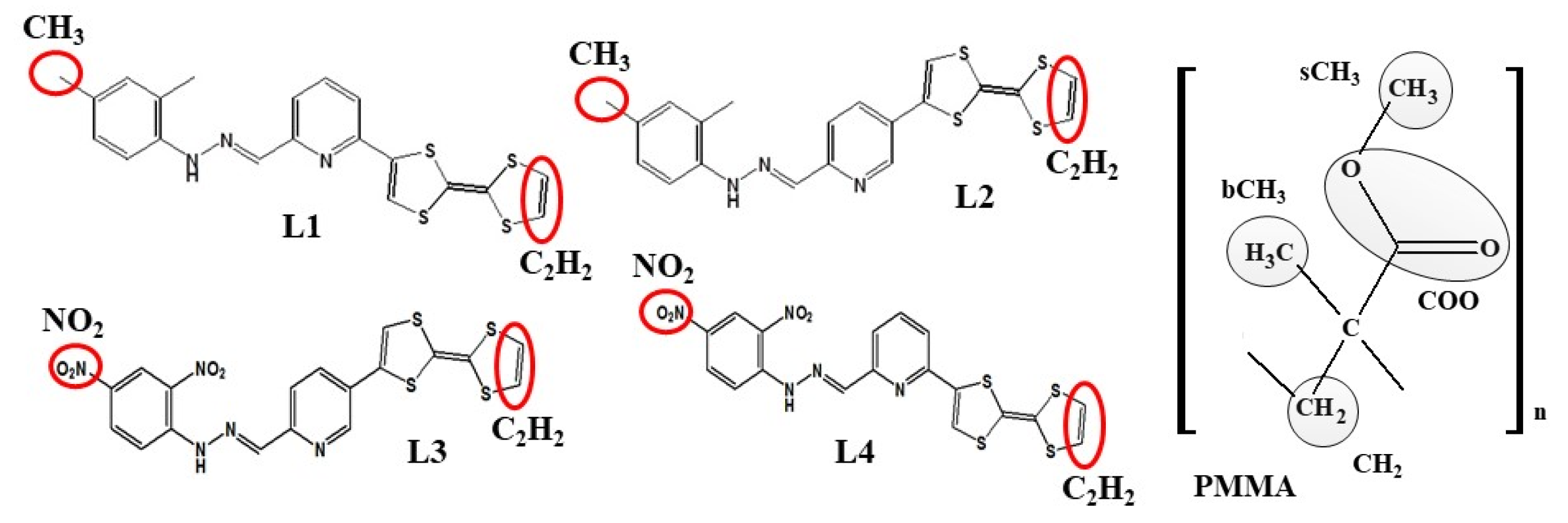
The structures of four investigated LX chromophores and mer of PMMA with marked groups of atoms used to construct the RDF function.
Figure 3.
The structures of four investigated LX chromophores and mer of PMMA with marked groups of atoms used to construct the RDF function.
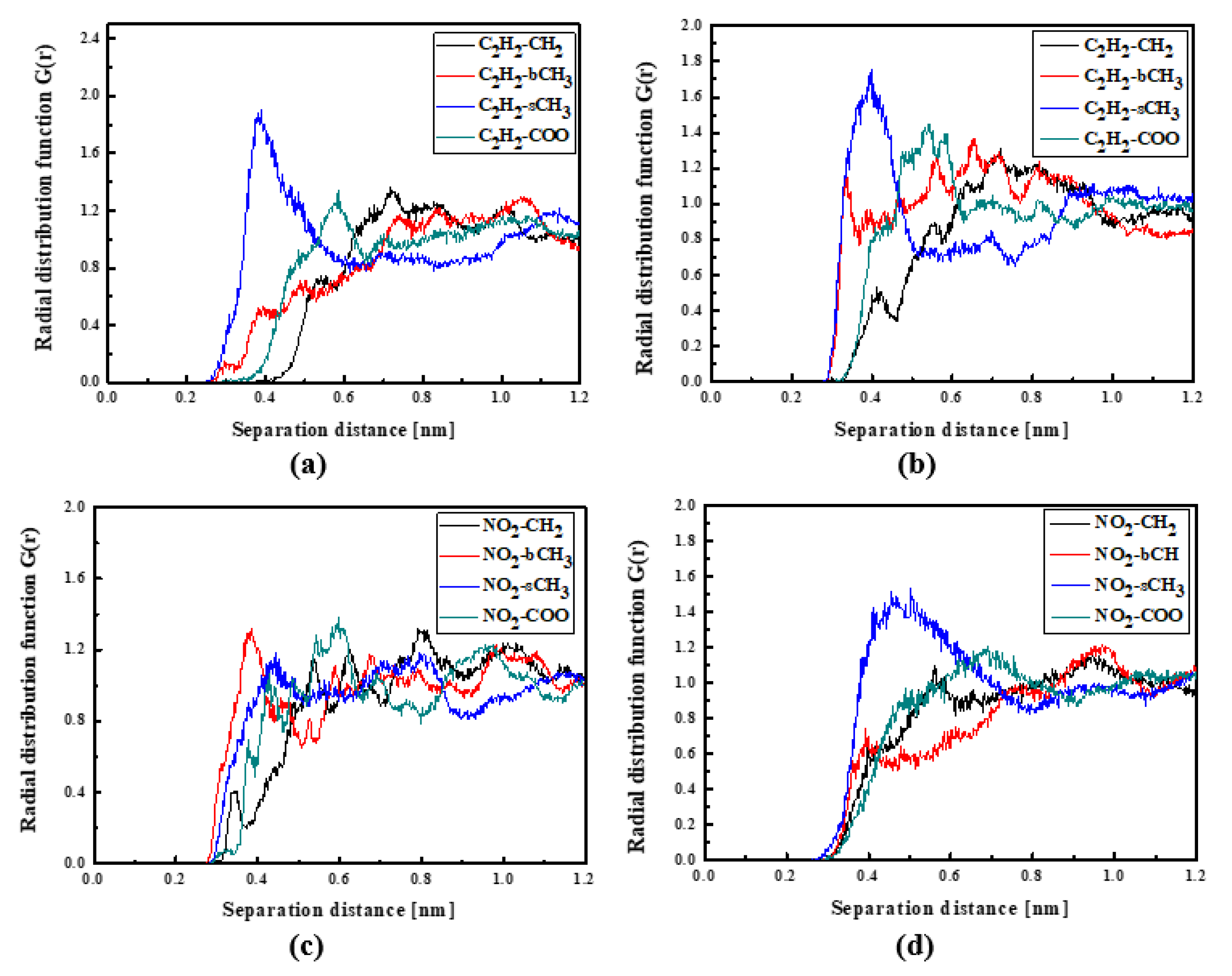
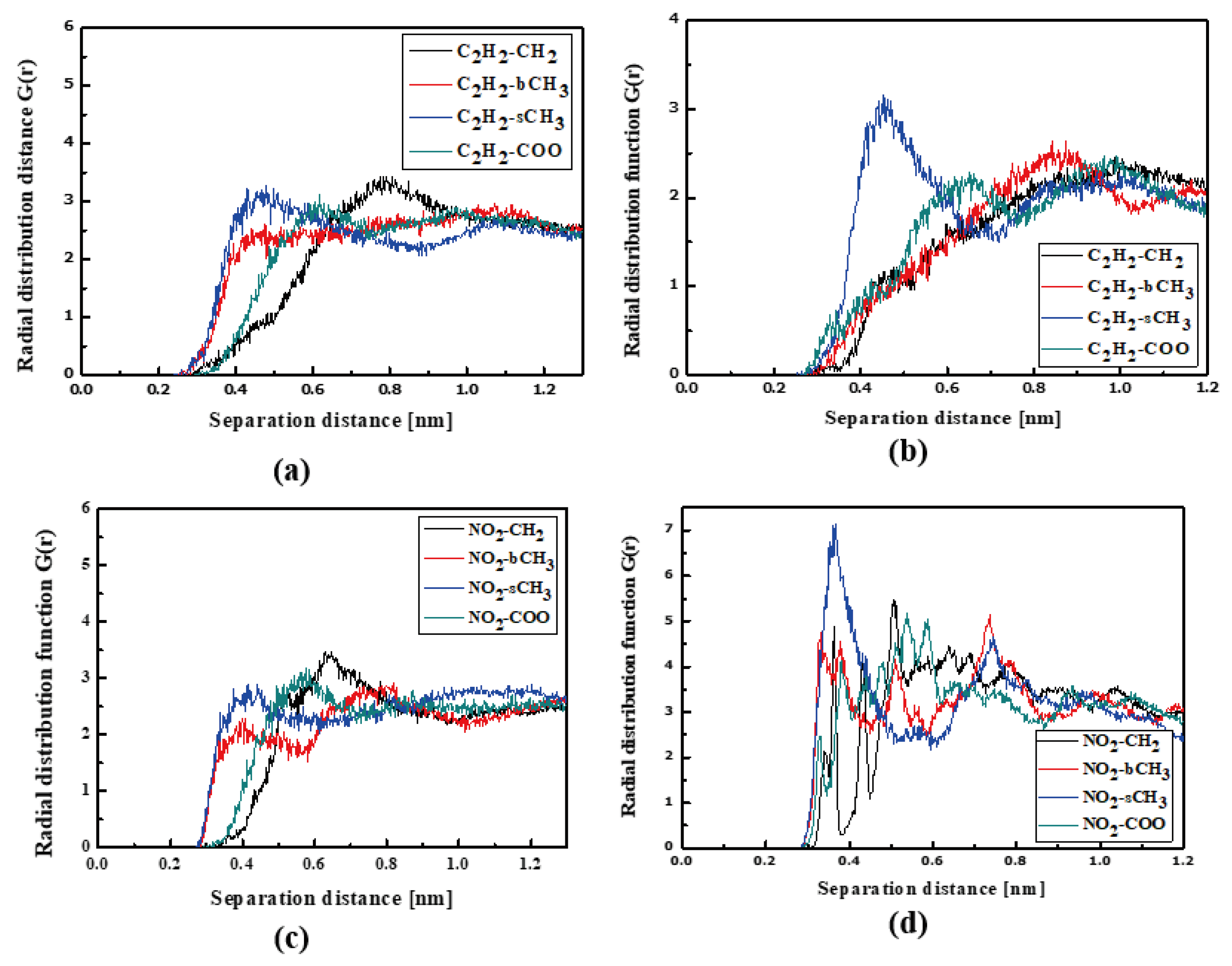
Figure 4.
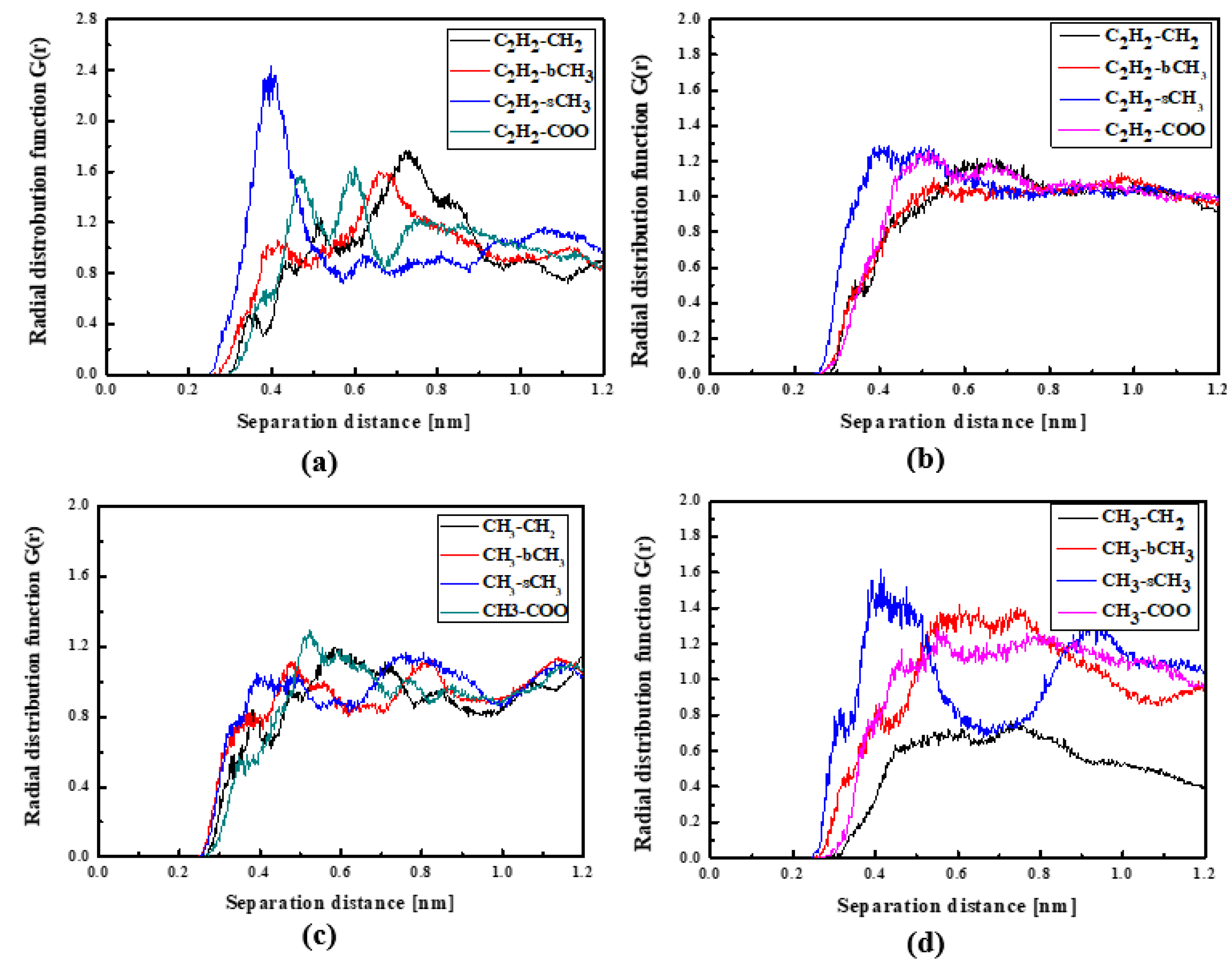
Partial RDFs calculated for distances between the center of mass of different moieties of the L1 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L1/PMMA system. Panels (a,b) present the RDFs for C2H2 of L1 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs for CH3 of L1 and different subunits of PMMA mer.
Figure 4.
Partial RDFs calculated for distances between the center of mass of different moieties of the L1 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L1/PMMA system. Panels (a,b) present the RDFs for C2H2 of L1 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs for CH3 of L1 and different subunits of PMMA mer.
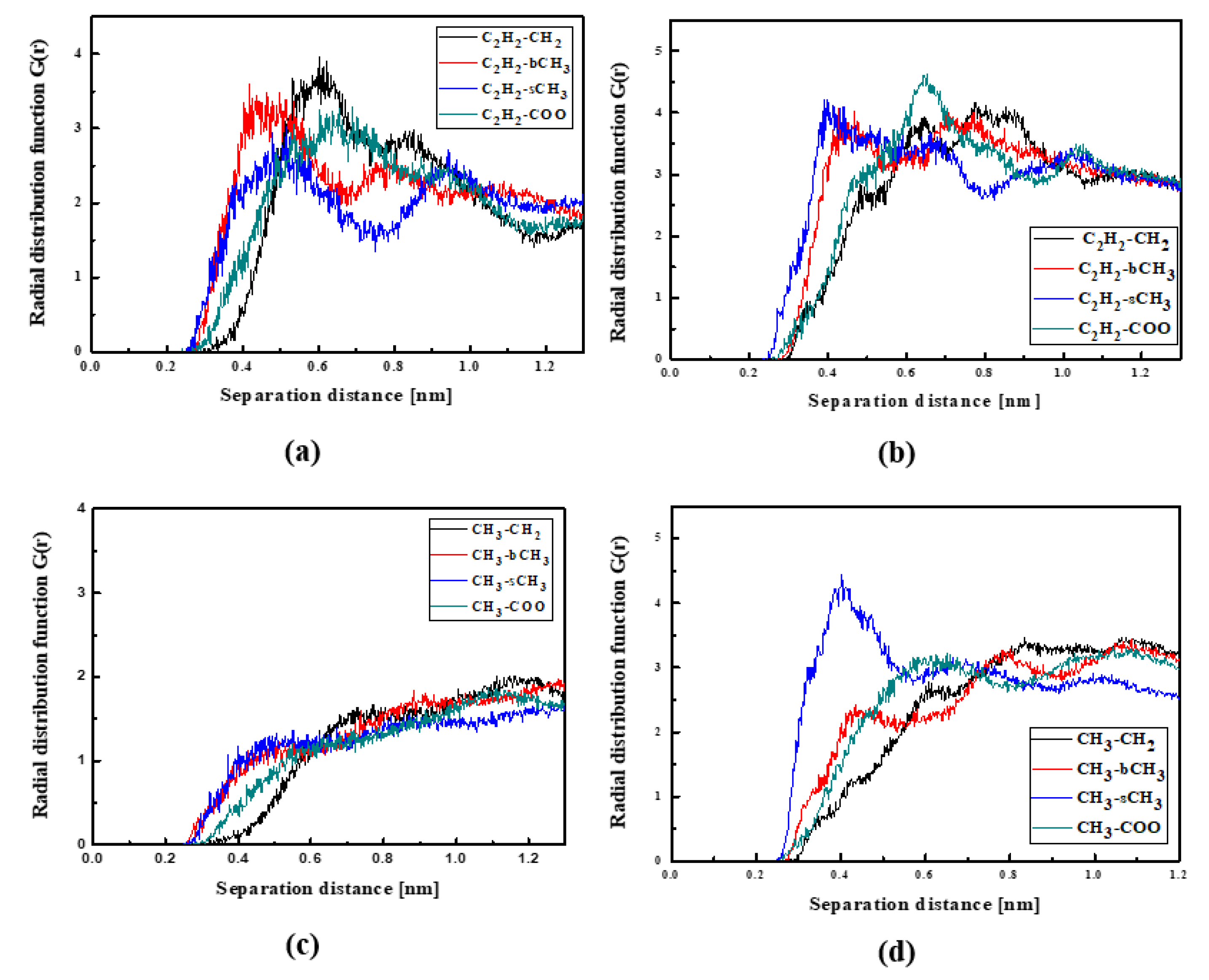
Figure 5.
Partial RDFs calculated for distances between the center of mass of different moieties of the L2 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L2/PMMA system. Panels (a,b) present the RDFs for C2H2 of L2 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L2 and different subunits of PMMA mer.
Figure 5.
Partial RDFs calculated for distances between the center of mass of different moieties of the L2 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L2/PMMA system. Panels (a,b) present the RDFs for C2H2 of L2 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L2 and different subunits of PMMA mer.
Figure 6.
Partial RDFs calculated for distances between the center of mass of different moieties of the L3 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L3/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L3 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs NO2 of L3 and different subunits of PMMA mer.
Figure 6.
Partial RDFs calculated for distances between the center of mass of different moieties of the L3 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L3/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L3 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs NO2 of L3 and different subunits of PMMA mer.
Figure 7.
Partial RDFs calculated for distances between the center of mass of different moieties of the L4 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L4/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L4 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs NO2 of L4 and different subunits of PMMA mer.
Figure 7.
Partial RDFs calculated for distances between the center of mass of different moieties of the L4 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the bulk L4/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L4 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs NO2 of L4 and different subunits of PMMA mer.
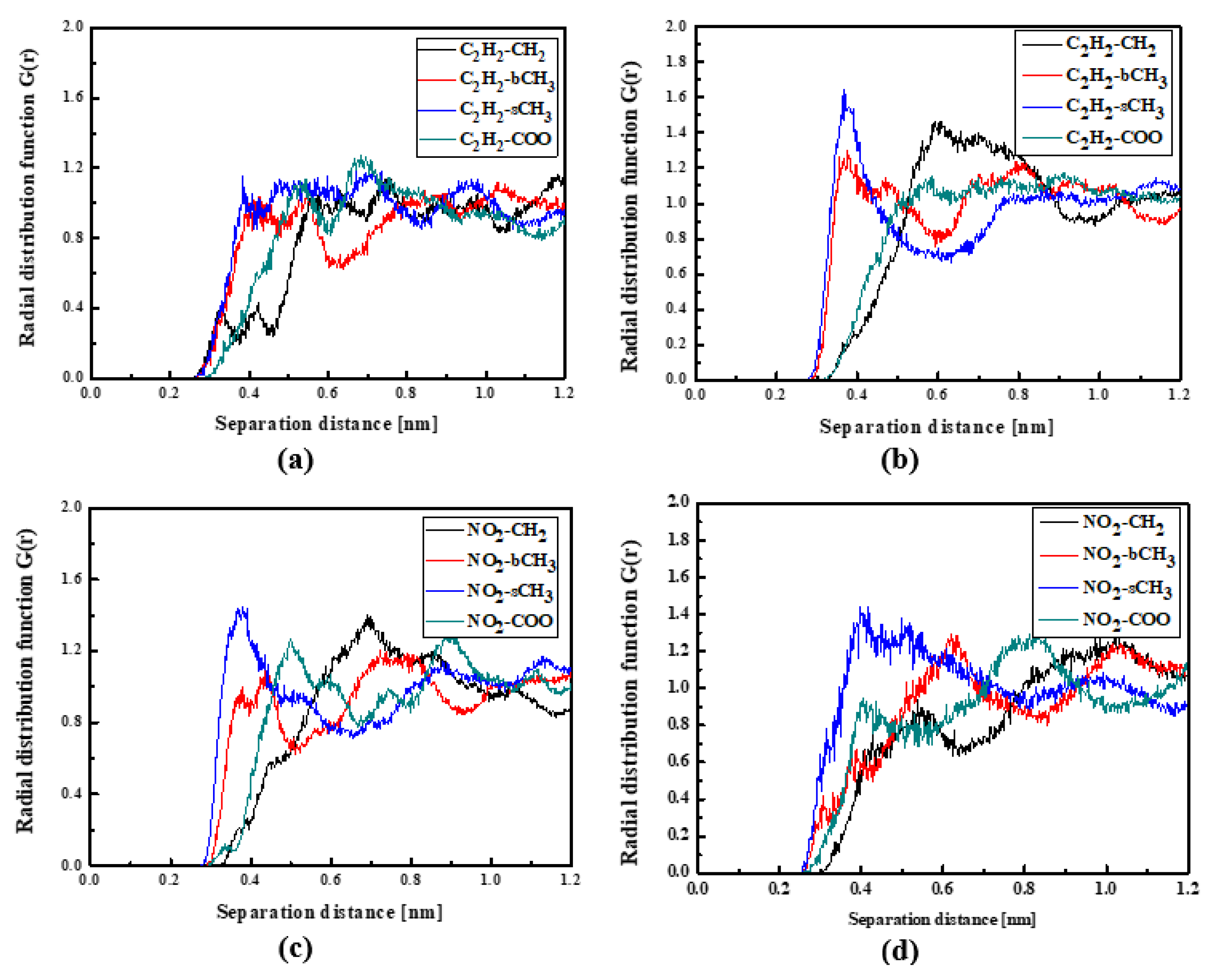
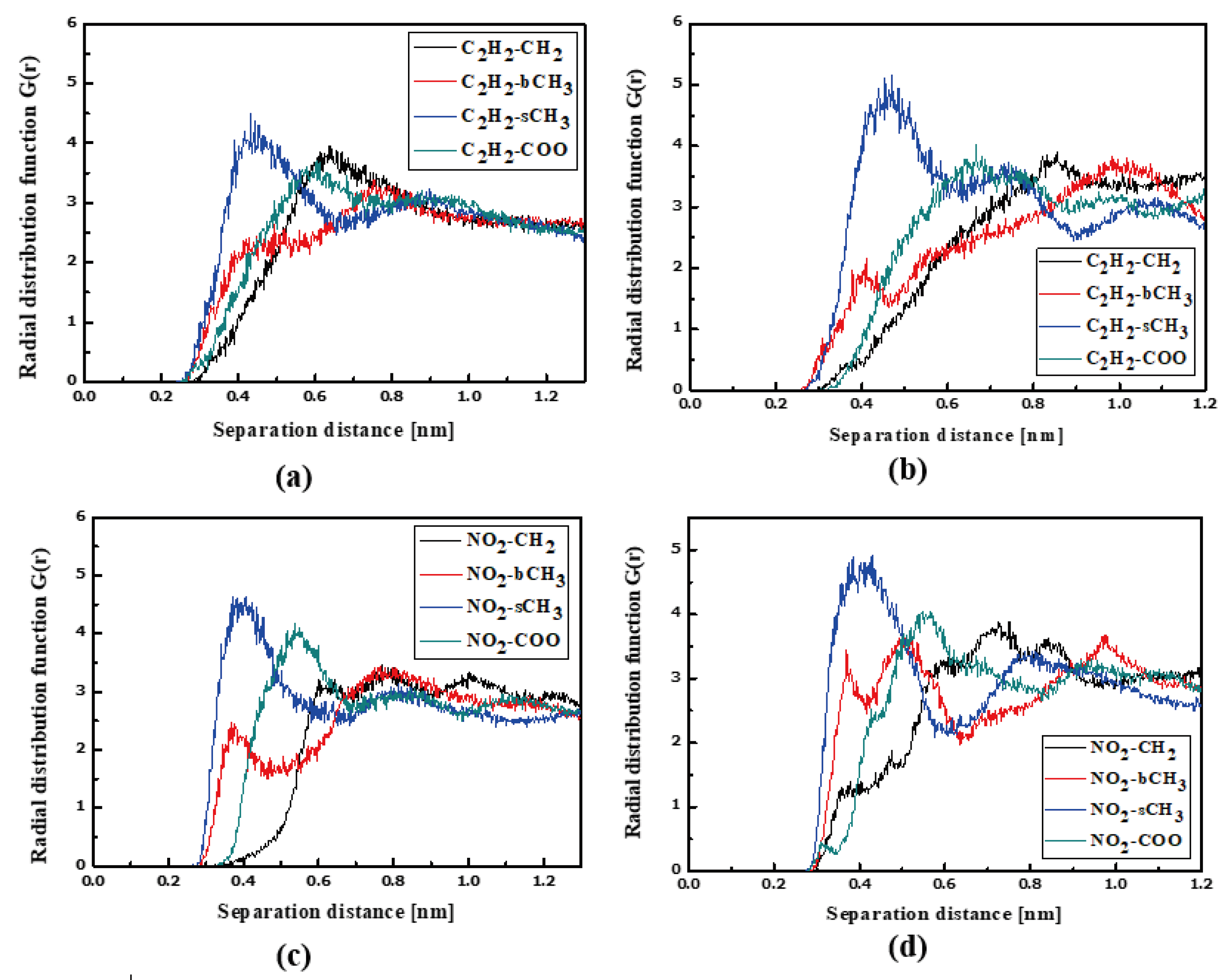
Figure 8.
Partial RDFs calculated for distances between the center of mass of different moieties of the L1 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L1/PMMA system. Panels (a,b) present the RDFs for C2H2 of L1 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L1 and different subunits of PMMA mer.
Figure 8.
Partial RDFs calculated for distances between the center of mass of different moieties of the L1 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L1/PMMA system. Panels (a,b) present the RDFs for C2H2 of L1 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L1 and different subunits of PMMA mer.
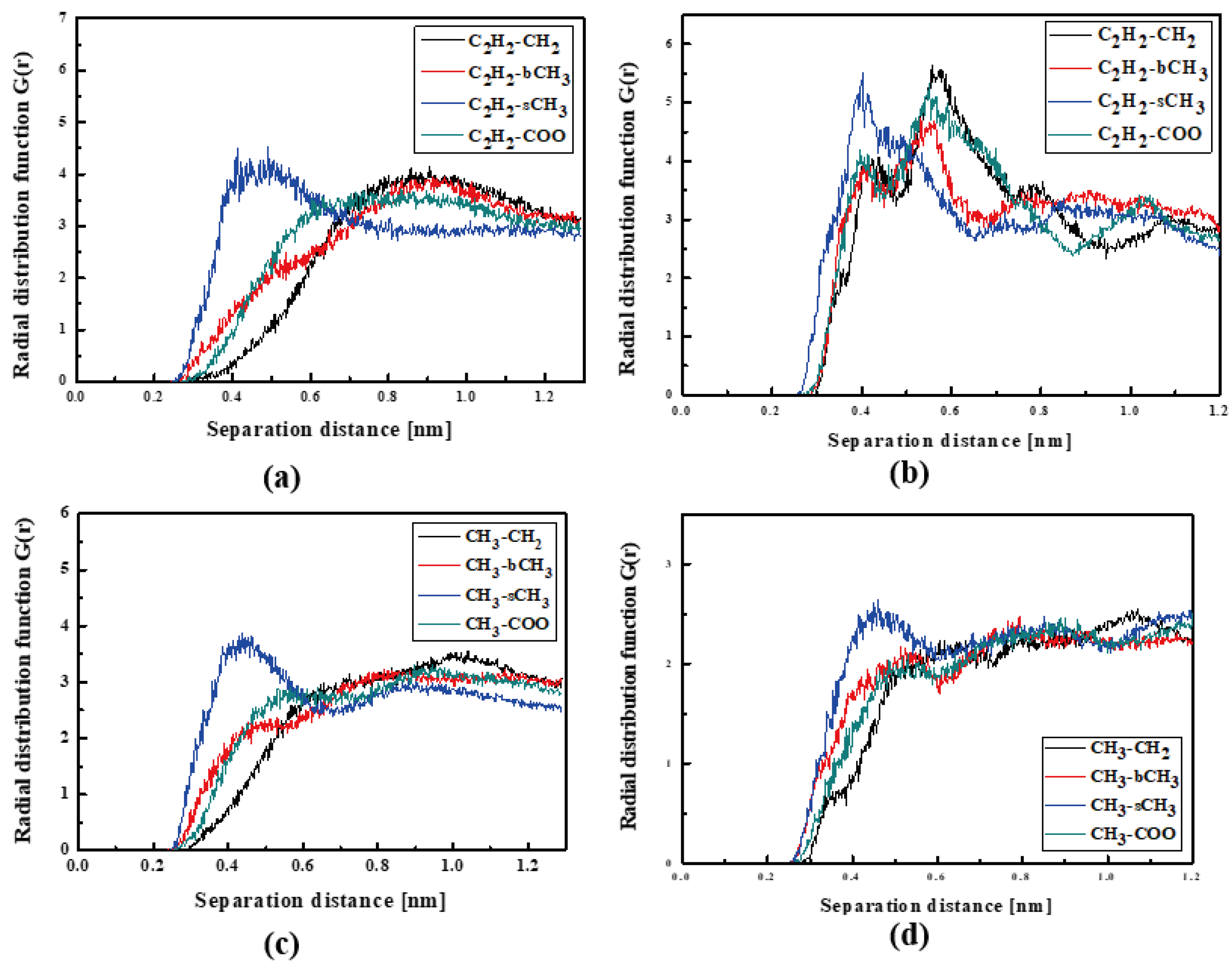
Figure 9.
Partial RDFs calculated for distances between the center of mass of different moieties of the L2 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L2/PMMA system. Panels (a,b) present the RDFs for C2H2 of L2 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L2 and different subunits of PMMA mer.
Figure 9.
Partial RDFs calculated for distances between the center of mass of different moieties of the L2 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L2/PMMA system. Panels (a,b) present the RDFs for C2H2 of L2 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs CH3 of L2 and different subunits of PMMA mer.
Figure 10.
Partial RDFs calculated for distances between the center of mass of different moieties of the L3 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L3/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L3 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs for NO2 of L3 and different subunits of PMMA mer.
Figure 10.
Partial RDFs calculated for distances between the center of mass of different moieties of the L3 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L3/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L3 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs for NO2 of L3 and different subunits of PMMA mer.
Figure 11.
Partial RDFs calculated for distances between the center of mass of different moieties of the L4 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L4/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L4 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs of NO2 of L4 and different subunits of PMMA mer.
Figure 11.
Partial RDFs calculated for distances between the center of mass of different moieties of the L4 molecule and different subunits of PMMA at T = 500 K (a,c) and T = 300 K (b,d) for the thin film L4/PMMA system. Panels (a,b) present the RDFs for C2H2 of the L4 molecule and different subunits of PMMA mer. Panels (c,d) present the RDFs of NO2 of L4 and different subunits of PMMA mer.
Figure 12.
Relationship between laboratory and molecular coordinate systems.
Figure 12.
Relationship between laboratory and molecular coordinate systems.
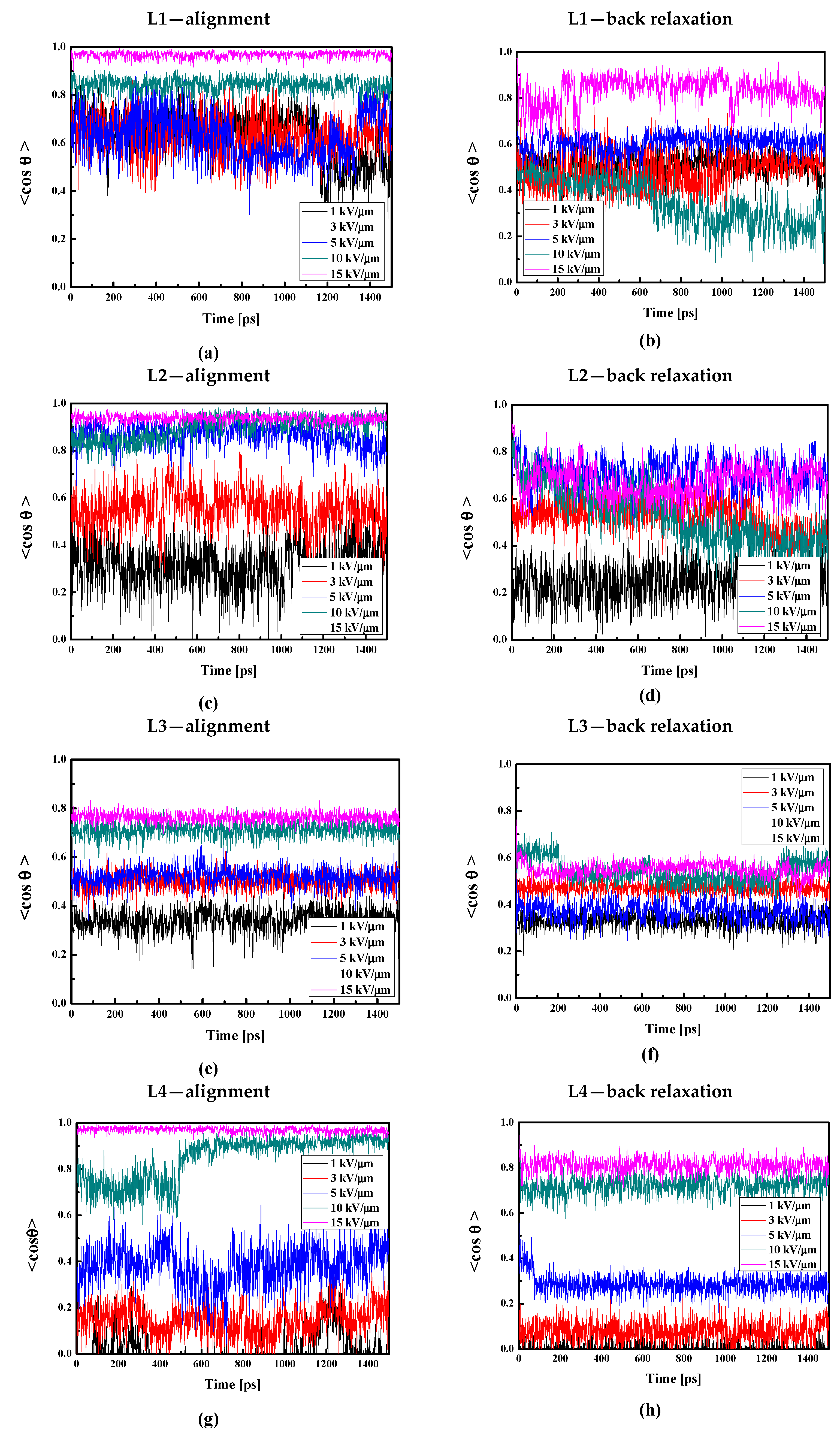
Figure 13.
Changes in the value of the order parameter <cosθ(t)> versus the time of simulation and applied external electric field calculated by the MD technique for the L1/PMMA (a,b), L2/PMMA (c,d), L3/PMMA (e,f), and L4/PMMA (g,h) composites in the volumetric form at temperatures of 500 K (a,c,e,g) and 300 K after the simulated annealing without external electric field (b,d,f,h).
Figure 13.
Changes in the value of the order parameter <cosθ(t)> versus the time of simulation and applied external electric field calculated by the MD technique for the L1/PMMA (a,b), L2/PMMA (c,d), L3/PMMA (e,f), and L4/PMMA (g,h) composites in the volumetric form at temperatures of 500 K (a,c,e,g) and 300 K after the simulated annealing without external electric field (b,d,f,h).
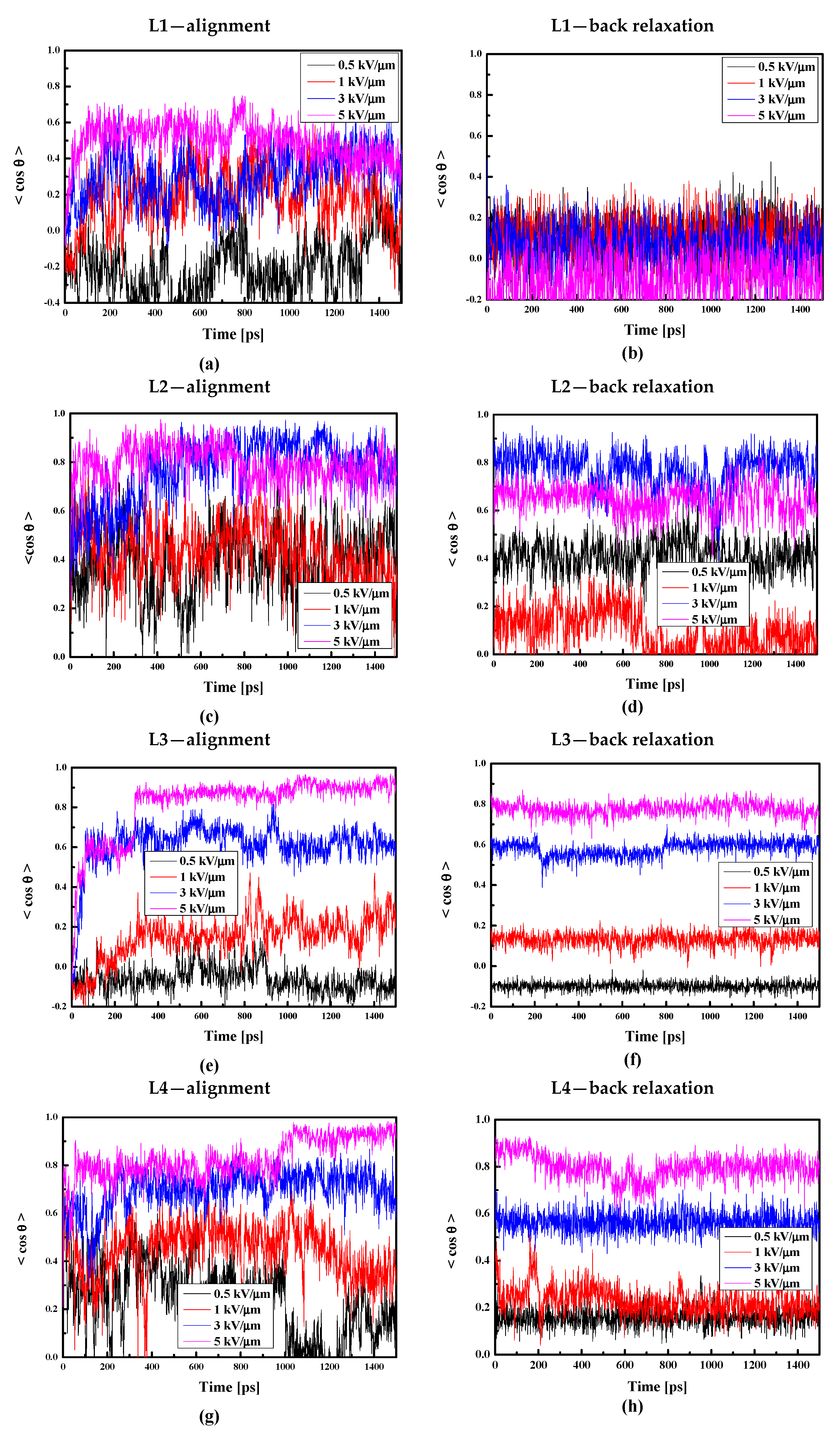
Figure 14.
Changes in the value of the order parameter <cosθ(t)> versus the time of simulation and applied external electric field calculated by the MD technique for the L1/PMMA (a,b), L2/PMMA (c,d), L3/PMMA (e,f), and L4/PMMA (g,h) composites in the thin film form at the temperature of 500 K (a,c,e,g) and in glassy state (300 K) after the simulated annealing (b,d,f,h).
Figure 14.
Changes in the value of the order parameter <cosθ(t)> versus the time of simulation and applied external electric field calculated by the MD technique for the L1/PMMA (a,b), L2/PMMA (c,d), L3/PMMA (e,f), and L4/PMMA (g,h) composites in the thin film form at the temperature of 500 K (a,c,e,g) and in glassy state (300 K) after the simulated annealing (b,d,f,h).
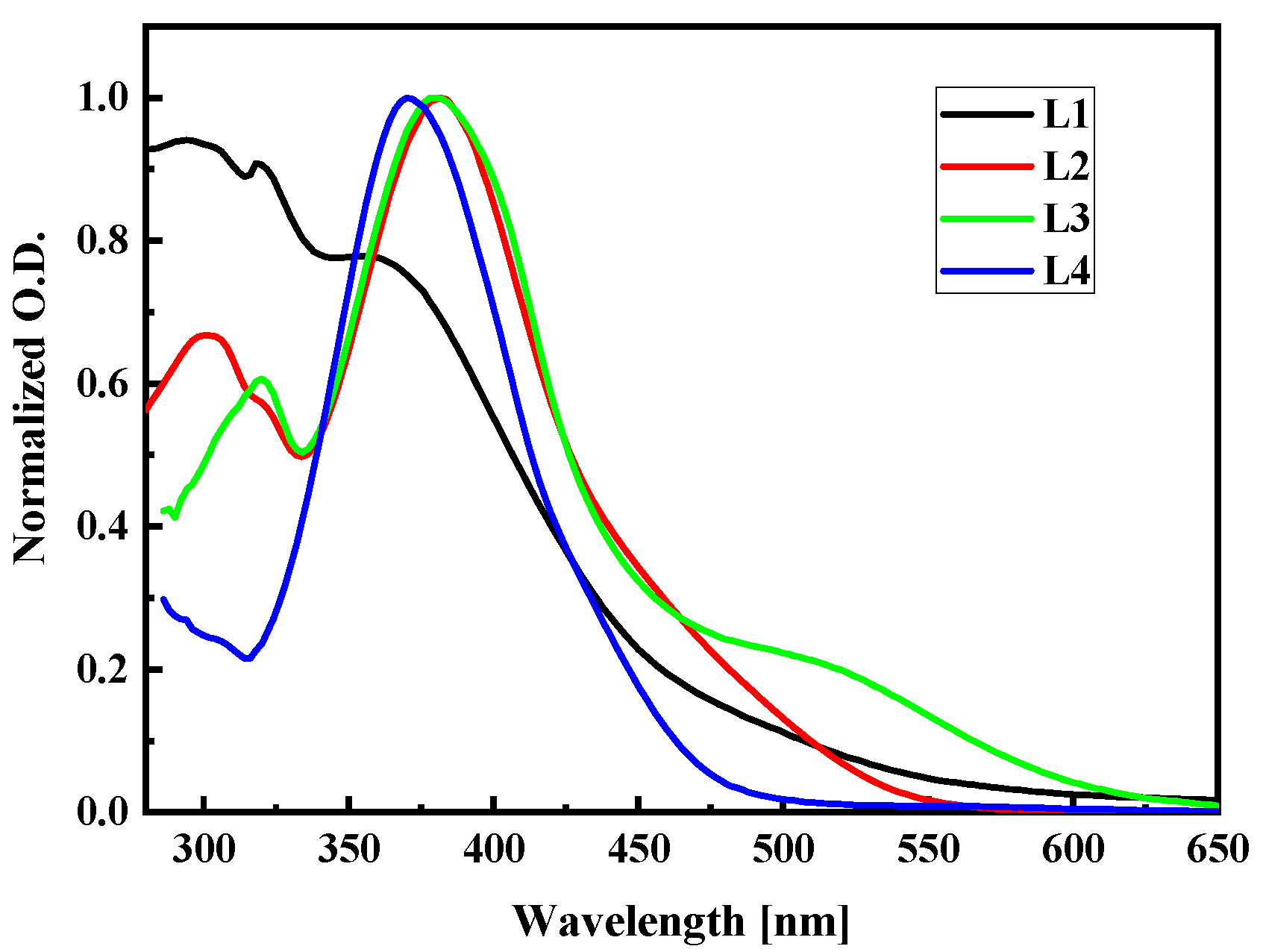
Figure 15.
Experimental UV-vis absorption spectra of L1, L2, L3, and L4 chromophores measured in dichloromethane (~C = 2.6 × 10−5 M) at room temperature.
Figure 15.
Experimental UV-vis absorption spectra of L1, L2, L3, and L4 chromophores measured in dichloromethane (~C = 2.6 × 10−5 M) at room temperature.
Figure 16.
The UV-vis absorption spectra calculated by DFT/B3LYP and DFT/LC-BLYP methods for L1 (a), L2 (b), L3 (c), and L4 (d) molecules in dichloromethane compared with experimentally obtained data. UV-vis absorption spectra of L1, L2, L3, and L4 chromophores measured in dichloromethane (~C = 2.6 × 10−5 M) at room temperature.
Figure 16.
The UV-vis absorption spectra calculated by DFT/B3LYP and DFT/LC-BLYP methods for L1 (a), L2 (b), L3 (c), and L4 (d) molecules in dichloromethane compared with experimentally obtained data. UV-vis absorption spectra of L1, L2, L3, and L4 chromophores measured in dichloromethane (~C = 2.6 × 10−5 M) at room temperature.
Table 1.
Structures and frontier molecular orbitals calculated for L1, L2, L3, and L4 molecules by DFT/B3LYP/6-311++G** methodology.
Table 2.
Electron and optical properties of the L1, L2, L3, and L4 molecules in conformation a and b calculated in vacuum and dichloromethane by DFT/B3LYP and DFT/LC-BLYP methods.
Table 2.
Electron and optical properties of the L1, L2, L3, and L4 molecules in conformation a and b calculated in vacuum and dichloromethane by DFT/B3LYP and DFT/LC-BLYP methods.
| Molecule | ΔEHOMO-LUMO [eV] | Dipole Moment [D] | λmax [nm] | λmax [nm] |
|---|
| In Vacuum | In Dichloromethane |
|---|
| B3LYP | LC-BLYP | B3LYP | LC-BLYP | B3LYP | LC-BLYP | B3LYP | LC-BLYP |
|---|
| L1a | 3.23 | 6.90 | 1.24 | 1.00 | 500.8 | 409.6 | 500.1 | 408.7 |
| L1b | 3.13 | 7.31 | 2.55 | 2.95 | 509.0 | 415.4 | 500.1 | 409.1 |
| L2a | 2.94 | 6.55 | 1.56 | 1.12 | 520.1 | 415.2 | 518.1 | 414.7 |
| L2b | 2.85 | 6.89 | 2.72 | 3.30 | 542.2 | 423.8 | 534.2 | 416.9 |
| L3a | 1.99 | 6.16 | 6.66 | 6.03 | 717.5 | 416.1 | 718.8 | 409.3 |
| L3b | 2.13 | 6.27 | 10.56 | 9.96 | 680.5 | 422.9 | 737.1 | 415.6 |
| L4a | 1.92 | 6.15 | 6.60 | 5.92 | 744.2 | 416.3 | 719.6 | 409.3 |
| L4b | 1.68 | 5.90 | 8.39 | 8.10 | 971.7 | 416.0 | 891.3 | 423.9 |
Table 3.
Optical properties of the L1 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
Table 3.
Optical properties of the L1 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
| Molecule | L1a | L1b |
|---|
| Fx, Fy, Fz [GV/m] | 0, 0, 0 | 0.63, −0.26, −0.27 | 0, 0, 0 | 0.63, −0.26, −0.27 |
| λ =
∞ nm |
| αvec | 348.22 | 348.63 | 340.75 | 341.15 |
| β(z;z,z) | −234.12 | −281.44 | −993.81 | −1192.27 |
| β(z) | 513.90 | 513.06 | −1023.06 | −1237.10 |
| βvec | −1065.28 | −1299.30 | −1084.00 | −1376.59 |
| γ(z;z,z,z) | 316,544.26 | 323,376.63 | 308,817.70 | 323,428.03 |
| γvec | 152,423.09 | 156,069.64 | 125,806.73 | 129,839.36 |
| λ = 1064 nm |
| αvec | 354.97 | 355.43 | 347.15 | 347.62 |
| β(z;z,z) | −234.54 | −286.65 | −1803.34 | −2134.06 |
| β(z) | 820.20 | 842.96 | −1876.65 | −2211.48 |
| βvec | −1529.15 | −1842.83 | −1964.15 | 2387.40 |
| γ(z;z,z,z) | 780,156.42 | 808,763.27 | 1,037,673.87 | 1,153,715.81 |
Table 4.
Optical properties of the L2 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
Table 4.
Optical properties of the L2 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
| Molecule | L2a | L2b |
|---|
| Fx, Fy, Fz [GV/m] | 0, 0, 0 | 1.00, 0.08, −0.24 | 0, 0, 0 | 1.00, 0.08, −0.24 |
| λ =
∞ nm |
| αvec | 350.71 | 350.10 | 355.06 | 355.30 |
| β(z;z,z) | 1044.65 | 537.31 | −2168.85 | −2639.76 |
| β(z) | 1523.84 | 724.76 | −2292.25 | −2819.59 |
| βvec | 1135.96 | 648.52 | −2265.53 | −2780.98 |
| γ(z;z,z,z) | 343,711.47 | 330,617.13 | 767,234.87 | 791,318.81 |
| γvec | 180,307.29 | 176,382.50 | 220,527.36 | 225,157.26 |
| λ = 1064 nm |
| αvec | 357.80 | 357.12 | 363.20 | 363.48 |
| β(z;z,z) | 1572.70 | 777.96 | −4137.86 | −4948.54 |
| β(z) | 2217.61 | 981.05 | −4330.76 | −5222.10 |
| βvec | 1788.29 | 906.73 | 4280.09 | −5153.42 |
| γ(z;z,z,z) | 993,705.63 | 945,275.89 | 3,724,893.11 | 3,973,896.27 |
Table 5.
Optical properties of the L3 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
Table 5.
Optical properties of the L3 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
| Molecule | L3a | L3b |
|---|
| Fx, Fy, Fz [GV/m] | 0, 0, 0 | 0.02, 0.13, −0.16 | 0, 0, 0 | 0.02, 0.13, −0.16 |
| λ =
∞ nm |
| αvec | 371.32 | 371.13 | 371.19 | 371.06 |
| β(z;z,z) | 1834.58 | 1533.67 | 1631.59 | 1537.15 |
| β(z) | 2686.05 | 2368.38 | 2458.76 | 2320.57 |
| βvec | 2809.73 | 2510.19 | 2533.30 | 2404.97 |
| γ(z;z,z,z) | 1,059,995.74 | 1,038,024.70 | 448,094.58 | 445,438.97 |
| γvec | 272,530.64 | 267,830.58 | 179,103.70 | 242,816.49 |
| λ = 1064 nm |
| αvec | 380.51 | 380.28 | 380.42 | 380.33 |
| β(z;z,z) | 3403.30 | 2892.62 | 2336.48 | 2178.77 |
| β(z) | 4565.24 | 4030.50 | 3773.85 | 3544.41 |
| βvec | 4705.35 | 4189.16 | 3862.52 | 3644.54 |
| γ(z;z,z,z) | 4,263,921.80 | 4,065,808.73 | 1,758,796.83 | 1,738,381.81 |
Table 6.
Optical properties of the L4 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
Table 6.
Optical properties of the L4 molecules calculated in vacuum (Fx, Fy, Fz = 0, 0, 0) and PMMA polymer environment (Fx, Fy, Fz ≠ 0, 0, 0) in volumetric form by using the DFT/LC-BLYP/6-31++G** method.
| Molecule | L4a | L4b |
|---|
| Fx, Fy, Fz [GV/m] | 0, 0, 0 | 0.03, 0.37, 0.43 | 0, 0, 0 | 0.03,0.37, 0.43 |
| λ =
∞ nm |
| αvec | 362.74 | 363.61 | 351.66 | 351.78 |
| β(z;z,z) | 197.62 | 576.51 | 664.40 | 886.57 |
| β(z) | 1440.12 | 2015.46 | 705.52 | 978.04 |
| βvec | 1698.35 | 2324.69 | 446.08 | 894.45 |
| γ(z;z,z,z) | 355,253.54 | 366,340.05 | 288,943.31 | 296,072.59 |
| γvec | 174,379.43 | 182,956.26 | 137,652.19 | 137,585.37 |
| λ = 1064 nm |
| αvec | 370.63 | 371.61 | 358.77 | 358.91 |
| β(z;z,z) | 528.86 | 1117.53 | 983.49 | 1321.85 |
| β(z) | 2258.99 | 3146.95 | 1138.44 | 1627.22 |
| βvec | 2601.77 | 3667.28 | 943.73 | 1460.30 |
| γ(z;z,z,z) | 1,018,411.52 | 1,093,392.47 | 814,810.82 | 848,786.26 |
Table 7.
Susceptibilities χ(2) and χ(3) measured experimentally for L1/PMMA, L2/PMMA, L3/PMMA, and L4/PMMA thin films.
Table 7.
Susceptibilities χ(2) and χ(3) measured experimentally for L1/PMMA, L2/PMMA, L3/PMMA, and L4/PMMA thin films.
| Sample | χ(2) [10−12 mV−1] | χ(3) [10−21 m2V−2] |
|---|
| L1 | 1.10 | 1.70 |
| L2 | 2.10 | 1.80 |
| L3 | 0.79 | 1.60 |
| L4 | 1.20 | 1.70 |
| Y-cut quartz | 1.00 | |
| Silica glass | | 0.20 |