Experimental and Modeling Study on Methane Hydrate Equilibrium Conditions in the Presence of Inorganic Salts
Abstract
1. Introduction
2. Results and Discussion
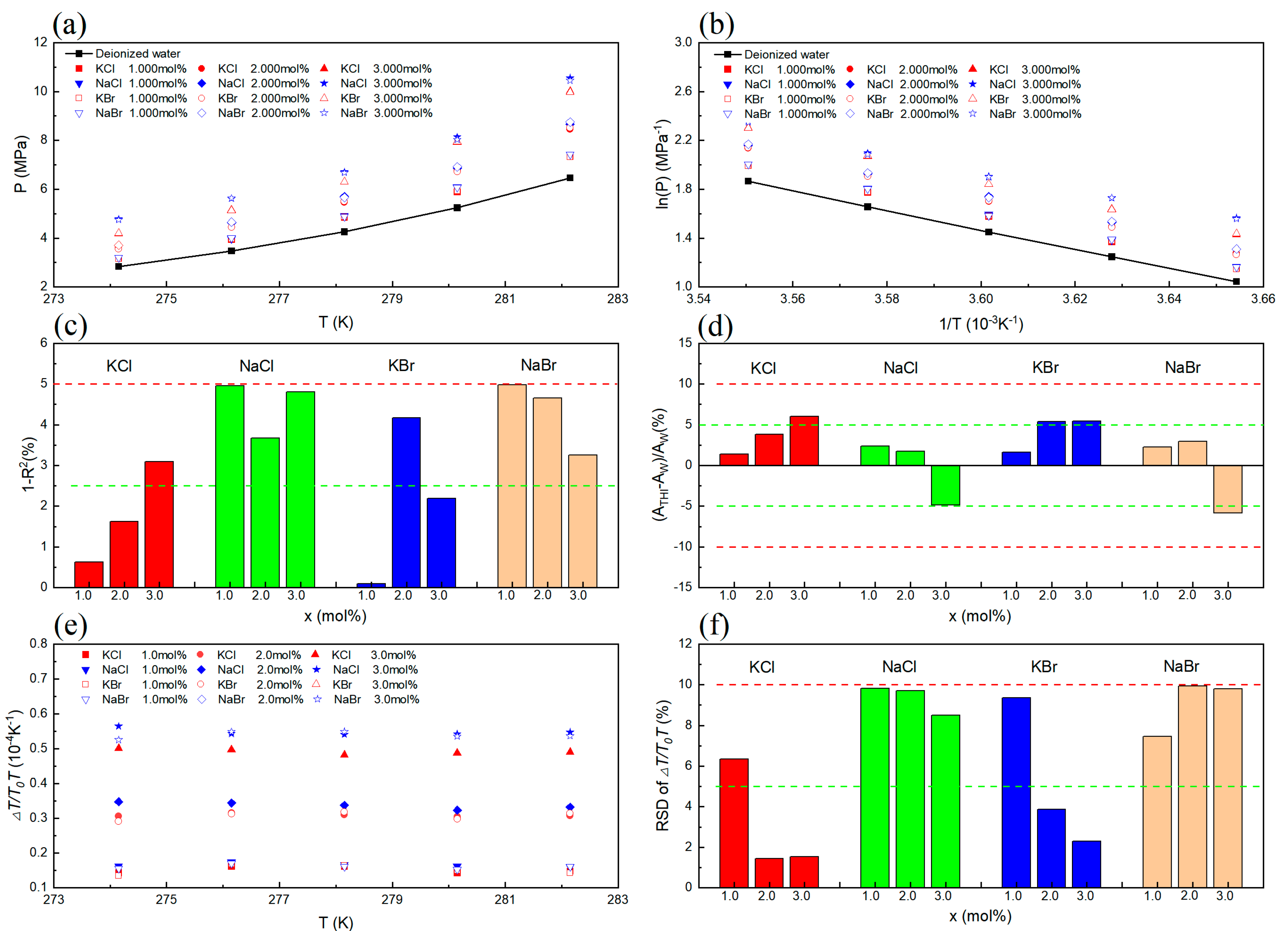
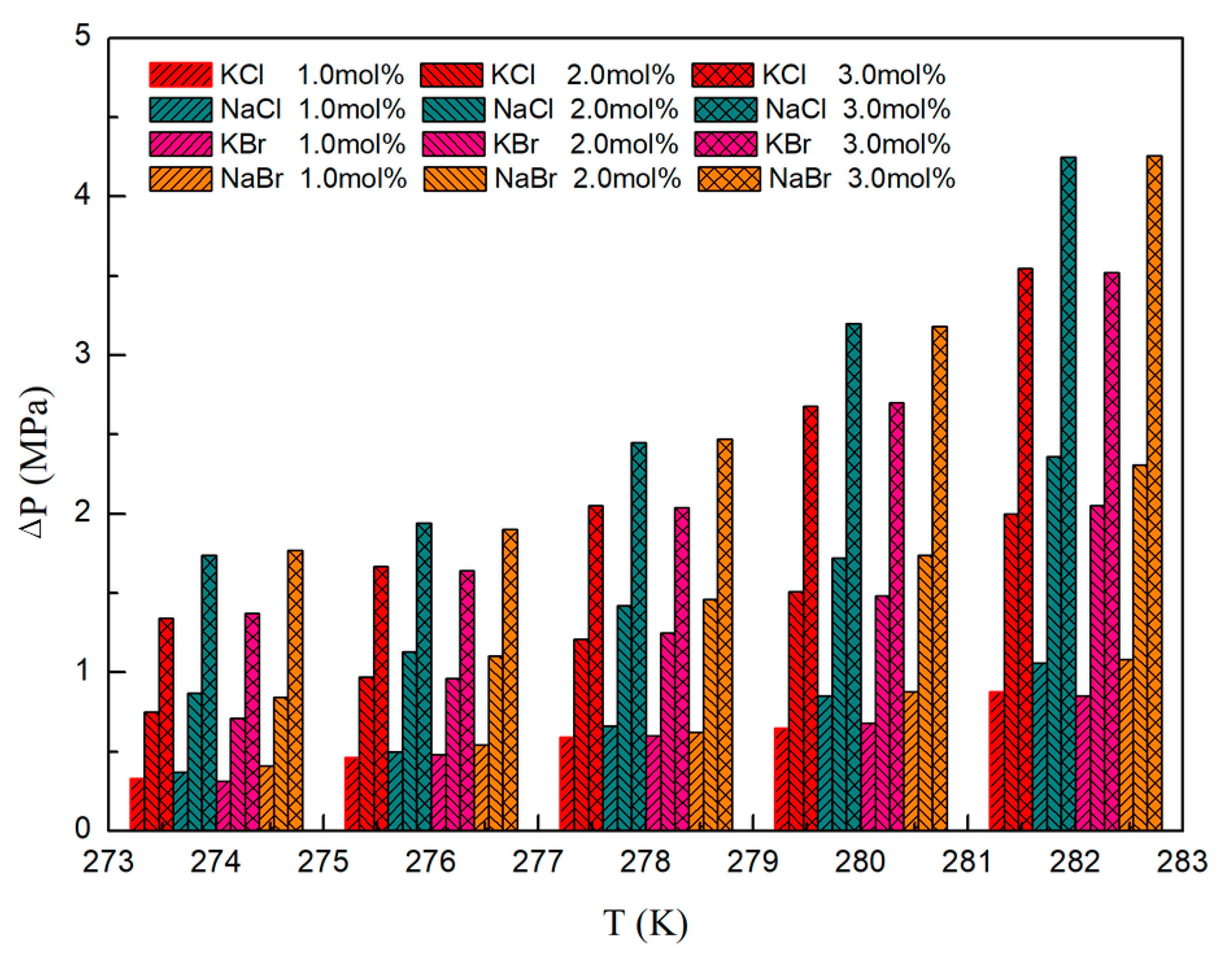
2.1. The VLWH Equilibrium Conditions in the Presence of Inorganic Salts
2.2. Thermodynamic Inhibition of Inorganic Salts on CH4 Hydrate
2.3. The Hydrate in the Presence of Inorganic Salts
2.4. Prediction of the VLWH Equilibrium Conditions
3. Materials and Methods
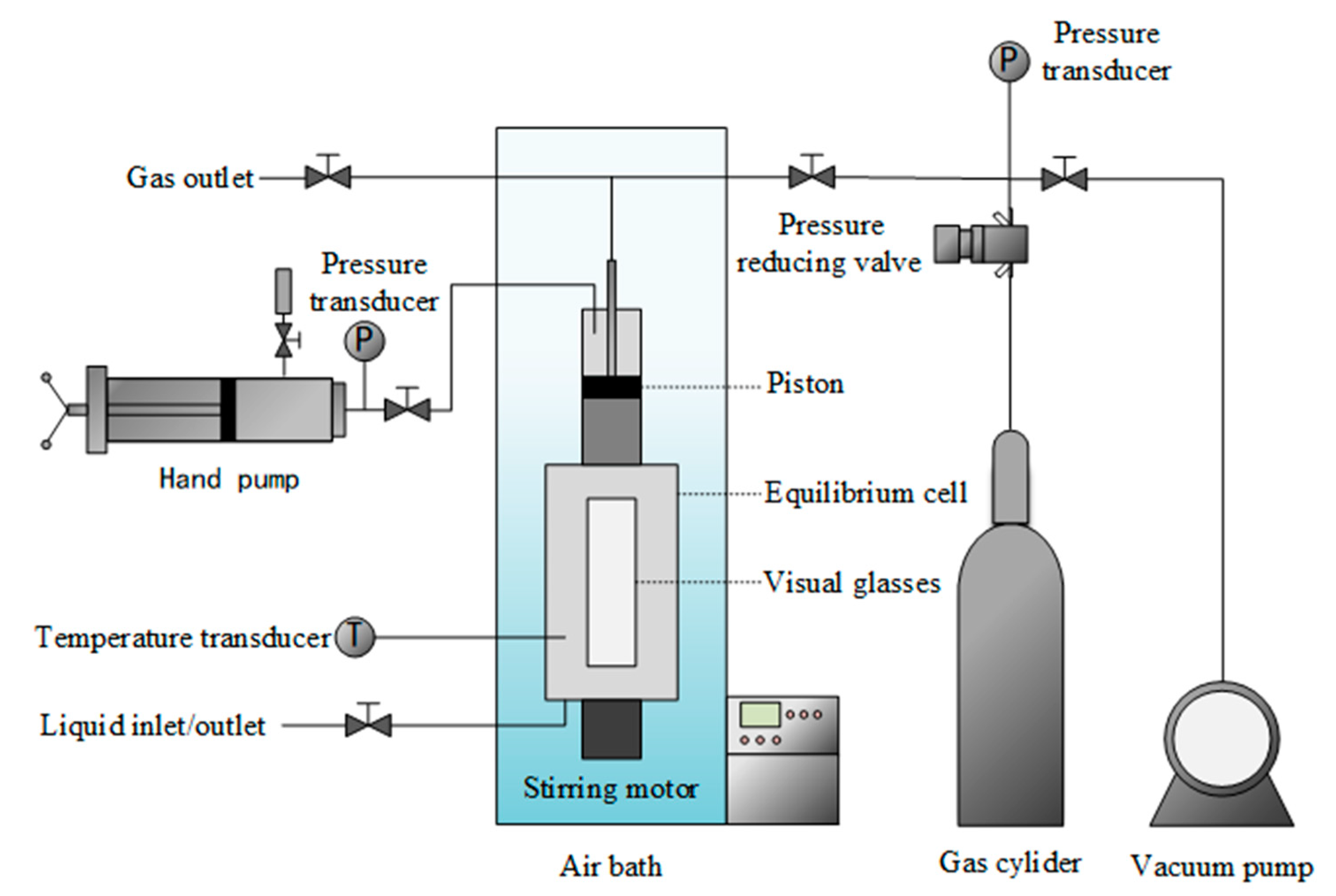
3.1. Experimental
3.1.1. Materials
3.1.2. Experimental Apparatus
3.1.3. Experimental Procedures
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix B.1. The Chen–Guo Thermodynamic Model
Appendix B.2. Water Activity () Model
References
- Song, Y.C.; Tian, M.R.; Zheng, J.N.; Yang, M.J. Thermodynamics analysis and ice behavior during the depressurization process of methane hydrate reservoir. Energy 2022, 250, 123801. [Google Scholar] [CrossRef]
- Pan, M.; Schicks, J.M. Unraveling the role of natural sediments in sII mixed gas hydrate formation: An experimental study. Molecules 2023, 28, 5887. [Google Scholar] [CrossRef] [PubMed]
- Jin, G.; Liu, J.; Su, Z.; Feng, C.; Cheng, S.; Zhai, H.; Liu, L. Gas production from a promising reservoir of the hydrate with associated and shallow gas layers in the low permeable sediments. Energy 2024, 295, 131079. [Google Scholar] [CrossRef]
- Gnezdilov, D.; Varfolomeev, M.; Farhadian, A.; Pavelyev, R.; Semenov, M.; Chirkova, Y.; Nazarychev, S.; Balachina, E.; Semenov, A.; Stoporev, A.; et al. Effective prevention of structure II gas hydrate formation using the newly synthesized kinetic inhibitors. Chem. Eng. Sci. 2024, 292, 119986. [Google Scholar] [CrossRef]
- Farhadian, A.; Rizi, Z.T.; Naeiji, P.; Mohammad-Taheri, M.; Shaabani, A.; Aminolroayaei, M.A.; Yang, M. Promising kinetic gas hydrate inhibitors for developing sour gas reservoirs. Energy 2023, 282, 128979. [Google Scholar] [CrossRef]
- Olarinoye, F.O.; Kang, S.-P.; Ajienka, J.A.; Ikiensikimama, S.S. Synergy between two natural inhibitors via pectin and mixed agro-waste-based amino acids for natural gas hydrate control. Geoenergy Sci. Eng. 2024, 239, 212967. [Google Scholar] [CrossRef]
- Kashish; Yusuf, M.; Beg, M.; Kamyab, H.; Muzammil, K.; Ahmad, M.; Gupta, M.; Kumar, S.; Rezania, S.; Ibrahim, H. Natural gas hydrates: A review of various inhibitors and respective mechanisms. J. Mol. Liq. 2024, 403, 124809. [Google Scholar] [CrossRef]
- Blazquez, S.; Vega, C.; Conde, M.M. Three phase equilibria of the methane hydrate in NaCl solutions: A simulation study. J. Mol. Liq. 2023, 383, 122031. [Google Scholar] [CrossRef]
- Liao, B.; Sun, J.; Wang, J.; Lv, X.; Wang, J.; Guo, J.; Lv, K.; Wang, R.; Zheng, J.; Chen, Z. Development of novel natural gas hydrate inhibitor and the synergistic inhibition mechanism with nacl: Experiments and molecular dynamics simulation. Fuel 2023, 353, 129162. [Google Scholar] [CrossRef]
- Semenov, A.P.; Mendgaziev, R.I.; Istomin, V.A.; Sergeeva, D.V.; Vinokurov, V.A.; Gong, Y.; Li, T.; Stoporev, A.S. Searching for synergy between alcohol and salt to produce more potent and environmentally benign gas hydrate inhibitors. Chem. Eng. Sci. 2024, 283, 119361. [Google Scholar] [CrossRef]
- Kannan, N.S.; Delgado-Linares, J.G.; Makogon, T.Y.; Koh, C.A. Synergistic effect of kinetic hydrate inhibitor (khi) and monoethylene glycol (meg) in gas hydrate management. Fuel 2024, 366, 131326. [Google Scholar] [CrossRef]
- Li, S.; Wang, J.; Lv, X.; Ge, K.; Jiang, Z.; Li, Y. Experimental measurement and thermodynamic modeling of methane hydrate phase equilibria in the presence of chloride salts. Chem. Eng. J. 2020, 395, 125126. [Google Scholar] [CrossRef]
- Semenov, A.P.; Tulegenov, T.B.; Mendgaziev, R.I.; Stoporev, A.S.; Istomin, V.A.; Sergeeva, D.V.; Lednev, D.A.; Vinokurov, V.A. Dual nature of methanol as a thermodynamic inhibitor and kinetic promoter of methane hydrate formation in a wide concentration range. J. Mol. Liq. 2024, 403, 124780. [Google Scholar] [CrossRef]
- Semenov, A.P.; Mendgaziev, R.I.; Istomin, V.A.; Sergeeva, D.V.; Vinokurov, V.A.; Gong, Y.; Li, T.; Stoporev, A.S. Data on searching for synergy between alcohol and salt to produce more potent and environmentally benign gas hydrate inhibitors. Data Br. 2024, 53, 110138. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Cai, W.J.; Li, Z.C.; Lu, H.L. Insights into behaviors of guest and host molecules in methane hydrate formation process in the presence of kinetic inhibitors via in-situ micro-Raman spectroscopy. Fuel 2024, 358, 130195. [Google Scholar] [CrossRef]
- Liang, H.Y.; Chu, J.W.; Liu, Y.Z.; Yang, L.; Shen, S.; Lv, X.; Song, Y.C. Kinetics and morphology of gas hydrate formation from meg solution in under-inhibited systems. Chem. Eng. J. 2024, 494, 152946. [Google Scholar] [CrossRef]
- Wu, G.Z.; Coulon, F.; Feng, J.C.; Yang, Z.F.; Jiang, Y.L.; Zhang, R.F. Machine learning models for fast selection of amino acids as green thermodynamic inhibitors for natural gas hydrate. J. Mol. Liq. 2023, 370, 120952. [Google Scholar] [CrossRef]
- Gong, Y.H.; Mendgaziev, R.I.; Hu, W.; Li, Y.Z.; Li, Z.; Stoporev, A.S.; Manakov, A.Y.; Vinokurov, V.A.; Li, T.D.; Semenov, A.P. Urea as a green thermodynamic inhibitor of sII gas hydrates. Chem. Eng. J. 2022, 429, 132386. [Google Scholar] [CrossRef]
- Das, S.; Mahto, V.; Udayabhanu, G.; Lall, M.V.; Singh, K.; Deepak, M. Evaluation of l-ascorbic acid as a green low dosage hydrate inhibitor in water-based drilling fluid for the drilling of gas hydrate reservoirs. J. Petrol. Sci. Eng. 2023, 220, 111156. [Google Scholar] [CrossRef]
- Pei, J.; Wang, Z.; Li, P.; Hu, J.; Tong, S.; Zhong, J.; Liu, P.; Fu, W. Experimental investigation on highly potent inhibitors of natural gas hydrate in oil-free flow system. Fuel 2023, 343, 127996. [Google Scholar] [CrossRef]
- Belosludov, R.V.; Gets, K.V.; Zhdanov, R.K.; Bozhko, Y.Y.; Belosludov, V.R.; Chen, L.-J.; Kawazoe, Y. Molecular dynamics study of clathrate-like ordering of water in supersaturated methane solution at low pressure. Molecules 2023, 28, 2960. [Google Scholar] [CrossRef] [PubMed]
- Teixeira, A.M.; Arinelli, L.D.O.; de Medeiros, J.L.; Araújo, O.D.Q.F. Sustainable offshore natural gas processing with thermodynamic gas-hydrate inhibitor reclamation: Supersonic separation affords carbon capture. Chem. Eng. Res. Des. 2022, 181, 55–73. [Google Scholar] [CrossRef]
- Okon, O.E.; Ajienka, J.A.; Ikiensikimama, S.S.; Akaranta, O.E.; Wachikwu-Elechi, V.U. Experimental investigation and comparative environmental impact analysis of conventional and naturally occurring kinetic hydrate inhibitors in offshore environments using toxicity and bioconcentration tools. Results Eng. 2024, 21, 101705. [Google Scholar] [CrossRef]
- Avula, V.R.; Nalajala, V.S.; Reddy, G.S.; Prince, M. Methane hydrate thermodynamic phase stability predictions in the presence of salt inhibitors and their mixture for offshore operations. Chem. Thermal Thermal Analys 2021, 3–4, 100022. [Google Scholar] [CrossRef]
- Sa, J.-H.; Hu, Y.; Sum, A.K. Assessing thermodynamic consistency of gas hydrates phase equilibrium data for inhibited systems. Fluid. Phase Equilibr 2018, 473, 294–299. [Google Scholar] [CrossRef]
- Sun, J.; Sun, R.; Chou, I.M.; Nguyen, A.V.; Jiang, L. Experimental measurement and thermodynamic modeling of dissociation conditions of hydrogen sulfide hydrate in the presence of electrolyte solutions. Chem. Eng. J. 2022, 431, 133821. [Google Scholar] [CrossRef]
- Xu, Z.; Wang, Y.; Kan, J.; Liu, A.; Sun, Q.; Chen, J.; Guo, X. Experimental measurement and model prediction on methane hydrate equilibrium conditions in the presence of organic carboxylic sodium salts. J. Chem. Thermodyn. 2023, 180, 107005. [Google Scholar] [CrossRef]
- Wu, C.; Chen, J.; Sun, J.; Chou, I.-M.; Mei, S.; Lin, J.; Jiang, L. Experimental determination and thermodynamic modeling of the hydrogen sulfide hydrate solubility in water. Chem. Eng. Sci. 2024, 284, 119474. [Google Scholar] [CrossRef]
- Cai, J.; Wang, X.-H.; Wu, Y.-W.; Tang, H.; Jun, L.; Xu, X.-J.; Ji, J.-F.; Sun, C.-Y.; Chen, G.-J. Modeling on gas hydrate phase equilibrium at high concentration of alcohols. J. Mol. Liq. 2024, 395, 123826. [Google Scholar] [CrossRef]
- Chen, G.J.; Guo, T.M. Thermodynamic modeling of hydrate formation based on new concepts. Fluid Phase Equilibria 1996, 122, 43–65. [Google Scholar] [CrossRef]
- Sun, C.Y.; Chen, G.J. Modelling the hydrate formation condition for sour gas and mixtures. Chem. Eng. Sci. 2005, 60, 4879–4885. [Google Scholar] [CrossRef]
- Chen, G.J.; Guo, T.M. A new approach to gas hydrate modelling. Chem. Eng. J. 1998, 71, 145–151. [Google Scholar] [CrossRef]
- Haghtalab, A.; Shojaeian, A.; Mazloumi, S.H. Nonelectrolyte NRTL-NRF model to study thermodynamics of strong and weak electrolyte solutions. J. Chem. Thermodyn. 2011, 43, 354–363. [Google Scholar] [CrossRef]
- Li, S.G.; Li, Y.J.; Wang, J.Q.; Ge, K.; Yang, L.B. Prediction of gas hydrate formation conditions in the presence of electrolytes using an N-NRTL-NRF activity coefficient model. Ind. Eng. Chem. Res. 2020, 59, 6269–6278. [Google Scholar] [CrossRef]
- Dholabhai, P.D.; Kalogerakis, N.; Bishnoi, P.R. Equilibrium conditions for carbon dioxide hydrate formation in aqueous electrolyte solutions. J. Chem. Eng. Data 1993, 38, 800–805. [Google Scholar] [CrossRef]

| System | 1.00 mol% | 2.00 % | 3.00% | |||
|---|---|---|---|---|---|---|
| T/K | P/MPa | T/K | P/MPa | T/K | P/MPa | |
| KCl | 274.15 | 3.17 | 274.15 | 3.59 | 274.15 | 4.18 |
| 276.15 | 3.94 | 276.15 | 4.45 | 276.15 | 5.15 | |
| 278.15 | 4.85 | 278.15 | 5.47 | 278.15 | 6.31 | |
| 280.15 | 5.89 | 280.15 | 6.75 | 280.15 | 7.92 | |
| 282.15 | 7.34 | 282.15 | 8.46 | 282.15 | 10.01 | |
| NaCl | 274.15 | 3.21 | 274.15 | 3.71 | 274.15 | 4.58 |
| 276.15 | 3.98 | 276.15 | 4.61 | 276.15 | 5.42 | |
| 278.15 | 4.92 | 278.15 | 5.68 | 278.15 | 6.71 | |
| 280.15 | 6.09 | 280.15 | 6.96 | 280.15 | 8.44 | |
| 282.15 | 7.52 | 282.15 | 8.82 | 282.15 | 10.71 | |
| KBr | 274.15 | 3.15 | 274.15 | 3.55 | 274.15 | 4.21 |
| 276.15 | 3.96 | 276.15 | 4.44 | 276.15 | 5.12 | |
| 278.15 | 4.86 | 278.15 | 5.51 | 278.15 | 6.3 | |
| 280.15 | 5.92 | 280.15 | 6.72 | 280.15 | 7.94 | |
| 282.15 | 7.31 | 282.15 | 8.51 | 282.15 | 9.98 | |
| NaBr | 274.15 | 3.25 | 274.15 | 3.68 | 274.15 | 4.61 |
| 276.15 | 4.02 | 276.15 | 4.58 | 276.15 | 5.38 | |
| 278.15 | 4.88 | 278.15 | 5.72 | 278.15 | 6.73 | |
| 280.15 | 6.12 | 280.15 | 6.98 | 280.15 | 8.42 | |
| 282.15 | 7.54 | 282.15 | 8.77 | 282.15 | 10.72 | |
| System | c/mol% | T/K | P/MPa | z | ∆Hdiss/kJ·mol−1 |
|---|---|---|---|---|---|
| Deionized water | / | 273.15–282.15 | 2.84~6.46 | 0.9309~0.8697 | −59.42 |
| KCl | 1.00 | 273.15–282.15 | 3.17~7.34 | 0.9231~0.8553 | −59.80 |
| 2.00 | 273.15–282.15 | 3.59~8.46 | 0.9133~0.8387 | −60.40 | |
| 3.00 | 273.15–282.15 | 4.18~10.01 | 0.8999~0.8195 | −62.21 | |
| NaCl | 1.00 | 273.15–282.15 | 3.21~7.42 | 0.9222~0.8330 | −59.30 |
| 2.00 | 273.15–282.15 | 3.71~8.65 | 0.9106~0.8121 | −57.84 | |
| 3.00 | 273.15–282.15 | 4.78~10.56 | 0.8865~0.7864 | −58.52 | |
| KBr | 1.00 | 273.15–282.15 | 3.15~7.31 | 0.9236~0.8350 | −58.98 |
| 2.00 | 273.15–282.15 | 3.55~8.51 | 0.9143~0.8143 | −60.11 | |
| 3.00 | 273.15–282.15 | 4.21~9.98 | 0.8992~0.7932 | −58.89 | |
| NaBr | 1.00 | 273.15–282.15 | 3.20~7.43 | 0.9224~0.8328 | −59.22 |
| 2.00 | 273.15–282.15 | 3.71~8.75 | 0.9106~0.8105 | −58.48 | |
| 3.00 | 273.15–282.15 | 4.75~10.46 | 0.8871~0.7875 | −58.03 |
| System | c/mol% | N | AARD/% |
|---|---|---|---|
| KCl | 1.00 | 5 | 1.24 |
| 2.00 | 5 | ||
| 3.00 | 5 | ||
| NaCl | 1.00 | 5 | 1.08 |
| 2.00 | 5 | ||
| 3.00 | 5 | ||
| KBr | 1.00 | 5 | 1.18 |
| 2.00 | 5 | ||
| 3.00 | 5 | ||
| NaBr | 1.00 | 5 | 1.21 |
| 2.00 | 5 | ||
| 3.00 | 5 |
| Chemical | Chemical Structure | Purity | Supplier | CAS |
|---|---|---|---|---|
| Potassium chloride | KCl | 99.99% | Shanghai Aladdin Biochemical Technology Co., Ltd. (Shanghai, China) | 7447-40-7 |
| Sodium chloride | NaCl | 99.99% | Shanghai Aladdin Biochemical Technology Co., Ltd. (Shanghai, China) | 7647-14-5 |
| Potassium bromide | KBr | 99.99% | Shanghai Aladdin Biochemical Technology Co., Ltd. (Shanghai, China) | 7758-02-3 |
| Sodium bromide | NaBr | 99.99% | Shanghai Aladdin Biochemical Technology Co., Ltd. (Shanghai, China) | 7647-15-6 |
| Deionized water | H2O | 18.0MΩ·cm | Smart-Q15 ultrapure water machine (Suzhou, China) | 732-18-5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, Q.; Chen, M.; Pang, W.; Xu, Z.; Liu, Z.; Wen, H.; Lei, X. Experimental and Modeling Study on Methane Hydrate Equilibrium Conditions in the Presence of Inorganic Salts. Molecules 2024, 29, 3702. https://doi.org/10.3390/molecules29153702
Fu Q, Chen M, Pang W, Xu Z, Liu Z, Wen H, Lei X. Experimental and Modeling Study on Methane Hydrate Equilibrium Conditions in the Presence of Inorganic Salts. Molecules. 2024; 29(15):3702. https://doi.org/10.3390/molecules29153702
Chicago/Turabian StyleFu, Qiang, Mingqiang Chen, Weixin Pang, Zhen Xu, Zengqi Liu, Huiyun Wen, and Xin Lei. 2024. "Experimental and Modeling Study on Methane Hydrate Equilibrium Conditions in the Presence of Inorganic Salts" Molecules 29, no. 15: 3702. https://doi.org/10.3390/molecules29153702
APA StyleFu, Q., Chen, M., Pang, W., Xu, Z., Liu, Z., Wen, H., & Lei, X. (2024). Experimental and Modeling Study on Methane Hydrate Equilibrium Conditions in the Presence of Inorganic Salts. Molecules, 29(15), 3702. https://doi.org/10.3390/molecules29153702








