Review of the Interfacial Structure and Properties of Surfactants in Petroleum Production and Geological Storage Systems from a Molecular Scale Perspective
Abstract
1. Introduction
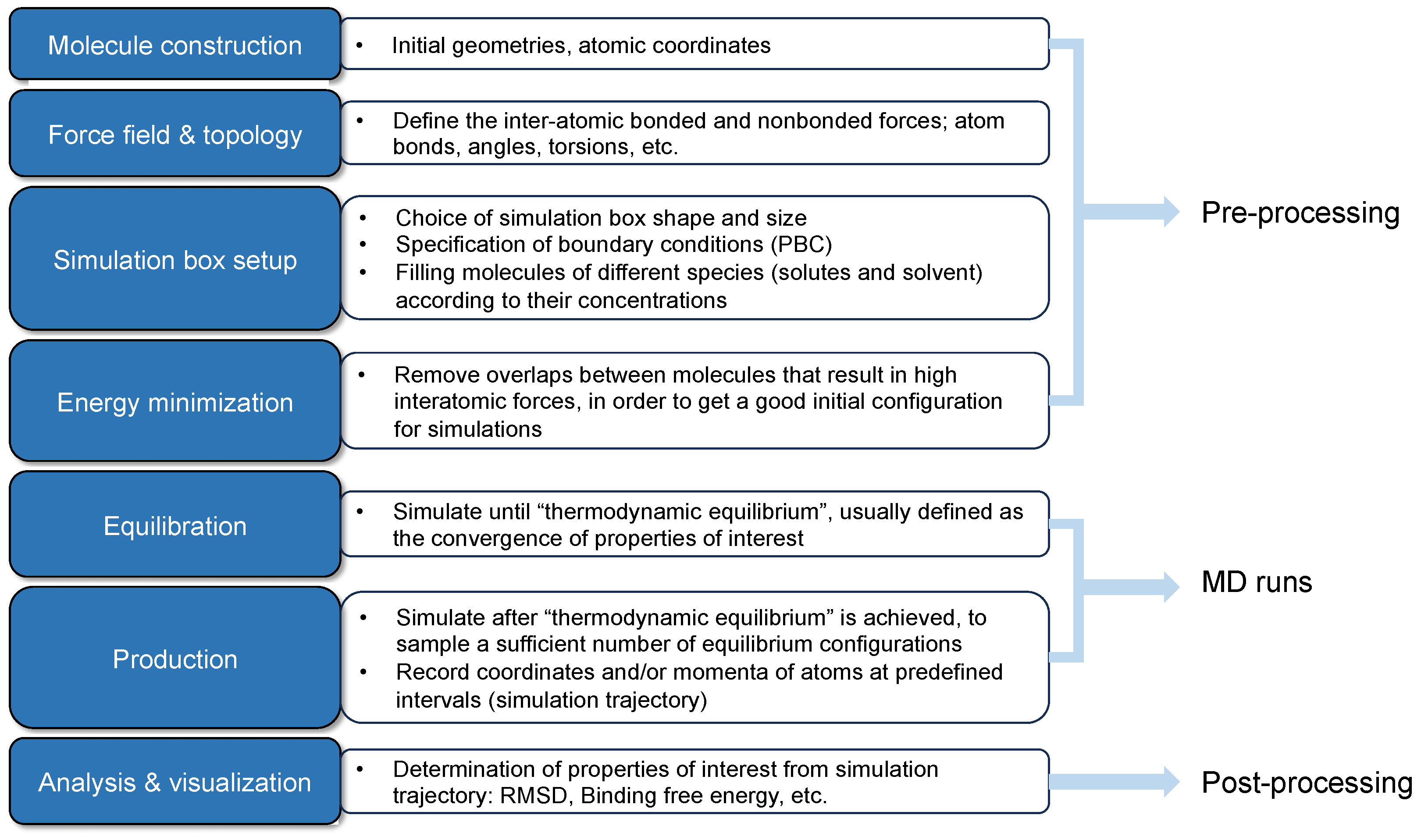
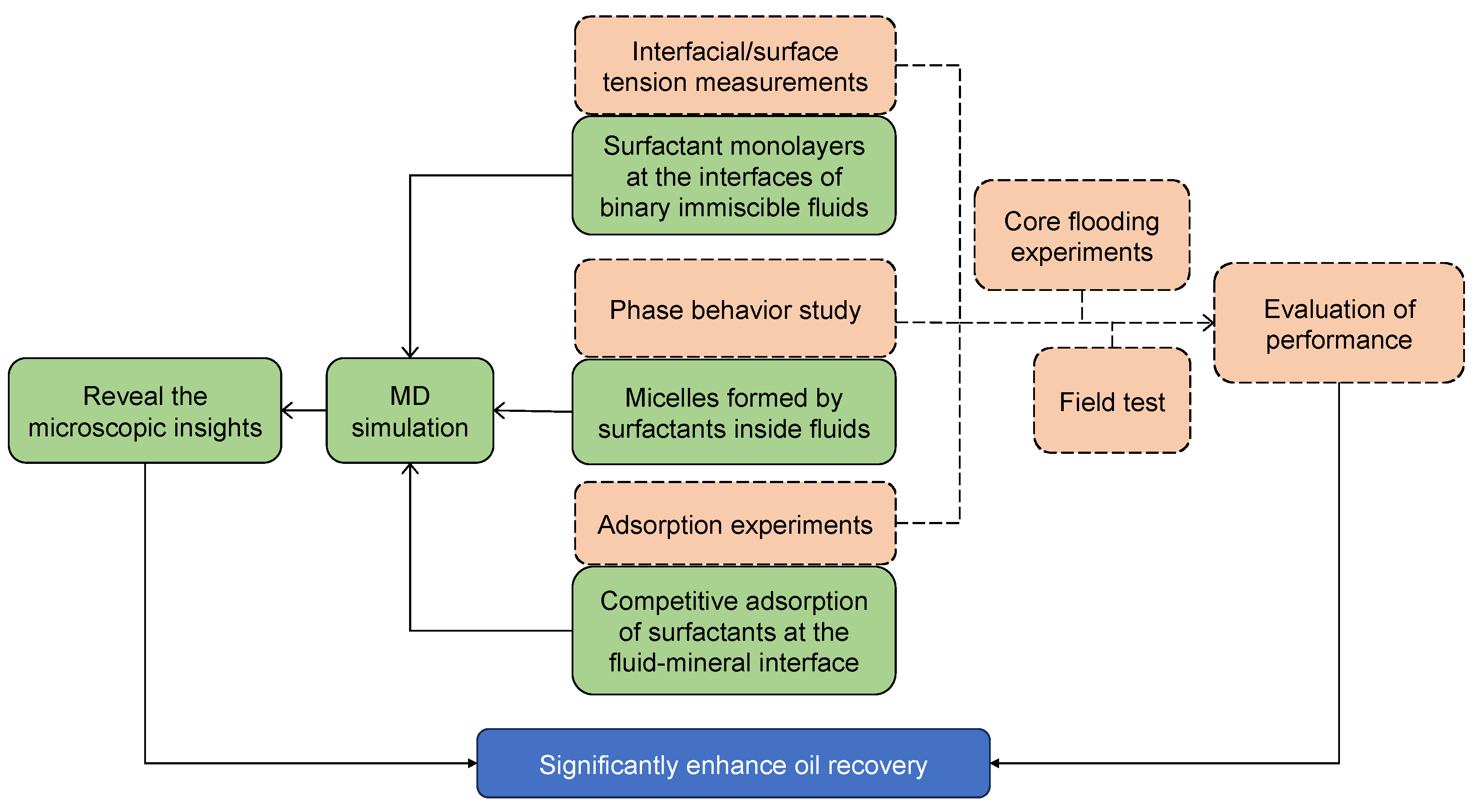
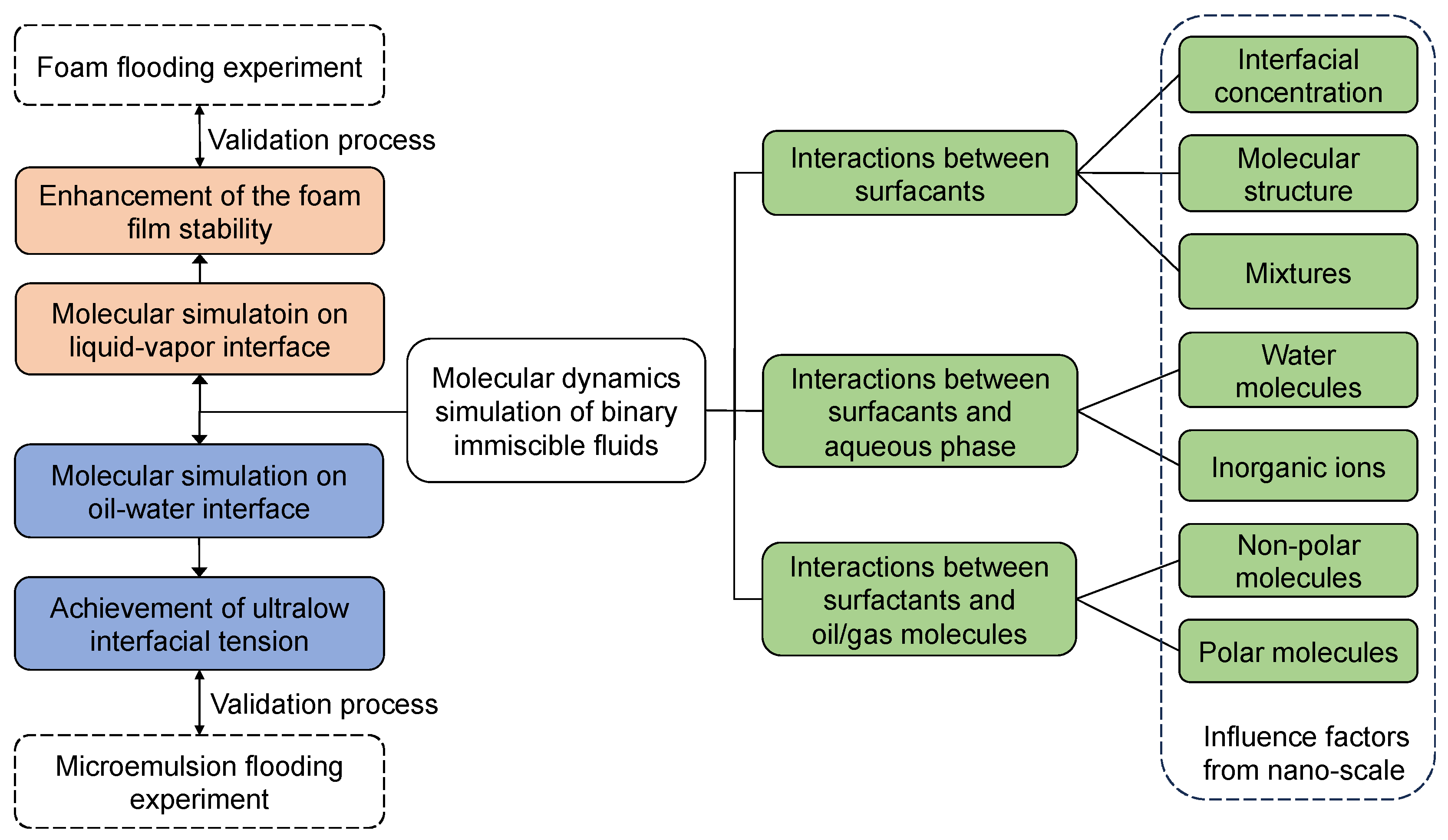
2. Research Progress
2.1. Interfacial Properties of the Surfactant-Formed Monolayers
2.1.1. Evaluation Method for Interfacial Properties
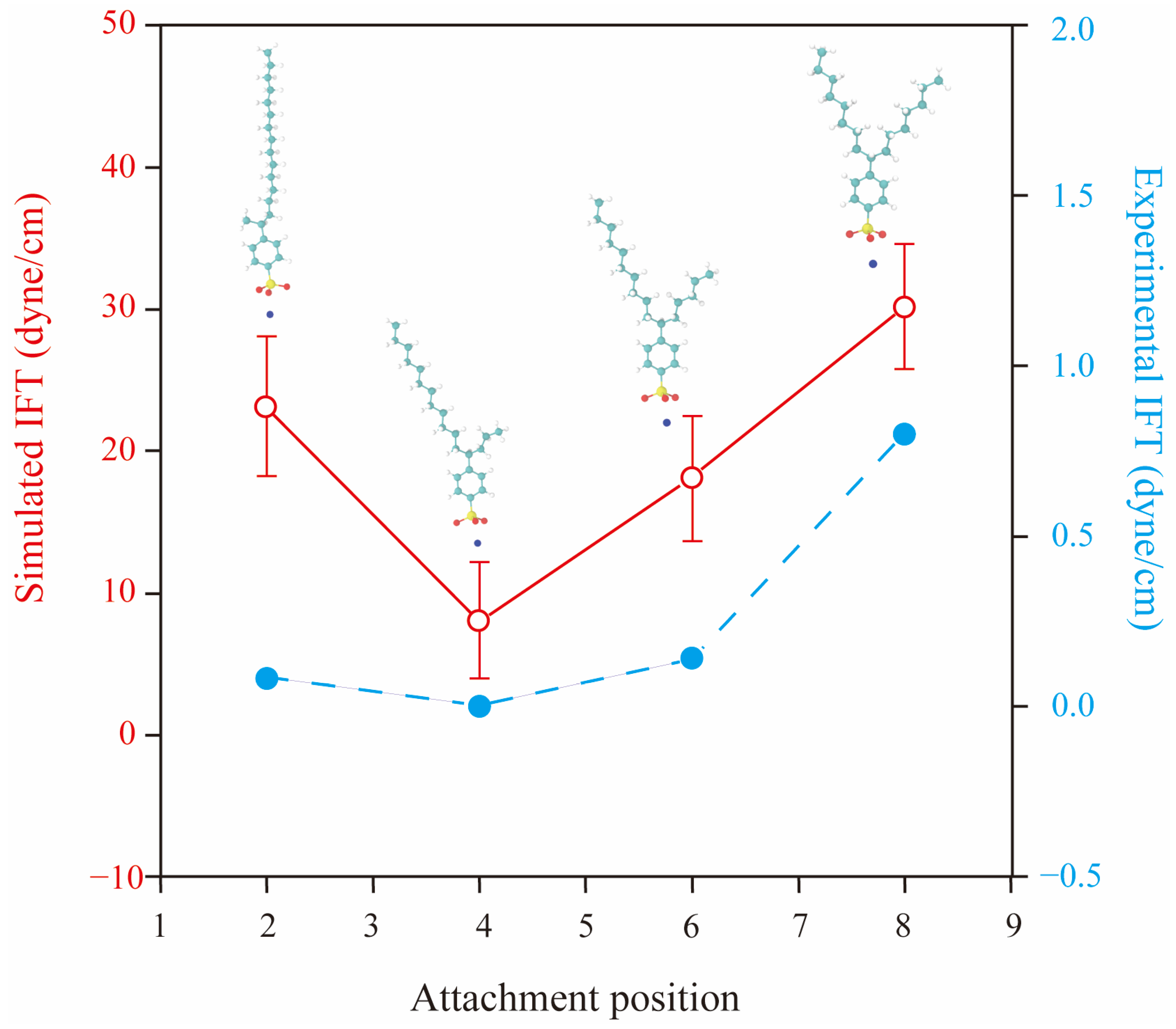
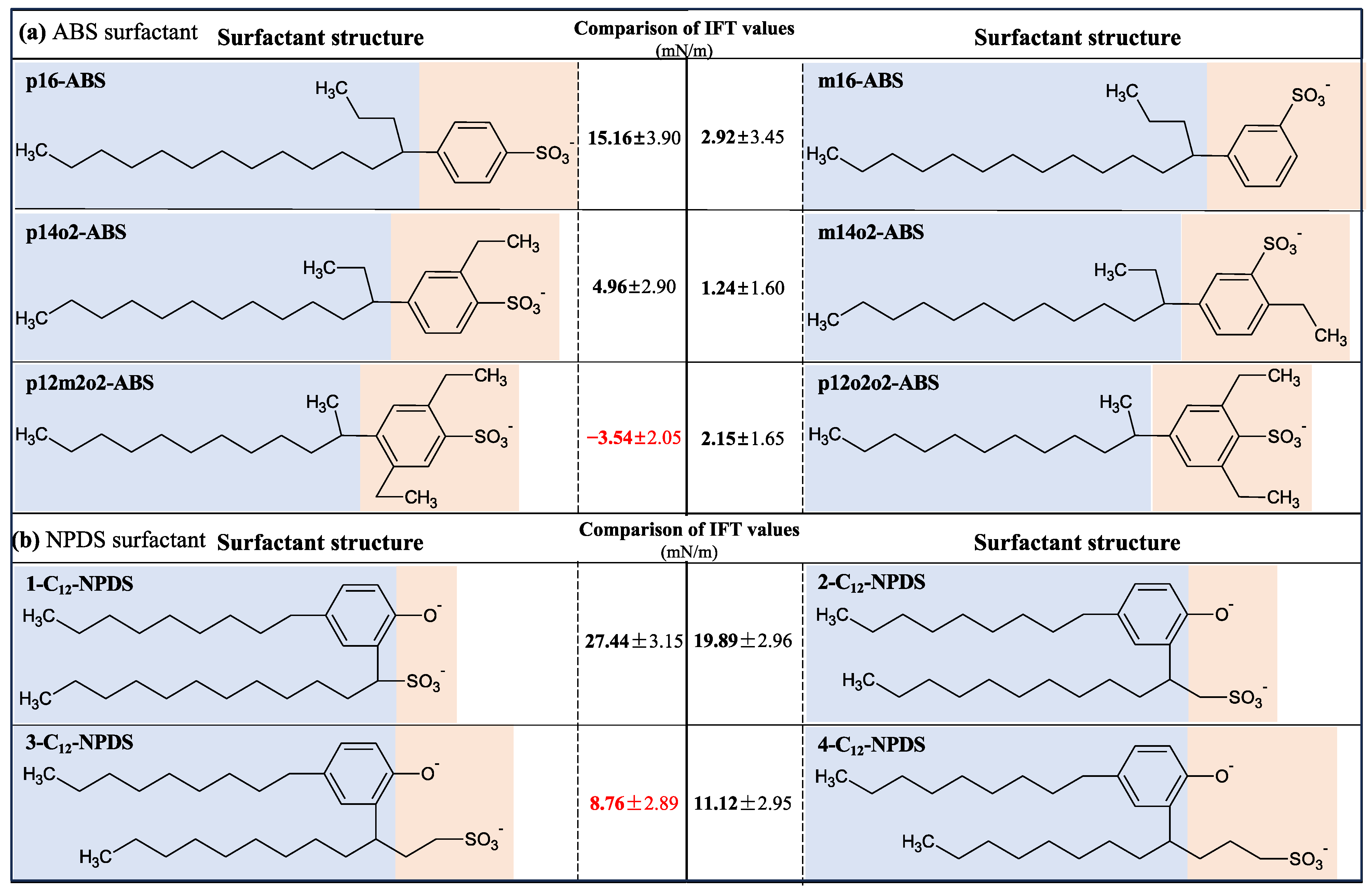
2.1.2. Effect of Interfacial Concentration and Molecular Structure
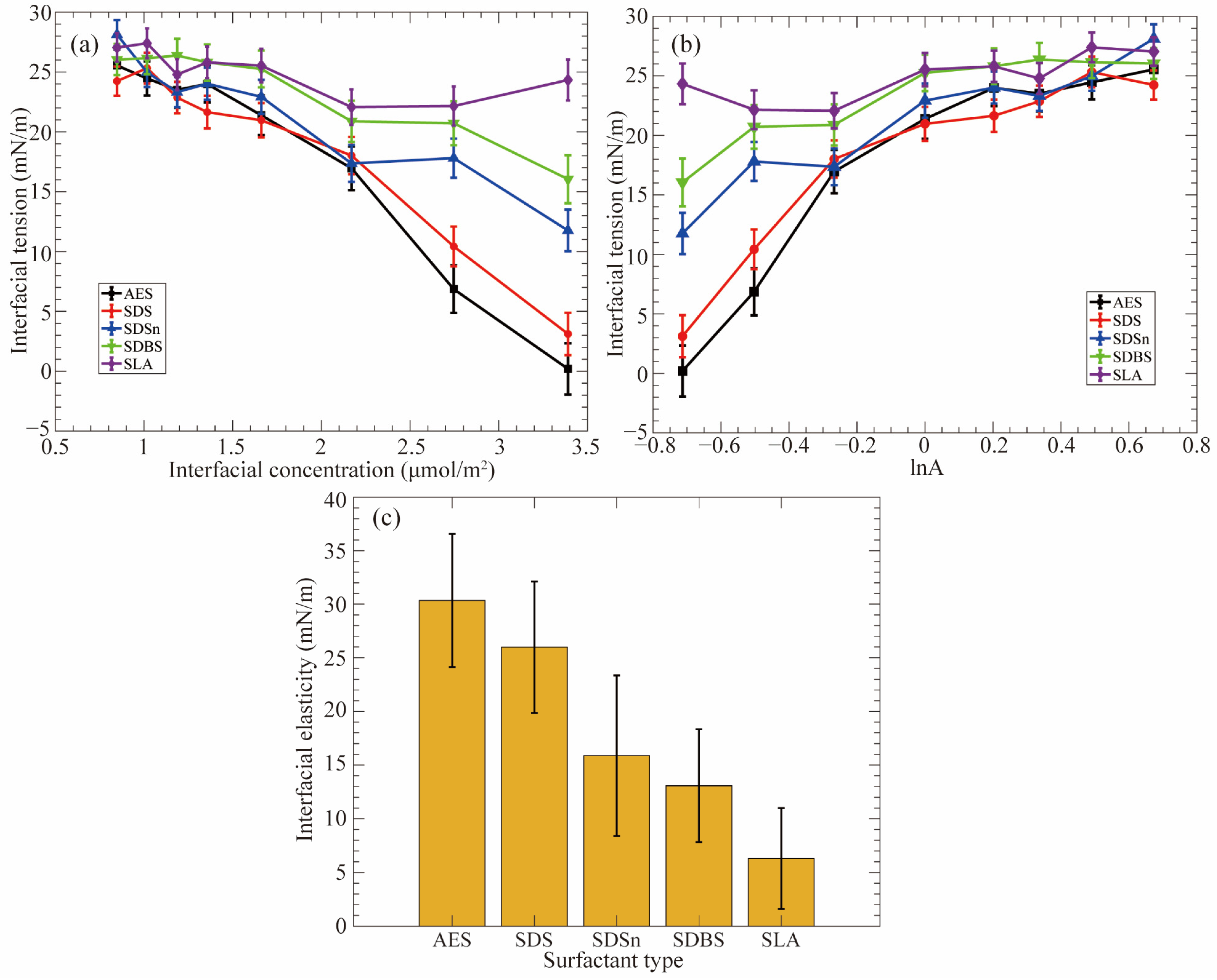
2.1.3. Synergistic Effect of Surfactant Mixtures
2.2. Molecular Views of the Interfacial Structure
2.2.1. Characterization of the Microstructure at the Interface
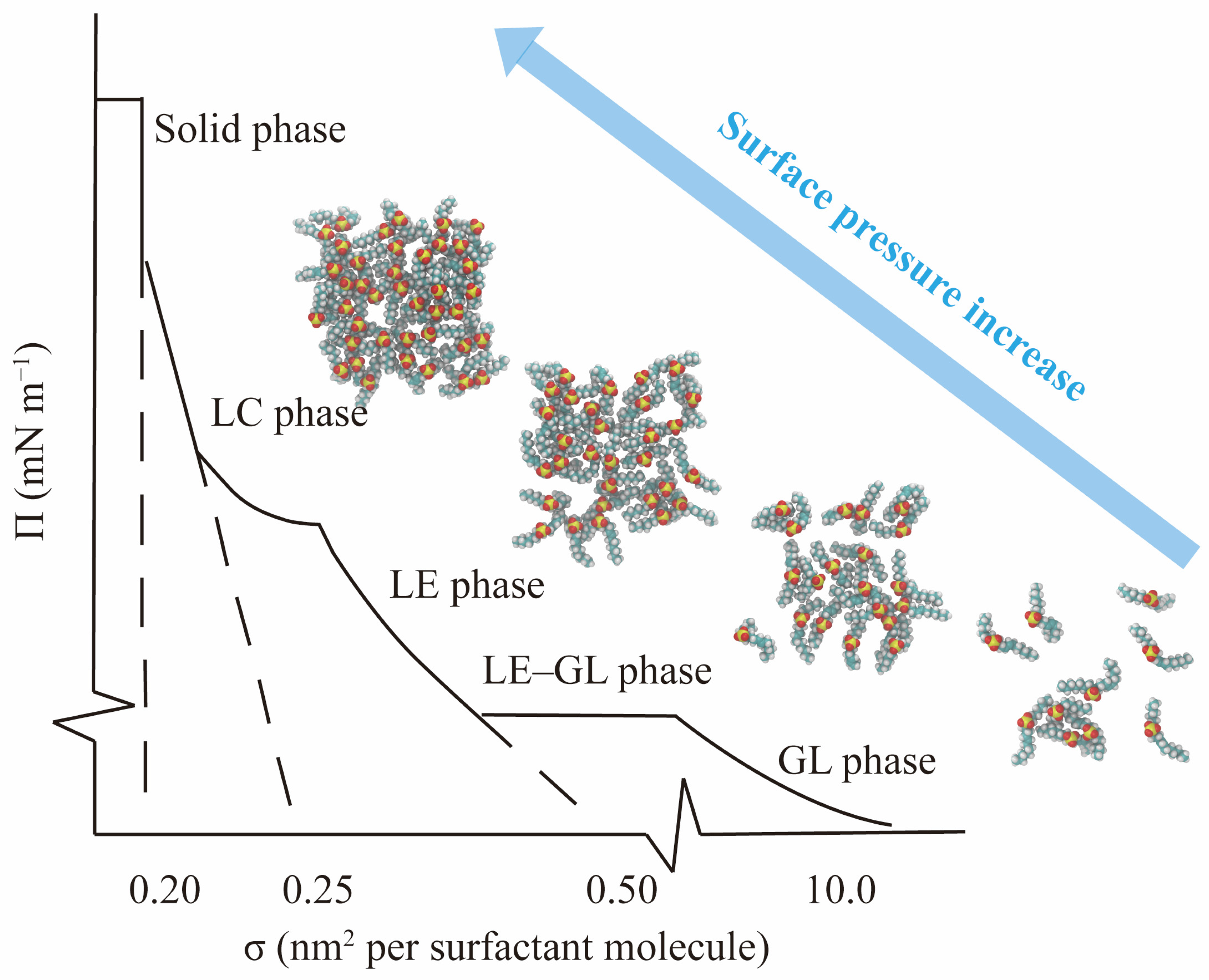
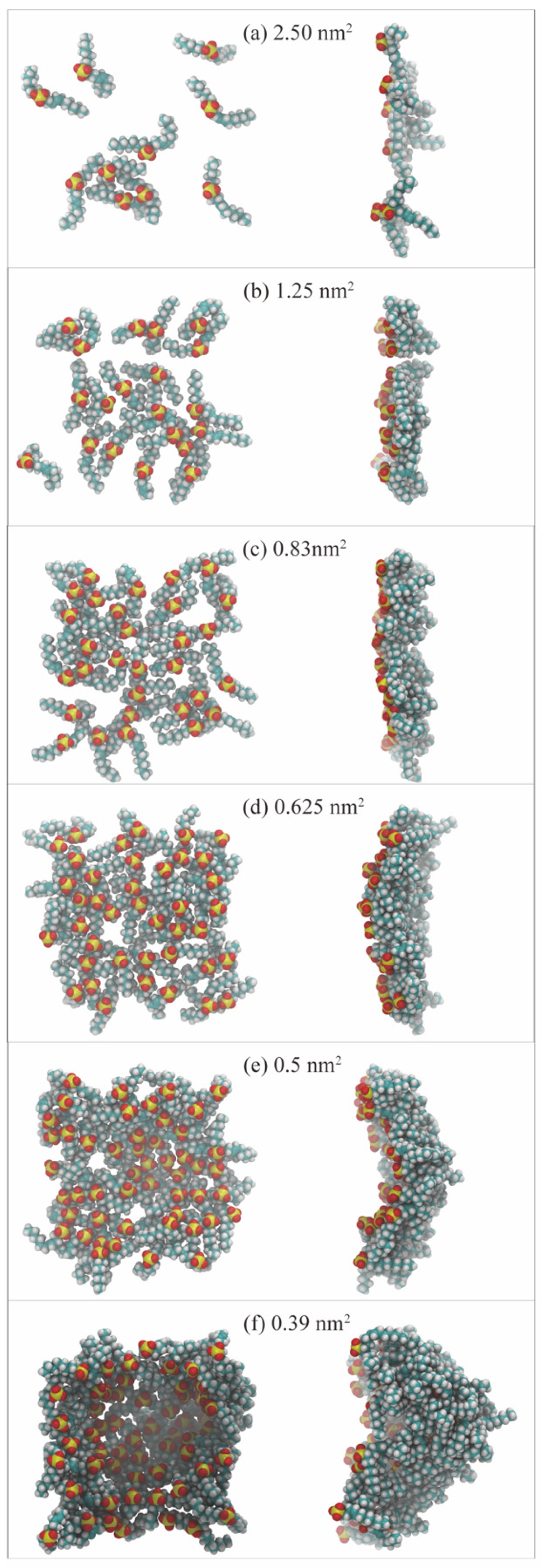
2.2.2. Effect of Interfacial Concentration on the Packing State of the Surfactants
- (1)
- When the number of surfactant molecules at the interface is very few, the SAPM value is large (2.5 and 1.25 nm2 per surfactant molecule), as shown in panels a and b. The separation distances between the molecules are relatively large. In this circumstance, the interaction force between each other can be negligible. This state is called the gas-like (GL) phase. Since the molecular arrangements of the monolayers are sparse and the resulting interfacial widths are small, the interfacial performance of the monolayer is poor, and many hydrocarbon molecules can directly contact water molecules at the intermediate region via the gap that the surfactant molecules are not occupying.
- (2)
- As the number of surfactant molecules increases at the interface, SAPM values decrease, as shown in panels c and d, and the interaction force between each surfactant molecule is enhanced. This state is called the liquid-expanded (LE) phase. At this moment, the monolayers become denser than those in the GL phase, and the orientation angles of surfactant alkyl tails are randomly distributed toward the oil/gas phase. The void space that remains in the monolayers allows for continued interaction between oil/gas and water molecules still occurs.
- (3)
- When the number of surfactant molecules reaches the saturation concentration at the interface, SAPM reaches the critical minimum point (0.5 nm2 per surfactant molecule), as shown in panel e. The molecular arrangement of the monolayers changes from a loosely packed pattern to a densely packed pattern, marking the transition to the liquid-condensed (LC) phase. In the LC phase, surfactant molecules are distributed close to each other, and most of the surfactant alkyl tails tend to be perpendicular to the interface. The absence of void space in the monolayers and the resulting largest interfacial widths allow for the best performance, effectively preventing the interactions and contacts between oil/gas and water molecules in the intermediate region.
- (4)
- When the interfacial concentration exceeds the concentration of saturation coverage, the interface becomes visibly curved (a concave surface), as shown in panel f. The interface becomes unstable and can undergo mechanical buckling to increase the interfacial area so that excessive surfactant molecules can be adsorbed at the contact surface between the oil/gas and water phases. In this circumstance, some surfactant molecules in the monolayers can also escape from the interface and form stable 3D structures such as vesicles and bilayers. As a result, the stability of the monolayer can recover. The interfacial properties change to different degrees as the shape of the surfactant monolayers changes over time.
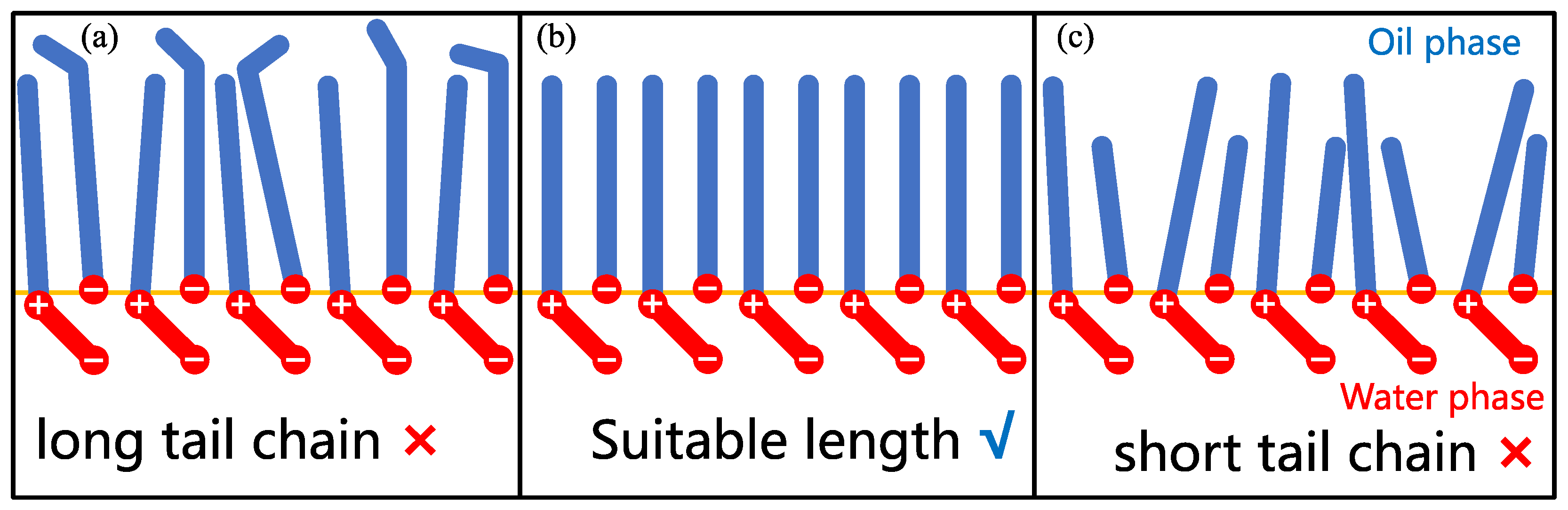
2.2.3. Effect of Molecular Structure and Synergism on Monolayers’ Morphology
2.3. Surfactant Headgroup Solvation and Counterion Effect in Aqueous Phase
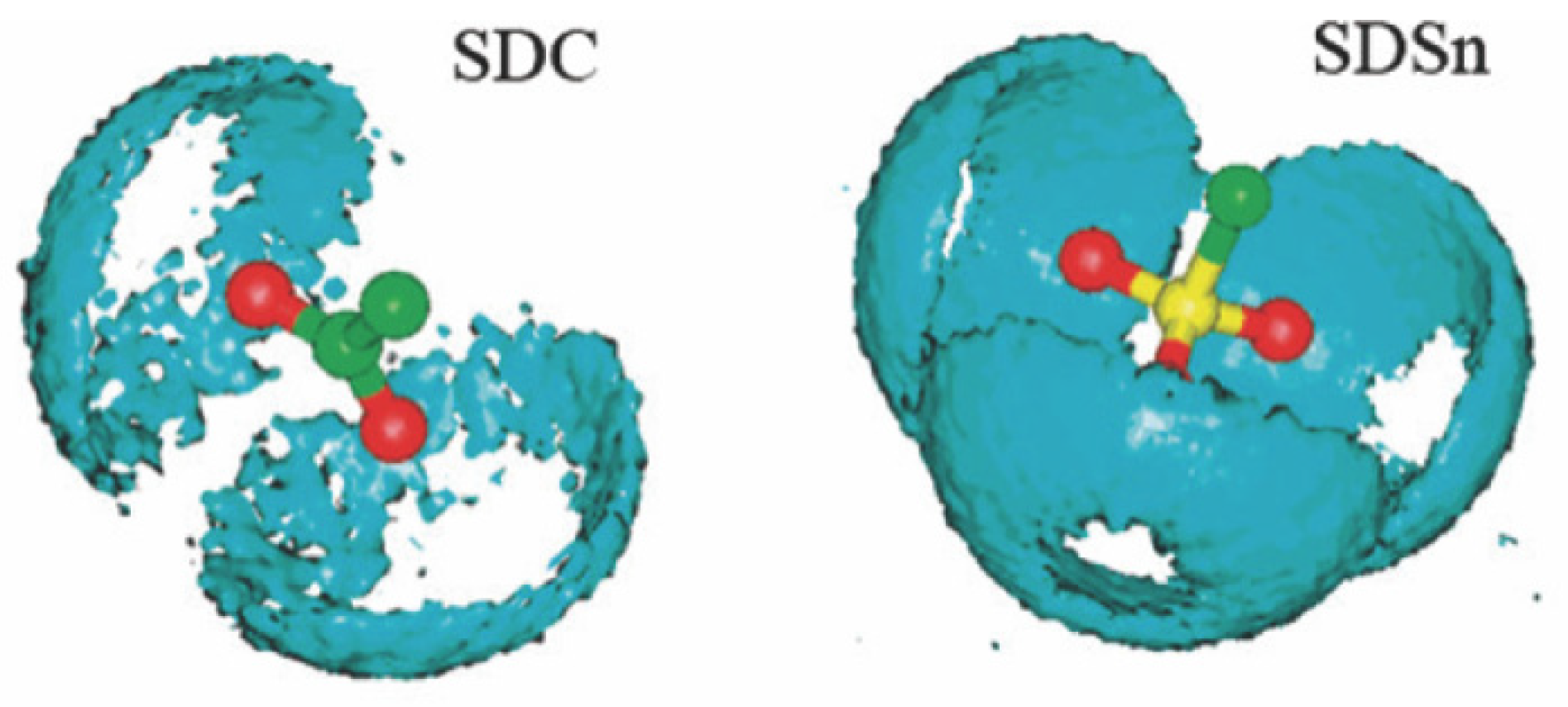
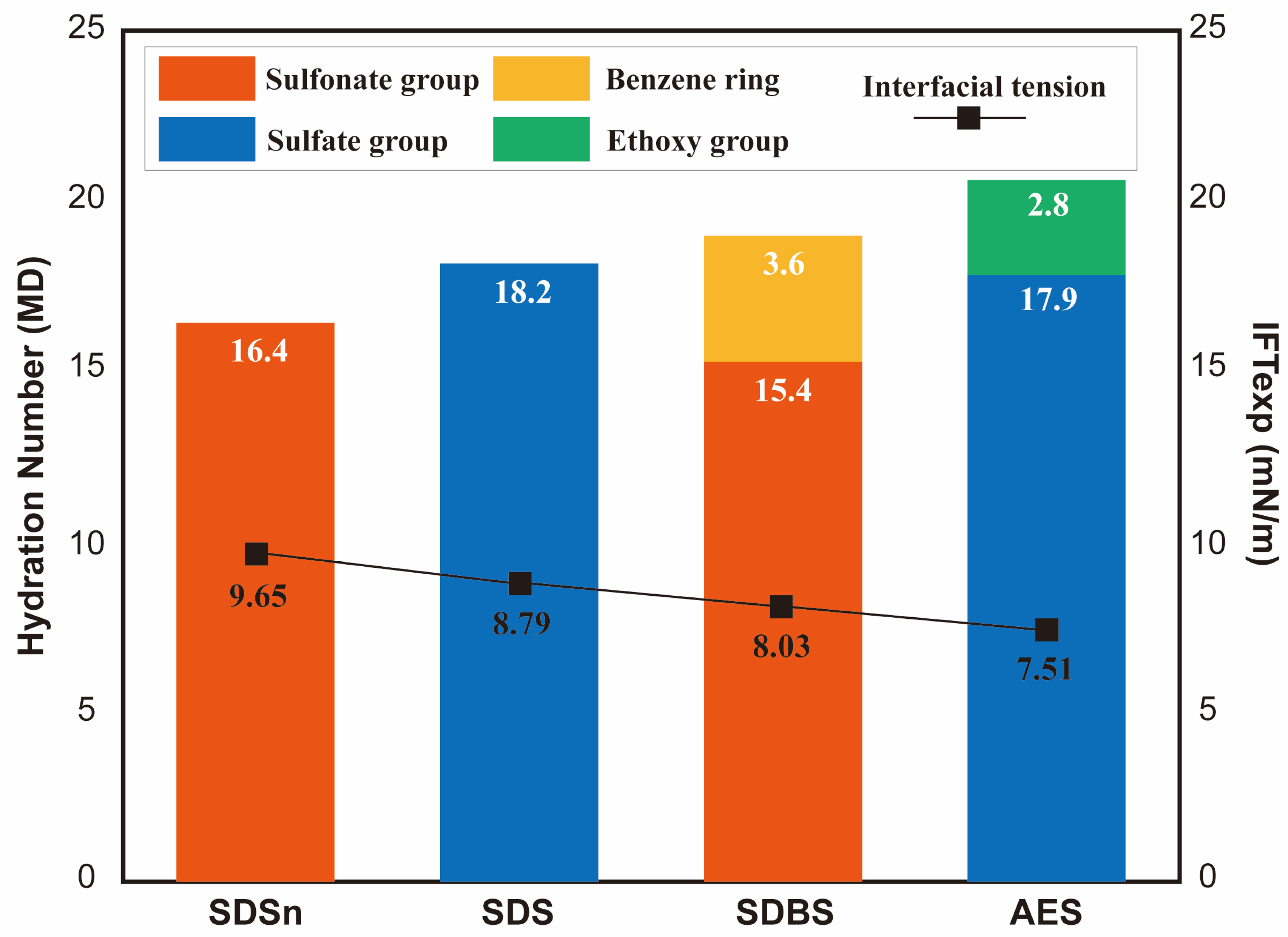
2.3.1. Hydration Shell Structure and Hydrogen Bonding
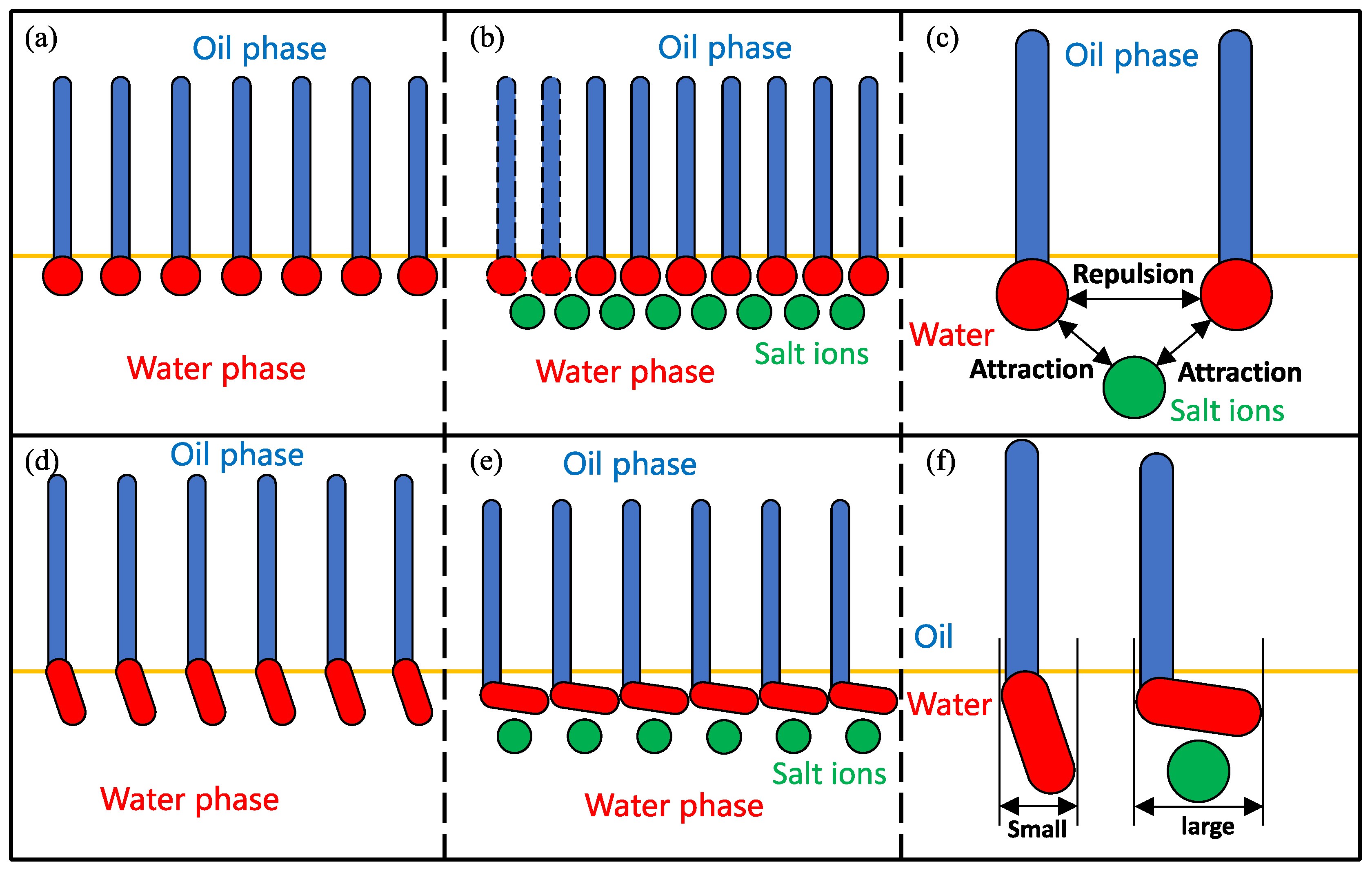
2.3.2. Influences of Inorganic Salt Ions
2.4. Interactions between Surfactant Alkyl Tails and Hydrophobic Phase
3. Conclusions and Outlook
- (1)
- Interactions between the surfactant molecules within the monolayers: with the increase in interfacial concentration, the formed monolayers undergo the process of “GL dispersion–LE phase–LC phase–undulation state–protruding bud structure–restoration of flatness”. In addition, modifying the molecular structure can enhance the interfacial performance of the surfactants. The measures include increasing the size of the headgroups, introducing extra hydrophilic radical groups, polymerizing the monomer molecules, as well as shortening and coarsening the linear-chain molecules. When applying the surfactant mixtures (i.e., synergistic effect), surfactant molecules of small size would be inserted into the gaps between the large surfactant molecules, improving the integrity degree of the monolayers, thus preventing the free diffusion of molecules and the contact between the two immiscible phases.
- (2)
- Interactions between the surfactant monolayers and the water phase: a clear hydration shell (which consists of bound water and captured water) exists near the hydrophilic headgroups of the surfactant. The number of water molecules in the hydrated layers and the number of hydrogen bonds, which quantitatively characterize the hydrophilicity of various headgroups, can be obtained from the MD simulation method. For ionic surfactant molecules, the inorganic salt ions shield the hydrophilic headgroups from electrostatic repulsions, which leads to more surfactant molecules being enriched at the interface. For nonionic surfactant molecules, the salt ions change the orientation of the hydrophilic headgroups, thus improving the degree of interfacial coverage of the monolayers.
- (3)
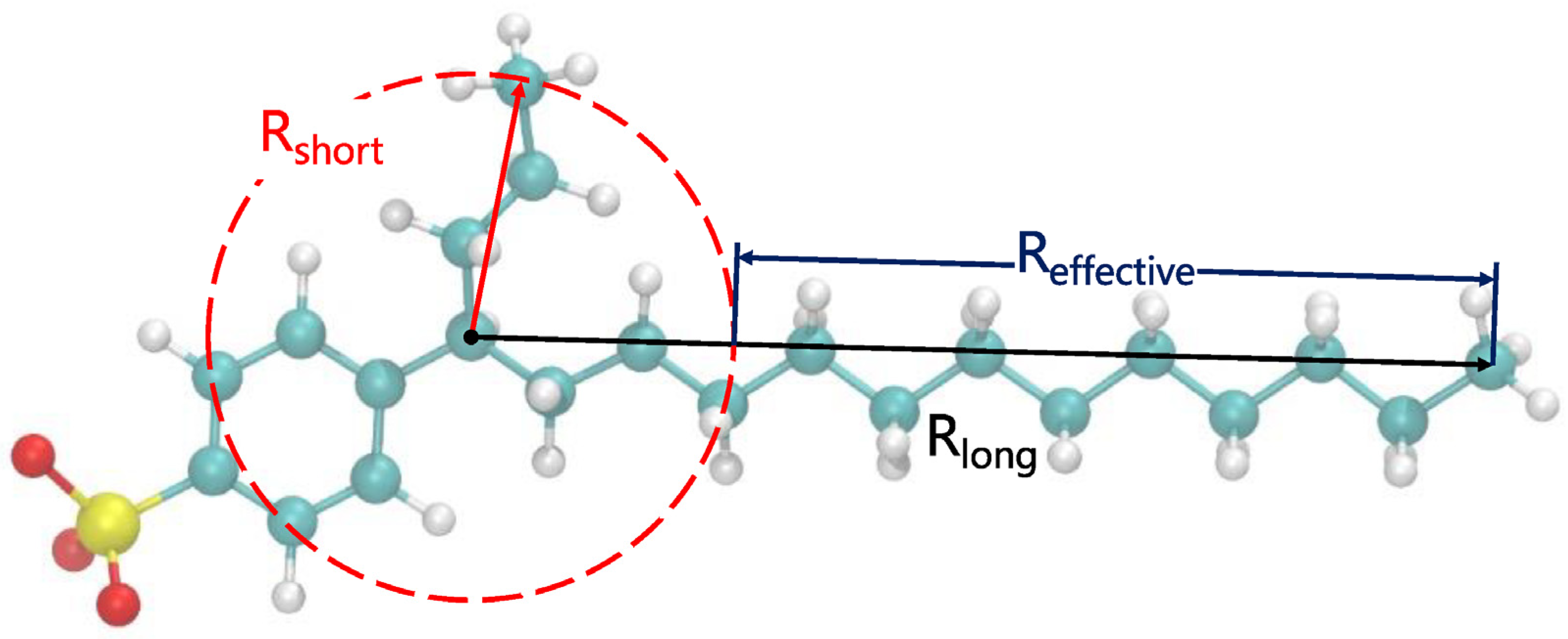
- Interactions between the surfactant monolayers and the hydrocarbon phase: most of the molecules (such as natural gas, paraffin, and aromatic hydrocarbons) are nonpolar, whereas resins and asphaltene are polar molecules. The nonpolar molecules would interact with the surfactant alkyl tail via van der Waals force. Thus, the molecular configurations at the gas–liquid interface are more disordered. As to nonpolar molecules in the oil phase (such as n-alkanes), the EATL method, using MD simulations, clarifies the matching relationship between the branched structures in the hydrophobic carbon tails and the components of the oil phase. The modeling of the crude oil composition by MD simulations has evolved from the initial pure n-alkanes to multicomponent simulated oils (i.e., digital oil) containing polar compounds. However, the influence of polar molecules with large sizes in crude oil on the interfacial properties of the surfactant monolayers still needs further study.
- (1)
- Upgrade the spatial and temporal scales. Currently, the dimensions of the simulated systems in most MD simulations are less than 20 nm for the sake of computational efficiency [36]. Expanding the spatial scale of simulations to hundreds of nanometers is crucial to eliminate the randomness of the predictions caused by the size effect. Meanwhile, only if a simulation is ergodic and long enough to allow the system to visit all its energetically relevant states can we derive meaningful information from it [47]. These are beneficial to describe the enrichment process of the surfactant molecules from the interior of the bulk phase to the interface and desorption from the interface under various conditions. Under these circumstances, coarse-grained MD and DPD simulations are recommended [122,123], which can model molecular behaviors from hundreds of nanometers to several micrometers (i.e., with a mesoscopic perspective).
- (2)
- Accurate description of the interface system. Unlike modeling of the bulk phase of the fluids, the intermediate regions in binary fluid systems are heterogeneous. Regarding van der Waals’ interaction, an insufficient cut-off distance for intermolecular interaction would lead to significant artifacts in microstructure and properties at the interfaces [124]. Furthermore, the cut-off scheme’s dispersion correction significantly affects the system’s adsorption process in which the Coulomb force is not strong enough. Lennard-Jones potential with the particle-mesh Ewald (LJ-PME) scheme is a potential solution for this issue [125]. In addition, the commonly used force fields [126,127,128] are developed for specific purposes (e.g., phase behaviors). The simulation results for the interface system may not be quantitatively compared with each other. The existing force fields should be continuously improved with reference to first-principles calculations and experimental values [129,130,131]. The combination of the MD simulation method and machine learning (ML) techniques may provide a fast and cost-effective IFT determination over multiple and complex fluid–fluid and fluid–solid interfaces (i.e., inhomogeneous systems) [132]. The relationship between the IFT, fluid composition, and thermodynamic conditions may involve several variables. In this context, machine learning can be a suitable approach to correlating physical and chemical properties in a single and robust model.
Funding
Acknowledgments
Conflicts of Interest
List of Abbreviations
| ABS | Alkyl Benzene Sulfonate |
| AEC | Alkyl Polyoxyethylene Carboxylate |
| AES | Sodium Polyoxyethylene Alkyl Ether Sulfate |
| AOS | Sodium α-olefin Sulfonate |
| BARR | Energy Barrier |
| CAPB | Cocamidopropyl Betaine |
| CAB | Lauramidopropyl Betaine |
| CM | Contact Minimum |
| CMC | Critical Micelle Concentration |
| C6C5E3 | Triethyleneglyco 6-Dodecyl Ether |
| C12E2 | Dodecyl Diethylene Glycol Ether |
| C12E3 | Triethyleneglycol 1-Dodecyl Ether |
| DTAB | Dodecyl Trimethylammonium Bromide |
| DSB | Dodecyl Sulfonate Betaine |
| EACN | Equivalent Alkane Carbon Number |
| EATL | Effective Alkyl Tail Length |
| EDL | Electric Double Layer |
| EOR | Enhanced Oil Recovery |
| GPU | Graphics Processing Units |
| GL | Gas-Like Phase |
| HLB | Hydrophile Lipophile Balance |
| HCB | Hydrophilic–CO2-philic Balance |
| IOS | Internal Olefin Sulfonate |
| IFE | Interface Formation Energy |
| IFT | Interfacial Tension |
| LAA | Lauryl Alkanolamide |
| LAS | Linear Alkylbenzene Sulfonate |
| LB | Lauryl Betaine |
| LE | Liquid-Expanded Phase |
| LC | Liquid-Condensed Phase |
| LJ | Lennard-Jones Potential |
| MD | Molecular Dynamics |
| ML | Machine Learning |
| NPnAT | Isobaric–Isothermal–Isointerface Area Ensemble |
| NPDS | Nonylphenol-Substituted Dodecyl sulfonates |
| ODC | 4,4′-Oxydianilinium Chloride |
| PFOS | Perfluorooctane Sulfonate |
| PMF | Potent Mean Force |
| PME | Particle-Mesh Ewald |
| QMR | Quantitative Molecular Representation |
| RDF | Radial Distribution Function |
| Rha-C10-C10 | Rhamnolipid |
| SAPM | Surface Area Per Molecule |
| SDF | Spatial Distribution Function |
| SDC | Dodecyl Carboxylate |
| SDS | Sodium Dodecyl Sulfate |
| SDSn | Sodium Decyl Sulfonate |
| SDBS | Sodium Dodecylbenzene Sulfonate |
| SHBS | Sodium Hexadecane Benzene Sulfonate |
| SLA | Sodium Laurate |
| SSM | Solvent-Separated Minimum |
| SOW | Surfactant–Oil–Water |
References
- Manrique, E.; Thomas, C.; Ravikiran, R.; Izadi, M.; Lantz, M.; Romero, J.; Alvarado, V. EOR: Current status and opportunities. In Proceedings of the SPE Improved Oil Recovery Symposium, Tulsa, OK, USA, 24 April 2010; OnePetro: Richardson, TX, USA, 2010; p. SPE-130113-MS. [Google Scholar] [CrossRef]
- Alfarge, D.; Wei, M.; Bai, B. IOR methods in unconventional reservoirs of North America: Comprehensive review. In Proceedings of the SPE Western Regional Meeting, Bakersfield, CA, USA, 23 April 2017; OnePetro: Richardson, TX, USA, 2017; p. SPE-185640-MS. [Google Scholar] [CrossRef]
- Al-Shargabi, M.; Davoodi, S.; Wood, D.A.; Rukavishnikov, V.S.; Minaev, K.M. Carbon dioxide applications for enhanced oil recovery assisted by nanoparticles: Recent developments. ACS Omega 2022, 7, 9984–9994. [Google Scholar] [CrossRef] [PubMed]
- Mokheimer, E.M.A.; Hamdy, M.; Abubakar, Z.; Shakeel, M.R.; Habib, M.A.; Mahmoud, M. A comprehensive review of thermal enhanced oil recovery: Techniques evaluation. J. Energy Resour. Technol. 2019, 141, 030801. [Google Scholar] [CrossRef]
- Kamal, M.S.; Hussein, I.A.; Sultan, A.S. Review on surfactant flooding: Phase behavior, retention, IFT, and field applications. Energy Fuels 2017, 31, 7701–7720. [Google Scholar] [CrossRef]
- Olajire, A.A. Review of ASP EOR (alkaline surfactant polymer enhanced oil recovery) technology in the petroleum industry: Prospects and challenges. Energy 2014, 77, 963–982. [Google Scholar] [CrossRef]
- Sagbana, P.I.; Abushaikha, A.S. A comprehensive review of the chemical-based conformance control methods in oil reservoirs. J. Petrol. Explor. Prod. Technol. 2021, 11, 2233–2257. [Google Scholar] [CrossRef]
- Nazar, M.F.; Shah, S.S.; Khosa, M.A. Microemulsions in enhanced oil recovery: A review. Pet. Sci. Technol. 2011, 29, 1353–1365. [Google Scholar] [CrossRef]
- Talebian, S.H.; Masoudi, R.; Tan, I.M.; Zitha, P.L.J. Foam assisted CO2-EOR: A review of concept, challenges, and future prospects. J. Petrol. Sci. Eng. 2014, 120, 202–215. [Google Scholar] [CrossRef]
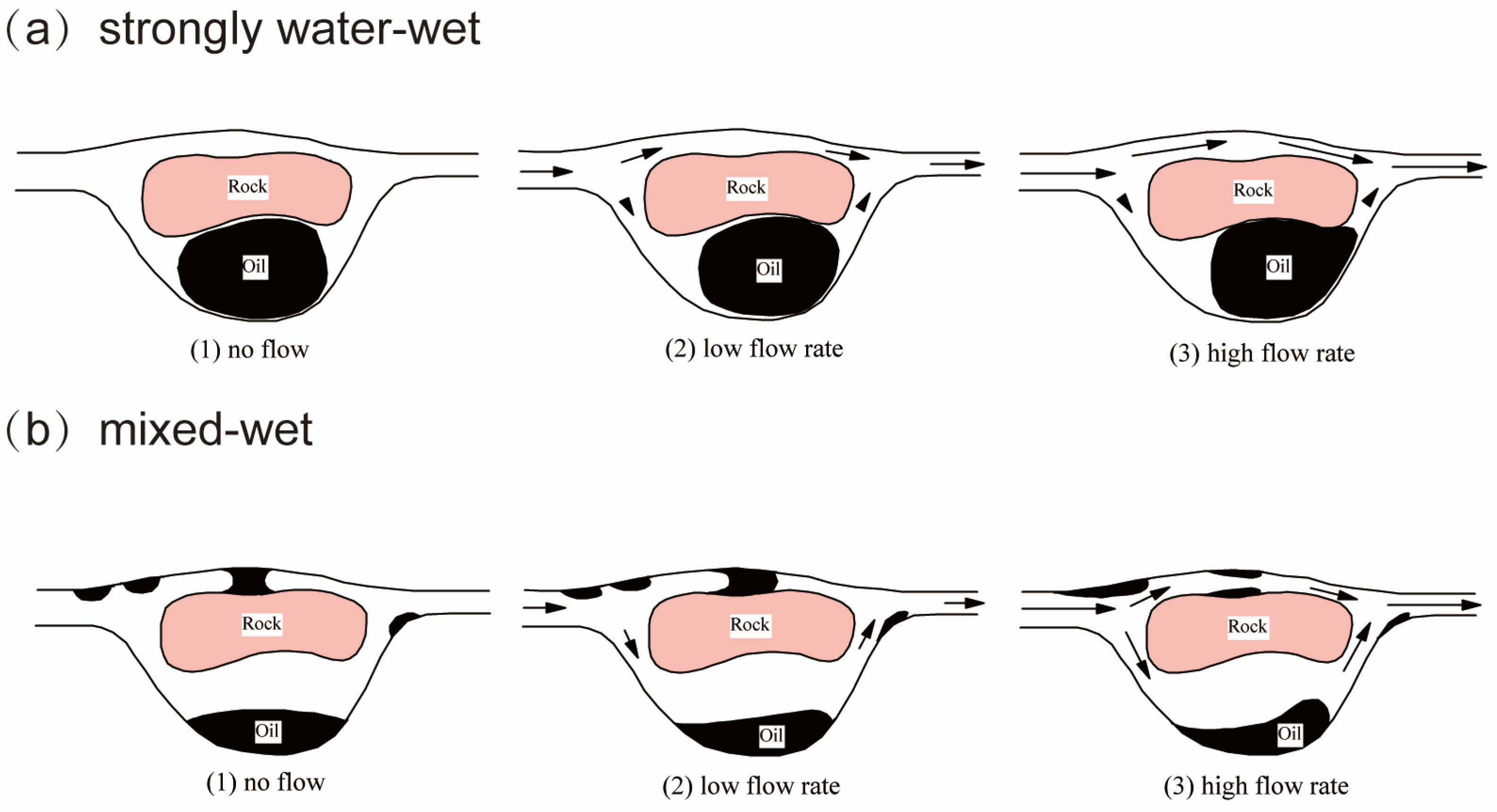
- Wang, J.X.; Buckley, J.S. Wettability and rate effects on end-point relative permeability to water. In Proceedings of the International Symposium of the Society of Core Analysts, Golden, CO, USA, 1–4 August 1999. [Google Scholar]
- Li, X.; Pu, C.; Chen, X.; Huang, F.; Zheng, H. Study on frequency optimization and mechanism of ultrasonic waves assisting water flooding in low-permeability reservoirs. Ultrason. Sonochem. 2021, 70, 105291. [Google Scholar] [CrossRef]
- Saxena, N.; Saxena, A.; Mandal, A. Synthesis, characterization and enhanced oil recovery potential analysis through simulation of a natural anionic surfactant. J. Mol. Liq. 2019, 282, 545–556. [Google Scholar] [CrossRef]
- Ahmadi, M.; Hou, Q.; Wang, Y.; Chen, Z. Interfacial and molecular interactions between fractions of heavy oil and surfactants in porous media: Comprehensive review. Adv. Colloid Interface Sci. 2020, 283, 102242. [Google Scholar] [CrossRef]
- Li, S.; Peng, D.; Feng, S.; Wang, Z.; Zhang, K. Dimethyl ether-steam assisted gravity drainage: Physical 2D heavy oil simulation. Fuel 2023, 342, 127821. [Google Scholar] [CrossRef]
- Sheng, J.J. Foams and their applications in enhancing oil recovery. In Enhanced Oil Recovery Field Case Studies; Sheng, J.J., Ed.; Gulf Professional Publishing: Boston, MA, USA, 2013; pp. 251–280. [Google Scholar] [CrossRef]
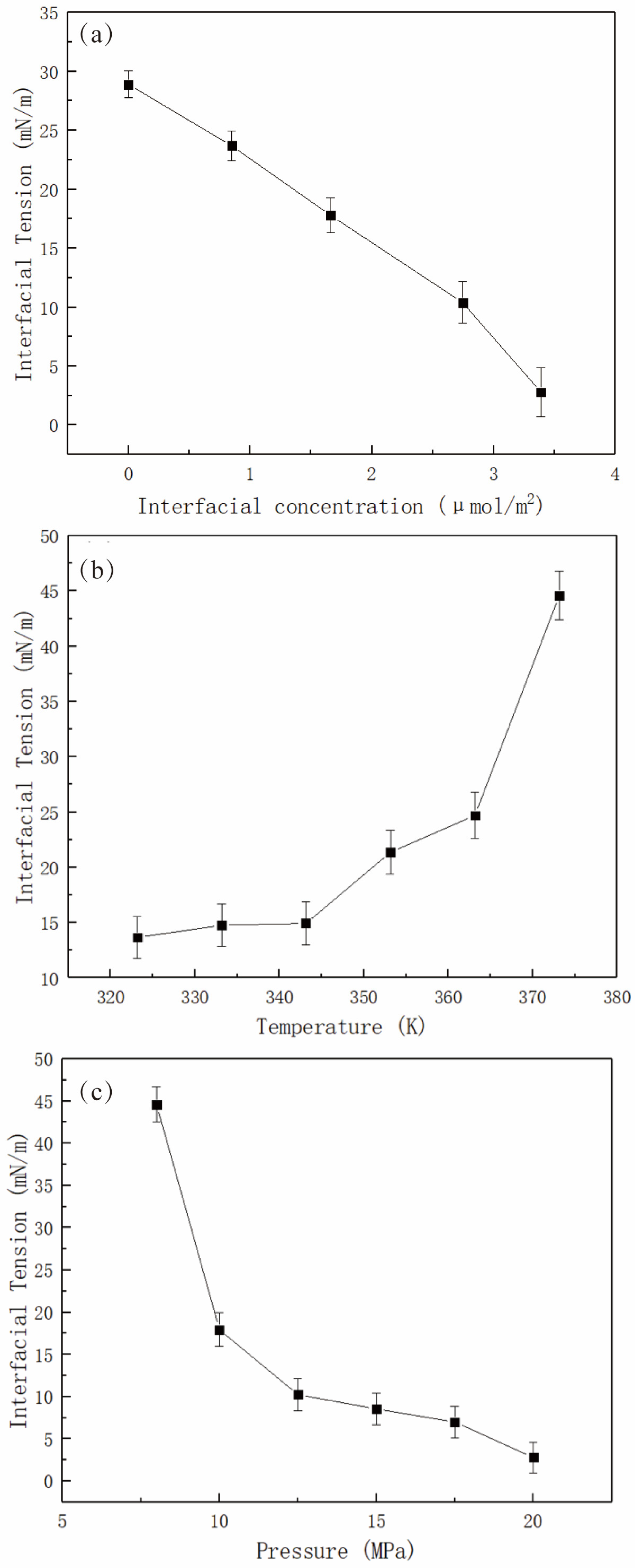
- Fan, C.; Jia, J.; Peng, B.; Liang, Y.; Li, J.; Liu, S. Molecular dynamics study on CO2 foam films with sodium dodecyl sulfate: Effects of surfactant concentration, temperature and pressure on the interfacial tension. Energy Fuels 2020, 34, 8562–8574. [Google Scholar] [CrossRef]
- Schramm, L.L. Foams: Fundamentals and Applications in the Petrleum Industry; American Chemical Society: Washington, DC, USA, 1994. [Google Scholar]
- Jia, J.; Cai, H.; Liang, Y.; Tsuji, T.; Lin, M.; Peng, B. Synergistic effect of hydrolyzed polyacrylamide and ionic surfactant to enhance the stability of CO2 foam: A molecular dynamics study. Petrol. Sci. Bull. 2023, 1, 69–86. [Google Scholar] [CrossRef]
- Jia, J.; Fan, C.; Li, J.; Peng, B.; Liang, Y.; Tsuji, T. Evaluation of the interfacial elasticity of surfactant monolayer at the CO2–water interface by molecular dynamics simulation: Screening surfactants to enhance the CO2 foam stability. Fuel 2024, 360, 130593. [Google Scholar] [CrossRef]
- Jang, S.S.; Goddard, W.A. Structures and properties of newton black films characterized using molecular dynamics simulations. J. Phys. Chem. B 2006, 110, 7992–8001. [Google Scholar] [CrossRef]
- Wang, L.; Asthagiri, D.; Zeng, Y.; Chapman, W.G. Simulation studies on the role of lauryl betaine in modulating the stability of AOS surfactant-stabilized foams used in enhanced oil recovery. Energy Fuels 2017, 31, 1512–1518. [Google Scholar] [CrossRef]
- Hirasaki, G.; Miller, C.A.; Puerto, M. Recent advances in surfactant EOR. SPE J. 2011, 16, 889–907. [Google Scholar] [CrossRef]
- Hocine, S.; Pousset, B.; Courtaud, T.; Degre, G. Long term thermal stability of chemical EOR surfactants. In Proceedings of the SPE EOR Conference at Oil and Gas West Asia, Muscat, Oman, 26–28 March 2018; OnePetro: Richardson, TX, USA, 2018; p. SPE-190361-MS. [Google Scholar] [CrossRef]
- Rosen, M.J.; Kunjappu, J.T. Surfactants and Interfacial Phenomena; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012; pp. 123–201. [Google Scholar] [CrossRef]
- Kalur, G.C.; Frounfelker, B.D.; Cipriano, B.H.; Norman, A.I.; Raghavan, S.R. Viscosity increase with temperature in cationic surfactant solutions due to the growth of wormlike micelles. Langmuir 2005, 21, 10998–11004. [Google Scholar] [CrossRef]
- Jia, J.; Li, J.; Liang, Y.; Peng, B. Molecular dynamics study on performance of olefin sulfonate at the decane—Water interface: Effect of molecular architecture. Fuel 2022, 308, 122013. [Google Scholar] [CrossRef]
- Doe, P.H.; Wade, W.H.; Schechter, R.S. Alkyl benzene sulfonates for producing low interfacial tensions between hydrocarbons and water. J. Colloid Interface Sci. 1977, 59, 525–531. [Google Scholar] [CrossRef]
- Janjua, A.N.; Sultan, A.S.; Kamal, M.S. Ultra-low interfacial tension, thermal stability and static adsorption of novel viscoelastic surfactant with heavy reservoir oil. In Proceedings of the SPE Kingdom of Saudi Arabia Annual Technical Symposium and Exhibition, Dammam, Saudi Arabia, 23 April 2018; OnePetro: Richardson, TX, USA, 2018; p. SPE-192322-MS. [Google Scholar] [CrossRef]
- Peng, M.; Nguyen, A.V. Adsorption of ionic surfactants at the air–water interface: The gap between theory and experiment. Adv. Colloid Interface Sci. 2020, 275, 102052. [Google Scholar] [CrossRef] [PubMed]
- Pal, N.; Alzahid, Y.; Alsofi, A.M.; Ali, M.; Hoteit, H. Review on microemulsions for conformance improvement technology: Fundamentals, design considerations, and perspectives. Energy Fuels 2023, 37, 858–875. [Google Scholar] [CrossRef]
- Wang, D.; Maubert, M.; Pope, G.A.; Liyanage, P.J.; Jang, S.H.; Upamali, K.A.; Chang, L.; Tagavifar, M.; Sharma, H.; Ren, G.; et al. Reduction of surfactant retention in limestones using sodium hydroxide. SPE J. 2019, 24, 92–115. [Google Scholar] [CrossRef]
- AlYousef, Z.A.; Almobarky, M.A.; Schechter, D.S. Surfactant and a mixture of surfactant and nanoparticles to stabilize CO2/brine foam, control gas mobility, and enhance oil recovery. J. Petrol. Explor. Prod. Technol. 2020, 10, 439–445. [Google Scholar] [CrossRef]
- Popova, H.; Milchev, A.; Egorov, S.A. Modeling the interfacial tension dependence on composition and stiffness of nonionic surfactants on liquid–liquid interfaces. Colloids Surf. A Physicochem. Eng. Asp. 2017, 519, 168–178. [Google Scholar] [CrossRef]
- Rai, B.; Pradip. Modeling self–assembly of surfactants at interfaces. Curr. Opin. Chem. Eng. 2017, 15, 84–94. [Google Scholar] [CrossRef]
- Wu, X.; Hou, Z.; Wu, G.; Sun, J.; Zheng, L. Aggregation behaviors of SDBSs at oil/water interface: Theoretical and experimental study. Chem. Phys. Lett. 2019, 730, 562–567. [Google Scholar] [CrossRef]
- Seyyedattar, M.; Zendehboudi, S.; Butt, S. Molecular dynamics simulations in reservoir analysis of offshore petroleum reserves: A systematic review of theory and applications. Earth Sci. Rev. 2019, 192, 194–213. [Google Scholar] [CrossRef]
- Katiyar, R.S.; Jha, P.K. Molecular simulations in drug delivery: Opportunities and challenges. WIRES Comput. Mol. Sci. 2018, 8, e1358. [Google Scholar] [CrossRef]
- Fraaije, J.G.; Tandon, K.; Jain, S.; Handgraaf, J.-W.; Buijse, M. Method of moments for computational microemulsion analysis and prediction in tertiary oil recovery. Langmuir 2013, 29, 2136–2151. [Google Scholar] [CrossRef]
- Wang, L.; Hu, Y.; Liu, R.; Liu, J.; Sun, W. Synergistic adsorption of DDA/alcohol mixtures at the air/water interface: A molecular dynamics simulation. J. Mol. Liq. 2017, 243, 1–8. [Google Scholar] [CrossRef]
- Wang, L.; Sun, N.; Wang, Z.; Han, H.; Yang, Y.; Liu, R.; Hu, Y.; Tang, H.; Sun, W. Self-assembly of mixed dodecylamine–dodecanol molecules at the air/water interface based on large-scale molecular dynamics. J. Mol. Liq. 2019, 276, 867–874. [Google Scholar] [CrossRef]
- Rucker, G.; Yu, X.; Zhang, L. Molecular dynamics investigation on n-alkane–air/water interfaces. Fuel 2020, 267, 117252. [Google Scholar] [CrossRef]
- Luft, C.M.; Munusamy, E.; Pemberton, J.E.; Schwartz, S.D. A classical molecular dynamics simulation study of interfacial and bulk solution aggregation properties of dirhamnolipids. J. Phys. Chem. B 2020, 124, 814–827. [Google Scholar] [CrossRef] [PubMed]
- Kartashynska, E.S.; Lylyk, S.V.; Aksenenko, E.V.; Makievski, A.V.; Vysotsky, Y.B.; Fainerman, V.B.; Miller, R. Surface tension at the interface between aqueous solution of surfactant and alkane: A comprehensive quantum chemical and thermodynamic approach. Colloids Surf. A Physicochem. Eng. Asp. 2020, 591, 124557. [Google Scholar] [CrossRef]
- Winsor, P.A. Binary and multicomponent solutions of amphiphilic compounds. Solubilization and the formation, structure, and theoretical significance of liquid crystalline solutions. Chem. Rev. 1968, 68, 1–40. [Google Scholar] [CrossRef]
- Creton, B.; Nieto-Draghi, C.; Pannacci, N. Prediction of surfactants’ properties using multiscale molecular modeling tools: A review. Oil Gas Sci. Technol. Rev. IFP Energ. Nouv. 2013, 67, 969–982. [Google Scholar] [CrossRef]
- Paredes, R.; Fariñas-Sánchez, A.I.; Medina-Rodríguez, B.; Samaniego, S.; Aray, Y.; Alvarez, L.J. Dynamics of surfactant clustering at interfaces and its influence on the interfacial tension: Atomistic simulation of a sodium hexadecane–benzene sulfonate–tetradecane–water system. Langmuir 2018, 34, 3146–3157. [Google Scholar] [CrossRef]
- Makimura, D.; Kunieda, M.; Liang, Y.; Matsuoka, T.; Takahashi, S.; Okabe, H. Application of molecular simulations to CO2-enhanced oil recovery: Phase equilibria and interfacial phenomena. SPE J. 2013, 18, 319–330. [Google Scholar] [CrossRef]
- Nakamura, T.; Kawamoto, S.; Shinoda, W. Precise calculation of the local pressure tensor in Cartesian and spherical coordinates in LAMMPS. Comput. Phys. Commun. 2015, 190, 120–128. [Google Scholar] [CrossRef]
- Irving, J.H.; Kirkwood, J.G. The statistical mechanical theory of transport processes. IV. The equations of hydrodynamics. J. Chem. Phys. 1950, 18, 817–829. [Google Scholar] [CrossRef]
- Muller, E.A.; Ervik, Å.; Mejía, A. A Guide to computing interfacial properties of fluids from molecular simulations [Article v1.0]. Living J. Comput. Mol. Sci. 2021, 2, 21385. [Google Scholar] [CrossRef]
- Huang, D.D.; Nikolov, A.; Wasan, D.T. Foams: Basic properties with applications to porous media. Langmuir 1986, 2, 672–677. [Google Scholar] [CrossRef]
- Jang, S.S.; Lin, S.; Maiti, P.K.; Blanco, M.; Goddard, W.A.; Shuler, P.; Tang, Y. Molecular dynamics study of a surfactant-mediated decane–water interface: Effect of molecular architecture of alkyl benzene sulfonate. J. Phys. Chem. B 2004, 108, 12130–12140. [Google Scholar] [CrossRef]
- Bera, A.; Ojha, K.; Mandal, A. Synergistic Effect of Mixed surfactant systems on Foam Behavior and Surface Tension. J. Surfact. Deterg. 2013, 16, 621–630. [Google Scholar] [CrossRef]
- Shi, W.; Guo, H. Structure, interfacial properties, and dynamics of the sodium alkyl sulfate type surfactant monolayer at the water/trichloroethylene interface: A molecular dynamics simulation study. J. Phys. Chem. B 2010, 114, 6365–6376. [Google Scholar] [CrossRef] [PubMed]
- He, X.; Guvench, O.; MacKerell, A.D.; Klein, M.L. Atomistic simulation study of linear alkylbenzene sulfonates at the water/air interface. J. Phys. Chem. B 2010, 114, 9787–9794. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Zhou, H.; Ge, J.; Xu, G. Aggregation behavior of double-chained anionic surfactant 1-Cm-C9-SO3Na at air/liquid interface: Molecular dynamics simulation. Acta Phys.-Chim. Sin. 2017, 33, 1214–1222. [Google Scholar] [CrossRef]
- Zhao, T.; Xu, G.; Yuan, S.; Chen, Y.; Yan, H. Molecular dynamics study of alkyl benzene sulfonate at air/water interface: Effect of inorganic salts. J. Phys. Chem. B 2010, 114, 5025–5033. [Google Scholar] [CrossRef]
- Alonso, G.; Gamallo, P.; Mejía, A.; Sayos, R. Assessing salt-surfactant synergistic effects on interfacial tension from molecular dynamics simulations. J. Mol. Liq. 2020, 299, 112223. [Google Scholar] [CrossRef]
- Adkins, S.S.; Chen, X.; Nguyen, Q.P.; Sanders, A.W.; Johnston, K.P. Effect of branching on the interfacial properties of nonionic hydrocarbon surfactants at the air–water and carbon dioxide–water interfaces. J. Colloid Interface Sci. 2010, 346, 455–463. [Google Scholar] [CrossRef]
- Hou, Y.; Ren, Q.; Dai, Z.; Zhou, H. Interaction mechanism of surfactant molecules with oil–water interfacial molecules. Acta Petrolei Sinica (Pet. Process.Sect.) 2018, 34, 108–114. [Google Scholar] [CrossRef]
- Hou, Y.; Ren, Q. Mesoscopic simulation of surfactant on oil–water interface. Acta Petrolei Sinica (Pet. Process.Sect.) 2019, 35, 91–98. [Google Scholar] [CrossRef]
- Shi, P.; Zhang, H.; Lin, L.; Song, C.; Chen, Q.; Li, Z. Molecular dynamics simulation of four typical surfactants at oil/water interface. J. Dispers. Sci. Technol. 2017, 39, 1258–1265. [Google Scholar] [CrossRef]
- Han, D.; Mao, J.; Zhao, J.; Zhang, H.; Wang, D.; Cao, H.; Yang, X.; Lin, C.; Zhang, Y. Dissipative particle dynamics simulation and experimental analysis of effects of Gemini surfactants with different spacer lengths on stability of emulsion systems. Colloids Surf. A Physicochem. Eng. Asp. 2022, 655, 130205. [Google Scholar] [CrossRef]
- Wang, P.; Pei, S.; Wang, M.; Yan, Y.; Sun, X.; Zhang, J. Coarse-grained molecular dynamics study on the self-assembly of Gemini surfactants: The effect of spacer length. Phys. Chem. Chem. Phys. 2017, 19, 4462. [Google Scholar] [CrossRef] [PubMed]
- Tan, J.S.J.; Zhang, L.; Lim, F.C.H.; Cheong, D.W. Interfacial properties and monolayer collapse of alkyl benzenesulfonate surfactant monolayers at the decane–water interface from molecular dynamics simulations. Langmuir 2017, 33, 4461–4476. [Google Scholar] [CrossRef] [PubMed]
- Gao, S.; Kang, Z.; Yuan, R.; Liu, N.; Zhu, P.; Wang, B. Molecular dynamics study of nonylphenol–substituted dodecyl sulfonate at air/water interface: Role of steric effect of surfactant headgroups. J. Mol. Struct. 2019, 1192, 35–41. [Google Scholar] [CrossRef]
- Gao, S.; Bao, X.; Yu, L.; Wang, H.; Li, J.; Chen, X. Molecular dynamics study of “quasi-gemini” surfactant at n-decane/water interface: The synergistic effect of hydrophilic headgroups and hydrophobic tails of surfactants on the interfacial properties. Colloids Surf. A Physicochem. Eng. Asp. 2022, 634, 127899. [Google Scholar] [CrossRef]
- Wade, W.H.; Morgan, J.C.; Jacobson, J.K.; Schechter, R.S. Low interfacial tensions involving mixtures of surfactants. SPE J. 1977, 17, 122–128. [Google Scholar] [CrossRef]
- Wang, C.; Cao, X.; Guo, L.; Xu, Z.; Zhang, L.; Gong, Q.; Zhang, L.; Zhao, S. Effect of molecular structure of catanionic surfactant mixtures on their interfacial properties. Colloids Surf. A Physicochem. Eng. Asp. 2016, 509, 601–612. [Google Scholar] [CrossRef]
- Wang, L.; Liu, R.; Hu, Y.; Sun, W. Adsorption of mixed DDA/NaOL surfactants at the air/water interface by molecular dynamics simulations. Chem. Eng. Sci. 2016, 155, 167–174. [Google Scholar] [CrossRef]
- Ergin, G.; Lbadaoui-Darvas, M.; Takahama, S. Molecular structure inhibiting synergism in charged surfactant mixtures: An atomistic molecular dynamics simulation study. Langmuir 2017, 33, 14093–14104. [Google Scholar] [CrossRef] [PubMed]
- Agneta, M.; Li, Z.; Zhang, C.; Gerald, G. Investigating synergism and antagonism of binary mixed surfactants for foam efficiency optimization in high salinity. J. Pet. Sci. Eng. 2019, 175, 489–494. [Google Scholar] [CrossRef]
- Li, J.; Han, Y.; Qu, G.; Cheng, J.; Xue, C.; Gao, X.; Sun, T.; Ding, W. Molecular dynamics simulation of the aggregation behavior of N-Dodecyl-N,N-Dimethyl-3-Ammonio-1-Propanesulfonate/sodium dodecyl benzene sulfonate surfactant mixed system at oil/water interface. Colloids Surf. A Physicochem. Eng. Asp. 2017, 531, 73–80. [Google Scholar] [CrossRef]
- Li, J.; Han, Y.; Qu, G.; Cheng, J.; Xue, C.; Ding, W. Effect of Ca2+ on aggregation behavior of mixed N-dodecyl-N,N-dimethy-3-ammonio-1-propanesulfonate (SB12-3)/sodium dodecyl benzene sulfonate at oil/water interface: A molecular dynamics study. Fine Chem. 2018, 35, 956–963. [Google Scholar] [CrossRef]
- Gao, F.; Liu, G.; Yuan, S. The effect of betaine on the foam stability: Molecular simulation. Appl. Surf. Sci. 2017, 407, 156–161. [Google Scholar] [CrossRef]
- Xu, J.; Zhang, Y.; Chen, H.; Wang, P.; Xie, Z.; Yao, Y.; Yan, Y.; Zhang, J. Effect of surfactant headgroups on the oil/water interface: An interfacial tension measurement and simulation study. J. Mol. Struct. 2013, 1052, 50–56. [Google Scholar] [CrossRef]
- Baoukina, S.; Mendez-Villuendas, E.; Tieleman, D.P. Molecular view of phase coexistence in lipid monolayers. J. Am. Chem. Soc. 2012, 134, 17543–17553. [Google Scholar] [CrossRef] [PubMed]
- Da Rocha, S.R.P.; Johnston, K.P.; Westacott, R.E.; Rossky, R.J. Molecular structure of the water–supercritical CO2 interface. J. Phys. Chem. B 2001, 105, 12092–12104. [Google Scholar] [CrossRef]
- Wei, Y.; Wang, H.; Xia, Q.; Yuan, S. A molecular dynamics study on the dependence of phase behaviors and structural properties of two-dimensional interfacial monolayer on surface area. Appl. Surf. Sci. 2018, 459, 741–748. [Google Scholar] [CrossRef]
- Mohammad-Aghaie, D.; Macé, E.; Sennoga, C.A.; Seddon, J.M.; Bresme, F. Molecular dynamics simulations of liquid condensed to liquid expanded transitions in DPPC monolayers. J. Phys. Chem. B 2010, 114, 1325–1335. [Google Scholar] [CrossRef]
- Munusamy, E.; Luft, C.M.; Pemberton, J.E.; Schwartz, S.D. Unraveling the differential aggregation of anionic and nonionic monorhamnolipids at air–water and oil–water interfaces: A classical molecular dynamics simulation study. J. Phys. Chem. B 2018, 122, 6403–6416. [Google Scholar] [CrossRef]
- Liu, Z.; Xu, Z.; Zhou, H.; Wang, Y.; Liao, Q.; Zhang, L.; Zhao, S. Interfacial behaviors of betaine and binary betaine/carboxylic acid mixtures in molecular dynamics simulation. J. Mol. Liq. 2017, 240, 412–419. [Google Scholar] [CrossRef]
- Cai, H.; Zhang, Y.; Liu, Z.; Li, J.; Gong, Q.; Liao, Q.; Zhang, L.; Zhao, S. Molecular dynamics simulation of binary betaine and anionic surfactant mixtures at decane–water interface. J. Mol. Liq. 2018, 266, 82–89. [Google Scholar] [CrossRef]
- Habartová, A.; Roeselová, M.; Cwiklik, L. Investigation of mixed surfactant films at water surface using molecular dynamics simulations. Langmuir 2015, 31, 11508–11515. [Google Scholar] [CrossRef]
- Jia, H.; Lian, P.; Yan, H.; Han, Y.; Wang, Q.; Dai, J.; Wang, S.; Tian, Z.; Wang, D. Insights into the assembly of the pseudogemini surfactant at the oil/water interface: A molecular simulation study. Langmuir 2020, 36, 1839–1847. [Google Scholar] [CrossRef]
- Yan, H.; Guo, X.; Yuan, S.; Liu, C. Molecular dynamics study of the effect of calcium ions on the monolayer of SDC and SDSn surfactants at the vapor/liquid interface. Langmuir 2011, 27, 5762–5771. [Google Scholar] [CrossRef]
- Zhang, L.; Wu, P.; Wei, Q.; Shen, J.; Liu, Z.; Ren, T.; Zhang, W.; Wang, X. The effect of spacer on the structure of surfactant at liquid/air interface: A molecular dynamics simulation study. J. Mol. Liq. 2016, 222, 988–994. [Google Scholar] [CrossRef]
- Goodarzi, F.; Zendehboudi, S. Effects of salt and surfactant on interfacial characteristics of water/oil systems: Molecular dynamic simulations and dissipative particle dynamics. Ind. Eng. Chem. Res. 2019, 58, 8817–8834. [Google Scholar] [CrossRef]
- Dong, Z.; Liu, S.; Nie, X.; Zhang, Y.; Dong, K.; Wang, J. Experimental and molecular simulation research on the effect of metal ions on the stability of SDS foam. Energy Fuels 2022, 36, 521–526. [Google Scholar] [CrossRef]
- Liu, B.; Hoopes, M.I.; Karttunen, M. Molecular dynamics simulations of DPPC/CTAB monolayers at the air/water interface. J. Phys. Chem. B 2014, 118, 11723–11737. [Google Scholar] [CrossRef]
- Allen, D.T.; Saaka, Y.; Pardo, L.C.; Lawrence, M.J.; Lorenz, C.D. Specific effects of monovalent counterions on the structural and interfacial properties of dodecyl sulfate monolayers. Phys. Chem. Chem. Phys. 2016, 18, 30394–30406. [Google Scholar] [CrossRef]
- Hu, S.; Ji, X.; Fan, Z.; Zhang, T.; Sun, S. Counterion effects on the properties of sulfate surfactants at the air/liquid interface by molecular dynamics simulation. Acta Phys.-Chim. Sin. 2015, 31, 83–89. [Google Scholar] [CrossRef]
- Yan, H.; Yuan, S.; Xu, G.; Liu, C. Effect of Ca2+ and Mg2+ ions on surfactant solutions investigated by molecular dynamics simulation. Langmuir 2010, 26, 10448–10459. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Y.; Li, C.; Zhan, D.; Cao, X.; Song, X.; Wang, Q.; Li, Y. Molecular array behavior and synergistic effect of sodium alcohol ether sulphate and carboxyl betaine/sulfobetaine in foam film under high salt conditions. Colloids Surf. A Physicochem. Eng. Asp. 2015, 480, 138–148. [Google Scholar] [CrossRef]
- Ghosh, T.; García, A.E.; Garde, S. Molecular dynamics simulations of pressure effects on hydrophobic interactions. J. Am. Chem. Soc. 2001, 123, 10997–11003. [Google Scholar] [CrossRef]
- Ghosh, T.; García, A.E.; Garde, S. Water-mediated three-particle interactions between hydrophobic solutes: size, pressure, and salt effects. J. Phys. Chem. B 2003, 107, 612–617. [Google Scholar] [CrossRef]
- Li, C.; Zhang, T.; Ji, X.; Wang, Z.; Sun, S.; Hu, S. Effect of Ca2+/Mg2+ on the stability of the foam system stabilized by an anionic surfactant: A molecular dynamics study. Colloids Surf. A Physicochem. Eng. Asp. 2016, 489, 423–432. [Google Scholar] [CrossRef]
- Liu, Z.; Liao, Q.; Jin, Z.; Zhang, L.; Zhang, L. Effect of electrolytes on the interfacial behavior of nonionic-anionic surfactant solutions using molecular dynamics simulations. Acta Phys.-Chim. Sin. 2016, 32, 1168–1174. [Google Scholar] [CrossRef]
- Riccardi, E.; Tichelkamp, T. Calcium ion effects on the water/oil interface in the presence of anionic surfactants. Colloids Surf. A Physicochem. Eng. Asp. 2019, 573, 246–254. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, L.; Cao, X.; Song, X.; Jin, Z.; Zhang, L.; Zhao, S. Effect of electrolytes on interfacial tensions of alkyl ether carboxylate solutions. Energy Fuels 2013, 27, 3122–3129. [Google Scholar] [CrossRef]
- Liu, Z.; Li, Z.; Song, X.; Zhang, J.; Zhang, L.; Zhang, L.; Zhao, S. Dynamic interfacial tensions of binary nonionic–anionic and nonionic surfactant mixtures at water–alkane interfaces. Fuel 2014, 135, 91–98. [Google Scholar] [CrossRef]
- Chanda, J.; Bandyopadhyay, S. Molecular dynamics study of surfactant monolayers adsorbed at the oil/water and air/water interfaces. J. Phys. Chem. B 2006, 110, 23482–23488. [Google Scholar] [CrossRef]
- Goodarzi, F.; Kondori, J.; Rezaei, N.; Zendehboudi, S. Meso- and molecular-scale modeling to provide new insights into interfacial and structural properties of hydrocarbon/water/surfactant systems. J. Mol. Liq. 2019, 295, 111357. [Google Scholar] [CrossRef]
- Jiang, R.; Luo, J.; Bai, R.; Jiang, B.; Zhou, G. Molecular dynamics simulation on behavior of common surfactants at the oil/water interface in complex systems. Chem. J. Chin. Univ. 2017, 38, 1804–1812. [Google Scholar] [CrossRef]
- Parra, J.G.; Domínguez, H.; Aray, Y.; Iza, P.; Zarate, X.; Schott, E. Structural and interfacial properties of the CO2–in–water foams prepared with sodium dodecyl sulfate (SDS): A molecular dynamics simulation study. Colloids Surf. A Physicochem. Eng. Asp. 2019, 578, 123615. [Google Scholar] [CrossRef]
- Sun, Y.; Qi, X.; Sun, H.; Zhao, H.; Li, Y. Understanding about how different foaming gases effect the interfacial array behaviors of surfactants and the foam properties. Langmuir 2016, 32, 7503–7511. [Google Scholar] [CrossRef]
- Creton, B.; Mougin, P. Equivalent alkane carbon number of live crude oil: A predictive model based on thermodynamics. Oil Gas Sci. Technol. Rev. IFP Energ. Nouv. 2016, 71, 62–71. [Google Scholar] [CrossRef]
- Lukowicz, T.; Illous, E.; Nardello-Rataj, V.; Aubry, J. Prediction of the equivalent alkane carbon number (EACN) of aprotic polar oils with COSMO-RS sigma–moments. Colloids Surf. A Physicochem. Eng. Asp. 2018, 536, 53–59. [Google Scholar] [CrossRef]
- Chang, L.; Pope, G.A.; Jang, S.H.; Tagavifar, M. Prediction of microemulsion phase behavior from surfactant and co-solvent structures. Fuel 2019, 237, 494–514. [Google Scholar] [CrossRef]
- Xiao, H.; Zhen, Z.; Sun, H.; Cao, X.; Li, Z.; Song, X.; Cui, X.; Liu, X. Molecular dynamics simulation of anionic surfactant at the water/n-alkane interface. Acta Phys.-Chim. Sin. 2010, 26, 422–428. [Google Scholar] [CrossRef]
- Barnes, J.R.; Smit, J.P.; Smit, J.R.; Shpakoff, G.; Raney, K.H.; Puerto, M.C. Development of surfactants for chemical flooding at difficult reservoir conditions. In Proceedings of the SPE Symposium on Improved Oil Recovery, Tulsa, OK, USA, 20–23 April 2008; OnePetro: Richardson, TX, USA, 2008; p. SPE-113313-MS. [Google Scholar] [CrossRef]
- Kunieda, M.; Nakaoka, K.; Liang, Y.; Miranda, C.R.; Ueda, A.; Takahashi, S.; Okabe, H.; Matsuoka, T. Self-accumulation of aromatics at the oil–water interface through weak hydrogen bonding. J. Am. Chem. Soc. 2010, 132, 18281–18286. [Google Scholar] [CrossRef] [PubMed]
- Fujita, K.; Liang, Y.; Mizuhara, J.; Masuda, Y.; Kobayashi, K.; Takabayashi, K.; Iwama, H.; Yonebayashi, H. Evaluation of asphaltene adsorption free energy at the oil–water interface: Effect of oil solvents. Energy Fuels 2022, 36, 1338–1349. [Google Scholar] [CrossRef]
- Sugiyama, S.; Liang, Y.; Murata, S.; Matsuoka, T.; Morimoto, M.; Ohata, T.; Nakano, M.; Boek, E.S. Construction, validation, and application of digital oil: Investigation of asphaltene association toward asphaltene-precipitation prediction. SPE J. 2018, 23, 952–968. [Google Scholar] [CrossRef]
- Iwase, M.; Sugiyama, S.; Liang, Y.; Masuda, Y.; Morimoto, M.; Matsuoka, T.; Boek, E.S.; Ueda, R.; Nakagawa, K. Development of digital oil for heavy crude oil: Molecular model and molecular dynamics simulations. Energy Fuels 2018, 32, 2781–2792. [Google Scholar] [CrossRef]
- Iwase, M.; Liang, Y.; Masuda, Y.; Morimoto, M.; Matsuoka, T.; Boek, E.S.; Kaito, Y.; Nakagawa, K. Application of a digital oil model to solvent-based enhanced oil recovery of heavy crude oil. Energy Fuels 2019, 33, 10868–10877. [Google Scholar] [CrossRef]
- Cui, W.; Huo, K.; Sugiyama, S.; Liang, Y.; Masuda, Y.; Morimoto, M.; Matsuoka, T.; Boek, E.S.; Kaito, Y.; Nakagawa, K.; et al. Microstructural evolution of bitumen during the glass transition: An application of digital oil. Fuel 2023, 335, 127048. [Google Scholar] [CrossRef]
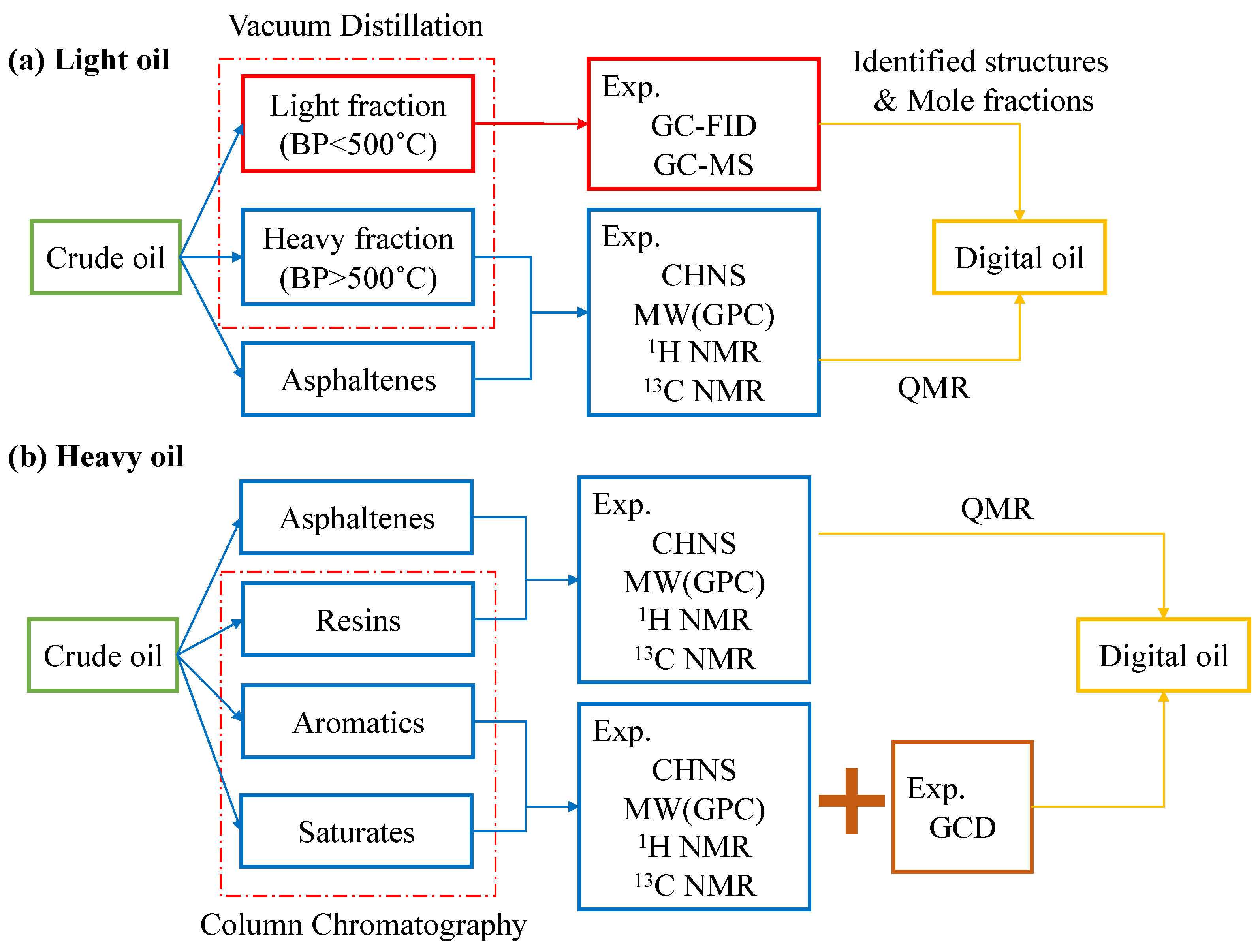
- Liang, Y. Development of digital oil for technological innovation in petroleum engineering. J. Jpn. Assoc. Petrol. Technol. 2019, 84, 425–436. [Google Scholar] [CrossRef]
- Mizuhara, J.; Liang, Y.; Masuda, Y.; Kobayashi, K.; Iwama, H.; Yonebayashi, H. Evaluation of asphaltene adsorption free energy at the oil–water interface: Role of heteroatoms. Energy Fuels 2020, 34, 5267–5280. [Google Scholar] [CrossRef]
- Gao, F.; Xu, Z.; Liu, G.; Yuan, S. Molecular dynamics simulation: The behavior of asphaltene in crude oil and at the oil/water interface. Energy Fuels 2014, 28, 7368–7376. [Google Scholar] [CrossRef]
- Lv, G.; Gao, F.; Liu, G.; Yuan, S. The properties of asphaltene at the oil–water interface: A molecular dynamics simulation. Colloids Surf. A Physicochem. Eng. Asp. 2017, 515, 34–40. [Google Scholar] [CrossRef]
- Truszkowski, A.; Epple, M.; Fiethen, A.; Zielesny, A.; Kuhn, H. Molecular fragment dynamics study on the water–air interface behavior of non-ionic polyoxyethylene alkyl ether surfactants. J. Colloid Interface Sci. 2013, 410, 140–145. [Google Scholar] [CrossRef]
- Khedr, A.; Striolo, A. DPD parameters estimation for simultaneously simulating water–oil interfaces and aqueous nonionic surfactants. J. Chem. Theory Comput. 2018, 14, 6460–6471. [Google Scholar] [CrossRef] [PubMed]
- Cao, J.; Liang, Y.; Masuda, Y.; Koga, H.; Tanaka, H.; Tamura, K.; Takagi, S.; Matsuoka, T. Molecular simulation of CH4 adsorption behavior in slit nanopores: Verification of simulation methods and models. AIChE J. 2019, 65, e16733. [Google Scholar] [CrossRef]
- Wennberg, C.L.; Murtola, T.; Hess, B.; Lindahl, E. Lennard-Jones lattice summation in bilayer simulations has critical effects on surface tension and lipid properties. J. Chem. Theory Comput. 2013, 9, 3527–3537. [Google Scholar] [CrossRef] [PubMed]
- Vanommeslaeghe, K.; Hatcher, E.; Acharya, C.; Kundu, S.; Zhong, S.; Shim, J.; Darian, E.; Guvench, O.; Lopes, P.; Vorobyov, I.; et al. CHARMM general force field: A force field for drug-like molecules compatible with the CHARMM all-atom additive biological force fields. J. Comput. Chem. 2010, 31, 671–690. [Google Scholar] [CrossRef]
- Jorgensen, W.L.; Maxwell, D.S.; Tirado-Rives, J. Development and testing of the OPLS all-atom force field on conformational energetics and properties of organic liquids. J. Am. Chem. Soc. 1996, 118, 11225–11236. [Google Scholar] [CrossRef]
- Scott, W.R.P.; Hünenberger, P.H.; Tironi, I.G.; Mark, A.E.; Billeter, S.R.; Fennen, J.; Torda, A.E.; Huber, T.; Kruger, P.; van Gunsteren, W.F. The GROMOS biomolecular simulation program package. J. Phys. Chem. A 1999, 103, 3596–3607. [Google Scholar] [CrossRef]
- Ríos-López, M.; Mendez-Bermúdez, J.G.; Domínguez, H. New force field parameters for the sodium dodecyl sulfate and alpha olefin sulfonate anionic surfactants. J. Phys. Chem. B 2018, 122, 4558–4565. [Google Scholar] [CrossRef]
- Illa-Tuset, S.; Malaspina, D.C.; Faraudo, J. Coarse-grained molecular dynamics simulation of the interface behaviour and self-assembly of CTAB cationic surfactants. Phys. Chem. Chem. Phys. 2018, 20, 26422–26430. [Google Scholar] [CrossRef] [PubMed]
- Servis, M.J.; McCue, A.; Casella, A.J.; Clark, A.E. The role of surfactant force field on the properties of liquid/liquid interfaces. Fluid Phase Equilibria 2020, 511, 112497. [Google Scholar] [CrossRef]
- Kirch, A.; Celaschi, Y.M.; Almeida, J.M.; Miranda, C.R. Brine–oil interfacial tension modeling: Assessment of machine learning techniques combined with molecular dynamics. ACS Appl. Mater. Interfaces 2020, 12, 15837–15843. [Google Scholar] [CrossRef] [PubMed]
- Blunt, M.J.; Bazylak, A.; Brook, M.; Muggeridge, A.; Orr, F.M. Research Needs in Porous Media for the Energy Transition. InterPore J. 2024, 1, ipj260424-2. Available online: https://ipjournal.interpore.org/index.php/interpore/article/view/14 (accessed on 7 June 2024). [CrossRef]





| Surfactants | Average Number of Hydrogen Bonds | Diffusion Coefficients ×10−6 (cm2/s) |
|---|---|---|
| PFOS | 1.070 | 2.588 |
| PFOS-CH2 | 1.081 | 2.348 |
| PFOS-S | 1.107 | 2.720 |
| PFOS-CO | 1.247 | 1.943 |
| PFOS-NH | 1.294 | 1.942 |
| PFOS-CONH | 1.290 | 1.678 |
| Surfactant | Dipolar Pair | CM (kJ/mol) | BARR (kJ/mol) | SSM (kJ/mol) | ΔE+ (kJ/mol) | ΔE− (kJ/mol) | K=ΔE+/ΔE− |
|---|---|---|---|---|---|---|---|
| SDS | −5.72 | 2.70 | −2.52 | 5.22 | 8.42 | 0.620 | |
| −7.68 | 13.38 | −3.75 | 17.13 | 21.06 | 0.813 | ||
| −8.31 | 28.29 | −4.70 | 32.99 | 36.60 | 0.901 | ||
| SDSn | −5.16 | 2.56 | −2.28 | 4.84 | 7.72 | 0.627 | |
| −5.87 | 18.84 | −2.76 | 21.60 | 24.71 | 0.874 | ||
| −8.65 | 29.00 | −5.13 | 34.13 | 37.65 | 0.907 | ||
| SDC | - | - | - | 6.53 | 9.60 | 0.680 | |
| - | - | - | 8.66 | 17.60 | 0.492 | ||
| SDSn | - | - | - | 7.78 | 9.82 | 0.792 | |
| - | - | - | 19.12 | 23.52 | 0.813 | ||
| AES + CAB | −10.32 | 5.80 | −3.00 | 8.80 | 16.12 | 0.546 | |
| −8.39 | 8.43 | −3.74 | 12.17 | 16.82 | 0.724 | ||
| AES + DSB | −10.59 | 5.23 | −5.45 | 10.68 | 15.82 | 0.675 | |
| −5.74 | 6.94 | −5.58 | 12.52 | 12.68 | 0.987 | ||
| AES | −31.41 | 53.31 | −19.93 | 73.24 | 84.72 | 0.860 | |
| −29.74 | 85.08 | −19.41 | 104.49 | 114.82 | 0.910 |
| Systems | Rnonane (nm) | Rdecane (nm) | Rhendecane (nm) | Rshort (nm) | Rlong (nm) | Reffective (nm) | IFT (mN/m) |
|---|---|---|---|---|---|---|---|
| nonane + pure water | 0.859 ± 0.014 | 0.370 ± 0.018 | 1.2241 ± 0.060 | 0.871 ± 0.078 | 33.14 ± 0.62 | ||
| decane + pure water | 0.997 ± 0.103 | 0.384 ± 0.019 | 1.337 ± 0.135 | 0.953 ± 0.136 | 8.02 ± 4.12 | ||
| hendecane + pure water | 1.041 ± 0.023 | 0.370 ± 0.017 | 1.231 ± 0.070 | 0.861 ± 0.087 | 31.10 ± 3.31 | ||
| nonane + brine (NaCl) | 0.859 ± 0.016 | 0.372 ± 0.013 | 1.224 ± 0.086 | 0.852 ± 0.099 | 31.41 ± 0.05 | ||
| decane + brine (NaCl) | 0.952 ± 0.018 | 0.369 ± 0.019 | 1.240 ± 0.067 | 0.871 ± 0.086 | 31.04 ± 0.32 | ||
| hendecane + brine (NaCl) | 1.040 ± 0.022 | 0.371 ± 0.014 | 1.229 ± 0.084 | 0.858 ± 0.098 | 31.08 ± 3.41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, J.; Yang, S.; Li, J.; Liang, Y.; Li, R.; Tsuji, T.; Niu, B.; Peng, B. Review of the Interfacial Structure and Properties of Surfactants in Petroleum Production and Geological Storage Systems from a Molecular Scale Perspective. Molecules 2024, 29, 3230. https://doi.org/10.3390/molecules29133230
Jia J, Yang S, Li J, Liang Y, Li R, Tsuji T, Niu B, Peng B. Review of the Interfacial Structure and Properties of Surfactants in Petroleum Production and Geological Storage Systems from a Molecular Scale Perspective. Molecules. 2024; 29(13):3230. https://doi.org/10.3390/molecules29133230
Chicago/Turabian StyleJia, Jihui, Shu Yang, Jingwei Li, Yunfeng Liang, Rongjuan Li, Takeshi Tsuji, Ben Niu, and Bo Peng. 2024. "Review of the Interfacial Structure and Properties of Surfactants in Petroleum Production and Geological Storage Systems from a Molecular Scale Perspective" Molecules 29, no. 13: 3230. https://doi.org/10.3390/molecules29133230
APA StyleJia, J., Yang, S., Li, J., Liang, Y., Li, R., Tsuji, T., Niu, B., & Peng, B. (2024). Review of the Interfacial Structure and Properties of Surfactants in Petroleum Production and Geological Storage Systems from a Molecular Scale Perspective. Molecules, 29(13), 3230. https://doi.org/10.3390/molecules29133230








