Exploring High-Spin Color Centers in Wide Band Gap Semiconductors SiC: A Comprehensive Magnetic Resonance Investigation (EPR and ENDOR Analysis)
Abstract
1. Introduction
2. Results and Discussion
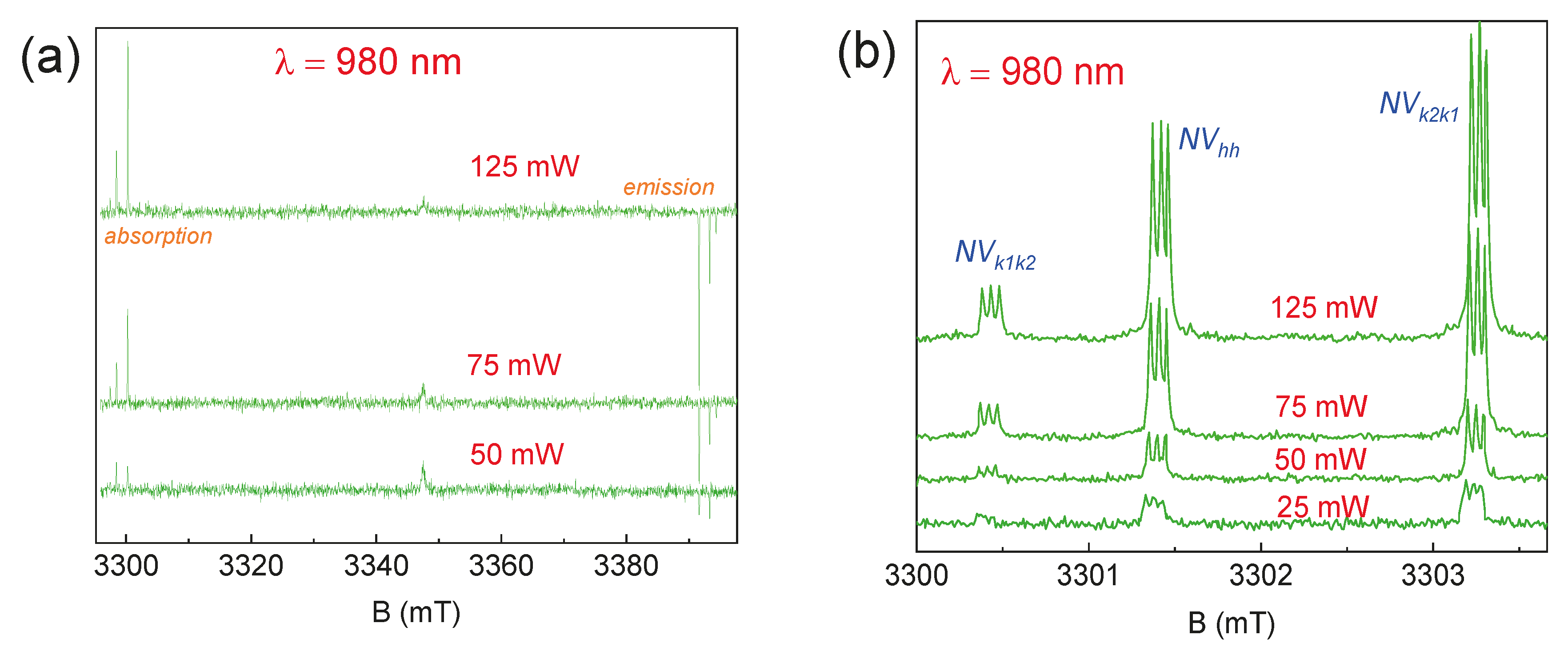
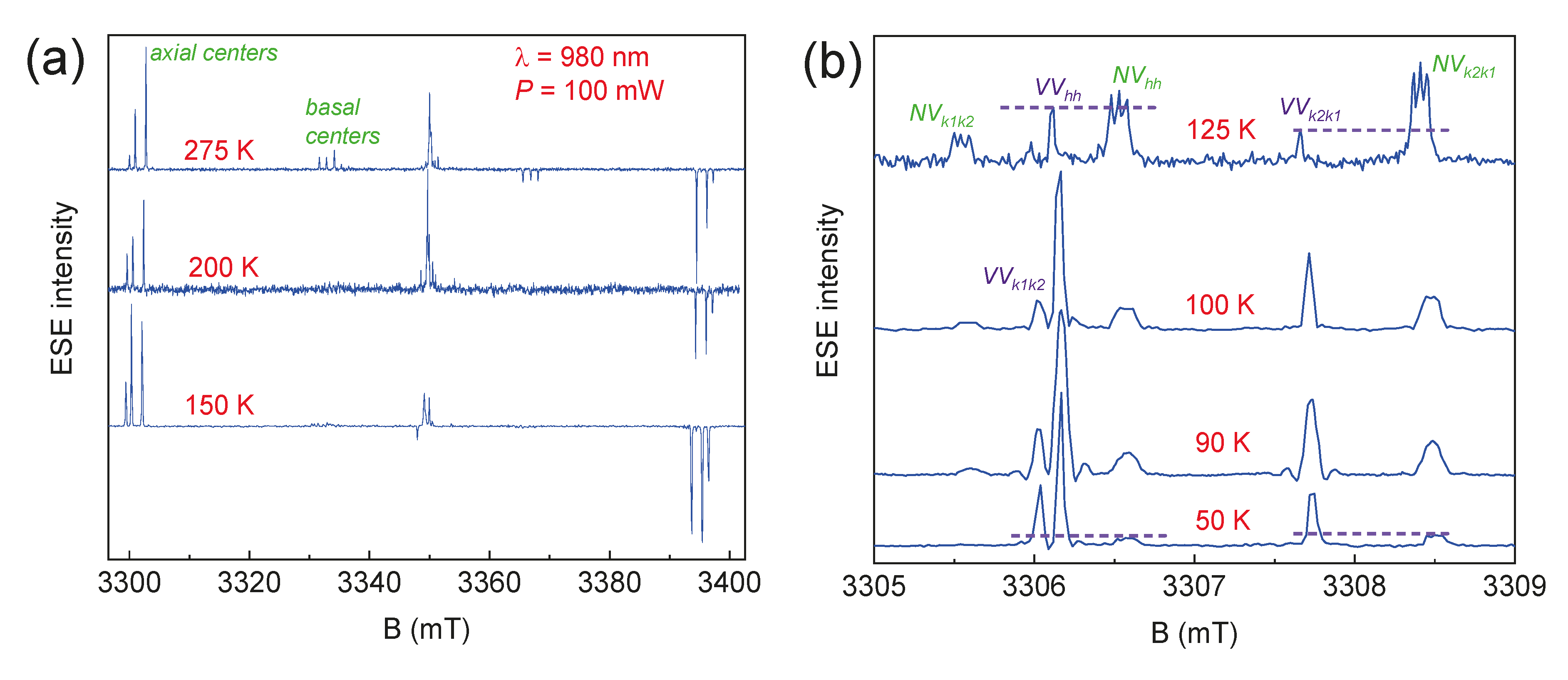
2.1. Spin-Optical Properties
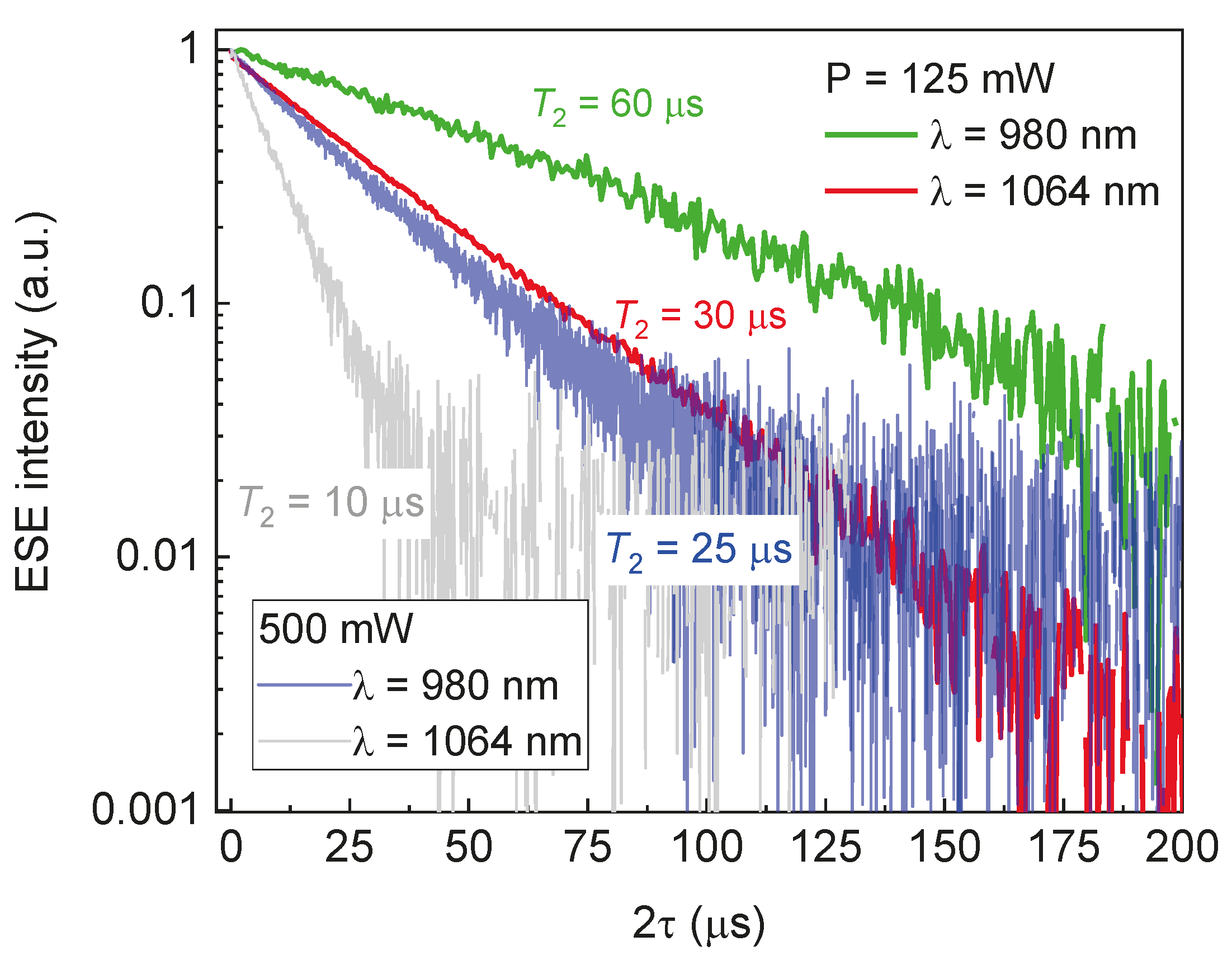
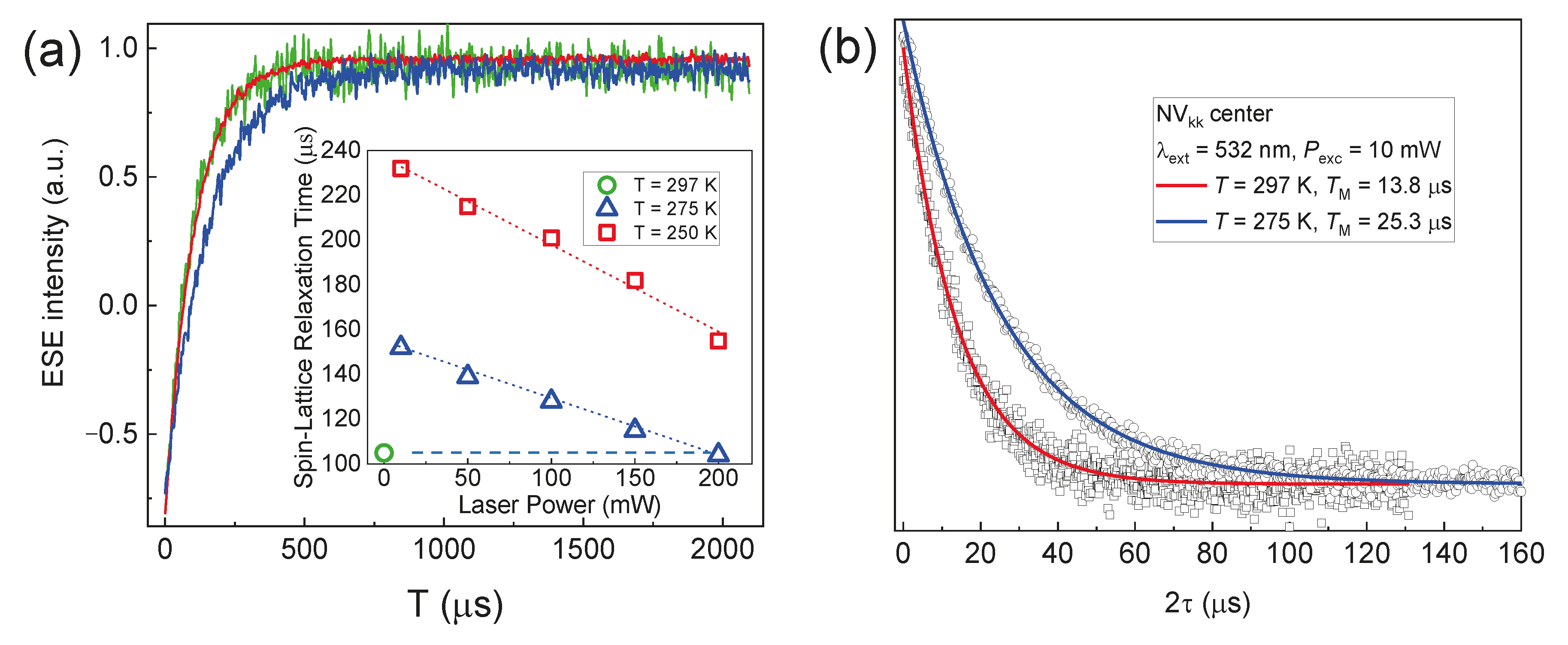
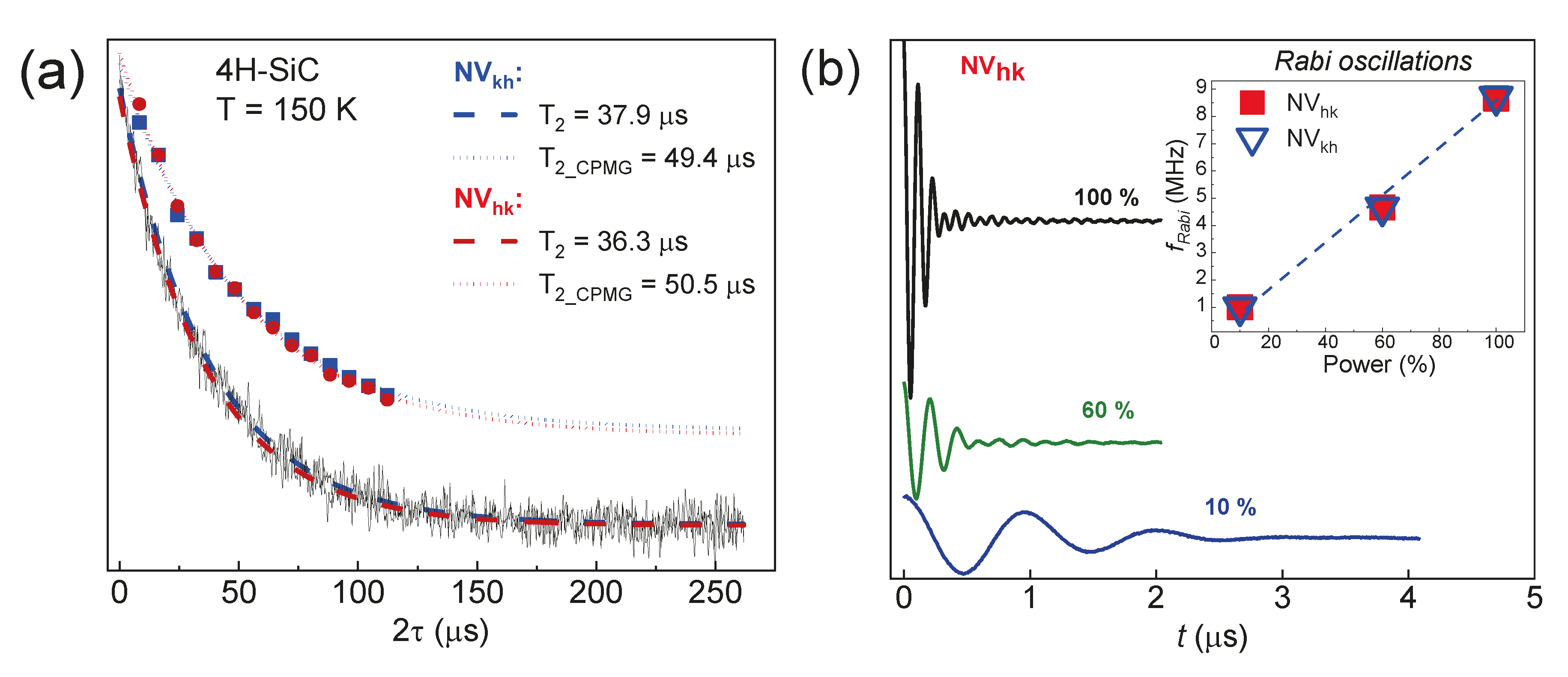
2.2. Relaxation Characteristics
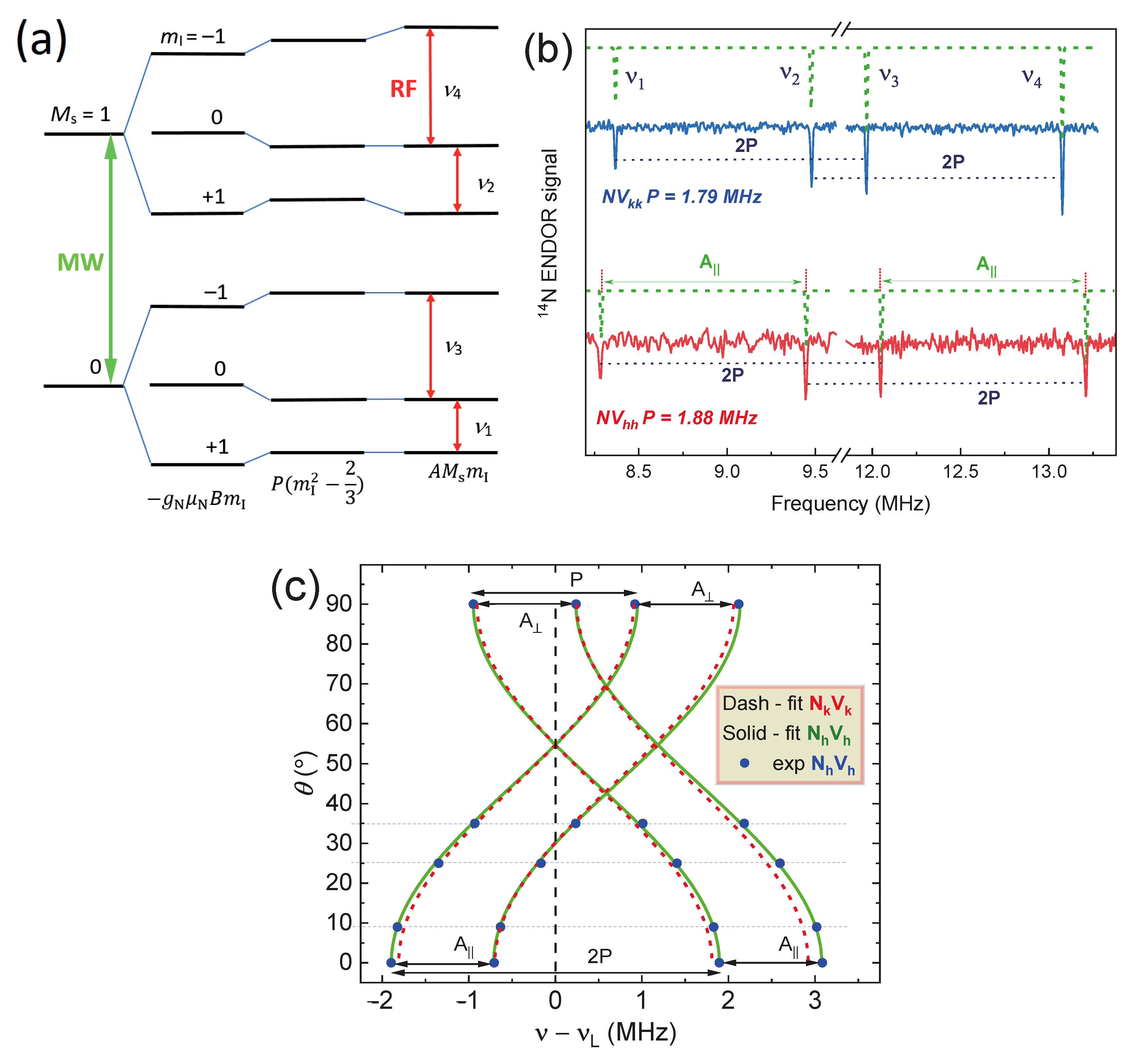
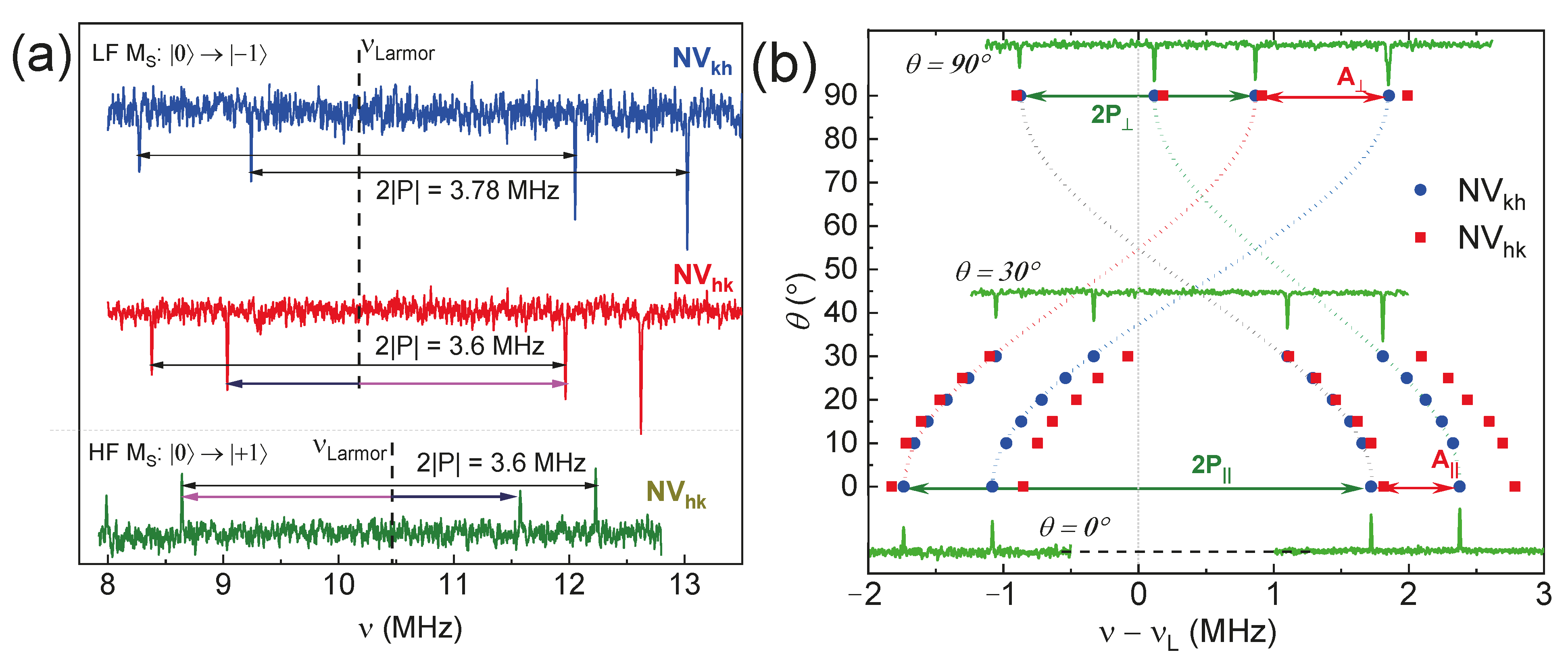
2.3. Electron–Nuclear Interactions (Axial and Basal Centers)
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ladd, T.D.; Jelezko, F.; Laflamme, R.; Nakamura, Y.; Monroe, C.; O’Brien, J.L. Quantum Computers. Nature 2010, 464, 45–53. [Google Scholar] [CrossRef] [PubMed]
- Tacchino, F.; Chiesa, A.; Carretta, S.; Gerace, D. Quantum Computers as Universal Quantum Simulators: State-of-the-Art and Perspectives. Adv. Quantum Technol. 2020, 3, 1900052. [Google Scholar] [CrossRef]
- Kumar, S. Fundamental Limits to Moore’s Law. arXiv 2015, arXiv:1511.05956. [Google Scholar]
- Powell, J.R. The Quantum Limit to Moore’s Law. Proc. IEEE 2008, 96, 1247–1248. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Computing 40 Years Later. In Feynman Lectures on Computation; CRC Press: Boca Raton, FL, USA, 2023. [Google Scholar]
- de Leon, N.P.; Itoh, K.M.; Kim, D.; Mehta, K.K.; Northup, T.E.; Paik, H.; Palmer, B.S.; Samarth, N.; Sangtawesin, S.; Steuerman, D.W. Materials Challenges and Opportunities for Quantum Computing Hardware. Science 2021, 372, eabb2823. [Google Scholar] [CrossRef] [PubMed]
- Chen, G.; Church, D.A.; Englert, B.-G.; Henkel, C.; Rohwedder, B.; Scully, M.O.; Zubairy, M.S. Quantum Computing Devices: Principles, Designs, and Analysis; Chapman and Hall/CRC: New York, NY, USA, 2006. [Google Scholar] [CrossRef]
- Perdomo-Ortiz, A.; Benedetti, M.; Realpe-Gómez, J.; Biswas, R. Opportunities and Challenges for Quantum-Assisted Machine Learning in near-Term Quantum Computers. Quantum Sci. Technol. 2018, 3, 030502. [Google Scholar] [CrossRef]
- Takui, T.; Berliner, L.; Hanson, G. Electron Spin Resonance (ESR) Based Quantum Computing; Springer: New York, NY, USA, 2016; p. 408. [Google Scholar]
- Roffe, J. Quantum Error Correction: An Introductory Guide. Contemp. Phys. 2019, 60, 226–245. [Google Scholar] [CrossRef]
- Terhal, B.M. Quantum Error Correction for Quantum Memories. Rev. Mod. Phys. 2015, 87, 307–346. [Google Scholar] [CrossRef]
- Häner, T.; Steiger, D.S.; Smelyanskiy, M.; Troyer, M. High Performance Emulation of Quantum Circuits. In Proceedings of the SC ’16: Proceedings of the International Conference for High Performance Computing, Networking, Storage and Analysis, Salt Lake City, UT, USA, 13–18 November 2016; pp. 866–874. [Google Scholar] [CrossRef]
- Wolfowicz, G.; Heremans, F.J.; Anderson, C.P.; Kanai, S.; Seo, H.; Gali, A.; Galli, G.; Awschalom, D.D. Quantum Guidelines for Solid-State Spin Defects. Nat. Rev. Mater. 2021, 6, 906–925. [Google Scholar] [CrossRef]
- Doherty, M.W.; Manson, N.B.; Delaney, P.; Jelezko, F.; Wrachtrup, J.; Hollenberg, L.C.L. The Nitrogen-Vacancy Colour Centre in Diamond. Phys. Rep. 2013, 528, 1–45. [Google Scholar] [CrossRef]
- Astakhov, G.V.; Simin, D.; Dyakonov, V.; Yavkin, B.V.; Orlinskii, S.B.; Proskuryakov, I.I.; Anisimov, A.N.; Soltamov, V.A.; Baranov, P.G. Spin Centres in SiC for Quantum Technologies. Appl. Magn. Reson. 2016, 47, 793–812. [Google Scholar] [CrossRef]
- Murzakhanov, F.F.; Mamin, G.V.; Orlinskii, S.B.; Gerstmann, U.; Schmidt, W.G.; Biktagirov, T.; Aharonovich, I.; Gottscholl, A.; Sperlich, A.; Dyakonov, V.; et al. Electron–Nuclear Coherent Coupling and Nuclear Spin Readout through Optically Polarized VB–Spin States in hBN. Nano Lett. 2022, 22, 2718–2724. [Google Scholar] [CrossRef] [PubMed]
- Awschalom, D.D.; Hanson, R.; Wrachtrup, J.; Zhou, B.B. Quantum Technologies with Optically Interfaced Solid-State Spins. Nat. Photonics 2018, 12, 516–527. [Google Scholar] [CrossRef]
- Weber, J.R.; Koehl, W.F.; Varley, J.B.; Janotti, A.; Buckley, B.B.; Van de Walle, C.G.; Awschalom, D.D. Quantum Computing with Defects. Proc. Natl. Acad. Sci. USA 2010, 107, 8513–8518. [Google Scholar] [CrossRef] [PubMed]
- Weber, J.R.; Koehl, W.F.; Varley, J.B.; Janotti, A.; Buckley, B.B.; Van de Walle, C.G.; Awschalom, D.D. Defects in SiC for Quantum Computing. J. Appl. Phys. 2011, 109, 102417. [Google Scholar] [CrossRef]
- Khazen, K.; von Bardeleben, H.J. NV-Centers in SiC: A Solution for Quantum Computing Technology? Front. Quantum Sci. Technol. 2023, 2, 1115039. [Google Scholar] [CrossRef]
- Dong, J.; Chen, A.-B. Fundamental Properties of SiC: Crystal Structure, Bonding Energy, Band Structure, and Lattice Vibrations. In SiC Power Materials: Devices and Applications; Feng, Z.C., Ed.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 63–87. [Google Scholar] [CrossRef]
- Magnusson, B.; Son, N.T.; Csóré, A.; Gällström, A.; Ohshima, T.; Gali, A.; Ivanov, I.G. Excitation Properties of the Divacancy in 4H-SiC. Phys. Rev. B 2018, 98, 195202. [Google Scholar] [CrossRef]
- Lefèvre, J.; Costantini, J.-M.; Gourier, D.; Esnouf, S.; Petite, G. Characterization of a Silicon-Related Defect Detected by Its Excited Triplet State in Electron-Irradiated 3C-SiC. Phys. Rev. B 2011, 83, 075201. [Google Scholar] [CrossRef]
- Spaeth, J.M.; Overhof, H. Point Defects in Semiconductors and Insulators: Determination of Atomic and Electronic Structure from Paramagnetic Hyperfine Interactions; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar] [CrossRef]
- Bradley, C.E.; Randall, J.; Abobeih, M.H.; Berrevoets, R.C.; Degen, M.J.; Bakker, M.A.; Markham, M.; Twitchen, D.J.; Taminiau, T.H. A Ten-Qubit Solid-State Spin Register with Quantum Memory up to One Minute. Phys. Rev. X 2019, 9, 031045. [Google Scholar] [CrossRef]
- Soykal, Ö.O.; Dev, P.; Economou, S.E. Silicon Vacancy Center in 4H-SiC: Electronic Structure and Spin-Photon Interfaces. Phys. Rev. B 2016, 93, 081207. [Google Scholar] [CrossRef]
- Hoang, T.M.; Ishiwata, H.; Masuyama, Y.; Yamazaki, Y.; Kojima, K.; Lee, S.-Y.; Ohshima, T.; Iwasaki, T.; Hisamoto, D.; Hatano, M. Thermometric Quantum Sensor Using Excited State of Silicon Vacancy Centers in 4H-SiC Devices. Appl. Phys. Lett. 2021, 118, 044001. [Google Scholar] [CrossRef]
- Luo, Q.-Y.; Li, Q.; Wang, J.-F.; Guo, P.-J.; Lin, W.-X.; Zhao, S.; Hu, Q.-C.; Zhu, Z.-Q.; Xu, J.-S.; Li, C.-F.; et al. Fabrication and Quantum Sensing of Spin Defects in Silicon Carbide. Front. Phys. 2023, 11, 1270602. [Google Scholar] [CrossRef]
- Soltamov, V.A.; Kasper, C.; Poshakinskiy, A.V.; Anisimov, A.N.; Mokhov, E.N.; Sperlich, A.; Tarasenko, S.A.; Baranov, P.G.; Astakhov, G.V.; Dyakonov, V. Excitation and Coherent Control of Spin Qudit Modes in Silicon Carbide at Room Temperature. Nat. Commun. 2019, 10, 1678. [Google Scholar] [CrossRef] [PubMed]
- Thiering, G.; Gali, A. Theory of the Optical Spin-Polarization Loop of the Nitrogen-Vacancy Center in Diamond. Phys. Rev. B 2018, 98, 085207. [Google Scholar] [CrossRef]
- Zhu, Y.; Kovos, B.; Onizhuk, M.; Awschalom, D.; Galli, G. Theoretical and Experimental Study of the Nitrogen-Vacancy Center in 4H-SiC. Phys. Rev. Mater. 2021, 5, 074602. [Google Scholar] [CrossRef]
- Castelletto, S.; Boretti, A. Silicon Carbide Color Centers for Quantum Applications. J. Phys. Photonics 2020, 2, 022001. [Google Scholar] [CrossRef]
- Zargaleh, S.A.; von Bardeleben, H.J.; Cantin, J.L.; Gerstmann, U.; Hameau, S.; Eblé, B.; Gao, W. Electron Paramagnetic Resonance Tagged High-Resolution Excitation Spectroscopy of NV-Centers in 4H-SiC. Phys. Rev. B 2018, 98, 214113. [Google Scholar] [CrossRef]
- Khazen, K.; von Bardeleben, H.J.; Zargaleh, S.A.; Cantin, J.L.; Zhao, M.; Gao, W.; Biktagirov, T.; Gerstmann, U. High-Resolution Resonant Excitation of NV Centers in 6H: A Matrix for Quantum Technology Applications. Phys. Rev. B 2019, 100, 205202. [Google Scholar] [CrossRef]
- von Bardeleben, H.J.; Cantin, J.L.; Csóré, A.; Gali, A.; Rauls, E.; Gerstmann, U. NV Centers in 3C,4H, and 6H Silicon Carbide: A Variable Platform for Solid-State Qubits and Nanosensors. Phys. Rev. B 2016, 94, 121202. [Google Scholar] [CrossRef]
- Childress, L.; Hanson, R. Diamond NV Centers for Quantum Computing and Quantum Networks. MRS Bull. 2013, 38, 134–138. [Google Scholar] [CrossRef]
- Zhang, J.; Hegde, S.S.; Suter, D. Pulse Sequences for Controlled Two- and Three-Qubit Gates in a Hybrid Quantum Register. Phys. Rev. A 2018, 98, 042302. [Google Scholar] [CrossRef]
- Singh, L.R. Site Symmetry Dependence on Luminescence Emission of Y2O3:Eu3+ Dispersed in Silica Matrix. Mater. Technol. 2022, 37, 1906–1913. [Google Scholar] [CrossRef]
- Murzakhanov, F.F.; Sadovnikova, M.A.; Gracheva, I.N.; Mamin, G.V.; Baibekov, E.I.; Mokhov, E.N. Exploring the Properties of the VB- Defect in hBN: Optical Spin Polarization, Rabi Oscillations, and Coherent Nuclei Modulation. Nanotechnology 2024, 35, 155001. [Google Scholar] [CrossRef] [PubMed]
- Moro, F.; Turyanska, L.; Wilman, J.; Fielding, A.J.; Fay, M.W.; Granwehr, J.; Patanè, A. Electron spin coherence near room temperature in magnetic quantum dots. Sci. Rep. 2015, 5, 10855. [Google Scholar] [CrossRef] [PubMed]
- Son, N.T.; Ivanov, I.G. Charge State Control of the Silicon Vacancy and Divacancy in Silicon Carbide. J. Appl. Phys. 2021, 129, 215702. [Google Scholar] [CrossRef]
- Christle, D.J.; Klimov, P.V.; de las Casas, C.F.; Szász, K.; Ivády, V.; Jokubavicius, V.; Ul Hassan, J.; Syväjärvi, M.; Koehl, W.F.; Ohshima, T.; et al. Isolated Spin Qubits in SiC with a High-Fidelity Infrared Spin-to-Photon Interface. Phys. Rev. X 2017, 7, 021046. [Google Scholar] [CrossRef]
- Yamamoto, Y.; Takata, K.; Utsunomiya, S. Quantum Computing vs. Coherent Computing. New Gener. Comput. 2012, 30, 327–356. [Google Scholar] [CrossRef]
- Agnello, S.; Boscaino, R.; Cannas, M.; Gelardi, F.M. Instantaneous Diffusion Effect on Spin-Echo Decay: Experimental Investigation by Spectral Selective Excitation. Phys. Rev. B 2001, 64, 174423. [Google Scholar] [CrossRef]
- Murzakhanov, F.F.; Yavkin, B.V.; Mamin, G.V.; Orlinskii, S.B.; von Bardeleben, H.J.; Biktagirov, T.; Gerstmann, U.; Soltamov, V.A. Hyperfine and Nuclear Quadrupole Splitting of the NV-Ground State in 4H-SiC. Phys. Rev. B 2021, 103, 245203. [Google Scholar] [CrossRef]
- Reynhardt, E.C.; High, G.L.; van Wyk, J.A. Temperature Dependence of Spin-Spin and Spin-Lattice Relaxation Times of Paramagnetic Nitrogen Defects in Diamond. J. Chem. Phys. 1998, 109, 8471–8477. [Google Scholar] [CrossRef]
- Goldfarb, D.; Stoll, S. EPR Spectroscopy: Fundamentals and Methods; John Wiley & Sons: Hoboken, NJ, USA, 2018. [Google Scholar]
- Zaripov, R.; Vavilova, E.; Miluykov, V.; Bezkishko, I.; Sinyashin, O.; Salikhov, K.; Kataev, V.; Büchner, B. Boosting the Electron Spin Coherence in Binuclear Mn Complexes by Multiple Microwave Pulses. Phys. Rev. B 2013, 88, 094418. [Google Scholar] [CrossRef]
- Ashhab, S.; Johansson, J.R.; Nori, F. Rabi Oscillations in a Qubit Coupled to a Quantum Two-Level System. New J. Phys. 2006, 8, 103. [Google Scholar] [CrossRef]
- Bourassa, A.; Anderson, C.P.; Miao, K.C.; Onizhuk, M.; Ma, H.; Crook, A.L.; Abe, H.; Ul-Hassan, J.; Ohshima, T.; Son, N.T.; et al. Entanglement and Control of Single Nuclear Spins in Isotopically Engineered Silicon Carbide. Nat. Mater. 2020, 19, 1319–1325. [Google Scholar] [CrossRef] [PubMed]
- Reiserer, A.; Kalb, N.; Blok, M.S.; van Bemmelen, K.J.M.; Taminiau, T.H.; Hanson, R.; Twitchen, D.J.; Markham, M. Robust Quantum-Network Memory Using Decoherence-Protected Subspaces of Nuclear Spins. Phys. Rev. X 2016, 6, 021040. [Google Scholar] [CrossRef]
- Blok, M.S.; Kalb, N.; Reiserer, A.; Taminiau, T.H.; Hanson, R. Towards Quantum Networks of Single Spins: Analysis of a Quantum Memory with an Optical Interface in Diamond. Faraday Discuss. 2015, 184, 173–182. [Google Scholar] [CrossRef]
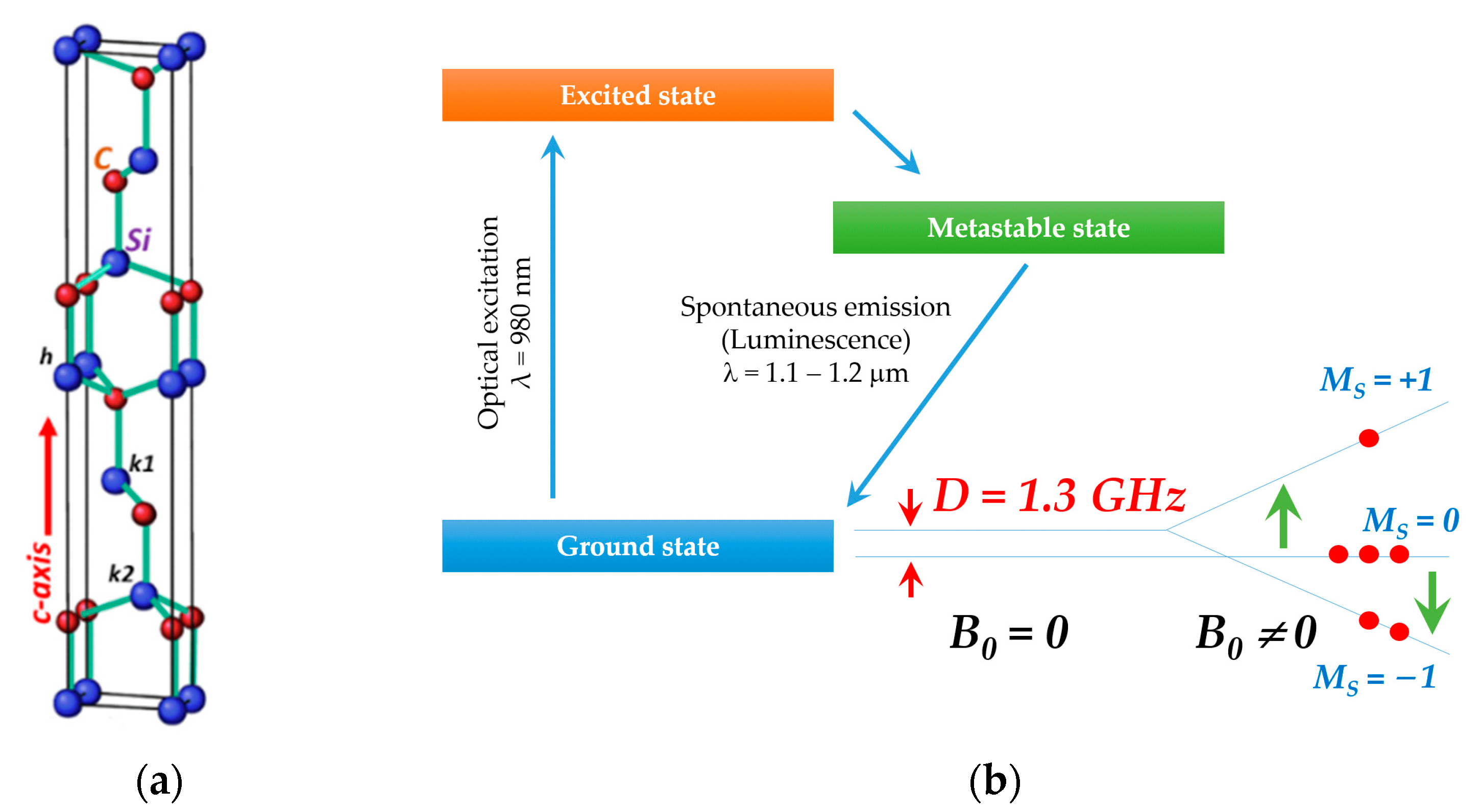
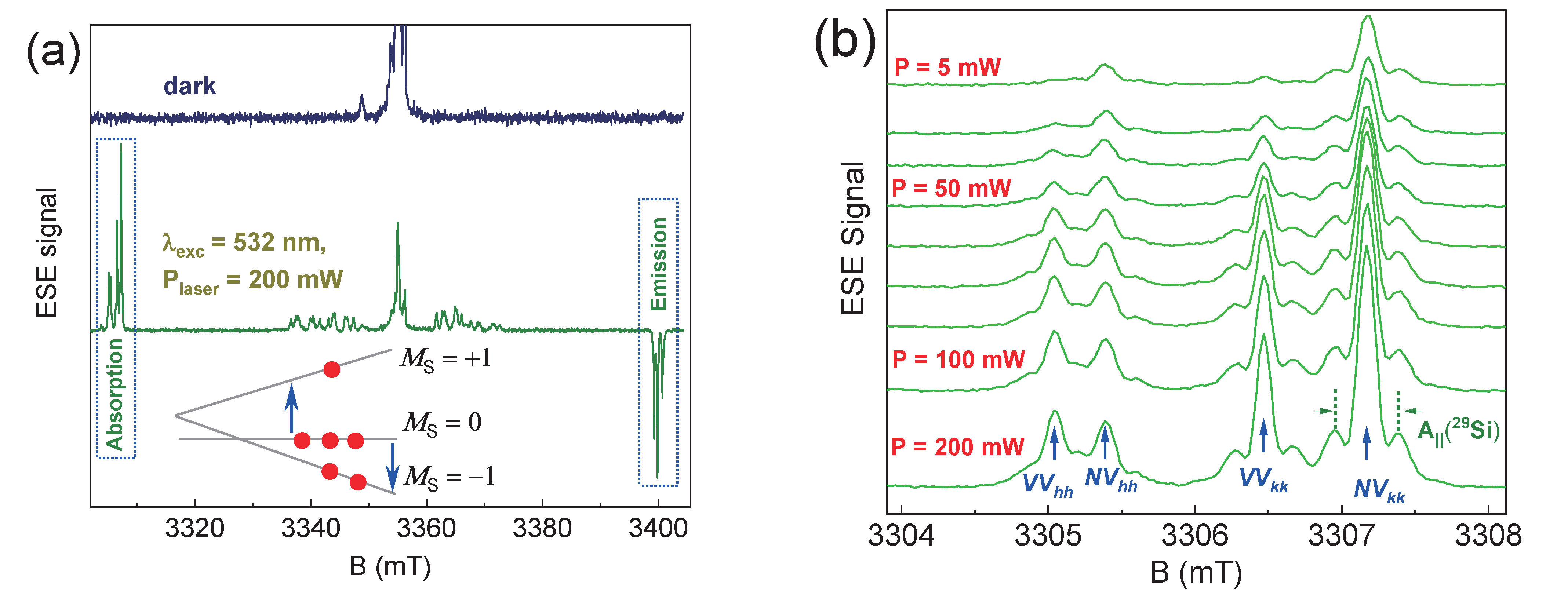
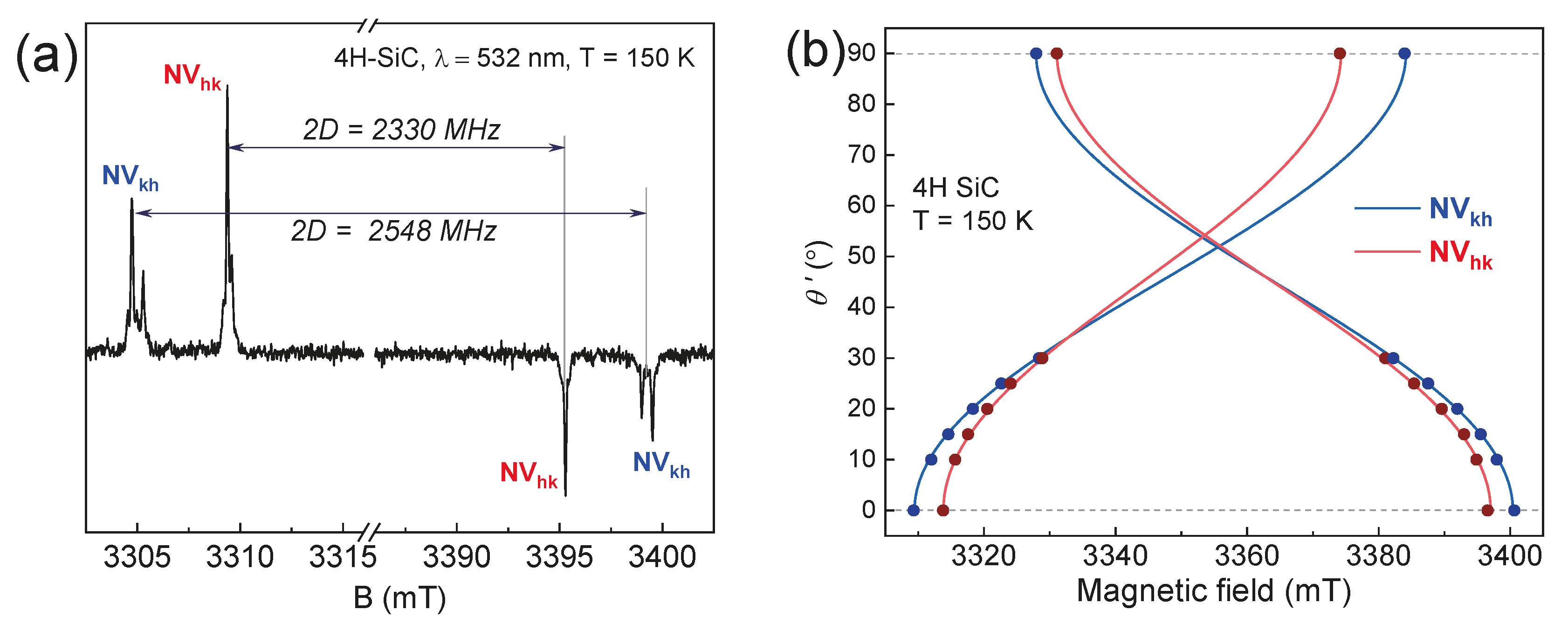

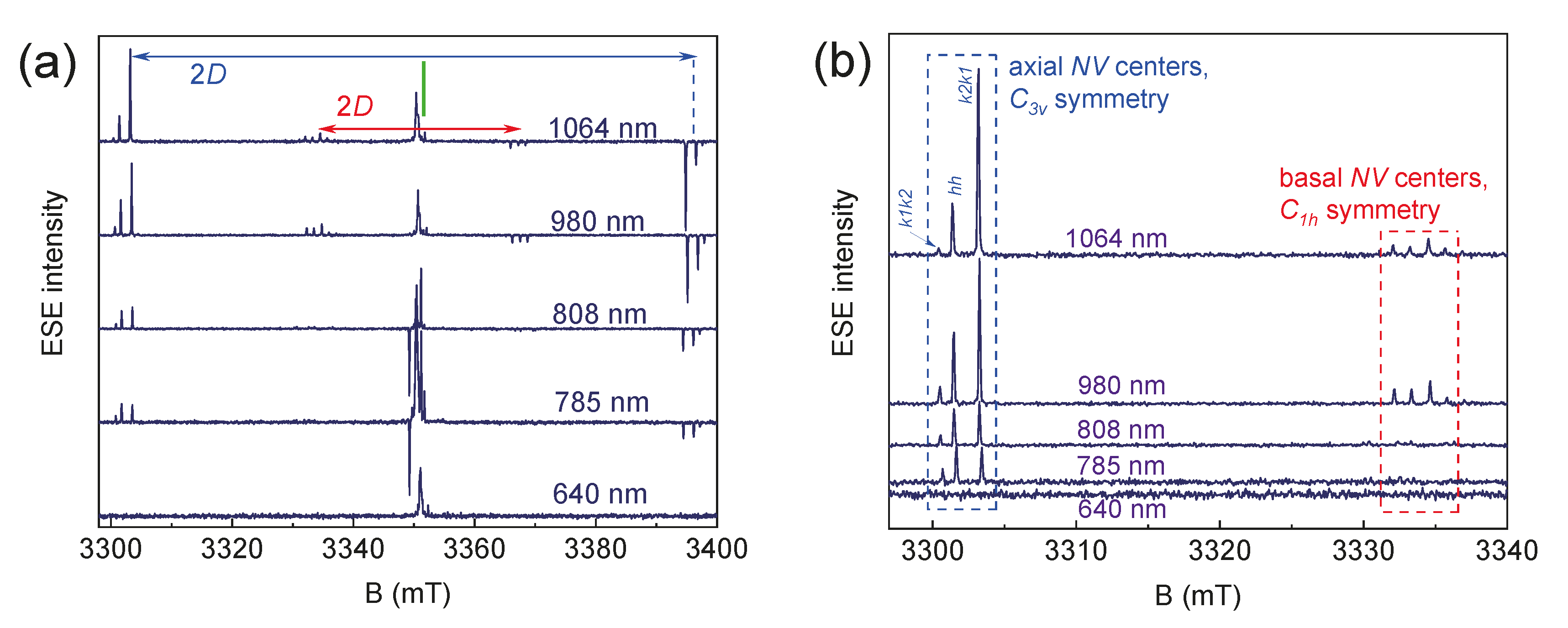
| g⊥ | g|| | D (MHz) | E (MHz) | |
|---|---|---|---|---|
| NVkk | 2.001(1) | 2.004(1) | 1299(10) | 0 |
| NVhh | 2.003(1) | 2.004(1) | 1349(10) | 0 |
| NVkh | 2.001(1) | 2.003(1) | 1274(20) | 13(2) |
| NVhk | 2.003(1) | 2.002(1) | 1165(20) | 110(5) |
| P (MHz) | Cq (MHz) | a (Isotropic, MHz) | b (Anisotropic, kHz) | |
|---|---|---|---|---|
| NVkk | 1.81 | 2.413 | −1.14 | 14 |
| NVhh | 1.895 | 2.53 | −1.185 | 10 |
| NVkh | 1.82(2) | 2.43(1) | −1.05 | 4 |
| NVhk | 1.73(2) | 2.31(1) | −0.87 | 11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Latypova, L.; Murzakhanov, F.; Mamin, G.; Sadovnikova, M.; von Bardeleben, H.J.; Rau, J.V.; Gafurov, M. Exploring High-Spin Color Centers in Wide Band Gap Semiconductors SiC: A Comprehensive Magnetic Resonance Investigation (EPR and ENDOR Analysis). Molecules 2024, 29, 3033. https://doi.org/10.3390/molecules29133033
Latypova L, Murzakhanov F, Mamin G, Sadovnikova M, von Bardeleben HJ, Rau JV, Gafurov M. Exploring High-Spin Color Centers in Wide Band Gap Semiconductors SiC: A Comprehensive Magnetic Resonance Investigation (EPR and ENDOR Analysis). Molecules. 2024; 29(13):3033. https://doi.org/10.3390/molecules29133033
Chicago/Turabian StyleLatypova, Larisa, Fadis Murzakhanov, George Mamin, Margarita Sadovnikova, Hans Jurgen von Bardeleben, Julietta V. Rau, and Marat Gafurov. 2024. "Exploring High-Spin Color Centers in Wide Band Gap Semiconductors SiC: A Comprehensive Magnetic Resonance Investigation (EPR and ENDOR Analysis)" Molecules 29, no. 13: 3033. https://doi.org/10.3390/molecules29133033
APA StyleLatypova, L., Murzakhanov, F., Mamin, G., Sadovnikova, M., von Bardeleben, H. J., Rau, J. V., & Gafurov, M. (2024). Exploring High-Spin Color Centers in Wide Band Gap Semiconductors SiC: A Comprehensive Magnetic Resonance Investigation (EPR and ENDOR Analysis). Molecules, 29(13), 3033. https://doi.org/10.3390/molecules29133033








