Theoretical Insight into Psittacofulvins and Their Derivatives
Abstract
1. Introduction
2. Computational Details
- (i)
- the global descriptors of the chemical activity: the ionization potential IP, electron affinity EA, chemical potential μ, absolute electronegativity χ, molecular hardness η and softness S, electrophilicity index ω, the electro-donating ω− and electro-accepting ω+ powers, and the Ra, Rd indexes [18,19,20];
- (ii)
- the thermodynamic descriptors: the bond dissociation enthalpy BDE, adiabatic ionization potential AIP, proton dissociation enthalpy PDE, proton affinity PA, electron transfer enthalpy ETE, the free Gibbs acidity Hacidity (in the gas phase) or Gacidity (in hydrophobic, i.e., benzene, and hydrophilic, i.e., water, solvents) [18,19,20].
BDE = H(R●) + H(H●) − H(R–H)
R–H●+ → R● + H+
AIP = H(R–H●+) + H(e−) − H(R–H)
PDE = H(R●) + H(H+) − H(R–H●+)
R− + X● + H+ → R● + X–H
PA = H(R−) + H(H+) − H(R–H)
ETE = H(R●) + H(e−) − H(R−)
3. Results and Discussion
- (i)
- the hydrophobic environment does not affect the antiradical activity of the tested compounds, which is comparable to that in a vacuum and characterized by the BDE parameter related to the HAT mechanism;
- (ii)
- in an aqueous medium, the antiradical activity of the derivatives analyzed increases, whereas the scavenging mechanism preferred changes to SPLET;
- (iii)
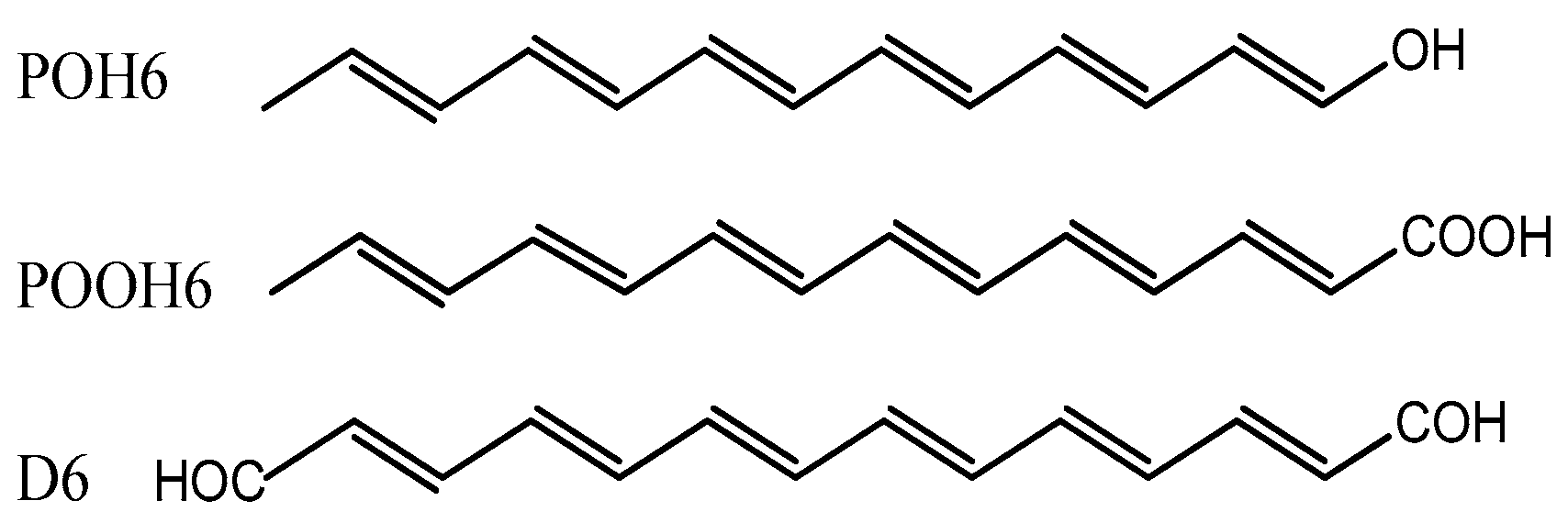
- the hydroxyl derivative POH6 of P6 shows less activity than the parent compound (the SPLET total energy PA + ETE = 145.27 [kcal mol−1]); therefore, it will not be considered at the second stage of calculations;
- (iv)
- D6 has (PA + ETE = 119.77 [kcal mol−1]) greater antiradical activity than P6, whereas POOH6 is a stronger metal chelation agent (Gacidity(H2O) = 286.42 [kcal mol−1]) than P6; therefore, the research will also cover their polyunsaturated derivatives based on the P7–9 parent compounds.
4. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krukenberg, C.F.W. Die Federfarbstoffe der Psittaciden. Vgl.-Physiol. Stud. Reihe Abtlg 1882, 2, 29–36. [Google Scholar]
- Stradi, R.; Pina, E.; Celentano, G. The chemical structure of the pigments in Ara macao plumage. Comp. Biochem. Physiol. Part B 2001, 130, 57–63. [Google Scholar] [CrossRef] [PubMed]
- McGraw, K.J.; Nogare, M.C. Carotenoid pigments and the selectivity of psittacofulvin based coloration systems in parrots. Comp. Biochem. Physiol. Part B 2004, 138, 229–233. [Google Scholar] [CrossRef] [PubMed]
- Tay, E.J.; Barnsley, J.E.; Thomas, D.B.; Gordon, K.C. Elucidating the resonance Raman spectra of psittacofulvins. Mol. Biomol. Spectrosc. 2021, 262, 120146. [Google Scholar] [CrossRef] [PubMed]
- Adamec, F.; Greco, J.A.; LaFountain, A.M.; Magdaong, N.M.; Fuciman, M.; Birge, R.R.; Polivka, T.; Frank, H.A. Spectroscopic investigation of a brightly colored psittacofulvin pigment from parrot feathers. Chem. Phys. Lett. 2016, 648, 195–199. [Google Scholar] [CrossRef]
- de Oliveira Neves, A.C.; Galván, I.; den Abeele, D.V. Impairment of mixed melanin-based pigmentation in parrots. J. Exp. Biol. 2020, 223, jeb225912. [Google Scholar] [CrossRef] [PubMed]
- Cooke, T.F.; Fischer, C.R.; Wu, P.; Jiang, T.-X.; Xie, K.T.; Kuo, J.; Doctorov, E.; Zehnder, A.; Khosla, C.; Chuong, C.-M.; et al. Genetic Mapping and Biochemical Basis of Yellow Feather Pigmentation in Budgerigars. Cell 2017, 171, 427–439.e21. [Google Scholar] [CrossRef]
- Roy, S.G.; Bakhart, A.; Abdu, M.; Afonso, A.; Pereira, P.; Carneiro, M.; Abdu, U. Mutations in SLC45A2 lead to loss of melanin in parrot feathers. G3 Genes Genomes Genet. 2024, 14, jkad254. [Google Scholar] [CrossRef]
- Ke, F.; van der Zwan, H.; Poon, E.S.; Cloutier, A.; Van den Abeele, D.; van der Sluis, R.; Sin, S.Y. Convergent evolution of parrot plumage coloration. PNAS Nexus 2024, 3, pgae107. [Google Scholar] [CrossRef]
- Mundy, N.I. Colouration Genetics: Pretty Polymorphic Parrots. Curr. Biol. 2018, 3, R113–R114. [Google Scholar] [CrossRef]
- Martinez, A. Donator acceptor map of psittacofulvins and anthocyanins: Are they good antioxidant substances? J. Phys. Chem. 2009, 113, 4915–4921. [Google Scholar] [CrossRef] [PubMed]
- Burtt, E.H., Jr.; Schroeder, M.R.; Smith, L.A.; Sroka, J.E.; McGraw, K.J. Colourful parrot feathers resist bacterial degradation. Biol. Lett. 2011, 7, 214–216. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Pini, E.; Bertelli, A.; Stradi, R.; Falchi, M. Biological activity of parrodienes, a new class of polyunsaturated linear aldehydes similar to carotenoids. Drugs Exp. Clin. Res. 2004, 30, 203–206. [Google Scholar] [PubMed]
- Flori, E.; Mastrofrancesco, A.; Kovacs, D.; Ramot, Y.; Briganti, S.; Bellei, B.; Paus, R.; Picardo, M. 2,4,6-Octatrienoic acid is a novel promoter of melanogenesis and antioxidant defence in normal human melanocytes via PPAR-γ activation. Pigment Cell Melanoma Res. 2011, 24, 618–630. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, S.; Ray, D.; Falchi, M.; Bertelli, A.; Pini, E.; Das, D.K. Cardioprotection with the parrodiene 2,4,6-octatrienal and its potassium salt through activation of the Akt-Bcl-2 survival pathway. J. Nat. Prod. 2009, 72, 871–875. [Google Scholar] [CrossRef] [PubMed]
- Tagliazucchi, D.; Verzelloni, E.; Pini, E.; Ronca, G.; Conte, A. Effect of chain length and aldehydic function on some biological properties of parropolyenes. Pharmacologyonline 2006, 3, 765–771. [Google Scholar]
- Morelli, R.; Loscalzo, R.; Stradi, R.; Bertelli, A.; Falchi, M. Evaluation of the antioxidant activity of new carotenoid-like compounds by electron paramagnetic resonance. Drugs Exp. Clin. Res. 2003, 29, 95–100. [Google Scholar] [PubMed]
- Molski, M. Density functional theory studies on the chemical reactivity of allyl mercaptan and its derivatives. Molecules 2024, 29, 668. [Google Scholar] [CrossRef] [PubMed]
- Molski, M. Theoretical study on the radical scavenging activity of gallic acid. Heliyon 2023, 9, e12806. [Google Scholar] [CrossRef]
- Rimarčik, J.; Lukeš, V.; Klein, E.; Ilčin, M. Study of the solvent effect on the enthalpies of homolytic and heterolytic N–H bond cleavage in p-phenylenediamine and tetracyano-p-phenylenediamine. J. Mol. Struct. THEOCHEM 2010, 952, 25–30. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16, Revision A.03; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef] [PubMed]
- Dunning, T.H. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 1989, 90, 1007–1023. [Google Scholar] [CrossRef]
- Weigend, F.; Ahlrichs, R.R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Account. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Becke, A.D. A new mixing of Hartree–Fock and local density-functional theories. J. Chem. Phys. 1993, 98, 1372–1377. [Google Scholar] [CrossRef]
- Cossi, M.; Rega, N.; Scalmani, G.; Barone, V. Energies, structures, and electronic properties of molecules in solution with the C-PCM solvation model. J. Comput. Chem. 2003, 24, 669–681. [Google Scholar] [CrossRef]
- Molski, M. Theoretical modeling of structure-toxicity relationship of cyanides. Toxicol. Lett. 2021, 349, 30–39. [Google Scholar] [CrossRef]
- Griffin, W.C. Classification of Surface-Active Agents by “HLB”. J. Soc. Cosmet. Chem. 1949, 1, 311–326. [Google Scholar]
- Davies, J.T. A quantitative kinetic theory of emulsion type, I. Physical chemistry of the emulsifying agent. Gas/Liquid and Liquid/Liquid Interface. In Proceedings of the International Congress of Surface Activity; Butterworths: London, UK, 1957; pp. 426–438. [Google Scholar]
- Stradi, R.; Bertelli, A.; Pini, E. Polyunsaturated Linear Aldehydes and Their Derivatives with Antiradical and Anti-Tumoral Activity. European Patent EP1501774B1, 2 February 2005. [Google Scholar]
- Gutmann, H.; Manz, U.; Schwieter, U. Polyene Compounds as Coloring Agents. U.S. Patent US3577464A, 4 May 1971. [Google Scholar]
- Leopoldini, M.; Russo, N.; Toscano, M. Gas and liquid phase acidity of natural antioxidants. J. Agric. Food Chem. 2006, 54, 3078–3085. [Google Scholar] [CrossRef]
- Li, X.; Ouyang, X.; Liang, M.; Chen, D. Comparative analysis of radical adduct formation (RAF) products and an tioxidant pathways between myricetin-3- O-galactoside and myricetin aglycone. Molecules 2019, 24, 2769. [Google Scholar] [CrossRef] [PubMed]
- Parr, R.G.; Yang, W. Density Functional Theory of Atoms and Molecules; Oxford University Press: Oxford, UK, 1989. [Google Scholar] [CrossRef]
- Pearson, R.G. Chemical Hardness—Applications from Molecules to Solids; Wiley: Weinheim, Germany, 1997; ISBN 978-3-527-60617-7. [Google Scholar]
- Sanchez-Marquez, J.; García, V.; Zorrilla, D.; Fernandez, M. On electronegativity, hardness, and reactivity descriptors: A new property-oriented basis set. J. Phys. Chem. A 2020, 124, 4700–4711. [Google Scholar] [CrossRef] [PubMed]
- Parr, R.G.; Szentpály, L.V.; Liu, S. Electrophilicity index. J. Am. Chem. Soc. 1999, 121, 1922–1924. [Google Scholar] [CrossRef]
- Gázquez, J.L.; Cedillo, A.; Vela, A.J. Electrodonating and electroaccepting powers. Phys. Chem. 2007, 111, 1966–1970. [Google Scholar] [CrossRef]
| Descriptor | P6 | POH6 | POOH6 | D6 |
|---|---|---|---|---|
| Medium | Vacuum | |||
| BDE | 74.43 | 100.47 | 102.89 | 72.13 |
| PA | 350.94 | 360.43 | 340.43 | 331.55 |
| ETE | 38.00 | 54.55 | 76.97 | 55.09 |
| AIP | 156.11 | 146.03 | 153.92 | 168.00 |
| PDE | 232.82 | 268.95 | 263.47 | 218.64 |
| Hacidity | 349.46 | 358.95 | 338.95 | 330.31 |
| Mechanism | HAT | HAT | HAT | HAT |
| Medium | Water | |||
| BDE | 76.56 | 99.17 | 86.15 | 73.67 |
| PA | 75.09 | 67.64 | 44.10 | 57.34 |
| ETE | 47.57 | 77.63 | 88.15 | 62.43 |
| PA + ETE | 122.66 | 145.27 | 132.25 | 119.77 |
| AIP | 95.28 | 89.55 | 94.58 | 105.64 |
| PDE | 27.38 | 55.71 | 37.66 | 14.13 |
| Gacidity | 317.98 | 311.24 | 286.42 | 300.59 |
| Mechanism | SPLET | SPLET | SPLET | SPLET |
| Medium | Benzene | |||
| BDE | 77.39 | 102.13 | 99.08 | 74.85 |
| PA | 119.50 | 122.35 | 99.33 | 101.26 |
| ETE | 55.52 | 77.41 | 97.38 | 71.21 |
| AIP | 93.79 | 126.66 | 133.18 | 144.79 |
| PDE | 81.23 | 73.09 | 63.53 | 27.69 |
| Gacidity | 331.68 | 335.08 | 310.94 | 313.85 |
| Mechanism | HAT | HAT | HAT + SPLET | HAT |
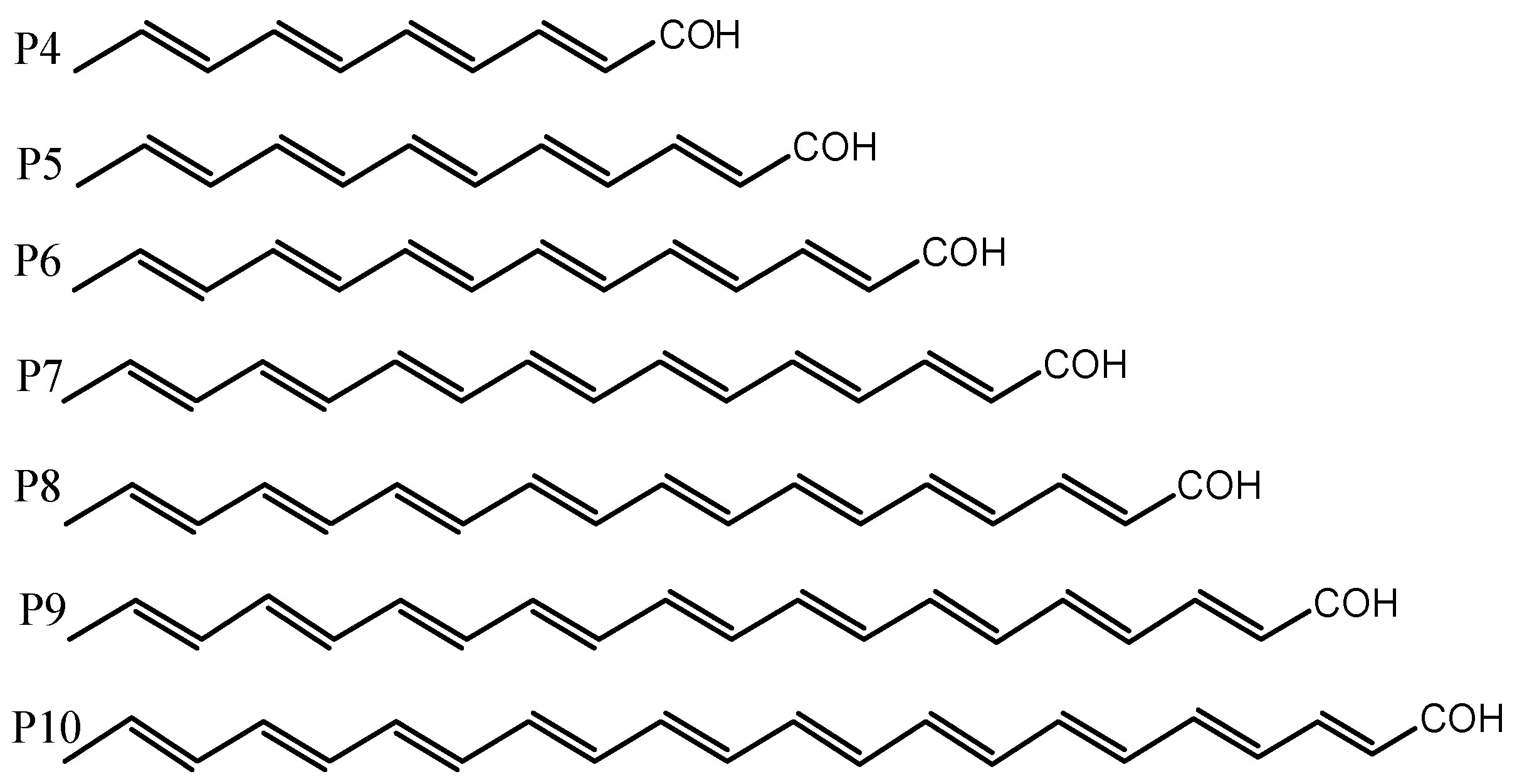
| Descriptor | P4 | P5 | P6 | P7 | P8 | P9 | P10 |
|---|---|---|---|---|---|---|---|
| BDE | 79.24 | 77.70 | 76.56 | 75.69 | 74.99 | 74.41 | 73.92 |
| PA | 83.02 | 78.45 | 75.09 | 72.51 | 70.47 | 68.85 | 67.50 |
| ETE | 42.32 | 45.35 | 47.57 | 49.27 | 50.60 | 51.66 | 52.52 |
| PA + ETE | 125.34 | 123.80 | 122.66 | 121.78 | 121.07 | 120.51 | 120.02 |
| AIP | 105.22 | 99.50 | 95.28 | 92.04 | 89.50 | 87.43 | 85.72 |
| PDE | 20.11 | 24.29 | 27.38 | 29.74 | 31.59 | 33.08 | 34.40 |
| Gacidity | 325.45 | 321.16 | 317.98 | 315.59 | 313.72 | 312.26 | 311.11 |
| d [a] | 10.37 | 11.61 | 12.69 | 13.64 | 14.47 | 15.18 | 15.80 |
| Mechanism | HAT | HAT-SPLET | SPLET | SPLET | SPLET | SPLET | SPLET |
| EA | 2.7141 | 2.8229 | 2.9029 | 2.9633 | 3.0112 | 3.0488 | 3.0792 |
| IP | 5.9095 | 5.6611 | 5.4771 | 5.3342 | 5.2235 | 5.1334 | 5.0572 |
| ΔE | 3.1954 | 2.8381 | 2.5742 | 2.3709 | 2.2123 | 2.0847 | 1.9779 |
| η | 1.5977 | 1.4191 | 1.2871 | 1.1855 | 1.1061 | 1.0423 | 0.9889 |
| S | 0.3129 | 0.3523 | 0.3885 | 0.4218 | 0.4520 | 0.4797 | 0.5056 |
| χ = −μ | 4.3118 | 4.2420 | 4.1900 | 4.1488 | 4.1174 | 4.0911 | 4.0682 |
| ω | 5.8181 | 6.3402 | 6.8201 | 7.2598 | 7.6629 | 8.0287 | 8.3673 |
| ω+ | 3.8620 | 4.3966 | 4.8859 | 5.3336 | 5.7425 | 6.1134 | 6.4568 |
| ω− | 8.1737 | 8.6386 | 9.0760 | 9.4824 | 9.8599 | 10.2045 | 10.5251 |
| Ra [b] | 1.1352 | 1.2924 | 1.4362 | 1.5678 | 1.6880 | 1.7970 | 1.8980 |
| Rd [b] | 2.3557 | 2.4896 | 2.6157 | 2.7328 | 2.8416 | 2.9409 | 3.0333 |
| Descriptor | POOH6 | POOH7 | POOH8 | POOH9 |
|---|---|---|---|---|
| BDE | 86.15 | 84.23 | 82.59 | 81.16 |
| PA | 44.10 | 44.11 | 44.12 | 44.13 |
| ETE | 88.15 | 86.22 | 84.57 | 83.13 |
| PA + ETE | 132.25 | 130.33 | 128.69 | 127.26 |
| AIP | 94.58 | 91.43 | 88.94 | 86.93 |
| PDE | 37.66 | 38.90 | 39.75 | 40.33 |
| Gacidity | 286.42 | 286.49 | 286.50 | 286.47 |
| Mechanism | SPLET | SPLET | SPLET | SPLET |
| EA | 2.7856 | 2.8572 | 2.9133 | 2.9581 |
| IP | 5.4453 | 5.3073 | 5.1987 | 5.1108 |
| ΔE | 2.6596 | 2.4501 | 2.2855 | 2.1527 |
| η | 1.3298 | 1.2251 | 1.1427 | 1.0763 |
| S | 0.3760 | 0.4081 | 0.4375 | 0.4645 |
| χ = −μ | 4.1155 | 4.0823 | 4.0560 | 4.0345 |
| ω | 6.3681 | 6.8016 | 7.1981 | 7.5613 |
| ω+ | 4.4766 | 4.9136 | 5.3129 | 5.6786 |
| ω− | 8.5921 | 8.9959 | 9.3689 | 9.7131 |
| Ra [a] | 1.3159 | 1.4444 | 1.5617 | 1.6692 |
| Rd [a] | 2.4762 | 2.5926 | 2.7001 | 2.7993 |
| Descriptor | D6 | D7 | D8 | D9 |
|---|---|---|---|---|
| BDE | 73.67 | 73.42 | 73.17 | 72.95 |
| PA | 57.34 | 57.04 | 56.80 | 56.60 |
| ETE | 62.43 | 62.47 | 62.47 | 62.44 |
| PA + ETE | 119.77 | 119.51 | 119.27 | 119.04 |
| AIP | 105.64 | 99.49 | 96.08 | 93.29 |
| PDE | 14.13 | 20.02 | 23.19 | 25.75 |
| Gacidity | 300.59 | 300.47 | 300.33 | 300.23 |
| Mechanism | SPLET | SPLET | SPLET | SPLET |
| EA | 3.2975 | 3.3007 | 3.3032 | 3.3051 |
| IP | 5.8379 | 5.6510 | 5.5019 | 5.3802 |
| ΔE | 2.5405 | 2.3502 | 2.1987 | 2.0751 |
| η | 1.2702 | 1.1751 | 1.0993 | 1.0376 |
| S | 0.3936 | 0.4255 | 0.4548 | 0.4819 |
| χ = −μ | 4.5677 | 4.4759 | 4.4025 | 4.3427 |
| ω | 8.2127 | 8.5239 | 8.8154 | 9.0879 |
| ω+ | 6.0876 | 6.4329 | 6.7516 | 7.0463 |
| ω− | 10.6553 | 10.9087 | 11.1541 | 11.3890 |
| Ra [a] | 1.7894 | 1.8909 | 1.9846 | 2.0712 |
| Rd [a] | 3.0709 | 3.1439 | 3.2146 | 3.2823 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Molski, M. Theoretical Insight into Psittacofulvins and Their Derivatives. Molecules 2024, 29, 2760. https://doi.org/10.3390/molecules29122760
Molski M. Theoretical Insight into Psittacofulvins and Their Derivatives. Molecules. 2024; 29(12):2760. https://doi.org/10.3390/molecules29122760
Chicago/Turabian StyleMolski, Marcin. 2024. "Theoretical Insight into Psittacofulvins and Their Derivatives" Molecules 29, no. 12: 2760. https://doi.org/10.3390/molecules29122760
APA StyleMolski, M. (2024). Theoretical Insight into Psittacofulvins and Their Derivatives. Molecules, 29(12), 2760. https://doi.org/10.3390/molecules29122760






