Robust Ferromagnetism in Hexagonal Honeycomb Transition Metal Nitride Monolayer
Abstract
1. Introduction
2. Results
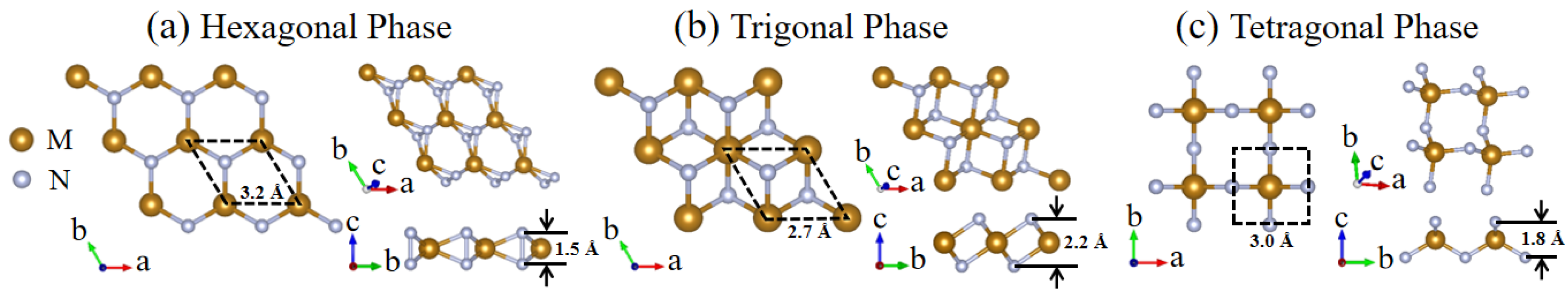
2.1. Atomic Structure
2.2. Evidence for Structural Stability
2.3. Electronic Structure
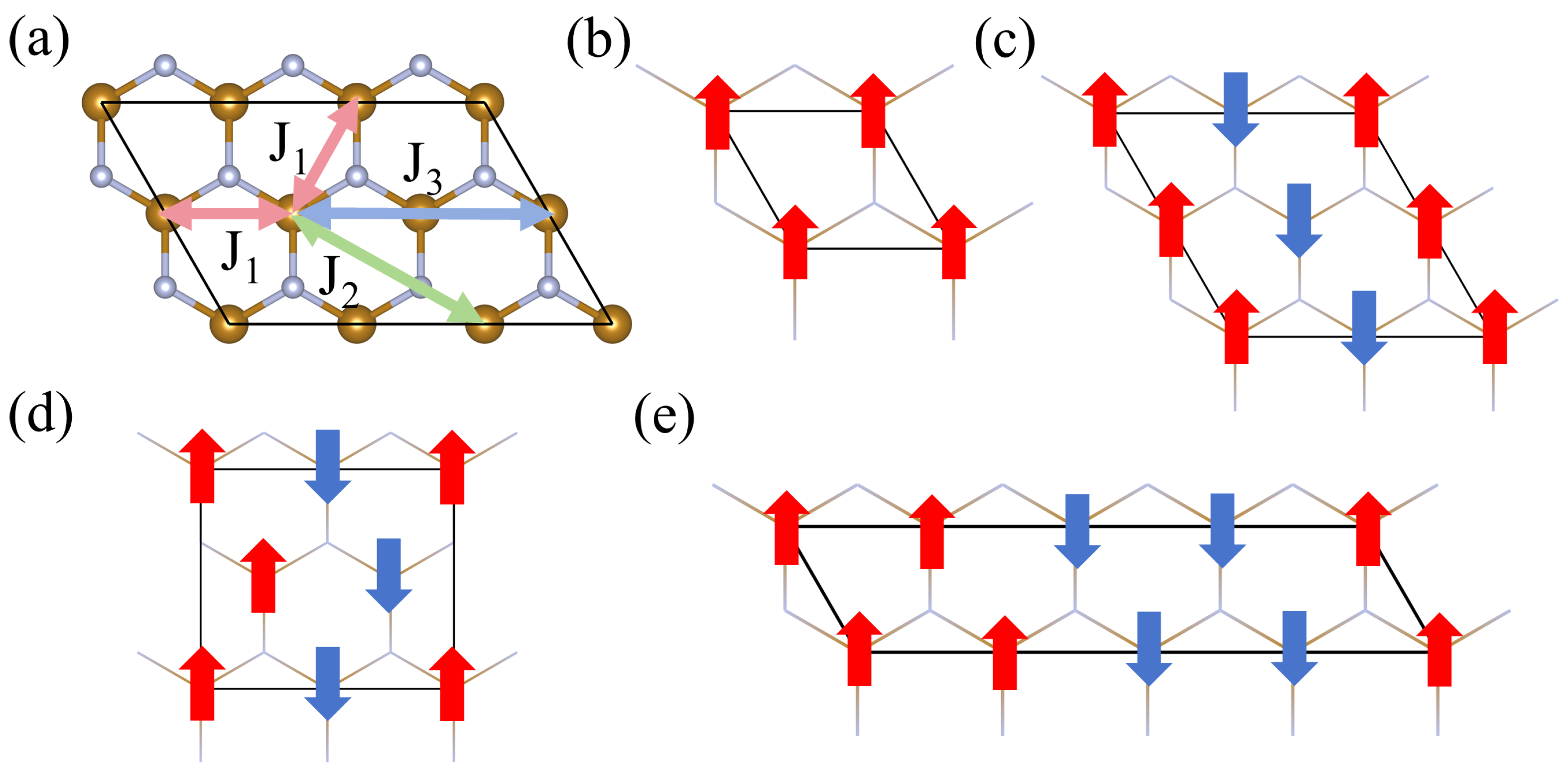
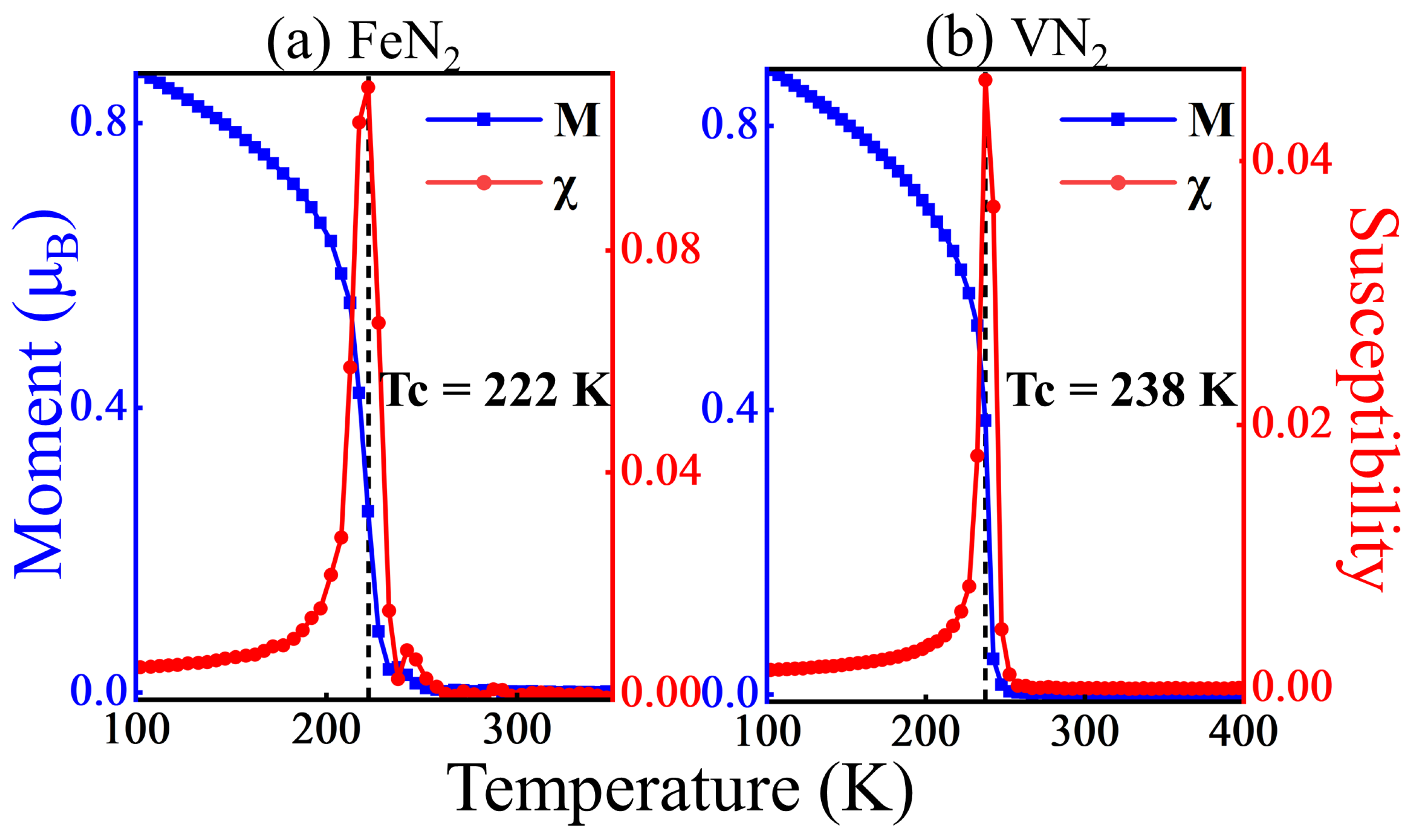
2.4. Ferromagnetism and Curie Temperature
3. Computational Methods
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef]
- Park, H.; Kim, T.K.; Cho, S.W.; Jang, H.S.; Lee, S.I.; Choi, S.Y. Large-scale synthesis of uniform hexagonal boron nitride films by plasma-enhanced atomic layer deposition. Sci. Rep. 2017, 7, 40091. [Google Scholar] [CrossRef] [PubMed]
- Lalmi, B.; Oughaddou, H.; Enriquez, H.; Kara, A.; Vizzini, S.; Ealet, B.; Aufray, B. Epitaxial growth of a silicene sheet. Appl. Phys. Lett. 2010, 97, 223109. [Google Scholar] [CrossRef]
- Mannix, A.J.; Zhou, X.F.; Kiraly, B.; Wood, J.D.; Alducin, D.; Myers, B.D.; Liu, X.; Fisher, B.L.; Santiago, U.; Guest, J.R.; et al. Synthesis of borophenes: Anisotropic, two-dimensional boron polymorphs. Science 2015, 350, 1513–1516. [Google Scholar] [CrossRef] [PubMed]
- Coleman, J.N.; Lotya, M.; O’Neill, A.; Bergin, S.D.; King, P.J.; Khan, U.; Young, K.; Gaucher, A.; De, S.; Smith, R.J.; et al. Two-Dimensional Nanosheets Produced by Liquid Exfoliation of Layered Materials. Science 2011, 331, 568–571. [Google Scholar] [CrossRef]
- Zheng, Y.; Li, X.; Pi, C.; Song, H.; Gao, B.; Chu, P.K.; Huo, K. Recent advances of two-dimensional transition metal nitrides for energy storage and conversion applications. FlatChem 2020, 19, 100149. [Google Scholar] [CrossRef]
- Liu, D.; Feng, P.; Gao, M.; Yan, X.W. CoN4C2: Two-dimensional cobalt carbonitride with a flat-band feature. Phys. Rev. B 2021, 103, 155411. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, S.; Gao, M.; Yan, X.W. Prediction of the two-dimensional cobalt carbonitride compounds CoN4C10, Co2N8C6, and Co2N6C6. Phys. Rev. B 2021, 103, 125407. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, S.; Gao, M.; Yan, X.W.; Xie, Z.Y. Robust ferromagnetism in single-atom-thick ternary chromium carbonitride CrN4C2. Appl. Phys. Lett. 2021, 118, 223104. [Google Scholar] [CrossRef]
- Wang, Y.; Ding, Y. Uncovering a Stable Phase in Group v Transition-metal Dinitride (MN2, M = Ta, Nb, V) Nanosheets and Their Electronic Properties via First-principles Investigations. J. Phys. Chem. C 2018, 122, 26748–26755. [Google Scholar] [CrossRef]
- Zheng, F.; Xiao, X.; Xie, J.; Zhou, L.; Li, Y.; Dong, H. Structures, properties and applications of two-dimensional metal nitrides: From nitride MXene to other metal nitrides. 2D Mater. 2022, 9, 022001. [Google Scholar] [CrossRef]
- Naguib, M.; Kurtoglu, M.; Presser, V.; Lu, J.; Niu, J.; Heon, M.; Hultman, L.; Gogotsi, Y.; Barsoum, M. Two-Dimensional Nanocrystals: Two-Dimensional Nanocrystals Produced by Exfoliation of Ti3AlC2 (Adv. Mater. 37/2011). Adv. Mater. 2011, 23, 4207. [Google Scholar] [CrossRef]
- Naguib, M.; Mochalin, V.N.; Barsoum, M.W.; Gogotsi, Y. 25th Anniversary Article: MXenes: A New Family of Two-Dimensional Materials. Adv. Mater. 2014, 26, 992–1005. [Google Scholar] [CrossRef] [PubMed]
- Khazaei, M.; Arai, M.; Sasaki, T.; Chung, C.Y.Y.; Venkataramanan, N.S.; Estili, M.; Sakka, Y.; Kawazoe, Y. Novel Electronic and Magnetic Properties of Two-Dimensional Transition Metal Carbides and Nitrides. Adv. Funct. Mater. 2013, 23, 2185–2192. [Google Scholar] [CrossRef]
- Sun, Q.; Li, J.; Li, Y.; Yang, Z.; Wu, R. Cr2NX2 MXene (X = O, F, OH): A 2D ferromagnetic half-metal. Appl. Phys. Lett. 2021, 119, 62404. [Google Scholar] [CrossRef]
- Onodera, M.; Kawamura, F.; Cuong, N.T.; Watanabe, K.; Moriya, R.; Masubuchi, S.; Taniguchi, T.; Okada, S.; Machida, T. Rhenium dinitride: Carrier transport in a novel transition metal dinitride layered crystal. APL Mater. 2019, 7, 101103. [Google Scholar] [CrossRef]
- Yu, Y.; Chen, X.; Liu, X.; Li, J.; Sanyal, B.; Kong, X.; Peeters, F.M.; Li, L. Ferromagnetism with in-plane magnetization, Dirac spin-gapless semiconducting properties, and tunable topological states in two-dimensional rare-earth metal dinitrides. Phys. Rev. B 2022, 105, 24407. [Google Scholar] [CrossRef]
- Mermin, N.D.; Wagner, H. Absence of ferromagnetism or antiferromagnetism in one- or two-dimensional isotropic Heisenberg models. Phys. Rev. Lett. 1966, 17, 1133–1136. [Google Scholar] [CrossRef]
- Huang, B.; Clark, G.; Navarro-Moratalla, E.; Klein, D.R.; Cheng, R.; Seyler, K.L.; Zhong, D.; Schmidgall, E.; McGuire, M.A.; Cobden, D.H.; et al. Layer-dependent ferromagnetism in a van der Waals crystal down to the monolayer limit. Nature 2017, 546, 270–273. [Google Scholar] [CrossRef]
- Gong, C.; Li, L.; Li, Z.; Ji, H.; Stern, A.; Xia, Y.; Cao, T.; Bao, W.; Wang, C.; Wang, Y.; et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature 2017, 546, 265–269. [Google Scholar] [CrossRef]
- Bonilla, M.; Kolekar, S.; Ma, Y.; Diaz, H.C.; Kalappattil, V.; Das, R.; Eggers, T.; Gutierrez, H.R.; Phan, M.H.; Batzill, M. Strong room-temperature ferromagnetism in VSe2 monolayers on van der Waals substrates. Nat. Nanotechnol. 2018, 13, 289–293. [Google Scholar] [CrossRef]
- Zhong, Y.; Peng, C.; Huang, H.; Guan, D.; Hwang, J.; Hsu, K.H.; Hu, Y.; Jia, C.; Moritz, B.; Lu, D.; et al. From Stoner to local moment magnetism in atomically thin Cr2Te3. Nat. Commun. 2023, 14, 5340. [Google Scholar] [CrossRef]
- Zhang, G.; Guo, F.; Wu, H.; Wen, X.; Yang, L.; Jin, W.; Zhang, W.; Chang, H. Above-room-temperature strong intrinsic ferromagnetism in 2D van der Waals Fe3GaTe2 with large perpendicular magnetic anisotropy. Nat. Commun. 2022, 13, 5067. [Google Scholar] [CrossRef] [PubMed]
- Houmes, M.J.A.; Baglioni, G.; Šiškins, M.; Lee, M.; Esteras, D.L.; Ruiz, A.M.; Mañas-Valero, S.; Boix-Constant, C.; Baldoví, J.J.; Coronado, E.; et al. Magnetic order in 2D antiferromagnets revealed by spontaneous anisotropic magnetostriction. Nat. Commun. 2023, 14, 8503. [Google Scholar] [CrossRef]
- Wilsdorf, H. Die Kristallstruktur des einwertigen Kupferazids, CuN3. Acta Crystallogr. 1948, 1, 115–118. [Google Scholar] [CrossRef]
- Crowhurst, J.C.; Goncharov, A.F.; Sadigh, B.; Evans, C.L.; Morrall, P.G.; Ferreira, J.L.; Nelson, A.J. Synthesis and characterization of the nitrides of platinum and iridium. Science 2006, 311, 1275–1278. [Google Scholar] [CrossRef]
- Groenewolt, M.; Antonietti, M. Synthesis of g-C3N4 Nanoparticles in Mesoporous Silica Host Matrices. Adv. Mater. 2005, 17, 1789–1792. [Google Scholar] [CrossRef]
- Cococcioni, M.; de Gironcoli, S. Linear response approach to the calculation of the effective interaction parameters in the LDA + U method. Phys. Rev. B 2005, 71, 35105. [Google Scholar] [CrossRef]
- Ma, F.; Lu, Z.Y.; Xiang, T. Arsenic-bridged antiferromagnetic superexchange interactions in LaFeAsO. Phys. Rev. B 2008, 78, 224517. [Google Scholar] [CrossRef]
- Ma, F.; Ji, W.; Hu, J.; Lu, Z.Y.; Xiang, T. First-Principles Calculations of the Electronic Structure of Tetragonal α -FeTe and α -FeSe Crystals: Evidence for a Bicollinear Antiferromagnetic Order. Phys. Rev. Lett. 2009, 102, 177003. [Google Scholar] [CrossRef]
- Yan, X.W.; Gao, M.; Lu, Z.Y.; Xiang, T. Electronic Structures and Magnetic Order of Ordered-Fe-Vacancy Ternary Iron Selenides TlFe1.5Se2 and AFe1.5Se2 (A = K, Ru, or Cs). Phys. Rev. Lett. 2011, 106, 087005. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.; Feng, P.; Liu, D.; Wu, H.; Gao, M.; Xu, T.; Xie, Z.Y.; Yan, X.W. Two-dimensional binary transition metal nitride MN$_4$(M= V, Cr, Mn, Fe, Co) with a graphenelike structure and strong magnetic properties. Phys. Rev. B 2022, 106, 235402. [Google Scholar] [CrossRef]
- Liu, D.; Feng, P.; Zhang, S.; Gao, M.; Ma, F.; Yan, X.W.; Xie, Z.Y. Prediction of single-atom-thick transition metal nitride CrN4 with a square-planar network and high-temperature ferromagnetism. Phys. Rev. B 2022, 106, 125421. [Google Scholar] [CrossRef]
- Xie, Z.Y.; Jiang, H.C.; Chen, Q.N.; Weng, Z.Y.; Xiang, T. Second Renormalization of Tensor-Network States. Phys. Rev. Lett. 2009, 103, 160601. [Google Scholar] [CrossRef]
- Guo, Y.; Wang, B.; Zhang, X.; Yuan, S.; Ma, L.; Wang, J. Magnetic two-dimensional layered crystals meet with ferromagnetic semiconductors. InfoMat 2020, 2, 639–655. [Google Scholar] [CrossRef]
- Liu, J.; Sun, Q.; Kawazoe, Y.; Jena, P. Exfoliating biocompatible ferromagnetic Cr-trihalide monolayers. Phys. Chem. Chem. Phys. 2016, 18, 8777–8784. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef]
- Sun, J.; Ruzsinszky, A.; Perdew, J.P. Strongly Constrained and Appropriately Normed Semilocal Density Functional. Phys. Rev. Lett. 2015, 115, 036402. [Google Scholar] [CrossRef] [PubMed]
- Togo, A.; Tanaka, I. First principles phonon calculations in materials science. Scr. Mater. 2015, 108, 1–5. [Google Scholar] [CrossRef]
- Martyna, G.J.; Klein, M.L.; Tuckerman, M. Nosé–Hoover chains: The canonical ensemble via continuous dynamics. J. Chem. Phys. 1992, 97, 2635–2643. [Google Scholar] [CrossRef]
- Liu, L.; Chen, S.; Lin, Z.; Zhang, X. A Symmetry-Breaking Phase in Two-Dimensional FeTe2 with Ferromagnetism above Room Temperature. J. Phys. Chem. Lett. 2020, 11, 7893–7900. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Zhang, J.; Liu, C.; Zhang, S.; Yuan, Y.; Li, P.; Wen, Y.; Jiang, Z.; Zhou, B.; Lei, Y.; et al. Chiral Helimagnetism and One-Dimensional Magnetic Solitons in a Cr-Intercalated Transition Metal Dichalcogenide. Adv. Mater. 2021, 33, 2101131. [Google Scholar] [CrossRef]
- Liu, C.; Jiang, J.; Zhang, C.; Wang, Q.; Zhang, H.; Zheng, D.; Li, Y.; Ma, Y.; Algaidi, H.; Gao, X.; et al. Controllable Skyrmionic Phase Transition between Néel Skyrmions and Bloch Skyrmionic Bubbles in van der Waals Ferromagnet Fe3-δGeTe2. Adv. Sci. 2023, 10, 2303443. [Google Scholar] [CrossRef]




| System | H-Phase (eV) | T-Phase (eV) | Tetra-Phase (eV) |
|---|---|---|---|
| FeN2 | −22.93576 | −21.42701 | −22.45834 |
| VN2 | −25.99425 | −25.15685 | −24.97182 |
| EFM | EAFM−I | EAFM−II | EAFM−III | M | J1 | J2 | J3 | MAE | ||
|---|---|---|---|---|---|---|---|---|---|---|
| (meV) | (meV) | (meV) | (meV) | () | (meV/S2) | (meV/S2) | (meV/S2) | (eV/S2) | (K) | |
| FeN2 | 0 | 86.74 | 68.45 | 33.29 | 3.3 | −19.65 | −2.07 | 3.57 | −144 | 222 |
| VN2 | 0 | 73.71 | 46.46 | 40.79 | 1.0 | −10.64 | −7.80 | 2.92 | −53 | 238 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, X.; Wang, Z.; Yue, Y.; Gao, M.; Ma, F.; Yan, X.-W. Robust Ferromagnetism in Hexagonal Honeycomb Transition Metal Nitride Monolayer. Molecules 2024, 29, 2322. https://doi.org/10.3390/molecules29102322
Ma X, Wang Z, Yue Y, Gao M, Ma F, Yan X-W. Robust Ferromagnetism in Hexagonal Honeycomb Transition Metal Nitride Monolayer. Molecules. 2024; 29(10):2322. https://doi.org/10.3390/molecules29102322
Chicago/Turabian StyleMa, Xiaolin, Zengqian Wang, Yuanfang Yue, Miao Gao, Fengjie Ma, and Xun-Wang Yan. 2024. "Robust Ferromagnetism in Hexagonal Honeycomb Transition Metal Nitride Monolayer" Molecules 29, no. 10: 2322. https://doi.org/10.3390/molecules29102322
APA StyleMa, X., Wang, Z., Yue, Y., Gao, M., Ma, F., & Yan, X.-W. (2024). Robust Ferromagnetism in Hexagonal Honeycomb Transition Metal Nitride Monolayer. Molecules, 29(10), 2322. https://doi.org/10.3390/molecules29102322








