Crystal Structure, Chemical Bond, and Microwave Dielectric Properties of Ba1−xSrx(Zn1/3Nb2/3)O3 Solid Solution Ceramics
Abstract
:1. Introduction
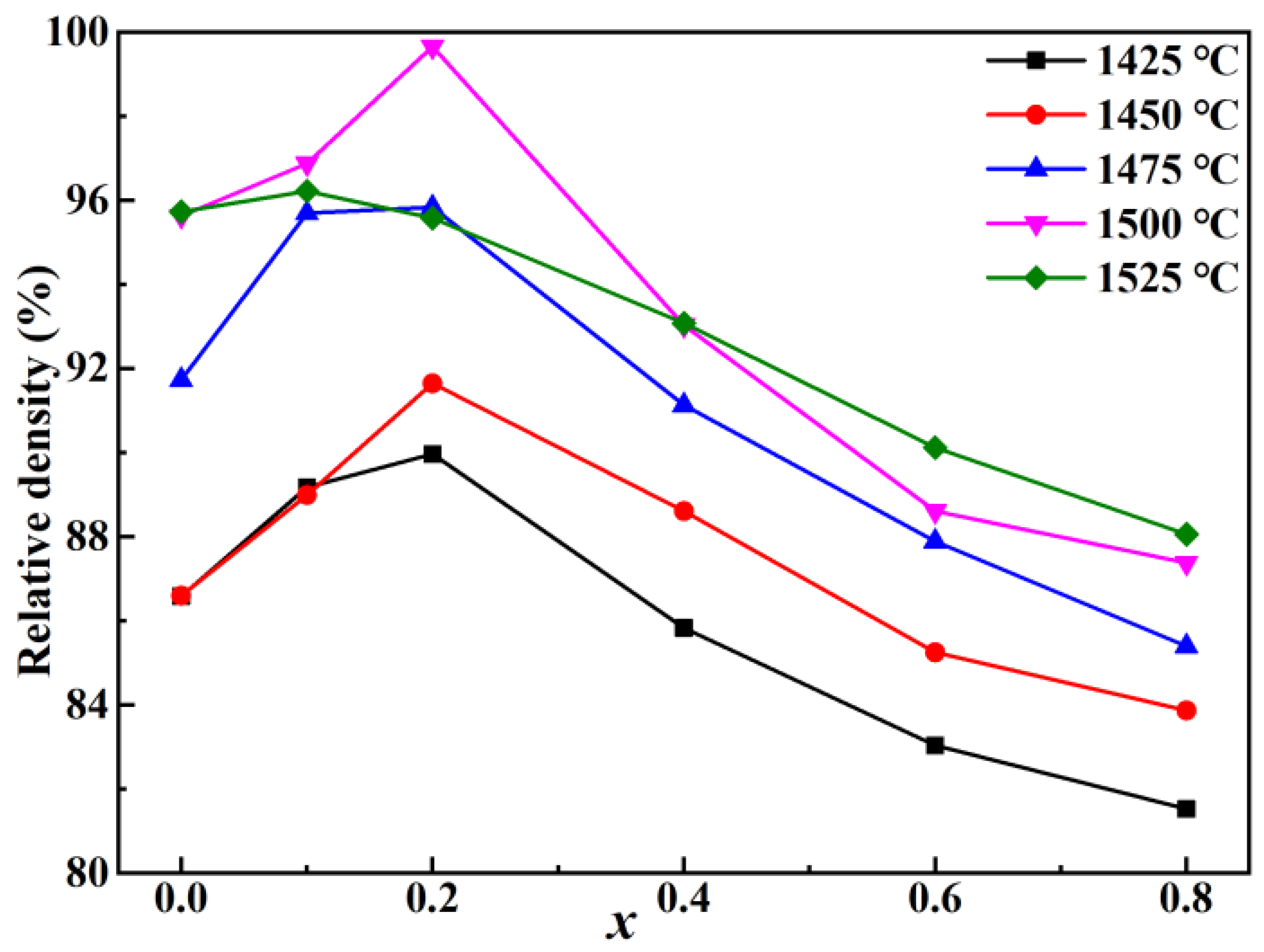
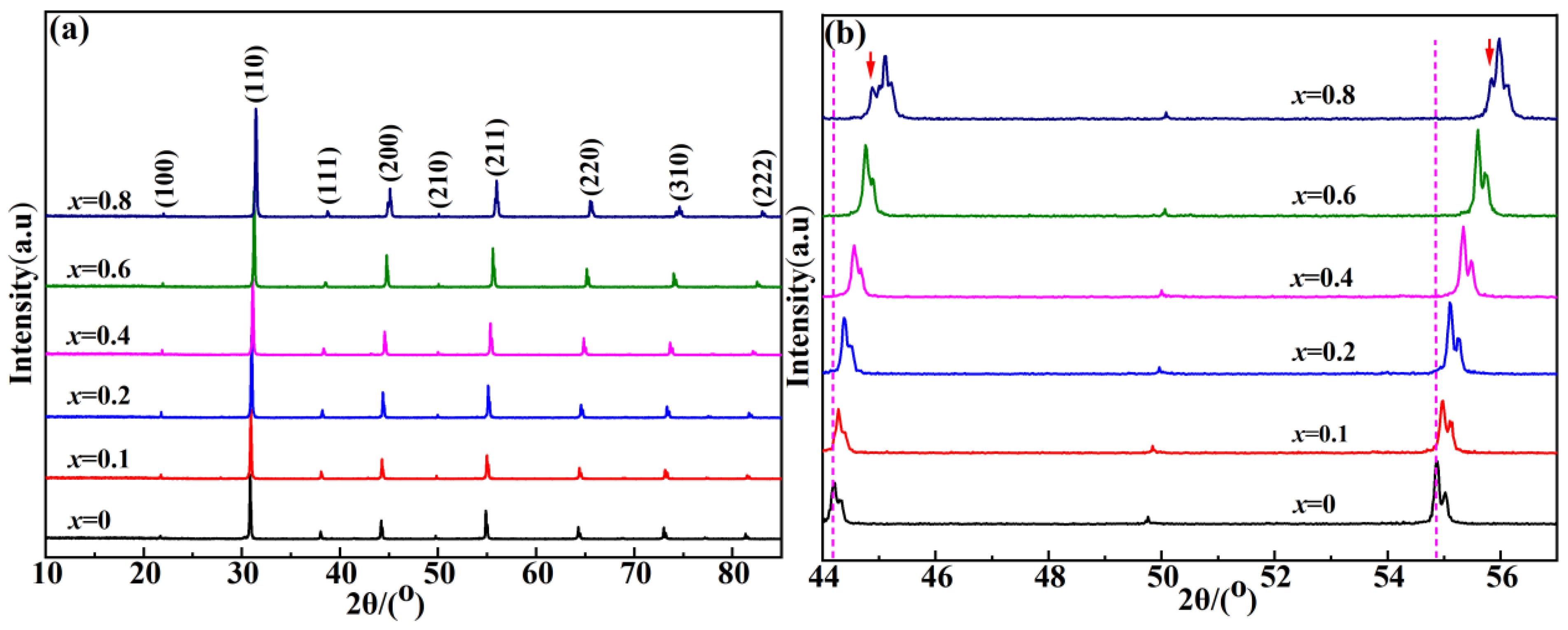
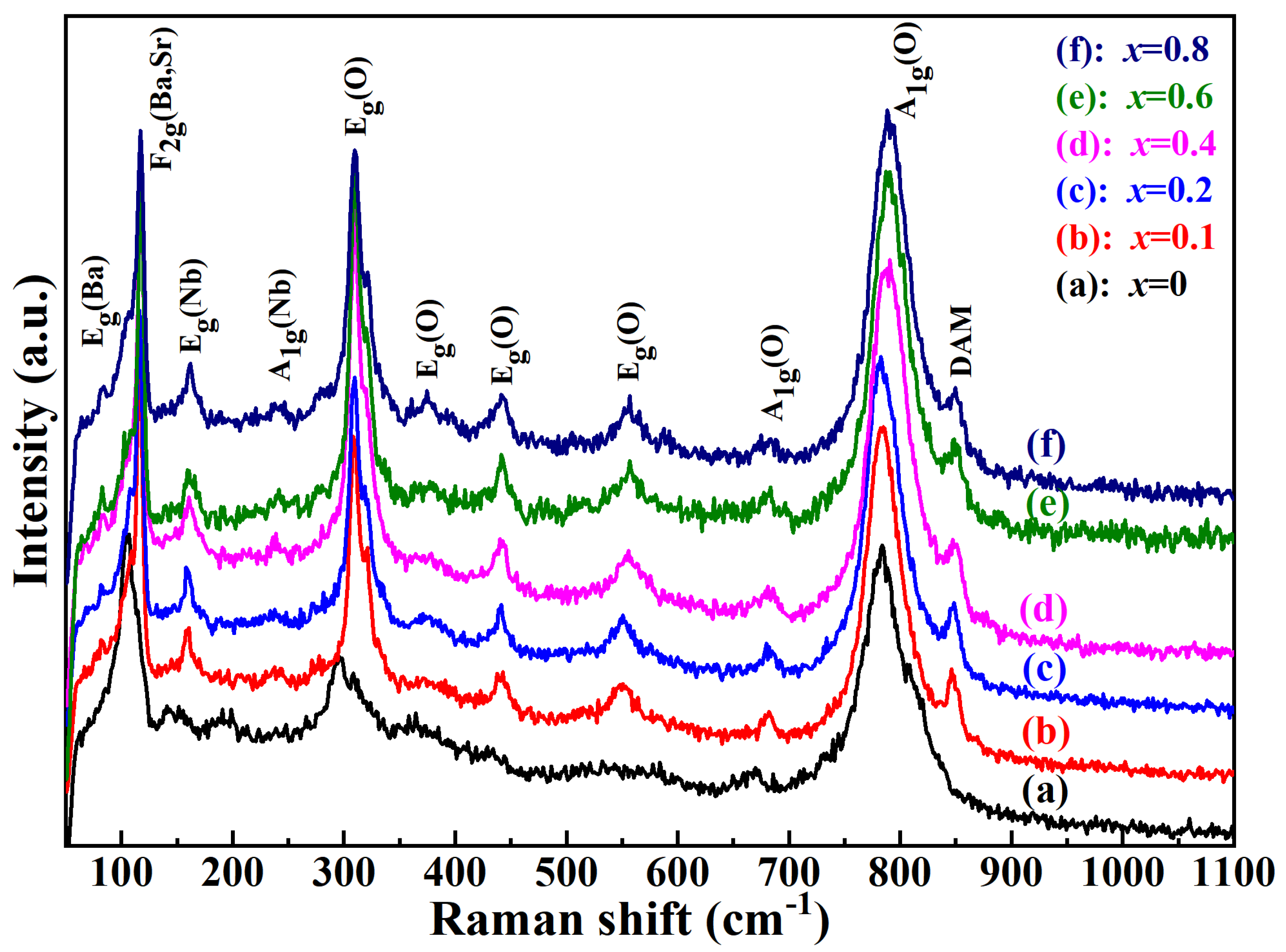
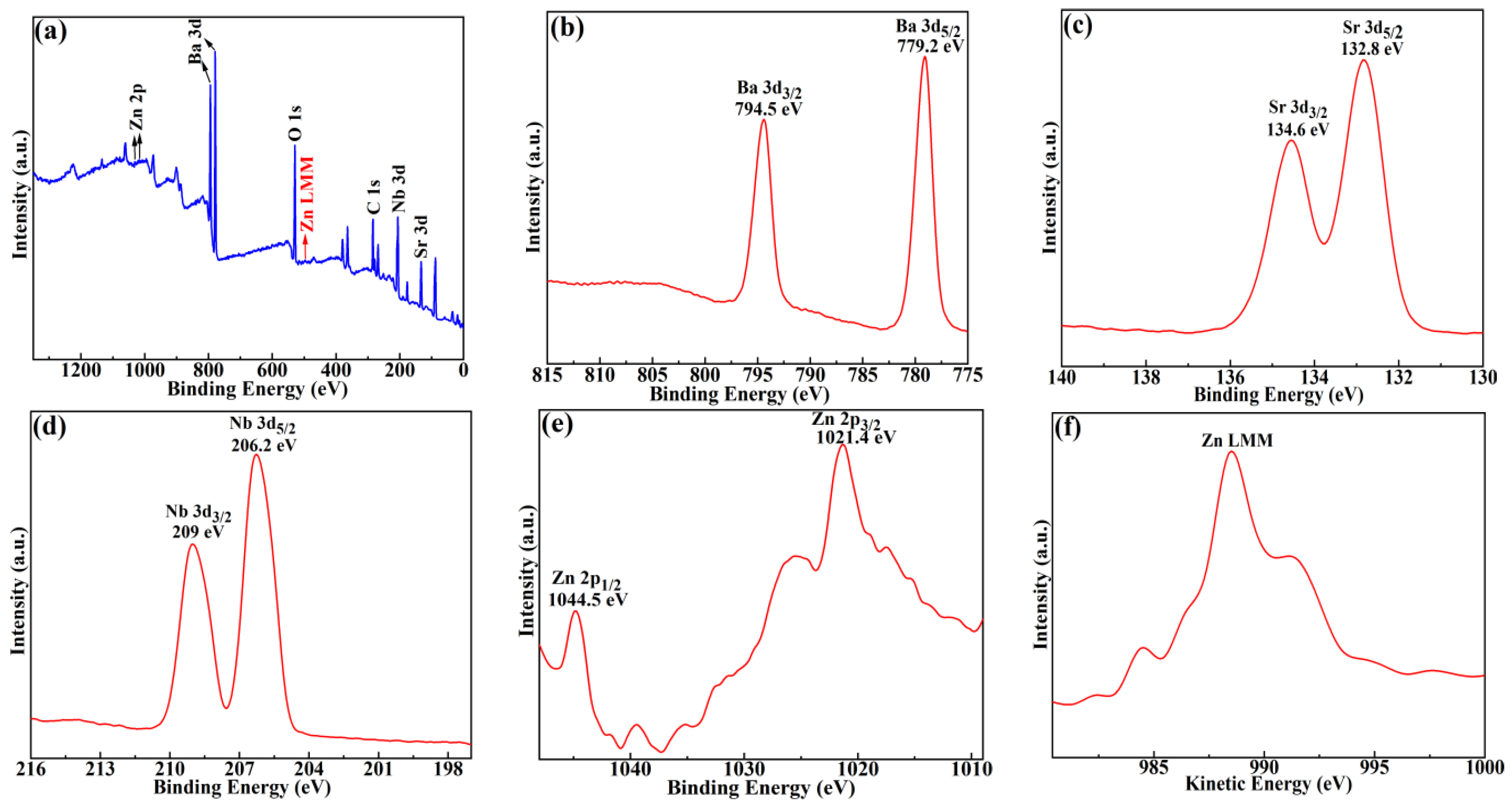
2. Results and Discussion
3. Materials and Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Onoda, M.; Kuwata, J.; Kaneta, K.; Toyama, K.; Nomura, S. Ba(Zn1/3Nb2/3)O3–Sr(Zn1/3Nb2/3)O3 solid solution ceramics with temperature-stable high dielectric constant and low microwave loss. Jpn. J. Appl. Phys. 1982, 21, 1707–1710. [Google Scholar] [CrossRef]
- Ma, P.P.; Chen, X.M. Further ordering structural investigation of Ba((Co, Zn, Mg)1/3Nb2/3)O3 perovskites by Raman spectroscopy. Mater. Charact. 2019, 158, 109938–109945. [Google Scholar] [CrossRef]
- Faruque, M.R.I.; Ahamed, E.; Rahman, M.A.; Islam, M.T. Flexible nickel aluminate (NiAl2O4) based dual-band double negative metamaterial for microwave applications. Results Phys. 2019, 14, 10252. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Y.; Xiang, M. Crystal structure and microwave dielectric characteristics of Zr-substituted CoTiNb2O8 ceramics. J. Am. Ceram. Soc. 2016, 36, 1945–1951. [Google Scholar] [CrossRef]
- Liu, D.; Chen, X.M.; Hu, X. Microwave dielectric ceramics in (Ca1−xBax)(Zn1/3Nb2/3)O3 system. Mater. Lett. 2007, 61, 1166–1169. [Google Scholar] [CrossRef]
- Liu, Y.C.; Chen, J.H.; Wang, H.W.; Liu, C.Y. Synthesis of (BaxSr1−x)(Zn1/3Nb2/3)O3 ceramics by reaction-sintering process and microstructure. Mater. Res. Bull. 2006, 41, 455–460. [Google Scholar] [CrossRef]
- Bai, J.H.; Liu, Q.L.; Li, X.; Wei, X.; Li, L.P. Optimization of Sintering Conditions to Enhance the Dielectric Performance of Gd3+ and Ho3+ Co-doped BaTiO3 Ceramics. Molecules 2022, 27, 7464. [Google Scholar] [CrossRef]
- Molodetsky, I.; Davies, P.K. Effect of Ba(Y1/2Nb1/2)O3 and BaZrO3 on the cation order and properties of Ba(Co1/3Nb2/3)O3 microwave ceramics. J. Eur. Ceram. Soc. 2001, 21, 2587–2591. [Google Scholar] [CrossRef]
- Koga, E.; Yamagishi, Y.; Moriwake, H.; Kakimoto, K.; Ohsato, H. Order-Disorder transition and its effect on microwave quality factor Q in Ba(Zn1/3Nb2/3)O3 System. J. Electroceram. 2006, 17, 375–379. [Google Scholar] [CrossRef]
- Chen, Y.C.; Syu, R.Y.; Ding, X.F. Enhancement quality factor by Zr4+ Substitution at B-site of ZnNiTiO4 microwave ceramics. Ceram. Int. 2017, 43, S301–S305. [Google Scholar] [CrossRef]
- Pan, C.-L.; Shen, C.-H.; Lin, S.-H.; Lin, Q.-Z. Tunable Microwave Dielectric Properties of Ca0.6La0.8/3TiO3 and Ca0.8Sm0.4/3TiO3-Modified (Mg0.6Zn0.4)0.95Ni0.05TiO3 Ceramics with a Near-Zero Temperature Coefficient. Molecules 2021, 26, 4715. [Google Scholar] [CrossRef] [PubMed]
- Li, L.X.; Jin, Y.X.; Dong, H.L.; Yu, S.H.; Xu, D. Effect of Sn4+ substitution on the dielectric properties of Bi2O3–ZnO–Nb2O5 pyrochlores. Ceram. Int. 2014, 40, 16133–16139. [Google Scholar] [CrossRef]
- Bian, J.J.; Dong, Y.F.; Song, G.X. Microwave Dielectric Properties of A-Site Modified Ba(Co0.7Zn0.3)1/3Nb2/3O3 by La3+. J. Eur. Ceram. Soc. 2008, 91, 1182–1187. [Google Scholar] [CrossRef]
- Surendran, K.P.; Mohanan, P.; Sebastion, M.T. The effect of glass additives on the microwave dielectric properties of Ba(Mg1/3Ta2/3)O3 ceramics. J. Solid State Chem. 2004, 177, 4031–4046. [Google Scholar] [CrossRef]
- Varma, M.R.; Sebastian, M.T. Effect of dopants on microwave dielectric properties of Ba(Zn1/3Nb2/3)O3 ceramics. J. Eur. Ceram. Soc. 2007, 27, 2827–2833. [Google Scholar] [CrossRef]
- Chia, C.T.; Chang, P.J.; Chen, M.Y.; Lin, I.N.; Ikawa, H.; Lin, L.J. Oxygen-octahedral phonon properties of xBaTiO3+(1-x)Ba(Mg1/3Ta2/3)O3 and xCa(Sc1/2Nb1/2)O3+(1−x)Ba(Sc1/2Nb1/2)O3 microwave ceramics. J. Appl. Phys. 2007, 101, 084115. [Google Scholar] [CrossRef]
- Qasrawi, A.F.; Sahin, E.I.; Abed, T.Y.; Emek, M. Structural and Dielectric Properties of Ba1−xLax(Zn1/3Nb2/3)O3 Solid Solutions. Phys. Status. Solidi B. 2020, 258, 2000419–2000425. [Google Scholar] [CrossRef]
- Varma, M.R.; Raghunandan, R.; Sebastian, M.T. Effect of dopants on microwave dielectric properties of Ba(Zn1/3Ta2/3)O3 Ceramics. Jpn. J. Appl. Phys. 2005, 44, 298–303. [Google Scholar] [CrossRef]
- Liu, X. Sr(Ga0.5Nb0.5)1−xTixO3 Low-Loss Microwave Dielectric Ceramics with Medium Dielectric Constant. J. Am. Ceram. Soc. 2015, 98, 2534–2540. [Google Scholar] [CrossRef]
- Diao, C.L.; Wang, C.H.; Luo, N.N.; Qi, Z.M.; Shao, T.; Wang, Y.Y.; Lu, J.; Wang, Q.C.; Kuang, X.J.; Fang, L.; et al. First-principle calculation and assignment for vibrational spectra of Ba(Mg1/3Nb2/3)O3 microwave dielectric ceramic. J. Appl. Phys. 2014, 115, 787–791. [Google Scholar] [CrossRef]
- Siny, I.G.; Tao, R.; Katiyar, R.S.; Guo, R.; Bhalla, A.S. Raman spectroscopy of Mg-Ta order-disorder in Ba(Mg1/3Ta2/3)O3. Phys. Chem. Solids. 1998, 59, 181–195. [Google Scholar] [CrossRef]
- Ratheesh, R.; Sreemoolanadhan, H.; Sebastian, M.T. Vibrational analysis of Ba5−xSrxNb4O15 microwave dielectric ceramic resonators. J. Solid State Chem. 1997, 131, 2–8. [Google Scholar] [CrossRef]
- Ratheesh, R.; Wohlecke, M.; Berge, B.; Wahlbrink, T.; Haeuseler, H.; Ruhl, E.; Blachnik, R.; Balan, P.; Santha, N.; Sbastian, M.T. Raman study of the ordering in Sr(B0.5′Nb0.5)O3 compounds. J. Appl. Phys. 2000, 88, 2813–2818. [Google Scholar] [CrossRef]
- Kim, E.S.; Chun, B.S.; Freer, R.; Cernik, R.J. Effects of packing fraction and bond valence on microwave dielectric properties of A2+B6+O4 (A2+: Ca, Pb, Ba; B6+: Mo, W) Ceramics. J. Eur. Ceram. Soc. 2010, 30, 1731–1736. [Google Scholar] [CrossRef]
- Huang, C.L.; Hsu, C.S.; Liu, S.J. Dielectric properties of 0.95Ba(Zn1/3Nb2/3)O3–0.05BaZrO3 ceramics at microwave frequency. Mater. Lett. 2003, 57, 3602–3605. [Google Scholar] [CrossRef]
- Yue, Z.; Zhao, F.; Zhang, Y.; Gui, Z.; Li, L. Microwave dielectric properties of Ba[(Zn1−xCox)1/3Nb2/3]O3 ceramics. Mater. Lett. 2004, 58, 1830–1834. [Google Scholar] [CrossRef]
- Fu, M.; Liu, X.; Chen, X. Effects of Mg Substitution on Microstructures and Microwave Dielectric Properties of Ba(Zn1/3Nb2/3)O3 Perovskite Ceramics. J. Am. Ceram. Soc. 2010, 93, 787–795. [Google Scholar] [CrossRef]
- Alkathy, M.S.; Rahman, A.; Zabotto, F.L.; Milton, F.P.; Raju, K.C.J.; Eiras, J.A. Room-temperature multiferroic behaviour in Co/Fe co-substituted layer-structured Aurivillius phase ceramics. Ceram. Int. 2022, 48, 30041–30051. [Google Scholar] [CrossRef]
- Jaiswal, S.K.; Hong, J.; Yoon, K.J.; Son, J.W.; Lee, J.H. Synthesis and conductivity behaviour of proton conducting (1-x)Ba0.6Sr0.4Ce0.75Zr0.10Y0.15O3δ-xGDC (x = 0, 0.2, 0.5) composite electrolytes. J. Am. Ceram. Soc. 2017, 100, 4710–4718. [Google Scholar] [CrossRef]
- Komai, S.; Hirano, M.; Ohtsu, N. Spectral analysis of Sr 3d XPS spectrum in Sr—containing hydroxyapatite. Surf. Interface Anal. 2020, 52, 823–828. [Google Scholar] [CrossRef]
- Chen, X.; Li, H.; Zhang, P.; Hu, H.; Chen, G.; Li, G. A low—permittivity microwave dielectric ceramic BaZnP2O7 and its performance modification. J. Am. Ceram. Soc. 2021, 104, 5214–5223. [Google Scholar] [CrossRef]
- Atuchin, V.V.; Kalabin, I.E.; Kesler, V.G.; Pervukhina, N.V. Nb 3d and O 1s core levels and chemical bonding in niobates. J. Elect. Spectr. Rel. Phenom. 2005, 142, 129–134. [Google Scholar] [CrossRef]
- Yu, S.Q.; Tang, B.; Zhang, X.; Zhang, S.R.; Zhou, X.H. Improved High-Q Microwave Dielectric Ceramics in CuO-Doped BaTi4O9-BaZn2Ti4O11 System. J. Am. Ceram. Soc. 2012, 95, 1939–1943. [Google Scholar] [CrossRef]
- Zhang, P.; Hao, M.M.; Xiao, M.; Zheng, Z.T. Crystal structure and microwave dielectric properties of novel BiMg2MO6 (M = P, V) ceramics with low sintering temperature. J. Mater. 2021, 7, 1344–1351. [Google Scholar] [CrossRef]
- Levine, B.F. Bond susceptibilities and ionicities in complex crystal structures. J. Chem. Phys. 1973, 59, 1463–1486. [Google Scholar] [CrossRef]
- Zhai, S.; Liu, P. Microwave dielectric properties of rock-salt structured Li7(Nb1−xTix)2O8-xF(0 ≤ x ≤ 0.10) system with low sintering temperature. Ceram. Int. 2022, 48, 28268–28273. [Google Scholar] [CrossRef]
- Shannon, R.D. Dielectric polarizabilities of ions in oxides and fluorides. J. Appl. Phys. 1993, 73, 348–366. [Google Scholar] [CrossRef]
- Kim, E.S.; Chun, B.S.; Yoon, K.H. Dielectric properties of [Ca1−x(Li1/2Nd1/2)x]1−yZnyTiO3 ceramics at microwave frequencies. Mater. Sci. Eng. B. 2003, 99, 93–97. [Google Scholar] [CrossRef]
- Shimada, Y.; Mutsukura, N.; Machi, Y. Dielectric and structural characteristics of Ba- and Sr-based complex perovskites as a function of tolerance factor. Jpn. J. App. Phys. 1992, 31, 1958–1963. [Google Scholar] [CrossRef]
- Liu, S.; Li, H.; Xiang, R.; Zhang, P.; Chen, X.; Wen, Q.; Hu, H. Effect of substituting Al3+ for Ti4+ on the microwave dielectric performance of Mg2Ti1−xAl4/3xO4 (0.01 ≤ x ≤ 0.09) ceramics. Ceram. Int. 2021, 47, 33064–33069. [Google Scholar] [CrossRef]

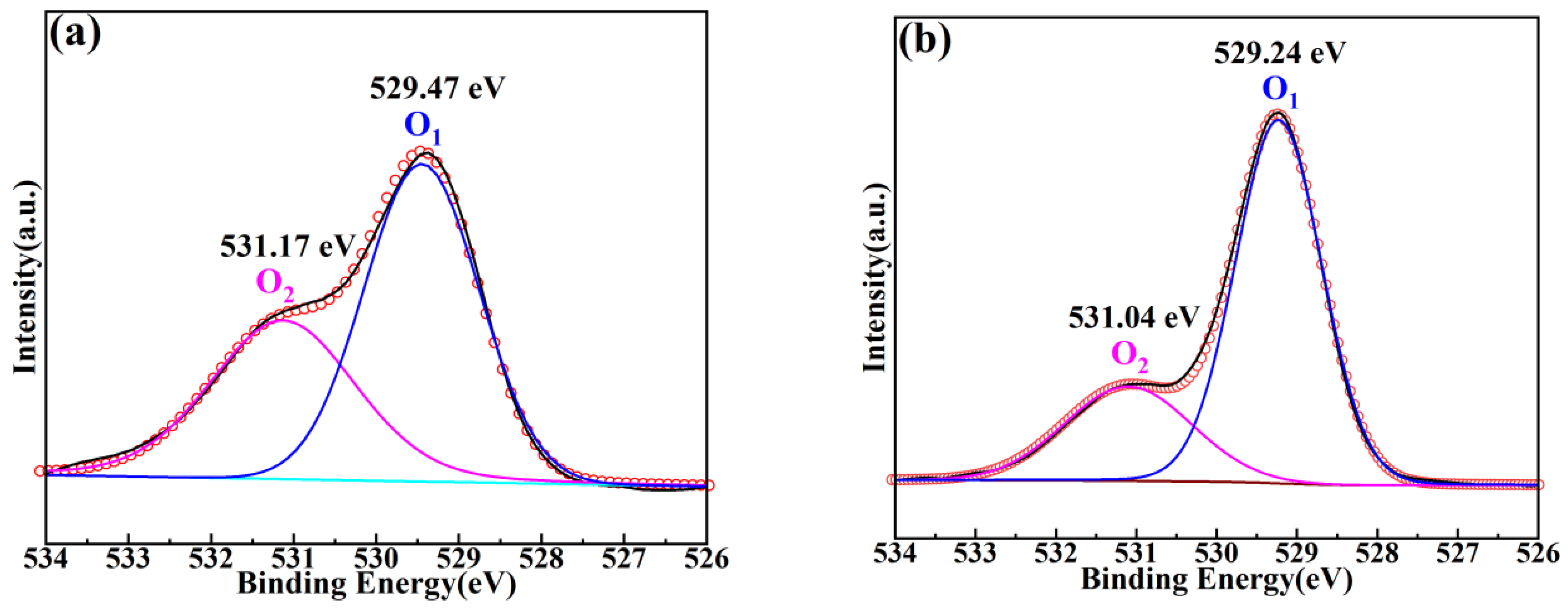
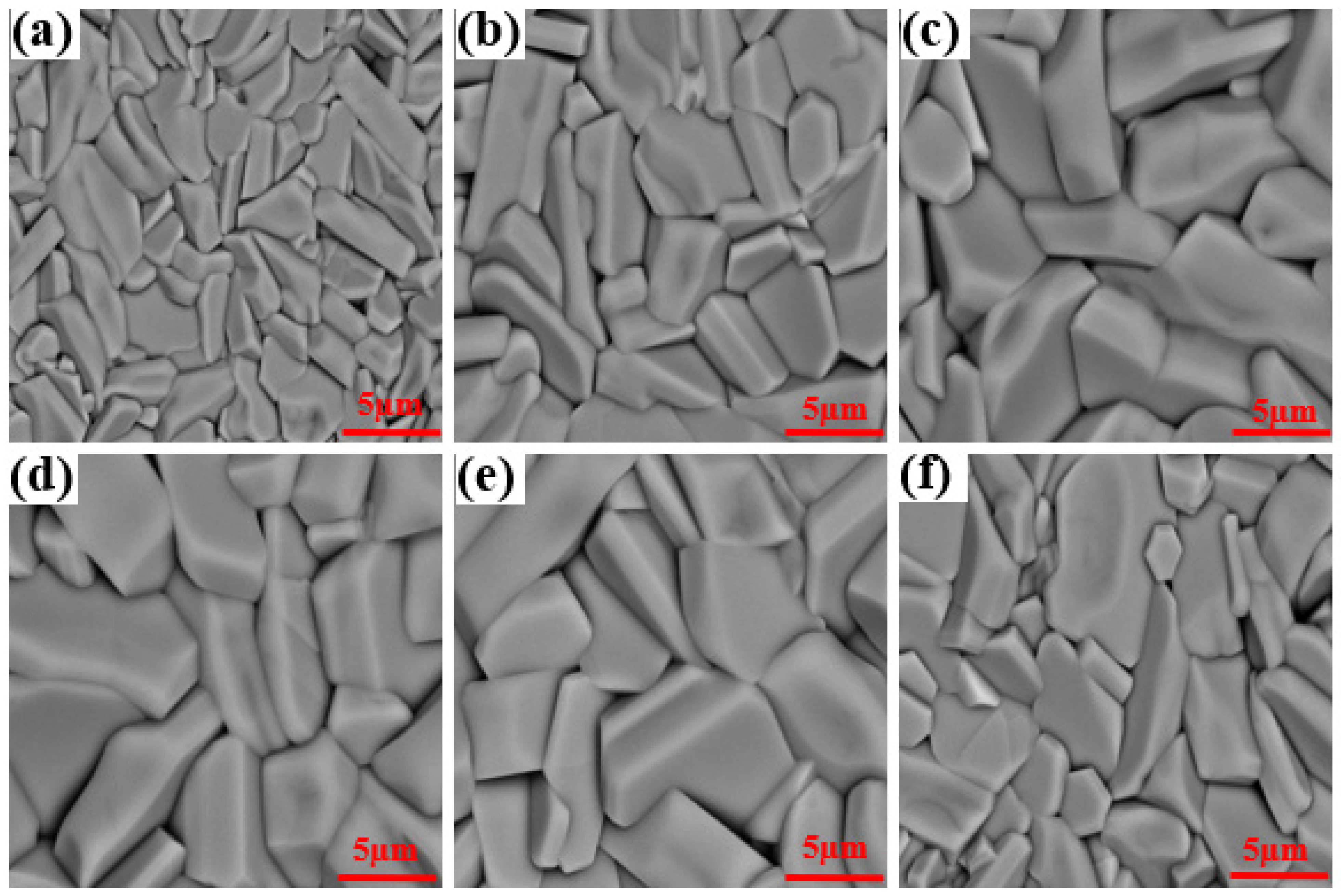

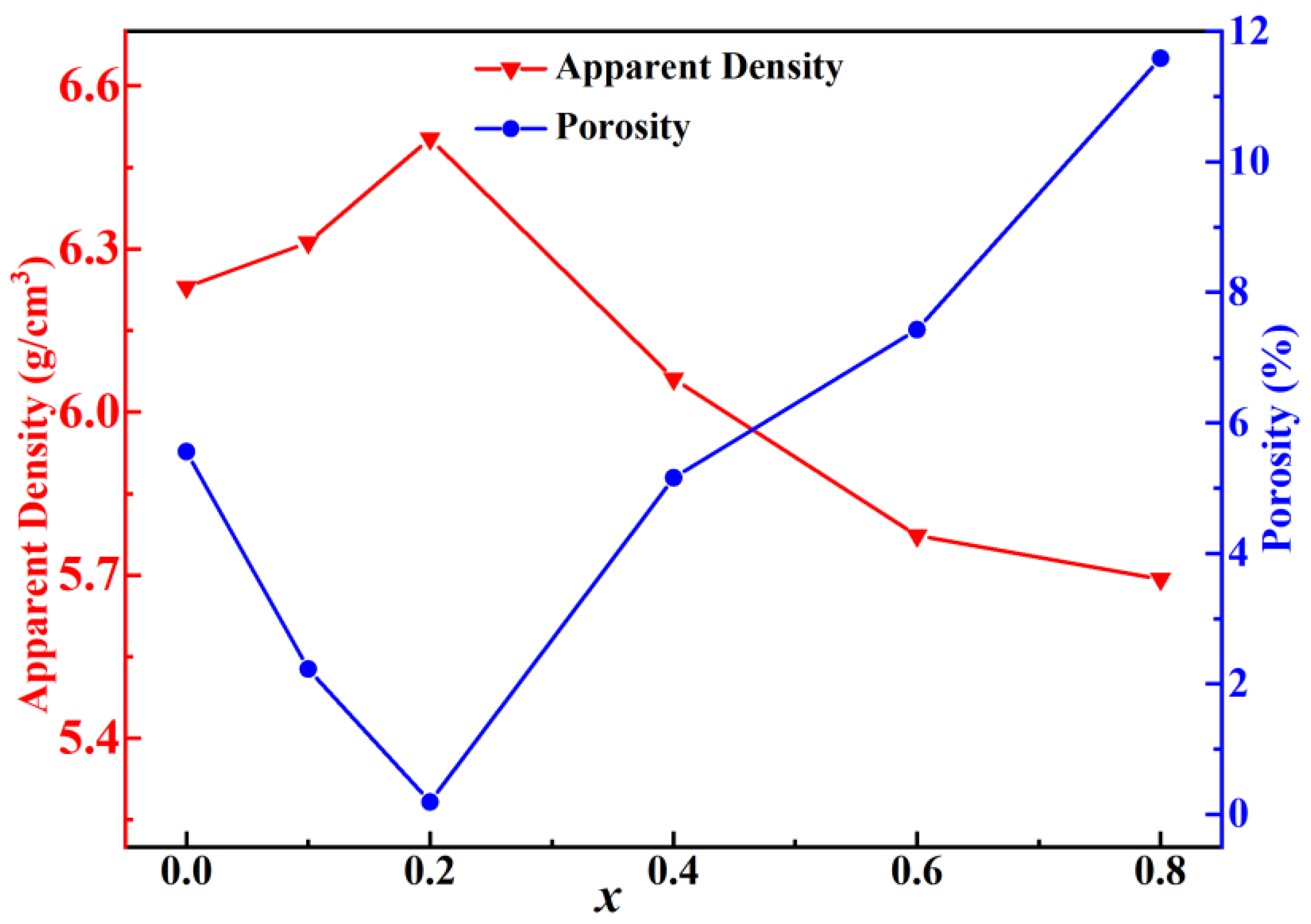
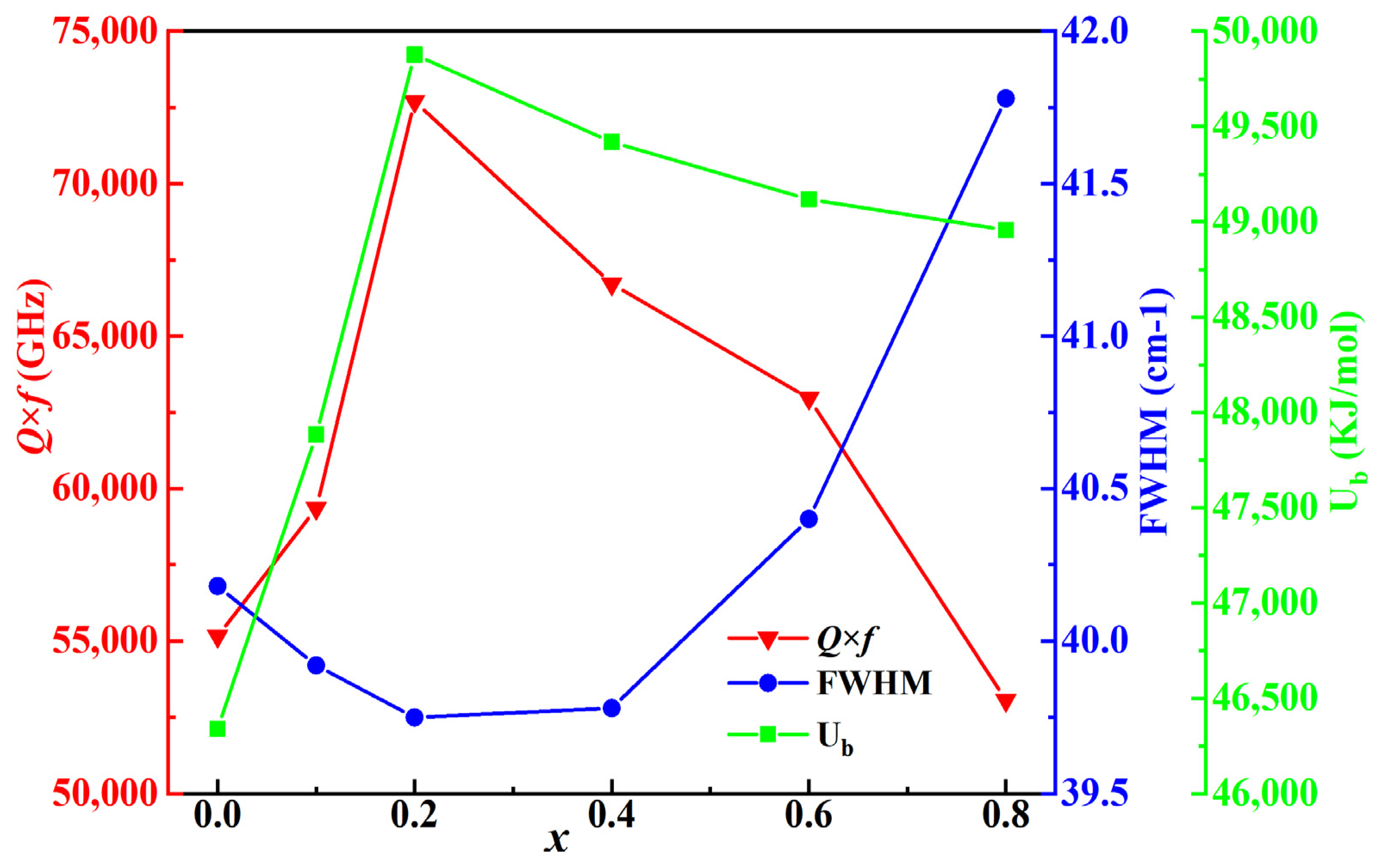
| x | a = b = c (Å) | α = β = γ (°) | Vcell (Å3) |
|---|---|---|---|
| 0 | 4.09548 | 90 | 68.65 |
| 0.1 | 4.08758 | 90 | 68.3 |
| 0.2 | 4.0796 | 90 | 67.9 |
| 0.4 | 4.0625 | 90 | 67.05 |
| 0.6 | 4.04586 | 90 | 66.23 |
| 0.8 | 4.02254 | 90 | 65.09 |
| x | Atomic Percentage (%) | ||||
|---|---|---|---|---|---|
| Ba | Sr | Zn | Nb | O | |
| 0 | 26.71 | / | 3.56 | 17.59 | 52.14 |
| 0.1 | 21.35 | 1.97 | 3.75 | 15.91 | 57.02 |
| 0.2 | 19.76 | 4.95 | 4.13 | 16.78 | 54.38 |
| 0.4 | 17.04 | 11.21 | 2.88 | 18.05 | 50.82 |
| 0.6 | 10.75 | 15.88 | 3.47 | 17.99 | 51.91 |
| 0.8 | 5.3 | 21.36 | 3.42 | 16.36 | 53.56 |
| Bond Type | Bond Length (Å) | fi (%) | fc (%) |
|---|---|---|---|
| Ba-O | 2.92 | 85.80 | 14.20 |
| Zn-O | 2.01 | 56.03 | 43.97 |
| Nb-O | 2.07 | 83.34 | 16.66 |
| x | εr | Vcell | Z | αtheo | αobs | Δ (%) |
|---|---|---|---|---|---|---|
| 0 | 40.91 | 68.65 | 1 | 15.7567 | 15.243 | 3.26 |
| 0.1 | 42.88 | 68.30 | 1 | 15.5407 | 15.215 | 2.1 |
| 0.2 | 45.25 | 67.90 | 1 | 15.3247 | 15.1807 | 0.94 |
| 0.4 | 44.60 | 67.05 | 1 | 14.8927 | 14.977 | 0.57 |
| 0.6 | 42.93 | 66.23 | 1 | 14.4607 | 14.7556 | 2.04 |
| 0.8 | 40.55 | 65.09 | 1 | 14.0287 | 14.4435 | 2.96 |
| x | dNbO (Å) | RNbO | VNbO | Δoctahedral | Eb (KJ/mol) | τf (ppm/°C) | |
|---|---|---|---|---|---|---|---|
| 0 | 2.07823 × 3 | 1.911 | 0.6364 × 3 | 3.9195 | 0.91 × 10−4 | 1060 | 27.72 |
| 2.0591 × 3 | 0.6701 × 3 | ||||||
| 0.1 | 2.05809 × 3 | 1.911 | 0.636 × 3 | 3.9239 | 1.03 × 10−4 | 1062 | 11.61 |
| 2.07844 × 3 | 0.672 × 3 | ||||||
| 0.2 | 2.06544 × 3 | 1.911 | 0.7106 × 3 | 3.9773 | 1.7 × 10−4 | 1074 | 1.26 |
| 1.89159 × 3 | 0.6152 × 3 | ||||||
| 0.4 | 2.02418 × 3 | 1.911 | 0.7347 × 3 | 5.3024 | 1.6 × 10−3 | 1034 | −5.16 |
| 1.8997 × 3 | 1.031 × 3 | ||||||
| 0.6 | 2.04764 × 3 | 1.911 | 0.6912 × 3 | 5.7635 | 3 × 10−3 | 1016 | −8.69 |
| 1.83442 × 3 | 1.2299 × 3 | ||||||
| 0.8 | 2.04507 × 3 | 1.911 | 0.696 × 3 | 6.3778 | 4.8 × 10−3 | 995 | −15.72 |
| 1.77869 × 3 | 1.4299 × 3 |
| Year | Ceramics Composition | Sintering Temperature (°C) | εr | Q × f (GHz) | τf (ppm/°C) | Reference |
|---|---|---|---|---|---|---|
| 1982 | Ba(Zn1/3Nb2/3)O3 | 1500 | 41 | 54,000 | +30 | [1] |
| 2003 | 0.95Ba(Zn1/3Nb2/3)O3 + 0.05 BaZrO3 | 1450 | 42 | 96,000 | +27 | [25] |
| 2004 | Ba[(Zn1−xCox)1/3Nb2/3]O3 (x = 0.8) | 1450 | 33 | 20,248 | −0.11 | [26] |
| 2010 | Ba(Zn1−xMgx)1/3Nb2/3)O3 (x = 0.4) | 1400 | 36 | 94,400 | +28.6 | [27] |
| 2008 | Ba1−xLa2x/3(Zn0.3Co0.7)1/3Nb2/3O3 (x = 0.015) | 1425 | 34 | 63,159 | 5.21 | [13] |
| 2023 | Ba1−xSrx(Zn1/3Nb2/3)O3 (x = 0.2) | 1500 | 45.25 | 72,704 | 1.26 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, L.; Deng, L.; Zhang, Y.; Wu, P.; Zeng, W.; Peng, S. Crystal Structure, Chemical Bond, and Microwave Dielectric Properties of Ba1−xSrx(Zn1/3Nb2/3)O3 Solid Solution Ceramics. Molecules 2023, 28, 3451. https://doi.org/10.3390/molecules28083451
Xiao L, Deng L, Zhang Y, Wu P, Zeng W, Peng S. Crystal Structure, Chemical Bond, and Microwave Dielectric Properties of Ba1−xSrx(Zn1/3Nb2/3)O3 Solid Solution Ceramics. Molecules. 2023; 28(8):3451. https://doi.org/10.3390/molecules28083451
Chicago/Turabian StyleXiao, Lei, Lianwen Deng, Yu Zhang, Ping Wu, Wenfei Zeng, and Sen Peng. 2023. "Crystal Structure, Chemical Bond, and Microwave Dielectric Properties of Ba1−xSrx(Zn1/3Nb2/3)O3 Solid Solution Ceramics" Molecules 28, no. 8: 3451. https://doi.org/10.3390/molecules28083451
APA StyleXiao, L., Deng, L., Zhang, Y., Wu, P., Zeng, W., & Peng, S. (2023). Crystal Structure, Chemical Bond, and Microwave Dielectric Properties of Ba1−xSrx(Zn1/3Nb2/3)O3 Solid Solution Ceramics. Molecules, 28(8), 3451. https://doi.org/10.3390/molecules28083451







