Systematic Theoretical Study on the pH-Dependent Absorption and Fluorescence Spectra of Flavins
Abstract
1. Introduction
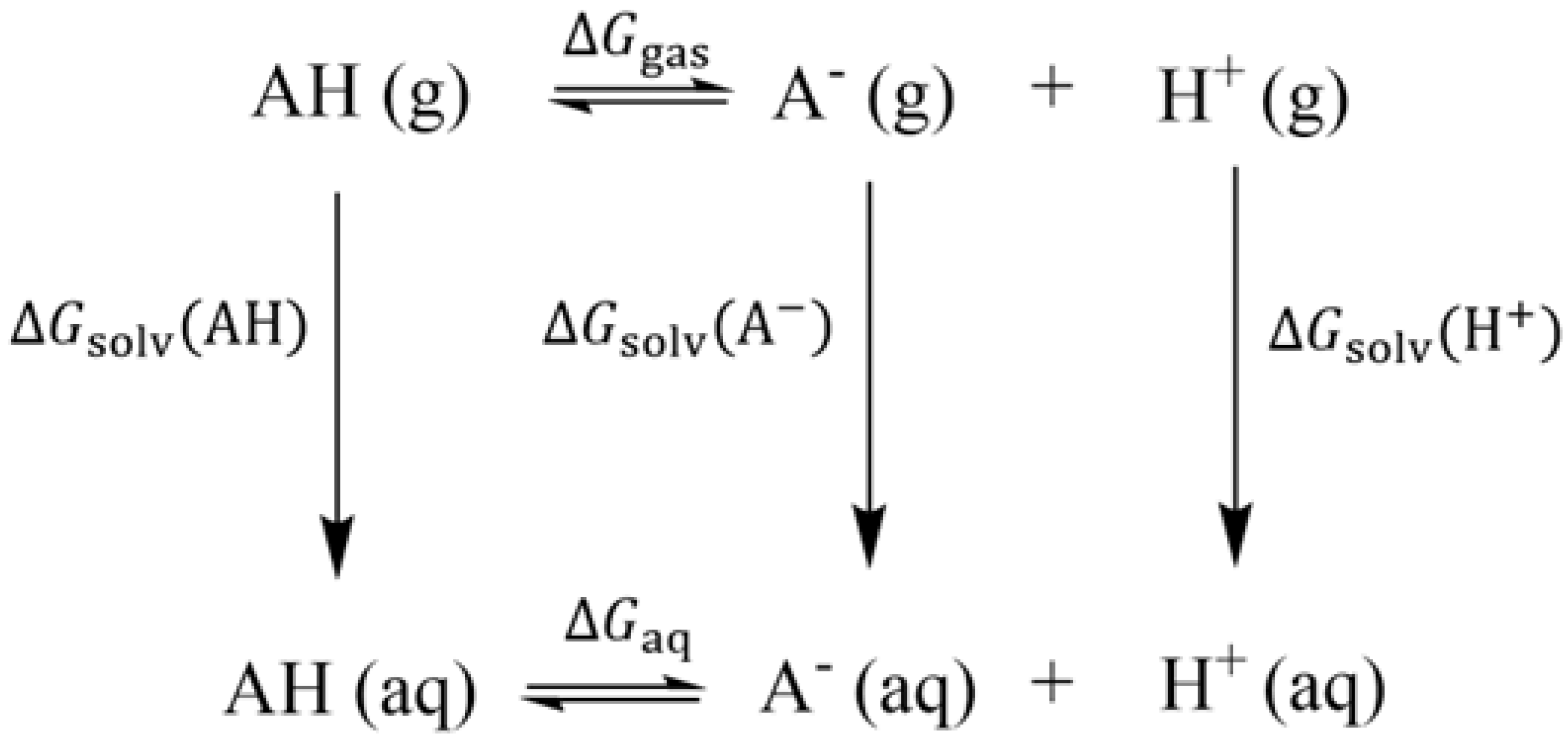
2. Computational Details
3. Results and Discussion
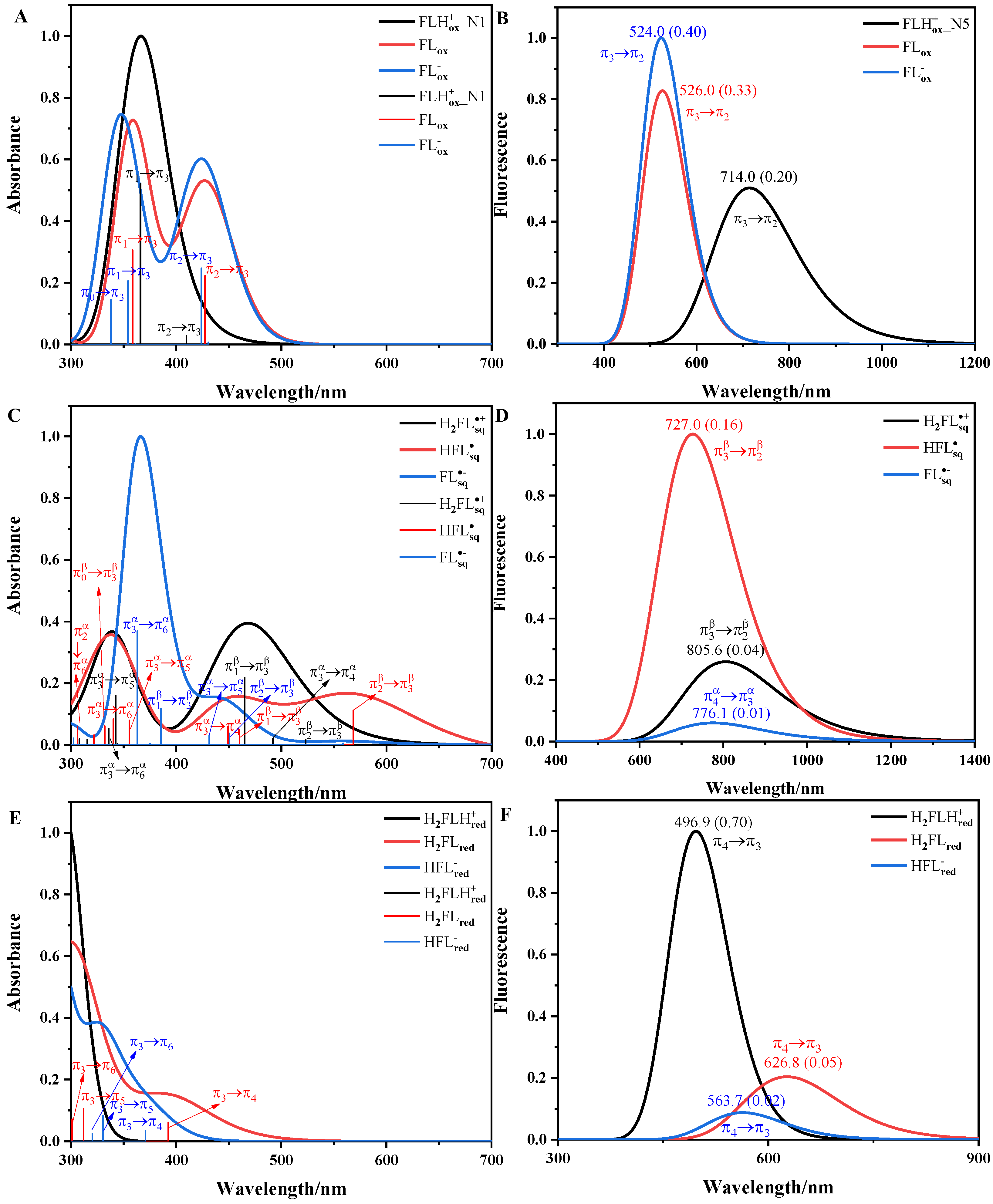
3.1. Absorption and Fluorescence Spectra of Flavins in Solution
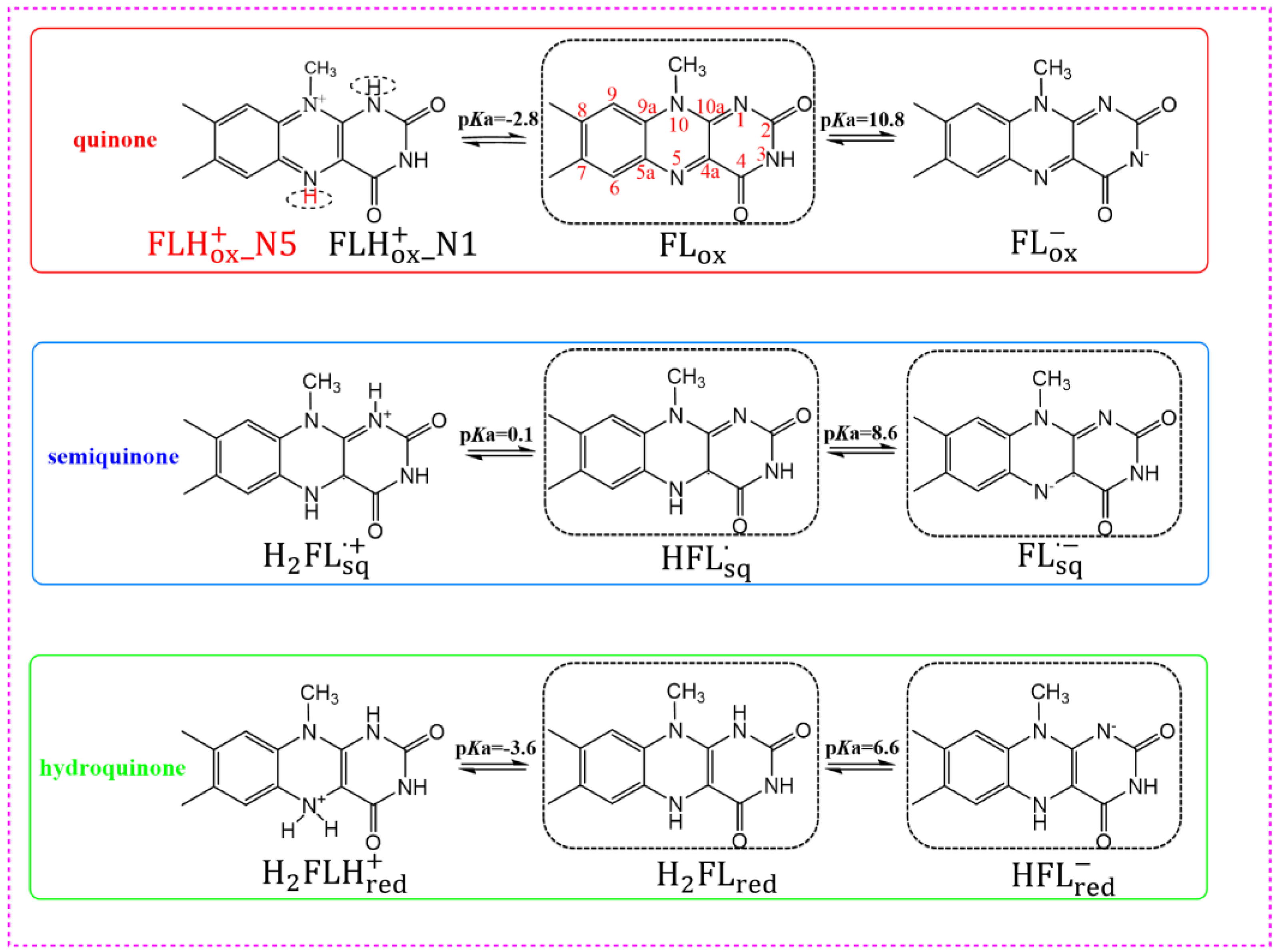
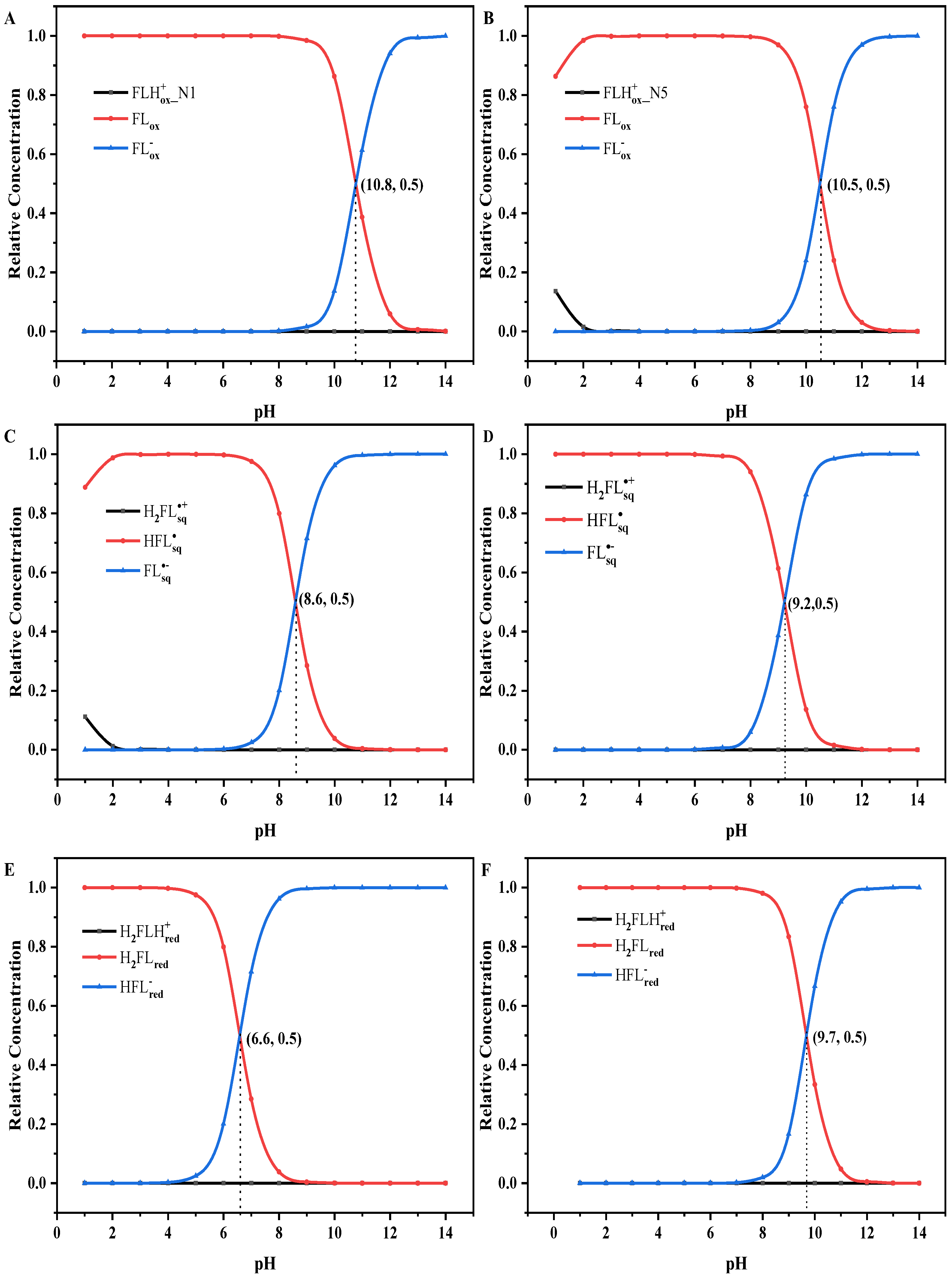
3.2. Chemical Equilibrium of Flavins in Solution
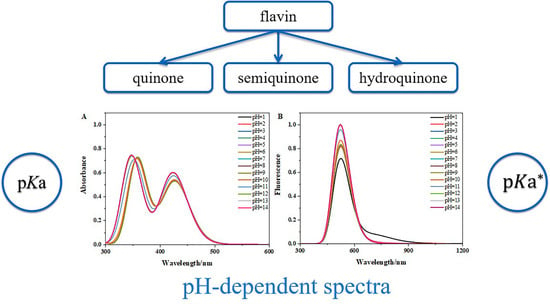
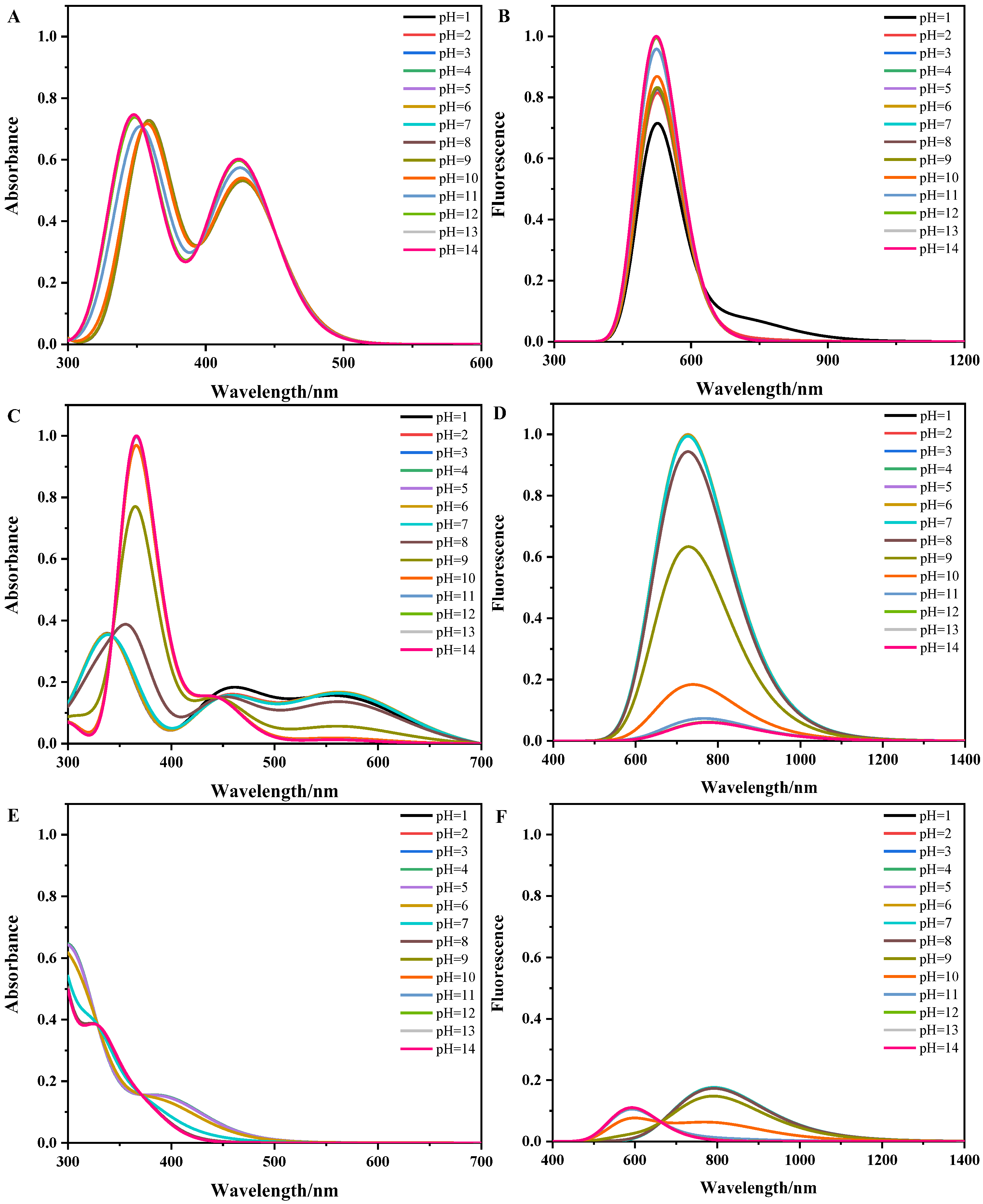
3.3. The pH-Dependent Absorption and Fluorescence Spectra of Flavins in Solution
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Huijbers, M.M.; Montersino, S.; Westphal, A.H.; Tischler, D.; van Berkel, W.J. Flavin Dependent Monoxygenases. Arch. Biochem. Biophys. 2014, 544, 2–17. [Google Scholar] [CrossRef] [PubMed]
- van Berkel, W.J.; Kamerbeek, N.M.; Fraaije, M.W. Flavoprotein Monooxygenases, A Diverse Class of Oxidative Biocatalysts. J. Biotechnol. 2006, 124, 670–689. [Google Scholar] [CrossRef] [PubMed]
- Koetter, J.W.A.; Schulz, G.E. Crystal Structure of 6-Hydroxy-d-nicotine Oxidase from Arthrobacter nicotinovorans. J. Mol. Biol. 2005, 352, 418–428. [Google Scholar] [CrossRef] [PubMed]
- Hassan-Abdallah, A.; Bruckner, R.C.; Zhao, G.; Jorns, M.S. Biosynthesis of Covalently Bound Flavin: Isolation and In vitro Flavinylation of the Monomeric Sarcosine Oxidase Apoprotein. Biochemistry 2005, 44, 6452–6462. [Google Scholar] [CrossRef]
- Liu, Y.-J. Understanding the Complete Bioluminescence Cycle from a Multiscale Computational Perspective: A review. J. Photochem. Photobiol. C 2022, 52, 1. [Google Scholar] [CrossRef]
- O’Kane, D.J.; Prasher, D.C. Evolutionary Origins of Bacterial Bioluminescence. Mol. Microbiol. 1992, 6, 443–449. [Google Scholar] [CrossRef]
- Mager, H.I.; Tu, S.C. Chemical Aspects of Bioluminescence. Photochem. Photobiol. 1995, 62, 607–614. [Google Scholar] [CrossRef]
- Colombo, B.; Saraceno, L.; Comi, G. Riboflavin and Migraine: The Bridge Over Troubled Mitochondria. Neurol. Sci. 2014, 35, 141–144. [Google Scholar] [CrossRef]
- Schulman, S.G. pH Dependence of Fluorescence of Riboflavin and Related Isoalloxazine Derivatives. J. Pharm. Sci. 1971, 60, 628–631. [Google Scholar] [CrossRef]
- Ahmad, I.; Anwar, Z.; Iqbal, K.; Ali, S.A.; Mirza, T.; Khurshid, A.; Khurshid, A.; Arsalan, A. Effect of Acetate and Carbonate buffers on the Photolysis of Riboflavin in Aqueous Solution: A Kinetic Study. AAPS PharmSciTech 2014, 15, 550–559. [Google Scholar] [CrossRef]
- Villabona-Monsalve, J.P.; Varnavski, O.; Palfey, B.A.; Goodson, T. Two-Photon Excitation of Flavins and Flavoproteins with Classical and Quantum Light. J. Am. Chem. Soc. 2018, 140, 14562–14566. [Google Scholar] [CrossRef] [PubMed]
- Ghisla, S.; Massey, V.; Lhoste, J.M.; Mayhew, S.G. Fluorescence and Optical Characteristics of Reduced Flavines and Flavoproteins. Biochemistry 1974, 13, 589. [Google Scholar] [CrossRef] [PubMed]
- Van den Berg, P.A.; Widengren, J.; Hink, M.A.; Rigler, R.; Visser, A.J. Fluorescence Correlation Spectroscopy of Flavins and Flavoenzymes: Photochemical and Photophysical Aspects. Spectrochim. Acta Part A 2001, 57, 2135–2144. [Google Scholar] [CrossRef]
- Ai, Y.; Zhao, C.; Xing, J.; Liu, Y.; Wang, Z.; Jin, J.; Xia, S.; Cui, G.; Wang, X. Excited-State Decay Pathways of Flavin Molecules in Five Redox Forms: The Role of Conical Intersections. J. Phys. Chem. A. 2018, 122, 7954–7961. [Google Scholar] [CrossRef] [PubMed]
- Tyagi, A.; Penzkofer, A. pH Dependence of the Absorption and Emission Behaviour of Lumiflavin in Aqueous Solution. J. Photochem. Photobiol. A 2010, 215, 108–117. [Google Scholar] [CrossRef]
- Song, S.H.; Dick, B.; Penzkofer, A.; Pokorny, R.; Batschauer, A.; Essen, L.O. Absorption and Fluorescence Spectroscopic Characterization of Cryptochrome 3 from Arabidopsis Thaliana. J. Photochem. Photobiol. B 2006, 85, 1–16. [Google Scholar] [CrossRef]
- Kabir, M.P.; Orozco-Gonzalez, Y.; Gozem, S. Electronic Spectra of Flavin in Different Redox and Protonation States: A Computational Perspective on the Effect of the Electrostatic Environment. Phys. Chem. Chem. Phys. 2019, 21, 16526–16537. [Google Scholar] [CrossRef]
- Schwinn, K.; Ferre, N.; Huix-Rotllant, M. UV-visible Absorption Spectrum of FAD and Its Reduced Forms Embedded in A Cryptochrome Protein. Phys. Chem. Chem. Phys. 2020, 22, 12447–12455. [Google Scholar] [CrossRef]
- Sato, K.; Nishina, Y.; Shiga, K. Decomposition of the Fluorescence Spectra of Two FAD Molecules in Electron-transferring Flavoprotein from Megasphaera elsdenii. J. Biochem. 2013, 154, 61–66. [Google Scholar] [CrossRef]
- Islam, M.S.; Honma, M.; Nakabayashi, T.; Kinjo, M.; Ohta, N. pH Dependence of the Fluorescence Lifetime of FAD in Solution and in Cells. Int. J. Mol. Sci. 2013, 14, 1952–1963. [Google Scholar] [CrossRef]
- Nakabayashi, T.; Islam, M.S.; Ohta, N. Fluorescence Decay Dynamics of Flavin Adenine Dinucleotide in a Mixture of Alcohol and Water in the Femtosecond and Nanosecond Time Range. J. Phys. Chem. B 2010, 114, 15254–15260. [Google Scholar] [CrossRef] [PubMed]
- Galban, J.; Sanz-Vicente, I.; Navarro, J.; de Marcos, S. The Intrinsic Fluorescence of FAD and Its Application in Analytical Chemistry: A Review. Methods Appl. Fluoresc. 2016, 4, 042005. [Google Scholar] [CrossRef] [PubMed]
- Su, D.; Kabir, M.P.; Orozco-Gonzalez, Y.; Gozem, S.; Gadda, G. Fluorescence Properties of Flavin Semiquinone Radicals in Nitronate Monooxygenase. Chembiochem 2019, 20, 1646–1652. [Google Scholar] [CrossRef]
- Perdew, J.P.; Ruzsinszky, A.; Tao, J.; Staroverov, V.N.; Scuseria, G.E.; Csonka, G.I. Prescription for the Design and Selection of Density Functional Approximations: More Constraint Satisfaction with Fewer Fits. J. Chem. Phys. 2005, 123, 062201. [Google Scholar] [CrossRef] [PubMed]
- Dreuw, A.; Head-Gordon, M. Single-reference ab Initio Methods for the Calculation of Excited States of Large Molecules. Chem. Rev. 2005, 105, 4009–4037. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A Consistent and Accurate ab initio Parametrization of Density Functional Dispersion Correction (DFT-D) for the 94 Elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed]
- Runge, E.; Gross, E.K.U. Density-Functional Theory for Time-Dependent Systems. Phys. Rev. Lett. 1984, 52, 997–1000. [Google Scholar] [CrossRef]
- Ghisla, S.; Massey, V. New Flavins for Old: Artificial Flavins as Active Site Probes of Flavoproteins. Biochem. J. 1986, 1, 1–12. [Google Scholar] [CrossRef]
- Kılıç, M.; Ensing, B. Acidity Constants of Lumiflavin from First Principles Molecular Dynamics Simulations. Phys. Chem. Chem. Phys. 2014, 16, 18993–19000. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. The M06 suite of Density Functionals for Main Group Thermochemistry, Thermochemical Kinetics, noncovalent Interactions, Excited states, and Transition Elements: Two New Functionals and Systematic Testing of Four M06-class Functionals and 12 Other Functionals. Theor. Chem. Acc. 2007, 120, 215–241. [Google Scholar]
- Marenich, A.V.; Cramer, C.J.; Truhlar, D.G. Universal Solvation Model Based on Solute Electron Density and on A Continuum Model of the Solvent Defined by the Bulk Dielectric Constant and Atomic Surface Tensions. J. Phys. Chem. B. 2009, 113, 6378–6396. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision D.01; Gaussian, Inc.: Wallingford, CT, USA, 2013. [Google Scholar]
- Haber, F. Betrachtungen zur theorie der warmetonung. Ber. Dtsch. Phys. Ges. 1919, 21, 750. [Google Scholar]
- Liptak, M.D.; Shields, G.C. Accurate pK(a) Calculations for Carboxylic Acids Using Complete Basis Set and Gaussian-n Models Combined with CPCM Continuum Solvation Methods. J. Am. Chem. Soc. 2001, 123, 7314–7319. [Google Scholar] [CrossRef] [PubMed]
- Rashin, A.A.; Topol, I.A.; Tawa, G.J.; Burt, S.K. Charge Distributions in Water and Ion-water Clusters. Chem. Phys. Lett. 2001, 335, 327–333. [Google Scholar] [CrossRef]
- Kelly, C.P.; Cramer, C.J.; Truhlar, D.G. Aqueous Solvation Free Energies of Ions and Ion-water Clusters Based on An Accurate Value for the Absolute Aqueous Solvation Free Energy of the Proton. J. Phys. Chem. B 2006, 110, 16066–16081. [Google Scholar] [CrossRef]
- Houari, Y.; Jacquemin, D.; Laurent, A.D. Methodological Keys for Accurate pKa* Simulations. Phys. Chem. Chem. Phys. 2013, 15, 11875–11882. [Google Scholar] [CrossRef]
- Mondal, P.; Schwinn, K.; Huix-Rotllant, M. Impact of The Redox State of Flavin Chromophores on the UV–vis Spectra, Redox and Acidity Constants and Electron Affinities. J. Photochem. Photobiol. A 2020, 387, 1. [Google Scholar] [CrossRef]
- McBride, R.A.; Barnard, D.T.; Jacoby-Morris, K.; Harun-Or-Rashid, M.; Stanley, R.J. Reduced Flavin in Aqueous Solution Is Nonfluorescent. Biochemistry 2023, 62, 759–769. [Google Scholar] [CrossRef]
- Sakai, M. One-electron Photoreduction of Flavin Mononucleotide: Time-resolved Resonance Raman and Absorption Study. J. Mol. Struct. 1996, 379, 9–18. [Google Scholar] [CrossRef]
- Kar, R.K.; Miller, A.F.; Mroginski, M.A. Understanding Flavin Electronic Structure and Spectra. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2021, 12, 2. [Google Scholar] [CrossRef]
- Choe, Y.K.; Nagase, S.; Nishimoto, K. Theoretical Study of the Electronic Spectra of Oxidized and Reduced States of Lumiflavin and Its derivative. J. Comput. Chem. 2007, 28, 727–739. [Google Scholar] [CrossRef] [PubMed]
- Kar, R.K.; Borin, V.A.; Ding, Y.; Matysik, J.; Schapiro, I. Spectroscopic Properties of Lumiflavin: A Quantum Chemical Study. Photochem. Photobiol. 2019, 95, 662–674. [Google Scholar] [CrossRef] [PubMed]
- Brisker-Klaiman, D.; Dreuw, A. On the Influence of Dimerisation of Lumiflavin in Aqueous Solution on Its Optical Spectra—A Quantum Chemical Study. Mol. Phys. 2019, 117, 2167–2178. [Google Scholar] [CrossRef]
- Land, E.J.; Swallow, A.J. One-electron Reactions in Biochemical Systems as Studied by Pulse Radiolysis. II. Riboflavin. Biochemistry 1969, 5, 2117. [Google Scholar] [CrossRef]
| TD State Order | Transition | f | ||||
|---|---|---|---|---|---|---|
| This paper | 1 | 427.5 | 0.22 | 445.0 | ||
| Ref. [38] | 391.0 | 0.25 | ||||
| Ref. [17] | 1 | 422.7 | 0.24 | |||
| This paper | 2 | 358.7 | 0.31 | 370.0 | ||
| Ref. [38] | 326.0 | 0.26 | ||||
| Ref. [17] | 4 | 345.3 | 0.25 | |||
| This paper | 1 | 568.6 | 0.11 | 571.0 | ||
| Ref. [38] | 535.0 | 0.13 | ||||
| Ref. [17] | 1 | 581.0 | 0.13 | |||
| This paper | 2 | 460.3 | 0.06 | 485.0 | ||
| Ref. [38] | 406.0 | 0.06 | ||||
| Ref. [17] | 3 | 431.3 | 0.06 | |||
| This paper | 6 | 340.2 | 0.08 | 340.0 | ||
| Ref. [38] | 296.0 | 0.11 | ||||
| Ref. [17] | 5 | 360.3 | 0.09 | |||
| This paper | 2 | 450.8 | 0.04 | 480.0 | ||
| Ref. [38] | 423.0 | 0.13 | ||||
| Ref. [17] | 3 | 437.5 | 0.14 | |||
| This paper | 7 | 363.1 | 0.37 | 370.0 | ||
| Ref. [38] | 359.0 | 0.101 | ||||
| Ref. [17] | 6 | 357.6 | 0.297 | |||
| This paper | 1 | 392.4 | 0.06 | 395.0 | ||
| Ref. [38] | 400.0 | 0.03 | ||||
| Ref. [17] | 1 | 411.4 | 0.03 | |||
| This paper | 2 | 330.4 | 0.08 | 342.0 | ||
| Ref. [38] | 347.0 | 0.12 | ||||
| Ref. [17] | 2 | 345.4 | 0.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Liu, Y. Systematic Theoretical Study on the pH-Dependent Absorption and Fluorescence Spectra of Flavins. Molecules 2023, 28, 3315. https://doi.org/10.3390/molecules28083315
Wang J, Liu Y. Systematic Theoretical Study on the pH-Dependent Absorption and Fluorescence Spectra of Flavins. Molecules. 2023; 28(8):3315. https://doi.org/10.3390/molecules28083315
Chicago/Turabian StyleWang, Jinyu, and Yajun Liu. 2023. "Systematic Theoretical Study on the pH-Dependent Absorption and Fluorescence Spectra of Flavins" Molecules 28, no. 8: 3315. https://doi.org/10.3390/molecules28083315
APA StyleWang, J., & Liu, Y. (2023). Systematic Theoretical Study on the pH-Dependent Absorption and Fluorescence Spectra of Flavins. Molecules, 28(8), 3315. https://doi.org/10.3390/molecules28083315